Nuclear Motion Is Classical: Spectra of Hydrogen Chloride and Ammonia
Abstract
1. Introduction
2. Methods
3. Results and Discussion
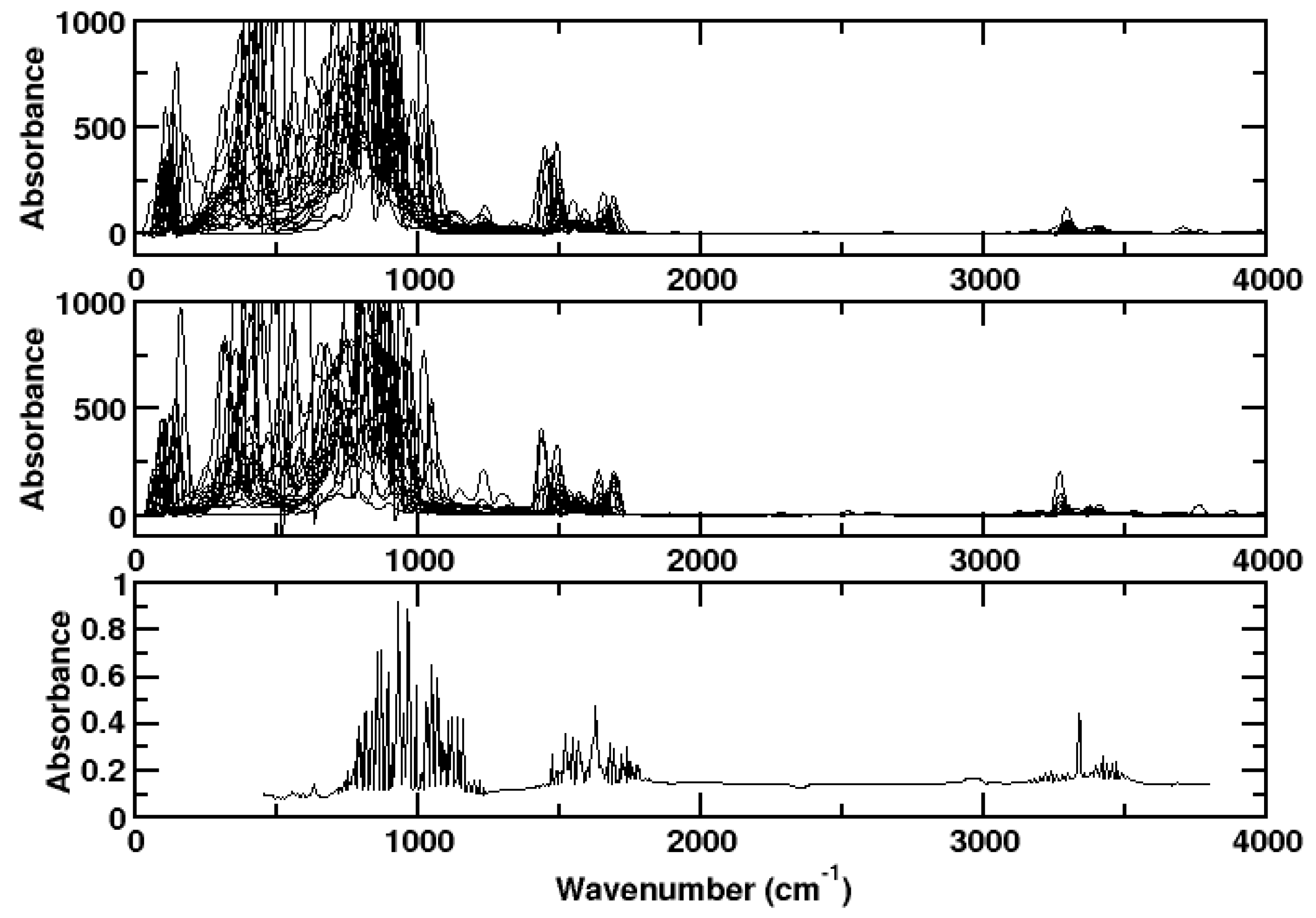
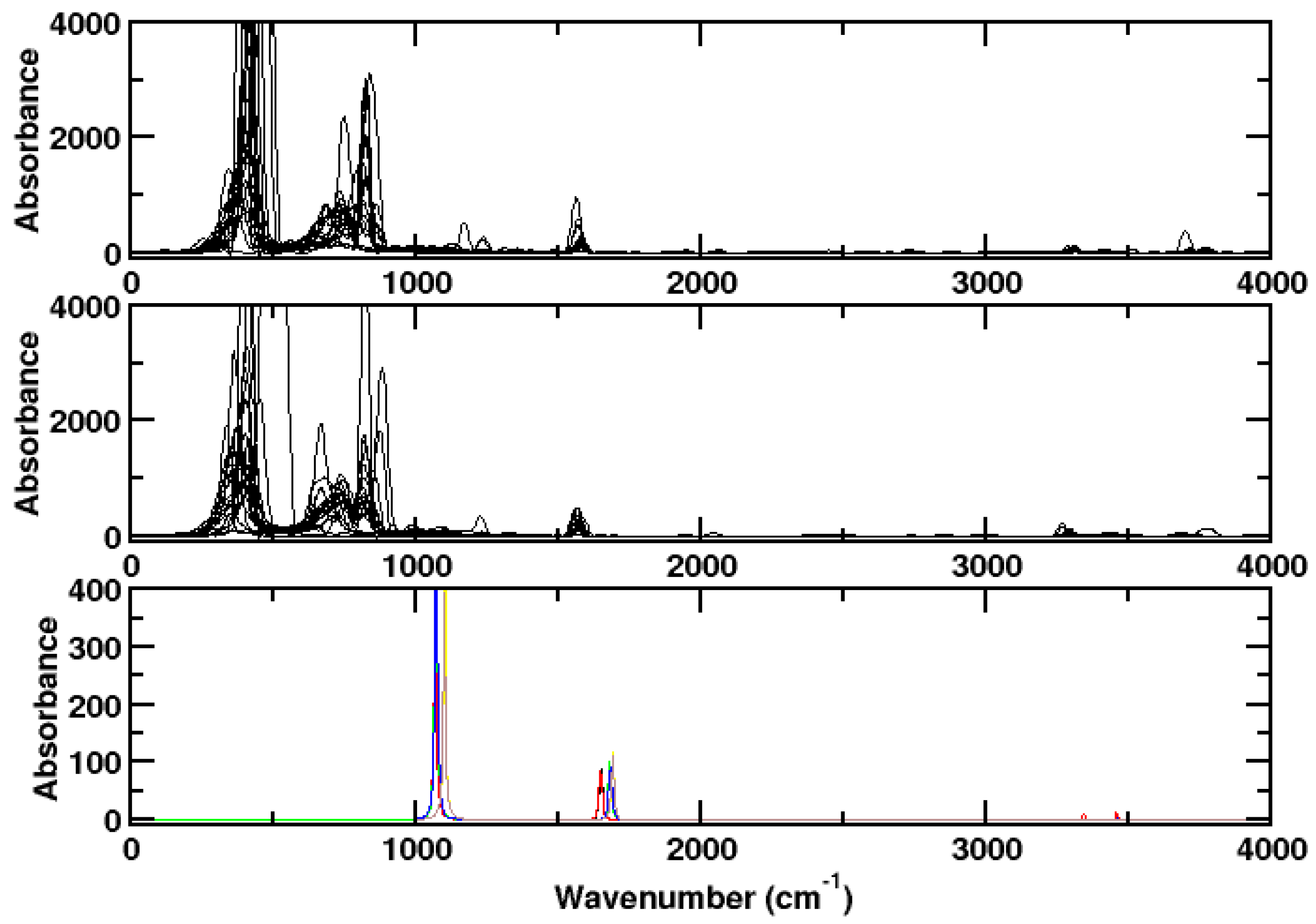
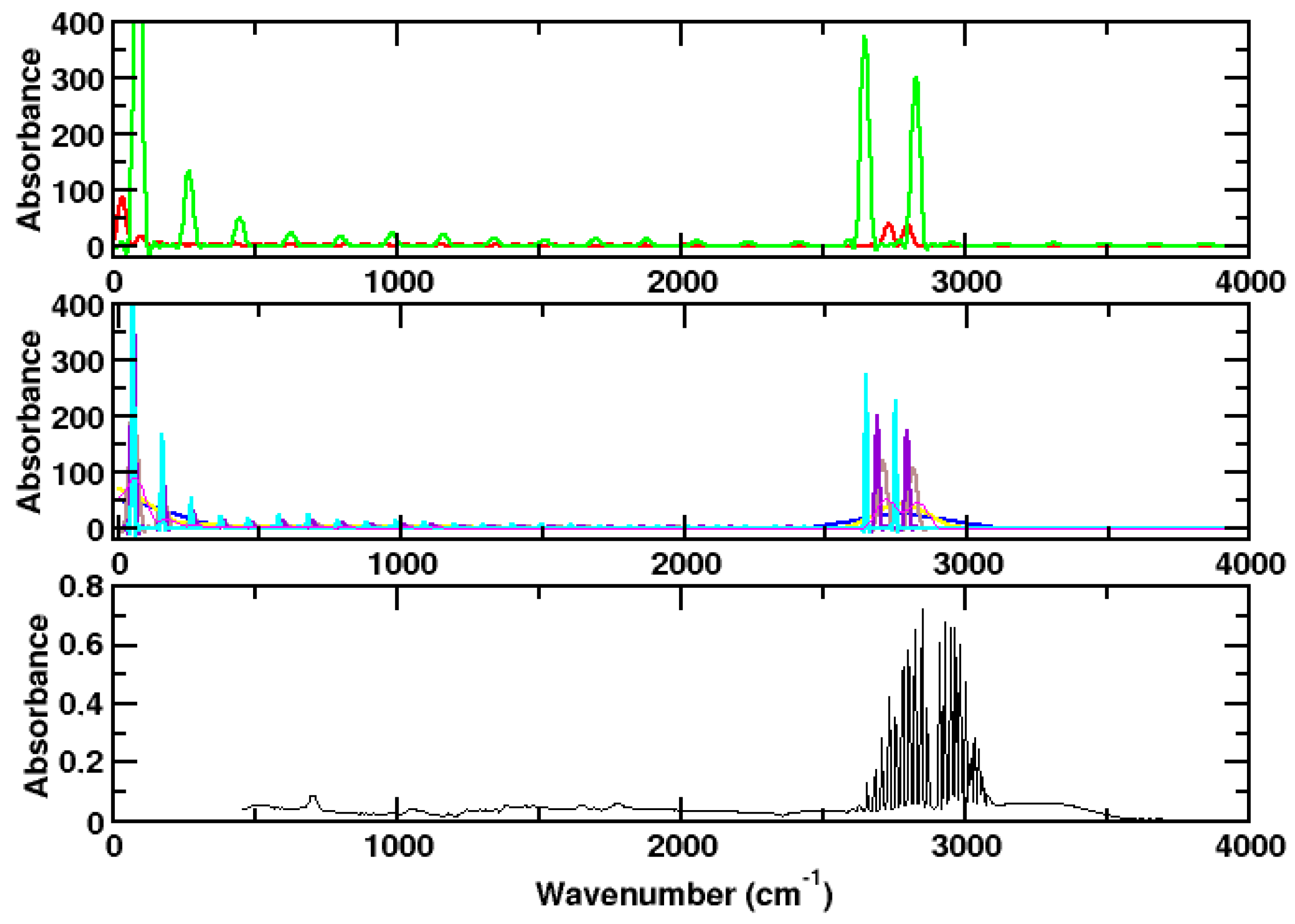
3.1. Ammonia
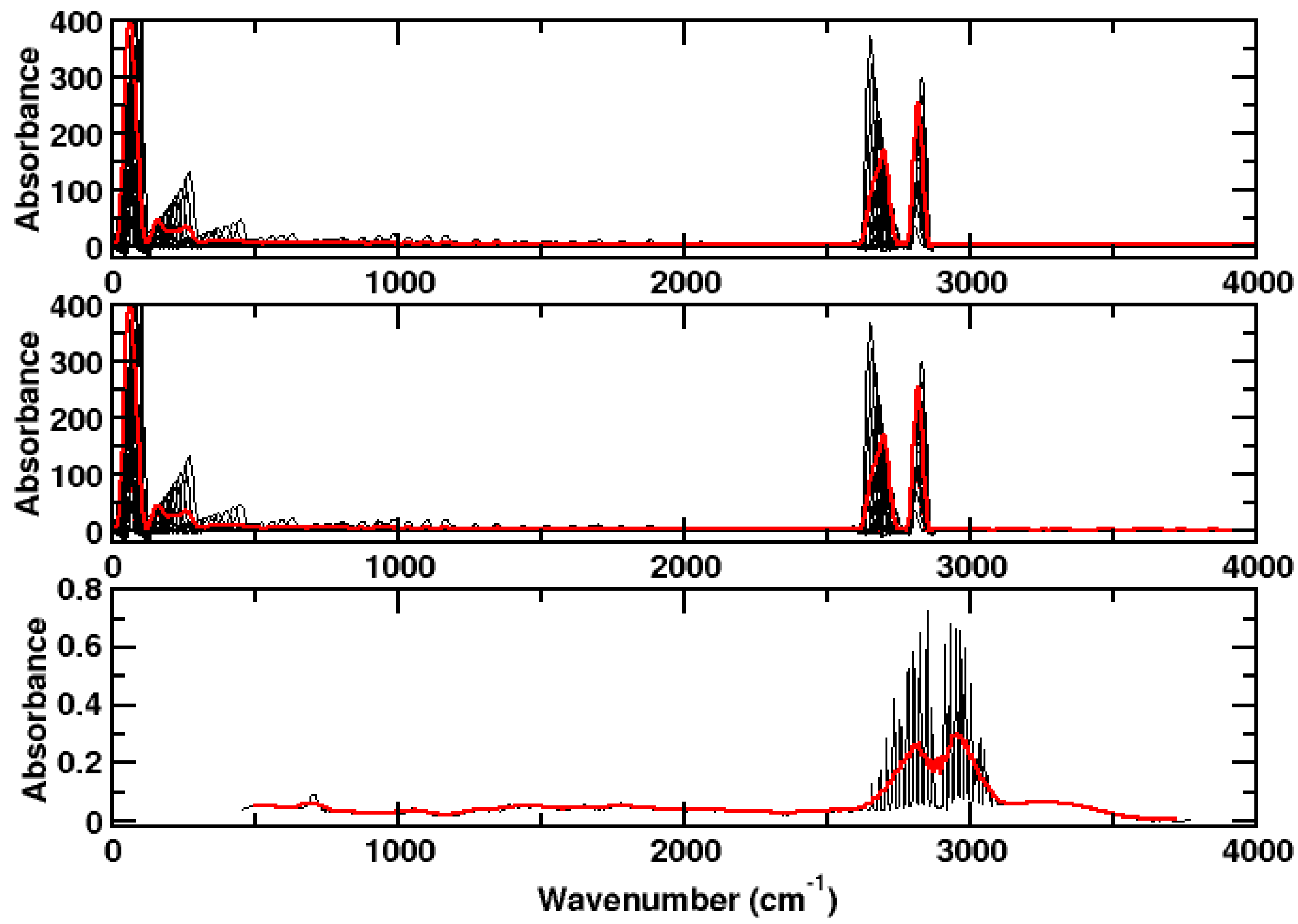
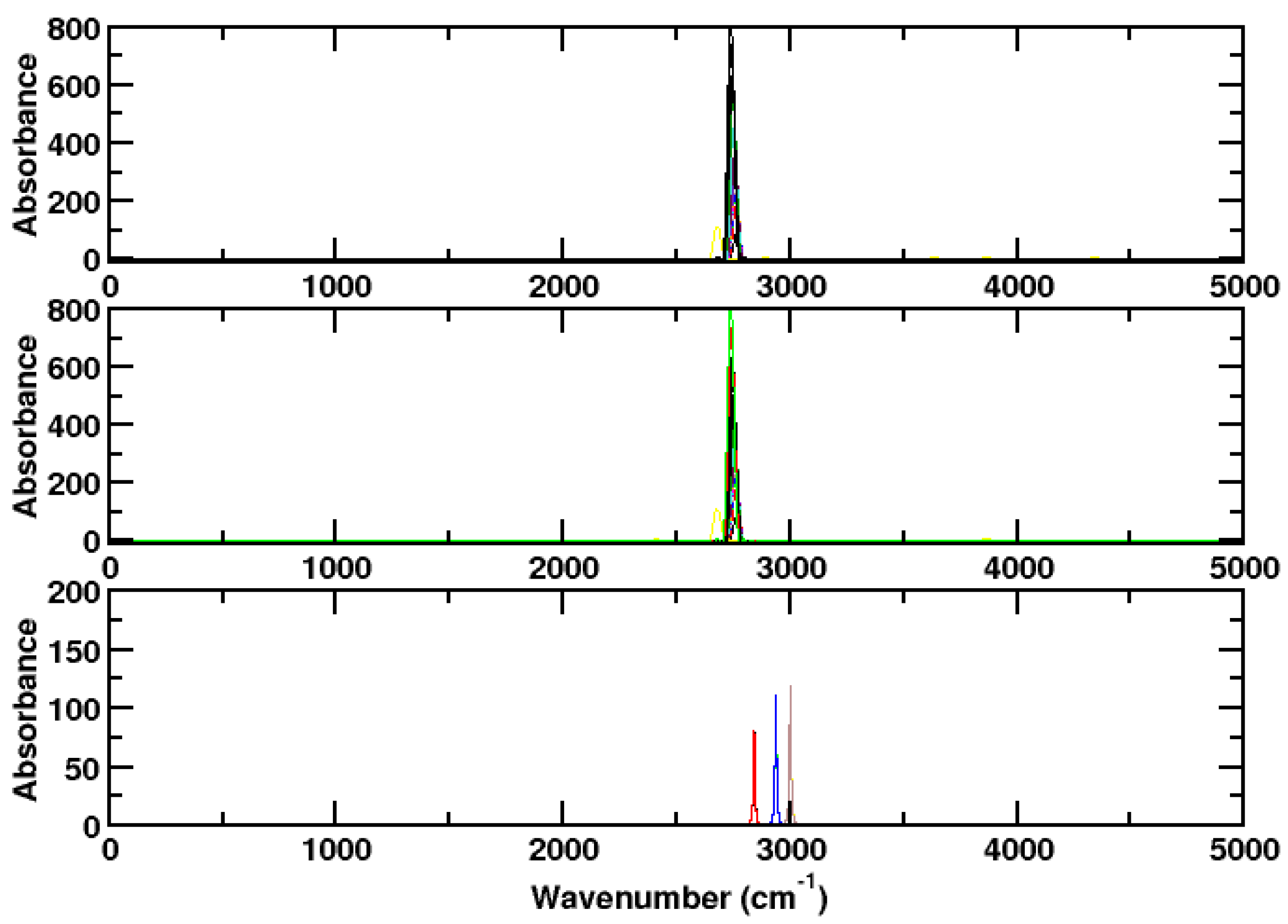
3.2. Hydrogen Chloride
4. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AIMD | Ab Initio Molecular Dynamics |
| BLYP | Becke–Lee–Yang–Parr density functional |
| B3LYP | Hybrid functional based on BLYP |
| B2PLYP | Double-hybrid functional based on B3LYP and MP2 |
| CPMD | Car–Parrinello Molecular Dynamics |
| -D3: | With dispersion correction |
| DFT | Density functional theory |
| MP2 | Moeller–Plesset second-order perturbation theory |
References
- Car, R.; Parrinello, M. Unified Approach for Molecular Dynamics and Density-Functional Theory. Phys. Rev. Lett. 1985, 55, 2471–2474. [Google Scholar] [CrossRef] [PubMed]
- Marx, D.; Hutter, J. Ab Initio Molecular Dynamics: Basic Theory and Advanced Methods; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Frank, I.; Genuit, S.; Matz, F.; Oschinski, H. Ammonia, water, and hydrogen: Can nuclear motion be described classically? Int. J. Quantum Chem. 2020, 120, e26142. [Google Scholar] [CrossRef]
- Frank, I. Classical motion of the nuclei in a molecule: A concept without alternatives. Chem. Select 2020, 5, 1872. [Google Scholar] [CrossRef]
- Büchel, R.C.; Rudolph, D.A.; Frank, I. Deterministic quantum mechanics: The role of the Maxwell-Boltzmann distribution. Int. J. Quantum Chem. 2021, 121, e26555. [Google Scholar] [CrossRef]
- Frank, I. Classical nuclear motion: Comparison to approaches with quantum mechanical nuclear motion. Hydrogen 2023, 4, 11–21. [Google Scholar] [CrossRef]
- Yurchenko, S.N.; Barber, R.J.; Tennyson, J.; Thiel, W.; Jensen, P. Towards efficient refinement of molecular potential energy surfaces: Ammonia as a case study. J. Mol. Spectrosc. 2011, 268, 123. [Google Scholar] [CrossRef]
- Marquardt, R.; Sagui, K.; Zheng, J.; Thiel, W.; Luckhaus, D.; Yurchenko, S.N.; Mariotti, F.; Quack, M. Global analytical potential energy surface for the electronic ground state of NH3 from high level ab initio calculations. J. Phys. Chem. A 2013, 117, 7502. [Google Scholar] [CrossRef]
- Fabri, C.; Marquardt, R.; Csaszar, A.G.; Quack, M. Controlling tunneling in ammonia isotopomers. J. Chem. Phys. 2019, 150, 014102. [Google Scholar] [CrossRef]
- Hutter, J.; Alavi, A.; Deutsch, T.; Bernasconi, M.; Goedecker, S.; Marx, D.; Tuckerman, M.; Parrinello, M. CPMD Version 4.3. Copyright IBM Corp 1990–2015. Copyright MPI für Festkörperforschung Stuttgart 1997–2001. Available online: https://github.com/CPMD-code/CPMD/releases/tag/4.3 (accessed on 11 May 2023).
- Grimme, S. Accurate Description of van der Waals Complexes by Density Functional Theory Including Empirical Corrections. J. Comput. Chem. 2004, 25, 1463. [Google Scholar] [CrossRef] [PubMed]
- Troullier, N.; Martins, J.L. Efficient Pseudopotentials for Plane-Wave Calculations. Phys. Rev. B 1991, 43, 1993. [Google Scholar] [CrossRef]
- Boero, M.; Parrinello, M.; Terakura, K.; Weiss, H. Car-Parrinello study of Ziegler-Natta heterogeneous catalysis: Stability and destabilization problems of the active site models. Mol. Phys. 2002, 100, 2935–2940. [Google Scholar] [CrossRef]
- Brehm, M.; Kirchner, B. TRAVIS—A free analyzer and visualizer for monte carlo and molecular dynamics trajectories. J. Chem. Inf. Model. 2011, 51, 2007. [Google Scholar] [CrossRef] [PubMed]
- Brehm, M.; Thomas, M.; Gehrke, S.; Kirchner, B. TRAVIS—A free analyzer for trajectories from molecular simulation. J. Chem. Phys. 2020, 152, 164105. [Google Scholar] [CrossRef] [PubMed]
- Thomas, M.; Brehm, M.; Fligg, R.; Vöhringer, P.; Kirchner, B. Computing vibrational spectra from ab initio molecular dynamics. Phys. Chem. Chem. Phys. 2013, 15, 6608. [Google Scholar] [CrossRef] [PubMed]
- Thomas, M.; Brehm, M.; Kirchner, B. Voronoi dipole moments for the simulation of bulk phase vibrational spectra. Phys. Chem. Chem. Phys. 2015, 17, 3207. [Google Scholar] [CrossRef] [PubMed]
- Marzari, N.; Vanderbilt, D. Maximally localized generalized Wannier functions for composite energy bands. Phys. Rev. B 1997, 56, 12847. [Google Scholar] [CrossRef]
- Ikeda, T.; Boero, M. Role of van der Waals corrections in first principles simulations of alkali metal ions in aqueous solutions. J. Chem. Phys. 2015, 143, 194510. [Google Scholar] [CrossRef] [PubMed]
- Silvestrelli, P.L.; Ambrosetti, A. Van der Waals interactions in DFT using Wannier functions without empirical parameters. J. Chem. Phys. 2019, 150, 164109. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian~16 Revision A.03; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Becke, A. Density-Functional Exchange-Energy Approximation with Correct Asymptotic Behavior. Phys. Rev. A 1988, 38, 3098. [Google Scholar] [CrossRef] [PubMed]
- Becke, A. A New Mixing of Hartree-Fock and Local-Density Functional Theories. J. Chem. Phys. 1993, 98, 1372. [Google Scholar] [CrossRef]
- Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 2006, 27, 1787. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S. Semiempirical hybrid density functional with perturbative second-order correlation. J. Chem. Phys. 2006, 124, 034108. [Google Scholar] [CrossRef] [PubMed]
- NIST Chemistry WebBook. Available online: https://webbook.nist.gov/ (accessed on 30 December 2022).
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frank, I. Nuclear Motion Is Classical: Spectra of Hydrogen Chloride and Ammonia. Hydrogen 2023, 4, 287-294. https://doi.org/10.3390/hydrogen4020020
Frank I. Nuclear Motion Is Classical: Spectra of Hydrogen Chloride and Ammonia. Hydrogen. 2023; 4(2):287-294. https://doi.org/10.3390/hydrogen4020020
Chicago/Turabian StyleFrank, Irmgard. 2023. "Nuclear Motion Is Classical: Spectra of Hydrogen Chloride and Ammonia" Hydrogen 4, no. 2: 287-294. https://doi.org/10.3390/hydrogen4020020
APA StyleFrank, I. (2023). Nuclear Motion Is Classical: Spectra of Hydrogen Chloride and Ammonia. Hydrogen, 4(2), 287-294. https://doi.org/10.3390/hydrogen4020020






