Experimental Study of the Parameter Mismatch Effects on the Low Frequency Circulating Currents of Parallel Three Phase Inverters
Abstract
1. Introduction
1.1. Potential Applications of Parallel Inverters
1.1.1. Active Front-End
1.1.2. Photovoltaic Inverters
1.1.3. Interlinking Converters in Microgrids
1.2. The Problem of Circulating Currents and the Main Contribution of This Work
2. Low-Frequency Circulating Currents’ Nature
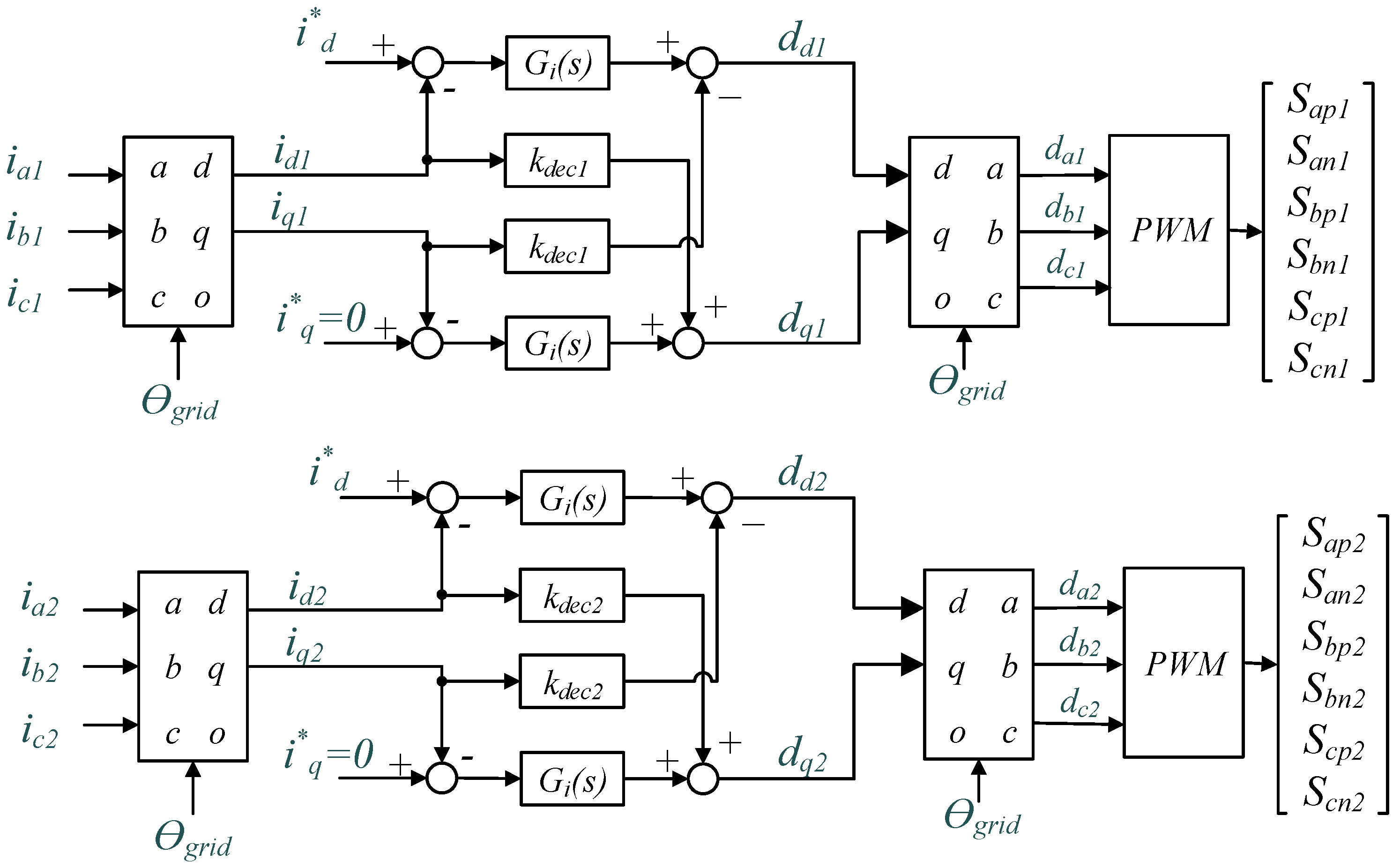
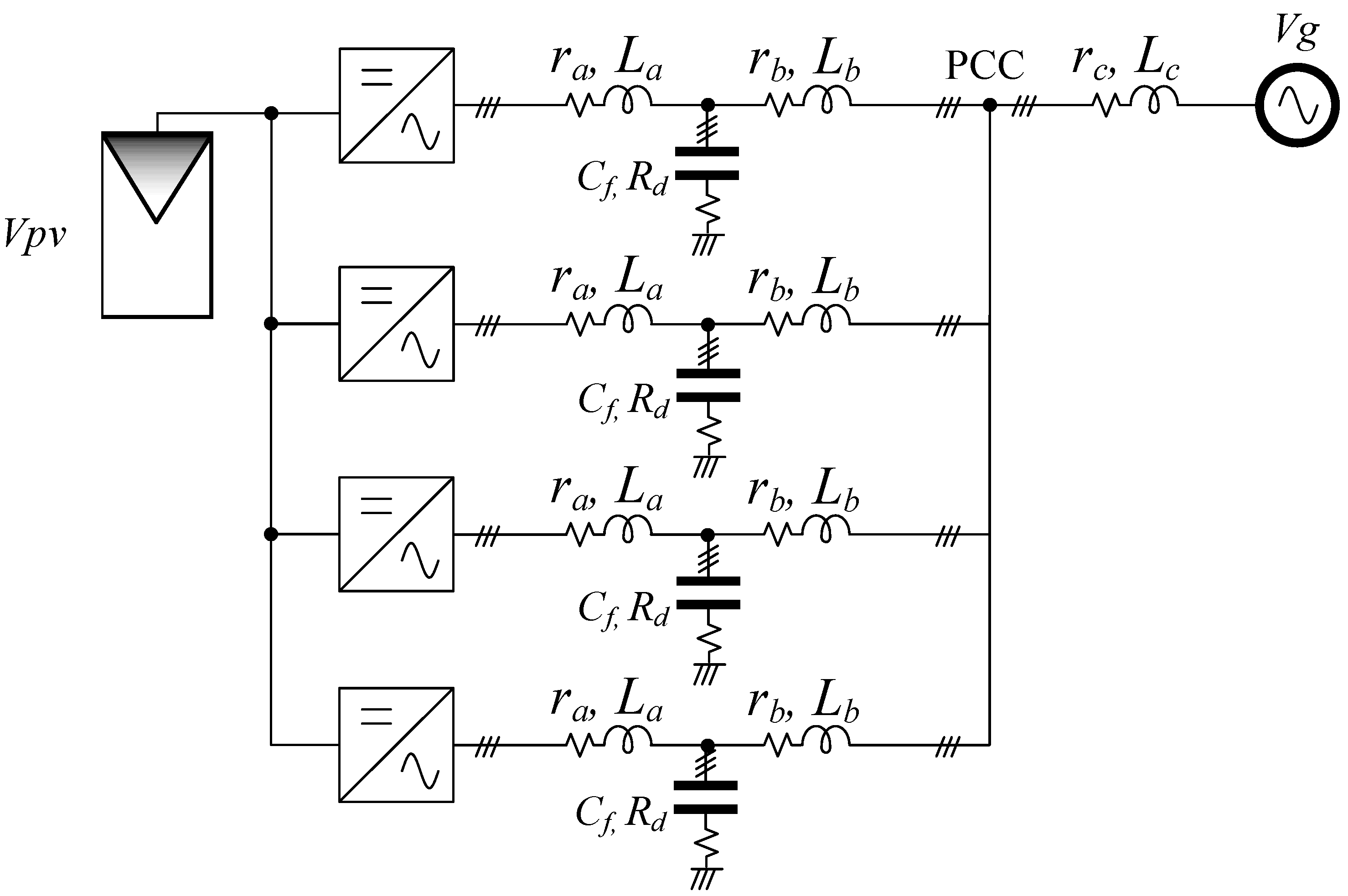
3. Simulation and Experimental Results
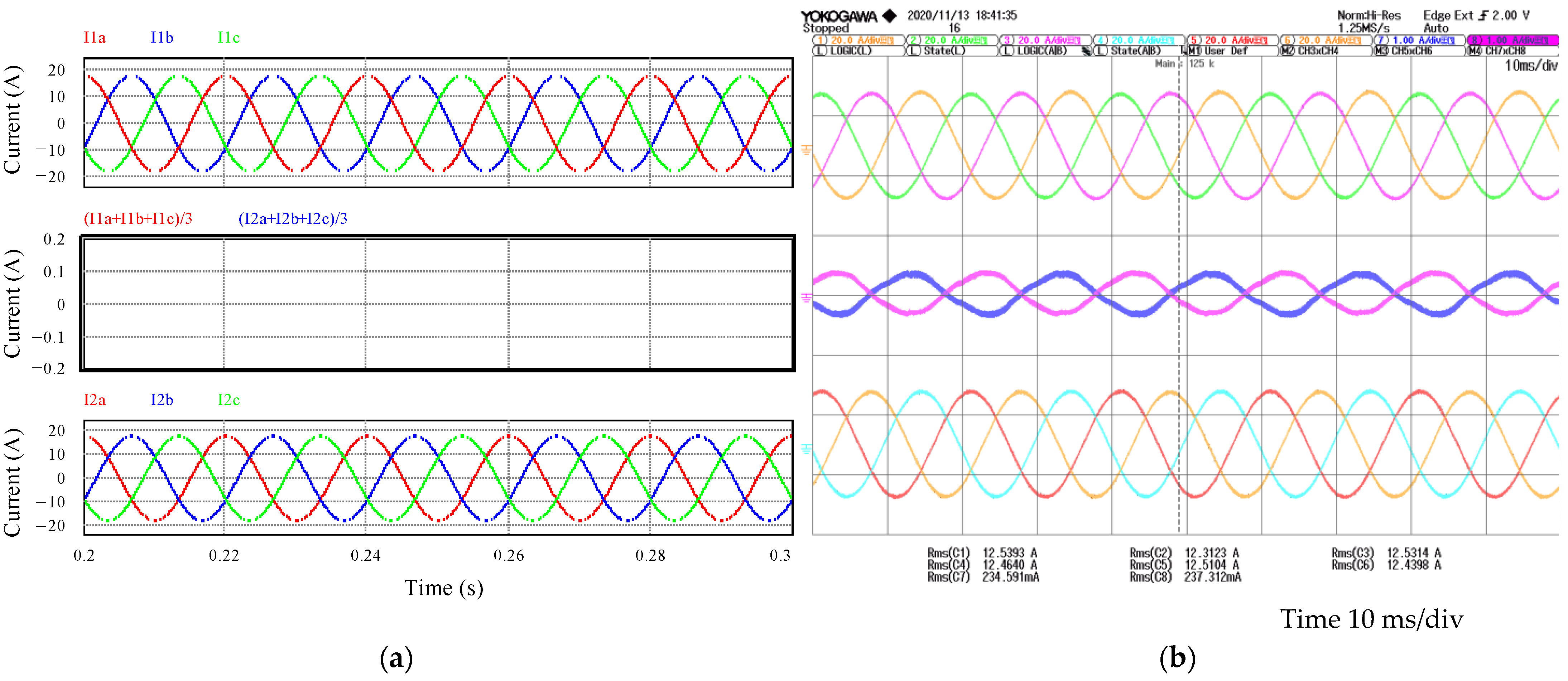
3.1. Circulating Currents in a Balanced System
3.2. Circulating Currents with a Mismatch between the Inductances of the Inverters
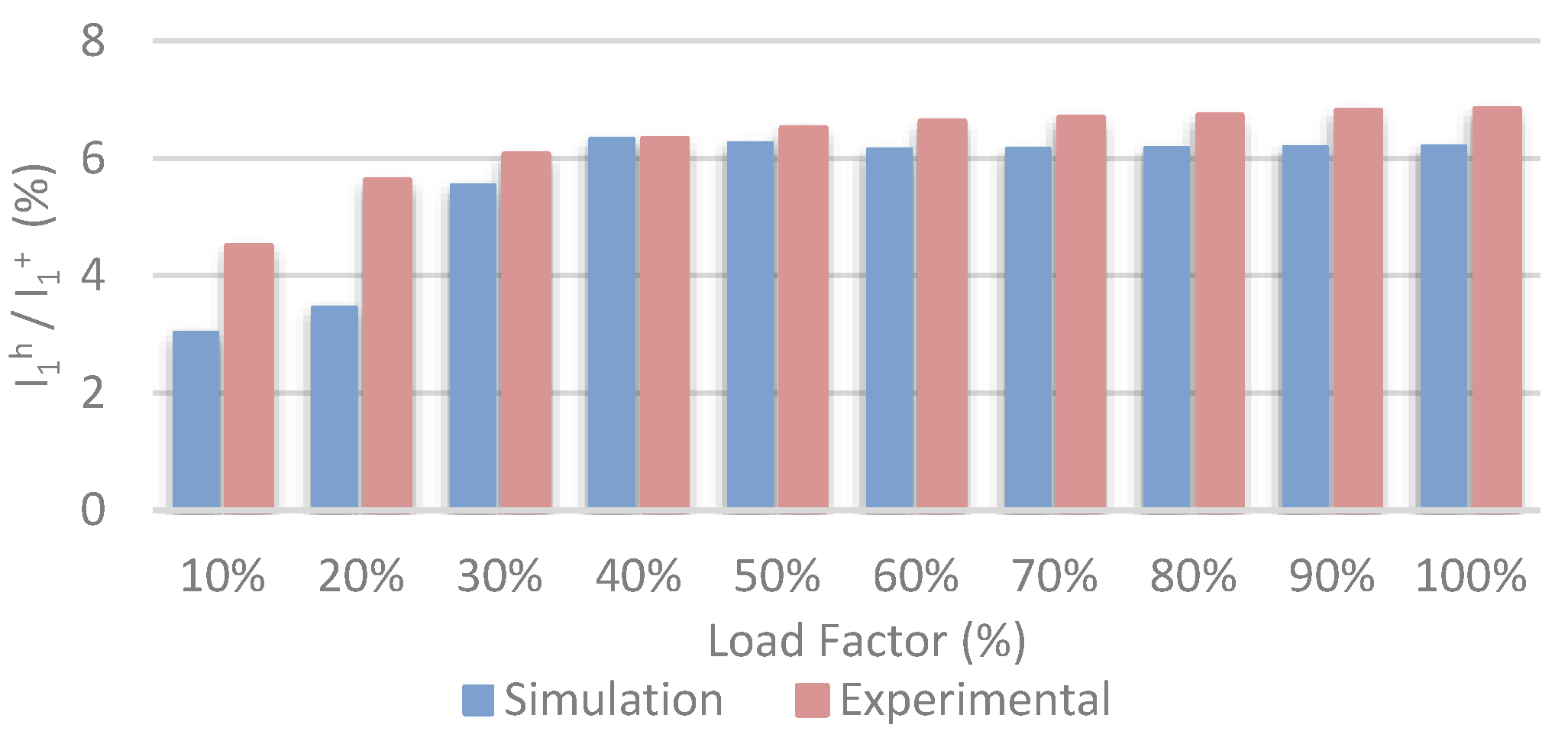
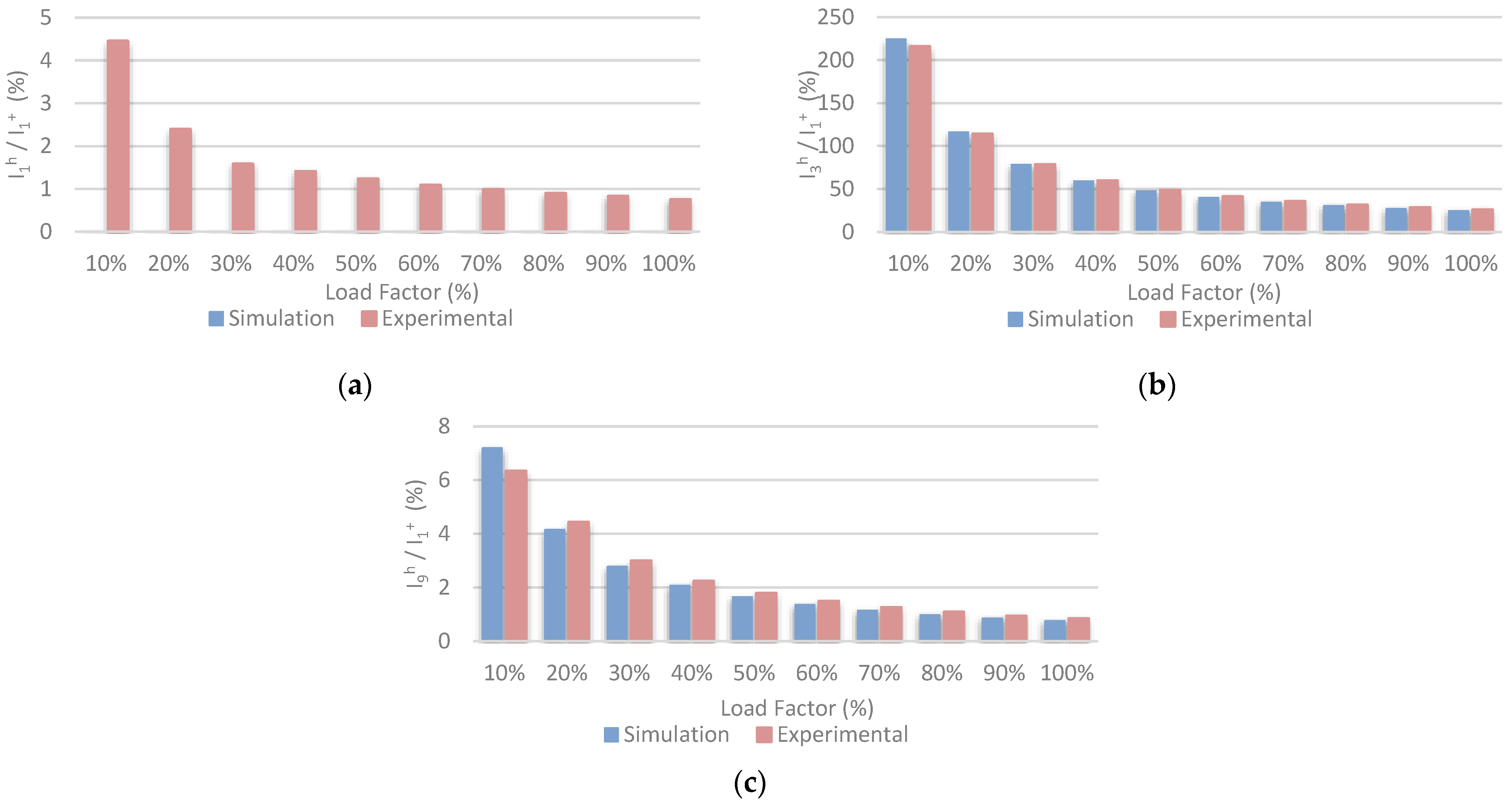
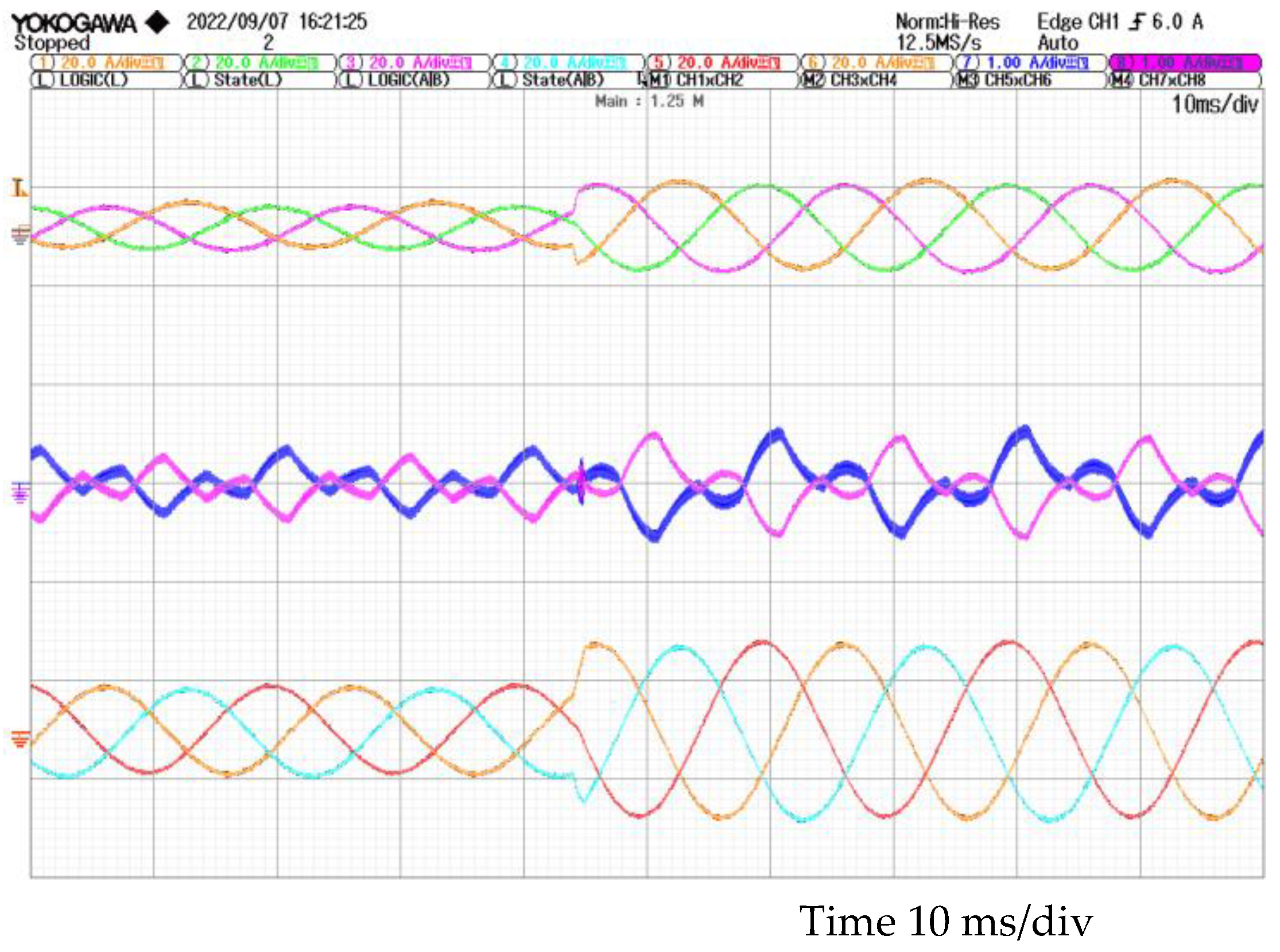
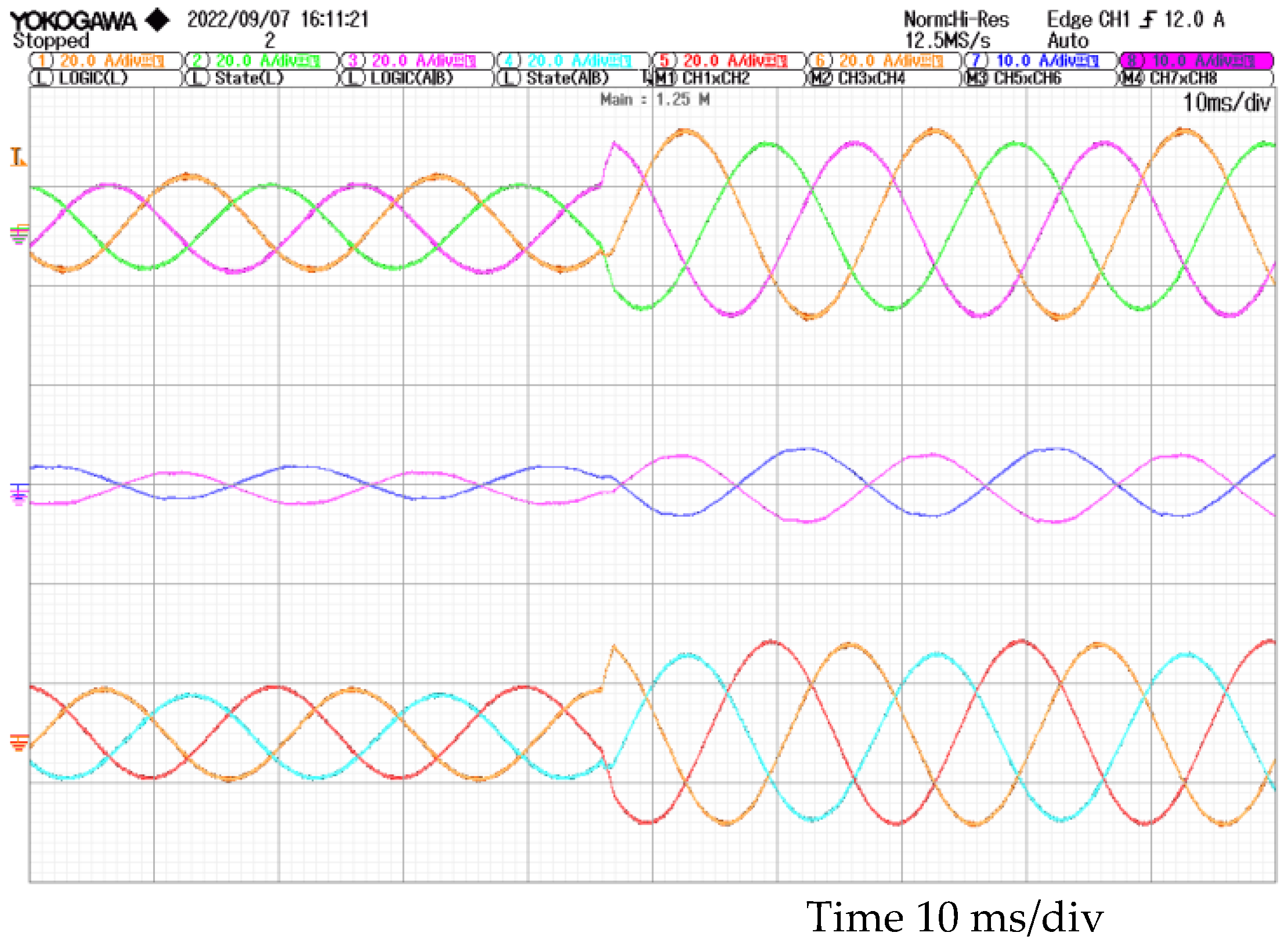
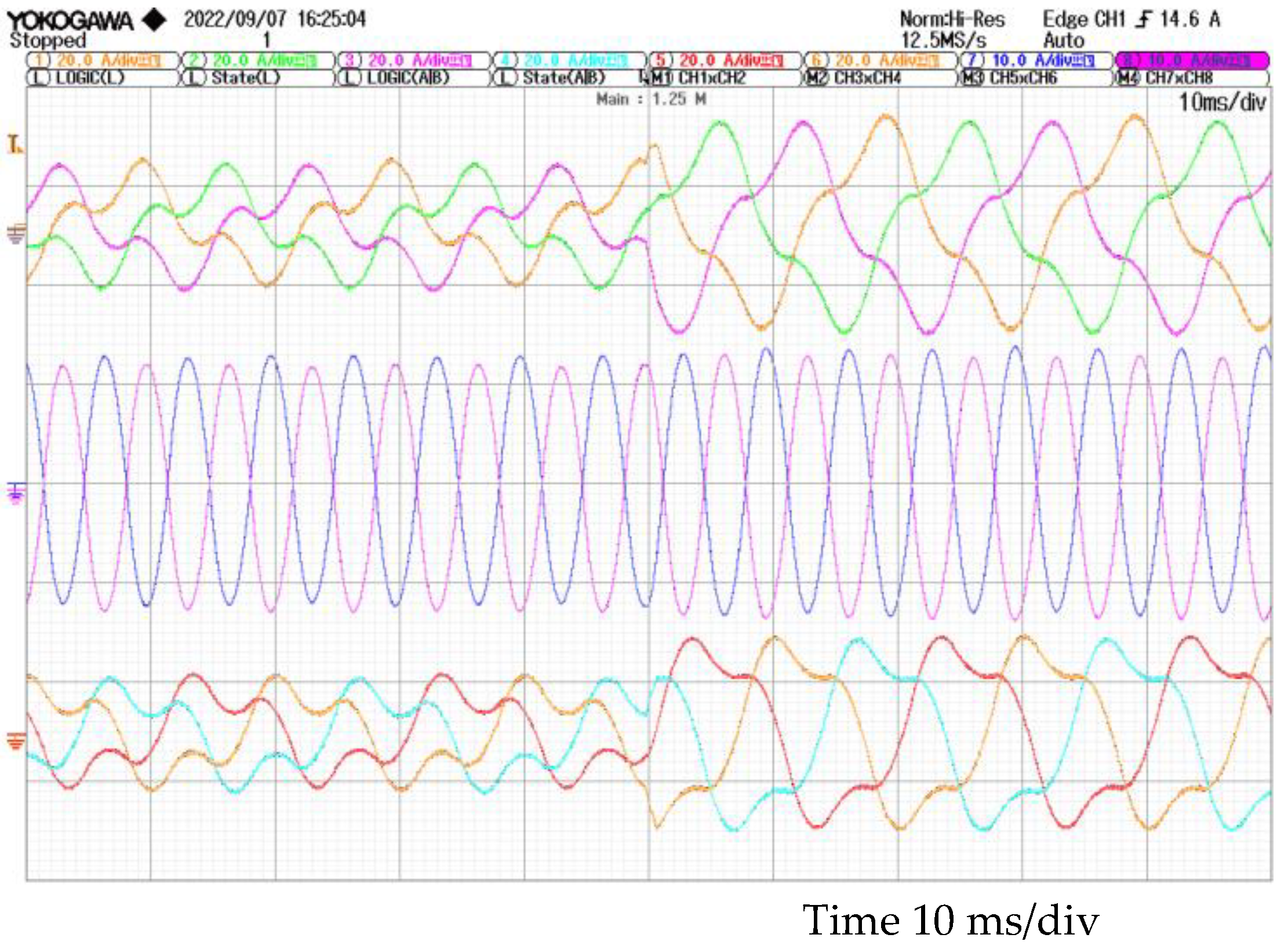
3.3. Circulating Currents with an Unbalanced Load Factor between Inverters
3.4. Circulating Currents with a Mismatch between the Inductances of the Same Inverter
3.5. Circulating Currents with Different Modulation Techniques between Inverters
3.6. Current Sharing in Dynamic Conditions
4. Circulating Currents in Photovoltaic High-Power Applications
5. Discussion and Comparison with Previous Works
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aleem, Z.; Shin, D.; Cha, H.; Lee, J.-P.; Yoo, D.-W.; Peng, F.Z. Parallel Operation of Inverter Using Trans-Z-Source Network. IET Power Electron. 2015, 8, 2176–2183. [Google Scholar] [CrossRef]
- Asiminoaei, L.; Aeloiza, E.; Enjeti, P.N.; Blaabjerg, F. Shunt Active-Power-Filter Topology Based on Parallel Interleaved Inverters. IEEE Trans. Ind. Electron. 2008, 55, 1175–1189. [Google Scholar] [CrossRef]
- Shao, Z.; Zhang, X.; Wang, F.; Cao, R. Modeling and Elimination of Zero-Sequence Circulating Currents in Parallel Three-Level T-Type Grid-Connected Inverters. IEEE Trans. Power Electron. 2015, 30, 1050–1063. [Google Scholar] [CrossRef]
- Abusara, M.A.; Sharkh, S.M. Design and Control of a Grid-Connected Interleaved Inverter. IEEE Trans. Power Electron. 2013, 28, 748–764. [Google Scholar] [CrossRef]
- Kim, K.-T.; Kwon, J.-M.; Kwon, B.-H. Parallel Operation of Photovoltaic Power Conditioning System Modules for Large-Scale Photovoltaic Power Generation. IET Power Electron. 2013, 7, 406–417. [Google Scholar] [CrossRef]
- Liberos, M.; González-Medina, R.; Garcerá, G.; Figueres, E. A Method to Enhance the Global Efficiency of High-Power Photovoltaic Inverters Connected in Parallel. Energies 2019, 12, 2219. [Google Scholar] [CrossRef]
- Matijević, E.; Sharma, R.; Zare, F. A Higher-Order Filter Approach to Implement Grid Current Based Active Damping in Active Front End Converters. In Proceedings of the 2020 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Jaipur, India, 16–19 December 2020; pp. 1–6. [Google Scholar]
- Zare, F.; Yaghoobi, J.; Gharani, K.; Kumar, D. Harmonic Cancellations in Parallel Active Front End Inverters in Distribution Networks: IEC 61000-3-16 and Phase-Angles. In Proceedings of the 2020 19th International Conference on Harmonics and Quality of Power (ICHQP), Dubai, United Arab Emirates, 6–7 July 2020; pp. 1–6. [Google Scholar]
- Hou, C.-C. A Multicarrier PWM for Parallel Three-Phase Active Front-End Converters. IEEE Trans. Power Electron. 2013, 28, 2753–2759. [Google Scholar] [CrossRef]
- Duman, T.; Marti, S.; Moonem, M.A.; Abdul Kader, A.A.R.; Krishnaswami, H. A Modular Multilevel Converter with Power Mismatch Control for Grid-Connected Photovoltaic Systems. Energies 2017, 10, 698. [Google Scholar] [CrossRef]
- Araujo, S.V.; Zacharias, P.; Mallwitz, R. Highly Efficient Single-Phase Transformerless Inverters for Grid-Connected Photovoltaic Systems. IEEE Trans. Ind. Electron. 2010, 57, 3118–3128. [Google Scholar] [CrossRef]
- Dagar, A.; Gupta, P.; Niranjan, V. Microgrid Protection: A Comprehensive Review. Renew. Sustain. Energy Rev. 2021, 149, 111401. [Google Scholar] [CrossRef]
- Zolfaghari, M.; Abedi, M.; Gharehpetian, G.B. Robust Nonlinear State Feedback Control of Bidirectional Interlink Power Converters in Grid-Connected Hybrid Microgrids. IEEE Syst. J. 2020, 14, 1117–1124. [Google Scholar] [CrossRef]
- Lyu, J.; Zhang, J.; Cai, X.; Wang, H.; Dai, J. Circulating Current Control Strategy for Parallel Full-Scale Wind Power Converters. IET Power Electron. 2016, 9, 639–647. [Google Scholar] [CrossRef]
- Jiang, W.; Ma, W.; Wang, J.; Wang, W.; Zhang, X.; Wang, L. Suppression of Zero Sequence Circulating Current for Parallel Three-Phase Grid-Connected Converters Using Hybrid Modulation Strategy. IEEE Trans. Ind. Electron. 2018, 65, 3017–3026. [Google Scholar] [CrossRef]
- Narimani, M.; Moschopoulos, G. Improved Method for Paralleling Reduced Switch VSI Modules: Harmonic Content and Circulating Current. IEEE Trans. Power Electron. 2014, 29, 3308–3317. [Google Scholar] [CrossRef]
- Fu, X.; Wang, H.; Guo, X.; Shi, C.; Jia, D.; Chen, C.; Guerrero, J.M. A Novel Circulating Current Suppression for Paralleled Current Source Converter Based on Virtual Impedance Concept. Energies 2022, 15, 1952. [Google Scholar] [CrossRef]
- Prasad, J.S.S.; Ghosh, R.; Narayanan, G. Common-Mode Injection PWM for Parallel Converters. IEEE Trans. Ind. Electron. 2015, 62, 789–794. [Google Scholar] [CrossRef]
- Abbes, M.; Mehouachi, I.; Chebbi, S. Circulating Current Reduction of a Grid-Connected Parallel Interleaved Converter Using Energy Shaping Control. Electr. Power Syst. Res. 2019, 170, 184–193. [Google Scholar] [CrossRef]
- Zhang, P.; Zhang, G.; Du, H. Circulating Current Suppression of Parallel Photovoltaic Grid-Connected Converters. IEEE Trans. Circuits Syst. II Express Briefs 2018, 65, 1214–1218. [Google Scholar] [CrossRef]
- Ye, Z.; Boroyevich, D.; Lee, F.C. Modeling and Control of Zero-Sequence Current in Parallel Multi-Phase Converters. In Proceedings of the 2000 IEEE 31st Annual Power Electronics Specialists Conference. Conference Proceedings (Cat. No.00CH37018), Galway, Ireland, 23 June 2000; Volume 2, pp. 680–685. [Google Scholar]
- Mazumder, S.K. A Novel Discrete Control Strategy for Independent Stabilization of Parallel Three-Phase Boost Converters by Combining Space-Vector Modulation with Variable-Structure Control. IEEE Trans. Power Electron. 2003, 18, 1070–1083. [Google Scholar] [CrossRef]
- Pan, C.-T.; Liao, Y.-H. Modeling and Control of Circulating Currents for Parallel Three-Phase Boost Rectifiers with Different Load Sharing. IEEE Trans. Ind. Electron. 2008, 55, 2776–2785. [Google Scholar] [CrossRef]
- Ogasawara, S.; Takagaki, J.; Akagi, H.; Nabae, A. A Novel Control Scheme of a Parallel Current-Controlled PWM Inverter. IEEE Trans. Ind. Appl. 1992, 28, 1023–1030. [Google Scholar] [CrossRef]
- Chen, T. Dual-Modulator Compensation Technique for Parallel Inverters Using Space-Vector Modulation. IEEE Trans. Ind. Electron. 2009, 56, 3004–3012. [Google Scholar] [CrossRef]
- Liberos, M.; González-Medina, R.; Patrao, I.; Garcerá, G.; Figueres, E. A Control Scheme to Suppress Circulating Currents in Parallel-Connected Three-Phase Inverters. Electronics 2022, 11, 3720. [Google Scholar] [CrossRef]
- Maheshwari, R.; Gohil, G.; Bede, L.; Munk-Nielsen, S. Analysis and Modelling of Circulating Current in Two Parallel-Connected Inverters. IET Power Electron. 2015, 8, 1273–1283. [Google Scholar] [CrossRef]
- Roslan, M.A.; Ahmad, M.S.; Isa, M.A.M.; Rahman, N.H.A. Circulating Current in Parallel Connected Inverter System. In Proceedings of the 2016 IEEE International Conference on Power and Energy (PECon), Melaka, Malaysia, 28–29 November 2016; pp. 172–177. [Google Scholar]
- Erickson, R.W.; Maksimovic, D. Fundamentals of Power Electronics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2001; ISBN 978-0-7923-7270-7. [Google Scholar]
- Figueres, E.; Garcera, G.; Sandia, J.; Gonzalez-Espin, F.; Calvo Rubio, J. Sensitivity Study of the Dynamics of Three-Phase Photovoltaic Inverters with an LCL Grid Filter. IEEE Trans. Ind. Electron. 2009, 56, 706–717. [Google Scholar] [CrossRef]
- Holmes, D.G.; Lipo, T.A. Pulse Width Modulation for Power Converters: Principles and Practice; John Wiley & Sons: Hoboken, NJ, USA, 2003; ISBN 978-0-471-20814-3. [Google Scholar]

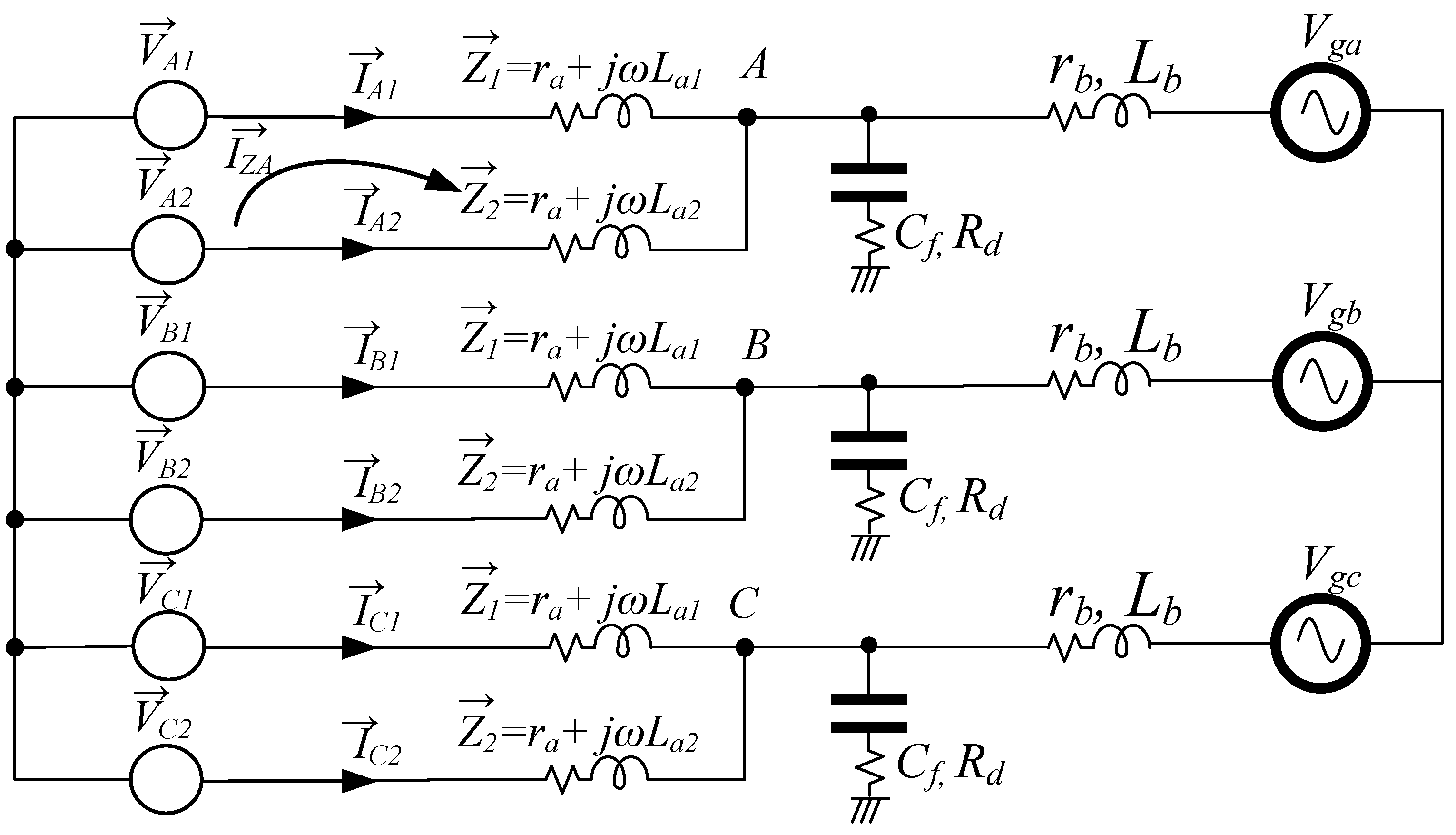










| Parameter | Nominal Value | Parameter | Nominal Value |
|---|---|---|---|
| Vg-RMS (phase-phase) | 230 V | Mb | −80 µH |
| Vdc | 500 V | ra | 50 mΩ |
| Pn | 5 kW | rb | 50 mΩ |
| fg | 50 Hz | Cf | 9 µF |
| Co | 1.2 mF | Rd | 4.4 Ω |
| La | 5 mH | fsw | 10 kHz |
| Lb | 320 µH |
| Parameter | Nominal Value | Parameter | Nominal Value |
|---|---|---|---|
| La_a1 | 5.14 mH | La_a2 | 5.1 mH |
| La_b1 | 5.14 mH | La_b2 | 4.85 mH |
| La_c1 | 5.27 mH | La_c2 | 5.03 mH |
| Parameter | 5-mH Inductor | Parameter | 5-mH Inductor | 2-mH Inductor | 7-mH Total Inductance |
|---|---|---|---|---|---|
| La_a1 | 5.14 mH | La_a2 | 5.1 mH | 2.06 mH | 7.16 mH |
| La_b1 | 5.14 mH | La_b2 | 4.85 mH | 2.13 mH | 6.98 mH |
| La_c1 | 5.27 mH | La_c2 | 5.03 mH | 2.09 mH | 7.12 mH |
| Parameter | Nominal Value | Real Value | Parameter | Nominal Value | Real Value |
|---|---|---|---|---|---|
| La_a1 | 5 mH | 5.14 mH | La_a2 | 7 mH | 7.16 mH |
| La_b1 | 5 mH | 5.14 mH | La_b2 | 5 mH | 4.85 mH |
| La_c1 | 5 mH | 5.27 mH | La_c2 | 5 mH | 5.03 mH |
| Parameter | Nominal Value | Parameter | Nominal Value |
|---|---|---|---|
| Vg-RMS (phase-phase) | 400 V | Mb | −15 µH |
| Vpv | [650–820] V | Mc | 0 |
| Pn | 500 kW | ra | 1 mΩ |
| fg | 50 Hz | rb | 1 mΩ |
| Co | 15 mF | rc | 1 mΩ |
| La | 160 µH | Cf | 500 µF |
| Lb | 60 µH | Rd | 0.12 Ω |
| Lc | [2.5–50] µH | fsw | 2 kHz |
| Ma | −40 µH |
| Mismatches | Inductances (Different Inverters) | Load Factor | Voltage Frequency | Voltage Phase | Inductances (Same Inverter) | Phase Shift PWM | Modulation Technique |
|---|---|---|---|---|---|---|---|
| Results obtained in this work | |||||||
| I1h/I1+ | 0.01% | 0.644% | - | - | 6.68% | - | 0.98% |
| I3h/I1+ | 0.03% | 0.44% | - | - | 0.007% | - | 35.41% |
| IZ RMS | 0.057 A | 0.08 A | - | - | 0.697 A | - | 3.14 A |
| THDi Ia | 0.58% | 1.25% | - | - | 0.64% | - | 30.91% |
| Previous work [27] | |||||||
| I1h/I1+ | - | - | - | - | - | 1.83% | - |
| I3h/I1+ | - | - | - | - | - | 6.25% | - |
| IZ RMS | - | - | - | - | - | 0.88 A | - |
| THDi Ia | - | - | - | - | - | - | - |
| Previous work [28] | |||||||
| I1h/I1+ | - | - | - | - | - | - | - |
| I3h/I1+ | - | - | - | - | - | - | - |
| IZ RMS | - | - | 0.096 A | 0.24A | - | - | - |
| THDi Ia | - | - | - | - | - | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liberos, M.; González-Medina, R.; Patrao, I.; Torán, E.; Garcerá, G.; Figueres, E. Experimental Study of the Parameter Mismatch Effects on the Low Frequency Circulating Currents of Parallel Three Phase Inverters. Eng 2023, 4, 1356-1376. https://doi.org/10.3390/eng4020079
Liberos M, González-Medina R, Patrao I, Torán E, Garcerá G, Figueres E. Experimental Study of the Parameter Mismatch Effects on the Low Frequency Circulating Currents of Parallel Three Phase Inverters. Eng. 2023; 4(2):1356-1376. https://doi.org/10.3390/eng4020079
Chicago/Turabian StyleLiberos, Marian, Raúl González-Medina, Iván Patrao, Enric Torán, Gabriel Garcerá, and Emilio Figueres. 2023. "Experimental Study of the Parameter Mismatch Effects on the Low Frequency Circulating Currents of Parallel Three Phase Inverters" Eng 4, no. 2: 1356-1376. https://doi.org/10.3390/eng4020079
APA StyleLiberos, M., González-Medina, R., Patrao, I., Torán, E., Garcerá, G., & Figueres, E. (2023). Experimental Study of the Parameter Mismatch Effects on the Low Frequency Circulating Currents of Parallel Three Phase Inverters. Eng, 4(2), 1356-1376. https://doi.org/10.3390/eng4020079






