Soil–Structure Interaction Effects on Seismic Responses of a Conventional and Isolated Bridge Subjected to Moderate Near-Fault and Far-Field Records
Abstract
1. Introduction
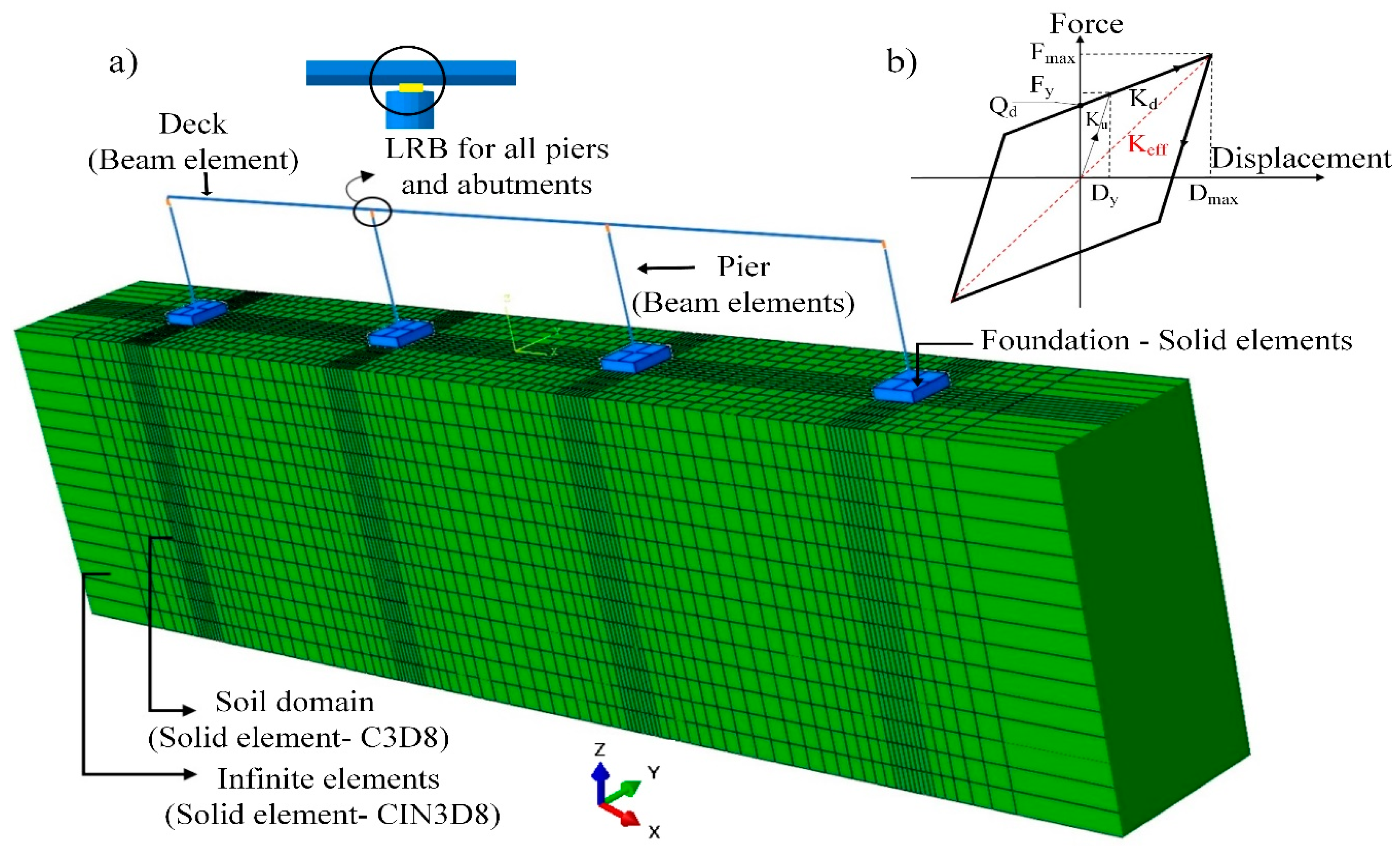
2. Numerical Modeling
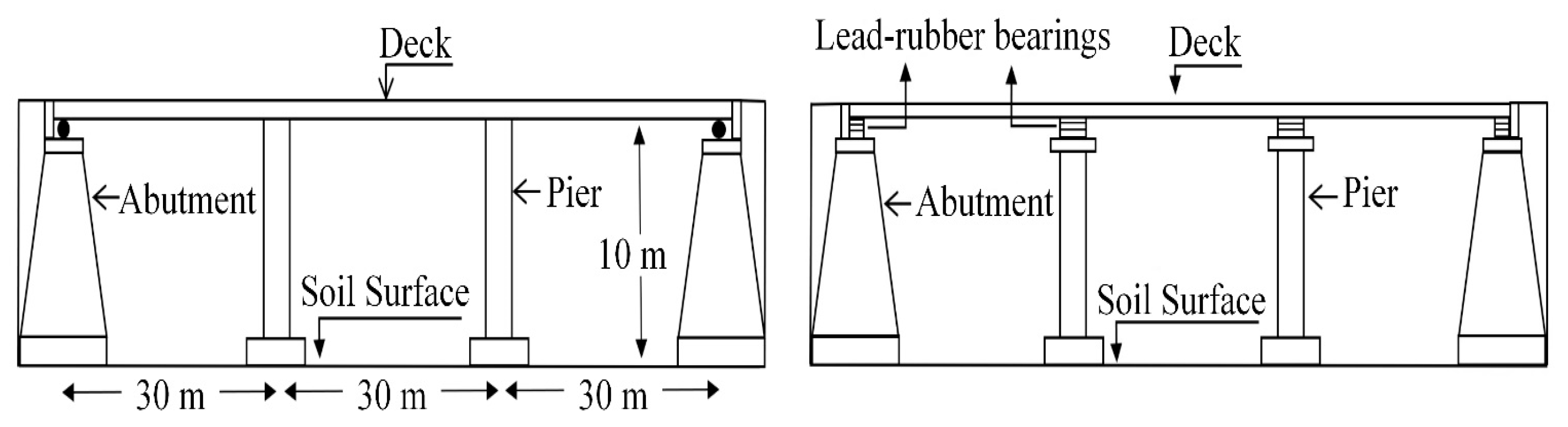
2.1. Case Study and Modeling of the Bridge
2.2. Isolation System
2.3. Direct Approach
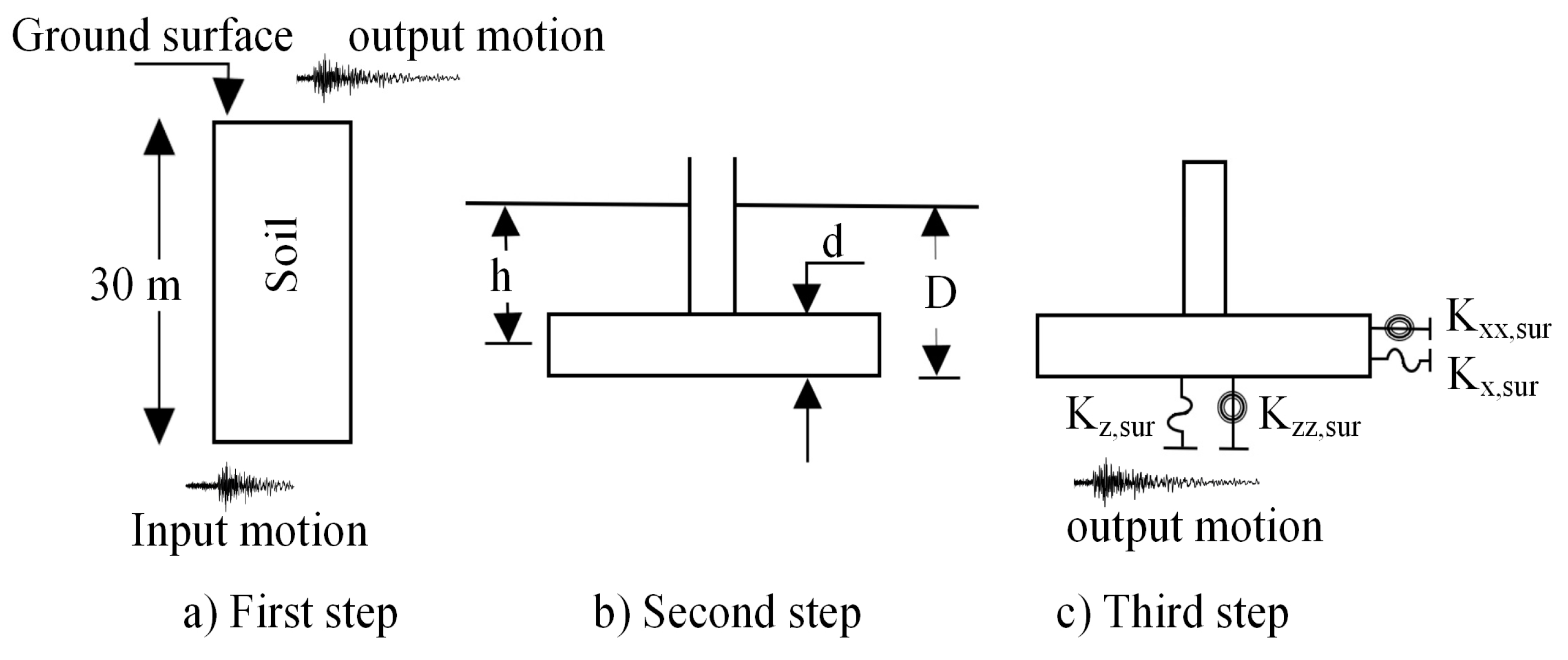
2.3.1. Soil Model and Properties
2.3.2. Soil Boundary Conditions
2.4. Simplified Approach
3. Seismic Analyses
3.1. Earthquake Record Selection and Calibration
3.2. Analysis Program and Procedure
4. Results and Discussion
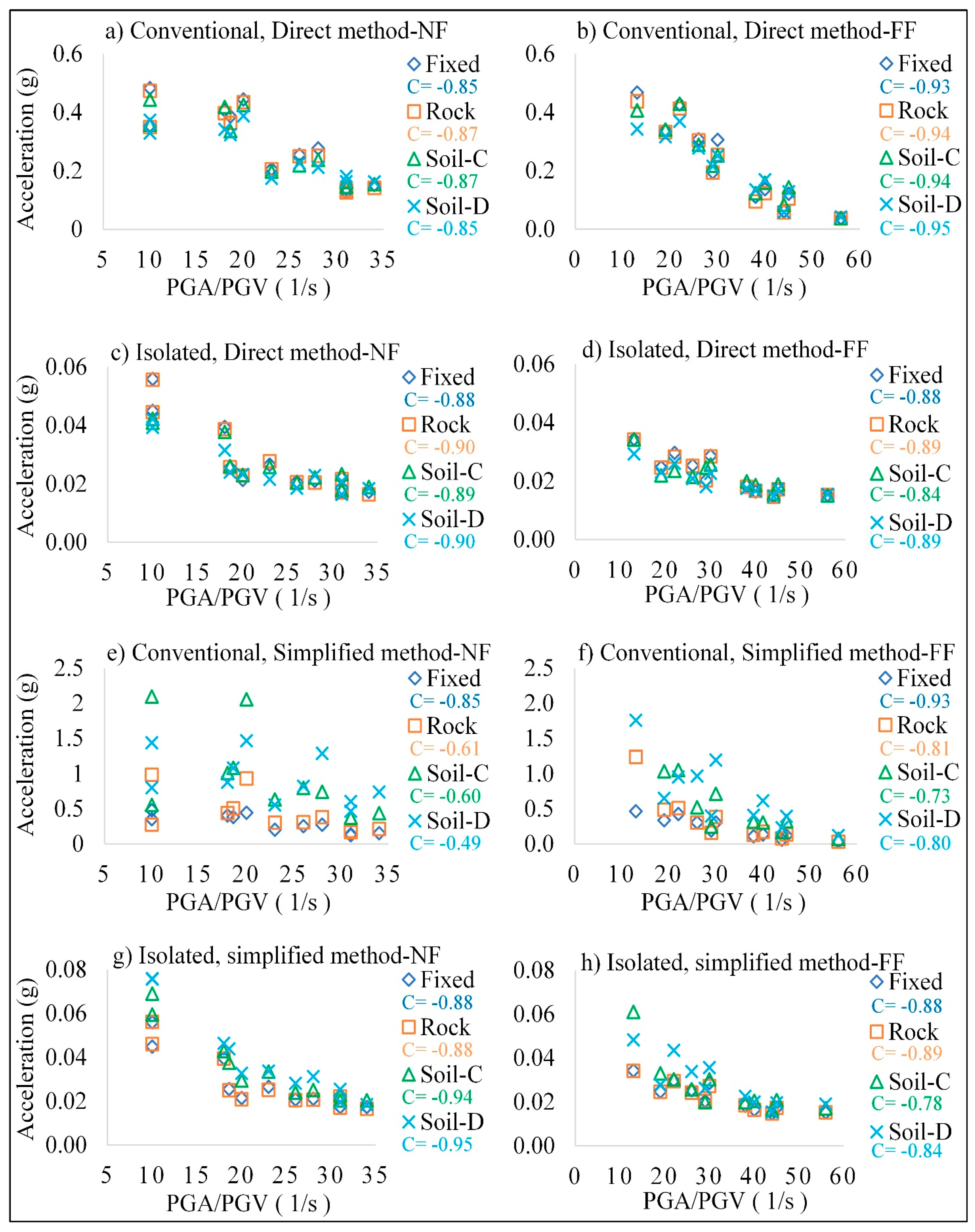
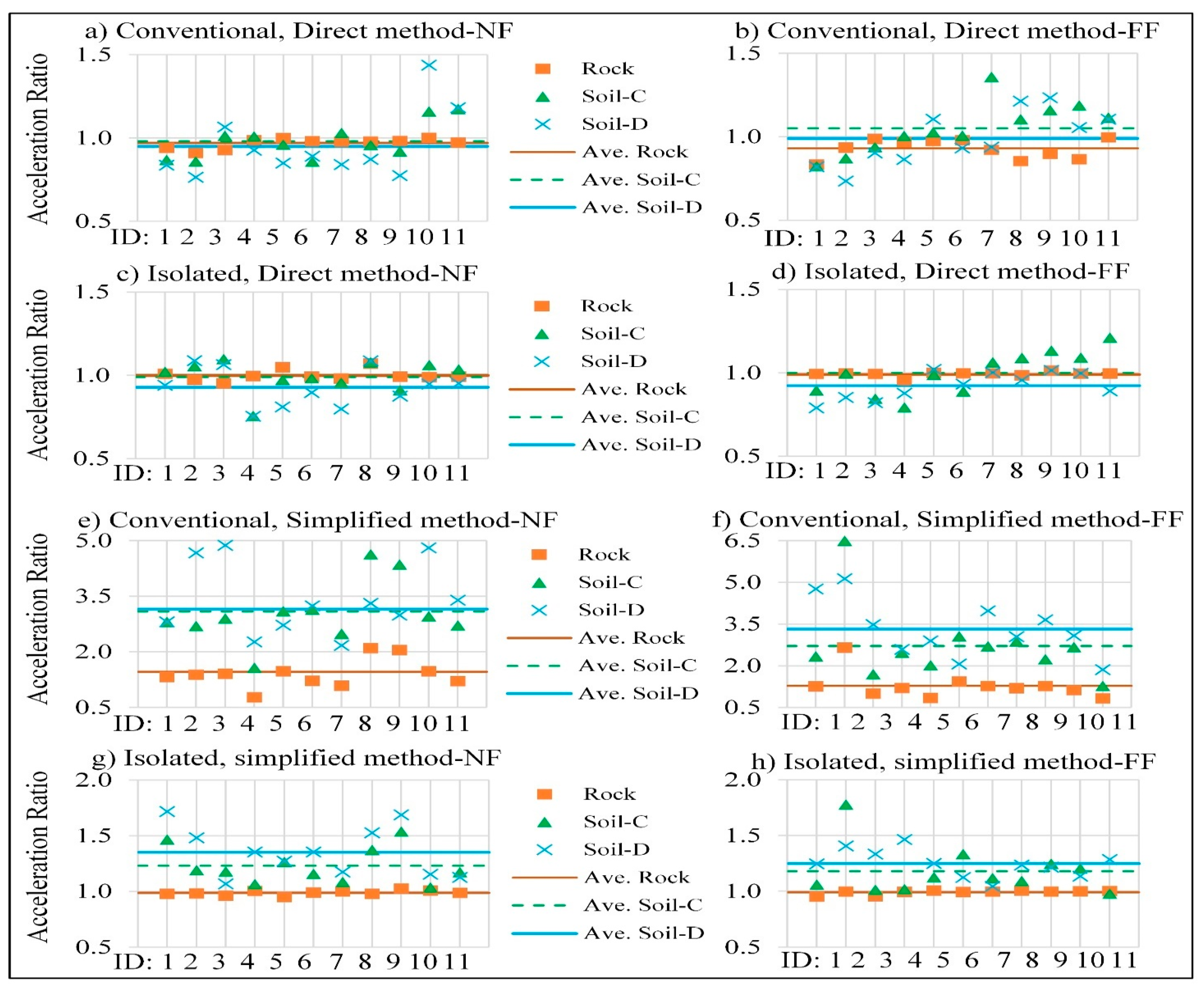
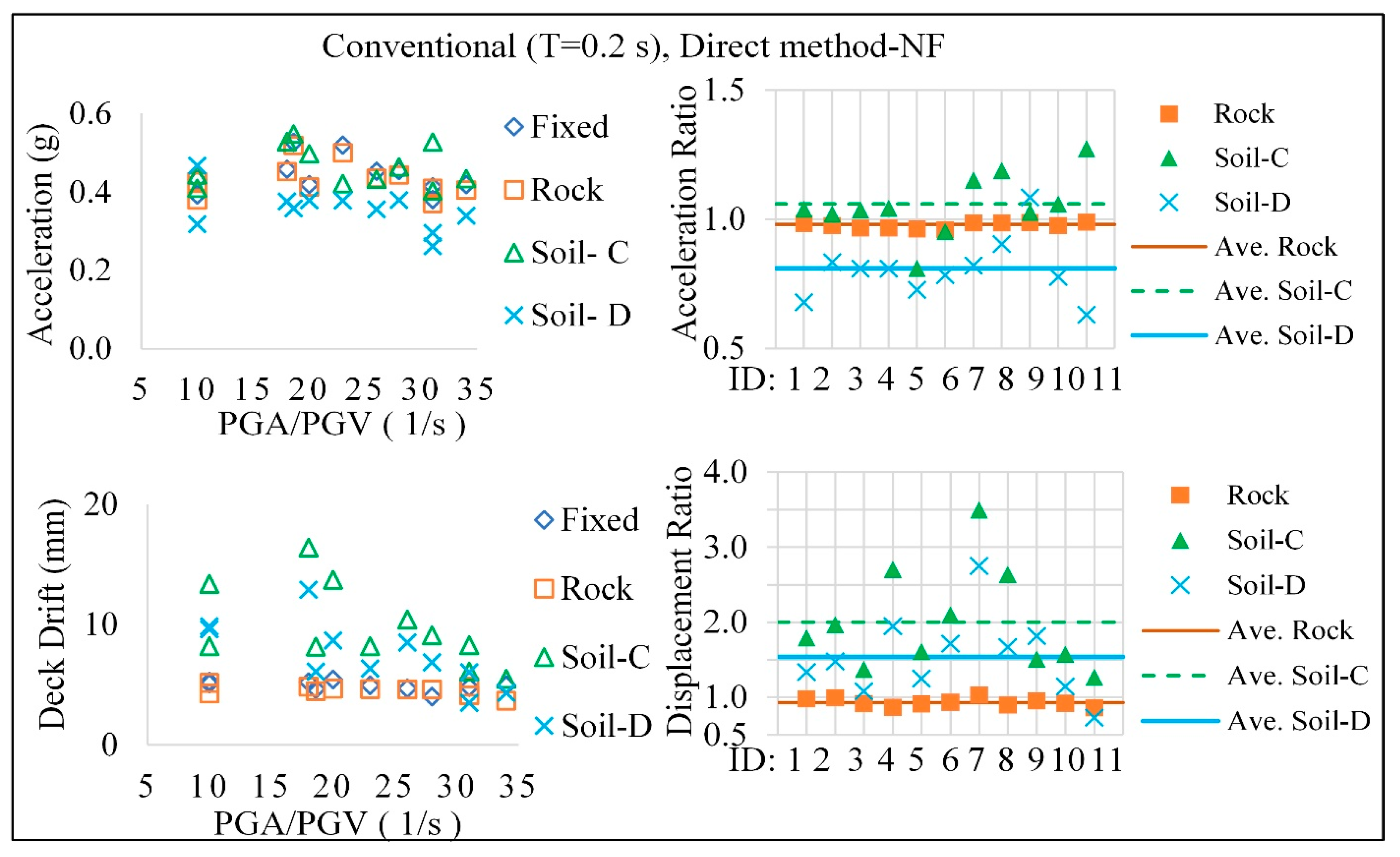
4.1. Effect of Earthquake Characteristics and SSI on the Acceleration Responses
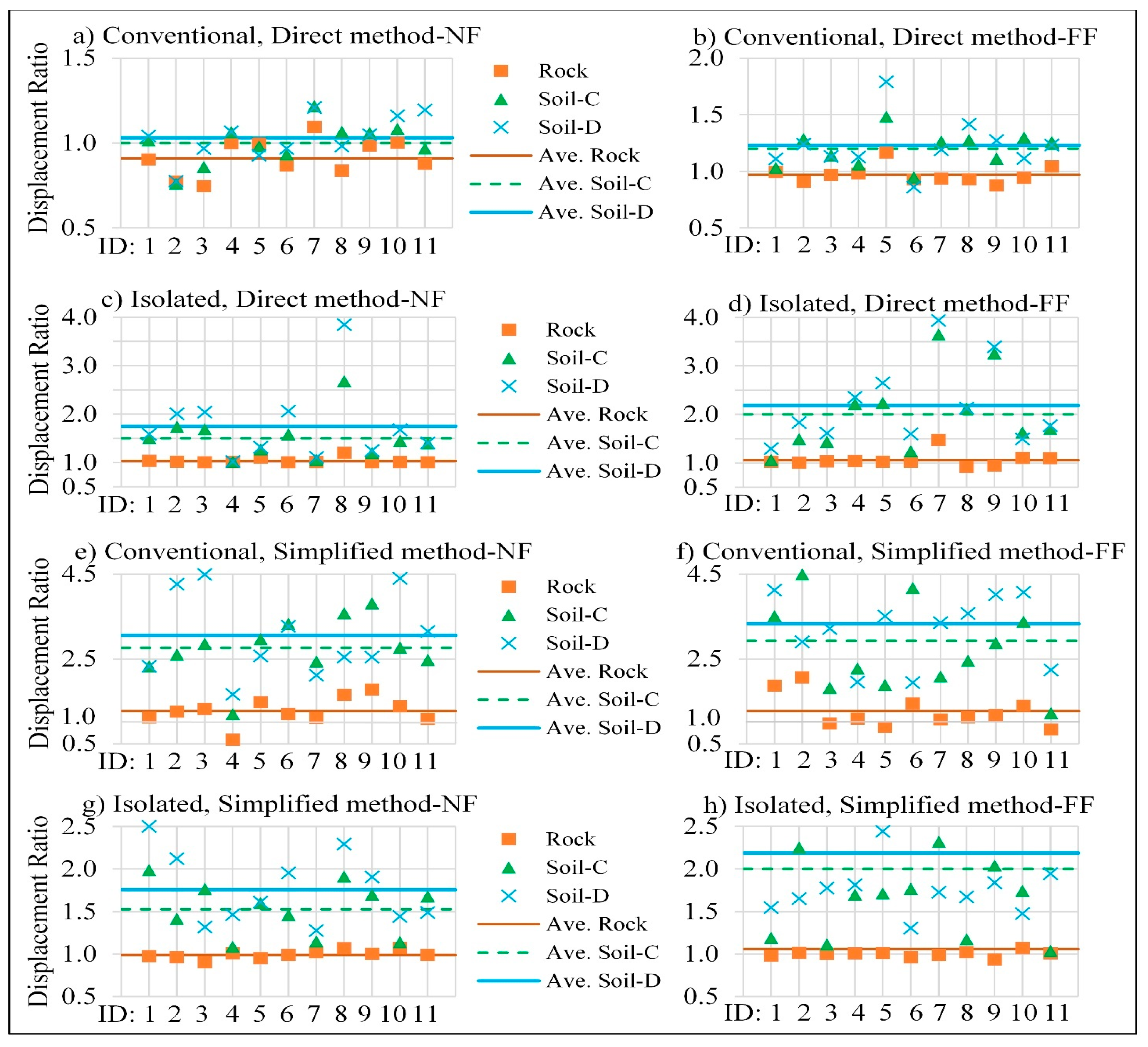
4.2. Effect of Earthquake Characteristics and SSI on the Displacement Responses
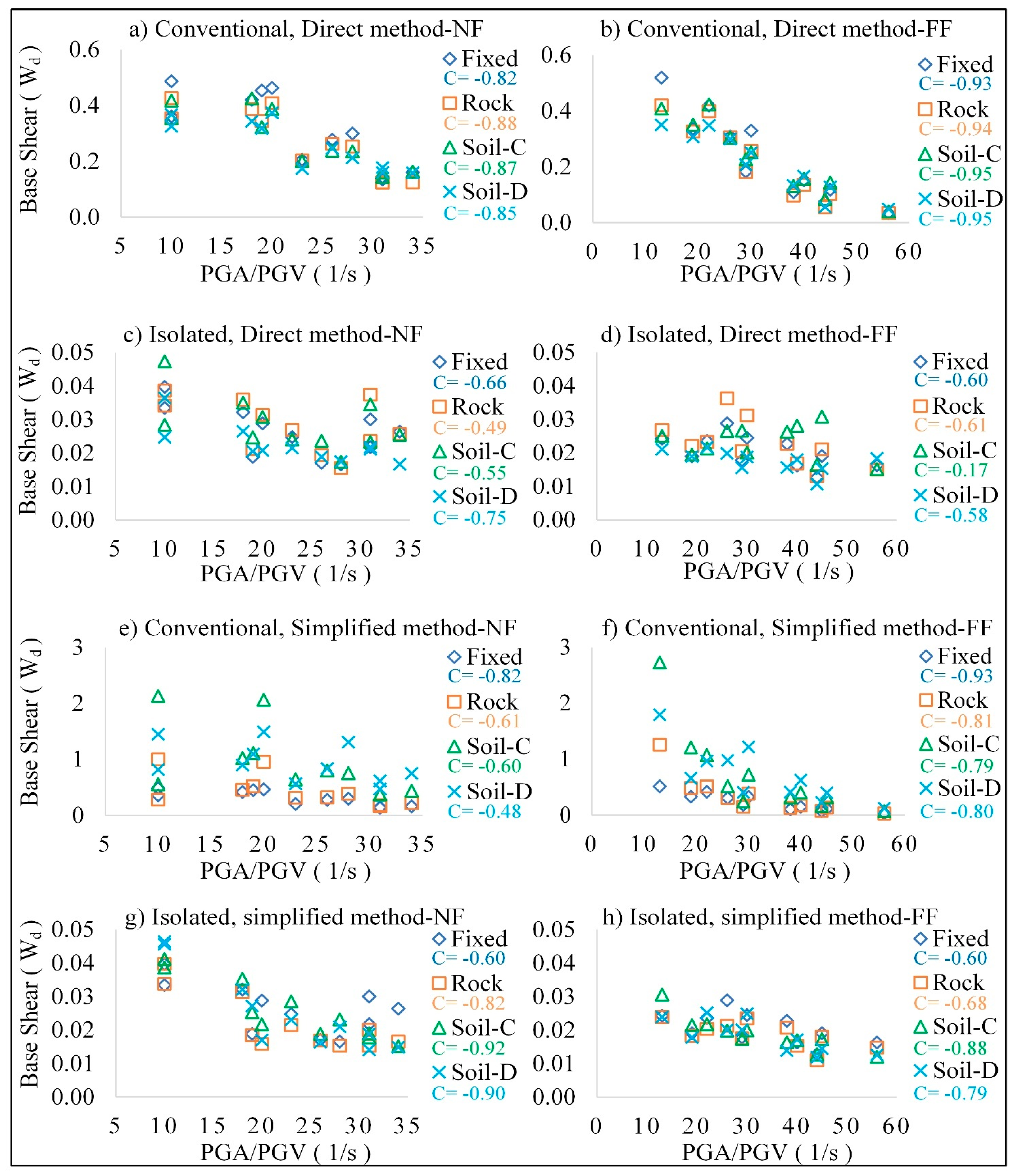
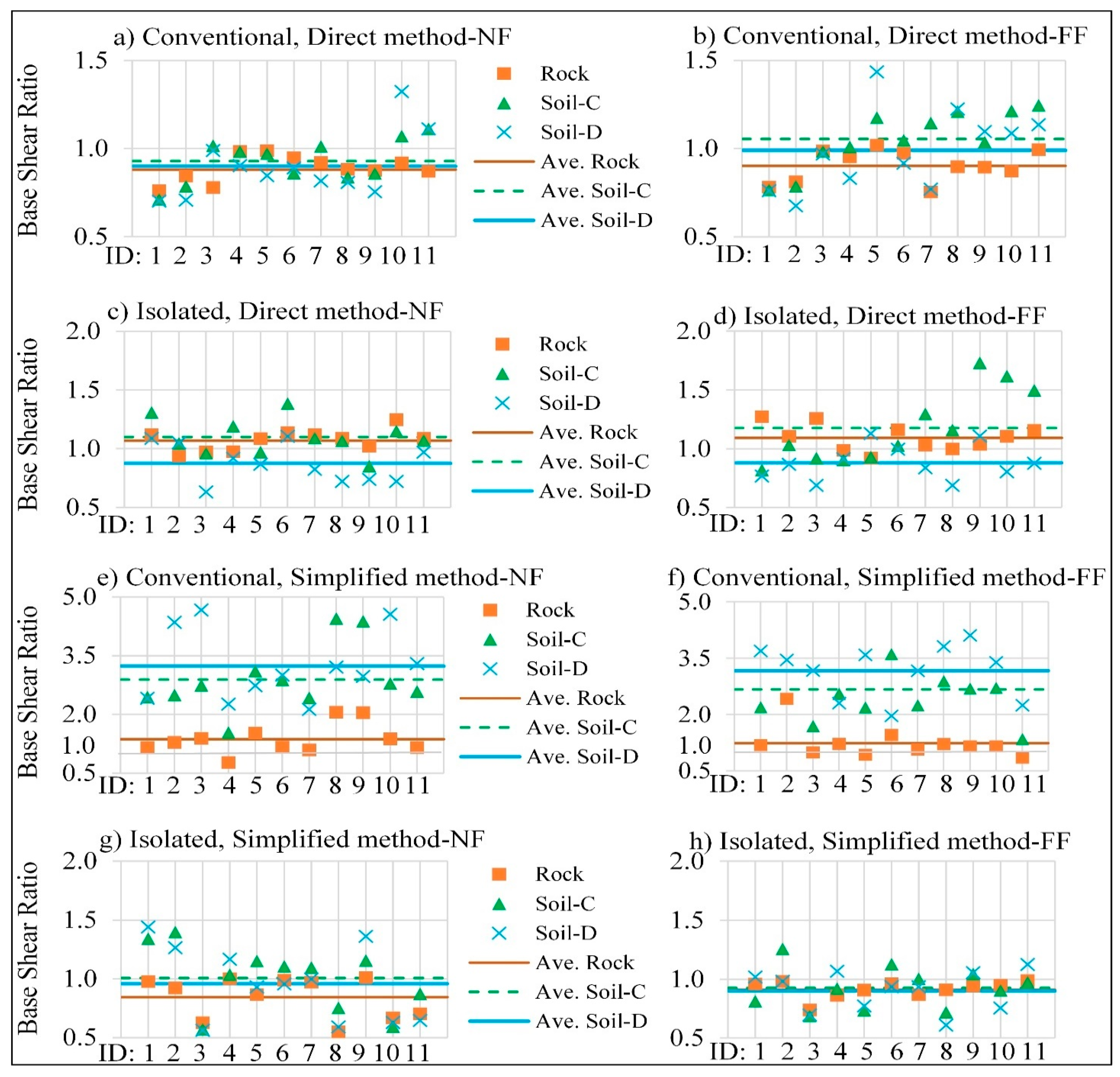
4.3. Effect of Earthquake Characteristics and SSI on the Base Shear Responses
5. Conclusions
- The fault distance does not play a decisive role in the dynamic responses of the bridge, and dynamic responses significantly depend on the low- or high-frequency contents of the records, regardless of the soil type, so the lower ratios of PGA/PGV cause the higher dynamic responses, and they diminish with the increasing of this ratio.
- With the same PGA, NF records which generally contain higher values of PGV cause higher dynamic responses compared to FF records for both conventional and isolated bridges on different soils.
- A considerable increase in the base shear response is observed in softer soils in a few individual records. For example, in the conventional bridge, there is a 43% increase in the maximum base share response on Soil-D for FF:5 compared to the fixed-base bridge (increasing by 0.015Wd, from 0.034Wd to 0.049Wd), while there is a 70% increase from the fixed-base isolated bridge to the isolated bridge in Soil-C (increasing by 0.012Wd, from 0.016Wd to 0.028Wd) in the case of FF:9. In fact, the base shear response is dominated by the uncertainty in the ground motion. Therefore, careful attention is needed at the design stage to anticipate the base shear demand depending on the frequency content, bridge condition and the underlying soil properties.
- In the isolated bridge and direct method, the maximum isolator displacements happen in the records with the lowest ratio of PGA/PGV. However, they increase drastically from Rock to Soil-C so that the displacement demand goes beyond the designed displacement. Therefore, ignoring the effect of the flexibility of soil and the SSI effects will result in underestimating the displacement demand of the isolated bridge and the possibility of destruction in the isolation system in earthquake-prone areas.
- The isolator displacement demand at the abutments is higher than the piers by up to three times in some individual records. Therefore, it should be carefully designed considering the soil effects and the characteristics of the records.
- As F(T) in the direct method is higher than the reduction factor proposed by CSA (S6-19) by the factor of 2.5, the site effect could lead to an underestimation of responses for rocks while it is conservative for soft soils.
- Using the simplified method in this study should be carried out alongside careful attention to the validity of using the equivalent linear method instead of the nonlinear method, because all the records with Soil-C and Soil-D were not eligible based on the limitation of the shear strain index (generally under 0.03%), and the responses were very scattered, especially in the conventional method. Therefore, the simplified method of using springs to represent the soil stratum is rather a simple approach to capture all the major mechanisms involved in soil, SSI, and characteristics of each earthquake ground motion.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tongaonkar, N.; Jangid, R. Seismic response of isolated bridges with soil–structure interaction. Soil Dyn. Earthq. Eng. 2003, 23, 287–302. [Google Scholar] [CrossRef]
- De Domenico, D.; Gandelli, E.; Quaglini, V. Adaptive isolation system combining low-friction sliding pendulum bearings and SMA-based gap dampers. Eng. Struct. 2020, 212, 110536. [Google Scholar] [CrossRef]
- Di Cesare, A.; Ponzo, F.C.; Telesca, A. Improving the earthquake resilience of isolated buildings with double concave curved surface sliders. Eng. Struct. 2020, 228, 111498. [Google Scholar] [CrossRef]
- Cardone, D.; Viggiani, L.; Perrone, G.; Telesca, A.; Di Cesare, A.; Ponzo, F.; Ragni, L.; Micozzi, F.; Dall’asta, A.; Furinghetti, M.; et al. Modelling and Seismic Response Analysis of Existing Italian Residential RC Buildings Retrofitted by Seismic Isolation. J. Earthq. Eng. 2022, 27, 1069–1093. [Google Scholar] [CrossRef]
- De Luca, A.; Guidi, L.G. State of art in the worldwide evolution of base isolation design. Soil Dyn. Earthq. Eng. 2019, 125, 105722. [Google Scholar] [CrossRef]
- Tsopelas, P.; Constantinou, M.; Okamoto, S.; Fujii, S.; Ozaki, D. Experimental study of bridge seismic sliding isolation systems. Eng. Struct. 1996, 18, 301–310. [Google Scholar] [CrossRef]
- Guizani, L.; Chaallal, O. Mise en conformité sismique des ponts par isolation de la base—Application au pont Madrid au Québec. Can. J. Civ. Eng. 2011, 38, 1–10. [Google Scholar] [CrossRef]
- Buckle, I.; Constantinou, M.; Dicleli, M.; Ghasemi, H. Seismic Isolation of Highway Bridges; MCEER-06-SP07; University at Buffalo: Buffalo, NY, USA; The State University of New York: New York, NY, USA, 2006; p. 190. [Google Scholar]
- Nassar, M.; Guizani, L.; Nollet, M.-J.; Tahan, A. Effects of temperature, analysis and modelling uncertainties on the reliability of base-isolated bridges in Eastern Canada. Structure 2022, 37, 295–304. [Google Scholar] [CrossRef]
- CSA. Canadian Highway Bridge Design Code(CHBDC), S6-19; Canadian Standards Association: Toronto, CA, Canada, 2019. [Google Scholar]
- De Domenico, D.; Losanno, D.; Vaiana, N. Experimental tests and numerical modeling of full-scale unbonded fiber reinforced elastomeric isolators (UFREIs) under bidirectional excitation. Eng. Struct. 2023, 274, 115118. [Google Scholar] [CrossRef]
- Nguyen, X.D.; Guizani, L. Analytical and numerical investigation of natural rubber bearings incorporating U-shaped dampers behaviour for seismic isolation. Eng. Struct. 2021, 243, 112647. [Google Scholar] [CrossRef]
- Ye, K.; Ji, J.; Brown, T. Design of a quasi-zero stiffness isolation system for supporting different loads. J. Sound Vib. 2020, 471, 115198. [Google Scholar] [CrossRef]
- Mordini, A.; Strauss, A. An innovative earthquake isolation system using fibre reinforced rubber bearings. Eng. Struct. 2008, 30, 2739–2751. [Google Scholar] [CrossRef]
- Nguyen, X.-D.; Guizani, L. Optimal seismic isolation characteristics for bridges in moderate and high seismicity areas. Can. J. Civ. Eng. 2021, 48, 642–655. [Google Scholar] [CrossRef]
- Ucak, A.; Tsopelas, P. Effect of Soil–Structure Interaction on Seismic Isolated Bridges. J. Struct. Eng. 2008, 134, 1154–1164. [Google Scholar] [CrossRef]
- Roussis, P.C.; Constantinou, M.C.; Erdik, M.; Durukal, E.; Dicleli, M. Assessment of Performance of Seismic Isolation System of Bolu Viaduct. J. Bridg. Eng. 2003, 8, 182–190. [Google Scholar] [CrossRef]
- Billah, A.H.M.M.; Alam, M.S.; Bhuiyan, M.A.R. Fragility Analysis of Retrofitted Multicolumn Bridge Bent Subjected to Near-Fault and Far-Field Ground Motion. J. Bridg. Eng. 2013, 18, 992–1004. [Google Scholar] [CrossRef]
- Bray, J.D.; Rodriguez-Marek, A. Characterization of forward-directivity ground motions in the near-fault region. Soil Dyn. Earthq. Eng. 2004, 24, 815–828. [Google Scholar] [CrossRef]
- Jia, H.; Liu, Z.; Xu, L.; Bai, H.; Bi, K.; Zhang, C.; Zheng, S. Dynamic response analyses of long-span cable-stayed bridges subjected to pulse-type ground motions. Soil Dyn. Earthq. Eng. 2023, 164, 107591. [Google Scholar] [CrossRef]
- Jiao, C.; Liu, W.; Wu, S.; Gui, X.; Huang, J.; Long, P.; Li, W. Shake table experimental study of curved bridges with consideration of girder-to-girder collision. Eng. Struct. 2021, 237, 112216. [Google Scholar] [CrossRef]
- Jiang, L.; Zhong, J.; Yuan, W. The pulse effect on the isolation device optimization of simply supported bridges in near-fault regions. Structures 2020, 27, 853–867. [Google Scholar] [CrossRef]
- Mangalathu, S.; Jeon, J.-S.; Jiang, J. Skew Adjustment Factors for Fragilities of California Box-Girder Bridges Subjected to near-Fault and Far-Field Ground Motions. J. Bridg. Eng. 2019, 24, 04018109. [Google Scholar] [CrossRef]
- Neethu, B.; Das, D. Effect of dynamic soil–structure interaction on the seismic response of bridges with elastomeric bearings. Asian J. Civ. Eng. 2018, 20, 197–207. [Google Scholar] [CrossRef]
- Ismail, M.; Rodellar, J.; Pozo, F. An isolation device for near-fault ground motions. Struct. Control Health Monit. 2014, 21, 249–268. [Google Scholar] [CrossRef]
- Chouw, N.; Hao, H. Study of SSI and non-uniform ground motion effect on pounding between bridge girders. Soil Dyn. Earthq. Eng. 2005, 25, 717–728. [Google Scholar] [CrossRef]
- Shen, J.; Tsai, M.-H.; Chang, K.-C.; Lee, G.C. Performance of a Seismically Isolated Bridge under Near-Fault Earthquake Ground Motions. J. Struct. Eng. 2004, 130, 861–868. [Google Scholar] [CrossRef]
- Liao, W.; Loh, C.; Wan, S.; Jean, W.; Chai, J. Dynamic responses of bridges subjected to near-fault ground motions. J. Chin. Inst. Eng. 2000, 23, 455–464. [Google Scholar] [CrossRef]
- Yang, T.; Yuan, X.; Zhong, J.; Yuan, W. Near-fault pulse seismic ductility spectra for bridge columns based on machine learning. Soil Dyn. Earthq. Eng. 2023, 164, 107582. [Google Scholar] [CrossRef]
- Zhong, J.; Yang, T.; Wang, W. Quantifying the impact of normalized period on seismic demand model of ductile columns under pulse-like ground motions. Bull. Earthq. Eng. 2022, 20, 6789–6812. [Google Scholar] [CrossRef]
- Yang, D.; Guo, G.; Liu, Y.; Zhang, J. Dimensional response analysis of bilinear SDOF systems under near-fault ground motions with intrinsic length scale. Soil Dyn. Earthq. Eng. 2019, 116, 397–408. [Google Scholar] [CrossRef]
- Tochaei, E.N.; Taylor, T.; Ansari, F. Effects of near-field ground motions and soil-structure interaction on dynamic response of a cable-stayed bridge. Soil Dyn. Earthq. Eng. 2020, 133, 106115. [Google Scholar] [CrossRef]
- Dezi, F.; Carbonari, S.; Tombari, A.; Leoni, G. Soil-structure interaction in the seismic response of an isolated three span motorway overcrossing founded on piles. Soil Dyn. Earthq. Eng. 2012, 41, 151–163. [Google Scholar] [CrossRef]
- Fatahi, B.; Tabatabaiefar, S.H.R.; Samali, B. Soil-structure interaction vs Site effect for seismic design of tall buildings on soft soil. Géoméch. Eng. 2014, 6, 293–320. [Google Scholar] [CrossRef]
- Saritaş, F.; Hasgür, Z. Dynamic behavior of an isolated bridge pier under earthquake effects for different soil layers and support conditions. Tek. Dergi 2014, 25, 1733–1756. [Google Scholar]
- Worku, A. Soil-structure-interaction provisions: A potential tool to consider for economical seismic design of buildings? J. South Afr. Inst. Civ. Eng. 2014, 56, 54–62. [Google Scholar]
- Stehmeyer, E.H.; Rizos, D.C. Considering dynamic soil structure interaction (SSI) effects on seismic isolation retrofit efficiency and the importance of natural frequency ratio. Soil Dyn. Earthq. Eng. 2008, 28, 468–479. [Google Scholar] [CrossRef]
- Kulkarni, J.A.; Jangid, R. Effects of Superstructure Flexibility on the Response of Base-Isolated Structures. Shock. Vib. 2003, 10, 1–13. [Google Scholar] [CrossRef]
- Chaudhary, M.; Abé, M.; Fujino, Y. Identification of soil–structure interaction effect in base-isolated bridges from earthquake records. Soil Dyn. Earthq. Eng. 2001, 21, 713–725. [Google Scholar] [CrossRef]
- Alam, A.; Bhuiyan, M. Effect of soil–structure interaction on seismic response of a seismically isolated highway bridge pier. J. Civ. Eng. 2013, 41, 179–199. [Google Scholar]
- Dicleli, M.; Buddaram, S. Effect of isolator and ground motion characteristics on the performance of seismic-isolated bridges. Earthq. Eng. Struct. Dyn. 2005, 35, 233–250. [Google Scholar] [CrossRef]
- Ates, S.; Constantinou, M.C. Example of application of response spectrum analysis for seismically isolated curved bridges including soil-foundation effects. Soil Dyn. Earthq. Eng. 2011, 31, 648–661. [Google Scholar] [CrossRef]
- Bi, K.; Hao, H.; Chouw, N. Influence of ground motion spatial variation, site condition and SSI on the required separation distances of bridge structures to avoid seismic pounding. Earthq. Eng. Struct. Dyn. 2010, 40, 1027–1043. [Google Scholar] [CrossRef]
- Hoseini, S.S.; Ghanbari, A.; Davoodi, M. A new approach to soil-pile-structure modeling of long-span bridges subjected to spatially varying earthquake ground motion. Bridg. Struct. 2019, 14, 63–79. [Google Scholar] [CrossRef]
- Vlassis, A.; Spyrakos, C. Seismically isolated bridge piers on shallow soil stratum with soil–structure interaction. Comput. Struct. 2001, 79, 2847–2861. [Google Scholar] [CrossRef]
- Soneji, B.; Jangid, R. Influence of soil–structure interaction on the response of seismically isolated cable-stayed bridge. Soil Dyn. Earthq. Eng. 2008, 28, 245–257. [Google Scholar] [CrossRef]
- Carbonari, S.; Dezi, F.; Leoni, G. Seismic soil-structure interaction in multi-span bridges: Application to a railway bridge. Earthq. Eng. Struct. Dyn. 2011, 40, 1219–1239. [Google Scholar] [CrossRef]
- Jeremić, B.; Kunnath, S.; Xiong, F. Influence of soil–foundation–structure interaction on seismic response of the I-880 viaduct. Eng. Struct. 2004, 26, 391–402. [Google Scholar] [CrossRef]
- Mylonakis, G.; Gazetas, G. Seismic soil-structure interaction: Beneficial or detrimental? J. Earthq. Eng. 2000, 4, 277–301. [Google Scholar] [CrossRef]
- Betti, R.; Abdel-Ghaffar, A.; Niazy, A. Kinematic soil–structure interaction for long-span cable-supported bridges. Earthq. Eng. Struct. Dyn. 1993, 22, 415–430. [Google Scholar] [CrossRef]
- Forcellini, D. Cost assessment of isolation technique applied to a benchmark bridge with soil structure interaction. Bull. Earthq. Eng. 2017, 15, 51–69. [Google Scholar] [CrossRef]
- Güllü, H.; Jaf, H.S. Full 3D nonlinear time history analysis of dynamic soil–structure interaction for a historical masonry arch bridge. Environ. Earth Sci. 2016, 75, 1421. [Google Scholar] [CrossRef]
- Canadian Standards Association. Commentary on CSA S6-19, Canadian, Highway Bridge Design Code; CSA Group: Toronto, CA, Canada, 2019. [Google Scholar]
- Elias, S.; Matsagar, V. Effectiveness of Tuned Mass Dampers in Seismic Response Control of Isolated Bridges Including Soil-Structure Interaction. Lat. Am. J. Solids Struct. 2017, 14, 2324–2341. [Google Scholar] [CrossRef]
- ABAQUS. Analysis User’s Manual, Version 6.14; Dassault Systemes Simulia, Inc.: Johnston, RI, USA, 2014. [Google Scholar]
- Earthquakes Canada. Earthquakes Canada, GSC, Earthquake Search (On-line Bulletin). 2022. Available online: http://earthquakescanada.nrcan.gc.ca/stndon/NEDB-BNDS/bulletin-en.php (accessed on 25 August 2022).
- Labuz, J.F.; Zang, A. Mohr–Coulomb failure criterion. Rock Mech. Rock Eng. 2012, 45, 975–979. [Google Scholar] [CrossRef]
- Tabatabaiefar, H.R.; Fatahi, B. Idealisation of soil–structure system to determine inelastic seismic response of mid-rise building frames. Soil Dyn. Earthq. Eng. 2014, 66, 339–351. [Google Scholar] [CrossRef]
- Rayhani, M.H.; El Naggar, M.H. Numerical Modeling of Seismic Response of Rigid Foundation on Soft Soil. Int. J. Géoméch. 2008, 8, 336–346. [Google Scholar] [CrossRef]
- Liu, G.R.; Quek Jerry, S.S. A non-reflecting boundary for analyzing wave propagation using the finite element method. Finite Elem. Anal. Des. 2003, 39, 403–417. [Google Scholar] [CrossRef]
- Tabatabaiefar, S.H.R.; Fatahi, B.; Samali, B. Seismic Behavior of Building Frames Considering Dynamic Soil-Structure Interaction. Int. J. Géoméch. 2013, 13, 409–420. [Google Scholar] [CrossRef]
- Dehghanpoor, A.; Thambiratnam, D.; Taciroglu, E.; Chan, T. Soil-pile-superstructure interaction effects in seismically isolated bridges under combined vertical and horizontal strong ground motions. Soil Dyn. Earthq. Eng. 2019, 126, 105753. [Google Scholar] [CrossRef]
- Khazaei, J.; Amiri, A.; Khalilpour, M. Seismic evaluation of soil-foundation-structure interaction: Direct and Cone model. Earthq. Struct. 2017, 12, 251–262. [Google Scholar] [CrossRef]
- Pando, M.A.; Ealy, C.D.; Filz, G.M.; Lesko, J.J.; Hoppe, E.J. A Laboratory and Field Study of Composite Piles for Bridge Substructures; Federal Highway Administration, Office of Infrastructure: Washington, DC, USA, 2006. [Google Scholar]
- Kimmerling, R. Geotechnical Engineering Circular No. 6 Shallow Foundations; Federal Highway Administration, Office of Bridge Technology: Washington, DC, USA, 2002. [Google Scholar]
- Manual, N.D.; Mechanics, S. Foundations and Earth Structures. NAVFAC DM-7; Department of the Navy, Naval Facilities Engineering Command: Washington, DC, USA, 1986; p. 982. [Google Scholar]
- Jesmani, M.; Fallahi, A.M.; Kashani, H.F. Effects of Geometrical Properties of Rectangular Trenches Intended for Passive Isolation in Sandy Soils. Earth Sci. Res. 2012, 1, 137. [Google Scholar] [CrossRef]
- Hashash, Y.M.A.; Musgrove, M.I.; Harmon, J.A.; Groholski, D.R.; Phillips, C.A.; Park, D. DEEPSOIL 6.1, User Manual; Board of Trustees of University of Illinois at Urbana-Champaign: Urbana, IL, USA, 2016. [Google Scholar]
- Kim, B.; Hashash, Y.M.A.; Stewart, J.P.; Rathje, E.M.; Harmon, J.A.; Musgrove, M.I.; Campbell, K.W.; Silva, W.J. Relative Differences between Nonlinear and Equivalent-Linear 1-D Site Response Analyses. Earthq. Spectra 2016, 32, 1845–1865. [Google Scholar] [CrossRef]
- United States Geological Survey “USGS”. Available online: https://www.usgs.gov/ (accessed on 25 August 2022).
- National Research Council of Canada. Fire, National Building Code of Canada: 2020; National Research Council of Canada: Montreal, QC, Canada, 2022. [Google Scholar]
- Makris, N.; Zhang, J. Seismic Response Analysis of a Highway Overcrossing Equipped with Elastomeric Bearings and Fluid Dampers. J. Struct. Eng. 2004, 130, 830–845. [Google Scholar] [CrossRef]




| Properties of the Bridge | Deck | Piers |
|---|---|---|
| Cross-sectional areas (m2) | 15.6 | 1.767 |
| Length or height (m) | 3 × 30 | 10 |
| Young’s modulus of elasticity (Gpa) | 36 | 36 |
| Mass density (kg/m3) | 2400 | 2400 |
| Compressive Strength (Mpa) | 30 | 30 |
| Poisson Ratio | 0.2 | 0.2 |
| Jangid 2003, Elias 2017 | Present Study | Difference % | |
|---|---|---|---|
| Period (s) | 0.53 | 0.54 | 1.85 |
| Base Shear/Wdeck | 1.439 | 1.388 | −3.54 |
| Deck acceleration (g) | 1.396 | 1.461 | 4.45 |
| Isolation System | T | Keff | Ku | Kd | Qd | Dmax |
|---|---|---|---|---|---|---|
| (s) | (KN/m) | (KN/m) | (KN/m) | (KN) | (mm) | |
| Piers | 2.5 | 7880 | 83,500 | 5600 | 140 | 60 |
| Abutments | 2.5 | 4000 | 30,000 | 2800 | 72 | 60 |
| Soil | E (MPa) | Ρ (kg/m3) | υ | C (KPa) | Ø (°) | Vs (m/s) | ψ | ξ (%) |
|---|---|---|---|---|---|---|---|---|
| Rock | 24,960 | 2600 | 0.2 | 25,000 | 48 | 2000 | 7 | 5 |
| Soil-C | 1323 | 2100 | 0.26 | 0 | 40 | 500 | 5 | 5 |
| Soil-D | 430 | 1900 | 0.32 | 0 | 35 | 300 | 4 | 5 |
| Direction | Stiffness. |
|---|---|
| Translation along x-axis | |
| Translation along y-axis | |
| Translation along z-axis | |
| Rocking about x-axis | |
| Rocking about y-axis | |
| Rocking about z-axis |
| Rock | Soil-C | Soil-D | |
|---|---|---|---|
| Foundation size (L × B × d) | 4.0 × 4.0 × 1 | 4.0 × 4.0 × 1 | 4.0 × 4.0 × 1 |
| Kx (GN/m) | 414.65 | 21.65 | 6.96 |
| Ky (GN/m) | 414.65 | 21.65 | 6.96 |
| Kz (GN/m) | 322.62 | 17.61 | 5.94 |
| Kxx (MN-m/rad) | 1644.43 | 89.74 | 30.3 |
| Kyy (GN-m/rad) | 1798.42 | 98.15 | 33.14 |
| Kzz (MN-m/rad) | 3451.86 | 174.25 | 54.06 |
| ID | Earthquake, Station | Component | Mw | Rrup (km) | PGA/PGV (1/s) | Scale Factor | Predominant Period (s) |
|---|---|---|---|---|---|---|---|
| NF:1 | Parkfield, Turkey Flat | 36529270 | 6 | 5.3 | 19 | 1.8 | 0.26 |
| 2 | 30,226,086, Warm Springs Dam | N2122090 | 4 | 8.8 | 28 | 14.8 | 0.28 |
| 3 | Hollister, Gilroy Array #1 | A-G01247 | 5.1 | 10.5 | 34 | 3.1 | 0.1 |
| 4 | Coyote Lake, Gilroy Array #1 | G01320 | 5.7 | 10.7 | 11 | 3.8 | 0.08 |
| 5 | San Francisco, Golden Gate | GGP100 | 5.3 | 11 | 23 | 4.7 | 0.22 |
| 6 | 21,530,368, Carmenet Vineyards | BKCVSHHE | 4.5 | 12.1 | 26 | 12.0 | 0.18 |
| 7 | Umbria, Gubbio | GBB090 | 5.6 | 15.7 | 19 | 6.6 | 0.24 |
| 8 | Northridge, Wonderland Ave | WON095 | 5.3 | 17.1 | 21 | 7.9 | 0.48 |
| 9 | Whittier Narrows, CIT Kresge | A-KRE090 | 6 | 18.1 | 11 | 4.0 | 0.36 |
| 10 | Lytle Creek, Allen Ranch | CSM095 | 5.3 | 19.4 | 32 | 10.8 | 0.14 |
| 11 | 14,151,344, Pinon Flats | AZPFOHLE | 5.2 | 19.6 | 32 | 1.9 | 0.12 |
| FF:1 | 14,095,628, Cattani Ranch | CITEHHLE | 5 | 20.6 | 31 | 17.8 | 0.26 |
| 2 | Northridge, Griffith Park | GPO000 | 5.3 | 21.7 | 13 | 13.8 | 0.14 |
| 3 | Whittier Narrows, Wonderland Av. | A-WON075 | 6 | 27.6 | 27 | 10.6 | 0.1 |
| 4 | 40,204,628, Mount Umunhum | NCJUMHNN | 5.5 | 30.8 | 22 | 18.5 | 0.08 |
| 5 | Anza, Keenwild Fire Station | 0604A180 | 4.9 | 32.1 | 56 | 15.3 | 0.22 |
| 6 | 21,530,368, Hamilton Field | NHFHNE | 4.5 | 35.1 | 19 | 15.9 | 0.18 |
| 7 | RiviereDuLoup, Riviere-Ouelle | CN.A16.HHE | 4.7 | 39 | 44 | 15.9 | 0.24 |
| 8 | Sierra Madre, Vasquez Rocks | VAS090 | 5.6 | 39.8 | 38 | 4.2 | 0.48 |
| 9 | Molise, Sannicandro | B-SCO000 | 5.7 | 51.3 | 40 | 12.0 | 0.36 |
| 10 | ValDesBois, Innes Road_ ON | CN.ORIO.HHE | 5.1 | 52.9 | 45 | 10.1 | 0.14 |
| 11 | Saguenay, US.DCKY | US.DCKY.HHE | 5.9 | 192.1 | 29 | 4.8 | 0.12 |
| NF Average | ….. | 5.3 | 13.5 | 23.2 | ….. | ….. | |
| FF Average | ….. | 5.3 | 49.4 | 33.2 | ….. | ….. |
| NF | FF | |||||
|---|---|---|---|---|---|---|
| Rock | Soil-C | Soil-D | Rock | Soil-C | Soil-D | |
| Conventional Bridge: | ||||||
| Acceleration (g) | ||||||
| Direct method | 0.28 | 0.28 | 0.26 | 0.21 | 0.23 | 0.21 |
| Simplified method | 0.43 | 0.92 | 0.92 | 0.33 | 0.74 | 0.7 |
| Difference (%) | +54 | +229 | +254 | +57 | +222 | +233 |
| Base Shear (Wd) | ||||||
| Direct method | 0.274 | 0.277 | 0.26 | 0.211 | 0.229 | 0.209 |
| Simplified method | 0.437 | 0.932 | 0.934 | 0.333 | 0.708 | 0.711 |
| Difference (%) | +60 | +236 | +260 | +58 | +209 | +240 |
| Deck drift (mm) | ||||||
| Direct method | 23 | 25.4 | 25.6 | 16.5 | 19.9 | 20 |
| Simplified method | 32 | 69.2 | 67.8 | 24.5 | 62.1 | 51.7 |
| Difference (%) | +39 | +172 | +166 | +49 | +211 | +158 |
| Isolated Bridge: | ||||||
| Acceleration (g) | ||||||
| Direct method | 0.029 | 0.027 | 0.025 | 0.022 | 0.022 | 0.02 |
| Simplified method | 0.028 | 0.035 | 0.039 | 0.021 | 0.027 | 0.028 |
| Difference (%) | −1 | +29 | +54 | −3 | +23 | +41 |
| Base Shear (Wd) | ||||||
| Direct method | 0.028 | 0.029 | 0.022 | 0.023 | 0.023 | 0.017 |
| Simplified method | 0.022 | 0.026 | 0.025 | 0.019 | 0.019 | 0.018 |
| Difference (%) | −21 | −9 | +12 | −18 | −20 | +5 |
| Isolation displacement, piers (mm) | ||||||
| Direct method | 34.2 | 43.6 | 48.3 | 20.5 | 33.6 | 37.8 |
| Simplified method | 33.4 | 47.6 | 56.6 | 19.9 | 32.5 | 33.4 |
| Difference (%) | −2 | +9 | +17 | −3 | −3 | −11 |
| Isolation displacement, abutments (mm) | ||||||
| Direct method | 38.2 | 102.5 | 109.9 | 23.3 | 96.4 | 96.1 |
| Simplified method | 34.4 | 49.1 | 58.6 | 20.3 | 31.2 | 34.6 |
| Difference (%) | −10 | −52 | −47 | −13 | −68 | −64 |
| Rock | Soil-C | Soil-D | ||||
|---|---|---|---|---|---|---|
| NF | FF | NF | FF | NF | FF | |
| Conventional Bridge: | ||||||
| CSA (S6-19) | 0.48 | 0.48 | 1 | 1 | 1.18 | 1.18 |
| Direct method | 1.01 | 0.9 | 1 | 1 | 0.93 | 0.95 |
| Simplified method | 0.46 | 0.49 | 1 | 1 | 1 | 1.33 |
| Isolated Bridge: | ||||||
| CSA (S6-19) | 0.4 | 0.4 | 1 | 1 | 1.35 | 1.35 |
| Direct method | 1.02 | 1.01 | 1 | 1 | 0.93 | 0.93 |
| Simplified method | 0.82 | 0.86 | 1 | 1 | 1.1 | 1.09 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheshmehkaboodi, N.; Guizani, L.; Ghlamallah, N. Soil–Structure Interaction Effects on Seismic Responses of a Conventional and Isolated Bridge Subjected to Moderate Near-Fault and Far-Field Records. CivilEng 2023, 4, 702-725. https://doi.org/10.3390/civileng4030040
Cheshmehkaboodi N, Guizani L, Ghlamallah N. Soil–Structure Interaction Effects on Seismic Responses of a Conventional and Isolated Bridge Subjected to Moderate Near-Fault and Far-Field Records. CivilEng. 2023; 4(3):702-725. https://doi.org/10.3390/civileng4030040
Chicago/Turabian StyleCheshmehkaboodi, Nastaran, Lotfi Guizani, and Noureddine Ghlamallah. 2023. "Soil–Structure Interaction Effects on Seismic Responses of a Conventional and Isolated Bridge Subjected to Moderate Near-Fault and Far-Field Records" CivilEng 4, no. 3: 702-725. https://doi.org/10.3390/civileng4030040
APA StyleCheshmehkaboodi, N., Guizani, L., & Ghlamallah, N. (2023). Soil–Structure Interaction Effects on Seismic Responses of a Conventional and Isolated Bridge Subjected to Moderate Near-Fault and Far-Field Records. CivilEng, 4(3), 702-725. https://doi.org/10.3390/civileng4030040









