Numerical and Code-Based Investigation on the Impact of Corrosion on the Ultimate Compressive Strength of Steel Angle Members Using Thickness Reduction Method
Abstract
1. Introduction
2. Methods
2.1. Code-Based Prediction of Compressive Capacity of Steel Angles
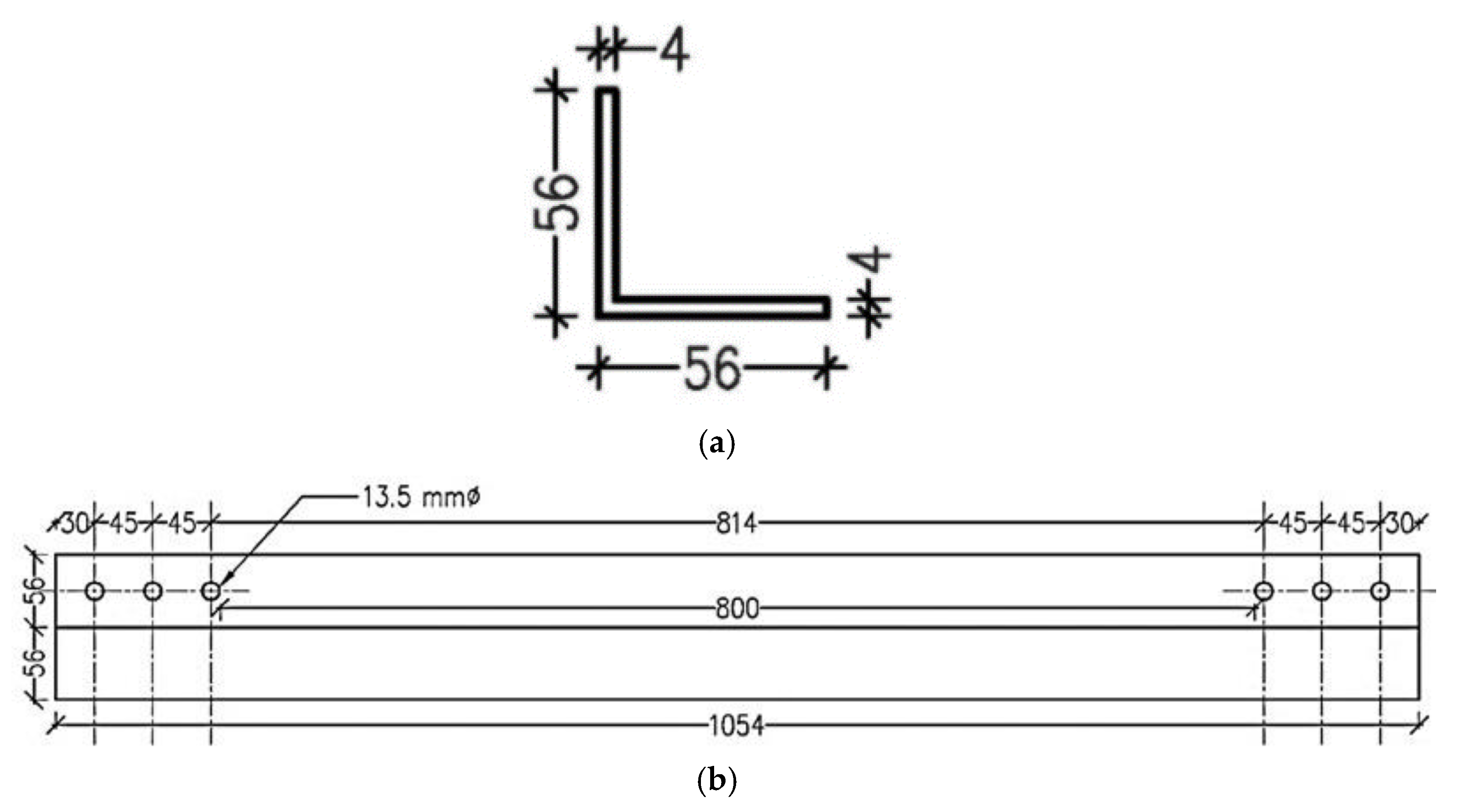
2.2. Numerical Model
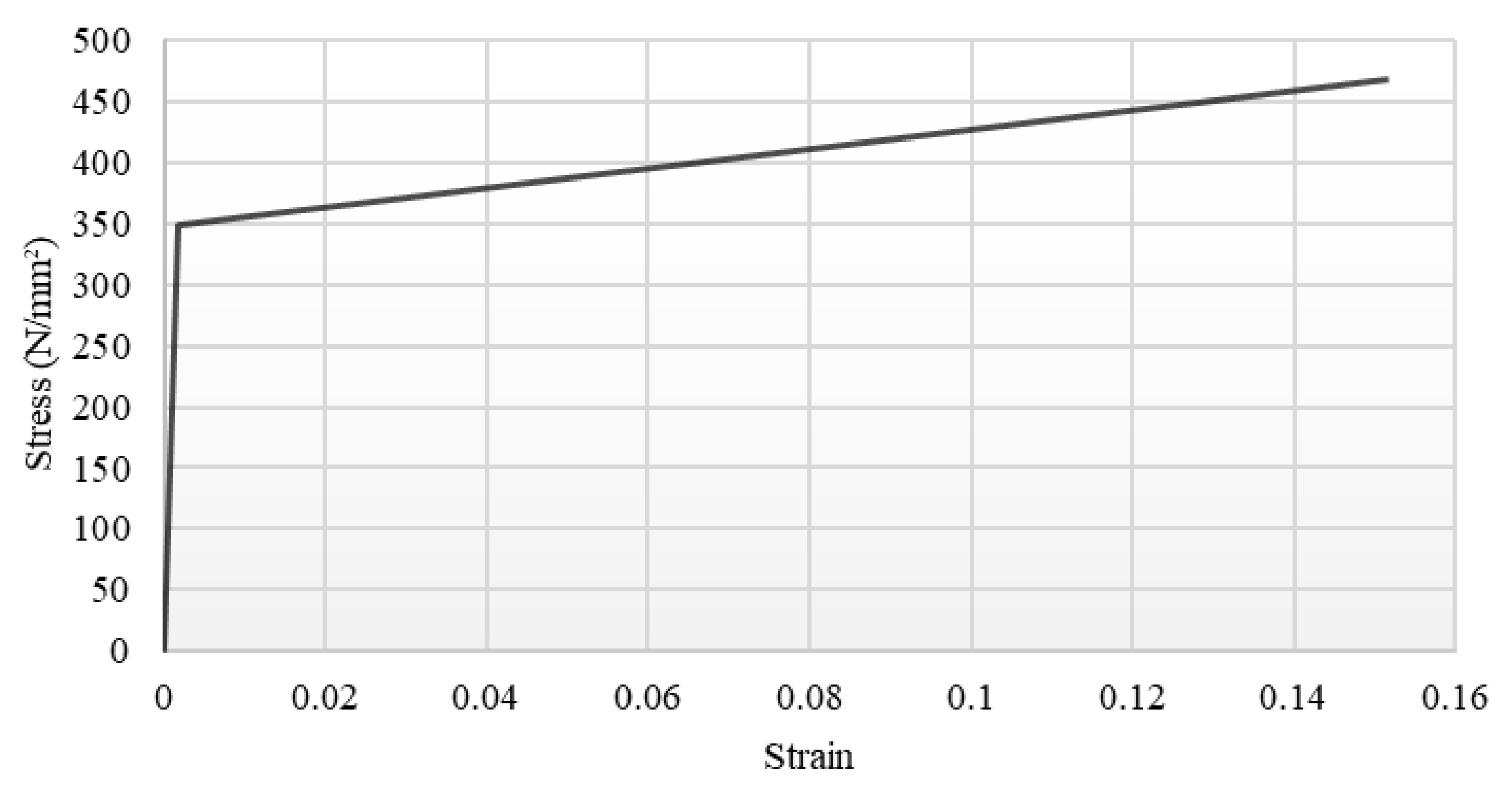
| Property | Value |
|---|---|
| Elastic Modulus | 206 GPa |
| Density | 7850 kg/m3 |
| Yield Strength | 350 MPa |
| Ultimate Strength | 468 MPa |
| Ultimate Strain | 0.15 |
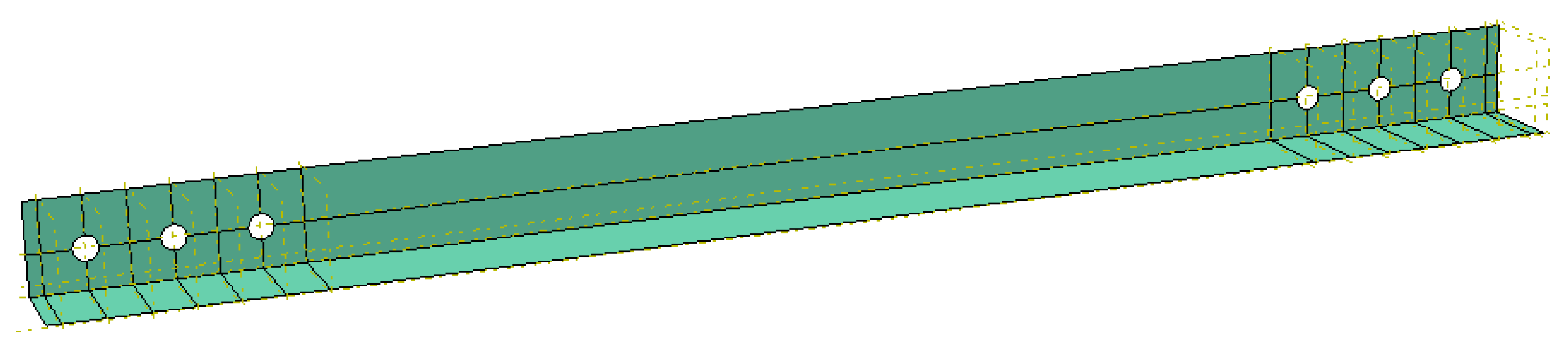
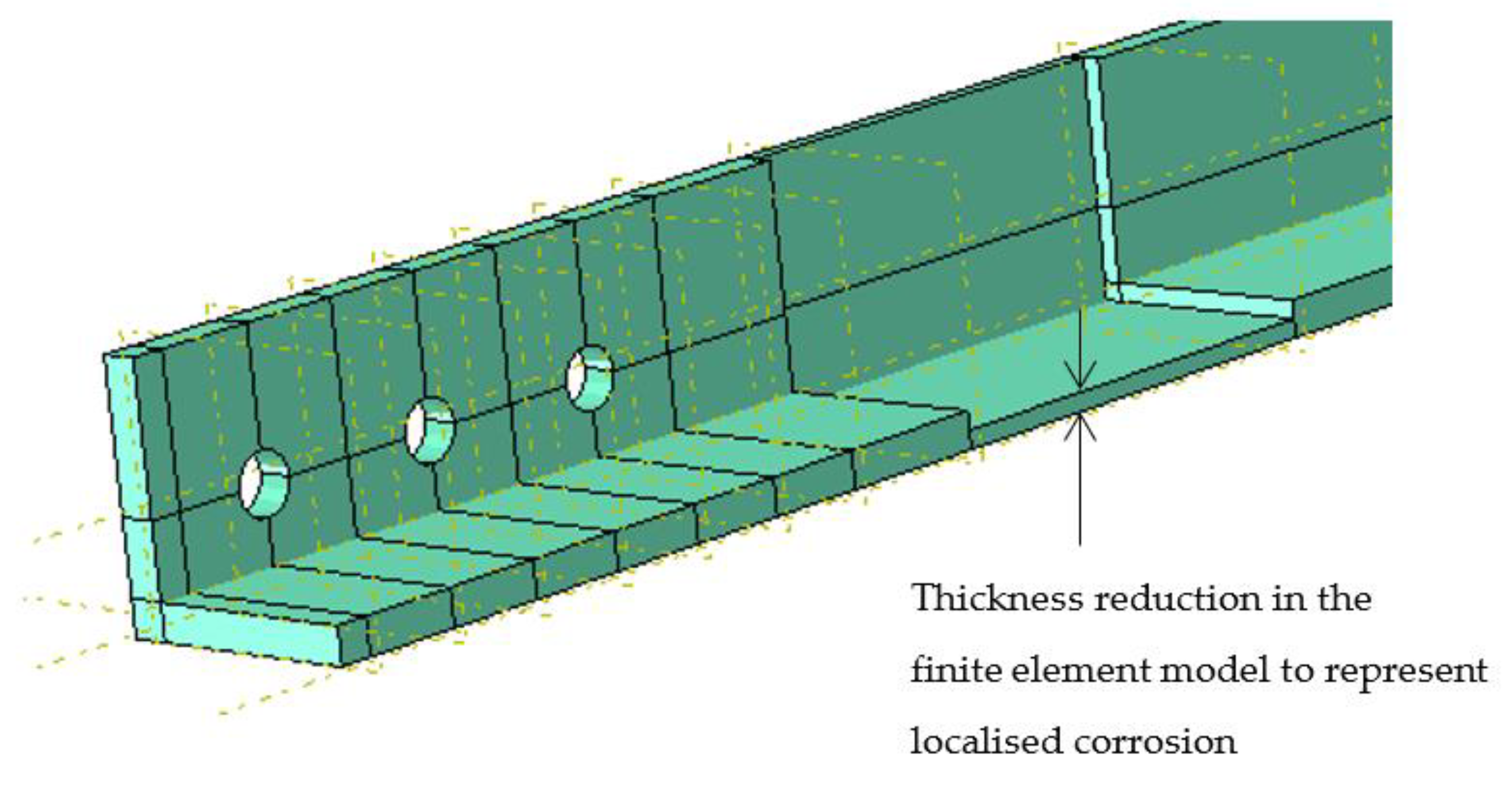
| Group No. | Specimen ID | Longitudinal Illustration | Cross Section | Corrosion Details | Assessment |
|---|---|---|---|---|---|
| 1 | 1A |  |  | Lcorr = 1054 mm tcorr = 1 mm | Identify the most critical leg (bolted or not) and side (outer or inner) of corrosion on the compression capacity |
| 1B |  |  | Lcorr = 1054 mm tcorr = 1 mm | ||
| 1C |  |  | Lcorr = 1054 mm tcorr = 1 mm | ||
| 1D |  |  | Lcorr = 1054 mm tcorr = 1 mm | ||
| 2 | 2A |  |   | Lcorr = 400 mm × 2 tcorr = 1 mm | The impact of rapidity of varying the corroded leg on the compression capacity. Similar patterns are experimentally tested by Ozvald and Dunai [20]. |
| 2B |  | Lcorr = 200 mm × 4 tcorr = 1 mm | |||
| 2C |  | Lcorr = 100 mm × 8 tcorr = 1 mm | |||
| 3 | 3A |  |  | Lcorr = 800 mm tcorr = 1 mm | Impact comparison of corner of the angle corrosion and edge of the width corrosion on the compression capacity. Similar patterns are experimentally tested by Ozvald and Dunai [20]. |
| 3B |  |  | Lcorr = 800 mm tcorr = 1 mm | ||
| 3C |  |  | Lcorr = 800 mm tcorr = 1 mm | ||
| 3D |  |  | Lcorr = 800 mm tcorr = 1 mm | ||
| 4 | 4A |  |  | Lcorr = 300 mm tcorr = 1 mm | Impact comparison of buckling area corrosion and bolted area corrosion on the compression capacity. |
| 4B |  |  | Lcorr = 150 mm × 2 tcorr = 1 mm | ||
| 5 | 5A |  |  | Lcorr = 100 mm tcorr = 3 mm | Impact of the concentration of the corroded volume on the compression capacity. Similar patterns are experimentally tested by Ozvald and Dunai [20]. |
| 5B |  |  | Lcorr = 200 mm tcorr = 1.5 mm | ||
| 5C |  |  | Lcorr = 300 mm tcorr = 1 mm | ||
| 5D |  |  | Lcorr = 600 mm tcorr = 0.5 mm | ||
| 6 | 6A |  |  | Lcorr = 100 mm tcorr = 1 mm | Impact of the local corrosion location on the compression capacity. Similar patterns are experimentally tested by Ozvald and Dunai [20]. |
| 6B |  | Lcorr = 100 mm tcorr = 1 mm | |||
| 6C |  | Lcorr = 100 mm tcorr = 1 mm | |||
| 6D |  | Lcorr = 100 mm tcorr = 1 mm | |||
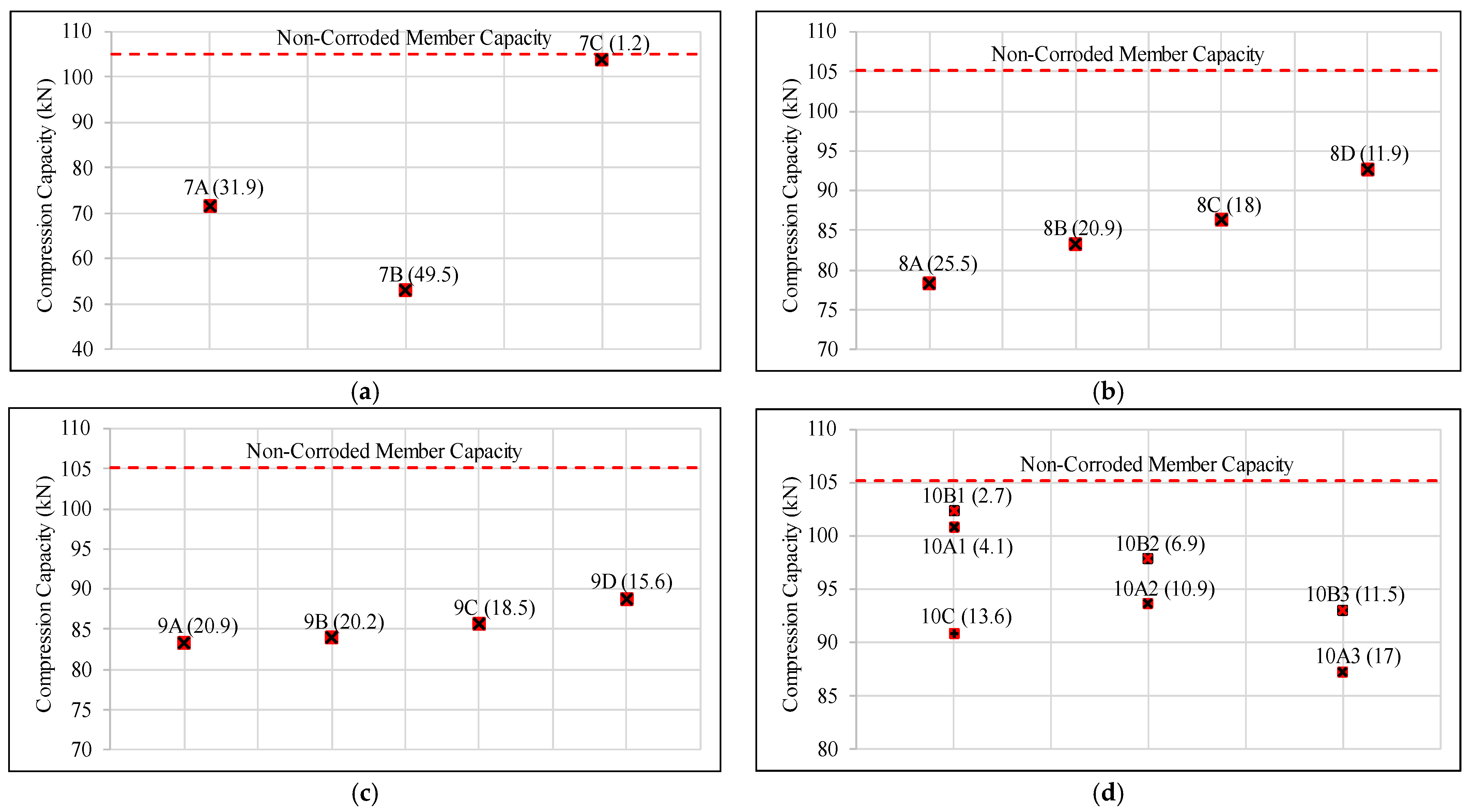
| 7 | 7A |  |  | D = 10 mm nper = 15 | The impact of perforated corrosion on the compression capacity. |
| 7B |  |  | Lcorr = 43 mm tcorr = 2 mm | ||
| 7C |  |  | Lcorr = 800 mm tcorr = 0.1053 mm | ||
| 8 | 8A |  |  | D = 8.66 mm nper = 8 | The impact of perforation pattern on the compression capacity. |
| 8B |  |  | D = 10 mm nper = 6 | ||
| 8C |  |  | D = 12.25 mm nper = 4 | ||
| 8D |  |  | D = 17.32 mm nper = 2 | ||
| 9 | 9A |  |  | D = 8.66 mm nper = 6 | The impact of perforation location on the compression capacity. |
| 9B |  | D = 8.66 mm nper = 6 | |||
| 9C |  | D = 8.66 mm nper = 6 | |||
| 9D |  | D = 8.66 mm nper = 6 | |||
| 10 | 10A1 |  |  | D = 15 mm npit = 66 tcorr = 1 mm | The impact of pitting corrosion compared to uniform corrosion on the compression capacity. |
| 10A2 |  |  | D = 15 mm npit = 66 tcorr = 2 mm | ||
| 10A3 |  |  | D = 15 mm npit = 66 tcorr = 3 mm | ||
| 10B1 |  |  | Lcorr = 768.5 mm tcorr = 0.292 mm | ||
| 10B2 |  |  | Lcorr = 768.5 mm tcorr = 0.58 4mm | ||
| 10B3 |  |  | Lcorr = 768.5 mm tcorr = 0.876 mm | ||
| 10C |  |  | Lcorr = 768.5 mm tcorr = 1 mm |
3. Results and Discussion
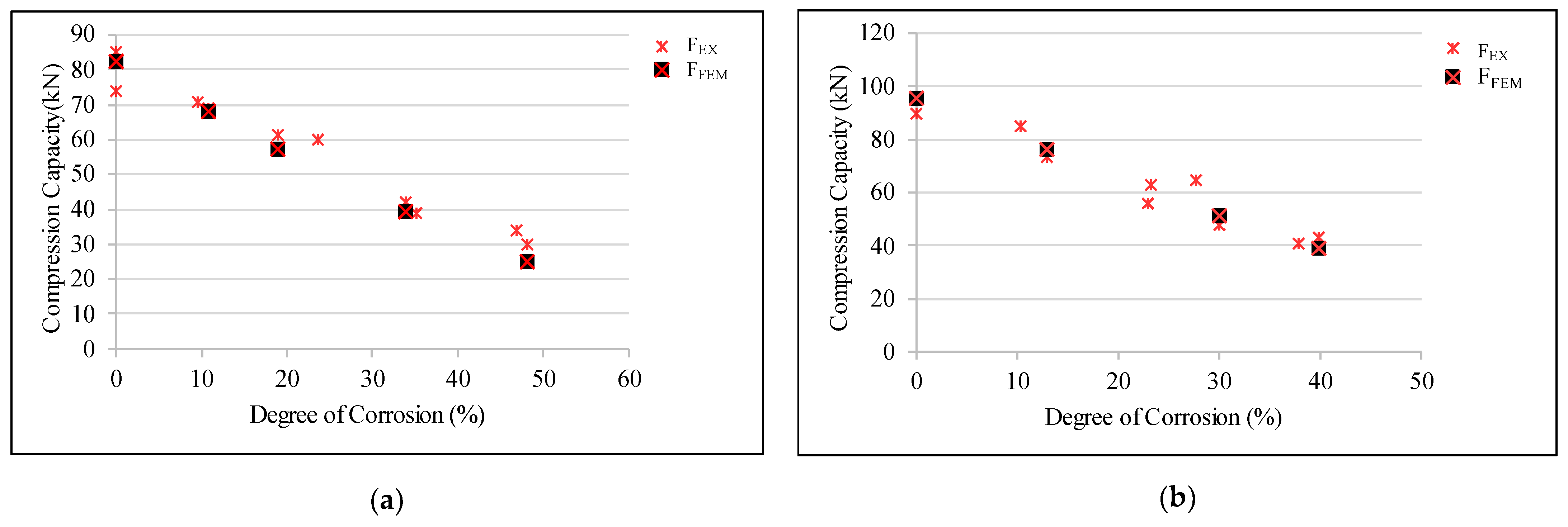
3.1. Code-Based Analysis

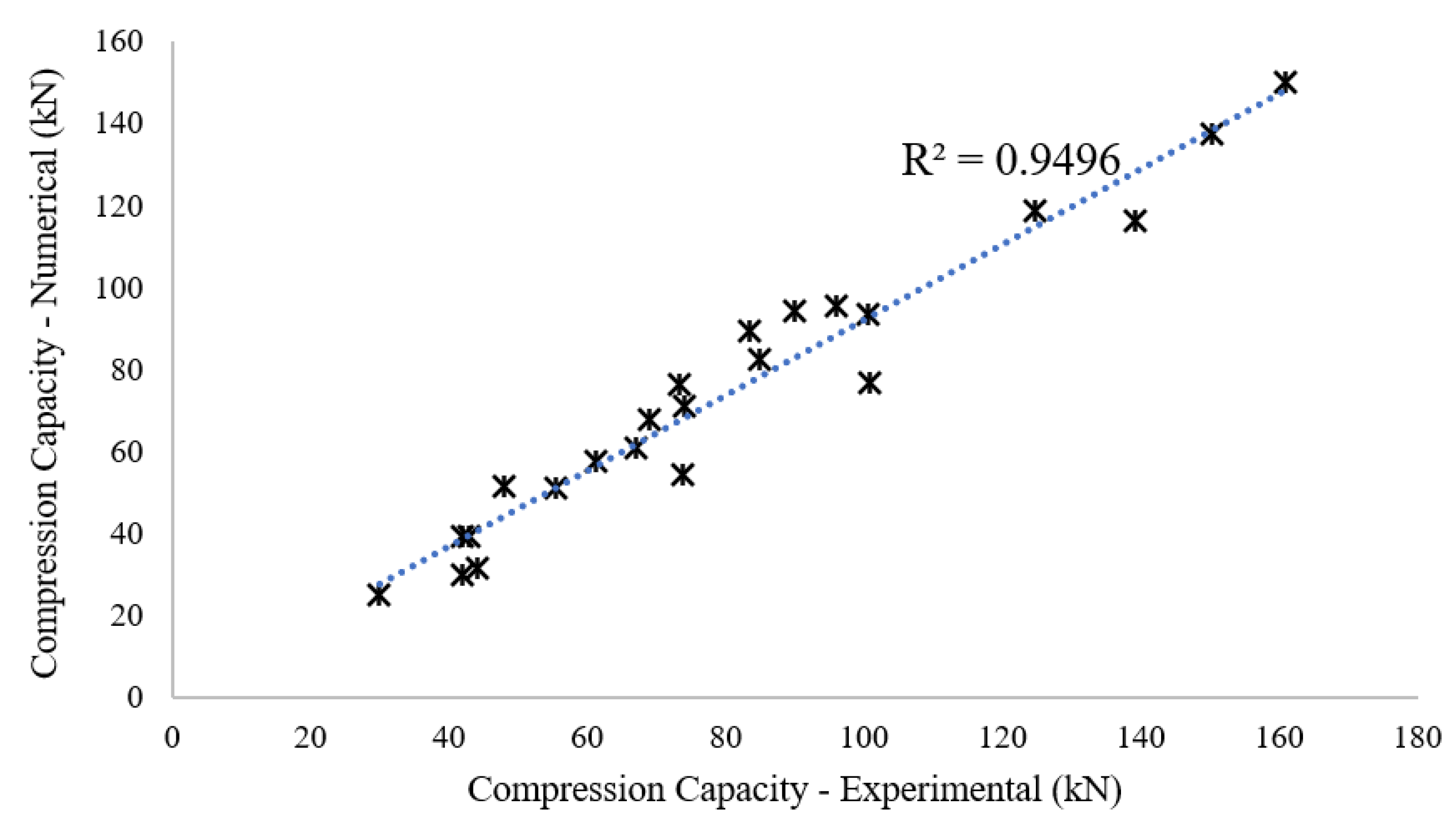
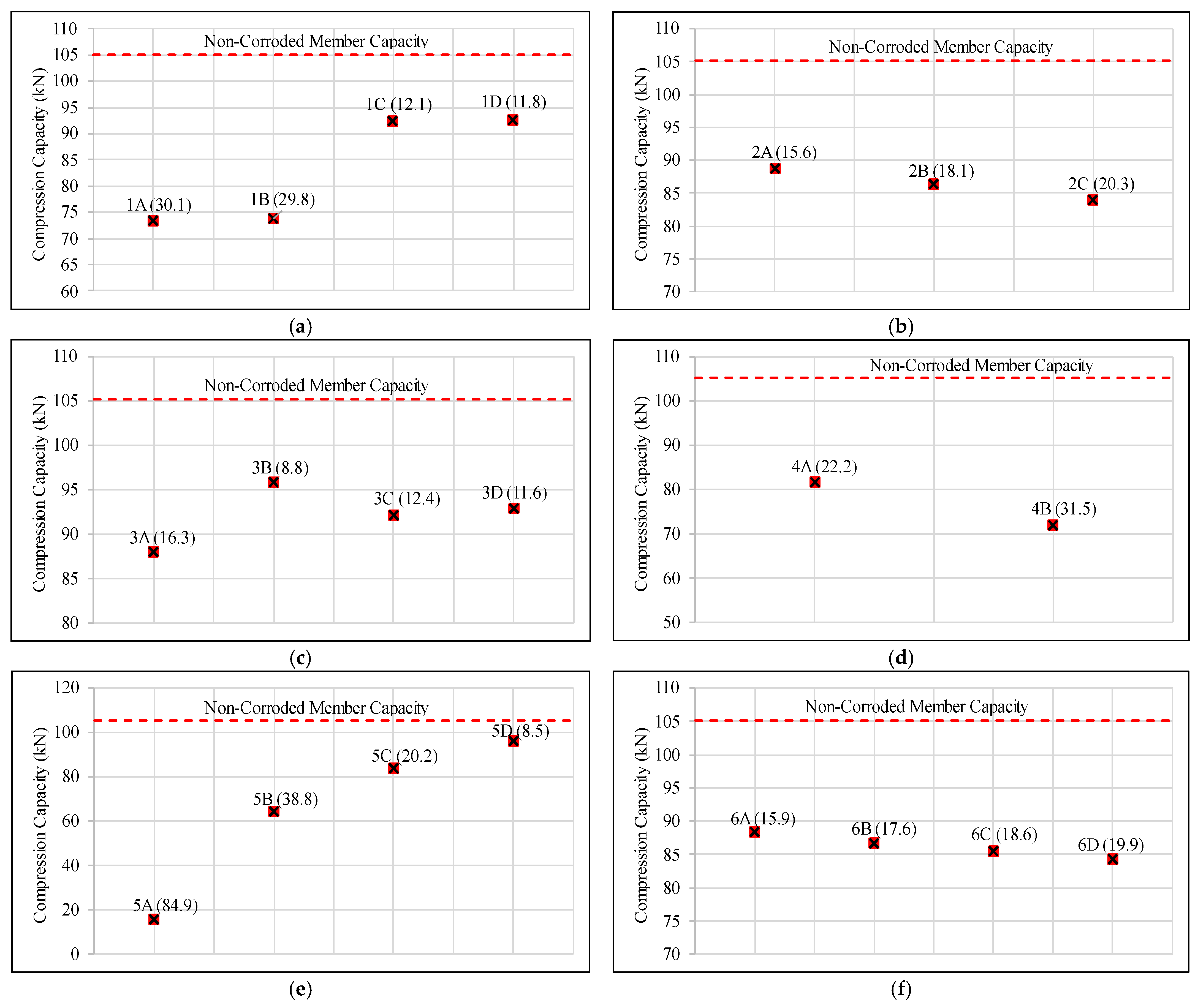
3.2. Numerical Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cantero, D.; Gonzalez, A. Bridge damage detection using weigh-in-motion technology. ASCE J. Bridge Eng. 2015, 20, 04014078. [Google Scholar] [CrossRef]
- Bandara, C.S.; Siriwardane, S.C.; Dissanayake, U.I.; Dissanayake, R. Full range S-N curves for fatigue life evaluation of steels using hardness measurements. Int. J. Fatigue 2016, 82, 325–331. [Google Scholar] [CrossRef]
- Shojai, S.; Schaumann, P.; Braun, M.; Ehlers, S. Influence of pitting corrosion on the fatigue strength of offshore steel structures based on 3D surface scans. Int. J. Fatigue 2022, 164, 107128. [Google Scholar] [CrossRef]
- Han, Q.; Zhao, N.; Xu, J. Recognition and location of steel structure surface corrosion based on unmanned aerial vehicle images. J. Civ. Struct. Health Monit. 2021, 11, 1375–1392. [Google Scholar] [CrossRef]
- Safiuddin, M. Occurrences of corrosion: Causes and prevention. BRAC Univ. J. 2006, III, 71–74. [Google Scholar]
- Wang, R.; Guo, H.; Shenoi, R.A. Experimental and numerical study of localized pitting effect on compressive behavior of tubular members. Mar. Struct. 2020, 72, 102784. [Google Scholar] [CrossRef]
- Aghoury, I.M.E. Numerical Tool for Fatigue Life Prediction of Corroded Steel Riveted Connections Using Various Damage Models. Ph.D. Thesis, Ain Shams University, Cairo, Egypt, April 2012. [Google Scholar]
- Abdulla, W.; Menzemer, C. Finite element analysis of heavy-duty riveted steel grating bridge deck. CivilEng 2021, 2, 485–501. [Google Scholar] [CrossRef]
- Shaheen, M.A.; Galal, M.A.; Cunningham, L.S.; Foster, A.S. New technique to improve the ductility of steel beam to column bolted connections: A numerical investigation. CivilEng 2021, 2, 929–942. [Google Scholar] [CrossRef]
- Leroux, P. Pitting Corrosion Measurement Using 3D Profilometry; Nanovea: Irvine, CA, USA, 2014. [Google Scholar]
- Xiao, L.F.; Peng, J.X.; Zhang, J.R. Mechanical properties of corroded high performance steel specimens based on 3d scanning. Adv. Steel Constr. 2019, 15, 129–136. [Google Scholar]
- Andrade, C.; Alonso, C. Test methods for on-site corrosion rate measurement of steel reinforcement in concrete by means of the polarization resistance method. Mater. Struct. 2004, 37, 623–643. [Google Scholar] [CrossRef]
- Chaminda, S.S.; Ohga, M.; Dissanayake, R.; Taniwaki, K. Different approaches for remaining fatigue life estimation of critical members in railway bridges. Steel Struct. 2007, 7, 263–276. [Google Scholar]
- Ostapenko, A.; Berger, T.W.; Chambers, S.L.; Hebor, M.F. Corrosion Damage Effect on Strength of Tubular Columns with Patch Corrosion; ATLSS: Bethlehem, Palestine, 1996. [Google Scholar]
- Hebor, M.F.; Ricles, J.M. Local buckling strength of patch corrosion damaged steel tubular bracing. Int. J. Steel Struct. 2002, 2, 59–70. [Google Scholar]
- Nazari, M.; Khedmati, M.R.; Khalaj, A.F. A numerical investigation into ultimate strength and buckling behavior of locally corroded steel tubular members. Lat. Am. J. Solids Struct. 2014, 11, 1063–1076. [Google Scholar] [CrossRef]
- Ahn, J.H.; Choi, W.R.; Jeon, S.H.; Kim, S.H.; Kim, I.T. Residual compressive strength of inclined steel tubular members with local corrosion. Appl. Ocean Res. 2016, 59, 498–509. [Google Scholar] [CrossRef]
- Shu, Q.; Wang, K.; Yuan, G.; Zhang, Y.; Lu, L.; Liu, Z. Assessing capacity of corroded angle members in steel structures based on experiment and simulation. Constr. Build. Mater. 2020, 244, 118210. [Google Scholar] [CrossRef]
- Beaulieu, L.V.; Legeron, F.; Langlois, S. Compression strength of corroded steel angle members. J. Constr. Steel Res. 2010, 66, 1366–1373. [Google Scholar] [CrossRef]
- Ozvald, K.; Dunai, L. Effect of corrosion on the buckling of steel angle members. Period. Polytech. Civ. Eng. 2012, 56, 175–183. [Google Scholar] [CrossRef]
- Chen, Z.; Li, J.; Liu, Y.; Wang, Y. Experimental study on axial compression behavior of square steel columns with artificial corrosion damage. J. Constr. Steel Res. 2017, 134, 78–86. [Google Scholar]
- BS5950-1; Structural Use of Steelwork in Building—Part 1: Code of Practice for Design Rolled and Welded Sections. British Standards Institution: London, UK, 2000.
- BS EN1993-1; Design of Steel Structures—Part 1: Design of Steel Structures. British Standards Institution: London, UK, 2000.
- ANSI/AISC 360-16; Specification for Structural Steel Buildings. American Institute of Steel Construction: Chicago, IL, USA, 2016.
- ASCE 10-15; Design of Latticed Steel Transmission Structures. American Society of Civil Engineering: Reston, VI, USA, 2015.
| Standard | BS 5950-1:2000 | BSEN 1993-1-1: 2005 | ANSI/AISC 360-16 | ASCE 10-15 |
|---|---|---|---|---|
| Design compression resistance calculation | ||||
| Design compressive stress calculation | But | But | When, , When, , | When, , When, , When, , When, |
| Slenderness calculation | λ is the greatest of, , but , but , but | Limiting width to thickness ratio for angle members, | For members with normal framing eccentricities at both ends of the unsupported panel, , For members partially restrained against rotation at both ends of the unsupported panel, , | |
| Abbreviations | pc—compressive strength Ag—gross sectional area py—design strength E—modulus of elasticity η—perry factor a = 5.5, for strut curve ‘c’ λ—slenderness λ0—limiting slenderness Lv, La, Lb—lengths about relevant axes rv, ra, rb—radii of gyration about relevant axes | χ—reduction factor for the relevant buckling mode for buckling curve ‘b’ —non-dimensional slenderness | Ae—summation of the effective areas Fcr—critical stress b—width of the element & for angle members λ—width to thickness ratio Fel—elastic local buckling stress Ag—gross sectional area E—modulus of elasticity Fe—elastic buckling stress Fy—specified minimum yield stress R—radius of gyration | Fy—minimum guaranteed yield stress E—modulus of elasticity L—unbraced length r—radius of gyration K—effective length coefficient w—flat width t—thickness of the leg Ψ (constant) = 2.62 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Senevirathna, C.R.; Bandara, C.S.; Siriwardane, S.C. Numerical and Code-Based Investigation on the Impact of Corrosion on the Ultimate Compressive Strength of Steel Angle Members Using Thickness Reduction Method. CivilEng 2023, 4, 506-521. https://doi.org/10.3390/civileng4020029
Senevirathna CR, Bandara CS, Siriwardane SC. Numerical and Code-Based Investigation on the Impact of Corrosion on the Ultimate Compressive Strength of Steel Angle Members Using Thickness Reduction Method. CivilEng. 2023; 4(2):506-521. https://doi.org/10.3390/civileng4020029
Chicago/Turabian StyleSenevirathna, Chamath Ravindu, Chaminda S. Bandara, and Sudath C. Siriwardane. 2023. "Numerical and Code-Based Investigation on the Impact of Corrosion on the Ultimate Compressive Strength of Steel Angle Members Using Thickness Reduction Method" CivilEng 4, no. 2: 506-521. https://doi.org/10.3390/civileng4020029
APA StyleSenevirathna, C. R., Bandara, C. S., & Siriwardane, S. C. (2023). Numerical and Code-Based Investigation on the Impact of Corrosion on the Ultimate Compressive Strength of Steel Angle Members Using Thickness Reduction Method. CivilEng, 4(2), 506-521. https://doi.org/10.3390/civileng4020029







