Evaluation of Concrete Material Properties at Early Age
Abstract
:1. Introduction
2. Materials and Methods
3. Results
4. Discussion
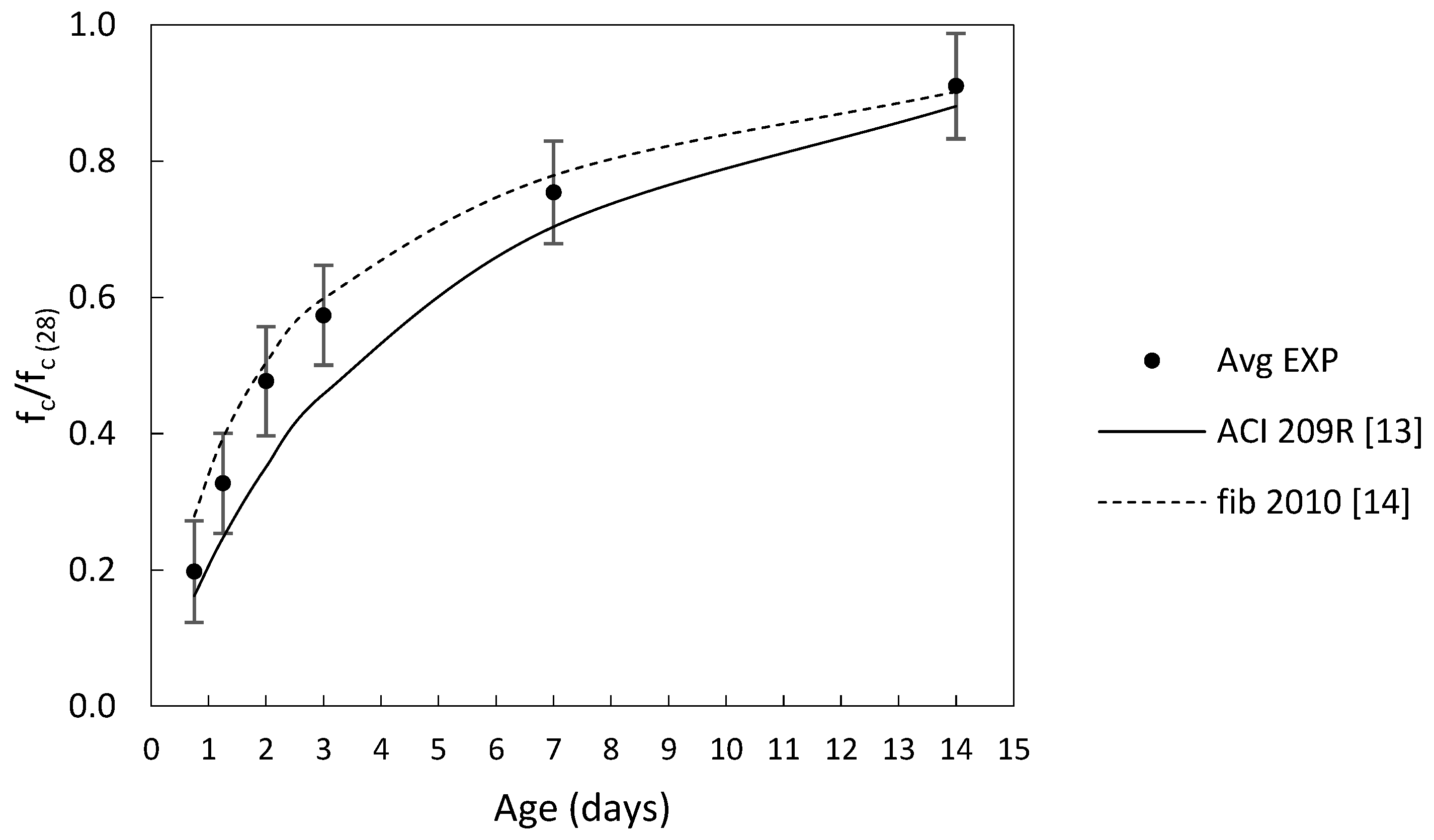
4.1. Compressive Strength Development with Time
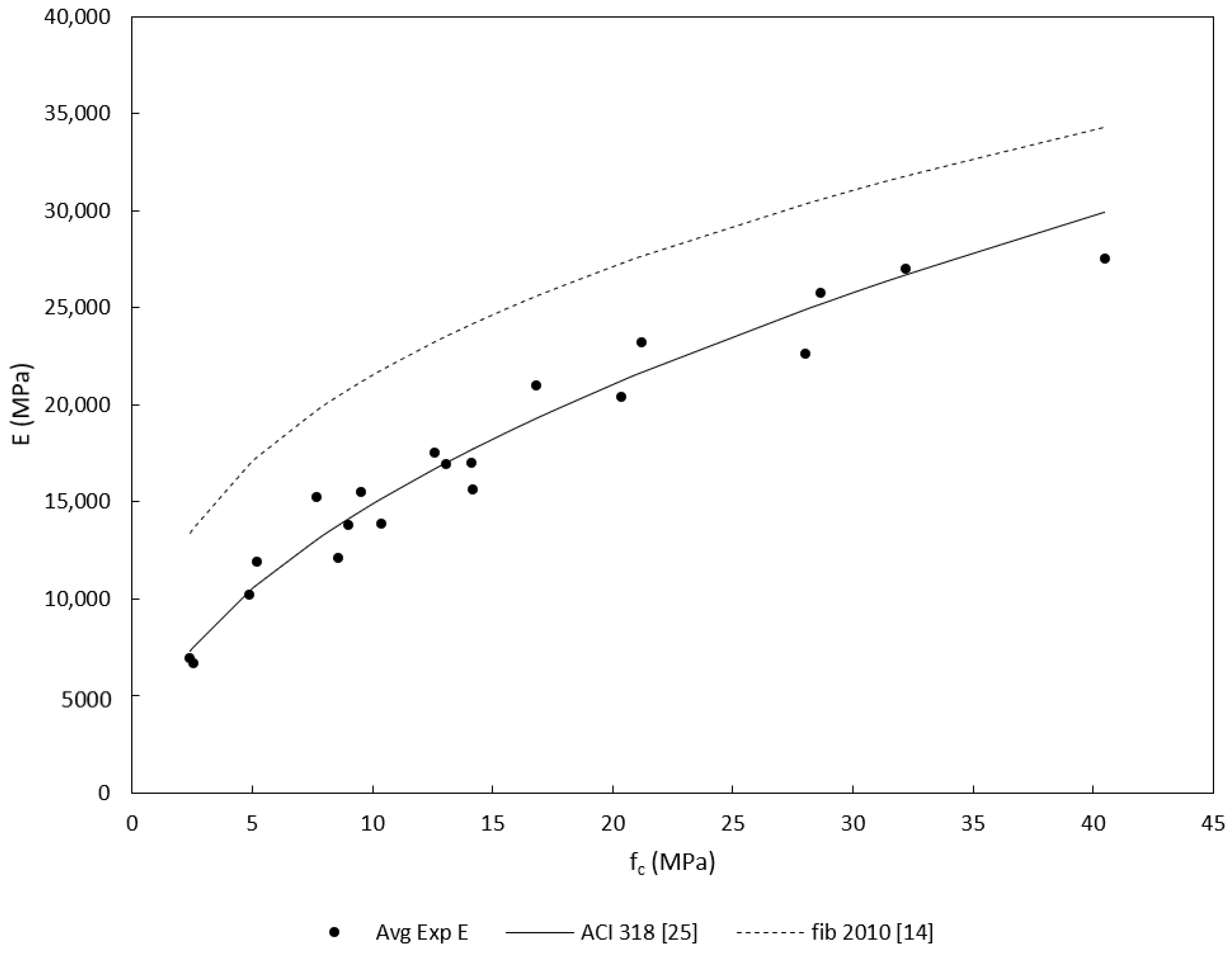
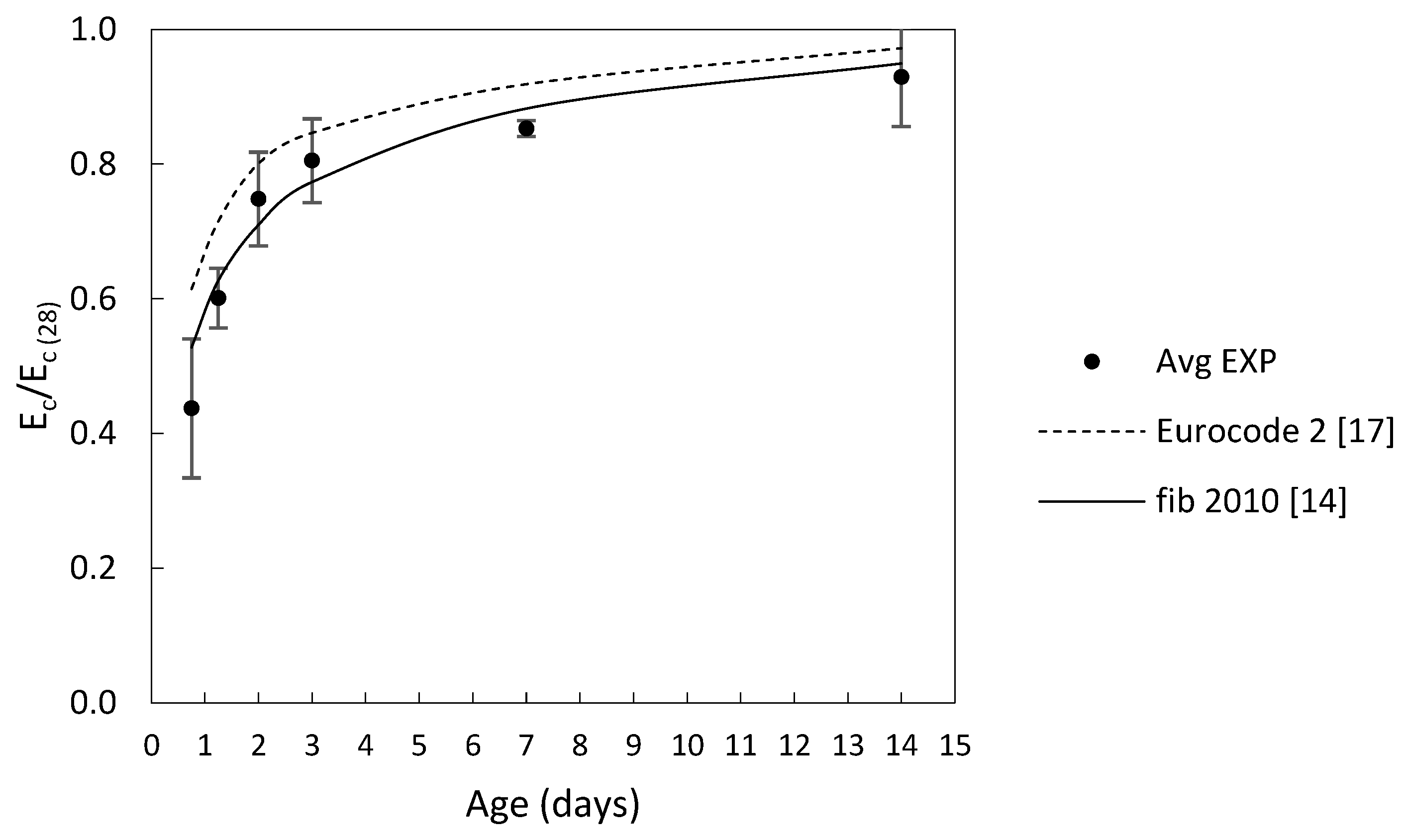
4.2. Modulus of Elasticity
4.3. Tensile Strength
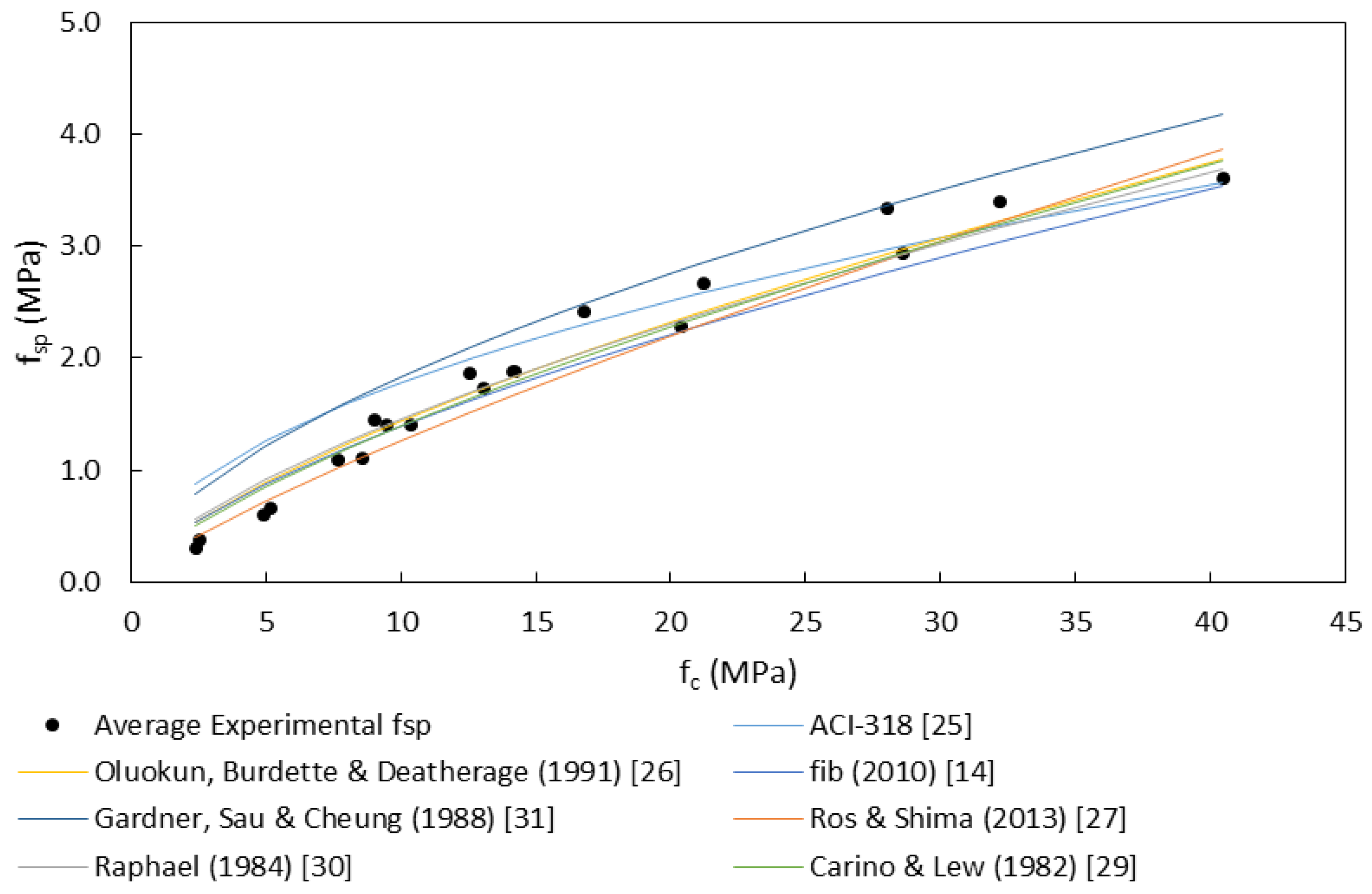
4.3.1. Splitting Tensile Strength
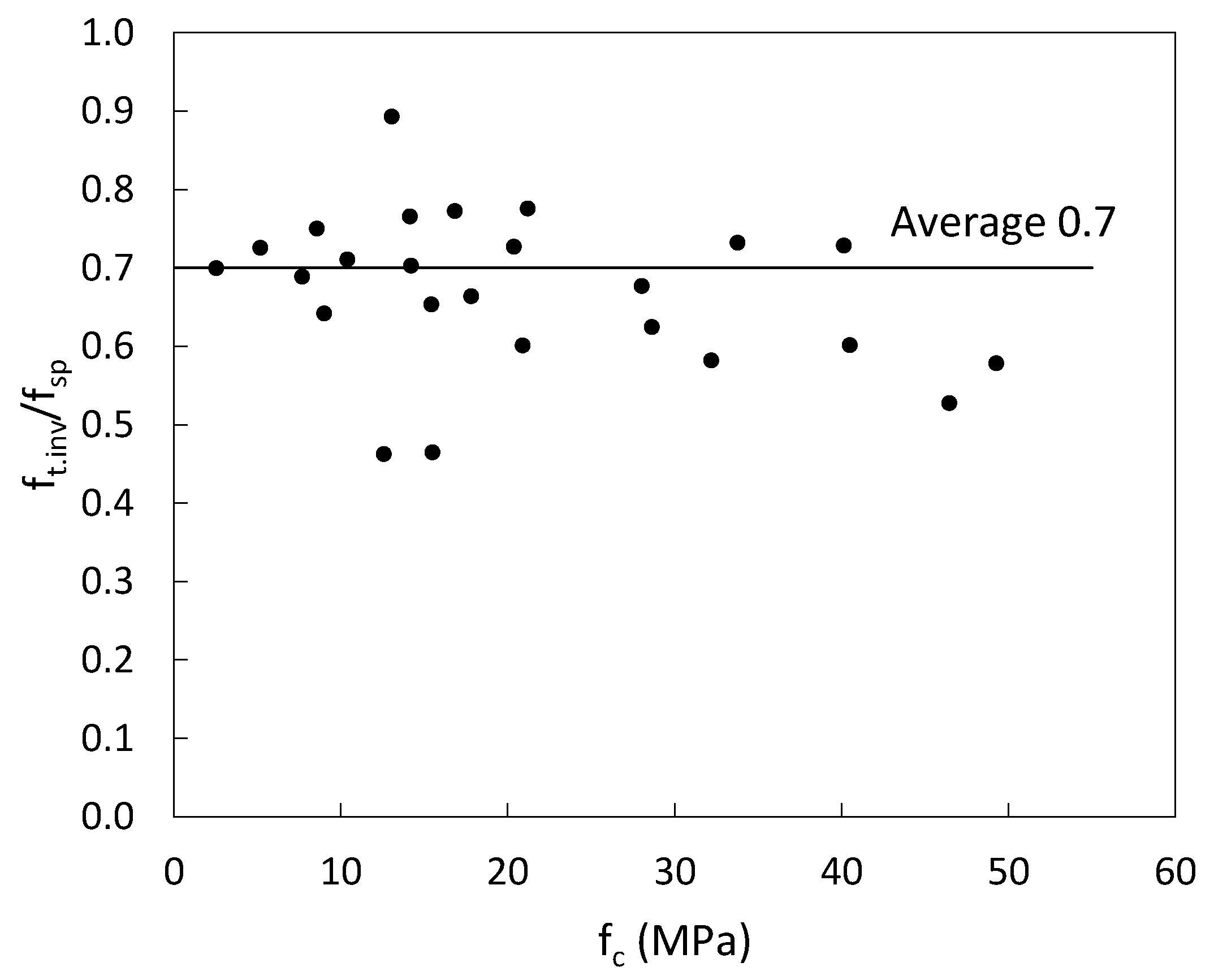
4.3.2. Inverse Analysis of the Wedge-Splitting Test
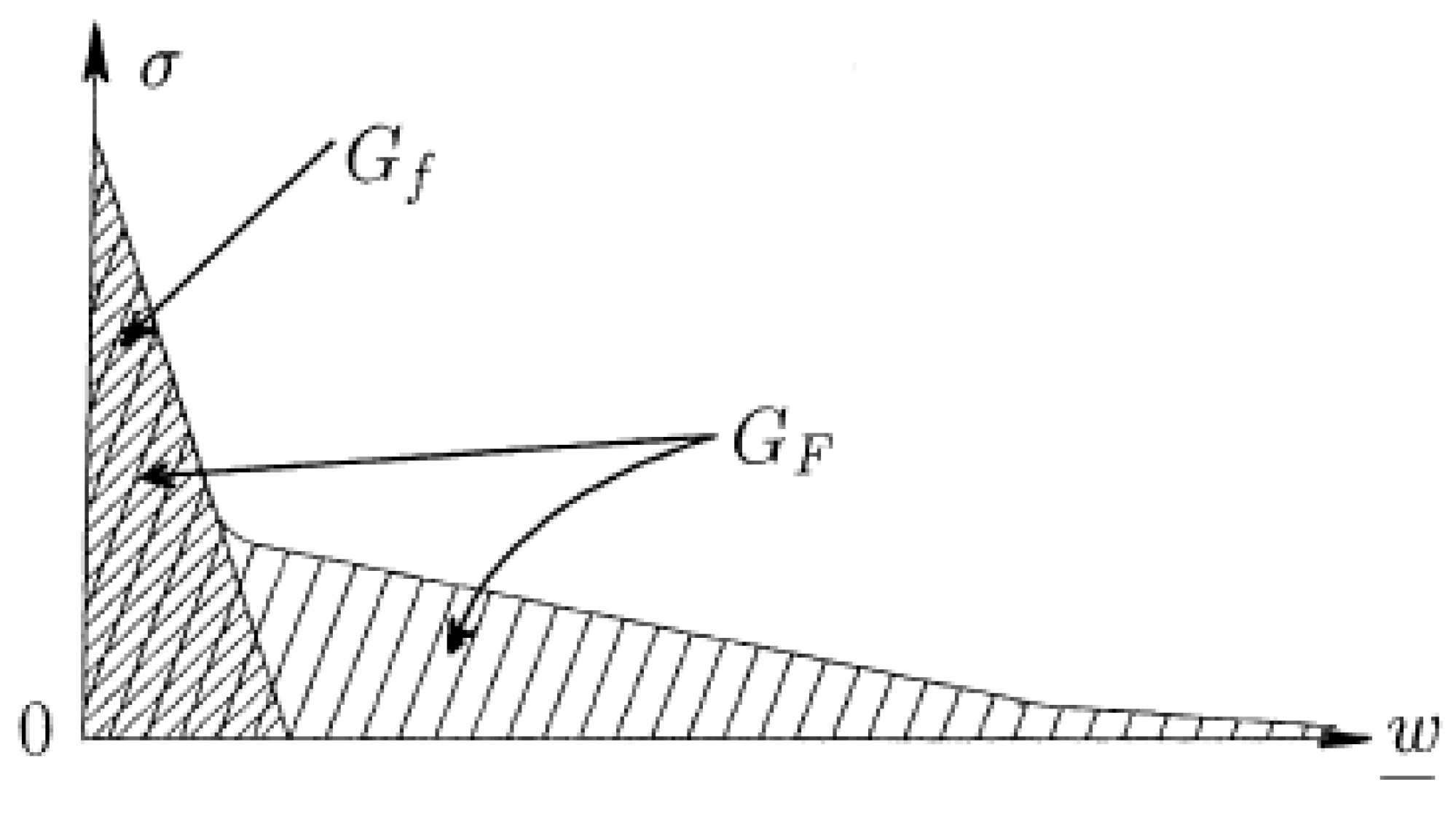
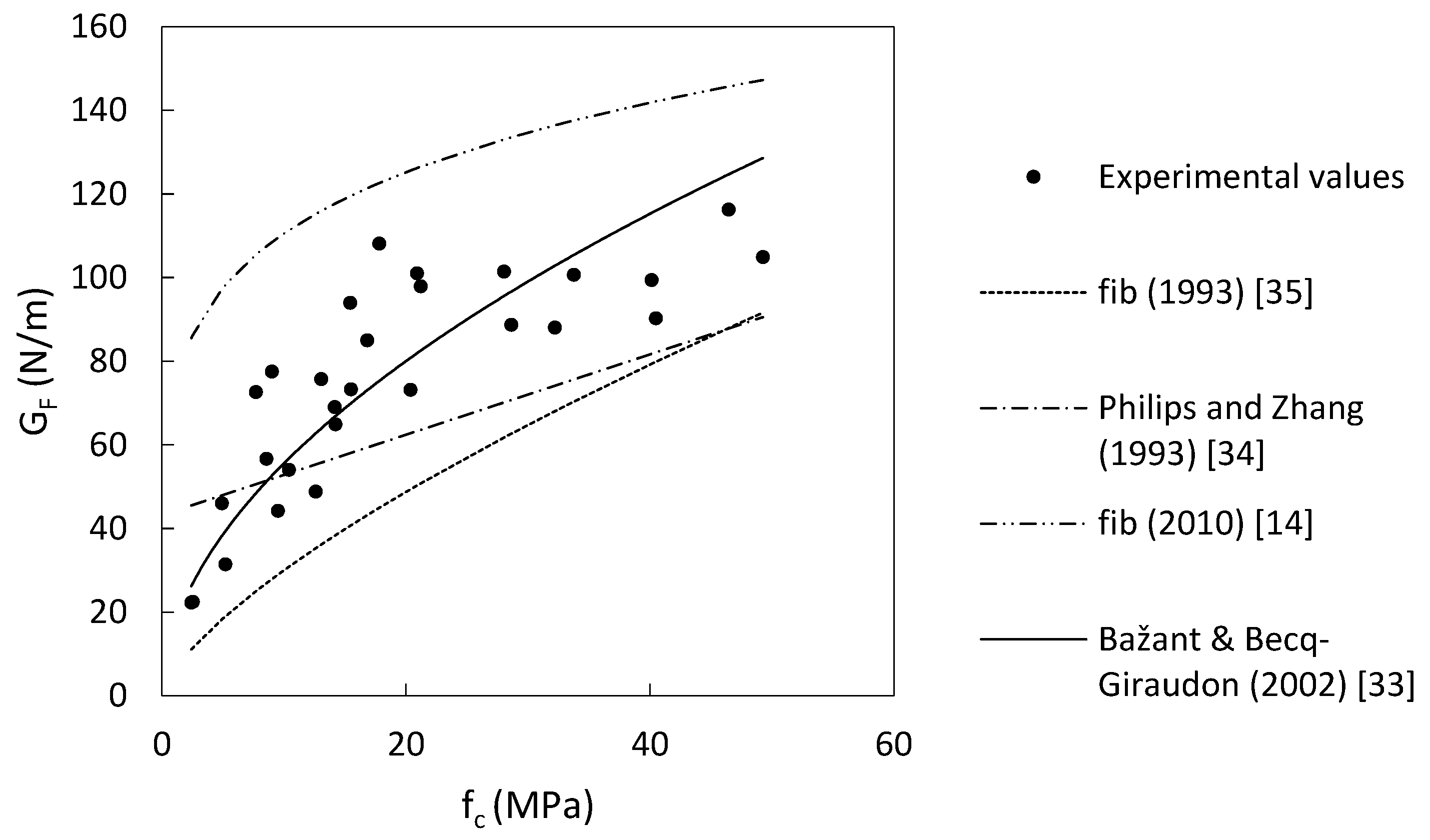
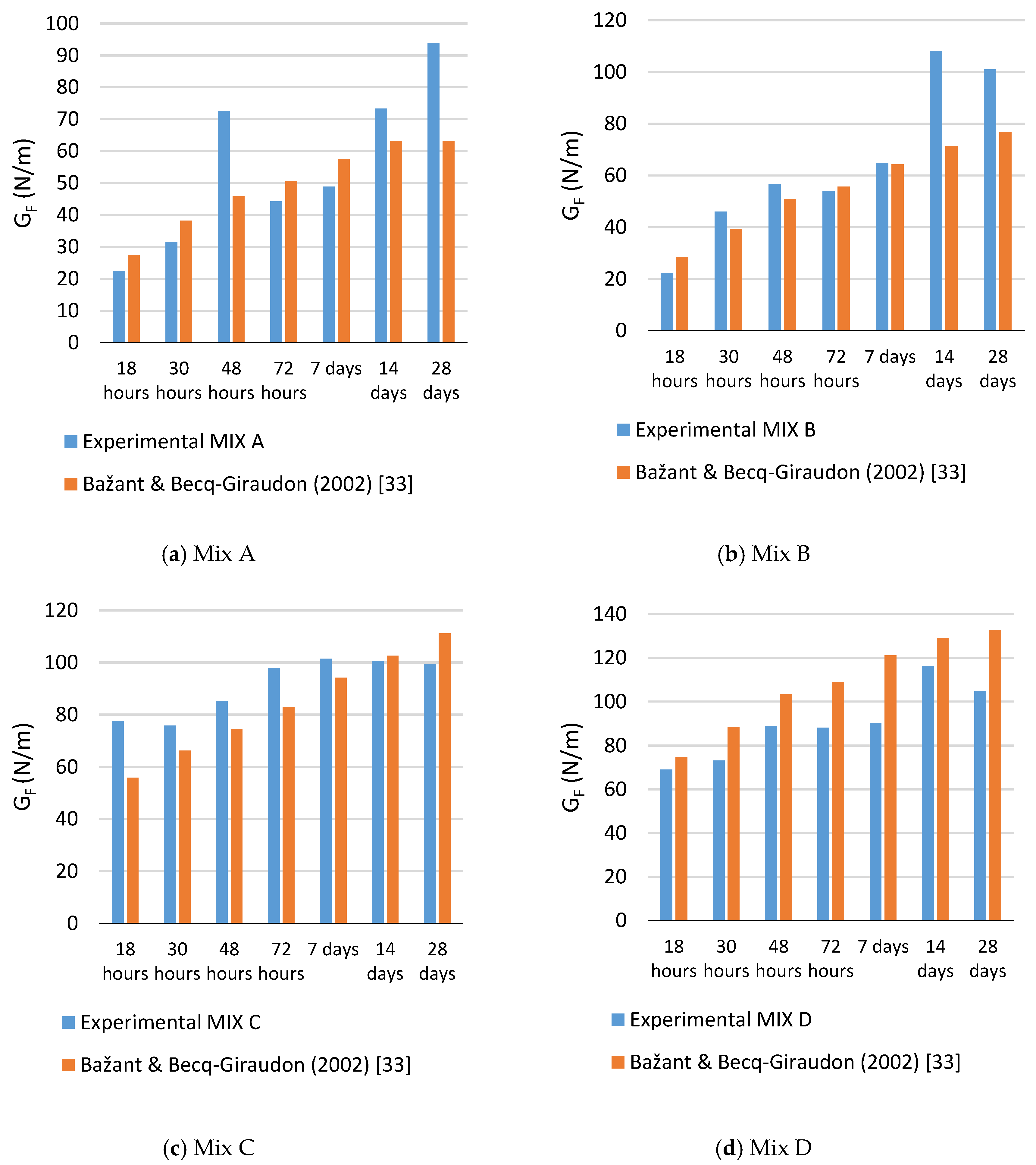
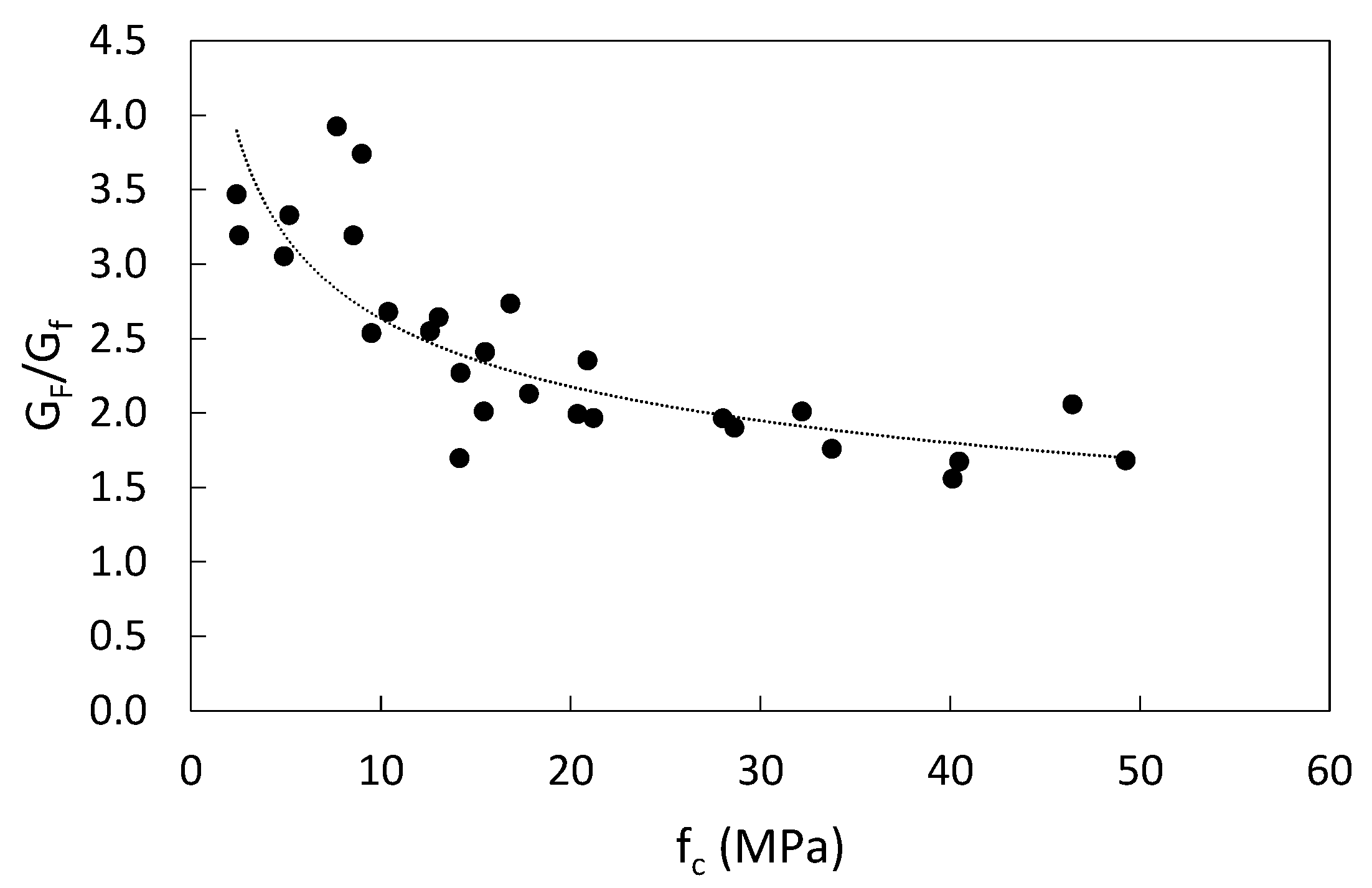
4.4. Fracture Energy
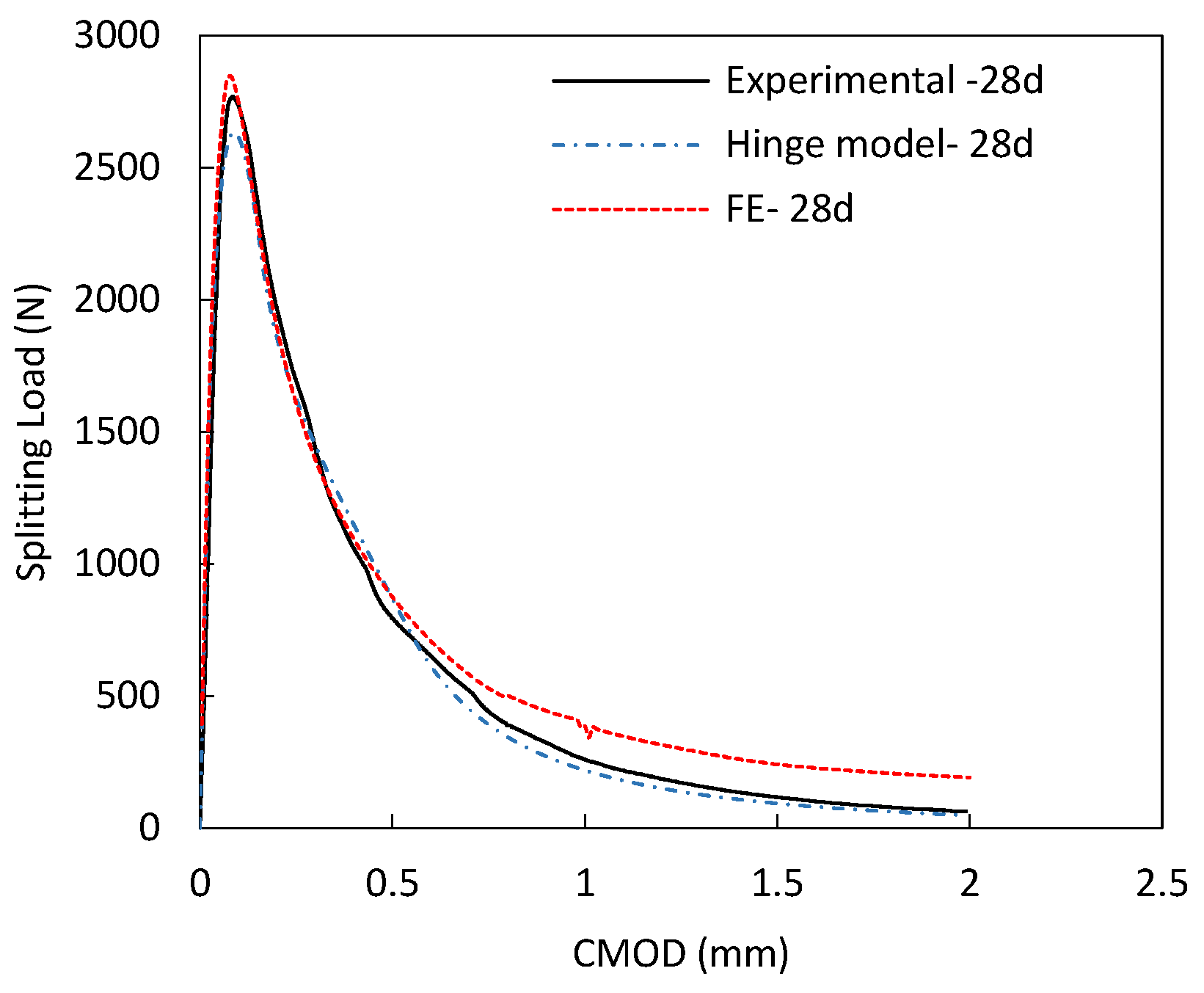
4.5. Finite Element Analysis of the Wedge-Splitting Test
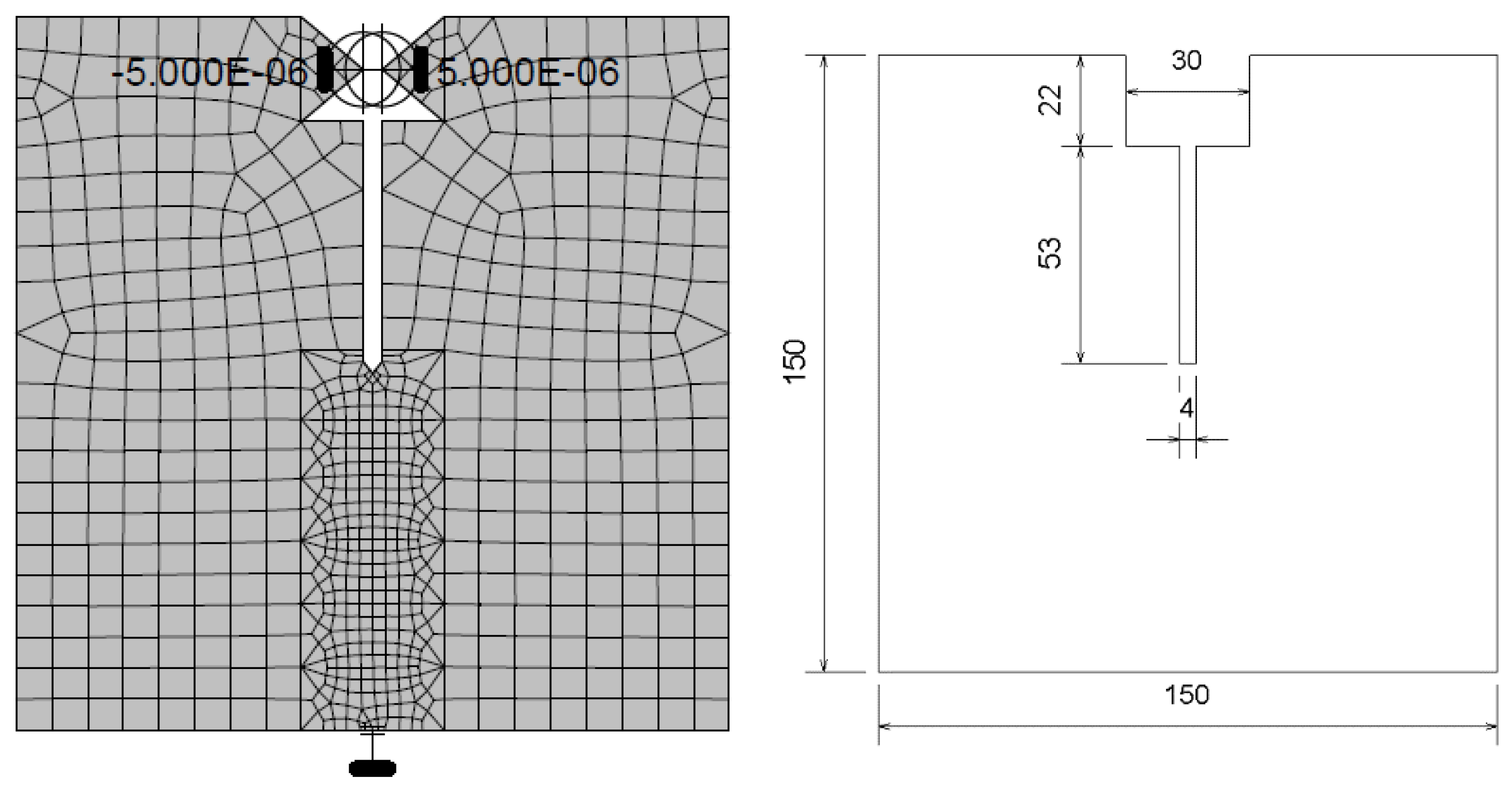
4.5.1. Finite Element Model and Boundary Conditions
4.5.2. Aims and Significance
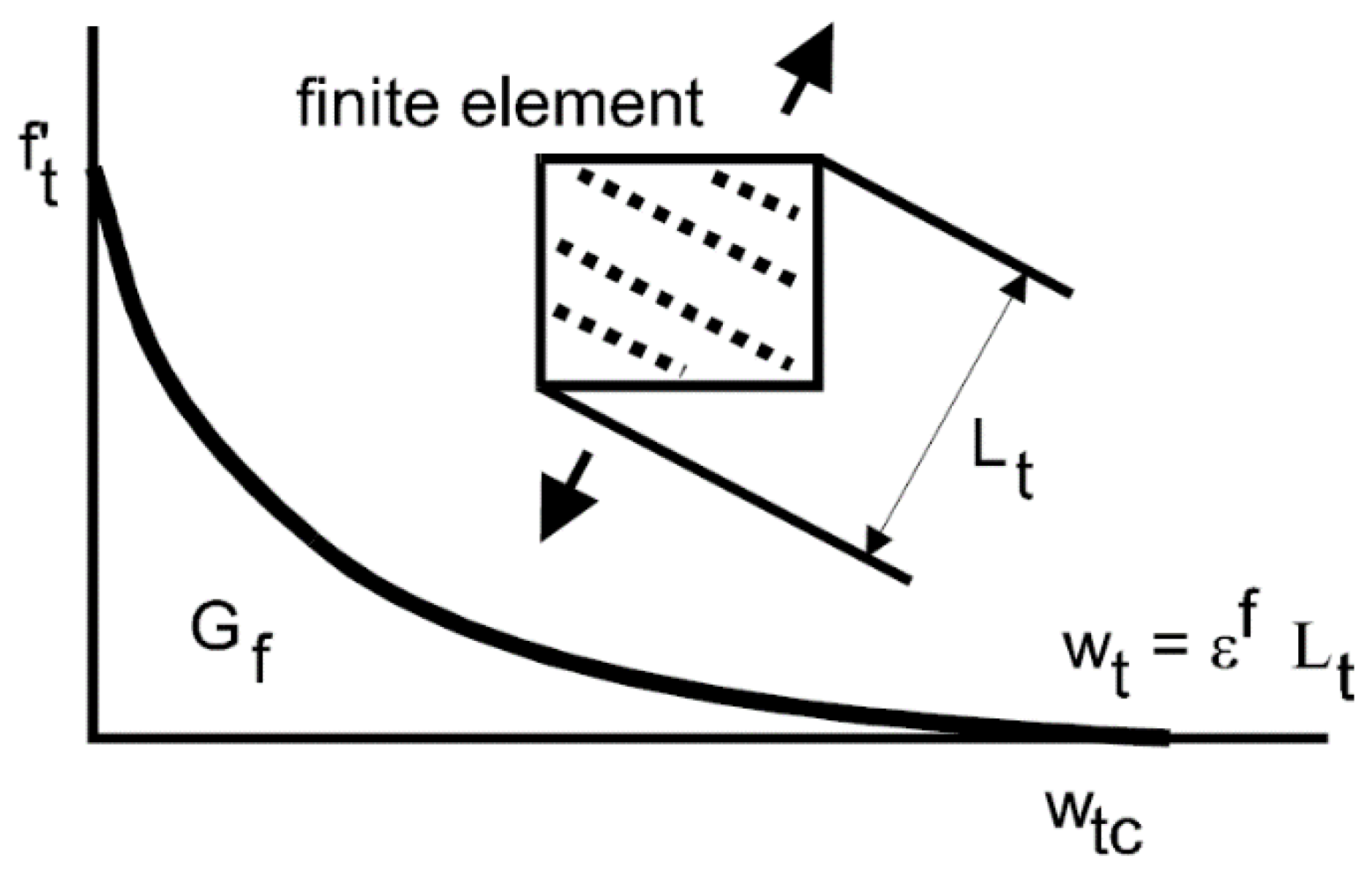
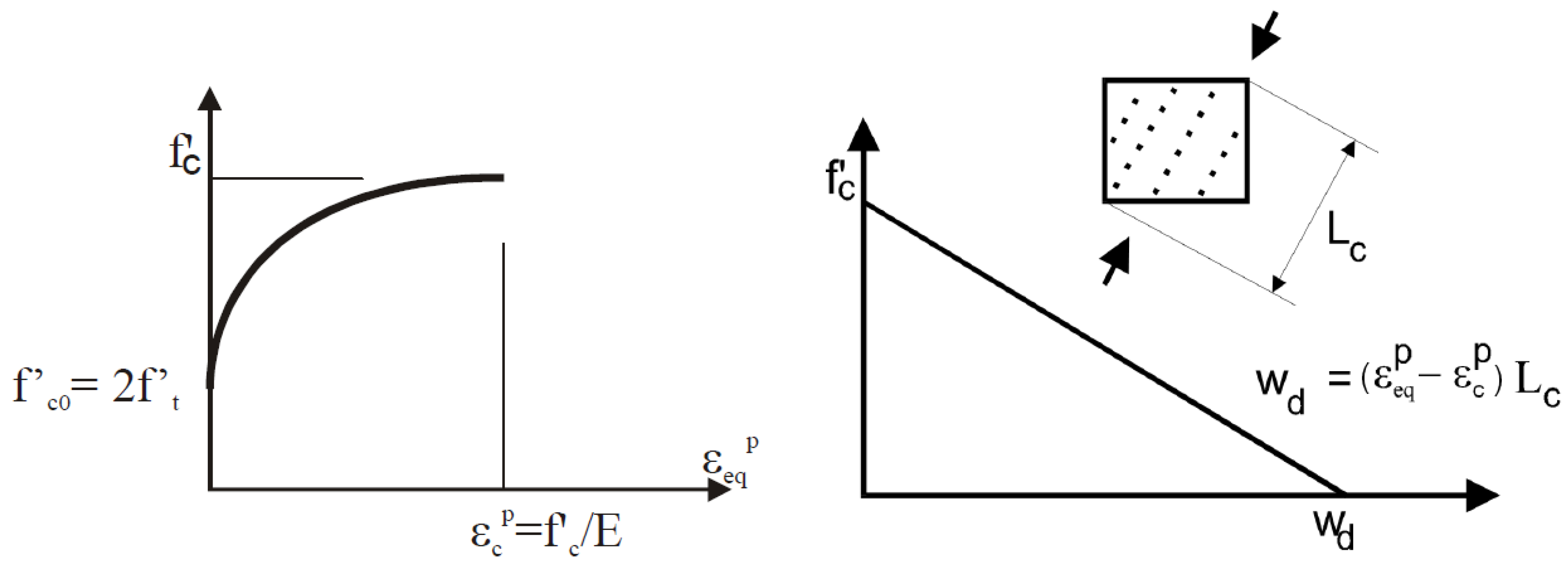
4.5.3. Material Properties
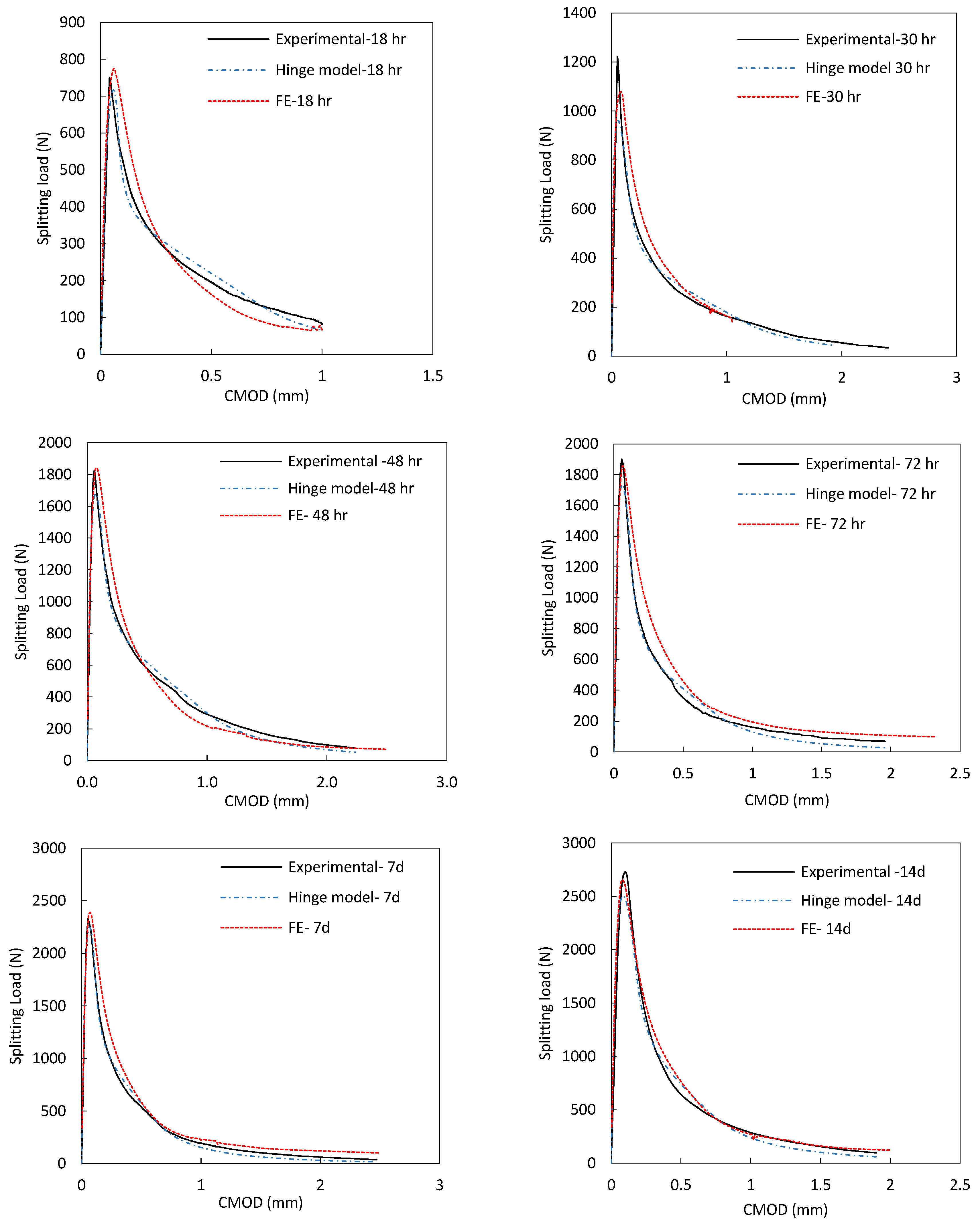
4.5.4. Results and Discussion
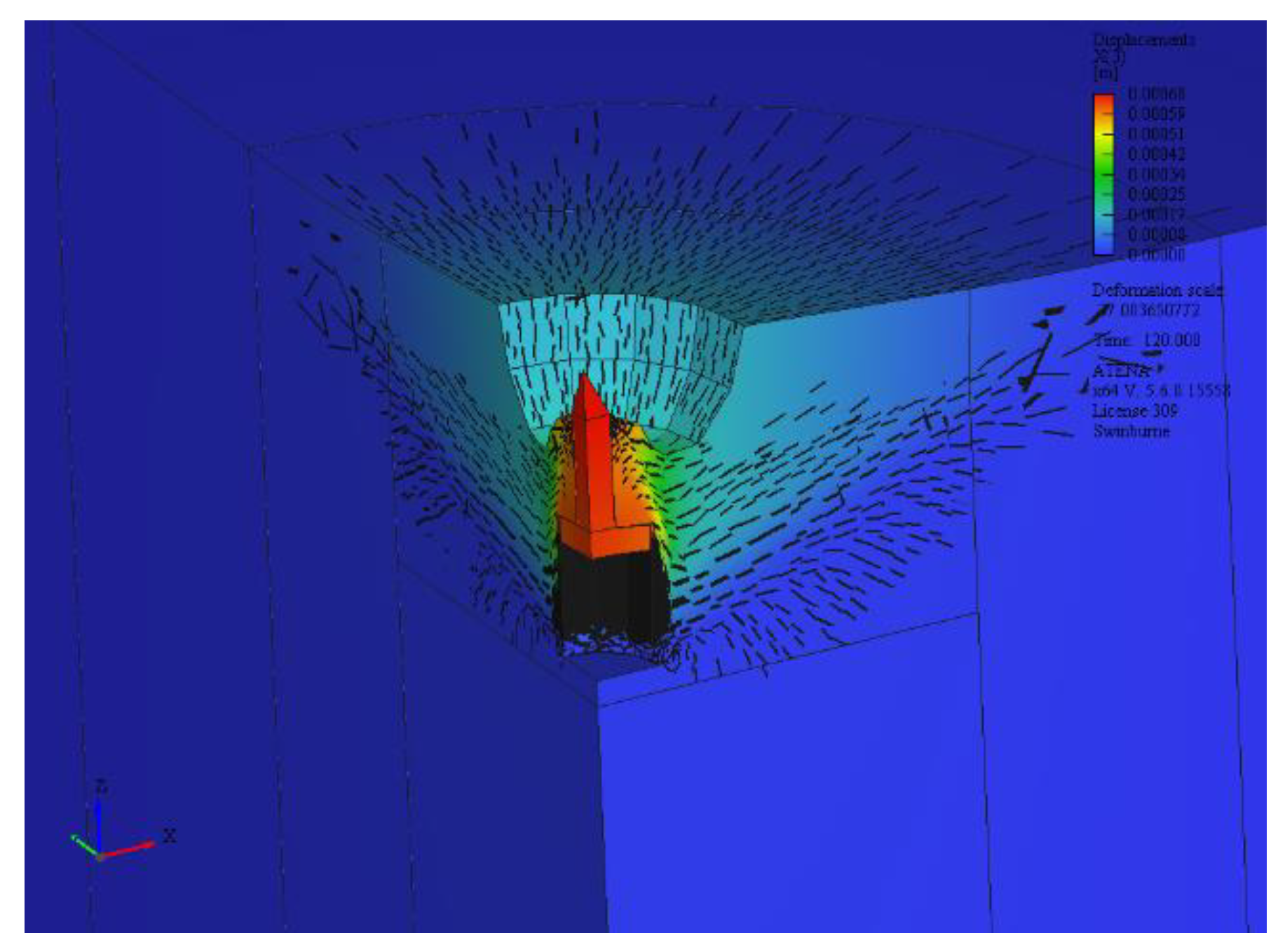
4.6. Finite Element Analysis of Anchor Pull-Out Test
4.6.1. Geometry and Setup
4.6.2. Material Properties
4.6.3. Discussion of Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nilforoush, R. Anchorage in Concrete Structures; Department of Civil, Environmental and Natural Resources Engineering, Lulea University of Technology: Lulea, Sweden, 2017. [Google Scholar]
- Fuchs, W.; Eligehausen, R.; Breen, E.J. Concrete Capacity Design (CCD) Approach for Fastening to Concrete. ACI Struct. J. 1995, 92, 73–94. [Google Scholar]
- Comité Euro-International du Béton. Fastenings to Concrete and Masonry Structures State of the Art Report; Thomas Telford: London, UK, 1994. [Google Scholar]
- Eligehausen, R.; Mallee, R.; Silva, J.F. Anchorage in Concrete Construction, 1st ed.; Wilhelm Ernst & Sohn Verlag fur Architektur und Technische: Berlin, Germany, 2006. [Google Scholar]
- Standards Australia. AS 3600-2009 Concrete Structures; Standards Australia: Sydney, Australia, 2009. [Google Scholar]
- Standards Australia. AS 1012.9 Method of Testing Concrete: Compressive Strength Tests—Concrete, Mortar and Grout Specimens; Standards Australia: Sydney, Australia, 2014. [Google Scholar]
- Standards Australia. AS 1012.10 Methods for Testing Concrete: Determination of Indirect Tensile Strength of Concrete Cylinders (Brazil or Splitting Test); Standards Australia: Sydney, Australia, 2014. [Google Scholar]
- Standards Australia. AS 1012.17 Methods of Testing Concrete: Determination of the Static Chord Modulus of Elasticity and Poisson’s Ratio of Concrete Specimens; Standards Australia: Sydney, Australia, 2014. [Google Scholar]
- Linsbauer, H.N.; Tschegg, E.K. Fracture energy determination of concrete with cube-shaped specimens. Zem. Beton 1986, 31, 38–40. [Google Scholar]
- Brühwiler, E.; Wittmann, F.H. The wedge splitting test, a new method of performing stable fracture mechanics tests. Eng. Fract. Mech. 1990, 35, 117–125. [Google Scholar] [CrossRef]
- Ostergaard, L. Early Age Fracture Mechanics and Cracking of Concrete: Experiments and Modelling; Technical University of Denmark: Lyngby, Denmark, 2003. [Google Scholar]
- Lofgren, I.; Olsen, J.F.; Flansbjer, M. Application of WST—Method for Fracture Testing of Fibre-Reinforced Concrete; Nordic Innovation Centre: Oslo, Norway, 2005. [Google Scholar]
- American Concrete Institute. Prediction of Creep, Shrinkage and Temperature Effects in Concrete Structures; ACI 209R-92; American Concrete Institute: Farmington Hills, MI, USA, 1997. [Google Scholar]
- International Federation for Structural Concrete (fib). Model Code 2010; International Federation for Structural Concrete: Lausanne, Switzerland, 2010. [Google Scholar]
- Mehta, P.K. Concrete: Microstructure, Properties, and Materials, 3rd ed.; McGraw-Hill: New York, NY, USA, 2006. [Google Scholar]
- Oluokun, F.; Burdette, E.; Deatherage, H. Elastic Modulus, Poisson’s Ratio, and Compressive Strength Relationships at Early Ages. ACI Mater. J. 1991, 88, 3–10. [Google Scholar]
- European Committee for Standardization CEN. Eurocode 2: Design of Concrete Structures; CEN: Brussels, Belgium, 2004. [Google Scholar]
- Hanjari, K. Evaluation of WST Method as a Fatigue Test for Plain and Fiber-Reinforced Concrete; Chalmers University of Technology: Goteborg, Sweden, 2006. [Google Scholar]
- Skocek, J. Fracture Propagation in Cementitious Materials; Technical University of Denmark: Lyngby, Denmark, 2010. [Google Scholar]
- Hodicky, K.; Hulin, T.; Schmidt, J.; Stang, H. Wedge Splitting Test on Fracture Behaviour of Fiber Reinforced and Regular High Performance Concretes. In Proceedings of the 13th International Conference on Fracture, Beijing, China, 16–21 June 2013. [Google Scholar]
- Abdalla, H.; Karihaloo, B. A method for constructing the bilinear tension softening diagram of concrete corresponding to its true fracture energy. Mag. Concr. Res. 2004, 56, 597–604. [Google Scholar] [CrossRef]
- Polies, W. Identification of Concrete Fracture Parameters Using Digital Image Correlation and Inverse Analysis; University of Wndsor: Windsor, ON, Canada, 2013. [Google Scholar]
- Li, Z. Advanced Concrete Technology; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Tang, T. Effects of load distributed width on split tension of unnotched and notched cylindrical specimens. J. Test. Eval. 1994, 22, 401–409. [Google Scholar]
- American Concrete Institute. Building code requirements for structural concrete (ACI 318-14 metric) and commentary. In ACI 318-11 (Metric); American Concrete Institute: Farmington Hills, MI, USA, 2014. [Google Scholar]
- Oluokun, F.A.; Burdette, E.G.; Deatherage, J.H. Splitting Tensile Strength and Compressive Strength Relationships at Early Ages. Mater. J. 1991, 88, 115–121. [Google Scholar]
- Ros, S.; Shima, H. Relationship between splitting tensile strength and compressive strength of concrete at early age with different types of cements and curing temperature histories. Concrete Inst. Proc. 2013, 35, 427–432. [Google Scholar]
- Chhorn, C.; Hong, S.J.; Lee, S.W. Relationship between compressive and tensile strengths of roller-compacted concrete. J. Traffic Transp. Eng. 2018, 5, 215–223. [Google Scholar] [CrossRef]
- Carino, N.J.; Lew, H.S. Re-examination of the Relation Between Splitting Tensile andCompressive Strength of Normal Weight Concrete. ACI J. 1982, 79, 214–219. [Google Scholar]
- Raphael, J.M. Tensile Strength of Concrete. ACI J. 1984, 81, 158–165. [Google Scholar]
- Gardner, N.J.; Sau, P.L.; Cheung, M.S. Strength Development and Durability of Concretes Cast and Cured at 0 C. Mater. J. 1988, 85, 529–536. [Google Scholar]
- Herdholdt, A.; Justesen, C.; Nepper-Chrostensen, P.; Nielsen, A. Beton-Bogen, 2nd ed.; Aalborg Portland: Aalborg, Denmark, 1985. [Google Scholar]
- Bažant, Z.P.; Becq-Giraudon, E. Statistical prediction of fracture parameters of concrete and implications for choice of testing standard. Cem. Concr. Res. 2002, 32, 529–556. [Google Scholar] [CrossRef]
- Phillips, D.V.; Zhang, B. Direct tension tests on notched and un-notched plain concrete specimens. Mag. Concr. Res. 1993, 45, 25–35. [Google Scholar] [CrossRef]
- Comité Euro-International du Béton; Fédération Internationale de la Précontrainte. CEB-FIP Model Code 1990 Design Code; Thomas Telford: London, UK, 1993. [Google Scholar]
- Hordijk, A. Local Approach to Fatigue of Concrete; Delft University of Technology: Delft, The Netherlands, 1991. [Google Scholar]
- Červenka, V.; Jendele, L.; Červenka, J. ATENA Program Documentation: Theory; Červenka Consulting: Prague, Czech Republic, 2017. [Google Scholar]
- Bazant, Z.P.; Oh, B. Crack Band Theory for Fracture of Concrete. Mater. Struct. 1983, 16, 155–177. [Google Scholar]
- Bui, T.T.; Limam, A.; Nana, W.S.A.; Arrieta, B.; Roure, T. Cast-in-place Headed Anchor Groups Under Shear: Experimental and Numerical Modelling. Structures 2018, 14, 178–196. [Google Scholar] [CrossRef]
- Poveda, E.; Ortega, J.J.; Ruiz, G.; Porras, R.; Carmona, J.R. Normal and tangential extraction of embedded anchor plates from precast façade concrete panels. Eng. Struct. 2016, 110, 21–35. [Google Scholar] [CrossRef]
- Barnat, J. Analysis of Bonded Anchor in Combined Concrete-Bond Failure Mode. In Recent Researches in Geography Geology Energy Environment and Biomedicine; WSEAS: Stevens Point, WI, USA, 2011. [Google Scholar]
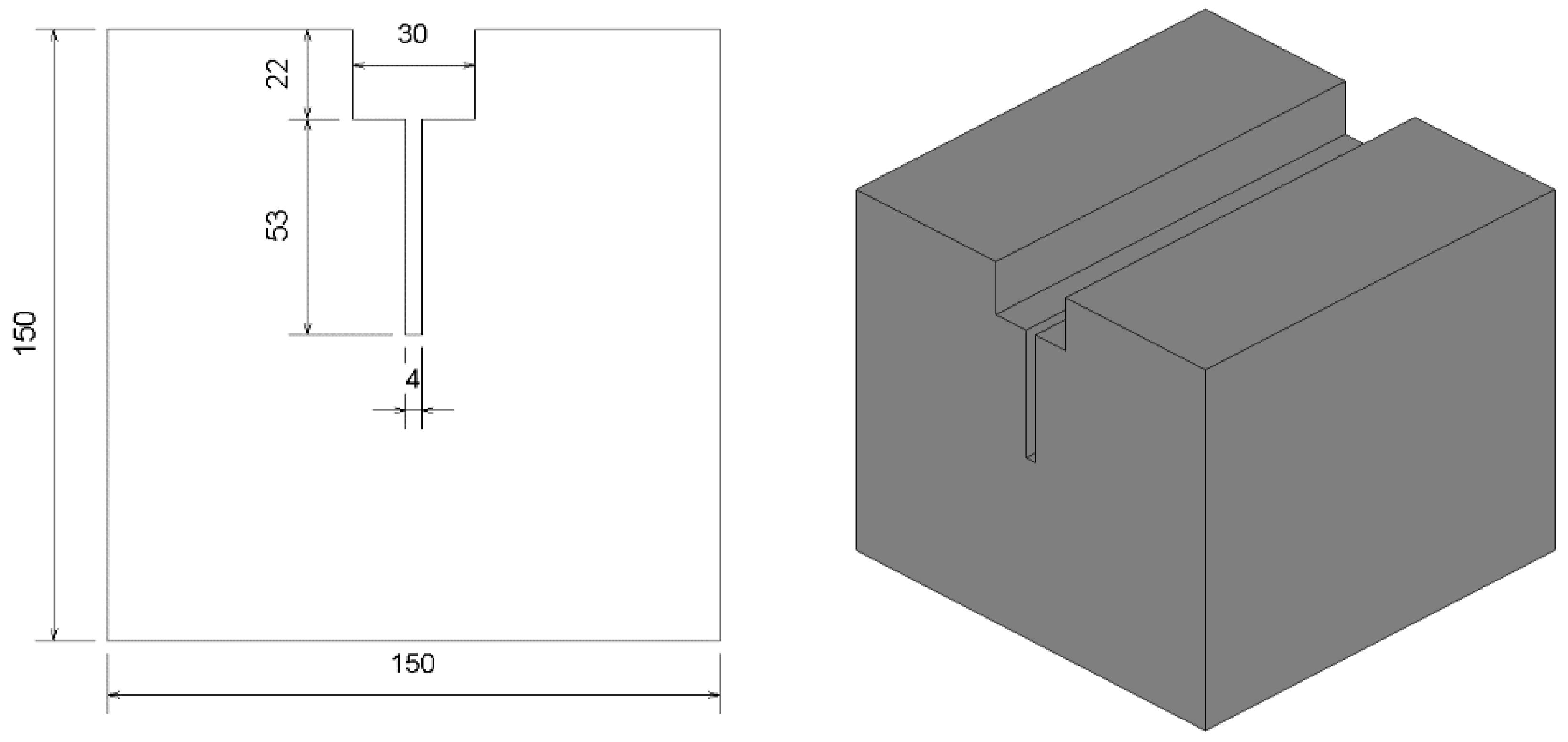

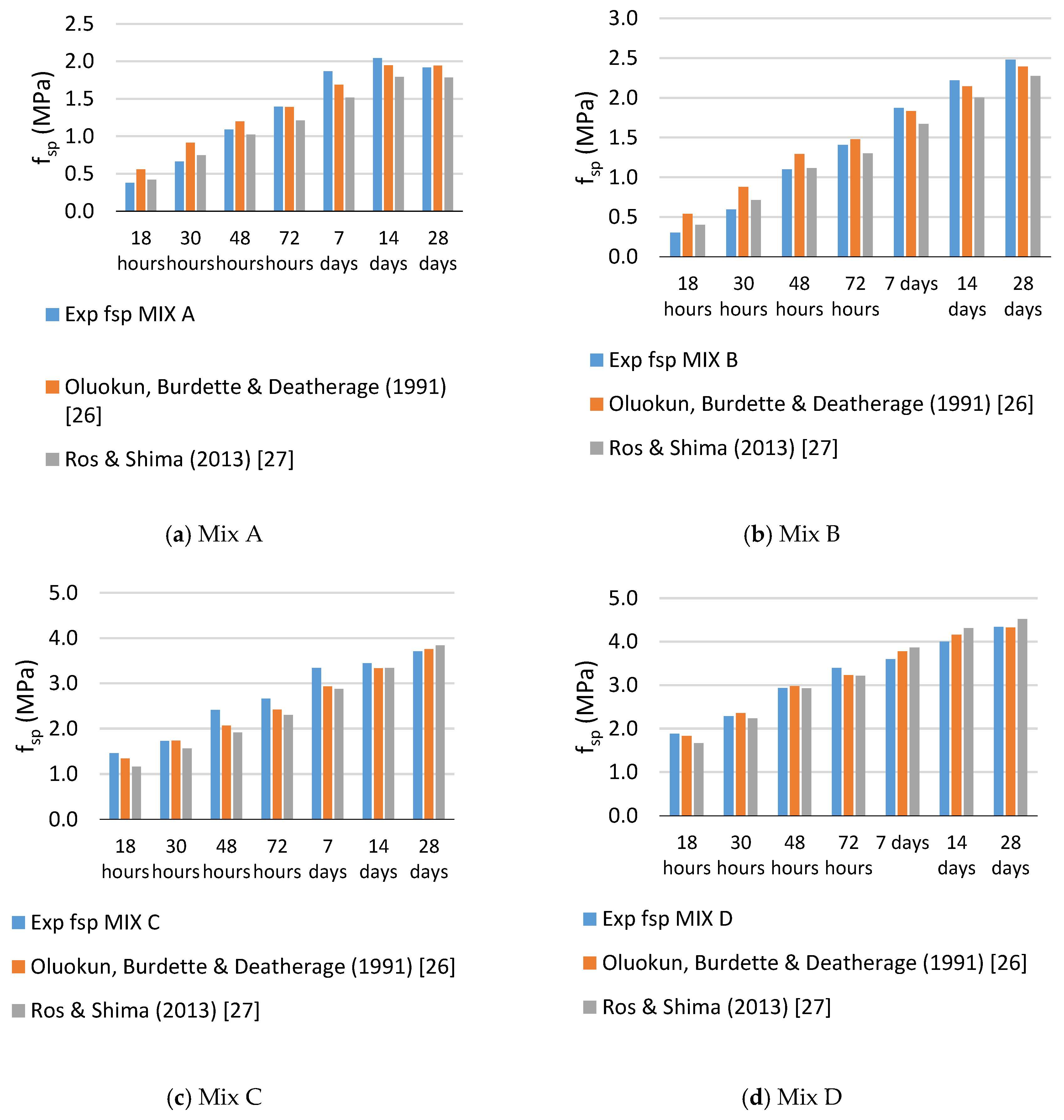
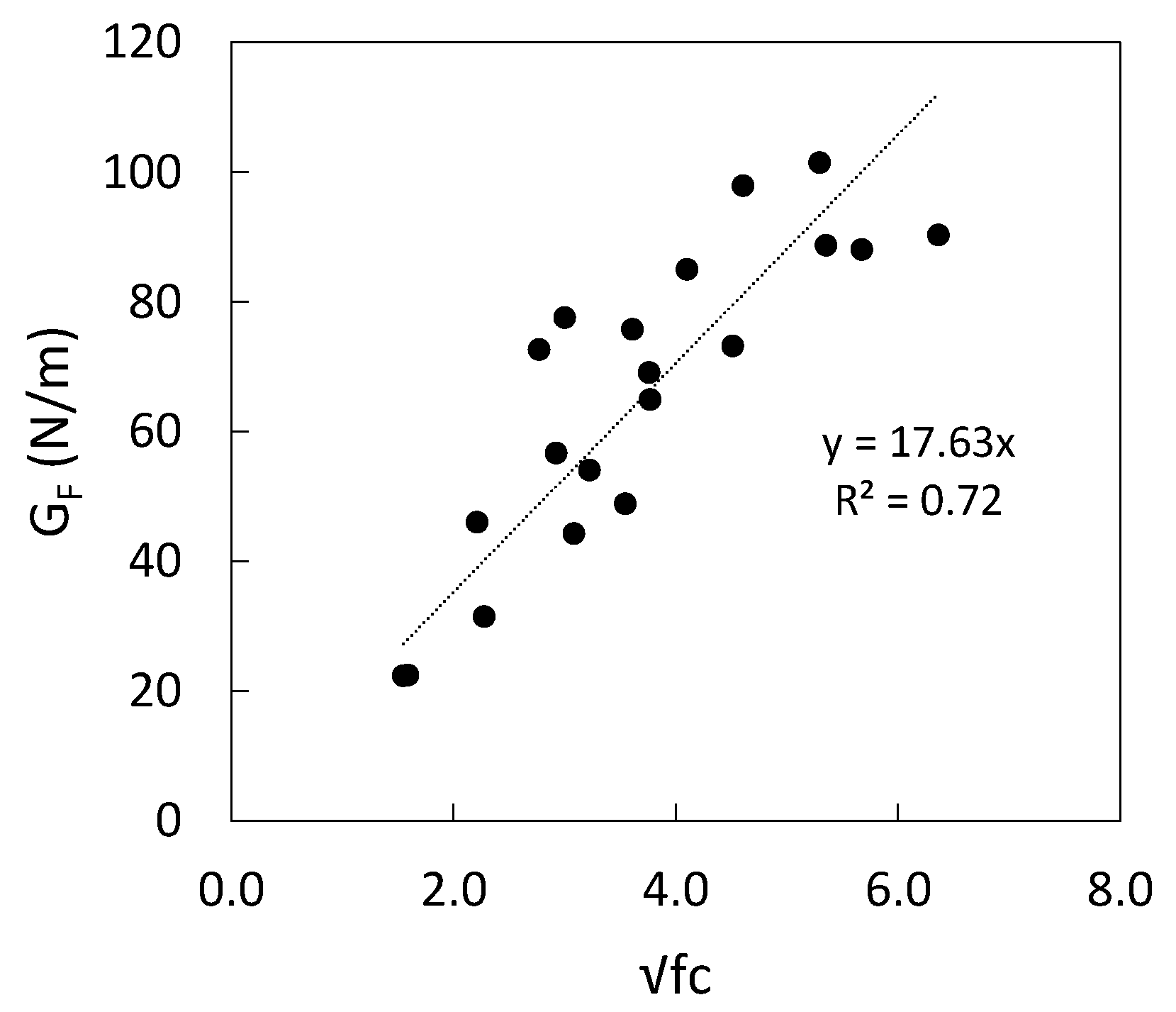





| Mix ID | Grade | GP Cement (kg) | Aggregates (kg) | Manufactured Sand (kg) | Sand (kg) | Nominal Water (lt) | Water Reducing Agent (ml) | w/c |
|---|---|---|---|---|---|---|---|---|
| A | N20 | 217 | 925 | 195 | 787 | 179 | 760 | 0.82 |
| B | N25 | 255 | 938 | 188 | 756 | 174 | 893 | 0.68 |
| C | N32 | 325 | 967 | 129 | 738 | 175 | 1138 | 0.54 |
| D | N40 | 443 | 956 | 36 | 697 | 183 | 1551 | 0.41 |
| Age | fcm (MPa) | COV | fsp (MPa) | COV | GF (N/m) | COV | Ec (MPa) | COV | ν |
|---|---|---|---|---|---|---|---|---|---|
| 18 h | 2.5 | 1.9% | 0.40 | 3.6% | 24 | / | 6669 | 7.0% | 0.15 |
| 30 h | 5.2 | 3.3% | 0.70 | 5.6% | 34 | / | 11,924 | 7.9% | 0.17 |
| 48 h | 7.7 | 2.6% | 1.15 | 2.5% | 41 | / | 15,277 | 6.1% | 0.18 |
| 72 h | 9.5 | 1.2% | 1.47 | 14.6% | 49 | / | 15,510 | 11.1% | 0.16 |
| 7 d | 12.6 | 11.6% | 1.97 | 3.7% | 54 | / | 17,567 | / | 0.17 |
| 14 d | 15.5 | 1.5% | 2.15 | 6.5% | 80 | / | 18,797 | 1.2% | 0.19 |
| 28 d | 15.4 | 3.9% | 2.02 | 4.2% | 108 | 7.5% | 20,420 | 3.7% | 0.19 |
| Age | fcm (MPa) | COV | fsp (MPa) | COV | GF (N/m) | COV | Ec (MPa) | COV | ν |
|---|---|---|---|---|---|---|---|---|---|
| 18 h | 2.40 | 5.0% | 0.32 | 3.2% | 26 | 13.3% | 6947 | 14.2% | 0.16 |
| 30 h | 4.89 | 3.1% | 0.63 | 9.6% | 49 | 18.5% | 10,188 | 6.1% | 0.21 |
| 48 h | 8.56 | 0.5% | 1.16 | 2.3% | 60 | / | 12,132 | 4.6% | 0.17 |
| 72 h | 10.39 | 1.8% | 1.48 | 4.9% | 61 | / | 13,878 | 9.3% | 0.18 |
| 7 d | 14.20 | 3.2% | 1.97 | 3.3% | 74 | 4.2% | 15,650 | 6.7% | 0.18 |
| 14 d | 17.81 | 5.6% | 2.34 | 9.4% | 100 | / | 15,664 | 2.6% | 0.18 |
| 28 d | 20.89 | 1.9% | 2.61 | 8.3% | 118 | 13.6% | 18,639 | 2.0% | 0.21 |
| Age | fcm (MPa) | COV | fsp (MPa) | COV | GF (N/m) | COV | Ec (MPa) | COV | ν |
|---|---|---|---|---|---|---|---|---|---|
| 18 h | 9.00 | 7.3% | 1.53 | 6.2% | 83 | 20.8% | 13,811 | 7.2% | 0.22 |
| 30 h | 13.04 | 5.2% | 1.82 | 6.5% | 83 | 12.4% | 16,953 | 2.2% | 0.20 |
| 48 h | 16.82 | 7.3% | 2.54 | 1.7% | 94 | 27.1% | 20,985 | 3.4% | 0.22 |
| 72 h | 21.20 | 3.4% | 2.80 | 7.2% | 109 | / | 23,249 | 10.6% | 0.23 |
| 7 d | 28.02 | 9.1% | 3.52 | 0.4% | 117 | 16.0% | 22,650 | 5.9% | 0.20 |
| 14 d | 33.76 | 4.8% | 3.62 | 7.0% | 119 | 4.3% | 27,301 | 5.6% | 0.24 |
| 28 d | 40.14 | 1.0% | 3.90 | 8.5% | 121 | / | 26,768 | 1.8% | 0.23 |
| Age | fcm (MPa) | COV | fsp (MPa) | COV | GF (N/m) | COV | Ec (MPa) | COV | ν |
|---|---|---|---|---|---|---|---|---|---|
| 18 h | 14.14 | 7.3% | 1.97 | 6.0% | 78 | / | 16,987 | 11.3% | 0.18 |
| 30 h | 20.37 | 5.9% | 2.40 | 9.7% | 77 | 15.7% | 20,393 | 3.0% | 0.18 |
| 48 h | 28.64 | 1.2% | 3.09 | 9.7% | 90 | / | 25,786 | 6.4% | 0.19 |
| 72 h | 32.19 | 7.8% | 3.57 | 7.7% | 89 | 18.5% | 26,998 | 4.6% | 0.19 |
| 7 d | 40.48 | 3.9% | 3.79 | 2.3% | 103 | 8.0% | 27,561 | 3.2% | 0.20 |
| 14 d | 46.46 | 2.0% | 4.21 | 3.3% | 119 | / | 29,834 | 1.5% | 0.21 |
| 28 d | 49.26 | 4.8% | 4.57 | 3.6% | 114 | 11.2% | 31,853 | 21.0% | 0.22 |
| Reference | Model | |
|---|---|---|
| [17] | Ec(t) = (fc(t) ⁄ fc(28))0.3·Ec(28) | (9) |
| [14] | Ec(t) = βE(t)·Ec(28) | (10) |
| βE(t) = [βcc (t)]0.5 | (11) | |
| βcc (t) = exp{0.25[1 − (28/t)(1/2)]} | (12) |
| Reference | Model |
|---|---|
| [25] | fsp = 0.56(fc)0.5 |
| [26] | fsp = 0.294(fc)0.69 |
| [27] | fsp = 0.2(fc)0.8 |
| [29] | fsp = 0.272(fc)0.71 |
| [14] | fsp = 0.3(fc)2/3 |
| [30] | fsp = 0.313(fc)2/3 |
| [31] | fsp = 0.47(fc)0.59 |
| Age | MIX A | MIX B | MIX C | MIX D | ||||
|---|---|---|---|---|---|---|---|---|
| ft inv (MPa) | ft inv/fsp | ft inv (MPa) | ft inv/fsp | ft inv (MPa) | ft inv/fsp | ft inv (MPa) | ft inv/fsp | |
| 18 h | 0.27 | 0.7 | 0.35 | 1.2 | 0.93 | 0.6 | 1.44 | 0.8 |
| 30 h | 0.48 | 0.7 | 0.57 | 1.0 | 1.54 | 0.9 | 1.66 | 0.7 |
| 48 h | 0.75 | 0.7 | 0.83 | 0.8 | 1.86 | 0.8 | 1.83 | 0.6 |
| 72 h | 0.60 | 0.4 | 1.00 | 0.7 | 2.07 | 0.8 | 1.98 | 0.6 |
| 7 d | 0.87 | 0.5 | 1.32 | 0.7 | 2.26 | 0.7 | 2.16 | 0.6 |
| 14 d | 0.95 | 0.5 | 1.47 | 0.7 | 2.52 | 0.7 | 2.11 | 0.5 |
| 28 d | 1.25 | 0.7 | 1.49 | 0.6 | 2.70 | 0.7 | 2.51 | 0.6 |
| Age | Mix A | Mix B | Mix C | Mix D | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GF N/m | Gf N/m | GF/Gf | GF N/m | Gf N/m | GF/Gf | GF N/m | Gf N/m | GF/Gf | GF N/m | Gf N/m | GF/Gf | |
| 18 h | 24 | 7 | 3.36 | 26 | 6 | 4.04 | 83 | 21 | 4.02 | 78 | 41 | 1.91 |
| 30 h | 34 | 9 | 3.59 | 49 | 15 | 3.22 | 83 | 29 | 2.90 | 77 | 37 | 2.09 |
| 48 h | 41 | 13 | 3.08 | 60 | 18 | 3.38 | 94 | 31 | 3.03 | 90 | 47 | 1.93 |
| 72 h | 49 | 17 | 2.80 | 61 | 20 | 3.00 | 109 | 50 | 2.18 | 89 | 44 | 2.04 |
| 7 d | 54 | 54 | 1.00 | 74 | 29 | 2.58 | 117 | 52 | 2.27 | 103 | 54 | 1.91 |
| 14 d | 80 | 30 | 2.64 | 100 | 35 | 2.84 | 119 | 57 | 2.08 | 119 | 57 | 2.10 |
| 28 d | 108 | 47 | 2.32 | 118 | 43 | 2.74 | 121 | 64 | 1.90 | 114 | 62 | 1.82 |
| Model | Reference |
|---|---|
| GF = 43.2 + 1.13 fcu | [34] |
| GF = Gfo (fcm/10)0.7 | * [35] |
| GF = 73fc0.18 | [14] |
| GF = 2.5 ∝0 (f’c/0.051)0.46 (1 + da/11.27) 0.22 (w/c)−0.30 | ** [33] |
| Specimen | Age (Hours) | fcm (MPa) | ft (MPa) | E (MPa) | Gf (N/m) |
|---|---|---|---|---|---|
| A | 43 | 7 | 0.72 | 11,941 | 25.85 |
| B | 91.5 | 11.2 | 1.0 | 14,292 | 35.8 |
| C | 166 | 14.2 | 1.17 | 15,400 | 42.15 |
| D | 334 | 17.8 | 1.37 | 16,610 | 49.25 |
| Label | Nu.test (kN) | δtest (mm) | Nu.FE (kN) | δFE (mm) | Nu.FE/Nu.test | δFE/δtest | Failure Mode |
|---|---|---|---|---|---|---|---|
| A | 11.78 | 0.64 | 12.6 | 0.41 | 1.07 | 0.64 | Plug (P-O) |
| B | 14.42 | 0.55 | 13.7 | 0.28 | 0.95 | 0.51 | Plug (P-O) |
| C | 16.14 | 0.54 | 17.9 | 0.39 | 1.11 | 0.72 | Plug (P-O) |
| D | 22.01 | 0.73 | 25.1 | 0.53 | 1.14 | 0.73 | Plug (P-O) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Obayes, O.; Gad, E.; Pokharel, T.; Lee, J.; Abdouka, K. Evaluation of Concrete Material Properties at Early Age. CivilEng 2020, 1, 326-350. https://doi.org/10.3390/civileng1030021
Obayes O, Gad E, Pokharel T, Lee J, Abdouka K. Evaluation of Concrete Material Properties at Early Age. CivilEng. 2020; 1(3):326-350. https://doi.org/10.3390/civileng1030021
Chicago/Turabian StyleObayes, Osamah, Emad Gad, Tilak Pokharel, Jessey Lee, and Kamiran Abdouka. 2020. "Evaluation of Concrete Material Properties at Early Age" CivilEng 1, no. 3: 326-350. https://doi.org/10.3390/civileng1030021
APA StyleObayes, O., Gad, E., Pokharel, T., Lee, J., & Abdouka, K. (2020). Evaluation of Concrete Material Properties at Early Age. CivilEng, 1(3), 326-350. https://doi.org/10.3390/civileng1030021







