Design and Analysis of Slotted Waveguide Antenna Radiating in a “Plasma-Shaped” Cavity of an ECR Ion Source
Abstract
1. Introduction and Motivation
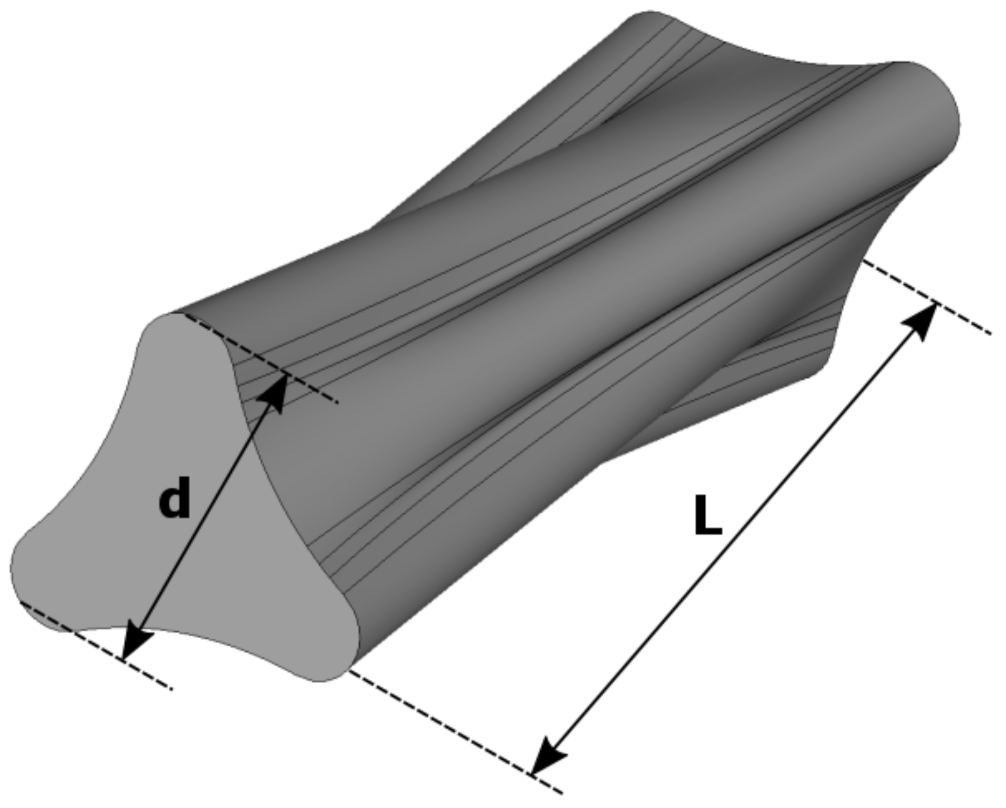
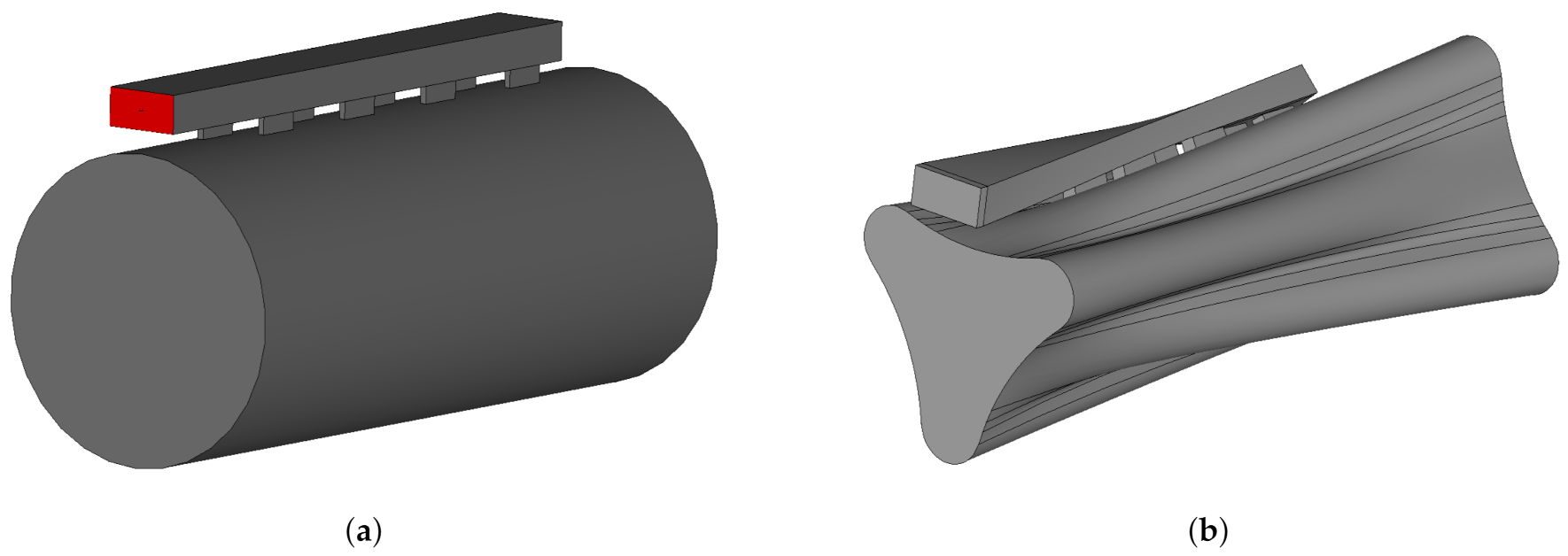
2. Plasma Shaped Cavity and Axial Microwave Injection
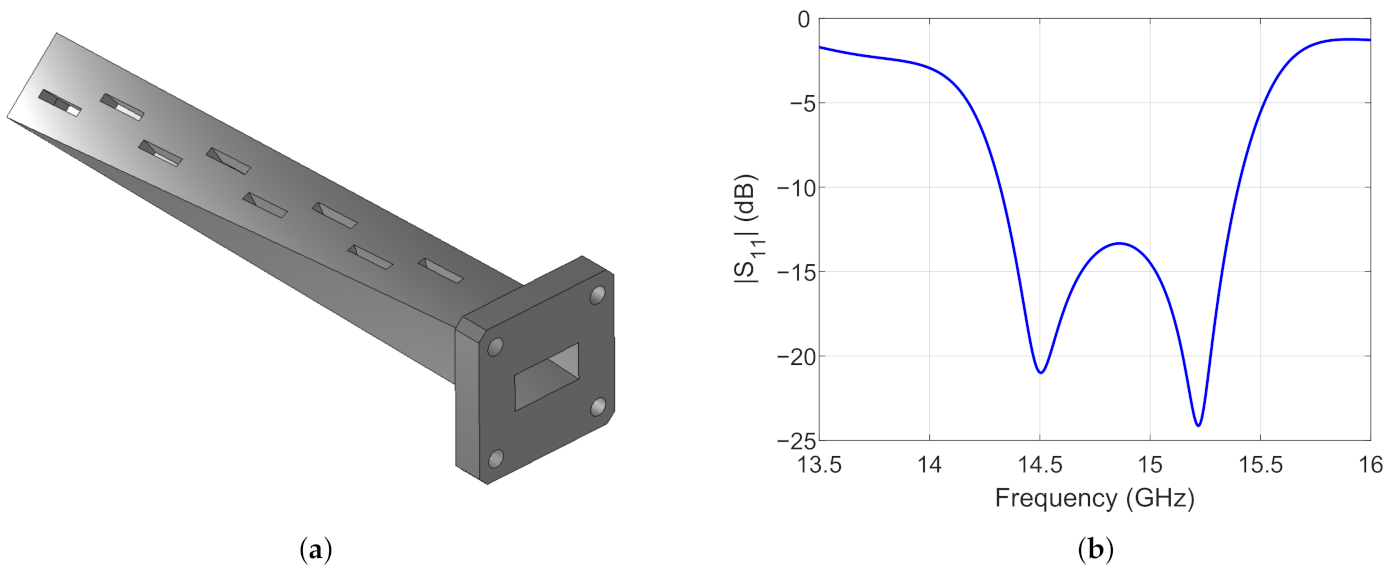
3. Slotted Waveguide Antenna Design
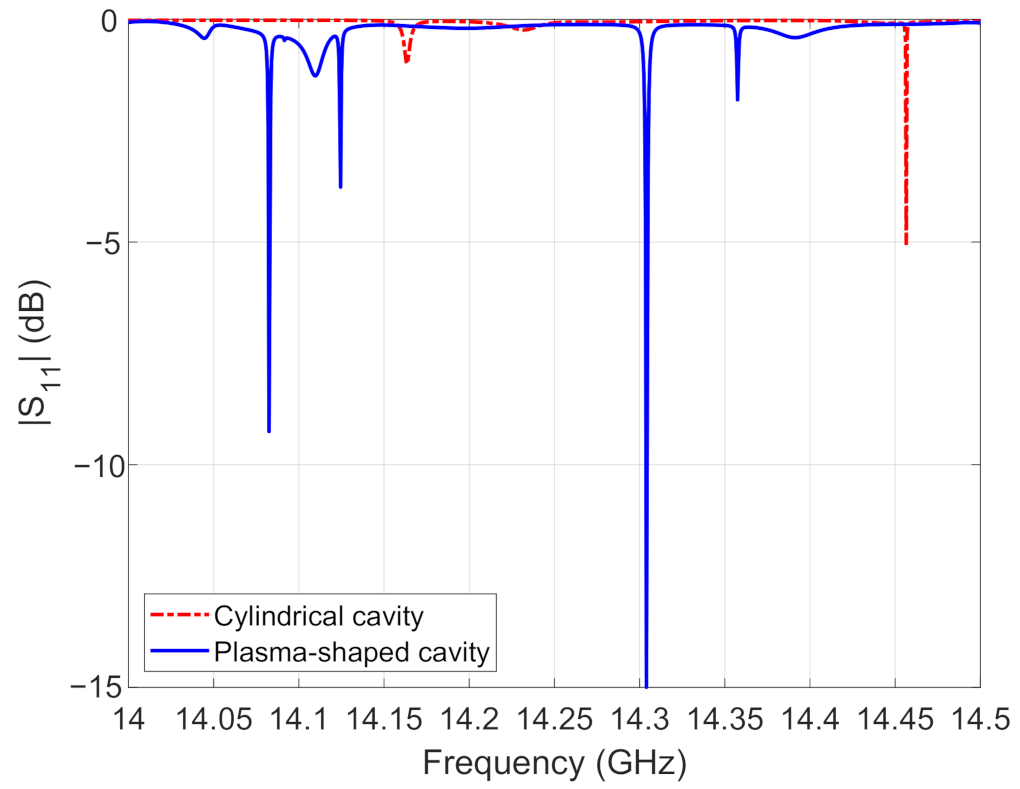
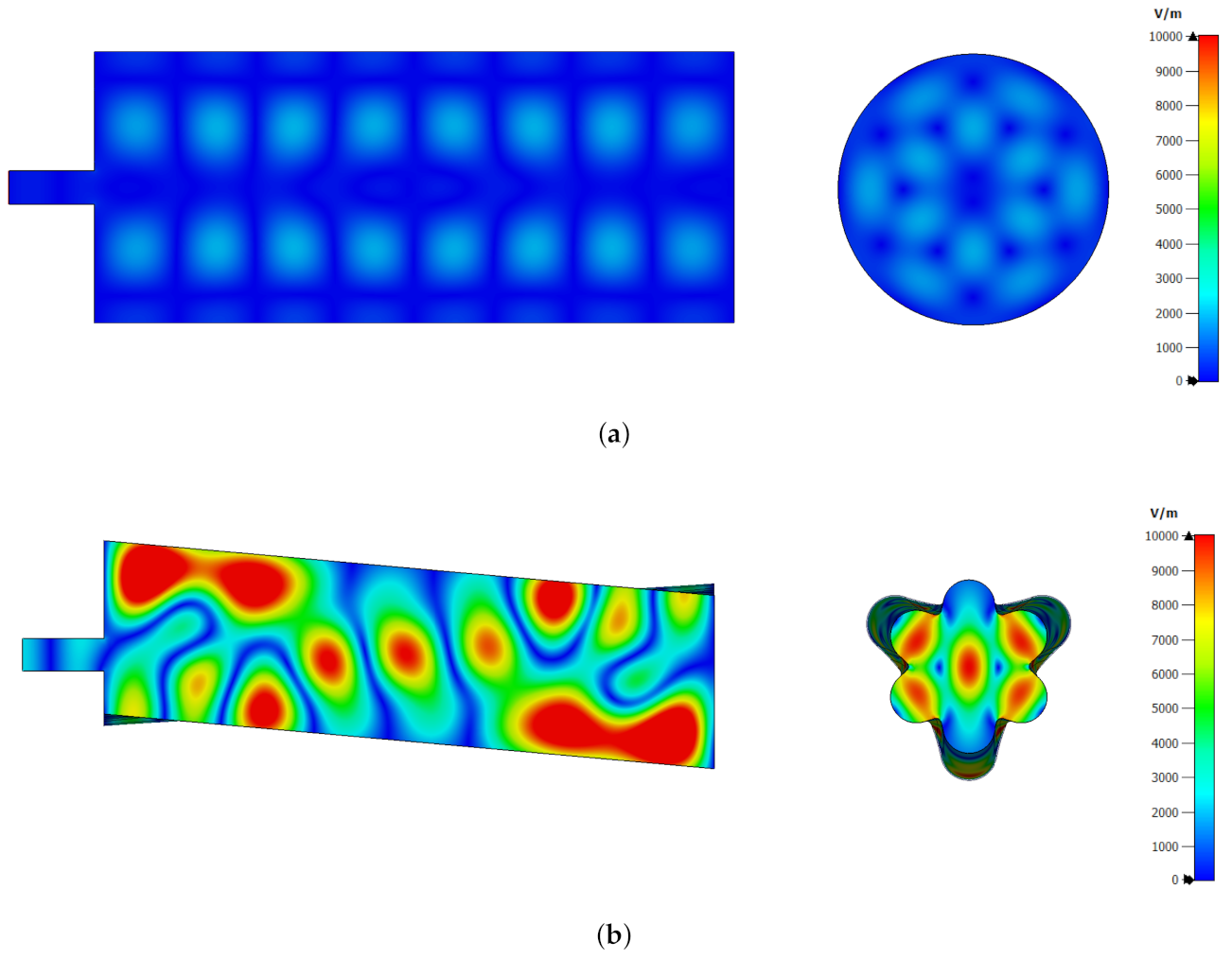
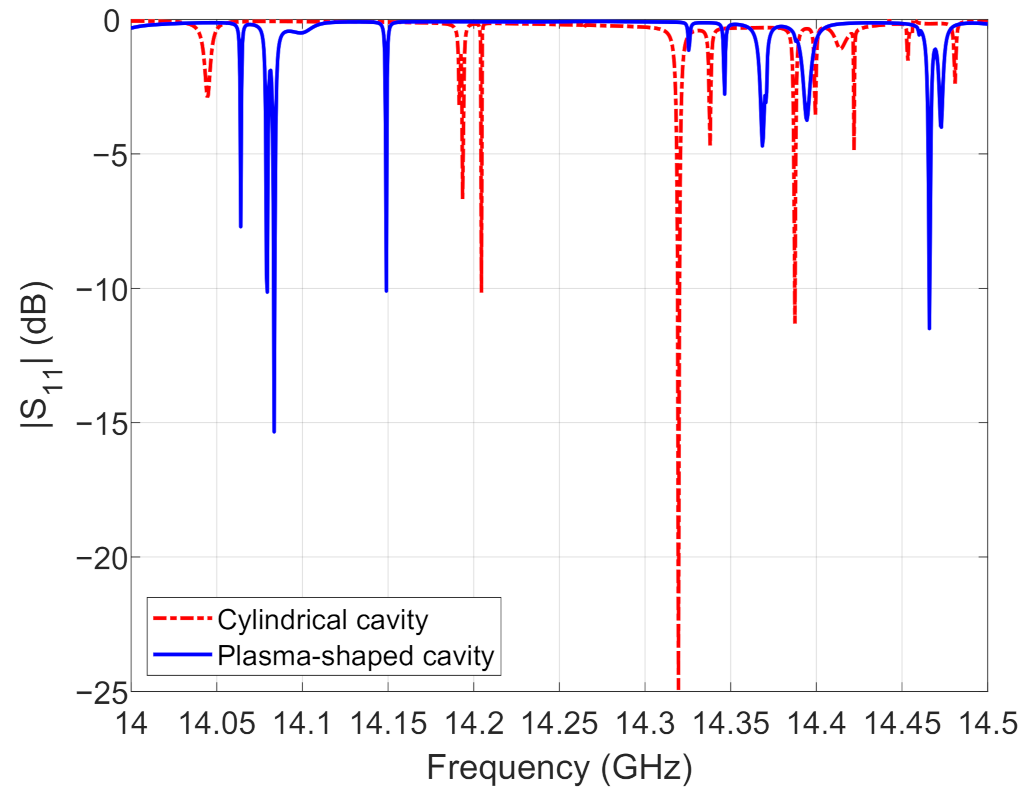
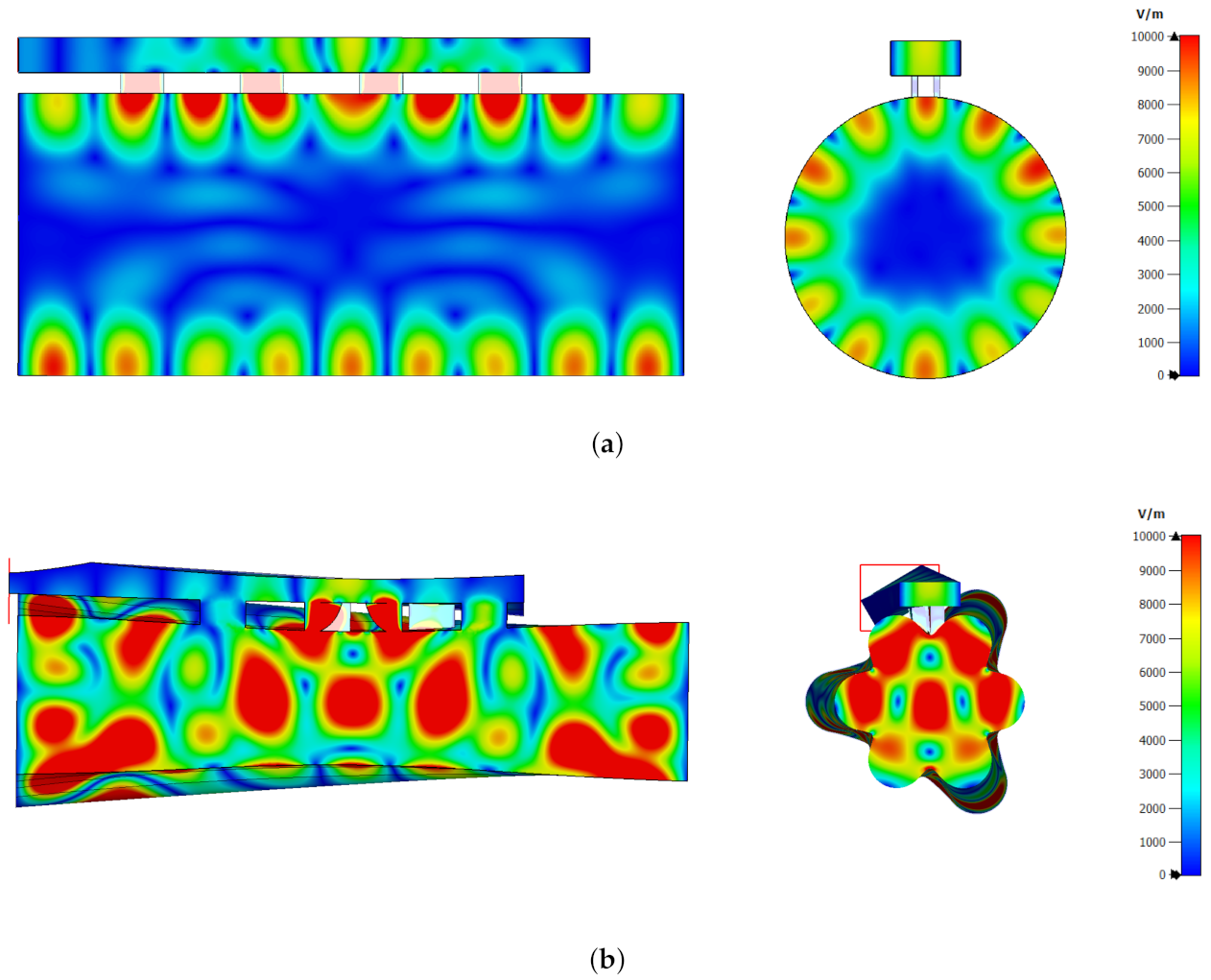
4. Slotted Waveguide Injection Simulations
5. Conclusions and Perspectives
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mascali, D.; Neri, L.; Gammino, S.; Celona, L.; Ciavola, G.; Gambino, N.; Miracoli, R.; Chikin, S. Plasma ion dynamics and beam formation in electron cyclotron resonance ion sources. Rev. Sci. Instrum. 2010, 81. [Google Scholar] [CrossRef] [PubMed]
- Gammino, S.; Ciavola, G.; Celona, L.G.; Mascali, D.; Maimone, F. Numerical simulations of the ECR heating with waves of different frequency in electron cyclotron resonance ion sources. IEEE Trans. Plasma Sci. 2008, 36, 1552–1568. [Google Scholar] [CrossRef]
- Koivisto, H.; Ikonen, A.; Kalvas, T.; Kosonen, S.; Kronholm, R.; Marttinen, M.; Tarvainen, O.; Toivanen, V. A new 18 GHz room temperature electron cyclotron resonance ion source for highly charged ion beams. Rev. Sci. Instrum. 2020, 91, 023303. [Google Scholar] [CrossRef] [PubMed]
- Hitz, D.; Girard, A.; Melin, G.; Gammino, S.; Ciavola, G.; Celona, L. Results and interpretation of high frequency experiments at 28 GHz in ECR ion sources, future prospects. Rev. Sci. Instrum. 2002, 73, 509–512. [Google Scholar] [CrossRef]
- Zhao, H.W.; Sun, L.T.; Guo, J.W.; Lu, W.; Xie, D.Z.; Hitz, D.; Zhang, X.Z.; Yang, Y. Intense highly charged ion beam production and operation with a superconducting electron cyclotron resonance ion source. Phys. Rev. Accel. Beams 2017, 20, 094801. [Google Scholar] [CrossRef]
- Xie, D.; Benitez, J.; Lu, W.; Lyneis, C.; Todd, D. Recent production of intense high charge ion beams with VENUS. In Proceedings of the 22nd International Workshop on ECR Ion Sources, Busan, Korea, 28 August 2017; p. THAO01. [Google Scholar] [CrossRef]
- Guo, J.W.; Sun, L.; Lu, W.; Zhang, W.H.; Feng, Y.C.; Shen, Z.; Li, L.X.; Li, J.B.; Zhang, X.Z.; Hitz, D.; Zhao, H.W. A new microwave coupling scheme for high intensity highly charged ion beam production by high power 24–28 GHz SECRAL ion source. Rev. Sci. Instrum. 2020, 91, 013322. [Google Scholar] [CrossRef] [PubMed]
- Galatà, A.; Mascali, D.; Gallo, C.S.; Torrisi, G. Self-consistent modeling of beam-plasma interaction in the charge breeding optimization process. Rev. Sci. Instrum. 2020, 91, 013506. [Google Scholar] [CrossRef] [PubMed]
- Someda, C. Electromagnetic Waves, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar] [CrossRef]
- Mascali, D.; Ando’, L.; Castro, G.; Celona, L.; Neri, L.; Gammino, S.; Romano, F.P.; Altana, C.; Caliri, C.; Sorbello, G.; Torrisi, G. Ecr Ion Sources Developments at Infn-Lns for the Production of High Brightness Highly Charged Ion Beams. Proceedings of LINAC2014, Geneva, Switzerland, 31 August–5 September 2014; pp. 254–256. [Google Scholar]
- Torrisi, G.; Mascali, D.; Sorbello, G.; Castro, G.; Celona, L.; Galatà, A.; Leonardi, O.; Naselli, E.; Gammino, S. Non-conventional microwave coupling of RF power in ECRIS plasmas. AIP Conf. Proc. 2018, 2011, 020014. [Google Scholar] [CrossRef]
- Mauro, G.S.; Torrisi, G.; Leonardi, O.; Galatà, A.; Gallo, C.S.; Sorbello, G.; Mascali, D. Slotted Antenna Waveguide for Microwave Injection in Ion Sources. In Proceedings of the 2020 XXXIIIrd General Assembly and Scientific Symposium of the International Union of Radio Science, Rome, Italy, 29 August–5 September 2020; pp. 1–4. [Google Scholar] [CrossRef]
- Werner, F.; Korzec, D.; Engemann, J. Slot antenna 2.45 GHz microwave plasma source. Plasma Sources Sci. Technol. 1994, 3, 473–481. [Google Scholar] [CrossRef]
- Woodard, A.; Shojaei, K.; Berrospe-Rodriguez, C.; Nava, G.; Mangolini, L. Electron emission from particles strongly affects the electron energy distribution in dusty plasmas. J. Vac. Sci. Technol. A 2020, 38. [Google Scholar] [CrossRef]
- Gatti, R.V.; Sorrentino, R. Slotted waveguide antennas with arbitrary radiation pattern. In Proceedings of the 34th European Microwave Conference, Amsterdam, The Netherlands, 12–14 October 2004; pp. 821–824. [Google Scholar]
- Elliott, R.S.; Kurtz, L.A. The design of small slot arrays. IEEE Trans. Antennas Propagat. 1978, 26, 214–219. [Google Scholar] [CrossRef]
- Elliott, R.S. The design of traveling wave fed longitudinal shunt slot arrays. IEEE Trans. Antennas Propagat. 1979, 27, 717–720. [Google Scholar] [CrossRef]
- Sekretarov, S.S.; Vavriv, D.M. A Wideband Slotted Waveguide Antenna Array for Sar Systems. Prog. Electromagn. Res. M 2010, 11, 165–176. [Google Scholar] [CrossRef]
- Celona, L.; Gammino, S.; Ciavola, G.; Maimone, F.; Mascali, D. Microwave to plasma coupling in electron cyclotron resonance and microwave ion sources. Rev. Sci. Instr. 2010, 81, 02A333. [Google Scholar] [CrossRef] [PubMed]
- Bernal, S.; Vega, F.; Roman, F.; Valero, A. A high-gain, broad-wall slotted waveguide antenna array to be used as part of a narrowband high power microwaves system. In Proceedings of the 2015 International Conference on Electromagnetics in Advanced Applications (ICEAA), Turin, Italy, 7–11 September 2015; pp. 618–621. [Google Scholar]
- Mascali, D.; Torrisi, G.; Neri, L.; Sorbello, G.; Castro, G.; Celona, L.; Gammino, S. 3D-full wave and kinetics numerical modelling of electron cyclotron resonance ion sources plasma: steps towards self-consistency. Eur. Phys. J. D 2015, 69, 27. [Google Scholar] [CrossRef]
- Torrisi, G.; Mascali, D.; Sorbello, G.; Neri, L.; Celona, L.; Castro, G.; Isernia, T.; Gammino, S. Full-wave FEM simulations of electromagnetic waves in strongly magnetized non-homogeneous plasma. J. Electromagn. Waves Appl. 2014, 28, 1085–1099. [Google Scholar] [CrossRef]

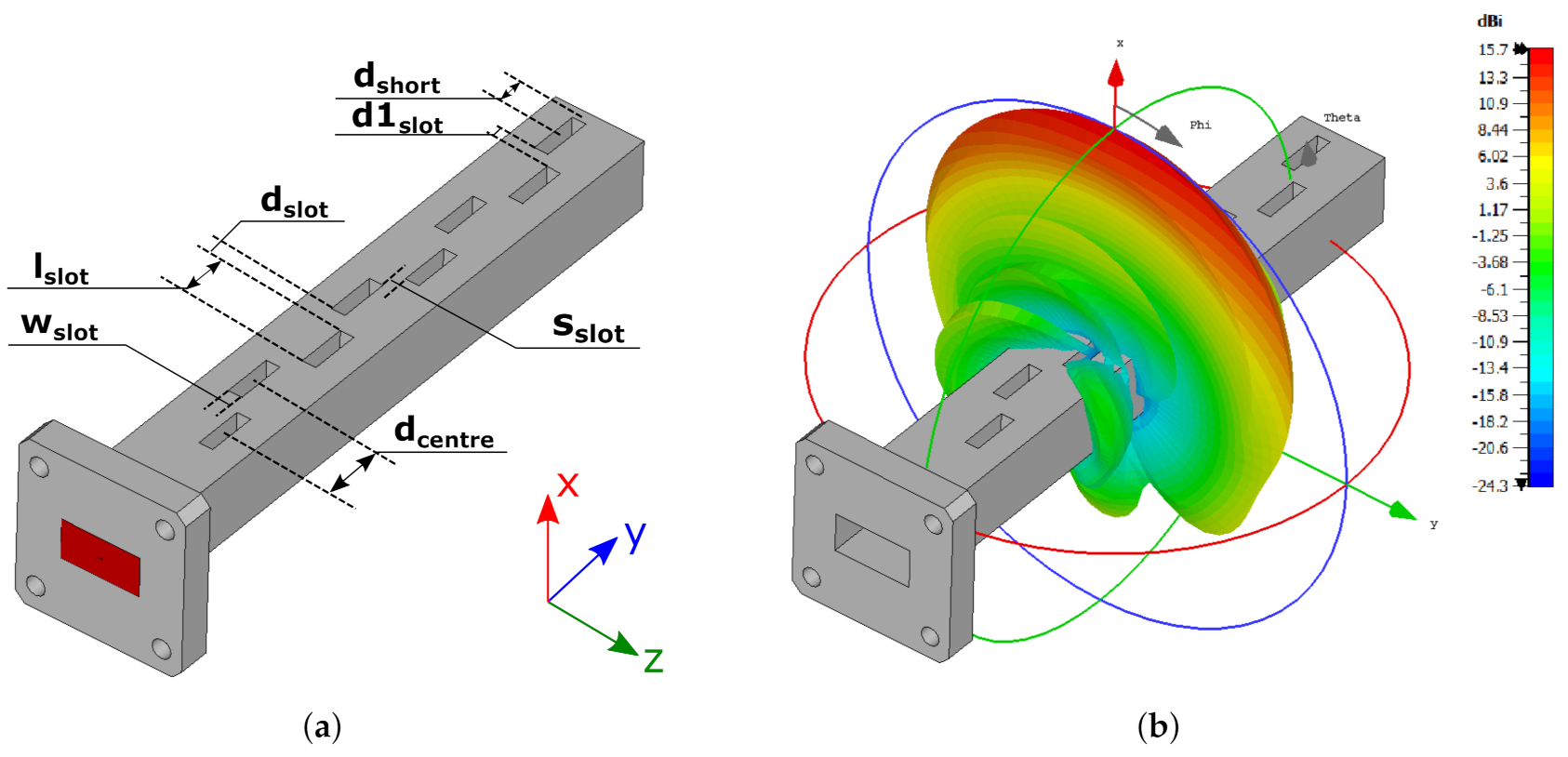

| Parameter | Value [mm] | Description |
|---|---|---|
| l | 9.92 | Slot length |
| w | 1.75 | Slot width |
| d | 13.45 | Slot centers distances |
| d | 3.53 | Longitudinal distance between slots |
| d1 | 2.53 | Last two slots distance |
| s | 2.75 | Transversal distance between slots |
| d | 6.455 | Last slot center to short wall distance |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mauro, G.S.; Torrisi, G.; Leonardi, O.; Pidatella, A.; Sorbello, G.; Mascali, D. Design and Analysis of Slotted Waveguide Antenna Radiating in a “Plasma-Shaped” Cavity of an ECR Ion Source. Telecom 2021, 2, 42-51. https://doi.org/10.3390/telecom2010004
Mauro GS, Torrisi G, Leonardi O, Pidatella A, Sorbello G, Mascali D. Design and Analysis of Slotted Waveguide Antenna Radiating in a “Plasma-Shaped” Cavity of an ECR Ion Source. Telecom. 2021; 2(1):42-51. https://doi.org/10.3390/telecom2010004
Chicago/Turabian StyleMauro, Giorgio Sebastiano, Giuseppe Torrisi, Ornella Leonardi, Angelo Pidatella, Gino Sorbello, and David Mascali. 2021. "Design and Analysis of Slotted Waveguide Antenna Radiating in a “Plasma-Shaped” Cavity of an ECR Ion Source" Telecom 2, no. 1: 42-51. https://doi.org/10.3390/telecom2010004
APA StyleMauro, G. S., Torrisi, G., Leonardi, O., Pidatella, A., Sorbello, G., & Mascali, D. (2021). Design and Analysis of Slotted Waveguide Antenna Radiating in a “Plasma-Shaped” Cavity of an ECR Ion Source. Telecom, 2(1), 42-51. https://doi.org/10.3390/telecom2010004








