Insulator Metal Transition-Based Selector in Crossbar Memory Arrays
Abstract
1. Introduction
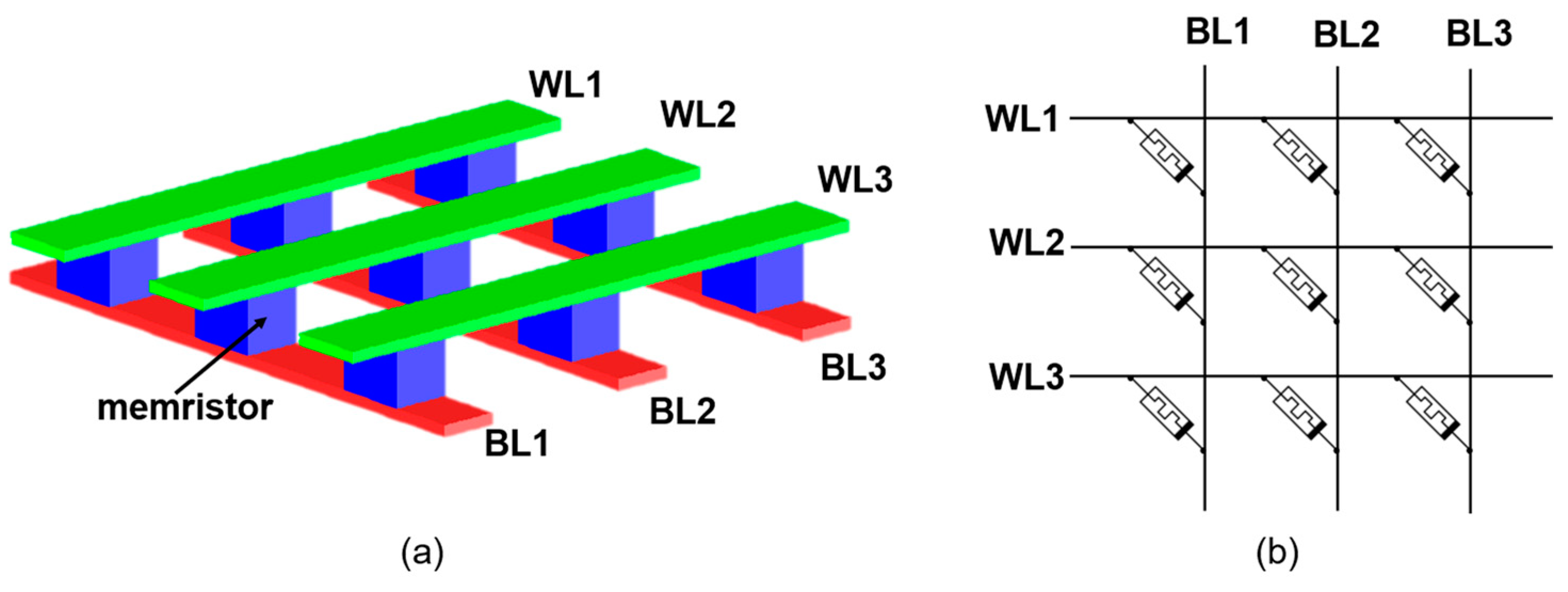
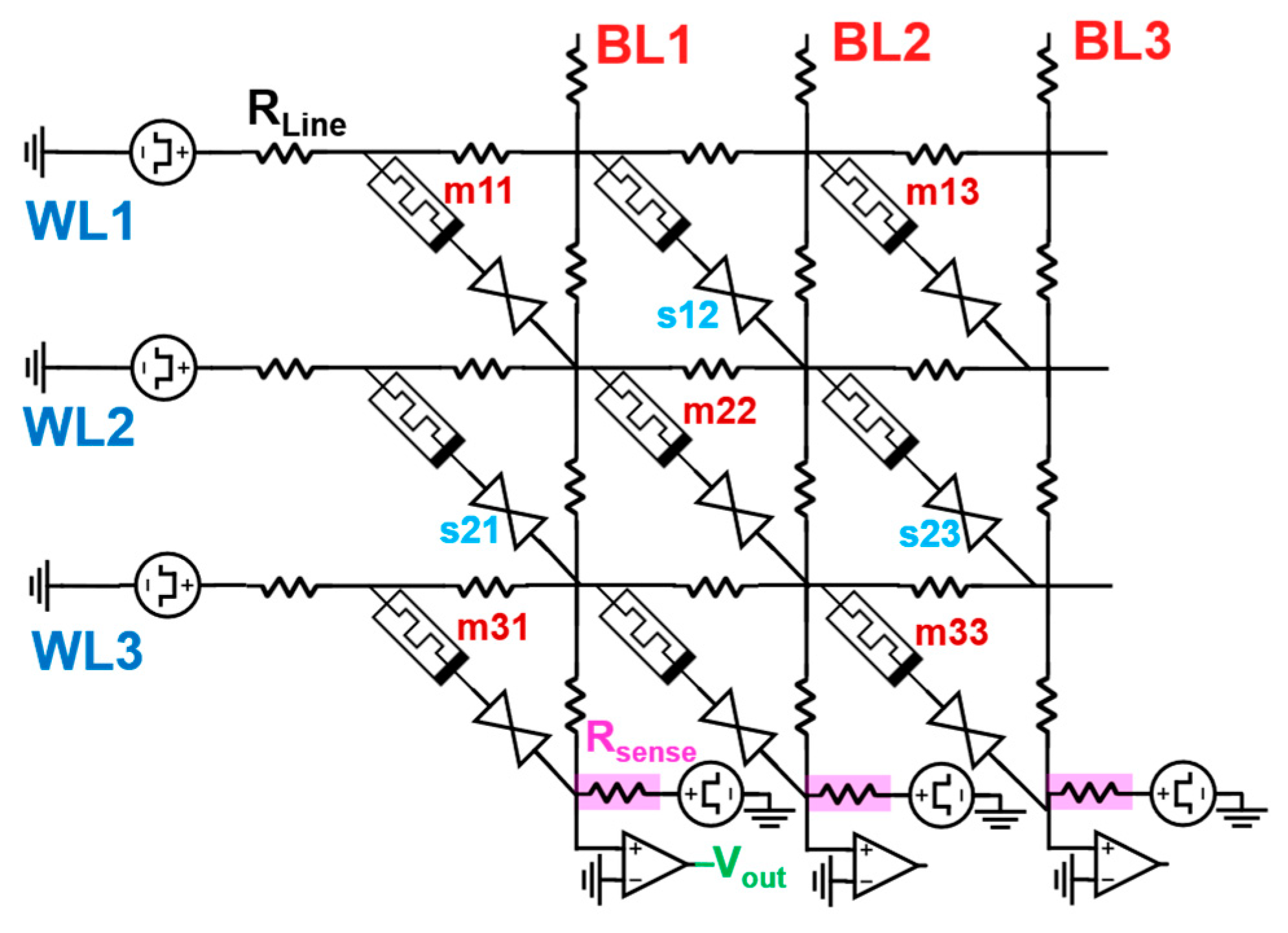
1.1. Crossbar Memory Arrays
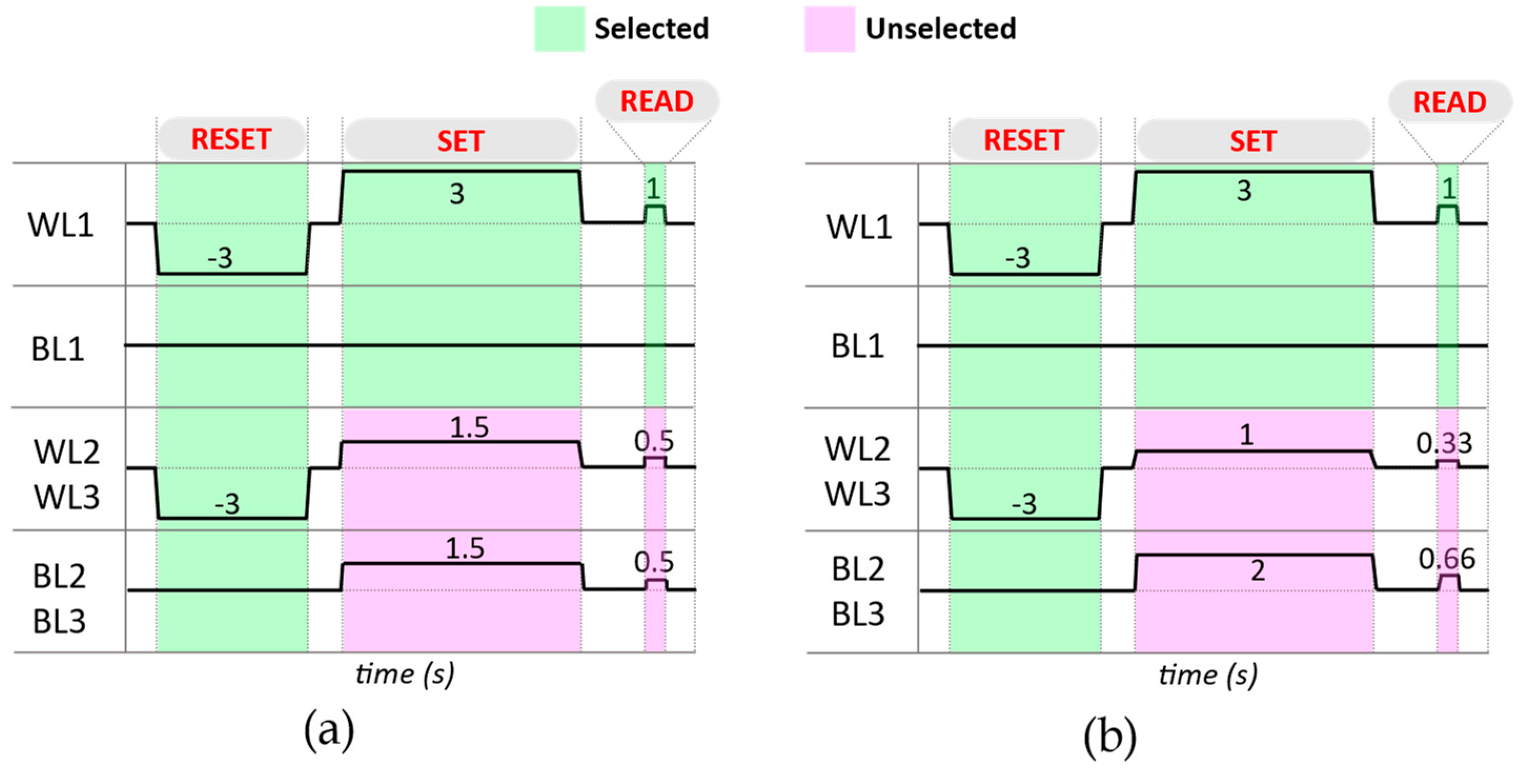
1.2. WRITE and READ Operations
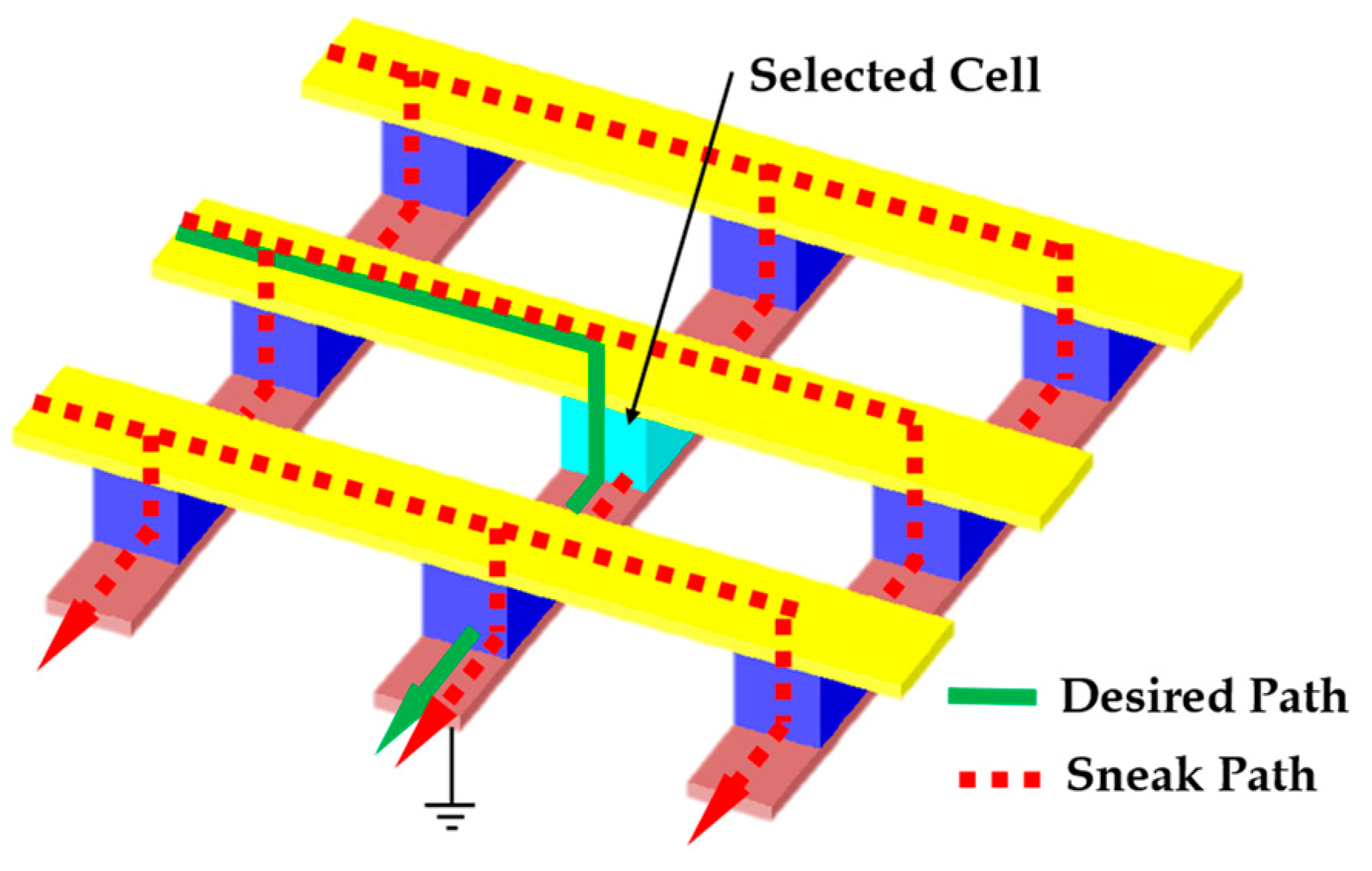
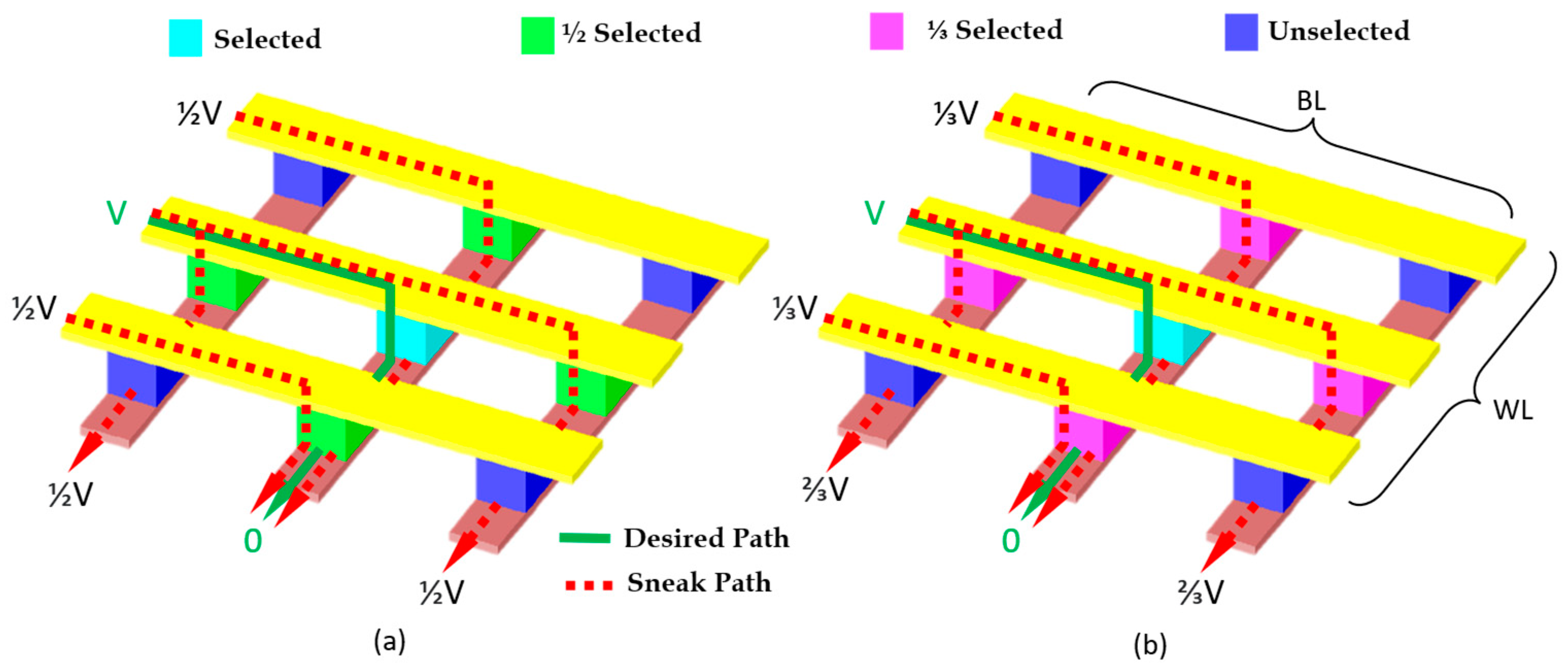
1.3. Challenges
2. Selectors in ReRAM Arrays
2.1. 1T1R
2.2. 1S1R
2.3. IMT Material as a Selector
3. Simulation Models
3.1. IMT Model
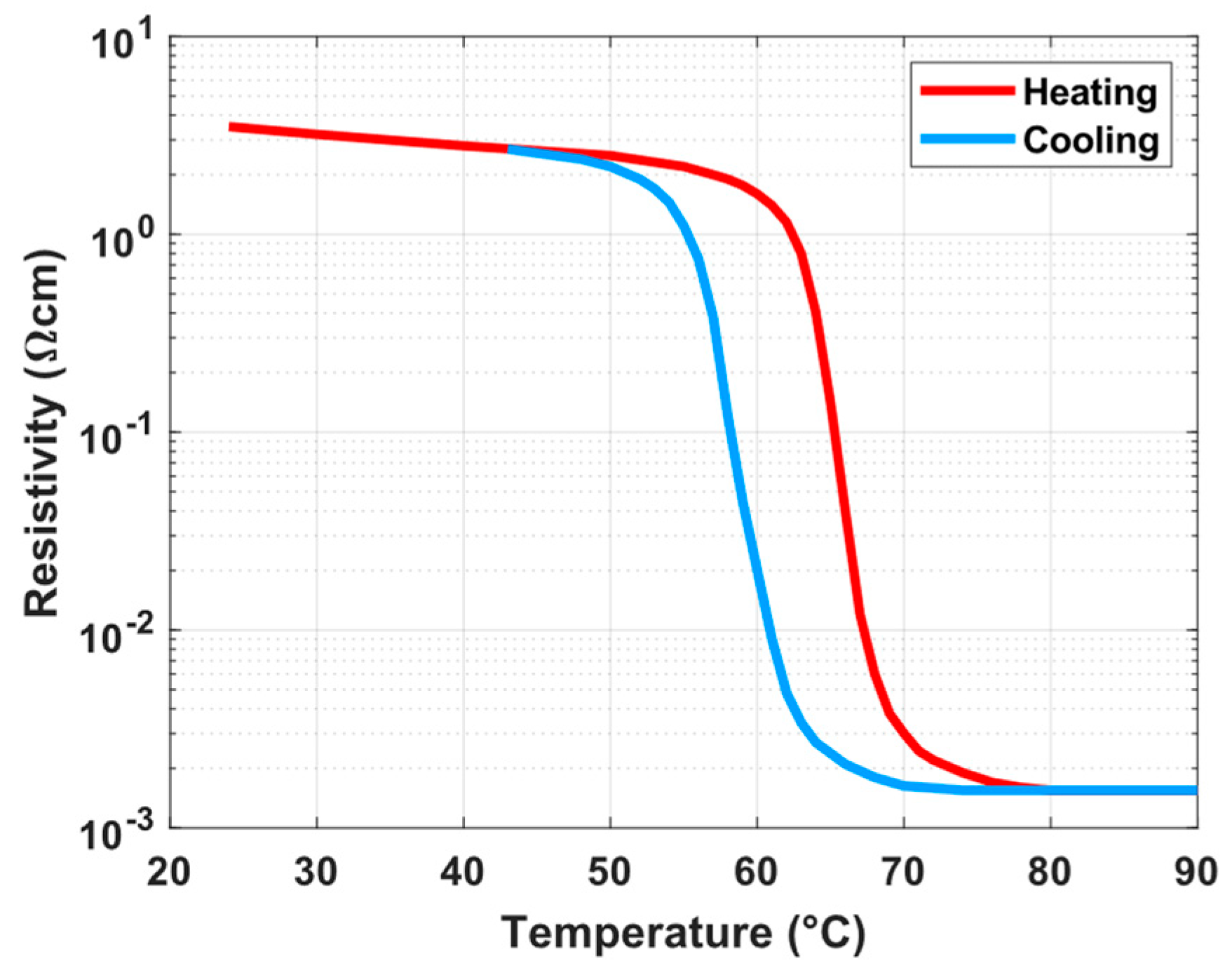
3.1.1. Temperature Evolving
3.1.2. Resistance Change with Temperature
3.1.3. Simulation Model Code
3.2. Memristor Model
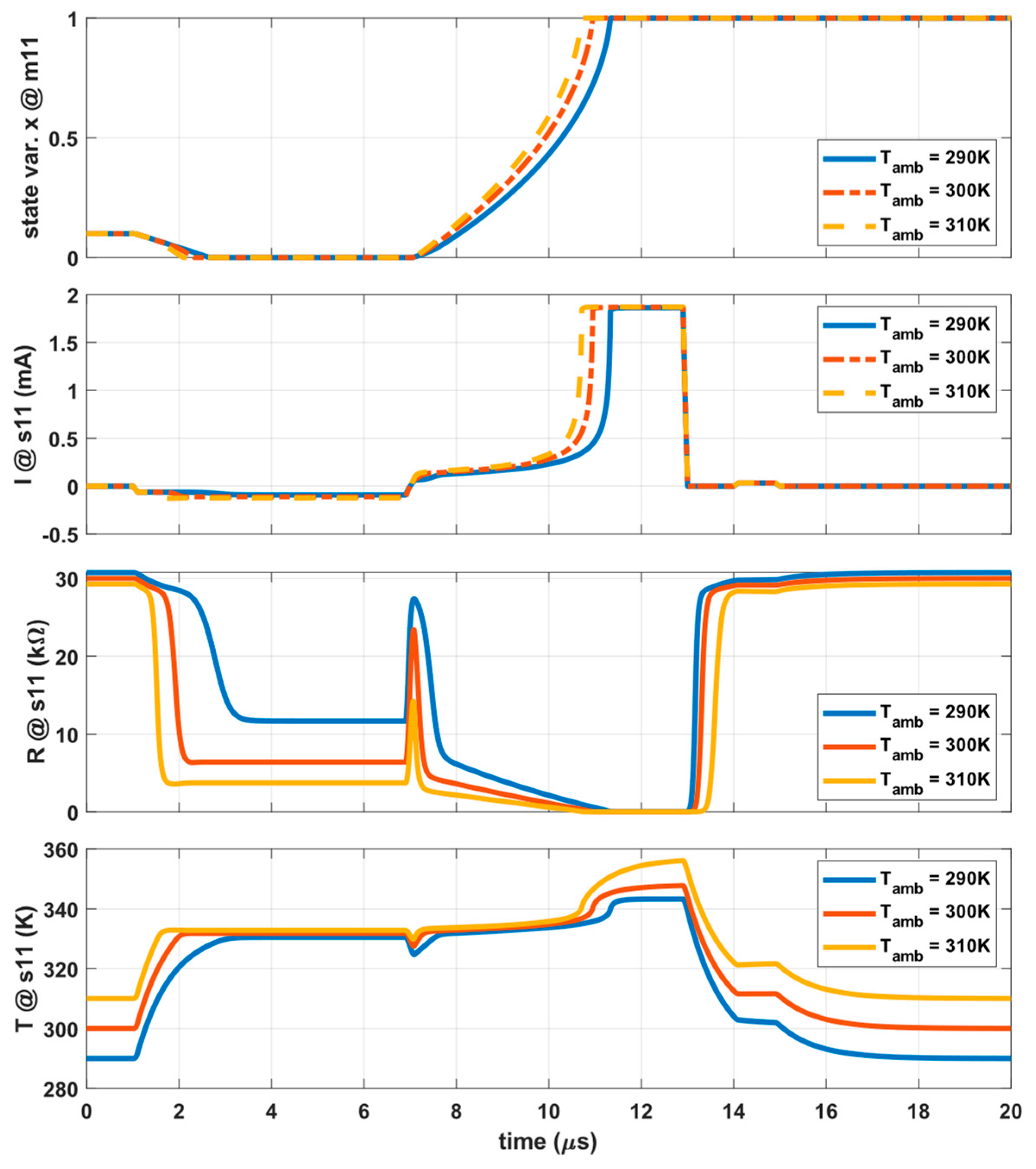
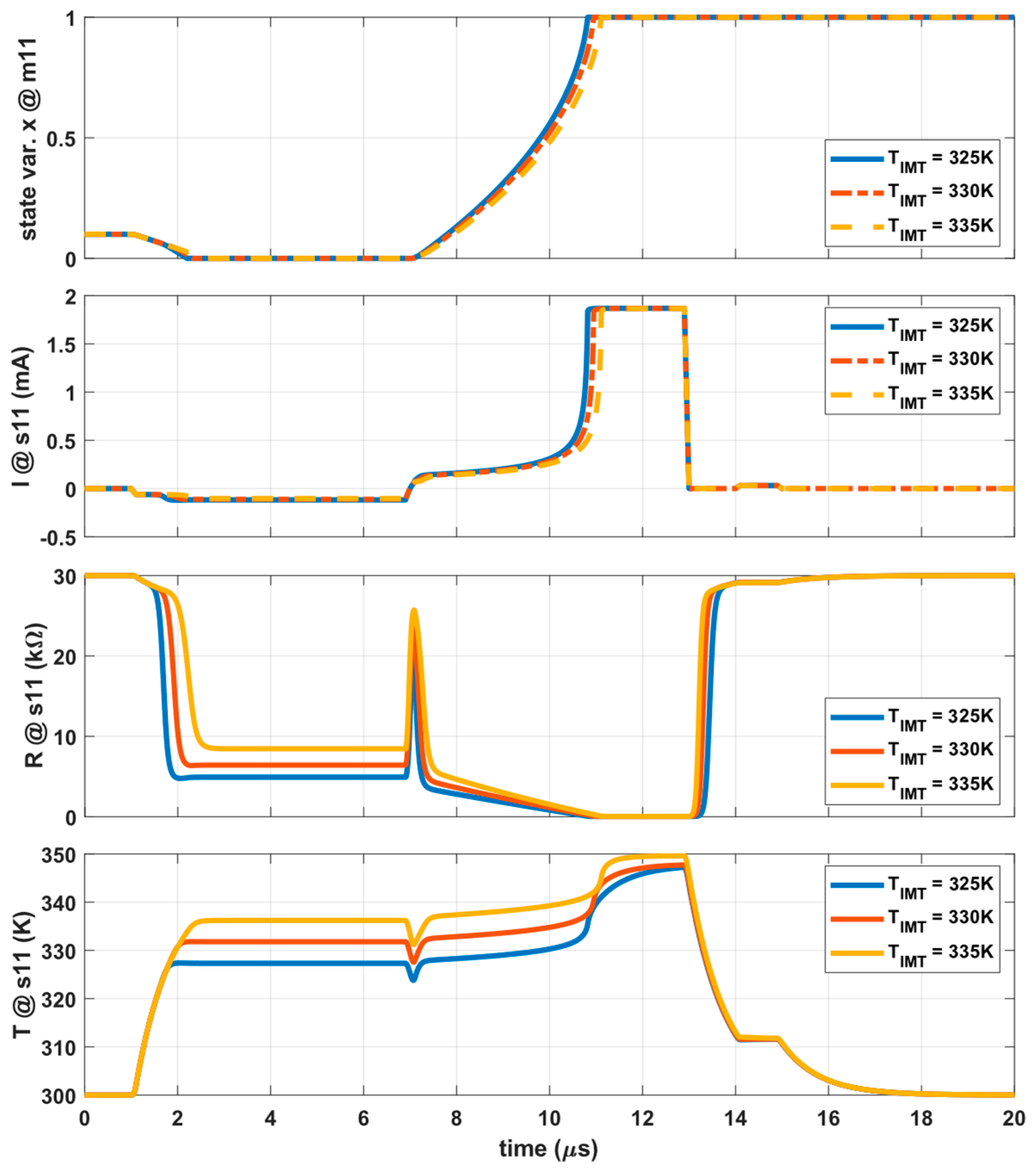
4. Simulation Setup and Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, F.; Koufaty, D.A.; Zhang, X. Understanding Intrinsic Characteristics and System Implications of Flash Memory Based Solid State Drives. SIGMETRICS Perform. Eval. Rev. 2009, 37, 181–192. [Google Scholar] [CrossRef]
- ReRAM. Available online: https://www.fujitsu.com/jp/group/fsm/en/products/reram/ (accessed on 25 October 2023).
- Heyman, K. ReRAM Seeks to Replace NOR. Available online: https://semiengineering.com/reram-seeks-to-replace-nor/ (accessed on 25 October 2023).
- Wainstein, N.; Adam, G.; Yalon, E.; Kvatinsky, S. Radiofrequency Switches Based on Emerging Resistive Memory Technologies—A Survey. Proc. IEEE 2021, 109, 77–95. [Google Scholar] [CrossRef]
- Dittmann, R.; Menzel, S.; Waser, R. Nanoionic Memristive Phenomena in Metal Oxides: The Valence Change Mechanism. Adv. Phys. 2021, 70, 155–349. [Google Scholar] [CrossRef]
- Strukov, D.B.; Snider, G.S.; Stewart, D.R.; Williams, R.S. The Missing Memristor Found. Nature 2008, 453, 80–83. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Wu, H.; Wang, K.; Wu, R.; Song, L.; Li, T.; Wang, J.; Yu, Z.; Qian, H. Stacked 3D RRAM Array with Graphene/CNT as Edge Electrodes. Sci. Rep. 2015, 5, 13785. [Google Scholar] [CrossRef] [PubMed]
- Shang, Y.; Ohsawa, T. Accurate Measurement of Sneak Current in ReRAM Crossbar Array with Data Storage Pattern Dependencies. In Proceedings of the 2019 International Symposium on VLSI Technology, Systems and Application (VLSI-TSA), Hsinchu, Taiwan, 22–25 April 2019; pp. 1–2. [Google Scholar]
- Demin, V.A.; Surazhevsky, I.A.; Emelyanov, A.V.; Kashkarov, P.K.; Kovalchuk, M.V. Sneak, Discharge, and Leakage Current Issues in a High-Dimensional 1T1M Memristive Crossbar. J. Comput. Electron. 2020, 19, 565–575. [Google Scholar] [CrossRef]
- Kannan, S.; Rajendran, J.; Karri, R.; Sinanoglu, O. Sneak-Path Testing of Crossbar-Based Nonvolatile Random Access Memories. IEEE Trans. Nanotechnol. 2013, 12, 413–426. [Google Scholar] [CrossRef]
- Chen, A. Memory Selector Devices and Crossbar Array Design: A Modeling-Based Assessment. J. Comput. Electron. 2017, 16, 1186–1200. [Google Scholar] [CrossRef]
- Lekshmi Jagath, A.; Kumar, T.N.; Almurib, H.A.; Jinesh, K.B.P. Analytical Modelling of Tantalum/Titanium Oxide-Based Multi-layer Selector to Eliminate Sneak Path Current in RRAM Arrays. IET Circuits Devices Syst. 2020, 14, 1092–1098. [Google Scholar] [CrossRef]
- Shi, L.; Zheng, G.; Tian, B.; Dkhil, B.; Duan, C. Research Progress on Solutions to the Sneak Path Issue in Memristor Crossbar Arrays. Nanoscale Adv. 2020, 2, 1811–1827. [Google Scholar] [CrossRef]
- Seok, J.Y.; Song, S.J.; Yoon, J.H.; Yoon, K.J.; Park, T.H.; Kwon, D.E.; Lim, H.; Kim, G.H.; Jeong, D.S.; Hwang, C.S. A Review of Three-Dimensional Resistive Switching Cross-Bar Array Memories from the Integration and Materials Property Points of View. Adv. Funct. Mater. 2014, 24, 5316–5339. [Google Scholar] [CrossRef]
- Ravi, V.; Singh, S.; Sofana Reka, S. Memristor-Based 2D1M Architecture: Solution to Sneak Paths in Multilevel Memory. Trans. Emerg. Telecommun. Technol. 2021, 32, e4143. [Google Scholar] [CrossRef]
- Li, Y.; Ang, K.-W. Hardware Implementation of Neuromorphic Computing Using Large-Scale Memristor Crossbar Arrays. Adv. Intell. Syst. 2021, 3, 2000137. [Google Scholar] [CrossRef]
- Zhang, L. Study of the Selector Element for Resistive Memory. Available online: https://lirias.kuleuven.be/1733031?limo=0 (accessed on 20 December 2023).
- Li, Y.; Gong, Q.; Li, R.; Jiang, X. A New Bipolar RRAM Selector Based on Anti-Parallel Connected Diodes for Crossbar Applications. Nanotechnology 2014, 25, 185201. [Google Scholar] [CrossRef]
- Lee, J.; Lee, S.; Kwak, M.; Choi, W.; Mosendz, O.; Hwang, H. Enhanced Switching Characteristics of an Ovonic Threshold Switching Device with an Ultra-Thin MgO Interfacial Layer. IEEE Electron. Device Lett. 2022, 43, 220–223. [Google Scholar] [CrossRef]
- Narayanan, P.; Burr, G.W.; Shenoy, R.S.; Stephens, S.; Virwani, K.; Padilla, A.; Kurdi, B.N.; Gopalakrishnan, K. Exploring the Design Space for Crossbar Arrays Built With Mixed-Ionic-Electronic-Conduction (MIEC) Access Devices. IEEE J. Electron. Devices Soc. 2015, 3, 423–434. [Google Scholar] [CrossRef]
- Farjadian, M.; Shalchian, M. Modeling and Design of a Mott Selector for a ReRAM-Based Non-Volatile Memory Cell in a Crossbar Architecture. J. Comput. Electron. 2022, 21, 535–549. [Google Scholar] [CrossRef]
- Jeon, D.S.; Dongale, T.D.; Kim, T.G. Low Power Ti-Doped NbO2-Based Selector Device with High Selectivity and Low OFF Current. J. Alloys Compd. 2021, 884, 161041. [Google Scholar] [CrossRef]
- Cha, E.; Woo, J.; Lee, D.; Lee, S.; Hwang, H. Selector Devices for 3-D Cross-Point ReRAM. In Proceedings of the 2014 IEEE International Symposium on Circuits and Systems (ISCAS), Melbourne, Australia, 1–5 June 2014; pp. 428–431. [Google Scholar]
- Dai, Y.; Tao, F.; Qi, M. Design of Selector-Based Insulator-Metal Transition Model for TiO2 Bipolar Resistive Random Access Memory. J. Appl. Phys. 2019, 126, 075705. [Google Scholar] [CrossRef]
- Nandi, S.K.; Liu, X.; Venkatachalam, D.K.; Elliman, R.G. Threshold Current Reduction for the Metal–Insulator Transition in NbO2-x-Selector Devices: The Effect of ReRAM Integration. J. Phys. D Appl. Phys. 2015, 48, 195105. [Google Scholar] [CrossRef]
- Mott, N.F. Metal-Insulator Transition. Rev. Mod. Phys. 1968, 40, 677–683. [Google Scholar] [CrossRef]
- Morin, F.J. Oxides Which Show a Metal-to-Insulator Transition at the Neel Temperature. Phys. Rev. Lett. 1959, 3, 34–36. [Google Scholar] [CrossRef]
- Darwish, M.; Neumann, P.; Mizsei, J.; Pohl, L. Electro-Thermal Simulation of Vertical VO2 Thermal-Electronic Circuit Elements. Energies 2020, 13, 3447. [Google Scholar] [CrossRef]
- Yang, Z.; Ko, C.; Ramanathan, S. Oxide Electronics Utilizing Ultrafast Metal-Insulator Transitions. Annu. Rev. Mater. Res. 2011, 41, 337–367. [Google Scholar] [CrossRef]
- Darwish, M.; Pohl, L. SPICE Modeling of Insulator-Metal Transition Devices with Hysteresis. In Proceedings of the 2023 29th International Workshop on Thermal Investigations of ICs and Systems (THERMINIC), Budapest, Hungary, 27–29 September 2023; pp. 1–5. [Google Scholar]
- Amer, S.; Hasan, M.S.; Adnan, M.M.; Rose, G.S. SPICE Modeling of Insulator Metal Transition: Model of the Critical Temperature. IEEE J. Electron Devices Soc. 2019, 7, 18–25. [Google Scholar] [CrossRef]
- Chua, L. Memristor-The Missing Circuit Element. IEEE Trans. Circuit Theory 1971, 18, 507–519. [Google Scholar] [CrossRef]
- Haase, J.; Lange, A. Hybrid Dynamical Systems for Memristor Modelling an Approach Avoiding the Terminal-State Problem. In Proceedings of the 2013 Forum on Specification and Design Languages (FDL), Paris, France, 24–26 September 2013; pp. 1–6. [Google Scholar]
- PartQuestTM Explore|Design, Modeling, Simulation and Analysis. Available online: https://explore.partquest.com/ (accessed on 13 December 2023).






| Operation | Writing 1 at m11 | Reading from m11 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Biasing Scheme | 1/2 | 1/3 | 1/2 | 1/3 | |||||
| Selector | without | with | without | with | without | with | without | with | |
| Main Current at m11 | 2003 | 1870 | 2062 | 1890 | 667 | 31.61 | 687.3 | 31.96 | |
| Sneak Current at | m12, m13 | 320.2 | 34.18 | 124.3 | 22.39 | 208.1 | 11.35 | 44.6 | 7.47 |
| m21, m31 | −99.4 | −27.08 | −110 | −38.49 | −26 | 9.25 | −36 | 5.84 | |
| m22, m23, m32, m33 | −25.7 | −1.05 | −60 | −21.25 | −16 | −0.349 | −21.8 | −7.04 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Darwish, M.; Pohl, L. Insulator Metal Transition-Based Selector in Crossbar Memory Arrays. Electron. Mater. 2024, 5, 17-29. https://doi.org/10.3390/electronicmat5010002
Darwish M, Pohl L. Insulator Metal Transition-Based Selector in Crossbar Memory Arrays. Electronic Materials. 2024; 5(1):17-29. https://doi.org/10.3390/electronicmat5010002
Chicago/Turabian StyleDarwish, Mahmoud, and László Pohl. 2024. "Insulator Metal Transition-Based Selector in Crossbar Memory Arrays" Electronic Materials 5, no. 1: 17-29. https://doi.org/10.3390/electronicmat5010002
APA StyleDarwish, M., & Pohl, L. (2024). Insulator Metal Transition-Based Selector in Crossbar Memory Arrays. Electronic Materials, 5(1), 17-29. https://doi.org/10.3390/electronicmat5010002







