Structural, Electronic, and Optical Properties of p-Type Semiconductors Cu₂O and ZnRh₂O₄: A Self-Consistent Hybrid Functional Investigation

Abstract

The p-type semiconductors Cu${}_2$O and ZnRh${}_2$O${}_4$ have been under investigation for potential applications as transparent conducting oxides. Here, we re-evaluate their structural, electronic, and optical properties by means of first-principles calculations employing density functional theory and a recently introduced self-consistent hybrid functional approach. Therein, the predefined fraction $\alpha$ of Hartree–Fock exact exchange is determined self-consistently via the inverse of the dielectric constant ${\epsilon }_{\infty }$. The structural, electronic, and optical properties will be discussed alongside experimental results, with a focus on possible technological applications.

1. Introduction

Transparent conducting oxides (TCOs) are one important building block of current technological devices, ranging from various display applications to solar cells. While the quest to find new and environmentally friendly TCOs has resulted in the identification of numerous n-type TCOs, their p-type counterparts are much harder to come by. This apparent scarcity of p-type TCOs suitable for device applications has led to a surge in experimental and theoretical investigations, trying to understand the underlying reasons and formulating some design principles for specific necessary material properties [1].

The present work focuses on two identified examples for p-type TCOs, namely cuprous oxide (Cu${}_2$O) and ZnRh${}_2$O${}_4$. Cu${}_2$O crystallises in the cubic crystal structure (space group $Pn\overline{3}m$, No. 224), whereas ZnRh${}_2$O${}_4$ crystallises in the so-called normal spinel crystal structure (space group $Fd\overline{3}m$, No. 227), respectively, similar to ZnFe${}_2$O${}_4$ [2], but at variance to NiFe${}_2$O${}_4$ and CoFe${}_2$O${}_4$ which crystallise in the (partially) inverse spinel structure [3]. In general, the spinel crystal structure offers a rich interplay of structural parameters [4], subsequently influencing the electronic and optical properties of materials and allowing for a plethora of potential technological applications [5].

While Cu${}_2$O has been known as a p-type semiconductor for decades, the spinel-type ZnRh${}_2$O${}_4$ received wider attention only fairly recently [6,7]. Subsequent first-principles calculations of the whole spinel series Zn$M_2$O${}_4$ (M = Co, Rh, and Ir) tried to identify their conduction mechanism, i.e., hole or polaron conduction [8,9,10]. These first-principles calculations employed a range of (semi)local and hybrid exchange and correlation functionals to investigate the structural, electronic, and optical properties of ZnRh${}_2$O${}_4$, respectively. Application of exchange and correlation functionals of increased complexity has been recognised as a viable tool for a better description of material properties [11].

The recent development of a self-consistent hybrid functional [12] provided the motivation for the current work, namely to re-evaluate the structural, electronic, and optical properties of Cu${}_2$O and ZnRh${}_2$O${}_4$, similar to other crystalline [13,14] and amorphous [15,16] oxide TCOs.

The paper is organised as follows. Section 2 shortly introduces the self-consistent hybrid functional approach and provides the necessary computational details. In Section 3 the results on the structural (Section 3.1), and electronic and optical (Section 3.2) properties are presented and discussed alongside experimental data and other theoretical investigations. Section 4 provides a short summary and outlook.

2. Theoretical Background

The first-principles calculations presented here employed both, the state-of-the-art meta-GGA SCAN functional [17] and a recently introduced self-consistent hybrid functional (scPBE0) [12,13]. While the SCAN functional fulfils all the necessary requirements of an exchange and correlation functional, it has been claimed that its accuracy in terms of predicting structural properties rivals that of computationally much more demanding hybrid exchange and correlation functionals [18]. On the other hand, the so-called hybrid exchange and correlation functionals admix a predefined fraction $\alpha$ of Hartree–Fock exact exchange into the underlying (semi)local functional and allow for a much improved description of electronic and optical properties of materials. Here, the recently introduced self-consistent hybrid functional has been employed, where the particular fraction of Hartree–Fock exact exchange $\alpha$ is treated as a material specific parameter, determined via an additional self-consistency cycle and employing the reciprocal relationship between $\alpha$ and the low-frequency dielectric constant ${\epsilon }_{\infty }$, i.e., $\alpha =1/{\epsilon }_{\infty }$. The dielectric constant is calculated for the identified ground state structure for each iteration, until their difference between successive iterations is smaller than 0.01 [13,14].

All the calculations of the present work have been performed using the Vienna Ab Initio Simulation Package (VASP 5.4.4) [19,20,21] together with the projector-augmented wave (PAW) formalism [22,23]. Standard PAW potentials supplied by VASP have been employed, contributing 11, 12, 15, and 6 valence electrons for the Cu, Zn, Rh, and O atoms, respectively.

Ground state structural properties have been obtained from analysing the total energy curves, i.e., monitoring changes in the total energy with respect to several fixed volumes around the ground state volume, followed by a subsequent analysis via Murnaghan’s equation of state [24,25], yielding ground state volumes and lattice parameters, and giving additional access to the bulk modulus B${}_0$ and its pressure derivative B${}_0^{\prime }$, respectively. From previous investigations on spinel ferrites it is known that both, lattice parameters and bulk modulus, have to agree with the experimental values in order to identify the correct ground state structure [26].

Based on the ground state structural properties the electronic (band structures) and optical properties (dielectric functions) have been calculated. For the latter, the number of empty bands in the calculations have been increased by a factor of three, in order to allow for a sufficient capture of the influence of higher lying energy bands on the optical properties.

Other calculational parameters include a 500 eV cutoff for the plane wave expansion, convergence criteria of 10${}^{-6}$ eV and 10${}^{-3}$ Å${}^{-1}$ for the calculated total energies and forces on the atoms, respectively, and a $6\times 6\times 6$$\mathsf{\Gamma }$-centred k-point grid, respectively, thus ensuring well-converged results for the structural, electronic, and optical properties.

3. Results and Discussion

3.1. Structural Properties

Figure 1 shows the total energy curves (upper panels) for Cu${}_2$O (left panel) and ZnRh${}_2$O${}_4$ (right panel), calculated with the SCAN (black), PBE0 (red), and scPBE0 (green) exchange and correlation functionals. The dashed lines indicate their respective ground state values, while the orange dashed line refers to the experimental results. Subsequent analysis of the total energy curves yields the ground state volumes and lattice parameters, the bulk modulus $B_0$ and its pressure derivative $B_0^{\prime }$, respectively, summarised and compared to available experimental results in

Table 1. Ground state structural properties for Cu${}_2$O and ZnRh${}_2$O${}_4$ for different exchange and correlation functionals in comparison to experimental data. Shown are the lattice constants a [Å], the internal anion displacement parameter u (for ZnRh${}_2$O${}_4$), the bulk modulus $B_0$ [GPa] and its pressure derivative $B_0^{\prime }$, the calculated dielectric constant ${\epsilon }_{\infty }$ and the corresponding $\alpha$ value, and the Kohn–Sham energy band gap $E_{\mathrm{KS}}$ [eV], respectively.

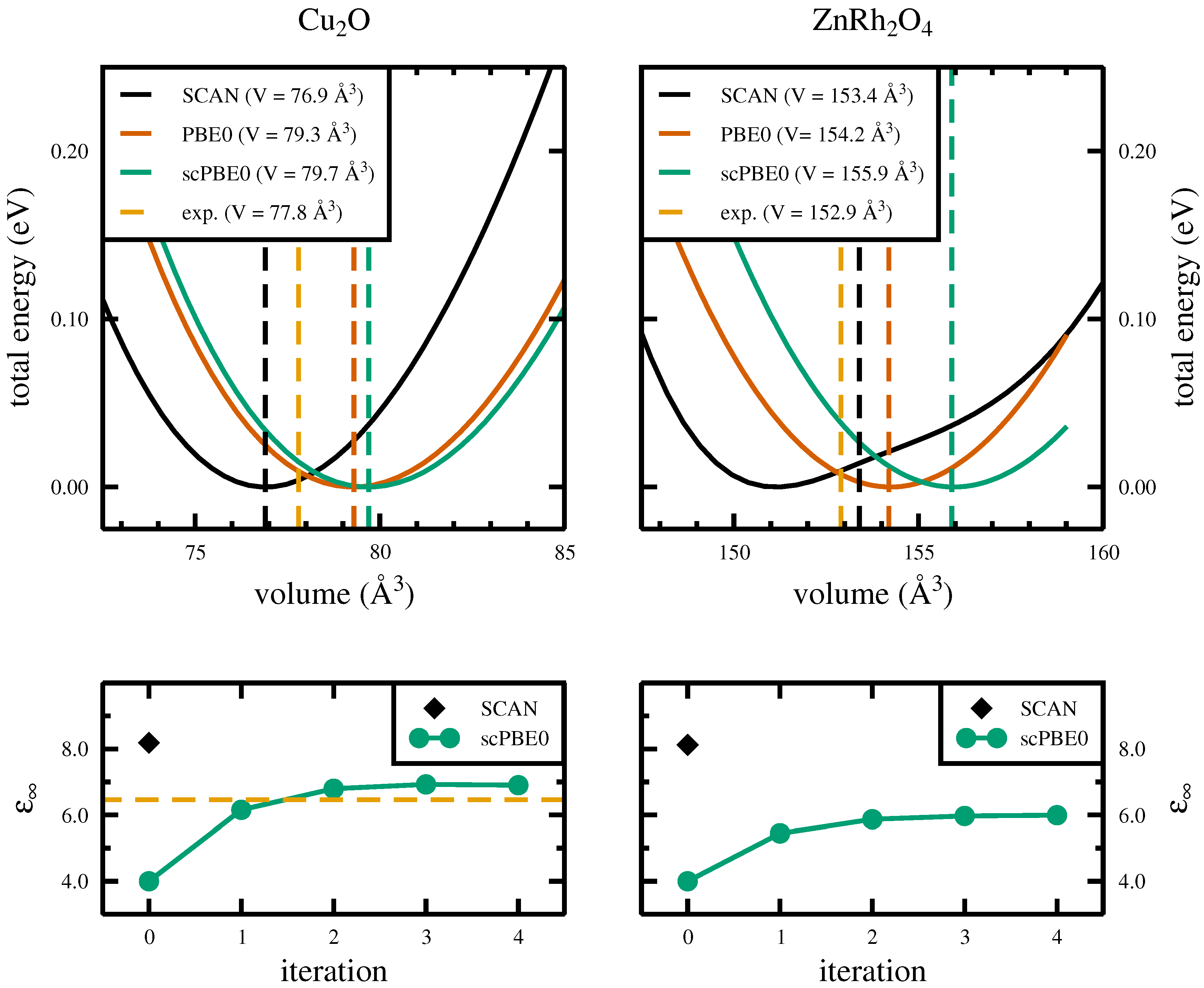
Cu${}_2$O	SCAN	PBE0	scPBE0	Exp.
a [Å]	4.252	4.296	4.303	4.2685 [27], 4.2696 [28]
$B_0$ [GPa]	122.0	110.8	109.8	110.6 [29], 111.96 [30]
$B_0^{\prime }$	4.9	4.6	4.6	4.46 [30]
${\epsilon }_{\infty }$	8.186	6.153	6.922	6.46 [28]
$\alpha$	0.122	0.250	0.147	0.15 [28]
$E_{\mathrm{KS}}$ [eV]	0.559	2.685	1.703	2.1720 [31]
ZnRh${}_2$O${}_4$	SCAN	PBE0	scPBE0	Exp.
a [Å]	8.498	8.513	8.544	8.489 [32]
u	0.260	0.261	0.261	−
$B_0$ [GPa]	196.9	205.4	198.3	−
$B_0^{\prime }$	6.9	3.6	3.6	−
${\epsilon }_{\infty }$	8.125	5.445	5.990	−
$\alpha$	0.123	0.250	0.168	−
$E_{\mathrm{KS}}$ [eV]	0.989	3.666	2.707	2.1 [33], 2.74 [32]

.

For Cu${}_2$O the lattice constants amount to 4.252 Å, 4.296 Å, and 4.303 Å for the SCAN, PBE0, and scPBE0 exchange and correlation functionals, in good agreement with the experimental lattice constants 4.2685 Å [27] and 4.2696 Å [28]. While the SCAN lattice constant is underestimated with respect to the experimental values, the PBE0 value is similarly good, and the scPBE0 value is slightly overestimated. The bulk moduli $B_0$ amount to 122.0 GPa, 110.8 GPa, and 109.8 GPa, again for the SCAN, PBE0, and scPBE0 exchange and correlation functionals. While the SCAN value is overestimated with respect to the experimental values of 110.6 GPa [29] and 111.96 GPa [30], PBE0 and scPBE0 values show similarly good agreement. The same holds for the pressure derivative $B_0^{\prime }$ of the bulk modulus, with PBE0 and scPBE0 values of 4.6 showing best agreement with the experimental value of 4.46 [30].

The ground state crystal structures served as a starting point for subsequent calculations of the dielectric constant ${\epsilon }_{\infty }$, amounting to 8.186 for the SCAN exchange and correlation functional, overestimated with respect to the experimental value of 6.46 [28], respectively. Much better agreement is obtained for the PBE0 exchange and correlation functional with ${\epsilon }_{\infty }$ amounting to 6.153, iterated to self-consistency within four steps for the scPBE0 exchange and correlation functional, amounting to 6.922, respectively, again in good agreement with the experimental value. The convergence of ${\epsilon }_{\infty }$ for Cu${}_2$O within the scPBE0 approach is shown in the lower left panel of Figure 1.

The lattice constants of ZnRh${}_2$O${}_4$ amount to 8.498 Å, 8.513 Å, and 8.544 Å, again calculated by means of the SCAN, PBE0, and scPBE0 exchange and correlation functionals. This is in favourable agreement with the experimental lattice constant of 8.489 Å [32]. Interestingly, the SCAN value shows the best agreement, and the scPBE0 value is again larger than the PBE0 one. The internal oxygen parameter u, the bulk modulus $B_0$ and its pressure derivative $B_0^{\prime }$ are all in agreement with other theoretical investigations employing various types of exchange and correlation functionals.

Again, the ground state crystal structures served as starting points for calculating the dielectric constant ${\epsilon }_{\infty }$, yielding a large 8.125 for the SCAN exchange and correlation functional. Similar to Cu${}_2$O, self-consistency in the calculation of ${\epsilon }_{\infty }$ is reached after four steps, starting from 5.445 (PBE0) to 5.990 (scPBE0), respectively.

3.2. Electronic and Optical Properties

The determined ground state structural properties allowed for subsequent analysis of the electronic and optical properties. The calculated electronic band structures are shown in the upper panels of Figure 2 for Cu${}_2$O (left) and ZnRh${}_2$O${}_4$ (right), respectively.

Shown are the valence (green) and conduction bands (red), calculated by means of the self-consistent hybrid functional scPBE0, with the valence band maximum set to zero. The shaded grey area depicts the calculations employing the SCAN exchange and correlation functional.

For Cu${}_2$O the band gap is direct and at the $\mathsf{\Gamma }$ point, amounting to 0.559 eV, 2.685 eV, and 1.703 eV for calculations employing the SCAN, PBE0, and scPBE0 exchange and correlation functionals. The best agreement with the experimental result of 2.1720 eV [31] is observed for the hybrid functionals PBE0 and scPBE0. Interestingly, iterating the fraction $\alpha$ of Hartree–Fock exact exchange to self-consistency reduces the band gap considerably and yields better agreement with the experiment.

For ZnRh${}_2$O${}_4$ the band gap is indirect with the valence band maximum at X and the conduction band minimum closely to X along the X—$\mathsf{\Gamma }$ line. The band gaps amount to 0.989 eV, 3.666 eV, and 2.707 eV for calculations employing the SCAN, PBE0, and scPBE0 exchange and correlation functionals. Again, the best agreement with the experimental band gap of 2.74 eV [32] is observed for the scPBE0 exchange and correlation functional.

This is consistent with earlier hybrid functional calculations yielding 2.87 eV [9]. Scanlon et al. [9] and Wahila et al. [34] also noted that the crystal field splittings play a crucial role for the band edges in the spinel materials Zn$M_2$O${}_4$ (M = Co, Rh, and Ir), and are difficult to treat with previously employed exchange and correlation functionals. As a possible solution, they suggested adjusting the fraction $\alpha$ of Hartree–Fock exact exchange in hybrid functional calculations. This serves as a further justification for the present work. Subsequently, a detailed self-consistent hybrid functional investigation for spinel materials Zn$M_2$O${}_4$ (M = Co, Rh, and Ir) is underway, and will be published elsewhere.

Following the calculation of the band structures, the optical properties have been calculated as well. The obtained ${\epsilon }_1$ real (red) and ${\epsilon }_2$ imaginary (green) parts of the dielectric functions are shown in the lower panels of Figure 2 for Cu${}_2$O (left) and ZnRh${}_2$O${}_4$ (right), respectively. These calculations have been performed employing the scPBE0 exchange and correlation functional, and give additional access to absorption coefficients and reflectivity for better comparison with experimental data.

4. Summary and Outlook

Here, a detailed first-principles investigation re-evaluated the structural, electronic, and optical properties of two p-type semiconductors, namely Cu${}_2$O and ZnRh${}_2$O${}_4$, by means of the self-consistent hybrid functional scPBE0. While the ground state structural properties have been better described with the standard PBE0 exchange and correlation functional, the electronic and optical properties calculated by means of the scPBE0 exchange and correlation functional are in best agreement with available experimental results. For ZnRh${}_2$O${}_4$ in particular, the question of calculating the ligand field splitting reliably by first-principles methods has been raised earlier. In order to address this question, investigations for the spinel materials Zn$M_2$O${}_4$ (M = Co, Rh, and Ir) are underway and will be published elsewhere.