Theoretical Prediction of Heterogeneous Integration of Dissimilar Semiconductor with Various Ultra-Thin Oxides and 2D Materials
Abstract
:1. Introduction
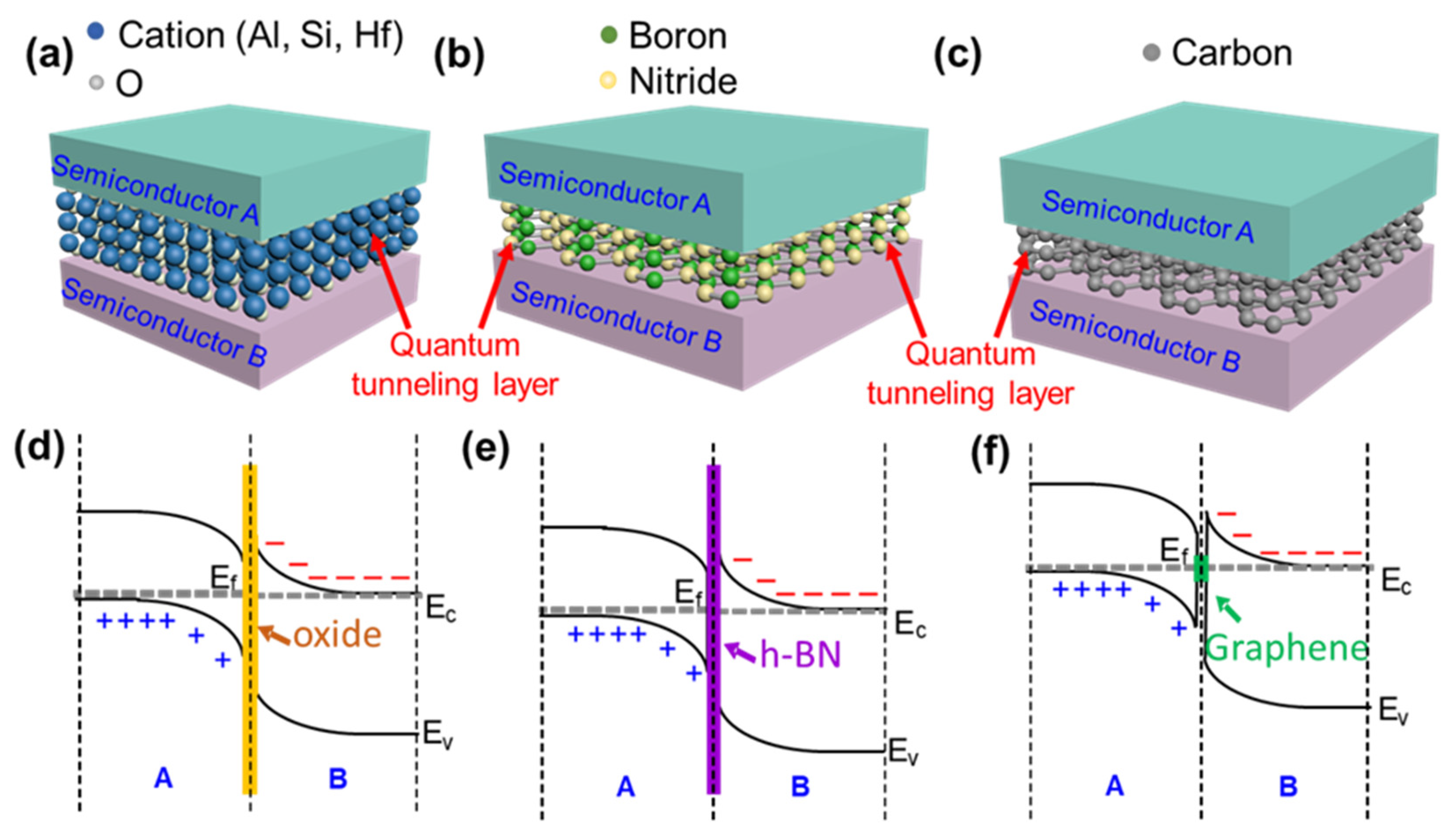
2. Materials and Methods
3. Results
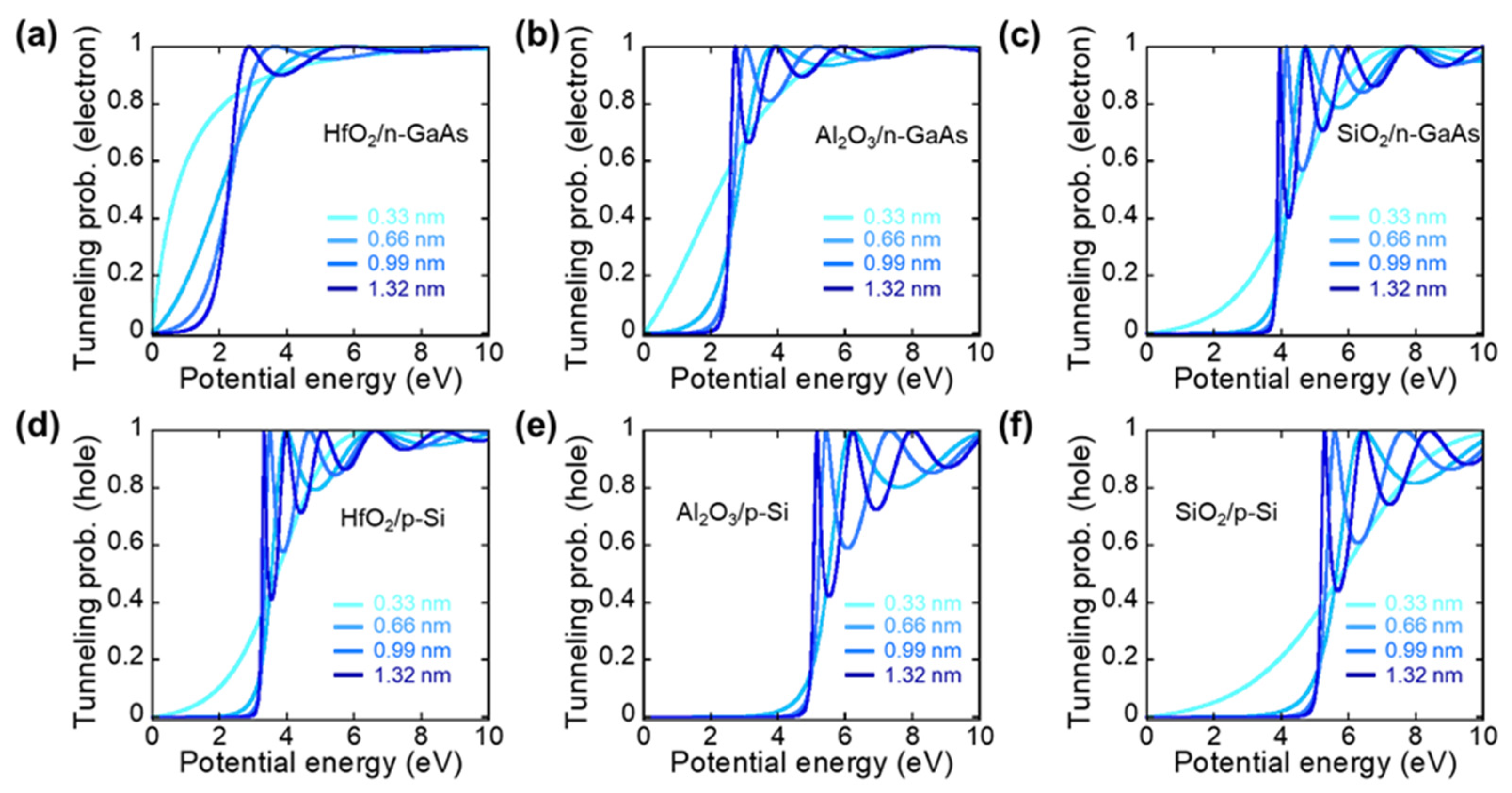
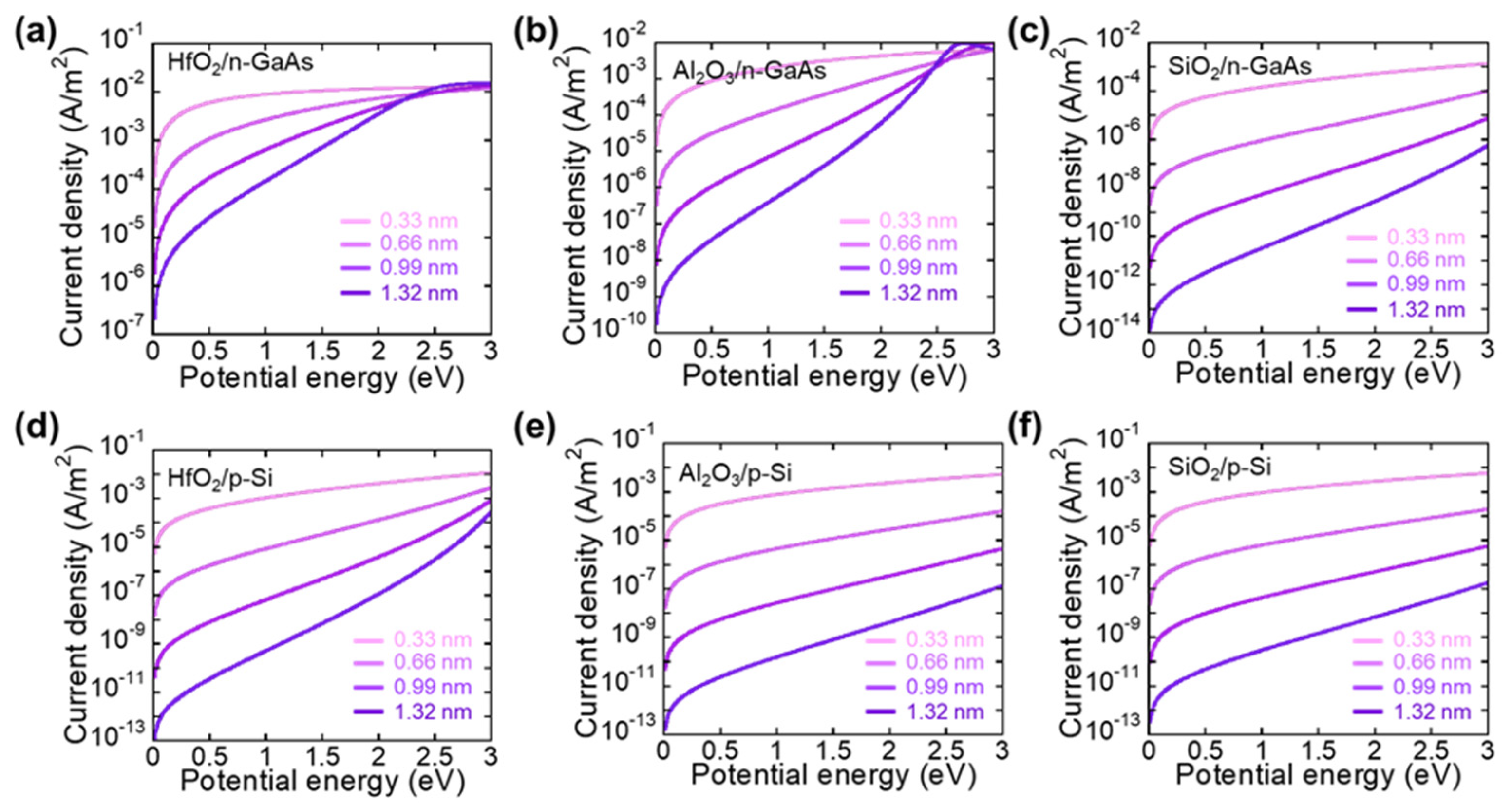
3.1. Tunneling Probability and Current Density with Ultra-Thin Oxide Intermediate Layers
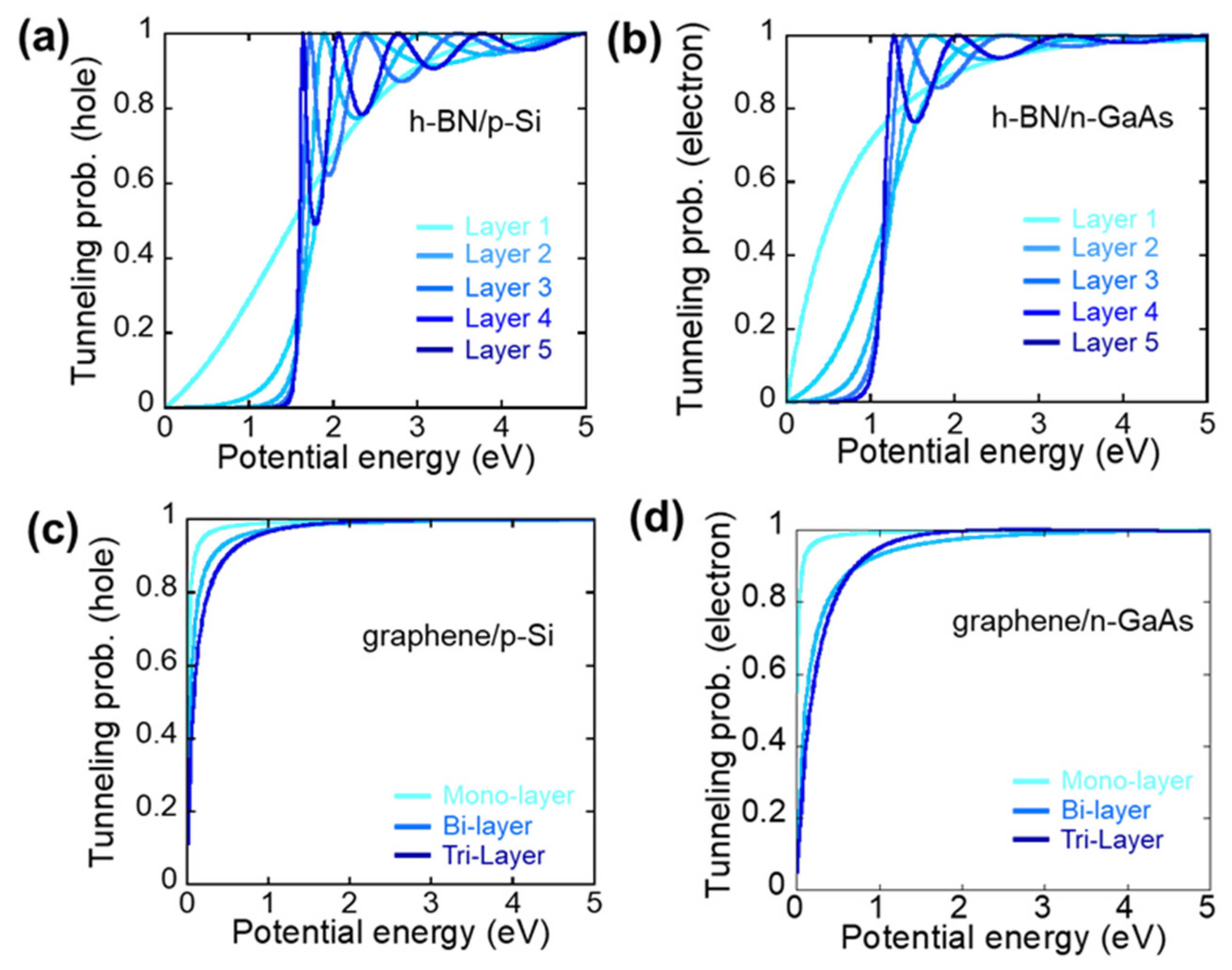
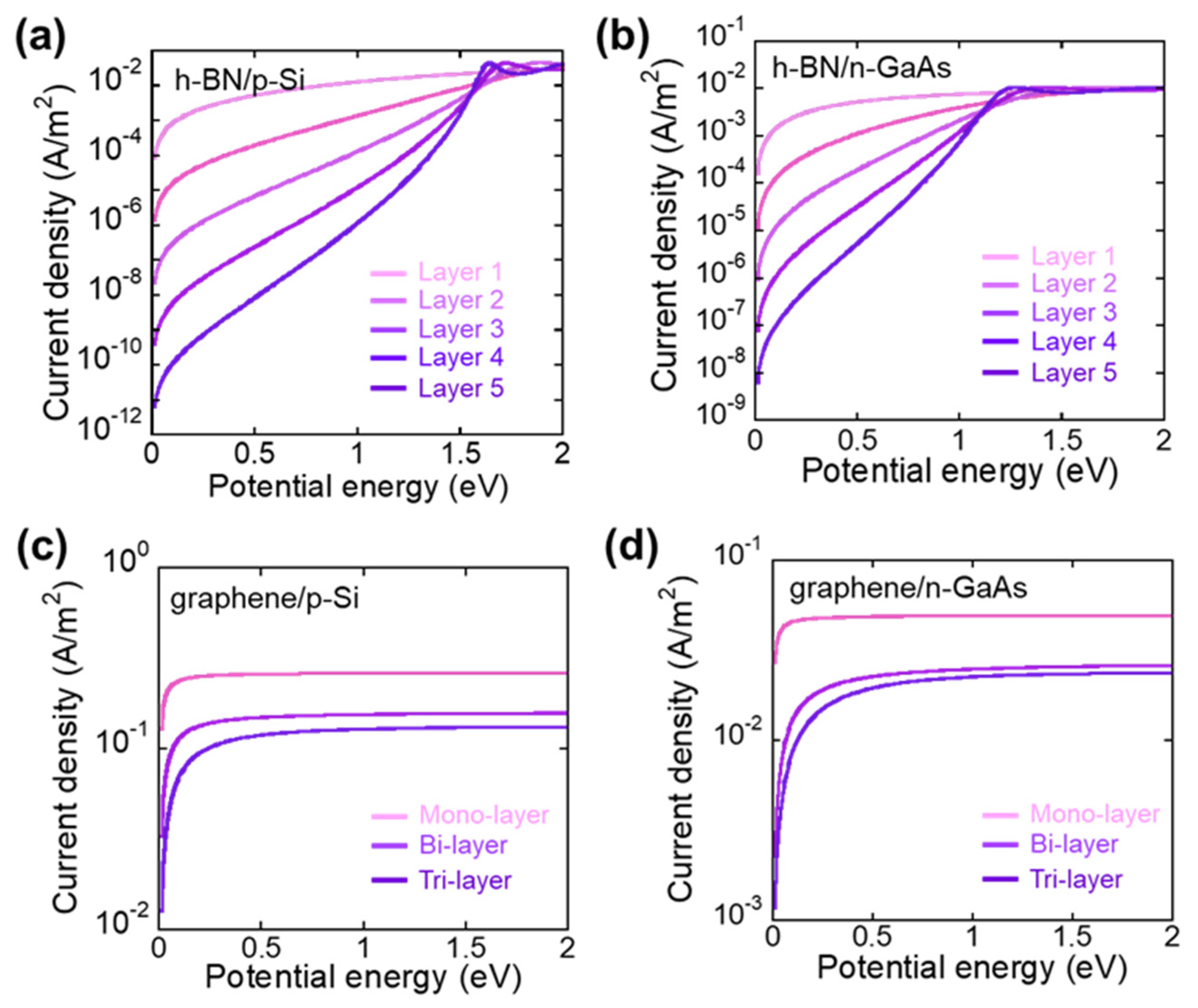
3.2. Tunneling Probability and Current Density with 2D Interface Layers
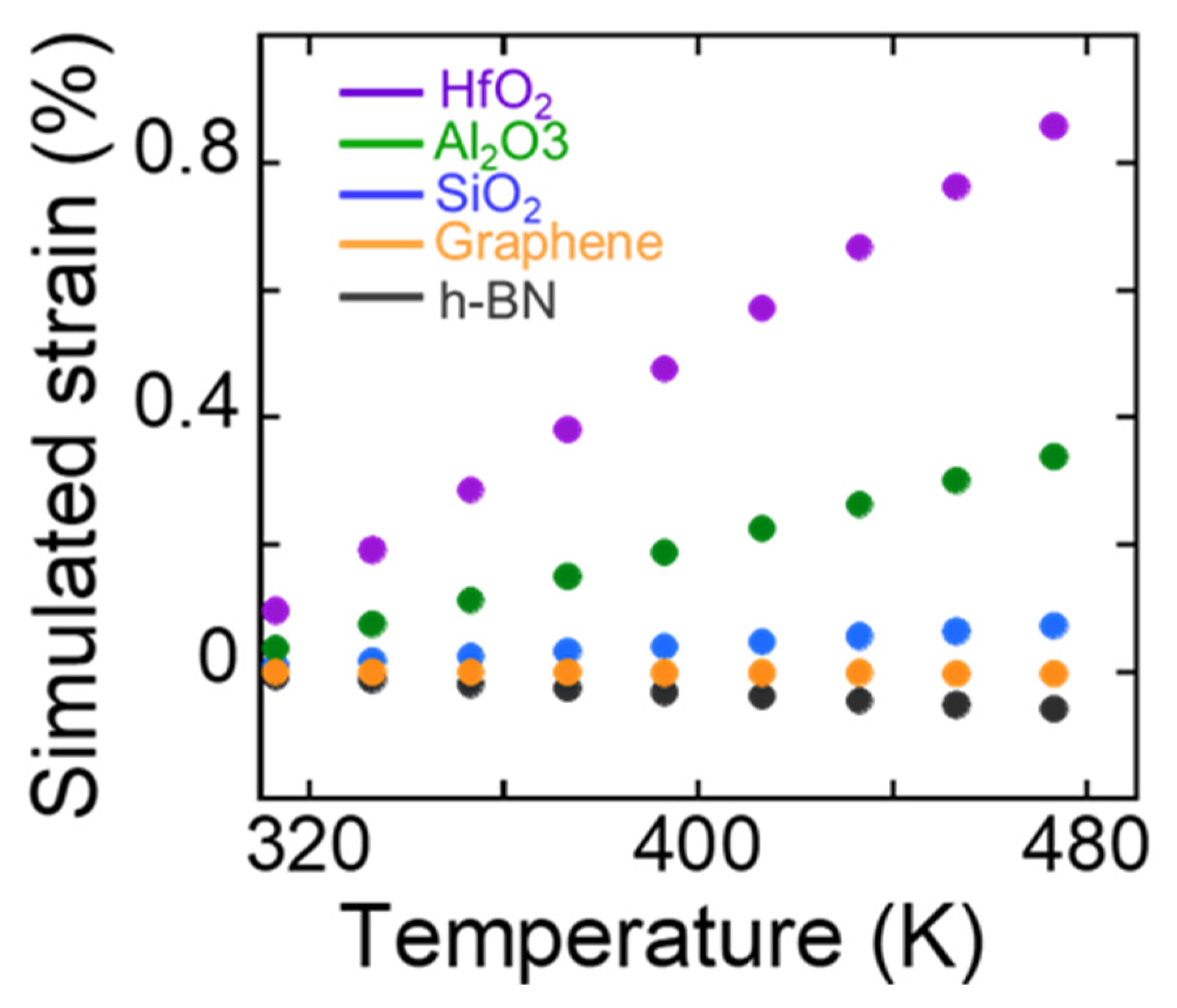
3.3. Simulated Strain under Temperature
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, D.; Cho, S.J.; Park, J.; Gong, J.; Seo, J.-H.; Dalmau, R.; Zhao, D.; Kim, K.; Kim, M.; Kalapala, A.R. 226 nm AlGaN/AlN UV LEDs using p-type Si for hole injection and UV reflection. Appl. Phys. Lett. 2018, 113, 011111. [Google Scholar] [CrossRef]
- Sun, G.; Soref, R.; Cheng, H. Design of an electrically pumped SiGeSn/GeSn/SiGeSn double-heterostructure midinfrared laser. J. Appl. Phys. 2010, 108, 033107. [Google Scholar] [CrossRef]
- Dong, Y.; Son, D.-H.; Dai, Q.; Lee, J.-H.; Won, C.-H.; Kim, J.-G.; Kang, S.-H.; Lee, J.-H.; Chen, D.; Lu, H. AlGaN/GaN heterostructure pH sensor with multi-sensing segments. Sens. Actuators B Chem. 2018, 260, 134–139. [Google Scholar] [CrossRef]
- Ma, Z.; Seo, J.-H. Hybrid Heterostructure Light Emitting Devices. U.S. Patent 9,425,351, 23 August 2016. [Google Scholar]
- Palacios, T.; Chini, A.; Buttari, D.; Heikman, S.; Chakraborty, A.; Keller, S.; DenBaars, S.P.; Mishra, U.K. Use of double-channel heterostructures to improve the access resistance and linearity in GaN-based HEMTs. IEEE Trans. Electron Devices 2006, 53, 562–565. [Google Scholar] [CrossRef]
- Mapel, J.; Singh, M.; Baldo, M.; Celebi, K. Plasmonic excitation of organic double heterostructure solar cells. Appl. Phys. Lett. 2007, 90, 121102. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.; Zhao, D.; Liu, S.; Liu, Y.; Seo, J.-H.; Ma, Z.; Zhou, W. Transfer printed nanomembranes for heterogeneously integrated membrane photonics. Photonics 2015, 2, 1081–1100. [Google Scholar] [CrossRef] [Green Version]
- Asif Khan, M.; Yang, J.; Knap, W.; Frayssinet, E.; Hu, X.; Simin, G.; Prystawko, P.; Leszczynski, M.; Grzegory, I.; Porowski, S. GaN–AlGaN heterostructure field-effect transistors over bulk GaN substrates. Appl. Phys. Lett. 2000, 76, 3807–3809. [Google Scholar] [CrossRef]
- Paul, D.J. Si/SiGe heterostructures: From material and physics to devices and circuits. Semicond. Sci. Technol. 2004, 19, R75. [Google Scholar] [CrossRef]
- Sandroff, C.; Nottenburg, R.; Bischoff, J.C.; Bhat, R. Dramatic enhancement in the gain of a GaAs/AlGaAs heterostructure bipolar transistor by surface chemical passivation. Appl. Phys. Lett. 1987, 51, 33–35. [Google Scholar] [CrossRef]
- Tong, Q.-Y.; Goesele, U. Semiconductor Wafer Bonding: Science and Technology; John Wiley: Hoboken, NJ, USA, 1999. [Google Scholar]
- Okuno, Y.; Uomi, K.; Aoki, M.; Tsuchiya, T. Direct wafer bonding of III-V compound semiconductors for free-material and free-orientation integration. IEEE J. Quantum Electron. 1997, 33, 959–969. [Google Scholar] [CrossRef]
- Black, A.; Hawkins, A.; Margalit, N.; Babic, D.; Holmes, A.; Chang, Y.-L.; Abraham, P.; Bowers, J.E.; Hu, E. Wafer fusion: Materials issues and device results. IEEE J. Sel. Top. Quantum Electron. 1997, 3, 943–951. [Google Scholar] [CrossRef]
- Pasquariello, D.; Hjort, K. Plasma-assisted InP-to-Si low temperature wafer bonding. IEEE J. Sel. Top. Quantum Electron. 2002, 8, 118–131. [Google Scholar] [CrossRef]
- Tanabe, K.; Watanabe, K.; Arakawa, Y. III-V/Si hybrid photonic devices by direct fusion bonding. Sci. Rep. 2012, 2, 1–6. [Google Scholar] [CrossRef]
- Howlader, M.; Watanabe, T.; Suga, T. Investigation of the bonding strength and interface current of p-Si/n-GaAs wafers bonded by surface activated bonding at room temperature. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. Process. Meas. Phenom. 2001, 19, 2114–2118. [Google Scholar] [CrossRef] [Green Version]
- Chung, T.R.; Yang, L.; Hosoda, N.; Takagi, H.; Suga, T. Wafer direct bonding of compound semiconductors and silicon at room temperature by the surface activated bonding method. Appl. Surf. Sci. 1997, 117, 808–812. [Google Scholar] [CrossRef]
- Liu, D.; Cho, S.J.; Seo, J.-H.; Kim, K.; Kim, M.; Shi, J.; Yin, X.; Choi, W.; Zhang, C.; Kim, J. Lattice-mismatched semiconductor heterostructures. arXiv 2018, arXiv:1812.10225. [Google Scholar]
- Cho, M.; Seo, J.-H.; Kim, M.; Lee, J.; Liu, D.; Zhou, W.; Yu, Z.; Ma, Z. Resonant cavity germanium photodetector via stacked single-crystalline nanomembranes. J. Vac. Sci. Technol. B Nanotechnol. Microelectron. Mater. Process. Meas. Phenom. 2016, 34, 040604. [Google Scholar] [CrossRef]
- Schenk, A.; Heiser, G. Modeling and simulation of tunneling through ultra-thin gate dielectrics. J. Appl. Phys. 1997, 81, 7900–7908. [Google Scholar] [CrossRef] [Green Version]
- Yoshida, T.; Imafuku, D.; Alay, J.L.; Miyazaki, S.; Hirose, M. Quantitative analysis of tunneling current through ultrathin gate oxides. Jpn. J. Appl. Phys. 1995, 34, L903. [Google Scholar] [CrossRef]
- Fermor, J. Quantum-mechanical tunneling. Am. J. Phys. 1966, 34, 1168–1170. [Google Scholar] [CrossRef]
- Chapter 1—Quantum Mechanical Tunnelling. In Tunnelling and Negative Resistance Phenomena in Semiconductors; Pamplin, B.R.; Roy, D.K. (Eds.) Pergamon: Oxford, UK, 1977; pp. 1–35. [Google Scholar]
- Thornton, S.T.; Rex, A. Modern Physics for Scientists and Engineers; Cengage Learning: Boston, MA, USA, 2012. [Google Scholar]
- Faddeev, L.D.; Merkuriev, S.P. Quantum Scattering Theory for Several Particle Systems; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 11. [Google Scholar]
- Ghetti, A.; Liu, C.-T.; Mastrapasqua, M.; Sangiorgi, E. Characterization of tunneling current in ultra-thin gate oxide. Solid-State Electron. 2000, 44, 1523–1531. [Google Scholar] [CrossRef]
- Xu, Y.-N.; Ching, W. Calculation of ground-state and optical properties of boron nitrides in the hexagonal, cubic, and wurtzite structures. Phys. Rev. B 1991, 44, 7787. [Google Scholar] [CrossRef] [PubMed]
- Arnaud, B.; Lebègue, S.; Rabiller, P.; Alouani, M. Huge Excitonic Effects in Layered Hexagonal Boron Nitride. Phys. Rev. Lett. 2006, 96, 026402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Avetisyan, A.; Partoens, B.; Peeters, F. Stacking order dependent electric field tuning of the band gap in graphene multilayers. Phys. Rev. B 2010, 81, 115432. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Feng, Y.; Shen, Z. Structural and electronic properties of h-BN. Phys. Rev. B 2003, 68, 104102. [Google Scholar] [CrossRef]
- Haggerty, R.P.; Sarin, P.; Apostolov, Z.D.; Driemeyer, P.E.; Kriven, W.M. Thermal expansion of HfO2 and ZrO2. J. Am. Ceram. Soc. 2014, 97, 2213–2222. [Google Scholar] [CrossRef]
- El-Kareh, B.; Hutter, L.N. Fundamentals of Semiconductor Processing Technology; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Wilk, G.D.; Wallace, R.M.; Anthony, J. High-κ gate dielectrics: Current status and materials properties considerations. J. Appl. Phys. 2001, 89, 5243–5275. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasan, M.N.; Li, C.; Lai, J.; Seo, J.-H. Theoretical Prediction of Heterogeneous Integration of Dissimilar Semiconductor with Various Ultra-Thin Oxides and 2D Materials. Electron. Mater. 2021, 2, 495-503. https://doi.org/10.3390/electronicmat2040034
Hasan MN, Li C, Lai J, Seo J-H. Theoretical Prediction of Heterogeneous Integration of Dissimilar Semiconductor with Various Ultra-Thin Oxides and 2D Materials. Electronic Materials. 2021; 2(4):495-503. https://doi.org/10.3390/electronicmat2040034
Chicago/Turabian StyleHasan, Md Nazmul, Chenxi Li, Junyu Lai, and Jung-Hun Seo. 2021. "Theoretical Prediction of Heterogeneous Integration of Dissimilar Semiconductor with Various Ultra-Thin Oxides and 2D Materials" Electronic Materials 2, no. 4: 495-503. https://doi.org/10.3390/electronicmat2040034
APA StyleHasan, M. N., Li, C., Lai, J., & Seo, J.-H. (2021). Theoretical Prediction of Heterogeneous Integration of Dissimilar Semiconductor with Various Ultra-Thin Oxides and 2D Materials. Electronic Materials, 2(4), 495-503. https://doi.org/10.3390/electronicmat2040034






