A CALPHAD-Informed Enthalpy Method for Multicomponent Alloy Systems with Phase Transitions
Abstract
1. Introduction
2. Enthalpy Models
2.1. The Enthalpy Method
2.2. Conventional Enthalpy Model for Multicomponent Systems
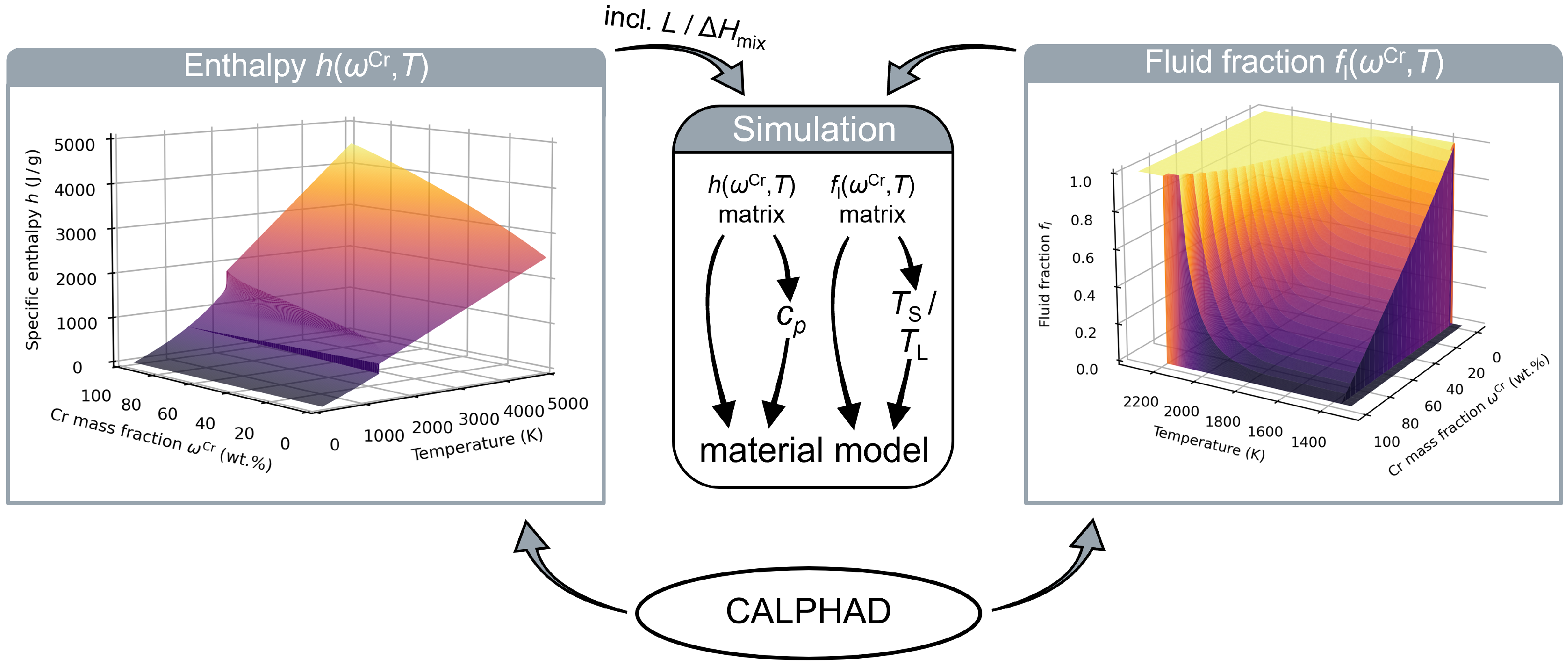
2.3. The CALPHAD-Informed Enthalpy Method
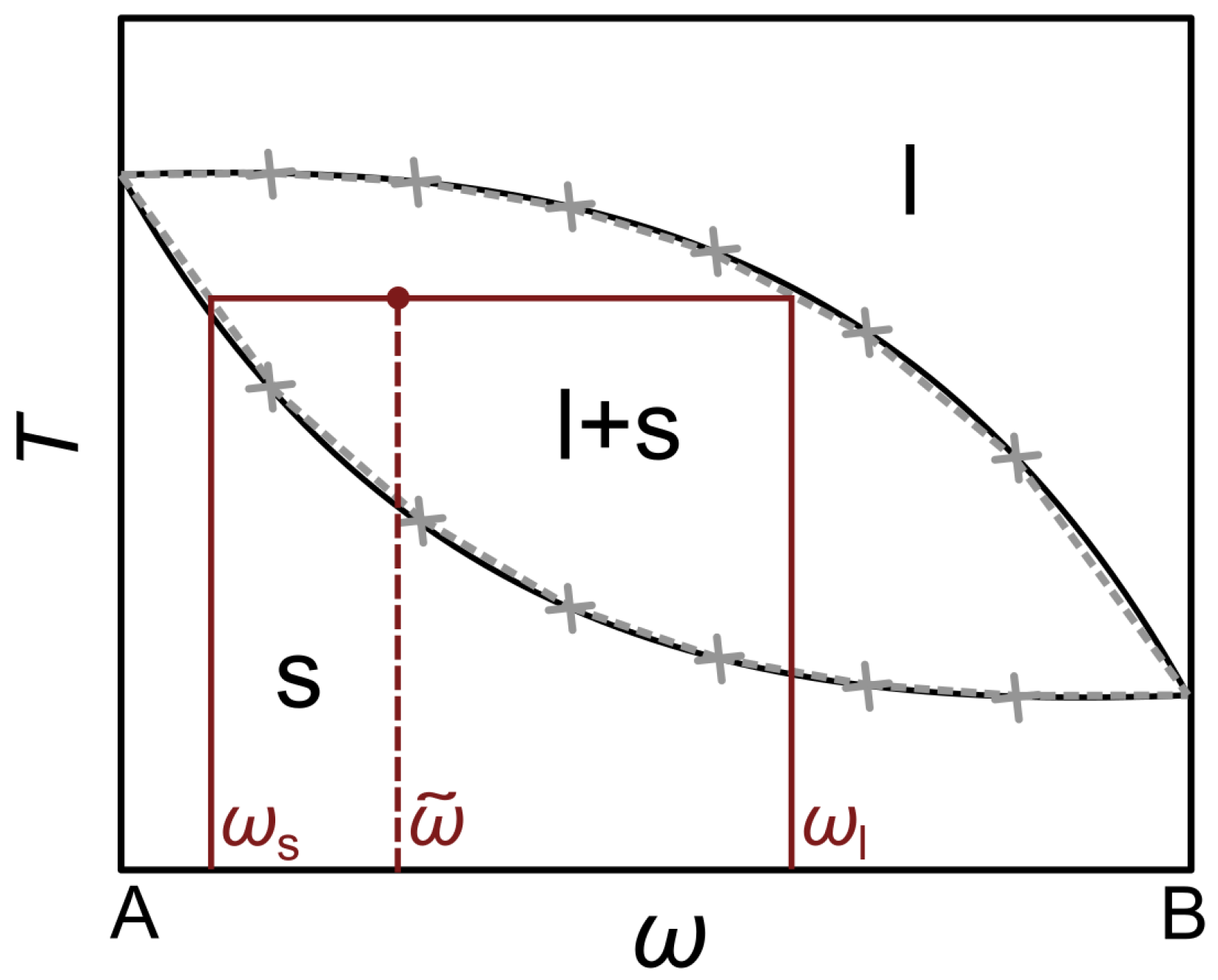
2.3.1. Generation of the CALPHAD Data
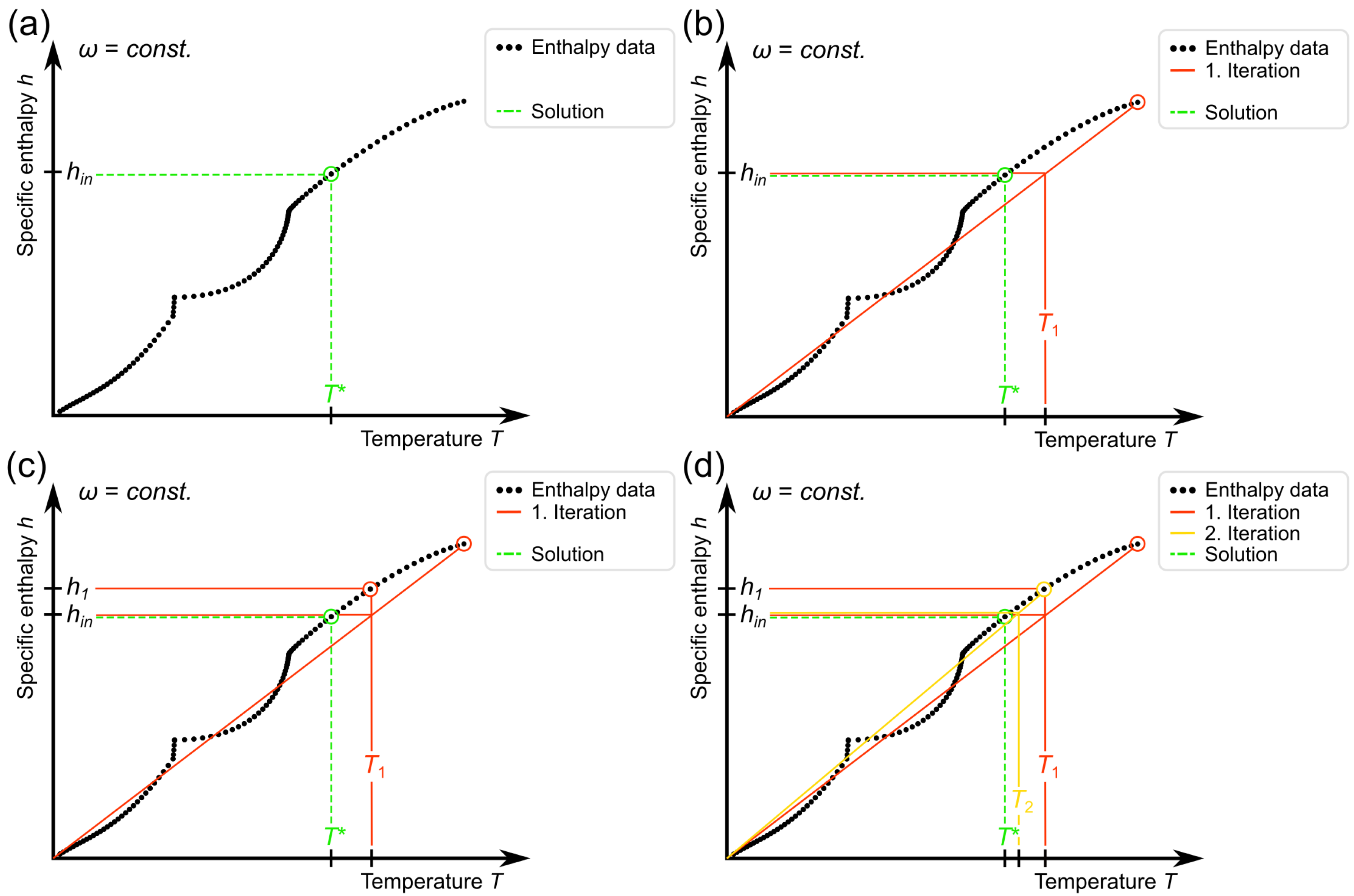
2.3.2. Numerical Implementation
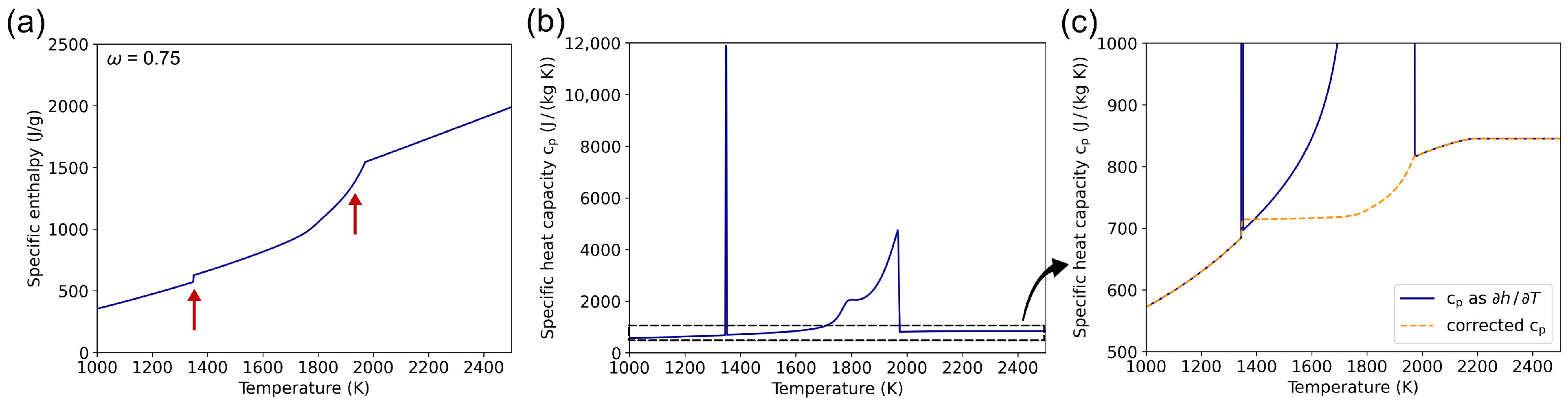
2.3.3. Handling of Phase Transitions
3. Numerical Solvers
3.1. Simulation Software
3.2. Finite Difference (FD) Solver
4. Verification Simulations
4.1. Diffusion Couple
4.2. Coupled Transport Phenomena
4.3. Stefan Problem
5. Application to Multi-Material Electron Beam Powder Bed Fusion
6. Summary
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Specific heat capacity | |
| Specific heat capacity of the liquid phase | |
| Specific heat capacity of the mushy zone | |
| Specific heat capacity of the solid phase | |
| Heat capacity | |
| Heat capacity of the liquid phase | |
| Heat capacity of the solid phase | |
| D | Diffusion coefficient |
| Fluid fraction | |
| h | Specific enthalpy |
| Enthalpy of mixing | |
| Specific enthalpy of mixing | |
| Normalised total specific enthalpy | |
| H | Enthalpy |
| Volume-specific enthalpy | |
| Diffusion flux | |
| l | Length |
| Diffusion length | |
| L | Latent heat of fusion |
| Thermal source term | |
| t | Time |
| T | Temperature |
| Average temperature | |
| Temperature of the liquid phase | |
| Liquidus temperature | |
| Melting point | |
| Temperature of the solid phase | |
| Solidus temperature | |
| Velocity | |
| x | Spatial coordinate |
| Position of the phase boundary | |
| Thermal diffusivity | |
| Thermal diffusivity of the liquid phase | |
| Thermal diffusivity of the solid phase | |
| Thermal conductivity | |
| Thermal conductivity of the liquid phase | |
| Thermal conductivity of the solid phase | |
| Density | |
| Density of the liquid phase | |
| Density of the solid phase | |
| Mass fraction | |
| Mass fraction of element A | |
| Mass fraction of element B | |
| Mass fraction of Chromium | |
| Composition of the liquid phase | |
| Composition of the solid phase |
References
- Kaufman, L.; Bernstein, H.L. Computer Calculation of Phase Diagrams with Special Reference to Refractory Metals; Refractory Materials. A Series of Monographs; Academic Press Inc.: New York, NY, USA, 1970. [Google Scholar]
- Saunders, N.; Miodownik, A.P. CALPHAD (Calculation of Phase Diagrams): A Comprehensive Guide; Pergamon Materials Series; Elsevier: Pergamon, UK, 1998; Volume 1. [Google Scholar]
- Lukas, H.; Fries, S.G.; Sundman, B. Computational Thermodynamics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar] [CrossRef]
- Lukas, H.; Henig, E.; Zimmermann, B. Optimization of phase diagrams by a least squares method using simultaneously different types of data. Calphad 1977, 1, 225–236. [Google Scholar] [CrossRef]
- Spencer, P. A brief history of CALPHAD. Calphad 2008, 32, 1–8. [Google Scholar] [CrossRef]
- Gaye, H.; Lupis, C. Computer calculations of multicomponent phase diagrams. Scr. Metall. 1970, 4, 685–691. [Google Scholar] [CrossRef]
- Counsell, J.F.; Lees, E.B.; Spencer, P.J. An Original Method for the Determination of Equilibrium Diagrams in Multicomponent Systems by Means of a Digital Computer. Met. Sci. J. 1971, 5, 210–213. [Google Scholar] [CrossRef]
- Grafe, U.; Böttger, B.; Tiaden, J.; Fries, S. Coupling of multicomponent thermodynamic databases to a phase field model: Application to solidification and solid state transformations of superalloys. Scr. Mater. 2000, 42, 1179–1186. [Google Scholar] [CrossRef]
- Steinbach, I.; Böttger, B.; Eiken, J.; Warnken, N.; Fries, S.G. CALPHAD and Phase-Field Modeling: A Successful Liaison. J. Phase Equilibria Diffus. 2007, 28, 101–106. [Google Scholar] [CrossRef]
- Kitashima, T. Coupling of the phase-field and CALPHAD methods for predicting multicomponent, solid-state phase transformations. Philos. Mag. 2008, 88, 1615–1637. [Google Scholar] [CrossRef]
- Steinbach, I. Second Symposium on Phase-Field Modelling in Materials Science. Int. J. Mater. Res. 2010, 101, 455. [Google Scholar] [CrossRef]
- Kattner, U.R. The CALPHAD method and its role in material and process development. Tecnol. Metal. Mater. Min. 2016, 13, 3–15. [Google Scholar] [CrossRef]
- Xiong, W.; Grönhagen, K.A.; Ågren, J.; Selleby, M.; Odqvist, J.; Chen, Q. Investigation of Spinodal Decomposition in Fe-Cr Alloys: CALPHAD Modeling and Phase Field Simulation. Solid State Phenom. 2011, 172–174, 1060–1065. [Google Scholar] [CrossRef]
- Kobayashi, H.; Ode, M.; Kim, S.G.; Kim, W.T.; Suzuki, T. Phase-field model for solidification of ternary alloys coupled with thermodynamic database. Scr. Mater. 2003, 48, 689–694. [Google Scholar] [CrossRef]
- Shamsundar, N.; Sparrow, E.M. Analysis of Multidimensional Conduction Phase Change via the Enthalpy Model. J. Heat Transf. 1975, 97, 333–340. [Google Scholar] [CrossRef]
- Date, A.W. A novel enthalpy formulation for multidimensional solidification and melting of a pure substance. Sadhana 1994, 19, 833–850. [Google Scholar] [CrossRef]
- Qin, L.; Shen, J.; Li, Q.; Shang, Z. Effects of convection patterns on freckle formation of directionally solidified Nickel-based superalloy casting with abruptly varying cross-sections. J. Cryst. Growth 2017, 466, 45–55. [Google Scholar] [CrossRef]
- Wu, M.; Liu, L.; Ding, J. An enthalpy method based on fixed-grid for quasi-steady modeling of solidification/melting processes of pure materials. Int. J. Heat Mass Transf. 2017, 108, 1383–1392. [Google Scholar] [CrossRef]
- Sudnik, W.; Radaj, D.; Erofeew, W. Computerized simulation of laser beam welding, modelling and verification. J. Phys. D Appl. Phys. 1996, 29, 2811. [Google Scholar] [CrossRef]
- Duggan, G.; Mirihanage, W.U.; Tong, M.; Browne, D.J. A combined enthalpy/front tracking method for modelling melting and solidification in laser welding. IOP Conf. Ser. Mater. Sci. Eng. 2012, 33, 012026. [Google Scholar] [CrossRef]
- Farias, R.; Teixeira, P.; Vilarinho, L. An efficient computational approach for heat source optimization in numerical simulations of arc welding processes. J. Constr. Steel Res. 2021, 176, 106382. [Google Scholar] [CrossRef]
- Attar, E.; Körner, C. Lattice Boltzmann model for thermal free surface flows with liquid–solid phase transition. Int. J. Heat Fluid Flow 2011, 32, 156–163. [Google Scholar] [CrossRef]
- Xu, P.; Xu, S.; Gao, Y.; Liu, P. A multicomponent multiphase enthalpy-based lattice Boltzmann method for droplet solidification on cold surface with different wettability. Int. J. Heat Mass Transf. 2018, 127, 136–140. [Google Scholar] [CrossRef]
- Gu, H.; Wei, C.; Li, L.; Han, Q.; Setchi, R.; Ryan, M.; Li, Q. Multi-physics modelling of molten pool development and track formation in multi-track, multi-layer and multi-material selective laser melting. Int. J. Heat Mass Transf. 2020, 151, 119458. [Google Scholar] [CrossRef]
- Zakirov, A.; Belousov, S.; Bogdanova, M.; Korneev, B.; Stepanov, A.; Perepelkina, A.; Levchenko, V.; Meshkov, A.; Potapkin, B. Predictive modeling of laser and electron beam powder bed fusion additive manufacturing of metals at the mesoscale. Addit. Manuf. 2020, 35, 101236. [Google Scholar] [CrossRef]
- Küng, V.E.; Scherr, R.; Markl, M.; Körner, C. Multi-material model for the simulation of powder bed fusion additive manufacturing. Comput. Mater. Sci. 2021, 194, 110415. [Google Scholar] [CrossRef]
- Li, E.; Wang, L.; Yu, A.; Zhou, Z. A three-phase model for simulation of heat transfer and melt pool behaviour in laser powder bed fusion process. Powder Technol. 2021, 381, 298–312. [Google Scholar] [CrossRef]
- Collins, P.; Banerjee, R.; Fraser, H. The influence of the enthalpy of mixing during the laser deposition of complex titanium alloys using elemental blends. Scr. Mater. 2003, 48, 1445–1450. [Google Scholar] [CrossRef]
- Chouhan, A.; Hesselmann, M.; Toenjes, A.; Mädler, L.; Ellendt, N. Numerical modelling of in-situ alloying of Al and Cu using the laser powder bed fusion process: A study on the effect of energy density and remelting on deposited track homogeneity. Addit. Manuf. 2022, 59, 103179. [Google Scholar] [CrossRef]
- Ohsasa, K. Numerical simulation of solidification for aluminum-base multicomponent alloy. J. Phase Equilibria 2001, 22, 498–503. [Google Scholar] [CrossRef]
- Ohsasa, K.; Shirosawa, H. Heat Transfer Analysis for Solidifying Ferrous Multi-component Alloys Using Computational Thermodynamics. ISIJ Int. 2009, 49, 1715–1721. [Google Scholar] [CrossRef][Green Version]
- Saad, A.; Gandin, C.A.; Bellet, M. Temperature-based energy solver coupled with tabulated thermodynamic properties—Application to the prediction of macrosegregation in multicomponent alloys. Comput. Mater. Sci. 2015, 99, 221–231. [Google Scholar] [CrossRef]
- Srinivas Shastri, S.; Allen, R. Method of lines and enthalpy method for solving moving boundary problems. Int. Commun. Heat Mass Transf. 1998, 25, 531–540. [Google Scholar] [CrossRef]
- Stefan, J. Über die Verdampfung und die Auflösung als Vorgänge der Diffusion. Ann. Phys. Chem. 1890, 277, 725–747. [Google Scholar] [CrossRef]
- Meyer, G.H. The numerical solution of Stefan problems with front-tracking and smoothing methods. Appl. Math. Comput. 1978, 4, 283–306. [Google Scholar] [CrossRef]
- Douglas, J., Jr.; Gallie, T.M., Jr. On the numerical integration of a parabolic differential equation subject to a moving boundary condition. Duke Math. J. 1955, 22, 557–571. [Google Scholar] [CrossRef]
- Murray, W.D.; Landis, F. Numerical and Machine Solutions of Transient Heat-Conduction Problems Involving Melting or Freezing: Part I—Method of Analysis and Sample Solutions. J. Heat Transf. 1959, 81, 106–112. [Google Scholar] [CrossRef]
- Atthey, D.R. A Finite Difference Scheme for Melting Problems. IMA J. Appl. Math. 1974, 13, 353–366. [Google Scholar] [CrossRef]
- Meyer, G.H. Multidimensional Stefan Problems. SIAM J. Numer. Anal. 1973, 10, 522–538. [Google Scholar] [CrossRef]
- Hunter, L.W.; Kuttler, J.R. The Enthalpy Method for Heat Conduction Problems With Moving Boundaries. J. Heat Transf. 1989, 111, 239–242. [Google Scholar] [CrossRef]
- Tang, C.; Yao, L.; Du, H. Computational framework for the simulation of multi material laser powder bed fusion. Int. J. Heat Mass Transf. 2022, 191, 122855. [Google Scholar] [CrossRef]
- Andersson, J.O.; Helander, T.; Höglund, L.; Shi, P.; Sundman, B. Thermo-Calc & DICTRA, computational tools for materials science. Calphad 2002, 26, 273–312. [Google Scholar] [CrossRef]
- Foundation of Computational Thermodynamics. TC-Python API Reference Documentation 2024a, 1995–2024. Available online: https://download.thermocalc.com/docs/tc-python/2024a/html/ (accessed on 13 December 2023).
- Liepold, P. CALPHAD-Basiertes Materialmodell für die Simulation der Multi-Material-Verarbeitung im EB-PBF-Prozess. Master’s Thesis, Friedrich-Alexander-Universität Erlangen-Nürnberg, Erlangen, Germany, 2021. [Google Scholar]
- Peterson, W.W. Addressing for Random-Access Storage. IBM J. Res. Dev. 1957, 1, 130–146. [Google Scholar] [CrossRef]
- Markl, M.; Rausch, A.; Küng, V.; Körner, C. SAMPLE: A Software Suite to Predict Consolidation and Microstructure for Powder Bed Fusion Additive Manufacturing. Adv. Eng. Mater. 2019, 22, 1901270. [Google Scholar] [CrossRef]
- Scherr, R.; Markl, M.; Körner, C. Volume of fluid based modeling of thermocapillary flow applied to a free surface lattice Boltzmann method. J. Comput. Phys. 2023, 492, 112441. [Google Scholar] [CrossRef]
- Chen, S.; Doolen, G.D. Lattice Boltzmann Method for Fluid Flows. Annu. Rev. Fluid Mech. 1998, 30, 329–364. [Google Scholar] [CrossRef]
- Zhang, R.; Fan, H.; Chen, H. A lattice Boltzmann approach for solving scalar transport equations. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2011, 369, 2264–2273. [Google Scholar] [CrossRef]
- Cook, A.W. Enthalpy diffusion in multicomponent flows. Phys. Fluids 2009, 21, 055109. [Google Scholar] [CrossRef]
- Osmanlic, F.; Körner, C. Lattice Boltzmann method for Oldroyd-B fluids. Comput. Fluids 2016, 124, 190–196. [Google Scholar] [CrossRef]
- Roache, P.J. Computational Fluid Dynamics, 1st ed.; Hermosa Publishers: Socorro, NM, USA, 1972. [Google Scholar]
- Huang, X.; Wang, L.; Jia, S.; Qian, Z.; Deng, J.; Shi, Z. Numerical Simulation of Thermal Characteristics of Anodes by Pure Metal and CuCr Alloy Material in Vacuum Arc. IEEE Trans. Plasma Sci. 2015, 43, 2283–2293. [Google Scholar] [CrossRef]
- Turchanin, M.; Nikolaenko, I. Enthalpies of solution of vanadium and chromium in liquid copper by high temperature calorimetry. J. Alloys Compd. 1996, 235, 128–132. [Google Scholar] [CrossRef]
- Turchanin, M.A. Phase equilibria and thermodynamics of binary copper systems with 3d-metals. III. Copper-chromium system. Powder Metall. Met. Ceram. 2006, 45, 457–467. [Google Scholar] [CrossRef]
- Alexiades, V.; Solomon, A.D. Mathematical Modeling of Melting and Freezing Processes; Routledge: London, UK, 2018. [Google Scholar] [CrossRef]
- Guo, D.Z.; Sun, D.L.; Li, Z.Y.; Tao, W.Q. Phase Change Heat Transfer Simulation for Boiling Bubbles Arising from a Vapor Film by the VOSET Method. Numer. Heat Transf. Part A Appl. 2011, 59, 857–881. [Google Scholar] [CrossRef]
- Ho, C.Y.; Powell, R.W.; Liley, P.E. Thermal Conductivity of the Elements. J. Phys. Chem. Ref. Data 1972, 1, 279–421. [Google Scholar] [CrossRef]
- Dinsdale, A. SGTE data for pure elements. Calphad 1991, 15, 317–425. [Google Scholar] [CrossRef]
- Klassen, A.; Bauereiß, A.; Körner, C. Modelling of electron beam absorption in complex geometries. J. Phys. D Appl. Phys. 2014, 47, 065307. [Google Scholar] [CrossRef]
- Breuning, C.; Arnold, C.; Markl, M.; Körner, C. A multivariate meltpool stability criterion for fabrication of complex geometries in electron beam powder bed fusion. Addit. Manuf. 2021, 45, 102051. [Google Scholar] [CrossRef]
- Pistor, J.; Breuning, C.; Körner, C. A Single Crystal Process Window for Electron Beam Powder Bed Fusion Additive Manufacturing of a CMSX-4 Type Ni-Based Superalloy. Materials 2021, 14, 3785. [Google Scholar] [CrossRef] [PubMed]






| Alloy | Quantity | T in K | in wt.% | in K | in wt.% |
|---|---|---|---|---|---|
| CuCr | 200–5000 | 0–100 | 2.4 | 1 | |
| 1300–2300 | 0–100 | 0.5 | 1 | ||
| TiAl | 200–5000 | 0–100 | 2.4 | 1 | |
| 900–2000 | 0–100 | 0.55 | 1 |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Diffusion coefficient | D | ||
| Thermal conductivity | 100 | W/(mK) | |
| Density | 5000 | ||
| Ambient temperature | 2400 | K | |
| Peak temperature | 2700 | K | |
| Standard deviation | 15 | µm | |
| Cell size | 2 | µm | |
| Time step (LB) | 20 | ns | |
| Time step (FD) | 5 | ns |
| Quantity | Powder Layer Configurations | |||||
|---|---|---|---|---|---|---|
| #1 | #2 | #3 | #4 | #5 | ||
| (t = 4 ms) (K) | − | 1561 | 1554 | 1551 | 1546 | 1554 |
| ✓ | 1630 | 1638 | 1599 | 1590 | 1625 | |
| () | − | 0.0254 | 0.0243 | 0.0238 | 0.0260 | 0.0253 |
| ✓ | 0.0279 | 0.0274 | 0.0256 | 0.0268 | 0.0290 | |
| (ms) | − | 2.16 | 1.19 | 1.31 | 1.26 | 1.30 |
| ✓ | 3.31 | 2.02 | 2.11 | 2.65 | 2.41 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scherr, R.; Liepold, P.; Markl, M.; Körner, C. A CALPHAD-Informed Enthalpy Method for Multicomponent Alloy Systems with Phase Transitions. Modelling 2024, 5, 367-391. https://doi.org/10.3390/modelling5010020
Scherr R, Liepold P, Markl M, Körner C. A CALPHAD-Informed Enthalpy Method for Multicomponent Alloy Systems with Phase Transitions. Modelling. 2024; 5(1):367-391. https://doi.org/10.3390/modelling5010020
Chicago/Turabian StyleScherr, Robert, Philipp Liepold, Matthias Markl, and Carolin Körner. 2024. "A CALPHAD-Informed Enthalpy Method for Multicomponent Alloy Systems with Phase Transitions" Modelling 5, no. 1: 367-391. https://doi.org/10.3390/modelling5010020
APA StyleScherr, R., Liepold, P., Markl, M., & Körner, C. (2024). A CALPHAD-Informed Enthalpy Method for Multicomponent Alloy Systems with Phase Transitions. Modelling, 5(1), 367-391. https://doi.org/10.3390/modelling5010020








