Simulating Nearshore Wave Processes Utilizing an Enhanced Boussinesq-Type Model
Abstract
:1. Introduction
2. Theoretical Background
2.1. Basic Equations
2.2. Irregular Multidirectional Wave Generation
2.3. Calculation of Wave Overtopping Discharges
- A “simplified parametric” methodology, where averaging of the wave surface elevation takes place before the initiation of wave reflection at the structure’s face occurs.
- A “reference domain” simulation, in which the presence of the structure is omitted and an indicative simulation is executed to extract the wave characteristics at the position where the structure’s toe is to be located.
3. Model Verification
3.1. Wave Breaking on a Plane Sloping Beach (Mase and Kirby, 1992)
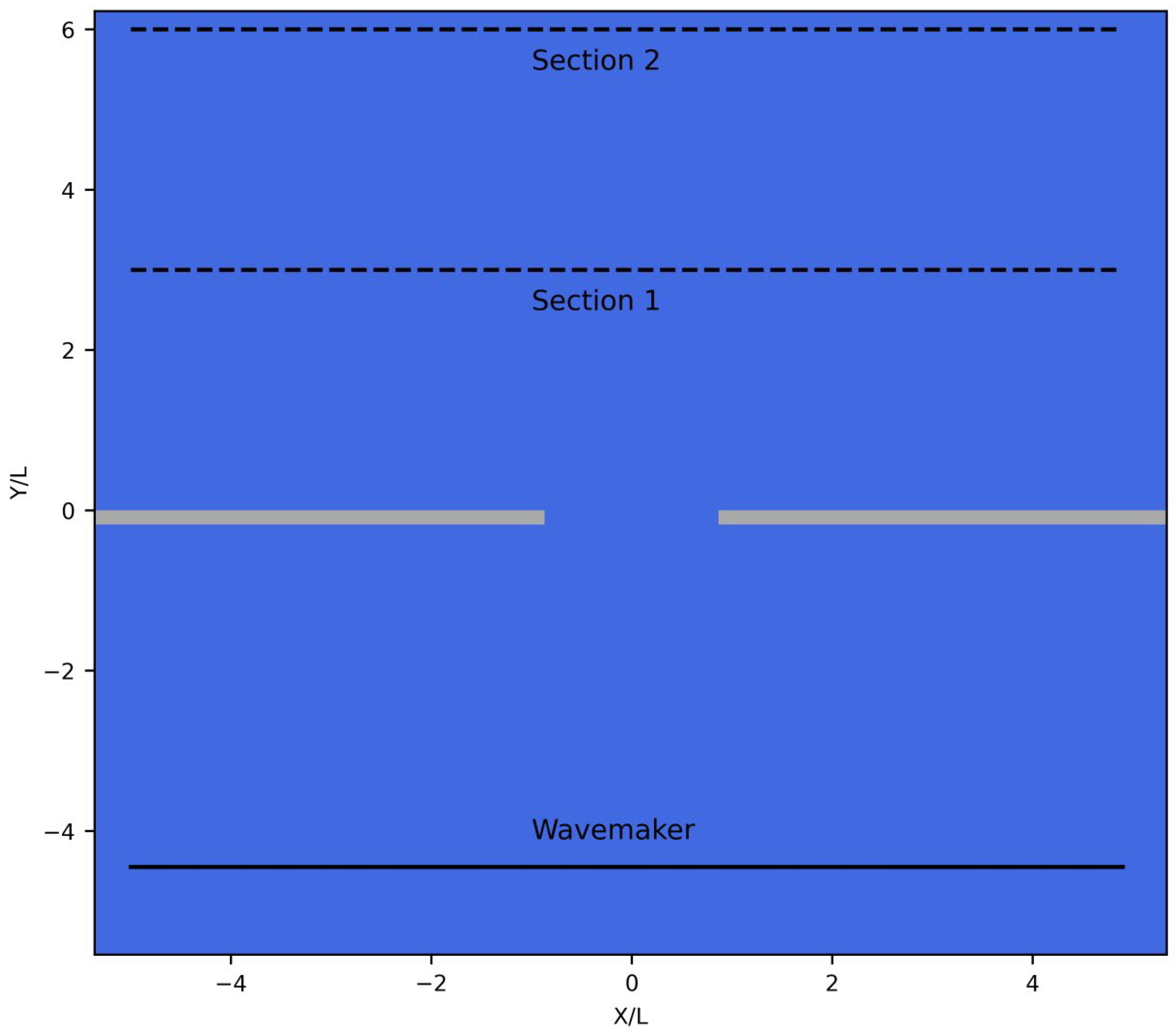
3.2. Irregular Wave Diffraction through a Breakwater Gap (Yu et al., 2000)
3.3. Wave Overtopping on a Smooth Sloping or Vertical Breakwater (Williams et al., 2019)
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Holthuijsen, L.H. Waves in Oceanic and Coastal Waters; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Pachang, V.; Wei, G.; Pearce, R.; Briggs, M. Numerical simulation of irregular wave propagation over a shoal. J. Waterw. Port. Coast. Ocean. Eng. 1990, 116, 324–340. [Google Scholar] [CrossRef]
- Chawla, A.; Özkan-Haller, H.T.; Kirby, J.T. Spectral Model for Wave Transformation and Breaking over Irregular Bathymetry. J. Waterw. Port Coastal Ocean Eng. 1998, 124, 189–198. [Google Scholar] [CrossRef]
- Chondros, M.; Metallinos, A.; Memos, C.; Karambas, T.; Papadimitriou, A. Concerted nonlinear mild-slope wave models for enhanced simulation of coastal processes. Appl. Math. Model. 2021, 91, 508–529. [Google Scholar] [CrossRef]
- Peregrine, D.H. Long waves on a beach. J. Fluid Mech. 1967, 27, 815–827. [Google Scholar] [CrossRef]
- Wei, G.; Kirby, J. Time-Dependent Numerical Code for Extended Boussinesq Equations. J. Waterw. Port. Coast. Ocean. Eng. 1995, 121, 251–261. [Google Scholar] [CrossRef]
- Karambas, T.V.; Memos, C.D. Boussinesq Model for Weakly Nonlinear Fully Dispersive Water Waves. J. Waterw. Port Coast. Ocean Eng. 2009, 135, 187–199. [Google Scholar] [CrossRef]
- Li, B. Wave Equations for Regular and Irregular Water Wave Propagation. J. Waterw. Port Coast. Ocean Eng. 2008, 134, 121–142. [Google Scholar] [CrossRef]
- Serre, F. Contribution à l’étude des écoulements permanents et variables dans les canaux. La Houille Blanche 1953, 39, 374–388. [Google Scholar] [CrossRef] [Green Version]
- Green, A.E.; Naghdi, P.M. A derivation of equations for wave propagation in water of variable depth. J. Fluid Mech. 1976, 78, 237–246. [Google Scholar] [CrossRef]
- Zelt, J. The run-up of nonbreaking and breaking solitary waves. Coast. Eng. 1991, 15, 205–246. [Google Scholar] [CrossRef]
- Karambas, T.; Koutitas, C. A breaking wave propagation model based on the Boussinesq equations. Coast. Eng. 1992, 18, 1–19. [Google Scholar] [CrossRef]
- Schäffer, H.A.; Madsen, P.A.; Deigaard, R. A Boussinesq model for waves breaking in shallow water. Coast. Eng. 1993, 20, 185–202. [Google Scholar] [CrossRef]
- Madsen, P.; Sørensen, O.; Schäffer, H. Surf zone dynamics simulated by a Boussinesq type model. Part I. Model description and cross-shore motion of regular waves. Coast. Eng. 1997, 32, 255–287. [Google Scholar] [CrossRef]
- Nwogu, O. Alternative Form of Boussinesq Equations for Nearshore Wave Propagation. J. Waterw. Port Coast. Ocean Eng. 1993, 119, 618–638. [Google Scholar] [CrossRef] [Green Version]
- Madsen, P.A.; Schäffer, H.A. Higher–Order Boussinesq–Type equations for surface gravity waves: Derivation and analysis. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1998, 356, 3123–3181. [Google Scholar] [CrossRef]
- Schäffer, H.A. Another step towards a post-Boussinesq wave model. In Proceedings of the 29th International Conference on Coastal Engineering, Lisbon, Portugal, 19–24 September 2004. [Google Scholar]
- Chondros, M.K.; Memos, C.D. A 2DH nonlinear Boussinesq-type wave model of improved dispersion, shoaling, and wave generation characteristics. Coast. Eng. 2014, 91, 99–122. [Google Scholar] [CrossRef]
- Metallinos, A.S.; Klonaris, G.; Memos, C.D.; Dimas, A. Hydrodynamic conditions in a submerged porous breakwater. Ocean Eng. 2019, 172, 712–725. [Google Scholar] [CrossRef]
- Cruz, E.; Isobe, M.; Watanabe, A. Boussinesq equations for wave transformation on porous beds. Coast. Eng. 1997, 30, 125–156. [Google Scholar] [CrossRef]
- Van Gent, M.R.A. Wave Interaction with Permeable Coastal Structures. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 12 December 1995. [Google Scholar]
- Sollitt, C.K.; Cross, R.H. Wave transmission through permeable breakwaters. In Proceedings of the 13th International Conference on Coastal Engineering, Vancouver, Canada, 10–14 July 1972. [Google Scholar]
- Losada, I.; Losada, M.; Martín, F. Experimental study of wave-induced flow in a porous structure. Coast. Eng. 1995, 26, 77–98. [Google Scholar] [CrossRef]
- Hsiao, S.-C.; Liu, P.; Chen, Y. Nonlinear water waves propagating over a permeable bed. Proc. R. Soc. Lond. A 2002, 458, 1291–1322. [Google Scholar] [CrossRef]
- Kennedy, A.; Chen, Q.; Kirby, J.T.; Dalrymple, R. Boussinesq Modeling of Wave Transformation, Breaking, and Runup. I: 1D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 39–47. [Google Scholar] [CrossRef] [Green Version]
- Chen, Q.; Kirby, J.; Dalrymple, R.; Kennedy, A.; Chawla, A. Boussinesq Modeling of Wave Transformation, Breaking, and Runup. II: 2D. J. Waterw. Port. Coast. Ocean. Eng. 2000, 126, 48–56. [Google Scholar] [CrossRef]
- Yu, Y.X.; Liu, S.X.; Li, L. Numerical simulation of multi-directional waves. In Proceedings of the International Society of Offshore and Polar Engineers Conference, Edinburgh, UK, 11–16 August 1991. [Google Scholar]
- Bouws, E.; Günther, H.; Rosenthal, W.; Vincent, C.L. Similarity of the wind wave spectrum in finite depth water: 1. Spectral form. J. Geophys. Res. Space Phys. 1985, 90, 975–986. [Google Scholar] [CrossRef]
- Goda, Y. Random Seas and Design of Maritime Structures, 2nd ed.; Advanced Series on Ocean Engineering; World Scientific Publishing Company: Singapore,, 2000; p. 464. [Google Scholar]
- EurOtop. Manual on Wave Overtopping of Sea Defences and Related Structures, 2nd ed.; EurOtop: Brussels, Belgium, 2018; p. 304. [Google Scholar]
- Besley, P.; Stewart, T.; Allsop, N.W.H. Overtopping of Vertical Structures: New Prediction Methods to Account for Shallow Water Conditions. In Coastlines, Structures and Breakwaters; Thomas Telford Ltd.: London, UK, 1998; pp. 46–57. [Google Scholar]
- Mase, H.; Kirby, J. Hybrid Frequency-Domain KdV Equation for Random Wave Transformation. In Proceedings of the 23rd International Conference on Coastal Engineering, Venice, Italy, 4–9 October 1992. [Google Scholar]
- Vincent, C.L.; Briggs, M.J. Refraction—Diffraction of Irregular Waves over a Mound. J. Waterw. Port Coast. Ocean Eng. 1989, 115, 269–284. [Google Scholar] [CrossRef]
- Yu, Y.-X.; Liu, S.-X.; Li, Y.; Wai, O.W. Refraction and diffraction of random waves through breakwater. Ocean Eng. 2000, 27, 489–509. [Google Scholar] [CrossRef]
- Williams, H.E.; Briganti, R.; Romano, A.; Dodd, N. Experimental Analysis of Wave Overtopping: A New Small Scale Laboratory Dataset for the Assessment of Uncertainty for Smooth Sloped and Vertical Coastal Structures. J. Mar. Sci. Eng. 2019, 7, 217. [Google Scholar] [CrossRef] [Green Version]
- Shi, F.; Dalrymple, R.; Kirby, J.; Chen, Q.; Kennedy, A. A fully nonlinear Boussinesq model in generalized curvilinear coor-dinates. Coast. Eng. 2001, 42, 337–358. [Google Scholar] [CrossRef]
- David, C.G.; Roeber, V.; Goseberg, N.; Schlurmann, T. Generation and propagation of ship-borne waves-Solutions from a Boussinesq-type model. Coast. Eng. 2017, 127, 170–187. [Google Scholar] [CrossRef] [Green Version]
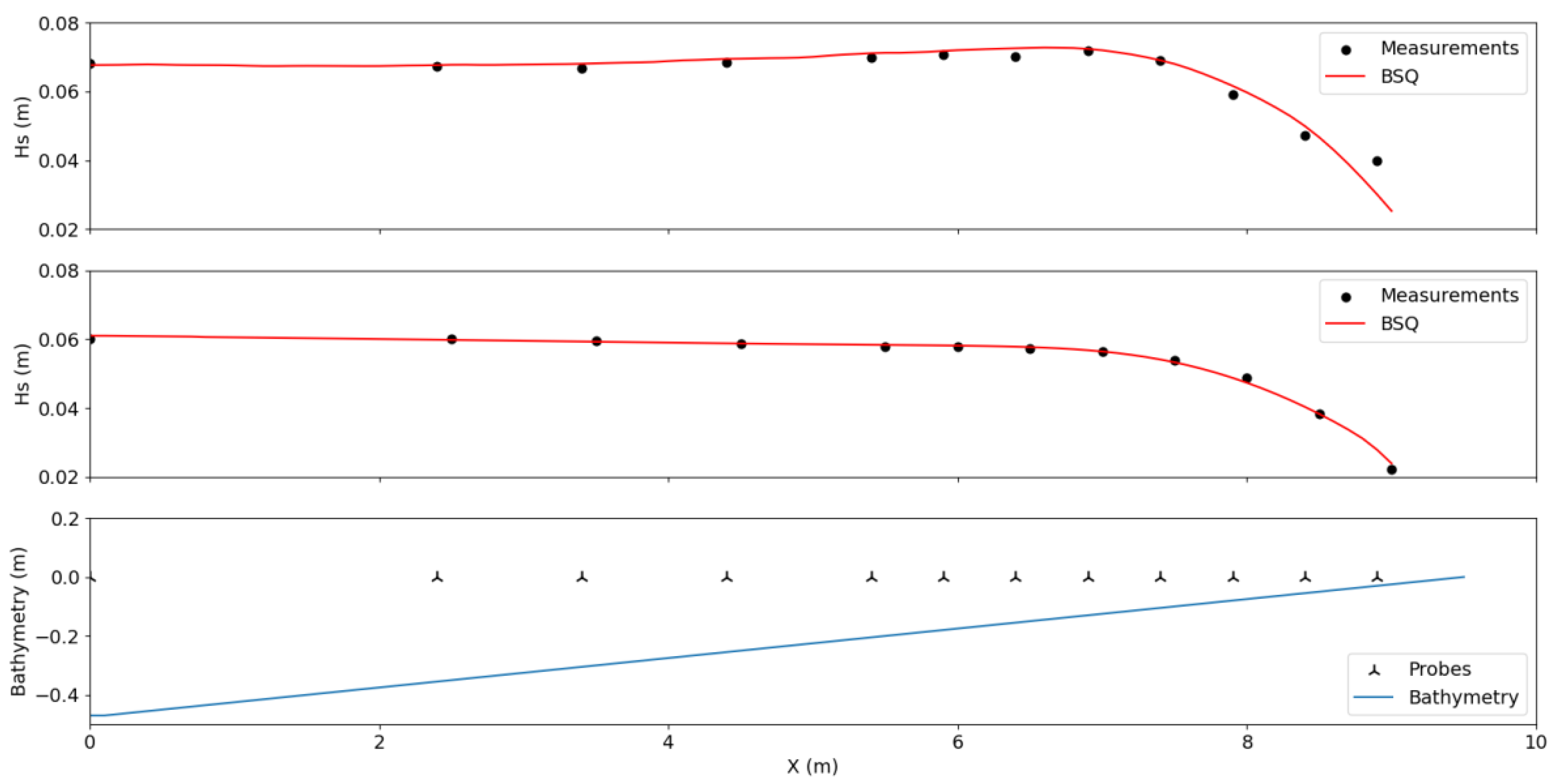

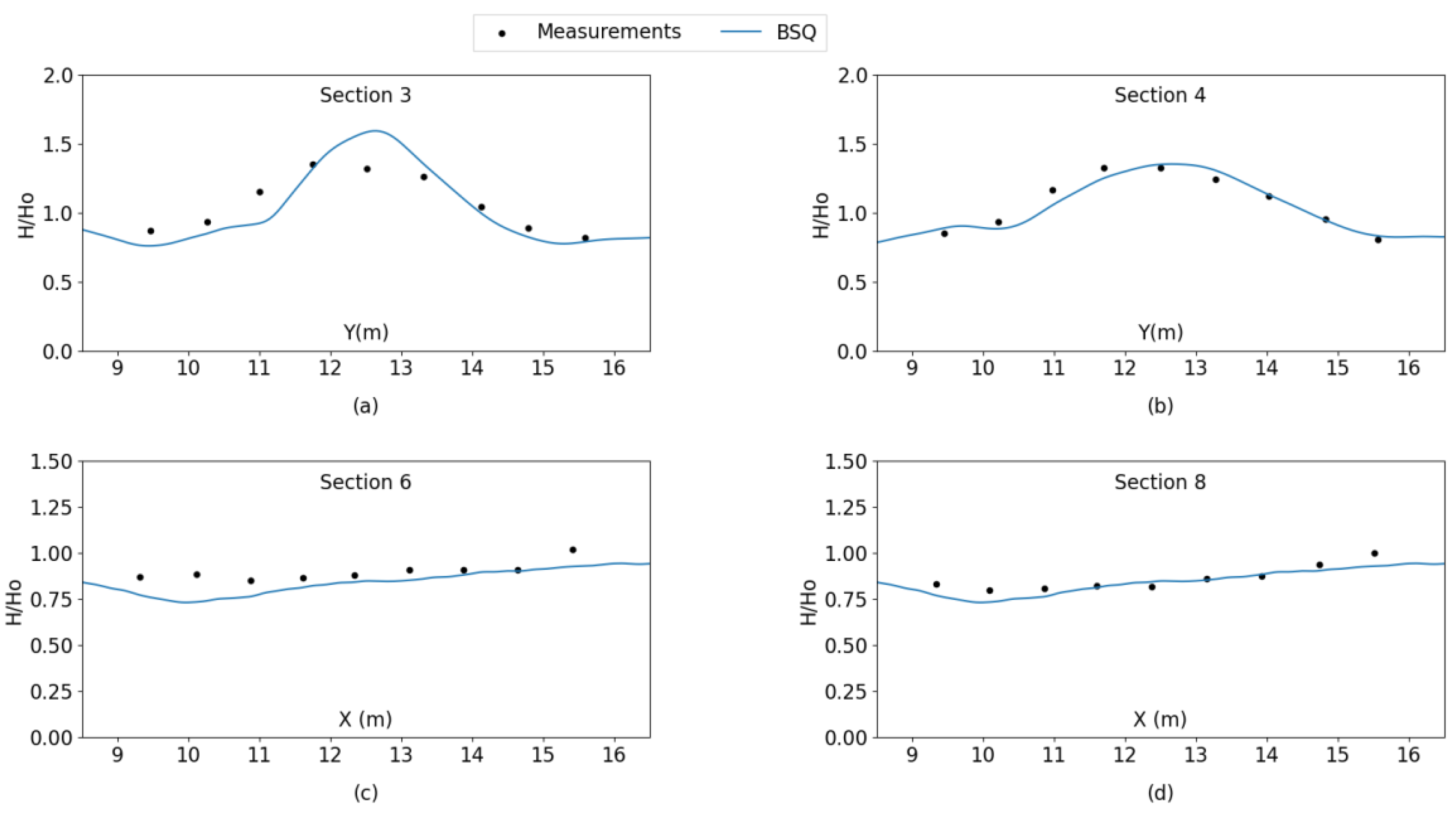
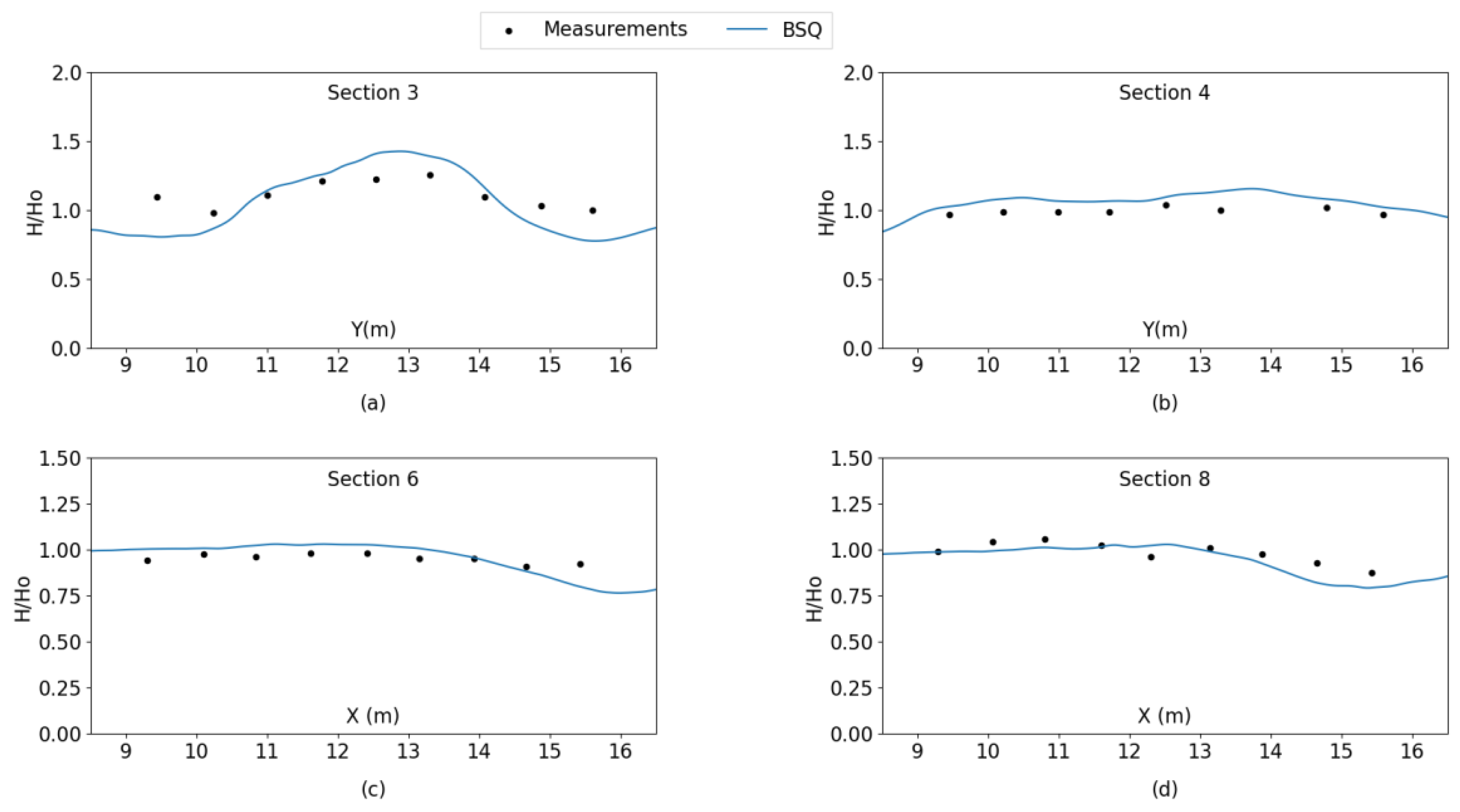
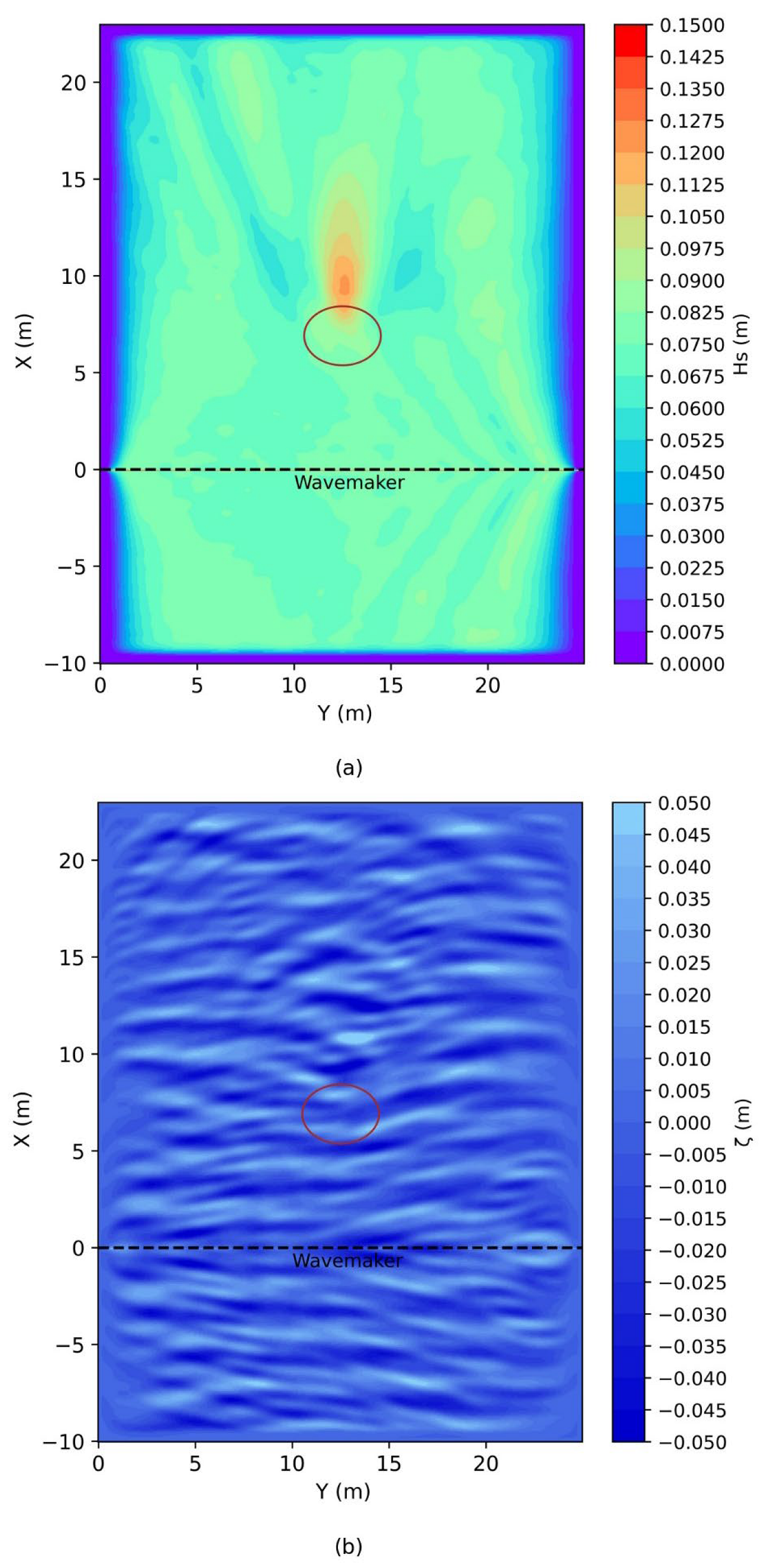



| Case | Ho (cm) | Tp (s) | α | Γ | σ (˚) |
|---|---|---|---|---|---|
| N1 | 7.75 | 1.30 | 0.0144 | 2 | 10 |
| B1 | 7.75 | 1.30 | 0.0144 | 2 | 30 |
| Case | α | Γ | s | |||
|---|---|---|---|---|---|---|
| U1 | 5.0 | 1.20 | 90 | 0.0081 | 4 | - |
| N1 | 5.0 | 1.20 | 90 | 0.0081 | 4 | 19 |
| B1 | 5.0 | 1.20 | 90 | 0.0081 | 4 | 6 |
| U2 | 5.0 | 1.20 | 45 | 0.0081 | 4 | - |
| N2 | 5.0 | 1.20 | 45 | 0.0081 | 4 | 19 |
| B2 | 5.0 | 1.20 | 45 | 0.0081 | 4 | 6 |
| Case | Structure Type | Hmo,i (cm) | Tp (s) | Rc (cm) | Rc/Hmo,i (-) |
|---|---|---|---|---|---|
| TS01-SS | Smooth Sloping | 6.0 | 1.01 | 6.0 | 1.00 |
| TS05-SS | Smooth Sloping | 5.0 | 0.93 | 6.0 | 1.20 |
| TS02-SS | Smooth Sloping | 4.0 | 0.86 | 6.0 | 1.50 |
| TS03-SS | Smooth Sloping | 3.0 | 0.70 | 6.0 | 2.00 |
| TS01-VW | Vertical Wall | 6.0 | 1.01 | 6.0 | 1.00 |
| TS07-VW | Vertical Wall | 5.0 | 1.24 | 6.0 | 1.20 |
| TS05-VW | Vertical Wall | 5.0 | 0.93 | 6.0 | 1.20 |
| TS02-VW | Vertical Wall | 4.0 | 0.86 | 6.0 | 1.50 |
| Case | Hmo,m (cm) | Hmo,c (cm) | Hmo,diff (%) | Q*,m (-) | Q*,c (cm) | Q*,diff (%) |
|---|---|---|---|---|---|---|
| TS01-SS | 4.30 | 4.36 | 1.44% | 0.008655 | 0.006929 | 19.94% |
| TS05-SS | 3.80 | 3.92 | 3.22% | 0.005362 | 0.004739 | 11.62% |
| TS02-SS | 3.20 | 3.33 | 4.06% | 0.00233 | 0.002358 | 1.20% |
| TS03-SS | 2.00 | 1.98 | 1.17% | 0.000543 | 0.000069 | 87.30% |
| TS01-VW | 4.30 | 4.24 | 1.42% | 0.004571 | 0.00098 | 78.62% |
| TS07-VW | 4.00 | 4.07 | 1.70% | 0.004268 | 0.00083 | 80.59% |
| TS05-VW | 3.80 | 3.72 | 2.11% | 0.002192 | 0.00056 | 74.24% |
| TS02-VW | 3.20 | 3.22 | 0.51% | 0.00082 | 0.00028 | 65.91% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Metallinos, A.; Chondros, M.; Papadimitriou, A. Simulating Nearshore Wave Processes Utilizing an Enhanced Boussinesq-Type Model. Modelling 2021, 2, 686-705. https://doi.org/10.3390/modelling2040037
Metallinos A, Chondros M, Papadimitriou A. Simulating Nearshore Wave Processes Utilizing an Enhanced Boussinesq-Type Model. Modelling. 2021; 2(4):686-705. https://doi.org/10.3390/modelling2040037
Chicago/Turabian StyleMetallinos, Anastasios, Michalis Chondros, and Andreas Papadimitriou. 2021. "Simulating Nearshore Wave Processes Utilizing an Enhanced Boussinesq-Type Model" Modelling 2, no. 4: 686-705. https://doi.org/10.3390/modelling2040037
APA StyleMetallinos, A., Chondros, M., & Papadimitriou, A. (2021). Simulating Nearshore Wave Processes Utilizing an Enhanced Boussinesq-Type Model. Modelling, 2(4), 686-705. https://doi.org/10.3390/modelling2040037






