Study of Superoscillating Functions Application to Overcome the Diffraction Limit with Suppressed Sidelobes
Abstract
:1. Introduction
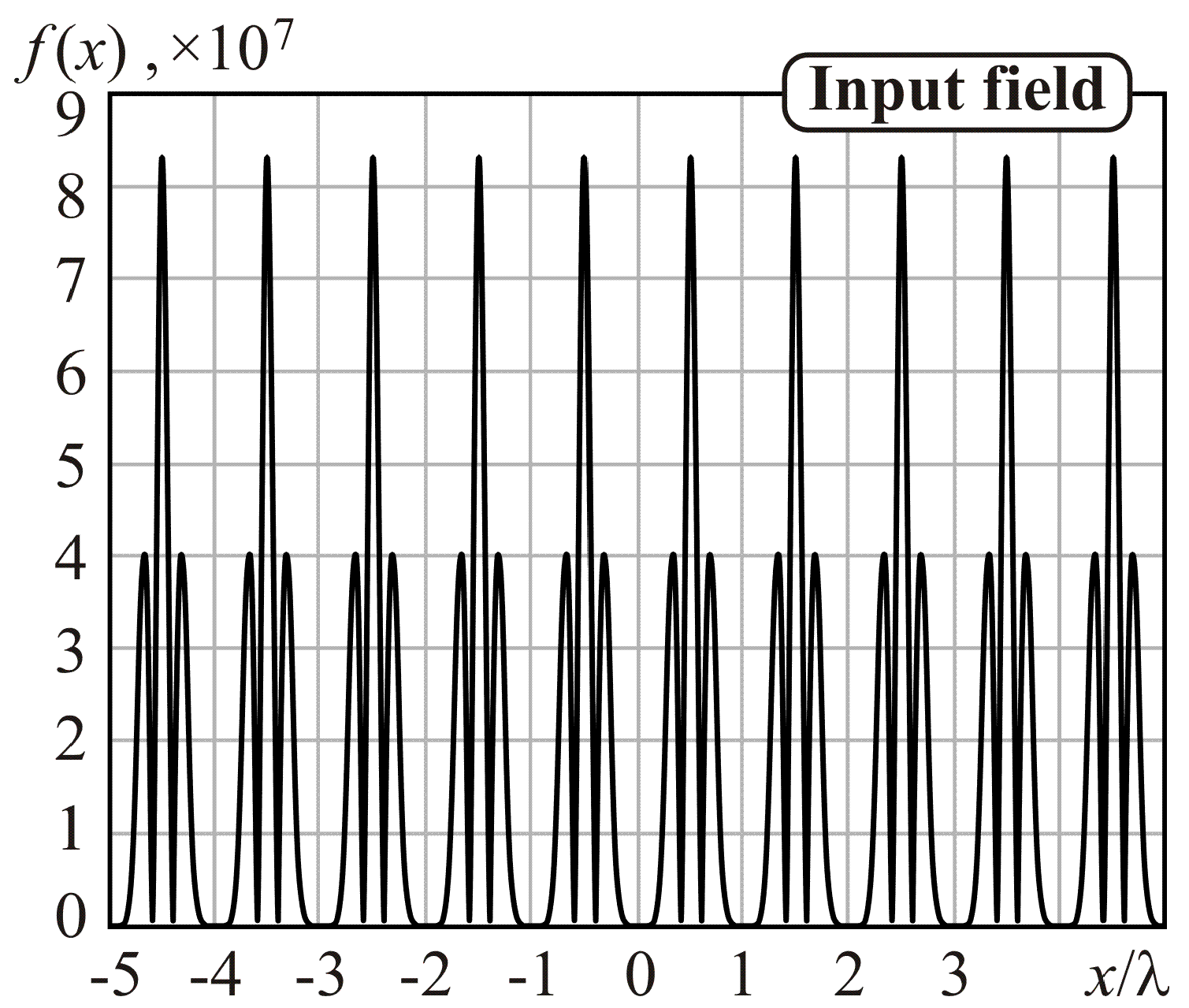
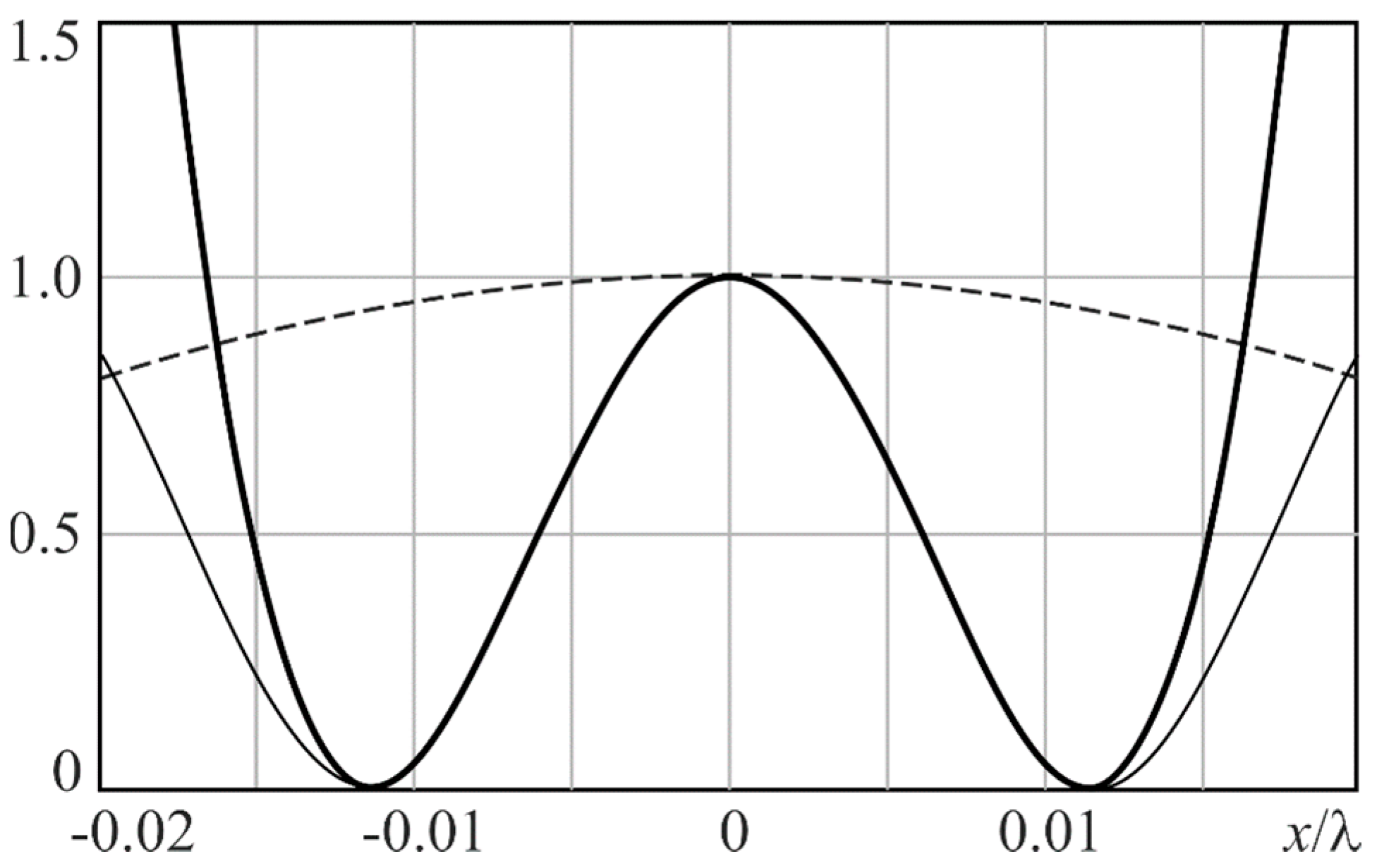
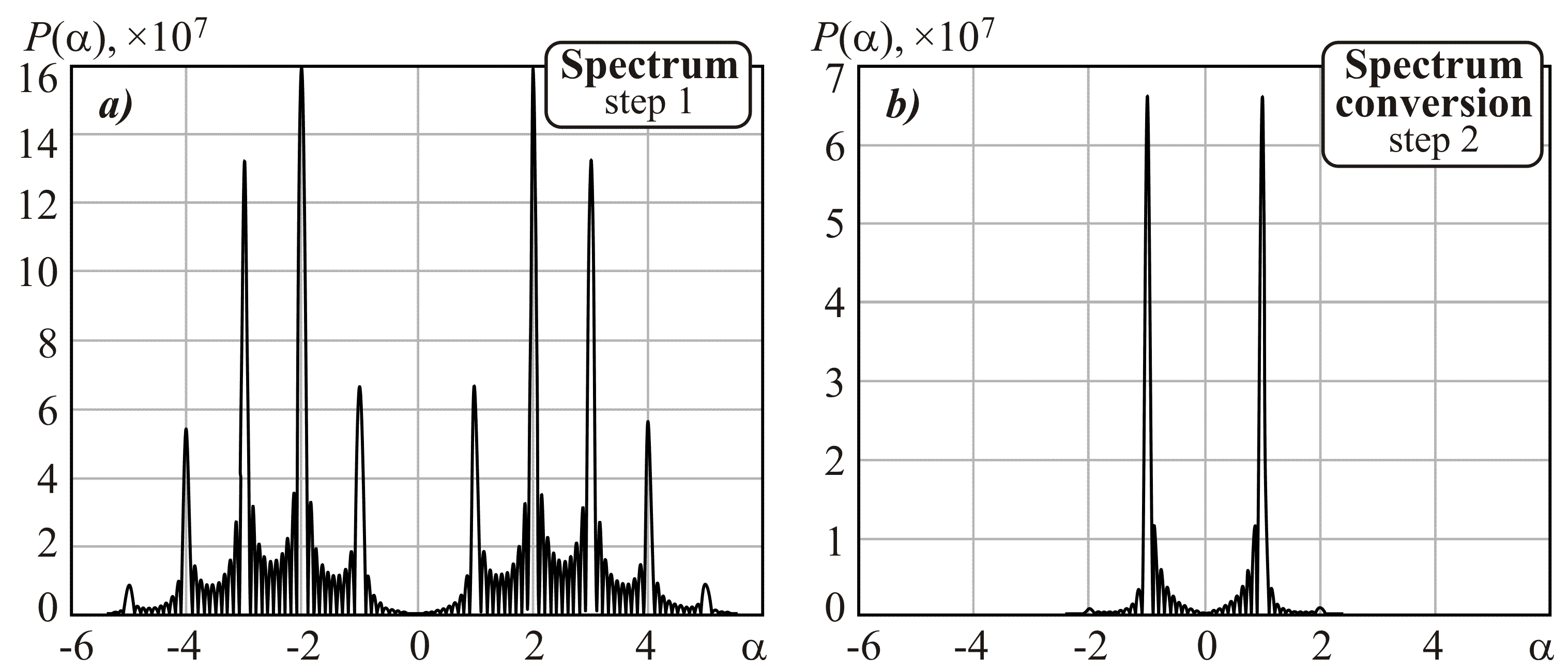
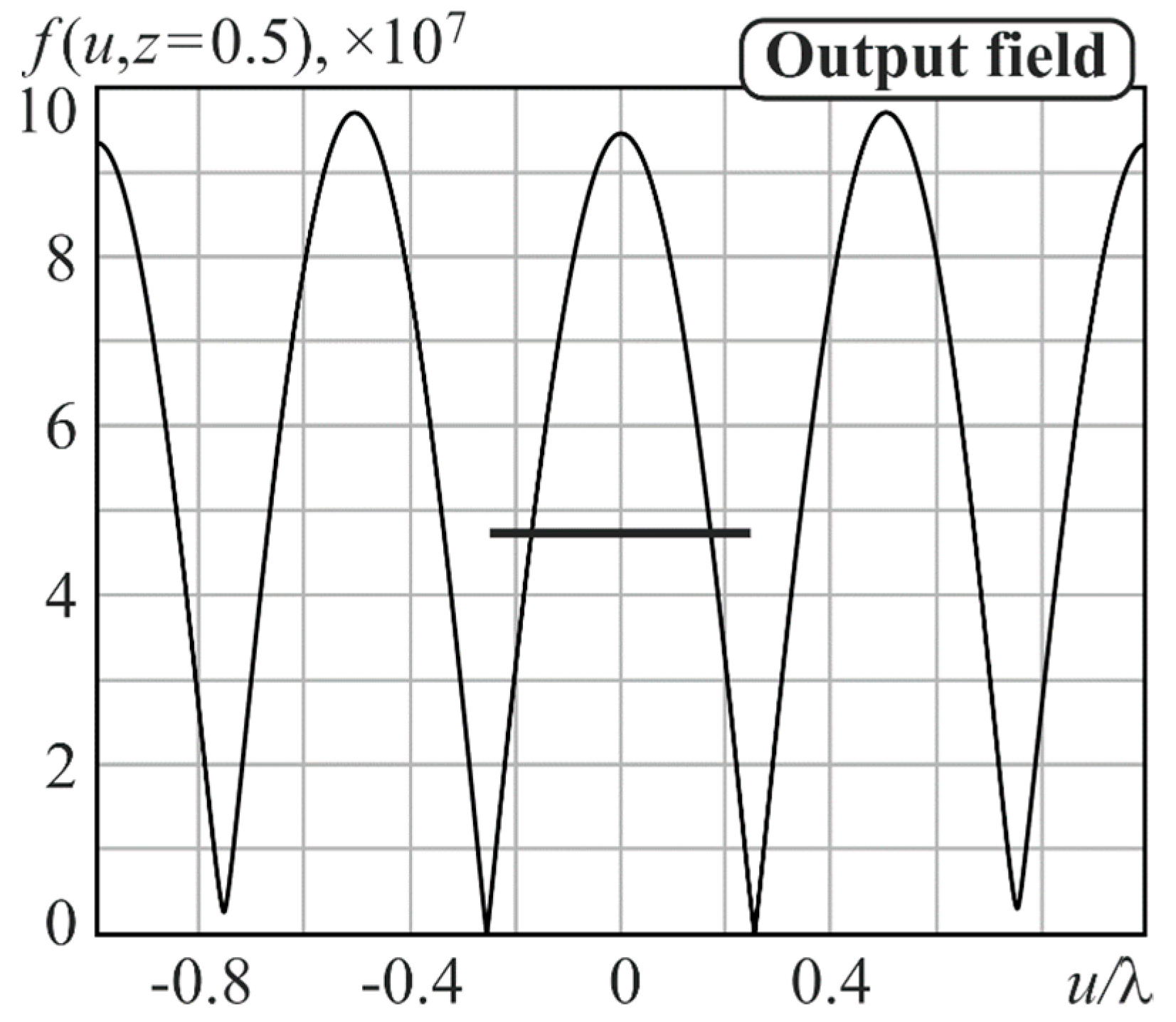
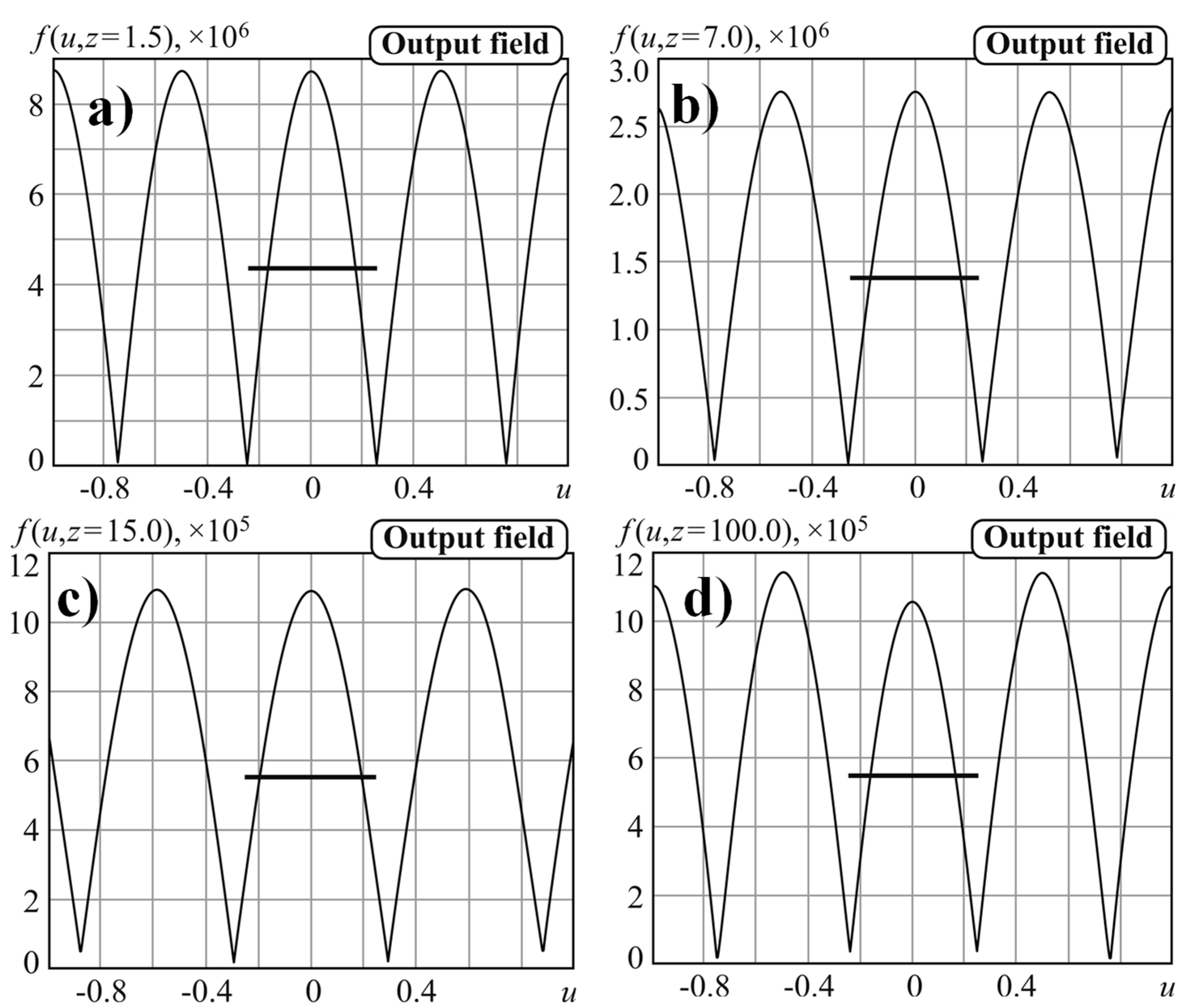
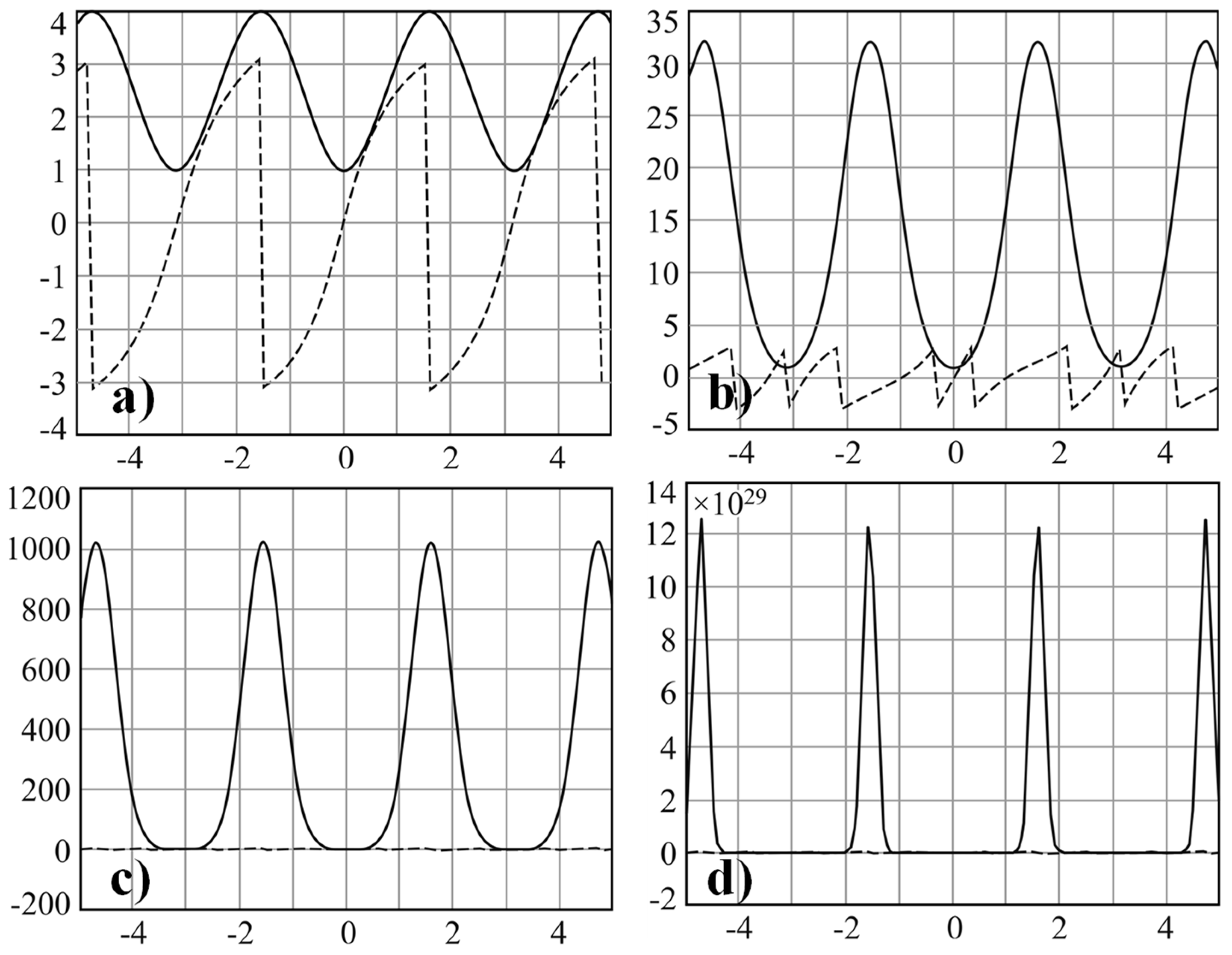
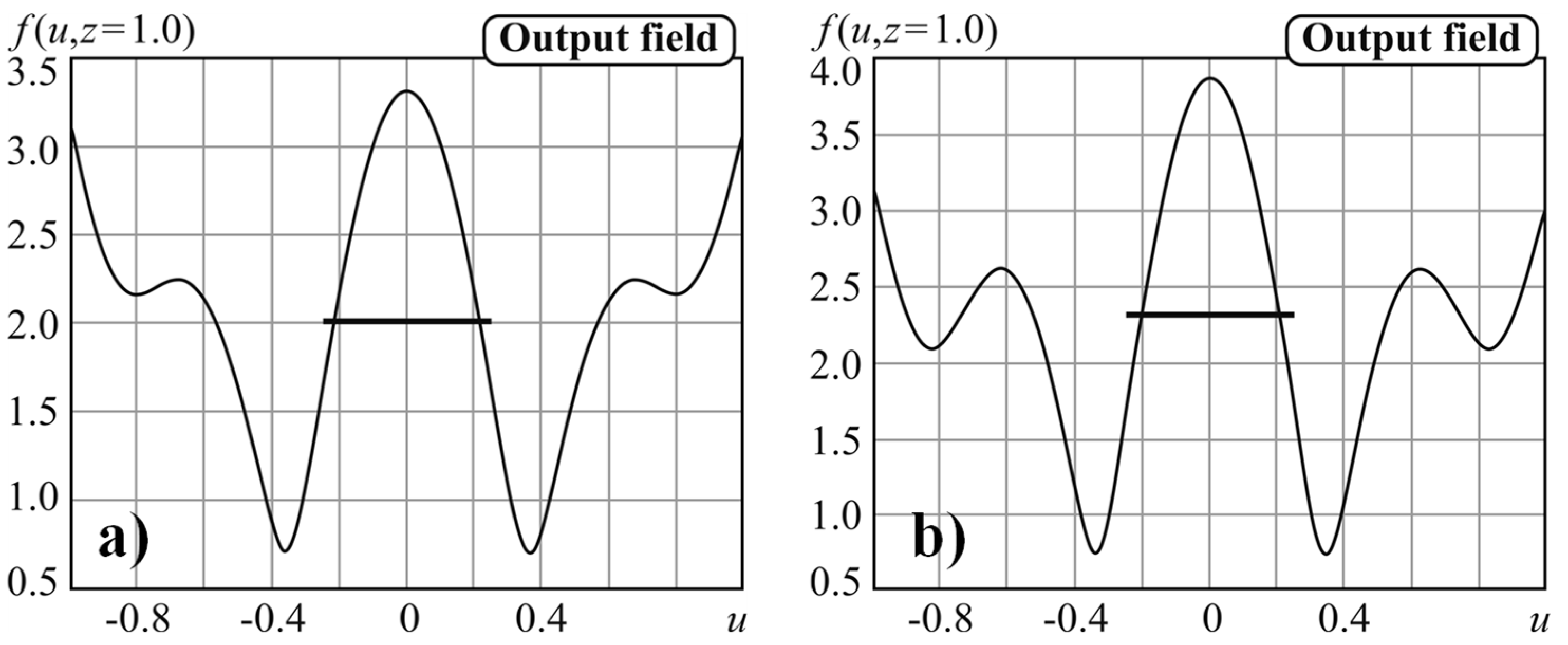
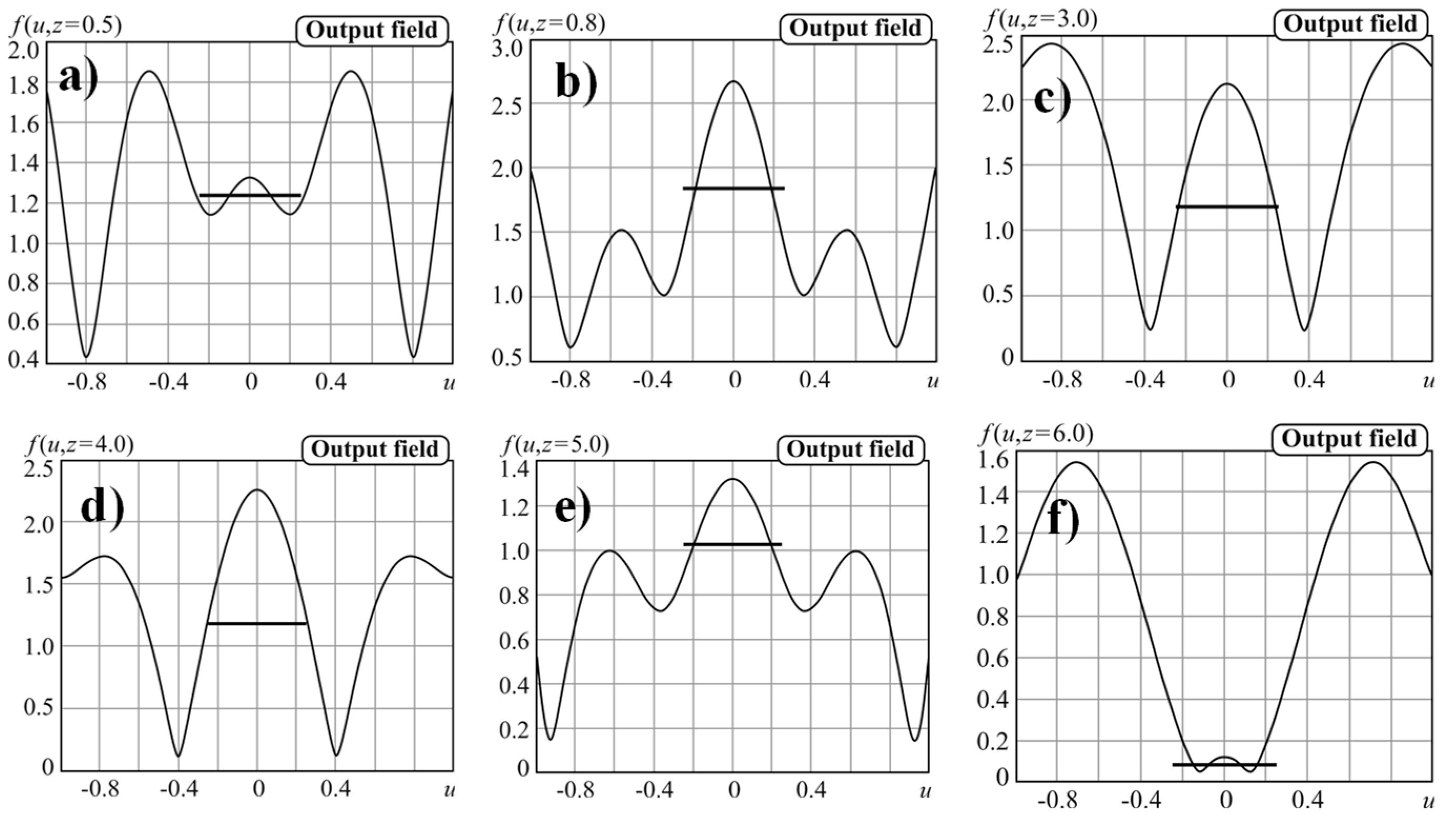
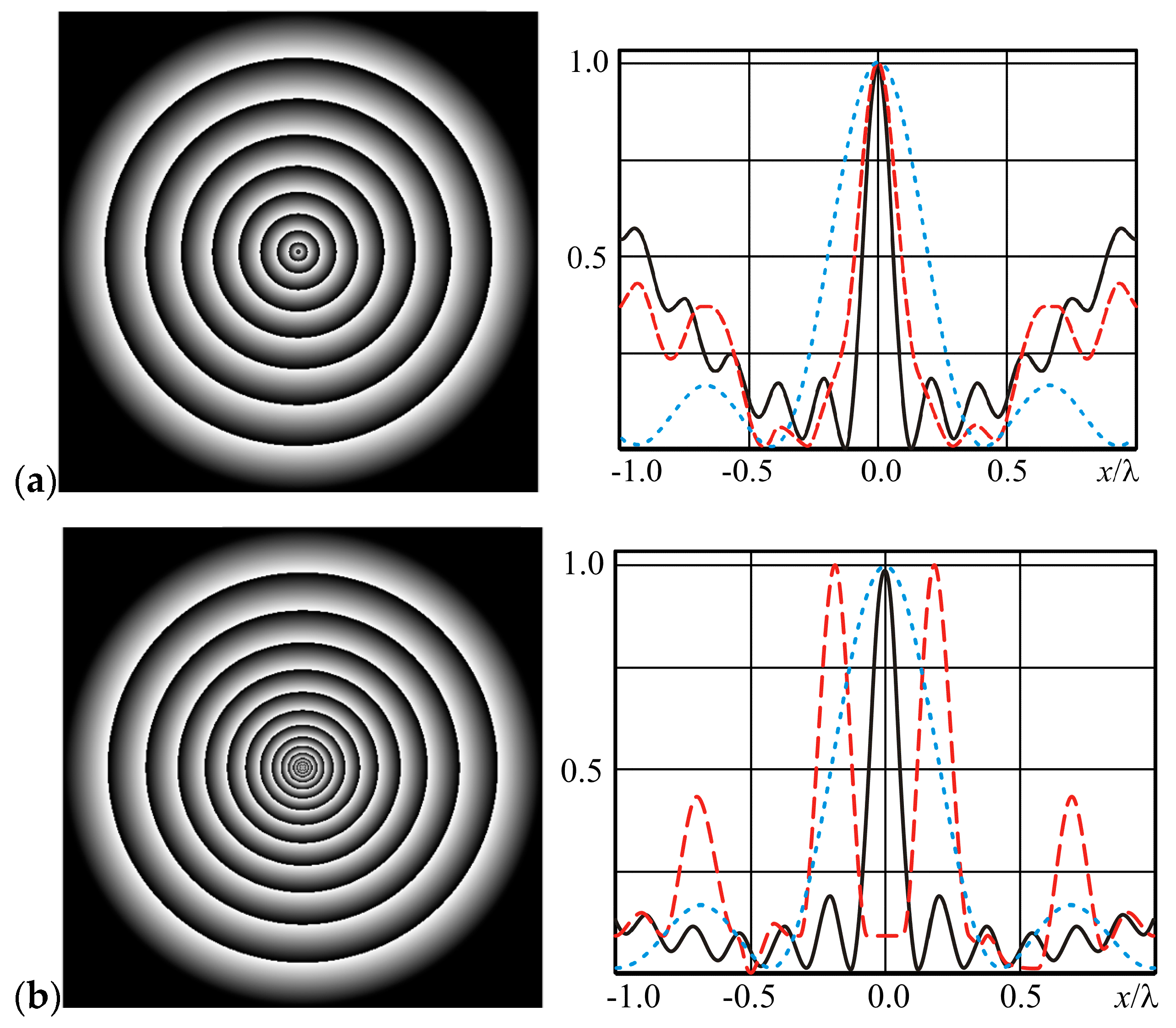
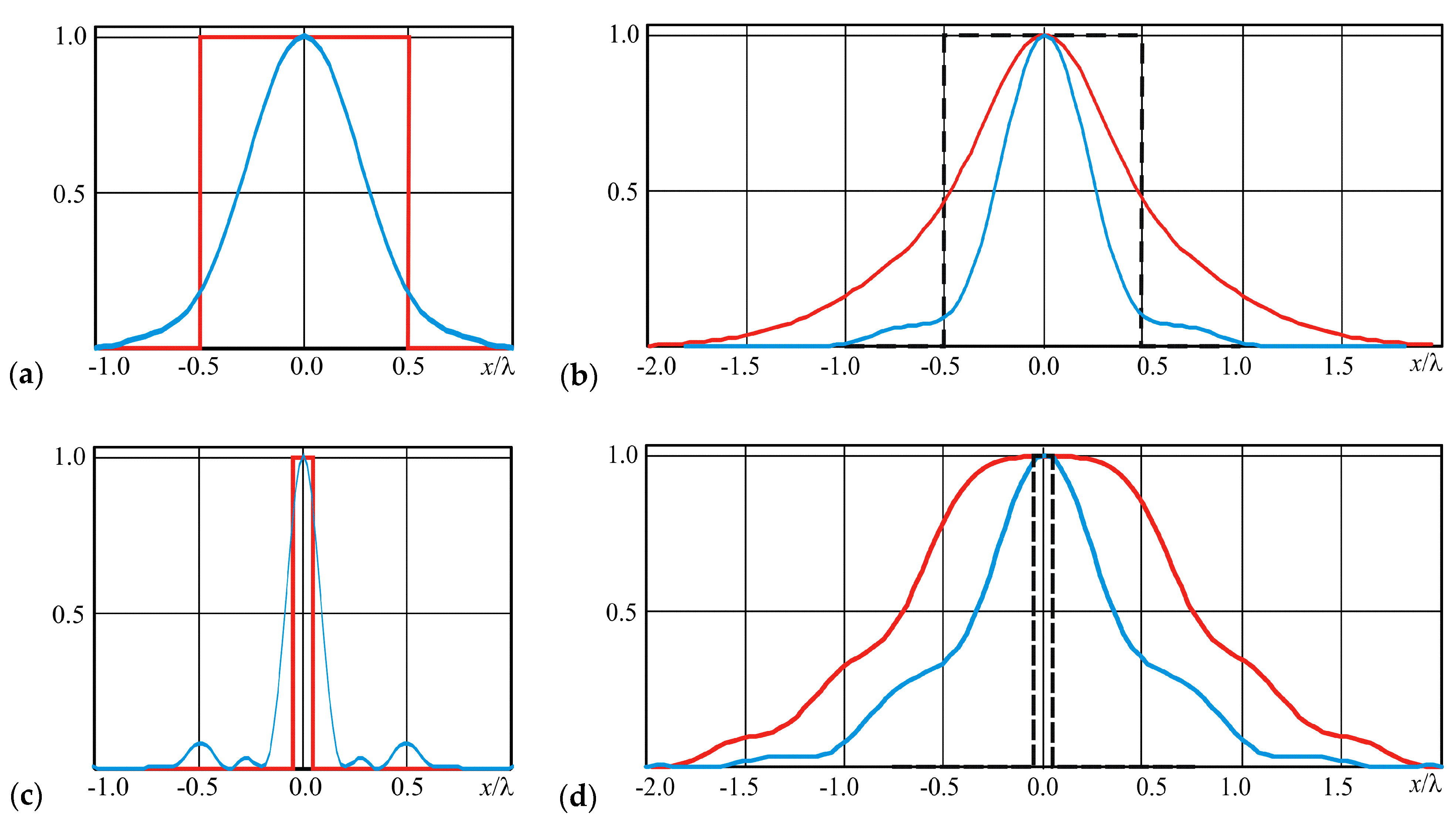
2. Superoscillating Signal Based on Superposition of Spatial Harmonics
3. Superoscillating Field Based on the Power of the Complex Function
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rayleigh, L. On the theory of optical images with special reference to the optical microscope. Philos. Mag. 1896, 5, 167–195. [Google Scholar] [CrossRef] [Green Version]
- Betzig, E.; Trautman, J.K.; Harris, T.D.; Weiner, J.S.; Kostelak, R.L. Breaking the diffraction barrier: Optical microscopy on a nanometric scale. Science 1991, 251, 1468–1470. [Google Scholar] [CrossRef] [PubMed]
- Betzig, E.; Trautman, J.K. Near-field optics: Microscopy, spectroscopy, and surface modification beyond the diffraction limit. Science 1992, 257, 189–195. [Google Scholar] [CrossRef] [Green Version]
- Heinzelmann, H.; Pohl, D. Scanning near-field optical microscopy. Appl. Phys. A 1994, 59, 89–101. [Google Scholar] [CrossRef]
- Girard, C.; Dereux, A. Near-field optics theories. Rep. Prog. Phys. 1996, 59, 657–699. [Google Scholar] [CrossRef] [Green Version]
- Hecht, B.; Sick, B.; Wild, U.P.; Deckert, V.; Zenobi, R.; Martin, O.J.F.; Pohl, D.W. Scanning near-field optical microscopy with aperture probes: Fundamentals and applications. J. Chem. Phys. 2000, 112, 7761–7774. [Google Scholar] [CrossRef]
- De Serio, M.; Zenobi, R.; Deckert, V. Looking at the nanoscale: Scanning near-field optical microscopy. TrAC Trend. Anal. Chem. 2003, 22, 70–77. [Google Scholar] [CrossRef]
- Shifa, W. Review of near field microscopy. Front. Phys. Chin. 2006, 1, 263–274. [Google Scholar]
- Lereu, A.; Passian, A.; Dumas, P. Near-field optical microscopy: A brief review. Int. J. Nanotechnol. 2012, 9, 3–7. [Google Scholar] [CrossRef]
- Degtyarev, S.A.; Khonina, S.N. Transmission of focused light signal through an apertured probe of a near-field scanning microscope. Pattern Recognit. Image Anal. 2015, 25, 306–313. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V. Very compact focal spot in the near-field of the fractional axicon. Opt. Commun. 2017, 391, 24–29. [Google Scholar] [CrossRef]
- Bazylewski, P.; Ezugwu, S.; Fanchini, G. A review of three-dimensional scanning near-field optical microscopy (3D-SNOM) and its applications in nanoscale light management. Appl. Sci. 2017, 7, 973. [Google Scholar] [CrossRef] [Green Version]
- Kowarz, M.W. Homogeneous and evanescent contributions in scalar near-field diffraction. Appl. Opt. 1995, 34, 3055–3063. [Google Scholar] [CrossRef] [PubMed]
- Katrich, A.B. Do evanescent waves really exist in free space? Opt. Commun. 2005, 255, 169–174. [Google Scholar] [CrossRef]
- Rasmussen, A.; Deckert, V. New dimension in nano-imaging:breaking through the diffraction limit with scanning near-field optical microscopy. Anal. Bioanal. Chem. 2005, 381, 165–172. [Google Scholar] [CrossRef]
- Rotenberg, N.; Kuipers, L. Mapping nanoscale light fields. Nat. Photonics 2014, 8, 919–926. [Google Scholar] [CrossRef]
- Degtyarev, S.A.; Ustinov, A.V.; Khonina, S.N. Nanofocusing by sharp edges. Comput. Opt. 2014, 38, 629–637. [Google Scholar] [CrossRef] [Green Version]
- Gramotnev, D.K.; Bozhevolnyi, S.A. Nanofocusing of electromagnetic radiation. Nat. Photonics 2014, 8, 13–22. [Google Scholar] [CrossRef]
- Degtyarev, S.A.; Porfirev, A.P.; Ustinov, A.V.; Khonina, S.N. Singular laser beams nanofocusing with dielectric nanostructures: Theoretical investigation. J. Opt. Soc. Am. B 2016, 33, 2480–2485. [Google Scholar] [CrossRef]
- Di Francia, G.T. Super-gain antennas and optical resolving power. Il Nuovo Cimento 1952, 9, 426–438. [Google Scholar] [CrossRef]
- Bucklew, J.A.; Saleh, B.E.A. Theorem for high-resolution high-contrast image synthesis. J. Opt. Soc. Am. A 1985, 2, 1233–1236. [Google Scholar] [CrossRef]
- Aharonov, Y.; Albert, D.Z.; Vaidman, L. How the result of a measurement of a component of the spin of a spin-1/2 particle can turn out to be 100. Am. Phys. Soc. 1988, 60, 1351–1354. [Google Scholar]
- Berry, M.V.; Popescu, S. Evolution of quantum superoscillations and optical superresolution without evanescent waves. J. Phys. A 2006, 39, 6965–6977. [Google Scholar] [CrossRef]
- Ferreira, P.J.S.G.; Kempf, A. Superoscillations: Faster than the Nyquist rate. IEEE Trans. Signal Process. 2006, 54, 3732–3740. [Google Scholar] [CrossRef] [Green Version]
- Huang, F.M.; Zheludev, N.I. Super-resolution without evanescent waves. Nano Lett. 2009, 9, 1249–1254. [Google Scholar] [CrossRef] [Green Version]
- Kant, R. Superresolution and increased depth of focus:an inverse problem of vector diffraction. J. Mod. Opt. 2000, 47, 905–916. [Google Scholar] [CrossRef]
- Rao, R.; Mitic, J.; Serov, A.; Leitgeb, R.A.; Lasser, T. Field confinement with aberration correction for solid immersion lens based fluorescence correlation spectroscopy. Opt. Commun. 2007, 271, 462–469. [Google Scholar] [CrossRef]
- Khonina, S.N.; Nesterenko, D.V.; Morozov, A.A.; Skidanov, R.V.; Soifer, V.A. Narrowing of a light spot at diffraction of linearly-polarized beam on binary asymmetric axicons. Opt. Mem. Neural Netw. 2012, 21, 17–26. [Google Scholar] [CrossRef]
- Hyvarinen, H.J.; Rehman, S.; Tervo, J.; Turunen, J.; Sheppard, C.J.R. Limitations of superoscillation filters in microscopy applications. Opt. Lett. 2012, 37, 903–905. [Google Scholar] [CrossRef]
- Khonina, S.N.; Volotovskiy, S.G. Minimizing the bright/shadow focal spot size with controlled side-lobe increase in high-numerical-aperture focusing systems. Adv. Opt. Technol. 2013, 2013, 267684. [Google Scholar] [CrossRef] [Green Version]
- Khonina, S.N.; Ustinov, A.V. Sharper focal spot for a radially polarized beam using ring aperture with phase jump. J. Eng. 2013, 2013, 512971. [Google Scholar] [CrossRef] [Green Version]
- Huang, F.M.; Chen, Y.; De Abajo, F.J.G.; Zheludev, N.I. Optical super-resolution through super-oscillations. J. Opt. A Pure Appl. Opt. 2007, 9, S285. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V.; Kovalyov, A.A.; Volotovsky, S.G. Near-field propagation of vortex beams: Models and computation algorithms. Opt. Mem. Neural Netw. 2014, 23, 50–73. [Google Scholar] [CrossRef]
- Khonina, S.N.; Kirilenko, M.S.; Volotovsky, S.G. Defined distribution forming in the near diffraction zone based on expansion of finite propagation operator eigenfunctions. Procedia Eng. 2017, 201, 53–60. [Google Scholar] [CrossRef]
- Boivin, R.; Boivin, A. Optimized amplitude filtering for superresolution over a restricted field: I. Achievement of maximum central irradiance under an energy constraint. Opt. Acta 1980, 27, 587–610. [Google Scholar]
- Quabis, S.; Dorn, R.; Eberler, M.; Glöckl, O.; Leuchs, G. Focusing light to tighter spot. Opt. Commun. 2000, 179, 1–7. [Google Scholar] [CrossRef]
- Reddy, A.N.K.; Khonina, S.N. Apodization for improving the two-point resolution of coherent optical systems with defect of focus. Appl. Phys. B 2018, 124, 229. [Google Scholar] [CrossRef]
- Sales, T.R.M.; Morris, G.M. Diffractive superresolution elements. J. Opt. Soc. Am. A 1997, 14, 1637–1646. [Google Scholar] [CrossRef]
- de Juana, D.M.; Oti, J.E.; Canales, V.F.; Cagigal, M.P. Design of superresolving continuous phase filters. Opt. Lett. 2003, 28, 607–609. [Google Scholar] [CrossRef]
- Sheppard, C.J.R. Filter performance parameters for high-aperture focusing. Opt. Lett. 2007, 32, 1653–1655. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V.; Pelevina, E.A. Analysis of wave aberration influence on reducing focal spot size in a high-aperture focusing system. J. Opt. 2011, 13, 095702. [Google Scholar] [CrossRef]
- Khonina, S.N. Simple phase optical elements for narrowing of a focal spot in high-numerical-aperture conditions. Opt. Eng. 2013, 52, 091711. [Google Scholar] [CrossRef]
- Ledesma, S.; Campos, J.; Escalera, J.C. Simple expressions for performance parameters of complex filters, with application to super-Gaussian phase filters. Opt. Lett. 2004, 29, 932–934. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.; Kuang, D.-F.; Fang, Z.-L. Properties of Fraunhofer Diffraction by an Annular Spiral Phase Plate for Sidelobe Suppression. Chin. Phys. Lett. 2009, 26, 094210. [Google Scholar]
- Kalosha, V.P.; Golub, I. Toward the subdiffraction focusing limit of optical superresolution. Opt. Lett. 2007, 32, 3540–3542. [Google Scholar] [CrossRef]
- Khonina, S.N.; Kazanskiy, N.L.; Karpeev, S.V.; Butt, M.A. Bessel Beam: Significance and Applications—A Progressive Review. Micromachines 2020, 11, 997. [Google Scholar] [CrossRef]
- Cagigal, M.P.; Oti, J.E.; Canales, V.F.; Valle, P.J. Analytical design of superresolving phase filters. Opt. Commun. 2004, 241, 249–253. [Google Scholar] [CrossRef]
- Liu, H.; Yan, Y.; Jin, G. Design theories and performance limits of diffractive superresolution elements with the highest sidelobe suppressed. J. Opt. Soc. Am. A 2005, 22, 828–838. [Google Scholar] [CrossRef]
- Ustinov, A.V.; Khonina, S.N. Fracxicon as hybrid element between the parabolic lens and the linear axicon. Comput. Opt. 2014, 38, 402–411. [Google Scholar] [CrossRef]
- Pierri, R.; Soldovieri, F. On the information content of the radiated fields in the near zone over bounded domains. Inverse Probl. 1998, 14, 321–337. [Google Scholar] [CrossRef]
- Miller, D.A.B. Communicating with waves between volumes: Evaluating orthogonal spatial channels and limits on coupling strengths. Appl. Opt. 2000, 39, 1681–1699. [Google Scholar] [CrossRef] [Green Version]
- Thaning, A.; Martinsson, P.; Karelin, M.; Friberg, A.T. Limits of diffractive optics by communication modes. J. Opt. A Pure Appl. Opt. 2003, 5, 153–158. [Google Scholar] [CrossRef]
- Mazilu, M.; Baumgartl, J.; Kosmeier, S.; Dholakia, K. Optical Eigenmodes; exploiting the quadratic nature of the energy flux and of scattering interactions. Opt. Express 2011, 19, 933–945. [Google Scholar] [CrossRef] [Green Version]
- Baumgartl, J.; Kosmeier, S.; Mazilu, M.; Rogers, E.T.F.; Zheludev, N.I.; Dholakia, K. Far field subwavelength focusing using optical eigenmodes. Appl. Phys. Lett. 2011, 98, 181109. [Google Scholar] [CrossRef] [Green Version]
- Kirilenko, M.S.; Khonina, S.N. Formation of signals matched with vortex eigenfunctions of bounded double lens system. Opt. Commun. 2018, 410, 153–159. [Google Scholar] [CrossRef]
- Slepian, D.; Pollak, H.O. Prolate spheroidal wave functions, Fourier analysis and uncertainty—I. Bell Syst. Technol. J. 1961, 40, 43–63. [Google Scholar] [CrossRef]
- Landau, H.J.; Pollak, H.O. Prolate spheroidal wave functions, Fourier analysis and uncertainty—II. Bell Syst. Technol. J. 1961, 40, 65–84. [Google Scholar] [CrossRef]
- Khonina, S.N.; Volotovskii, S.G.; Soifer, V.A. A method for computing the eigenvalues of prolate spheroidal functions of order zero. Dokl. Math. 2001, 63, 136–138. [Google Scholar]
- Karoui, A.; Moumni, T. Spectral analysis of the finite Hankel transform and circular prolate spheroidal wave functions. J. Comput. Appl. Math. 2009, 233, 315–333. [Google Scholar] [CrossRef] [Green Version]
- Kirilenko, M.S.; Zubtsov, R.O.; Khonina, S.N. Calculation of eigenfunctions of a bounded fractional Fourier transform. Comput. Opt. 2015, 39, 332–338. [Google Scholar] [CrossRef] [Green Version]
- Kirilenko, M.S.; Zubtsov, R.O.; Khonina, S.N. Calculation of eigenfunctions of bounded waveguide with quadratic refractive index. J. Phys. Conf. Ser. 2016, 735, 012002. [Google Scholar] [CrossRef] [Green Version]
- Gallager, R.G. Information Theory and Reliable Communication; John Wiley & Sons, Inc.: New York, NY, USA, 1968; ISBN 978-0-471-29048-3. [Google Scholar]
- Di Francia, G.T. Degrees of freedom of an image. J. Opt. Soc. Am. 1969, 59, 799–804. [Google Scholar] [CrossRef] [PubMed]


| Apodization (Amplitude and Phase Distribution) | Distribution in the Focal Plane (Intensity and Graph of Cross-Section) |
|---|---|
Without apodization |  FWHM = 0.544λ, S = 0.16 |
Narrow ring | 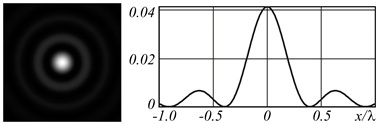 FWHM = 0.378λ, S = 0.162 |
Ring with phase jump | 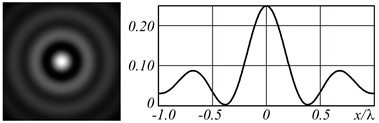 FWHM = 0.371λ, S = 0.351 |
Optimized function | 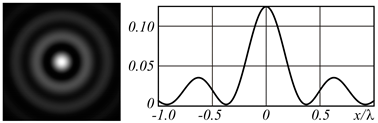 FWHM = 0.359λ, S = 0.28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khonina, S.N.; Ponomareva, E.D.; Butt, M.A. Study of Superoscillating Functions Application to Overcome the Diffraction Limit with Suppressed Sidelobes. Optics 2021, 2, 155-168. https://doi.org/10.3390/opt2030015
Khonina SN, Ponomareva ED, Butt MA. Study of Superoscillating Functions Application to Overcome the Diffraction Limit with Suppressed Sidelobes. Optics. 2021; 2(3):155-168. https://doi.org/10.3390/opt2030015
Chicago/Turabian StyleKhonina, Svetlana N., Ekaterina D. Ponomareva, and Muhammad A. Butt. 2021. "Study of Superoscillating Functions Application to Overcome the Diffraction Limit with Suppressed Sidelobes" Optics 2, no. 3: 155-168. https://doi.org/10.3390/opt2030015
APA StyleKhonina, S. N., Ponomareva, E. D., & Butt, M. A. (2021). Study of Superoscillating Functions Application to Overcome the Diffraction Limit with Suppressed Sidelobes. Optics, 2(3), 155-168. https://doi.org/10.3390/opt2030015








