An Integrated Approach to Control the Penetration Depth of 3D-Printed Hollow Microneedles
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
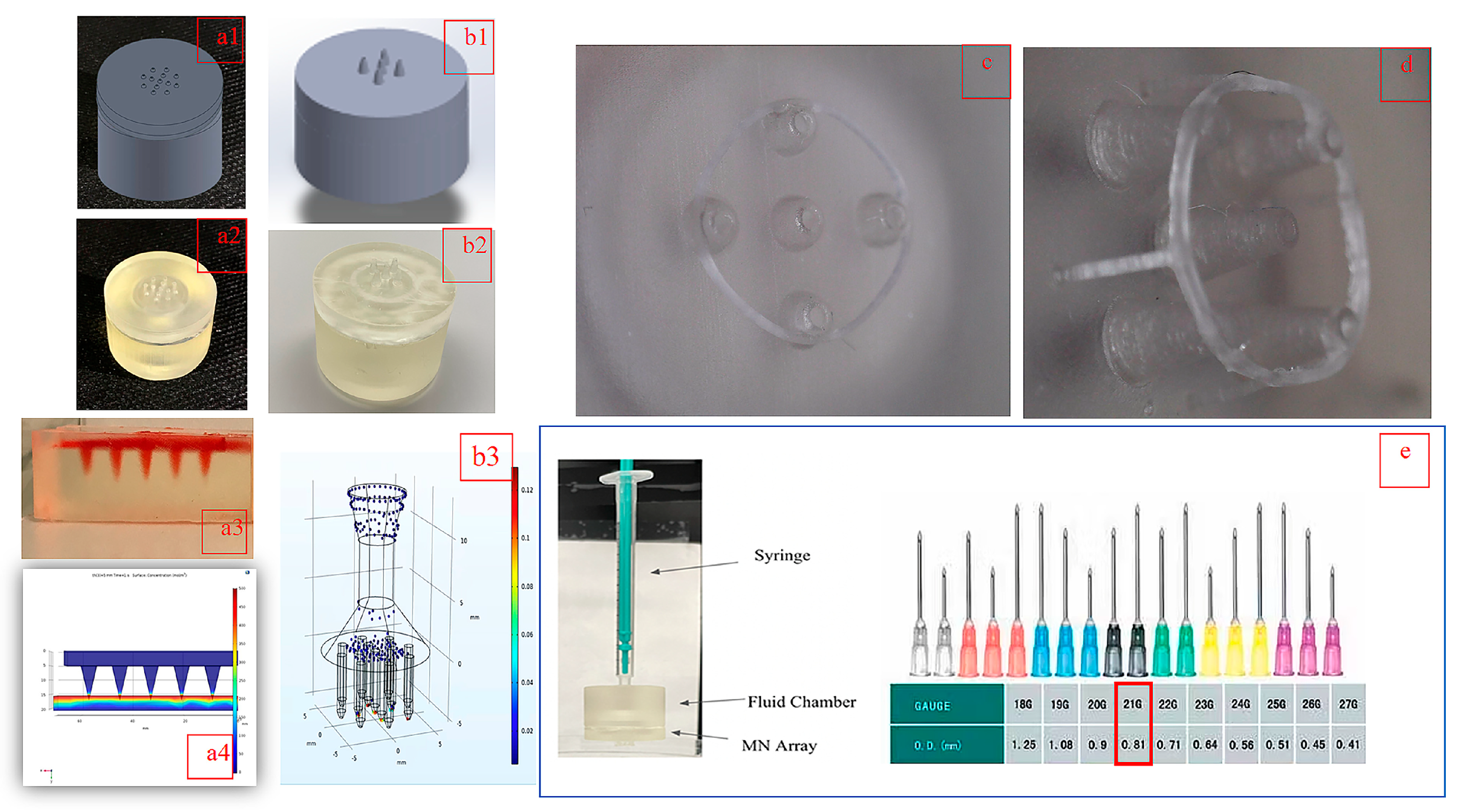
2.2.1. Device Design Printing and Assembly
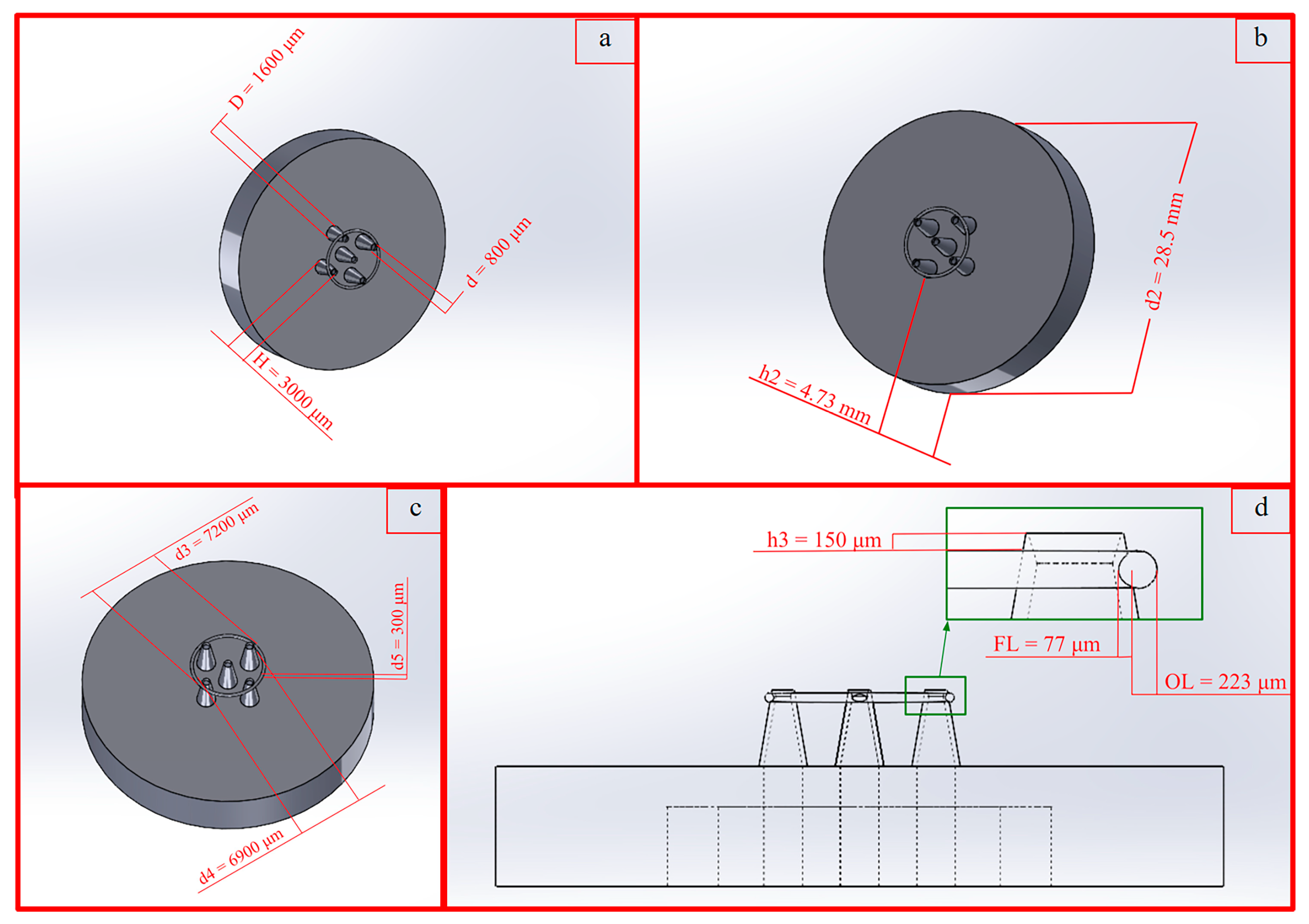
- Design
- 2.
- 3D Printing
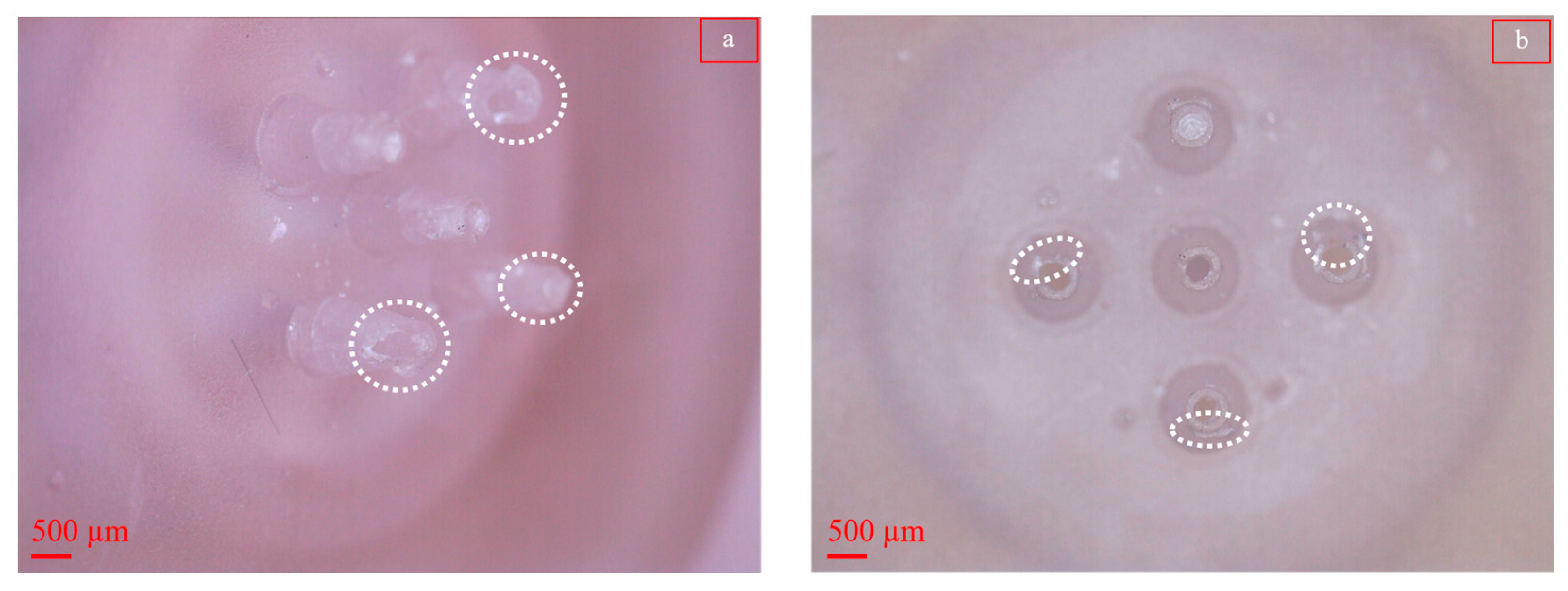
2.2.2. Inspection of 3D-Printed Parts
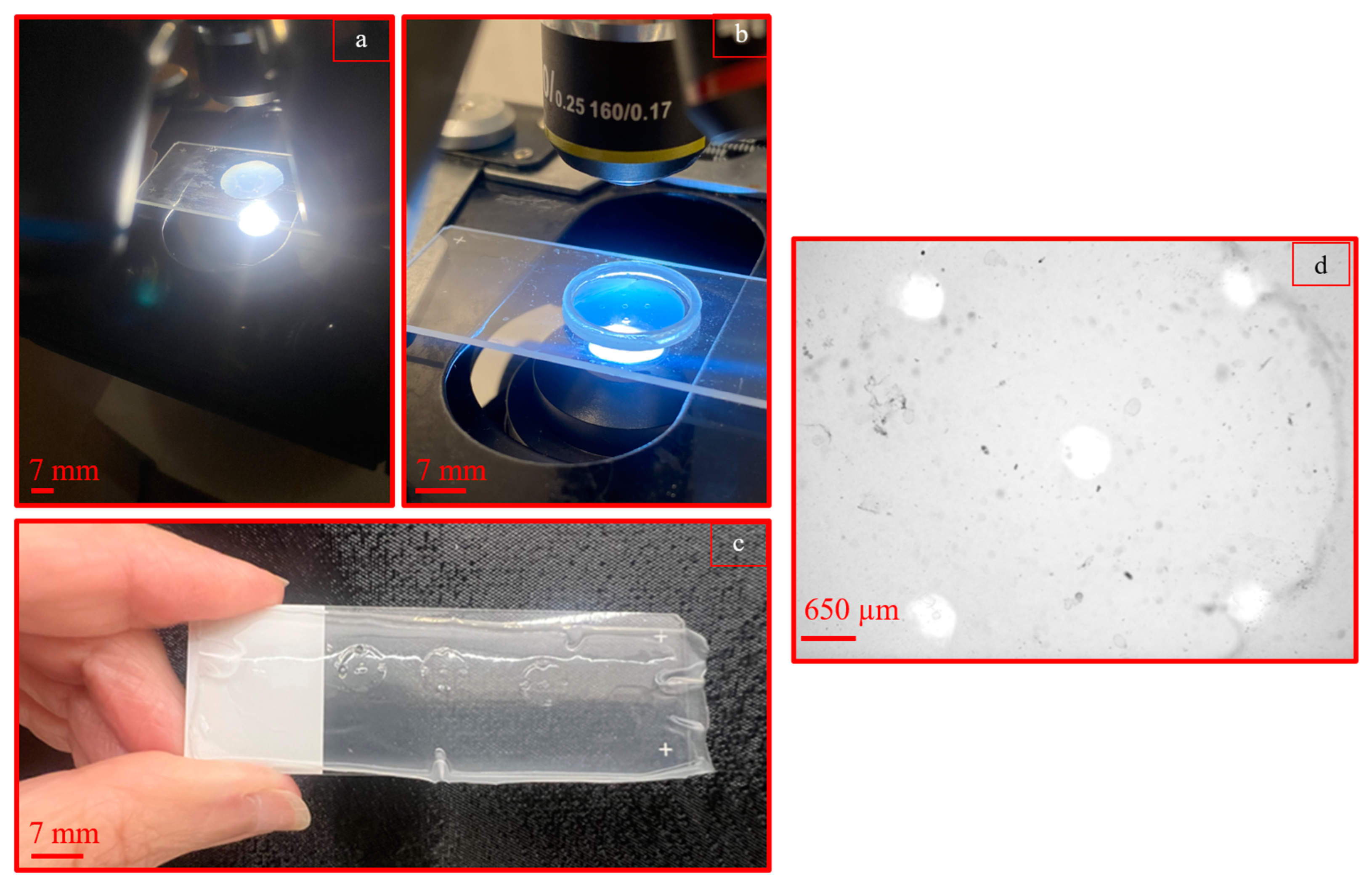
2.2.3. Skin Phantom Fabrication
2.2.4. Phantom Dimensional Verification
2.2.5. Profilometry
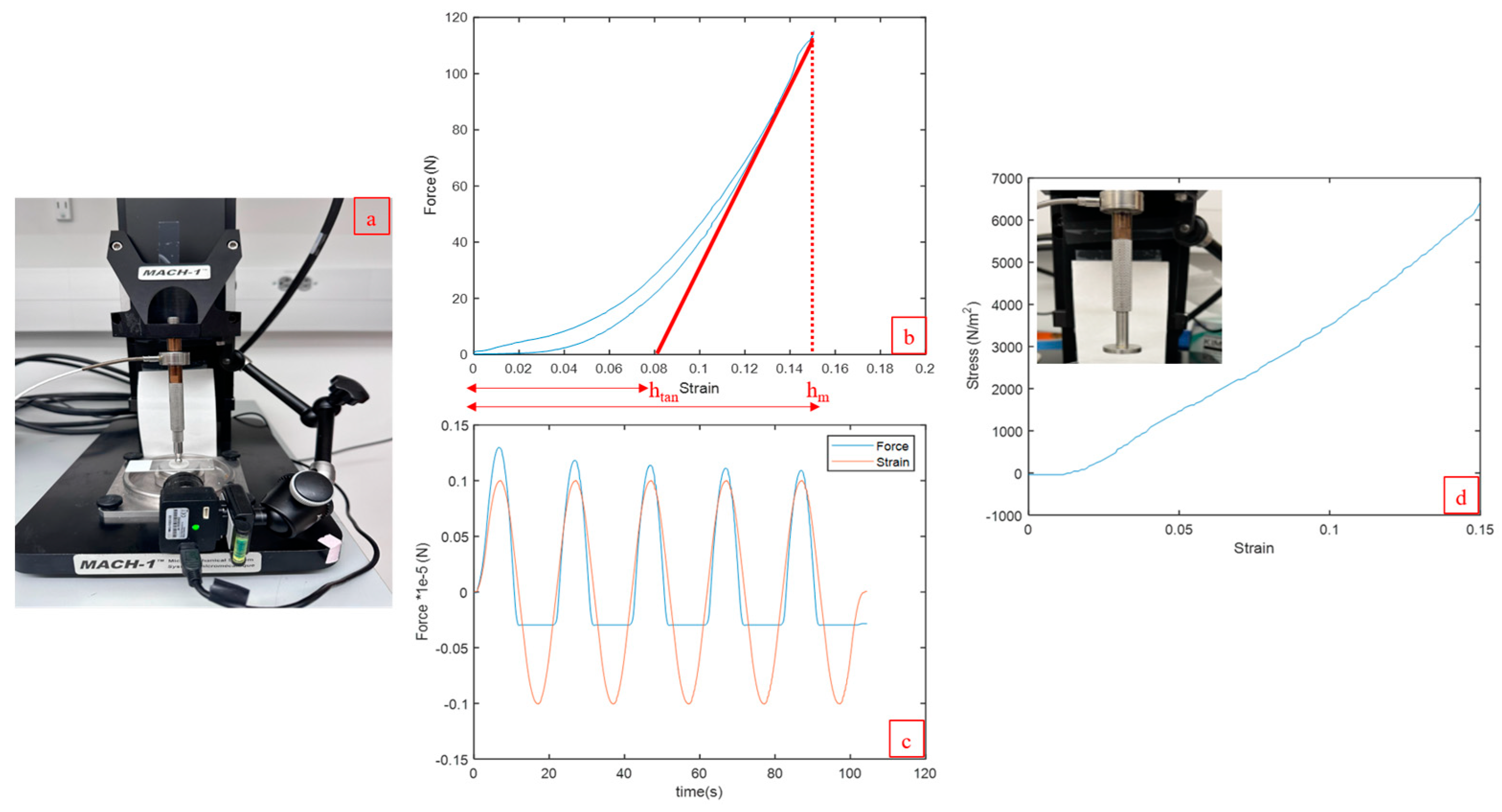
2.2.6. Mechanical Testing
- Base Substrate Characterization
- 2.
- Viscoelasticity Characterization
- 3.
- Indentation Parameters
2.2.7. Pycnometry
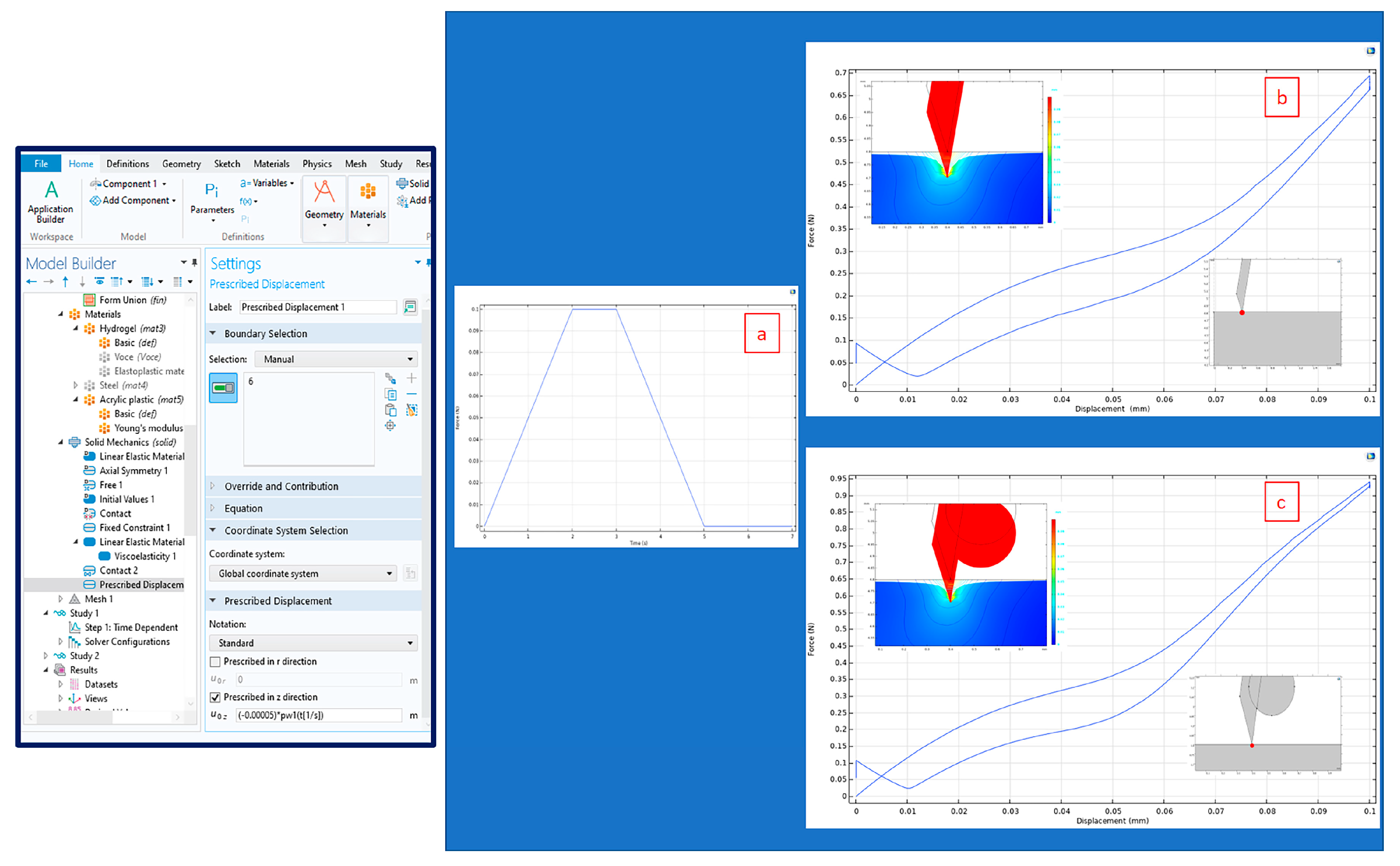
2.2.8. Simulation
2.2.9. Statistical Analysis
3. Results
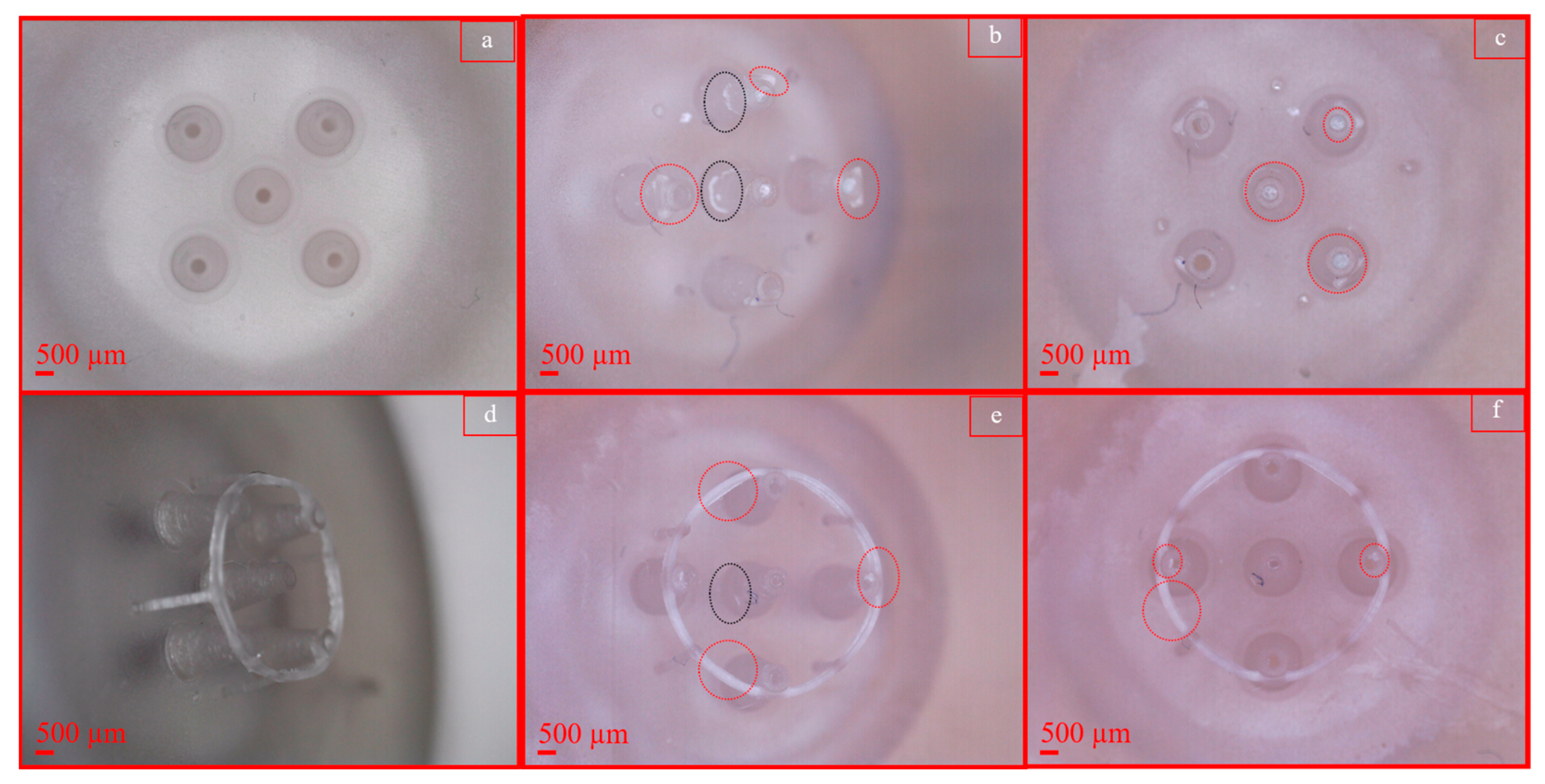
3.1. Dimensional Inspection of 3D-Printed Parts
3.2. Skin Phantom Fabrication and Dimensional Verifcation
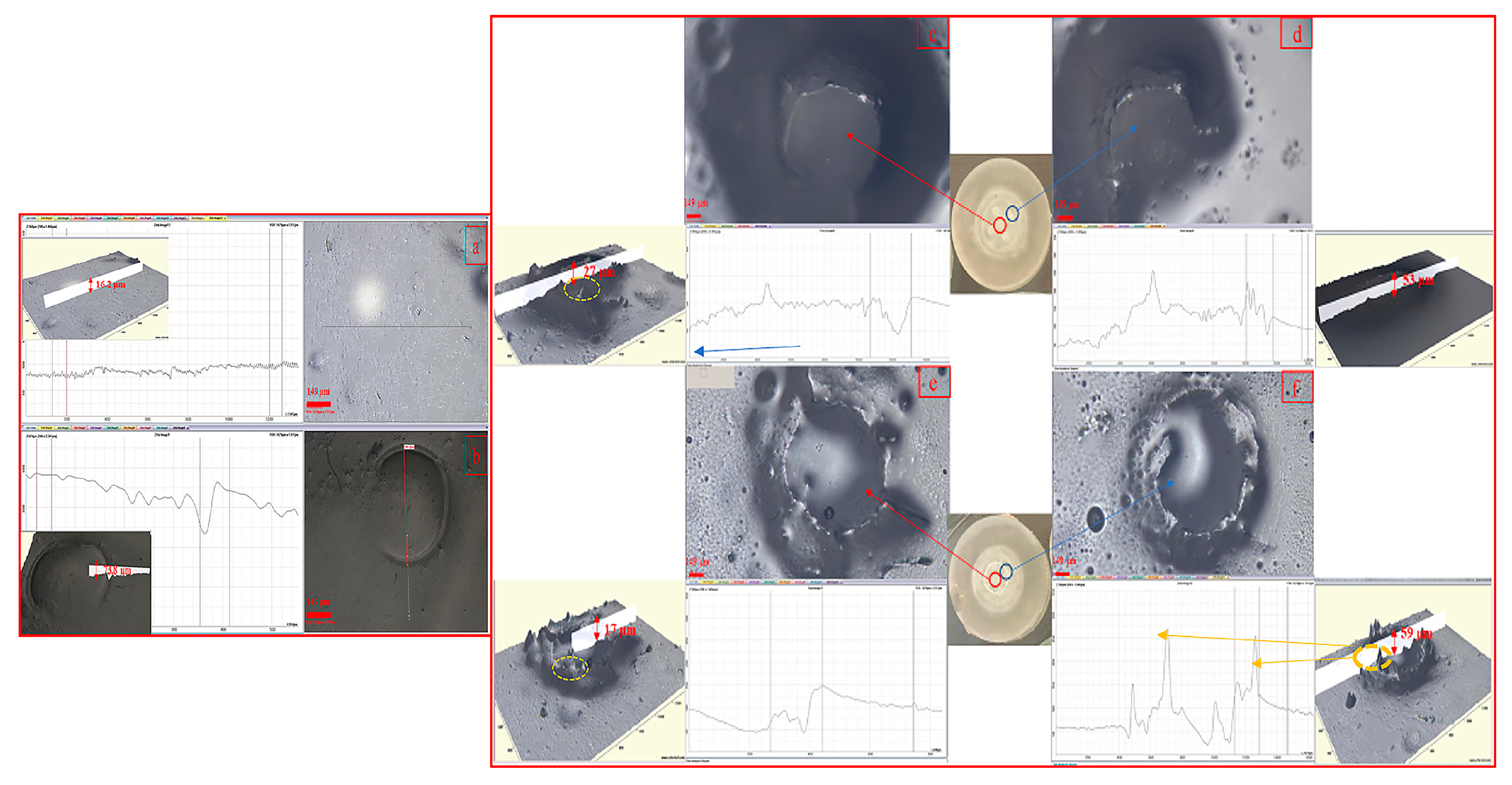
3.3. Profilometry
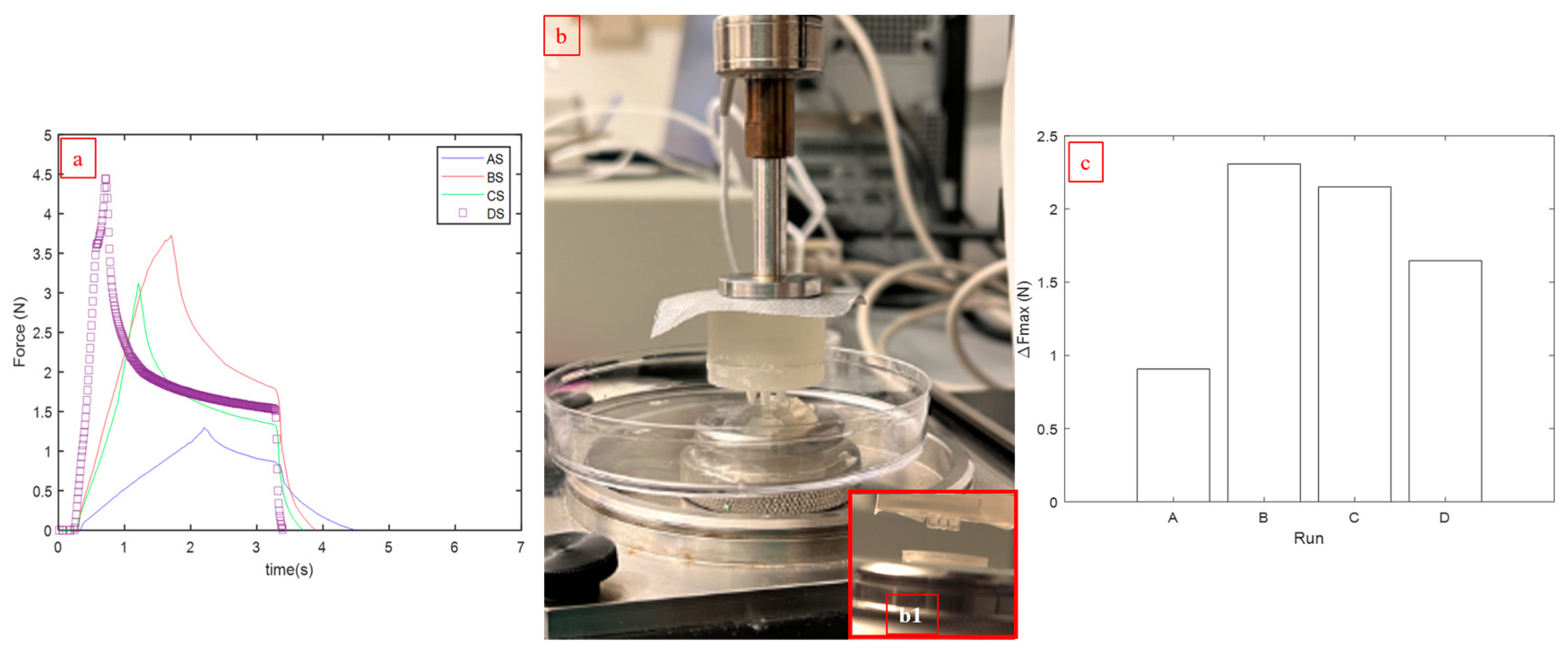
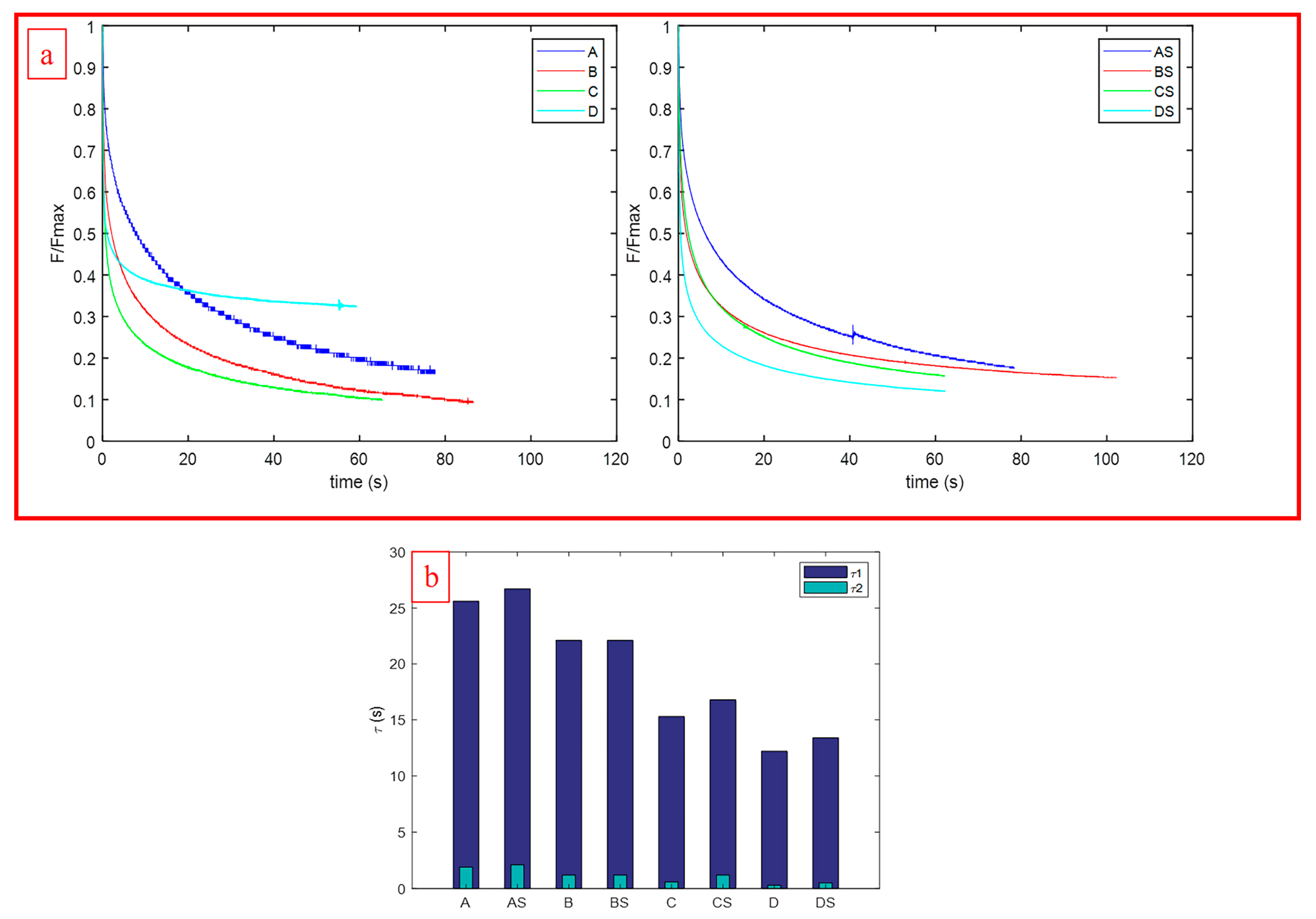
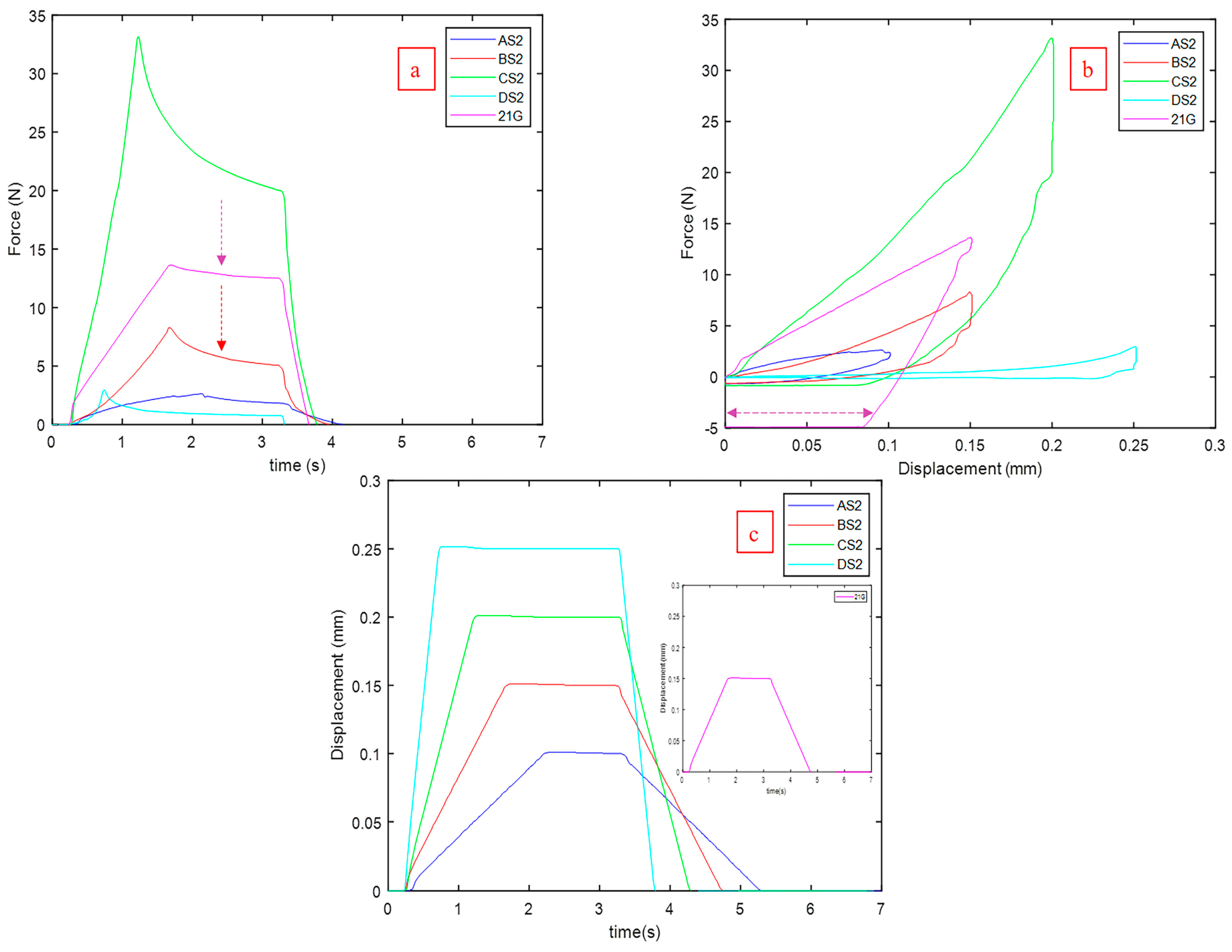
3.4. Mechanical Testing
- Substrate Characterization
- 2.
- Viscoelastic Characterization Using Microneedles
- 3.
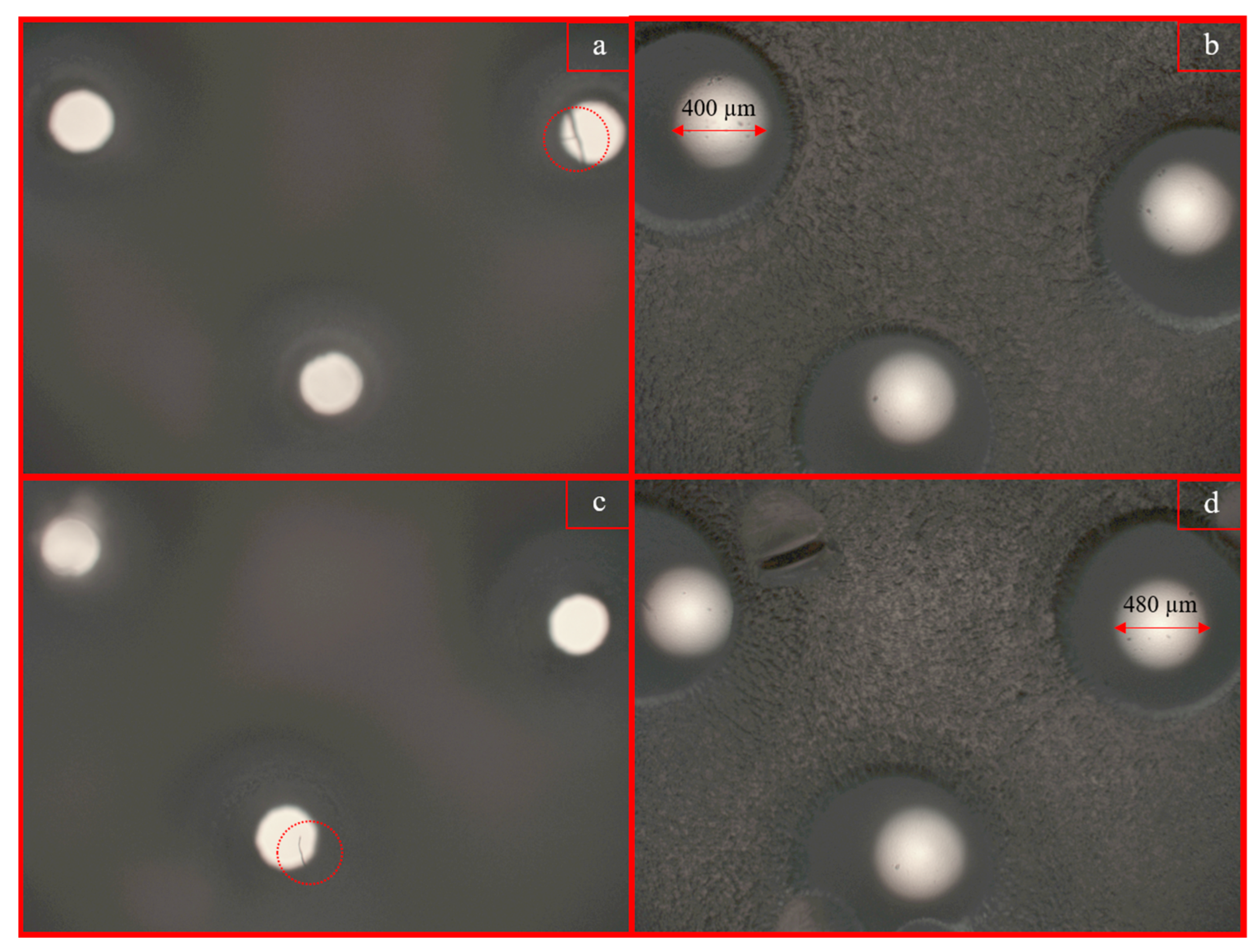
- Microneedle Inspection Post-Indentation
3.5. Pycnometry
3.6. Validation of Puncture
3.7. Simulation
4. Discussion
4.1. Dimensional Inspection of 3D-Printed Parts
4.2. Profilometry
4.3. Mechanical Testing
4.4. Pycnometry
4.5. Simulation
4.6. Implications of the Research
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Chen, B.Z.; Zhao, Z.Q.; Shahbazi, M.A.; Guo, X.D. Microneedle-based technology for cell therapy: Current status and future directions. Nanoscale Horiz. 2022, 7, 715–728. [Google Scholar] [CrossRef] [PubMed]
- Yeung, C.; Chen, S.; King, B.; Lin, H.; King, K.; Akhtar, F.; Emaminejad, S.A. 3D-printed microfluidic-enabled hollow microneedle architecture for transdermal drug delivery. Biomicrofluidics 2019, 13, 064125. [Google Scholar] [CrossRef] [PubMed]
- Economidou, S.N.; Uddin, J.; Marques, M.J.; Douroumis, D.; Sow, W.T.; Li, H.; Reid, A.; Windmill, J.F.C.; Podoleanu, A. A novel 3D printed hollow microneedle microelectromechanical system for controlled, personalized transdermal drug delivery. Addit. Manuf. 2021, 38, 101815. [Google Scholar] [CrossRef]
- Hu, Z.; Meduri, C.S.; Ingrole, R.S.J.; Gill, H.S.; Kumar, G. Solid and hollow metallic glass microneedles for transdermal drug-delivery. Appl. Phys. Lett. 2020, 116, 203703. [Google Scholar] [CrossRef]
- Ren, Y.; Li, J.; Chen, Y.; Wang, J.; Chen, Y.; Wang, Z.; Zhang, Z.; Chen, Y.; Shi, X.; Cao, L.; et al. Customized flexible hollow microneedles for psoriasis treatment with reduced-dose drug. Bioeng. Transl. Med. 2023, 8, e10530. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, H.X.; Nguyen, C.N. Microneedle-Mediated Transdermal Delivery of Biopharmaceuticals. Pharmaceutics 2023, 15, 277. [Google Scholar] [CrossRef] [PubMed]
- Gualeni, B.; Coulman, S.A.; Shah, D.; Eng, P.F.; Ashraf, H.; Vescovo, P.; Blayney, G.J.; Piveteau, L.-D.; Guy, O.J.; Birchall, J.C. Minimally invasive and targeted therapeutic cell delivery to the skin using microneedle devices. Br. J. Derm. 2018, 178, 731–739. [Google Scholar] [CrossRef] [PubMed]
- Iliescu, F.S.; Teo, J.C.M.; Vrtacnik, D.; Taylor, H.; Iliescu, C. Cell therapy using an array of ultrathin hollow microneedles. Microsyst. Technol. 2018, 24, 2905–2912. [Google Scholar] [CrossRef]
- Chang, H.; Chew, S.W.T.; Zheng, M.; Lio, D.C.S.; Wiraja, C.; Mei, Y.; Ning, X.; Cui, M.; Than, A.; Shi, P.; et al. Cryomicroneedles for transdermal cell delivery. Nat. Biomed. Eng. 2021, 5, 1008–1018. [Google Scholar] [CrossRef]
- Xenikakis, I.; Tsongas, K.; Tzimtzimis, E.K.; Katsamenis, O.L.; Demiri, E.; Zacharis, C.K.; Georgiou, D.; Kalogianni, E.P.; Tzetzis, D.; Fatouros, D.G. Transdermal delivery of insulin across human skin in vitro with 3D printed hollow microneedles. J. Drug Deliv. Sci. Technol. 2022, 67, 102891. [Google Scholar] [CrossRef]
- Resnik, D.; Možek, M.; Pečar, B.; Janež, A.; Urbančič, V.; Iliescu, C.; Vrtačnik, D. In Vivo Experimental Study of Noninvasive Insulin Microinjection through Hollow Si Microneedle Array. Micromachines 2018, 9, 40. [Google Scholar] [CrossRef] [PubMed]
- Abd-El-Azim, H.; Tekko, I.A.; Ali, A.; Ramadan, A.; Nafee, N.; Khalafallah, N.; Rahman, T.; Mcdaid, W.; Aly, R.G.; Vora, L.K.; et al. Hollow microneedle assisted intradermal delivery of hypericin lipid nanocapsules with light enabled photodynamic therapy against skin cancer. J. Control. Release 2022, 348, 849–869. [Google Scholar] [CrossRef] [PubMed]
- Weng, J.; Zheng, G.; Wen, J.; Yang, J.; Yang, Q.; Zheng, X.; Yan, Q. Construction and application of microneedle-mediated photothermal therapy and immunotherapy combined anti-tumor drug delivery system. Drug Deliv. 2023, 30, 2232950. [Google Scholar] [CrossRef] [PubMed]
- Fang, A.; Wang, Y.; Guan, N.; Zuo, Y.; Lin, L.; Guo, B.; Mo, A.; Wu, Y.; Lin, X.; Cai, W.; et al. Porous microneedle patch with sustained delivery of extracellular vesicles mitigates severe spinal cord injury. Nat. Commun. 2023, 14, 4011. [Google Scholar] [CrossRef] [PubMed]
- Makvandi, P.; Kirkby, M.; Hutton, A.R.J.; Shabani, M.; Yiu, C.K.Y.; Baghbantaraghdari, Z.; Jamaledin, R.; Carlotti, M.; Mazzolai, B.; Mattoli, V.; et al. Engineering Microneedle Patches for Improved Penetration: Analysis, Skin Models and Factors Affecting Needle Insertion. Nano-Micro Lett. 2021, 13, 93. [Google Scholar] [CrossRef] [PubMed]
- Ali, M.; Namjoshi, S.; Benson, H.A.E.; Kumeria, T.; Mohammed, Y. Skin biomechanics: Breaking the dermal barriers with microneedles. Nano TransMed 2022, 1, 9130002. [Google Scholar] [CrossRef]
- Abd, E.; Yousef, S.A.; Pastore, M.N.; Telaprolu, K.; Mohammed, Y.H.; Namjoshi, S.; Grice, J.E.; Roberts, M.S. Skin models for the testing of transdermal drugs. Clin. Pharmacol. Adv. Appl. 2016, 8, 163–176. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Liu, X.; Fu, Y.; Song, Y. Recent advances of microneedles for biomedical applications: Drug delivery and beyond. Acta Pharm. Sin. B 2019, 9, 469–483. [Google Scholar] [CrossRef]
- Kulkarni, D.; Gadade, D.; Chapaitkar, N.; Shelke, S.; Pekamwar, S.; Aher, R.; Ahire, A.; Avhale, M.; Badgule, R.; Bansode, R.; et al. Polymeric Microneedles: An Emerging Paradigm for Advanced Biomedical Applications. Sci. Pharm. 2023, 91, 27. [Google Scholar] [CrossRef]
- Kyser, A.J.; Fotouh, B.; Mahmoud, M.Y.; Frieboes, H.B. Rising role of 3D-printing in delivery of therapeutics for infectious disease. J. Control. Release 2024, 366, 349–365. [Google Scholar] [CrossRef]
- Farias, C.; Lyman, R.; Hemingway, C.; Chau, H.; Mahacek, A.; Bouzos, E.; Mobed-Miremadi, M. Three-Dimensional (3D) Printed Microneedles for Microencapsulated Cell Extrusion. Bioengineering 2018, 5, 59. [Google Scholar] [CrossRef] [PubMed]
- Boehm, R.D.; Jaipan, P.; Yang, K.-H.; Stewart, T.N.; Narayan, R.J. Microstereolithography-fabricated Microneedles for Fluid Sampling of Histamine-contaminated Tuna. Int. J. Bioprinting 2016, 2, 72–80. [Google Scholar] [CrossRef]
- Lim, S.H.; Ng, J.Y.; Kang, L. Three-dimensional Printing of a Microneedle Array on Personalized Curved Surfaces for Dual-pronged Treatment of Trigger Finger. Biofabrication 2017, 9, 015010. [Google Scholar] [CrossRef] [PubMed]
- Faraji Rad, Z.; Nordon, R.; Anthony, C.; Bilston, L.; Prewett, P.; Arns, J.-Y.; Arns, C.; Zhang, L.; Davies, G. High Fidelity Replication of Thermoplastic Microneedles with Open Microfluidic Channels. Microsyst. Nanoeng. 2017, 3, 17034. [Google Scholar] [CrossRef] [PubMed]
- Razzaghi, M.; Akbari, M. The Effect of 3D Printing Tilt Angle on the Penetration of 3D-Printed Microneedle Arrays. Micromachines 2023, 14, 1157. [Google Scholar] [CrossRef] [PubMed]
- Patel, P.; Dhal, K.; Gupta, R.; Tappa, K.; Rybicki, F.J.; Ravi, P. Medical 3D Printing Using Desktop Inverted Vat Photopolymerization: Background, Clinical Applications, and Challenges. Bioengineering 2023, 10, 782. [Google Scholar] [CrossRef] [PubMed]
- Gatto, M.L.; Mengucci, P.; Mattioli-Belmonte, M.; Munteanu, D.; Nasini, R.; Tognoli, E.; Denti, L.; Gatto, A. Features of Vat-Photopolymerized Masters for Microfluidic Device Manufacturing. Bioengineering 2024, 11, 80. [Google Scholar] [CrossRef] [PubMed]
- Gao, Z.; Sheng, T.; Zhang, W.; Feng, H.; Yu, J.; Gu, Z.; Zhang, Y. Microneedle-Mediated Cell Therapy. Adv. Sci. 2024, 11, 2304124. [Google Scholar] [CrossRef]
- Mbituyimana, B.; Adhikari, M.; Qi, F.; Shi, Z.; Fu, L.; Yang, G. Microneedle-based cell delivery and cell sampling for biomedical applications. J. Control. Release 2023, 362, 692–714. [Google Scholar] [CrossRef]
- Chen, Y.-H.; Wang, F.-Y.; Chan, Y.-S.; Huang, C.; Huang, Y.-Y. Biofabricating hollow microneedle array with controllable microstructure for cell transplantation. J. Biomed. Mater. Res. 2022, 110, 1997–2005. [Google Scholar] [CrossRef]
- Tang, J.; Wang, J.; Huang, K.; Ye, Y.; Su, T.; Qiao, L.; Hensley, M.T.; Caranasos, T.G.; Zhang, J.; Gu, Z.; et al. Cardiac cell-integrated microneedle patch for treating myocardial infarction. Sci. Adv. 2018, 4, eaat9365. [Google Scholar] [CrossRef]
- Zheng, M.; Hu, T.; Yang, Y.; Qie, X.; Yang, H.; Zhang, Y.; Zhang, Q.; Yong, K.-T.; Liu, W.; Xu, C. In situ-formed cryomicroneedles for intradermal cell delivery. NPG Asia Mater. 2024, 16, 11. [Google Scholar] [CrossRef]
- Ye, Y.; Yu, J.; Wang, C.; Nguyen, N.Y.; Walker, G.M.; Buse, J.B.; Gu, Z. Microneedles Integrated with Pancreatic Cells and Synthetic Glucose-Signal Amplifiers for Smart Insulin Delivery. Adv. Mater. 2016, 28, 3115–3121. [Google Scholar] [CrossRef]
- Kho, A.S.K.; Béguin, S.; O’Cearbhaill, E.D.; Annaidh, A.N. Mechanical characterization of commercial artificial skin models. J. Mech. Behav. Biomed. Mater. 2023, 147, 106090. [Google Scholar] [CrossRef]
- Chang, T.M.S. Artificial Cells for Cell and Organ Replacements. Artif. Organs 2004, 28, 265–270. [Google Scholar] [CrossRef]
- Kang, S.M.; Lee, J.H.; Huh, Y.S.; Takayama, S. Alginate Microencapsulation for Three-Dimensional In Vitro Cell Culture. ACS Biomater. Sci. Eng. 2021, 7, 2864–2879. [Google Scholar] [CrossRef]
- Desai, T.; Shea, L. Advances in islet encapsulation technologies. Nat. Rev. Drug Discov. 2017, 16, 338–350. [Google Scholar] [CrossRef]
- Tan, J.; Luo, Y.; Guo, Y.; Zhou, Y.; Liao, X.; Li, D.; Lai, X.; Liu, Y. Development of alginate-based hydrogels: Crosslinking strategies and biomedical applications. Int. J. Biol. Macromol. 2023, 239, 124275. [Google Scholar] [CrossRef]
- Zinkovska, N.; Pekar, M.; Smilek, J. Gradient Hydrogels-Overview of Techniques Demonstrating the Existence of a Gradient. Polymers 2022, 14, 866. [Google Scholar] [CrossRef]
- Freeman, F.E.; Kelly, D.J. Tuning Alginate Bioink Stiffness and Composition for Controlled Growth Factor Delivery and to Spatially Direct MSC Fate within Bioprinted Tissues. Sci. Rep. 2017, 7, 17042. [Google Scholar] [CrossRef]
- Luo, T.; Tan, B.; Zhu, L.; Wang, Y.; Liao, J. A Review on the Design of Hydrogels with Different Stiffness and Their Effects on Tissue Repair. Front. Bioeng. Biotechnol. 2022, 10, 817391. [Google Scholar] [CrossRef]
- Garrido, C.A.; Garske, D.S.; Thiele, M.; Amini, S.; Real, S.; Duda, G.N.; Schmidt-Bleek, K.; Cipitria, A. Hydrogels with stiffness-degradation spatial patterns control anisotropic 3D cell response. Biomater. Adv. 2023, 151, 213423. [Google Scholar] [CrossRef]
- Kim, B.; Park, J.; Lee, J.Y. Conductive double-network hydrogel composed of sodium alginate, polyacrylamide, and reduced graphene oxide. Korean J. Chem. Eng. 2023, 40, 352–360. [Google Scholar] [CrossRef]
- Liu, T.; Zhang, R.; Liu, J.; Zhao, L.; Yu, Y. High strength and conductive hydrogel with fully interpenetrated structure from alginate and acrylamide. e-Polymers 2021, 21, 391–397. [Google Scholar] [CrossRef]
- Ji, D.; Park, J.M.; Oh, M.S.; Nguyen, T.L.; Shin, H.; Kim, J.S.; Kim, D.; Park, H.S.; Kim, J. Superstrong, superstiff, and conductive alginate hydrogels. Nat. Commun. 2022, 13, 3019. [Google Scholar] [CrossRef]
- Cui, W.; Zheng, Y.; Zhu, R.; Mu, Q.; Wang, X.; Wang, Z.; Liu, S.; Li, M.; Ran, R. Strong Tough Conductive Hydrogels via the Synergy of Ion-Induced Cross-Linking and Salting-Out. Adv. Funct. Mater. 2022, 32, 2204823. [Google Scholar] [CrossRef]
- Conci, A.; Brazil, A.L.; Popovici, D.; Jiga, G.; Lebon, F. Modeling the behavior of human body tissues on penetration. AIP Conf. Proc. 2018, 1932, 020006. [Google Scholar] [CrossRef]
- Trączyński, M.; Patalas, A.; Rosłan, K.; Suszyński, M.; Talar, R. Assessment of needle–tissue force models based on ex vivo measurements. J. Mech. Behav. Biomed. Mater. 2024, 150, 106247. [Google Scholar] [CrossRef]
- Okamura, A.M.; Simone, C.; O’Leary, M.D. Force modeling for needle insertion into soft tissue. IEEE Trans. Biomed. Eng. 2004, 51, 1707–1716. [Google Scholar] [CrossRef]
- Choo, S.; Jin, S.; Jung, J. Fabricating High-Resolution and High-Dimensional Microneedle Mold through the Resolution Improvement of Stereolithography 3D Printing. Pharmaceutics 2022, 14, 766. [Google Scholar] [CrossRef]
- Gordon, A.; Kim, I.; Barnett, A.C.; Moore, J.Z. Needle Insertion Force Model for Haptic Simulation. In Proceedings of the ASME 2015 International Manufacturing Science and Engineering Conference, Charlotte, NC, USA, 8–12 June 2015; Volume 2: Materials; Biomanufacturing; Properties, Applications and Systems; Sustainable Manufacturing; ASME: New York, NY, USA, 2015; p. V002T03A003. [Google Scholar] [CrossRef]
- Machekposhti, S.A.; Kadian, S.; Vanderwal, L.; Stafslien, S.; Narayan, R.J. Novel hollow biodegradable microneedle for amphotericin B delivery. MedComm 2023, 4, e321. [Google Scholar] [CrossRef]
- Dardano, P.; De Martino, S.; Battisti, M.; Miranda, B.; Rea, I.; De Stefano, L. One-Shot Fabrication of Polymeric Hollow Microneedles by Standard Photolithography. Polymers 2021, 13, 520. [Google Scholar] [CrossRef]
- Henriquez, F.; Morales-Ferreiro, J.O.; Celentano, D. Structural Evaluation by the Finite-Element Method of Hollow Microneedle Geometries for Drug Delivery. Adv. Eng. Mater. 2022, 24, 2200049. [Google Scholar] [CrossRef]
- Tamez-Tamez, J.I.; Vázquez-Lepe, E.; Rodriguez, C.A.; Martínez-López, J.I.; García-López, E. Assessment of geometrical dimensions and puncture feasibility of microneedles manufactured by micromilling. Int. J. Adv. Manuf. Technol. 2023, 126, 4983–4996. [Google Scholar] [CrossRef]
- Anbazhagan, G.; Suseela, S.B.; Sankararajan, R. Effect of hollow microneedle geometry structure on mechanical stability and microfluidic flow for transdermal drug delivery applications. Microfluid. Nanofluid 2023, 27, 25. [Google Scholar] [CrossRef]
- Quisling, S.; Vestal, L.; Enstrom, A. Flow Visualization of Bolus Microcapsule Delivery through 3D Printed Microneedles. Bachelor’s Thesis, Santa Clara University, Santa Clara, CA, USA, June 2021. Available online: https://scholarcommons.scu.edu/bioe_senior/105 (accessed on 13 March 2024).
- Defelippi, K.; Johnson, D. Shear Detection of Microencapsulated Cells for Monoclonal Antibody Production Scaleup. Bachelor’s Thesis, Santa Clara University, Santa Clara, CA, USA, June 2022. Available online: https://scholarcommons.scu.edu/bioe_senior/120 (accessed on 13 March 2024).
- Matheny, M.; Dubus, M.; Eribes, L. Microneedles for Wound Healing. Bachelor’s Thesis, Santa Clara University, Santa Clara, CA, USA, June 2023. Available online: https://scholarcommons.scu.edu/bioe_senior/125/ (accessed on 13 March 2024).
- Johnson, D.; Kim, U.; Mobed-Miremadi, M. Nanocomposite films as electrochemical sensors for detection of catalase activity. Front. Mol. Biosci. 2022, 9, 972008. [Google Scholar] [CrossRef]
- Compression Measurement of Foam with Microindentation. Available online: https://nanovea.com/App-Notes/compressionmeasurement.pdf (accessed on 31 January 2024).
- Xu, D.; Harvey, T.; Begiristain, E.; Domínguez, C.; Sánchez-Abella, L.; Browne, M.; Cook, R.B. Measuring the elastic modulus of soft biomaterials using nanoindentation. J. Mech. Behav. Biomed. Mater. 2022, 133, 105329. [Google Scholar] [CrossRef]
- Webber, R.E.; Shull, K.R. Strain Dependence of the Viscoelastic Properties of Alginate Hydrogels. Macromolecules 2004, 37, 6153–6160. [Google Scholar] [CrossRef]
- Caccavo, D.; Cascone, S.; Lamerti, G.; Barba, A.A. Hydrogels: Experimental characterization and mathematical modelling of their mechanical and diffusive behaviour. Chem. Soc. Rev. 2018, 47, 2357–2373. [Google Scholar] [CrossRef]
- Serra-Aguila, A.; Puigoriol-Forcada, J.M.; Reyes, G.; Menacho, J. Viscoelastic models revisited: Characteristics and interconversion formulas for generalized Kelvin–Voigt and Maxwell models. Acta Mech. Sin. 2019, 35, 1191–1209. [Google Scholar] [CrossRef]
- Analysis of Deformation in Solid Mechanics. Available online: https://www.comsol.com/multiphysics/analysis-of-deformation#strain-tensors (accessed on 31 January 2024).
- Structural Contact Modeling Guidelines. Available online: https://www.comsol.com/support/knowledgebase/1102 (accessed on 31 January 2024).
- Clear Resin. Available online: https://formlabs.com/store/materials/clear-resin/ (accessed on 31 January 2024).
- Teotia, M.; Chauhan, M.; Choudhary, P.; Soni, R.K. Photocured characteristics of fast photocurable acrylic formulations and investigations by differential photo calorimeter. J. Therm. Anal. Calorim. 2019, 137, 133–141. [Google Scholar] [CrossRef]
- Kury, M.; Ehrmann, K.; Gorsche, C.; Dorfinger, P.; Koch, T.; Stampfl, J.; Liska, R. Regulated acrylate networks as tough photocurable materials for additive manufacturing. Polym. Int. 2022, 71, 897–905. [Google Scholar] [CrossRef]
- Plexiglas® G Cell-Cast Acrylic Sheet. Available online: https://associatedplastics.com/forms/acrylic_plastics_data.pdf (accessed on 31 January 2024).
- COI Durometer Conversion Chart. Available online: https://www.coirubber.com/wp-content/uploads/2017/07/COI-Durometer-Conversion-Chart.pdf (accessed on 31 January 2024).
- Rubber Hardness Chart. Available online: https://mykin.com/rubber-hardness-chart (accessed on 31 January 2024).
- ASTM D2240 Durometer Hardness. Available online: https://www.plantech.com/wp-content/uploads/2017/05/ASTM-D2240-Durometer-Hardness.pdf (accessed on 31 January 2024).
- Malektaj, H.; Drozdov, A.D.; deClaville Christiansen, J. Mechanical Properties of Alginate Hydrogels Cross-Linked with Multivalent Cations. Polymers 2023, 15, 3012. [Google Scholar] [CrossRef]
- Brus, J.; Urbanova, M.; Czernek, J.; Pavelkova, M.; Kubova, K.; Vyslouzil, J.; Abbrent, S.; Konefal, R.; Horsky, J.; Vetchy, D.; et al. Structure and Dynamics of Alginate Gels Cross-Linked by Polyvalent Ions Probed via Solid State NMR Spectroscopy. Biomacromolecules 2017, 18, 2478–2488. [Google Scholar] [CrossRef]
- Tong, R.; Ma, Z.; Yao, R.; Gu, R.; Li, T.; Liu, L.; Guo, F.; Zeng, M.; Xu, J. Stretchable and transparent alginate ionic gel film for multifunctional sensors and devices. Int. J. Biol. Macromol. 2023, 246, 125667. [Google Scholar] [CrossRef]
- Gryshkov, O.; Pogozhykh, D.; Hofmann, N.; Pogozhykh, O.; Mueller, T.; Glasmacher, B. Encapsulating Non-Human Primate Multipotent Stromal Cells in Alginate via High Voltage for Cell-Based Therapies and Cryopreservation. PLoS ONE 2014, 9, e0107911. [Google Scholar] [CrossRef]
- Hwang, Y.S.; Cho, J.; Tay, F.; Heng, J.Y.; Ho, R.; Kazarian, S.G.; Williams, D.R.; Boccaccini, A.R.; Polak, J.M.; Mantalaris, A. The use of murine embryonic stem cells, alginate encapsulation, and rotary microgravity bioreactor in bone tissue engineering. Biomaterials 2009, 30, 499–507. [Google Scholar] [CrossRef]
- Chan, E.S.; Lim, T.; Voo, W.; Ravindra, P.; Tey, B.T.; Zhang, Z. Effect of formulation of alginate beads on their mechanical behavior and stiffness. Particuology 2011, 9, 228–234. [Google Scholar] [CrossRef]
- Kaklamani, G.; Cheneler, D.; Grover, L.M.; Adams, M.J.; Bowen, J. Mechanical properties of alginate hydrogels manufactured using external gelation. J. Mech. Behav. Biomed. Mater. 2014, 36, 135–142. [Google Scholar] [CrossRef]
- del Pilar Martin, M.; Weldon, W.C.; Zarnitsyn, V.G.; Koutsonanos, D.G.; Akbari, H.; Skountzou, I.; Jacob, J.; Prausnitz, M.R.; Compans, R.W. Local response to microneedle-based influenza immunization in the skin. mBio 2012, 3, e00012-12. [Google Scholar] [CrossRef]
- Strange, D.G.T.; Fletcher, T.L.; Tonsomboom, K.; Brawn, H.; Zhao, X.; Oyen, M.L. Separating poroviscoelastic deformation mechanisms in hydrogels. Appl. Phys. Lett. 2013, 102, 031913. [Google Scholar] [CrossRef]
- Cai, S.; Hu, Y.; Zhao, X.; Suo, Z. Poroelasticity of a covalently crosslinked alginate hydrogel under compression. J. Appl. Phys. 2010, 180, 113514. [Google Scholar] [CrossRef]
- Ahearne, M.; Siamantouras, E.; Yang, Y.; Lui, K.-K. Mechanical Characterization of biomimetic membranes by micro-shaft. Poking. J. R. Soc. Interface 2009, 6, 471–478. [Google Scholar] [CrossRef]



| Disease/ Application | Cell Type | Microneedle Type | Material/ Fabrication | Penetration Depth | Citation |
|---|---|---|---|---|---|
| Melanoma tumors | Ovalbumin-pulsed dendritic cells | CryoMN | Cryogenic medium (PBS supplemented with 2.5% DMSO and 100 mM sucrose), molding | ~20 μm to 200 μm | [9] |
| Cell transplantation | Human epidermal melanocytes, follicle dermal papilla cells, corneal keratocytes, corneal epithelial cells | HMN | Poly(methyl methacrylate) (PMMA), molding | 300 μm to 500 μm | [30] |
| Heart regeneration after acute myocardial infarction (MI) | Cardiac stromal cells (CSCs) | Porous MN | Poly(vinyl alcohol) (PVA), micromolding | 400 μm to 500 μm | [31] |
| Loading and intradermal delivery | Mesenchymal stem cells (MSCs), melanoctyes, antigen-pulsed dendritic cells | Porous MN | Methacrylated hyaluronic acid (MeHA), molding | ~50 μm to 200 μm | [32] |
| Insulin delivery (diabetes mellitus) | Alginate-encapsulated pancreatic β-cells | Hydrogel MN | Hyaluronic acid (HA) matrix containing glucose-signal amplifiers (GSAs), micromolding | ~200 μm | [33] |
| Material | Dimensions | Substrate | Index of Biomechanics | Citation |
|---|---|---|---|---|
| Thermoplastic | H = 700 μm Tip length = 150 μm Tip taper angle = 63.4° Reservoir depth = 180 μm Open channel = 30 μm * | Rabbit Skin | Bending force, dynamic loading tests and yield strength, axial compression testing of ~10 N for displacement of ~400 μm | [24] |
| Stainless steel and poly(lactic-co-glycolic) acid (PLGA) | H = 600 μm Dbase = 300 μm Dbore = 90 μm | N/A ** | Buckling analysis for asymmetric hollow structures, failure of stainless steel HMN at 0.16 N and PLGA HMN at 0.19 N | [54] |
| Polyvinyl alcohol | H = 600 μm Dbase = 200 μm Dtip = 30 μm Dbore = 25 μm | N/A ** | Axial and bending loading, force, and stress analysis, with bending force of 0.1788 N at the tip of HMN | [56] |
| Clear resin V4 (acrylate based) | H = 600 μm Dbase = 1000 μm Dtip = 400 μm | N/A ** | Defective and clogged orifices | [57] |
| Variable | Setting |
|---|---|
| d * (µm) | 800 |
| H * (mm) | 3 |
| Print Angle (°) | 0 |
| Cleaning time (min) | 20 |
| Curing time (min) | 10 |
| h3 * (µm) | 150 |
| AR * | 1.875 |
| Runs | Amp (mm) | Rate (mm/s) | Delay (s) |
|---|---|---|---|
| A | 0.10 | 0.05 | 1.0 |
| B | 0.15 | 0.10 | 1.5 |
| C | 0.20 | 0.20 | 2.0 |
| D | 0.25 | 0.50 | 2.5 |
| Film | S (N/mm) | H (N/mm2) | δ (°) | E (Pa) | ν |
|---|---|---|---|---|---|
| 1 | 900 | 31 | 11 | 41,000 | 0.39 |
| 2 | 1225 | 36.5 | 5 | 46,500 | 0.39 |
| Run | F∞ (N) | Fmax (N) | R2 | Run | F∞ (N) | Fmax (N) | R2 |
|---|---|---|---|---|---|---|---|
| A | 0.0734 | 0.4851 | 0.9973 | AS | 0.2239 | 1.23 | 0.9967 |
| B | 0.1233 | 1.2691 | 0.9934 | BS | 0.5514 | 3.648 | 0.9922 |
| C | 0.1771 | 1.6807 | 0.9902 | CS | 0.461 | 3.1164 | 0.9962 |
| D | 0.9256 | 2.8028 | 0.9832 | DS | 0.5627 | 4.4492 | 0.998 |
| Run | E∞ (Pa) | Emax (Pa) | E1 (Pa) | E2 (Pa) | τ1 (s) | τ2 (s) |
|---|---|---|---|---|---|---|
| A | 4.04 × 104 | 2.67 × 105 | 1.23 × 105 | 7.87 × 104 | 1.9 | 25.6 |
| B | 6.23 × 104 | 6.41 × 105 | 2.52 × 105 | 2.27 × 105 | 1.2 | 22.1 |
| C | 8.24 × 104 | 7.82 × 105 | 3.70 × 105 | 2.07 × 105 | 0.6 | 15.3 |
| D | 3.68 × 105 | 1.12 × 106 | 1.64 × 105 | 3.26 × 104 | 0.3 | 12.2 |
| AS | 1.23 × 105 | 6.78 × 105 | 2.94 × 105 | 2.49 × 105 | 2.1 | 26.7 |
| BS | 2.65 × 105 | 1.75 × 106 | 4.49 × 105 | 6.74 × 105 | 1.2 | 22.1 |
| CS | 1.97 × 105 | 1.33 × 106 | 5.10 × 105 | 3.95 × 105 | 1.2 | 16.8 |
| DS | 2.03 × 105 | 1.61 × 106 | 7.30 × 105 | 3.82 × 105 | 0.5 | 13.4 |
| Run | HMNS Baseline ρ (g/cm3) | HMNS Post ρ (g/cm3) | p-Value (α = 0.05) | HMN Baseline ρ (g/cm3) | HMN Post ρ (g/cm3) | p-Value (α = 0.05) |
|---|---|---|---|---|---|---|
| BS/B | 1.4120 ± 0.0261 | 0.1691 ± 0.0010 | 9.69 × 10−5 | 1.4092 ± 0.0085 | 0.1500 ± 0.0017 | 6.08 × 10−6 |
| Run | Actual Force (N) | Simulated Original Configuration (N) | Simulated Proposed Configuration (N) |
|---|---|---|---|
| A | 0.485 | 0.7 * | N/A ** |
| B | 1.27 | 5.5 | N/A ** |
| C | 1.68 | 2700 | N/A ** |
| AS | 1.23 | 0.95 * | 1.52 |
| BS | 3.65 | 0.28 | 1.75 |
| CS | 3.12 | 0.6 | 1.85 |
| Area | Key Findings | Recommendations |
|---|---|---|
| SLA and Dimensional Verification |
|
|
| Skin Phantom Fabrication and Biomechanical Tests |
|
|
| Puncture Characterization |
|
|
| Simulation |
|
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Defelippi, K.M.; Kwong, A.Y.S.; Appleget, J.R.; Altay, R.; Matheny, M.B.; Dubus, M.M.; Eribes, L.M.; Mobed-Miremadi, M. An Integrated Approach to Control the Penetration Depth of 3D-Printed Hollow Microneedles. Appl. Mech. 2024, 5, 233-259. https://doi.org/10.3390/applmech5020015
Defelippi KM, Kwong AYS, Appleget JR, Altay R, Matheny MB, Dubus MM, Eribes LM, Mobed-Miremadi M. An Integrated Approach to Control the Penetration Depth of 3D-Printed Hollow Microneedles. Applied Mechanics. 2024; 5(2):233-259. https://doi.org/10.3390/applmech5020015
Chicago/Turabian StyleDefelippi, Kendall Marie, Allyson Yuuka Saumei Kwong, Julia Rose Appleget, Rana Altay, Maya Bree Matheny, Mary Margaret Dubus, Lily Marie Eribes, and Maryam Mobed-Miremadi. 2024. "An Integrated Approach to Control the Penetration Depth of 3D-Printed Hollow Microneedles" Applied Mechanics 5, no. 2: 233-259. https://doi.org/10.3390/applmech5020015
APA StyleDefelippi, K. M., Kwong, A. Y. S., Appleget, J. R., Altay, R., Matheny, M. B., Dubus, M. M., Eribes, L. M., & Mobed-Miremadi, M. (2024). An Integrated Approach to Control the Penetration Depth of 3D-Printed Hollow Microneedles. Applied Mechanics, 5(2), 233-259. https://doi.org/10.3390/applmech5020015





