High Expansion Auxetic Skin Graft Simulants for Severe Burn Injury Mitigation
Abstract
1. Introduction
2. Materials and Methods
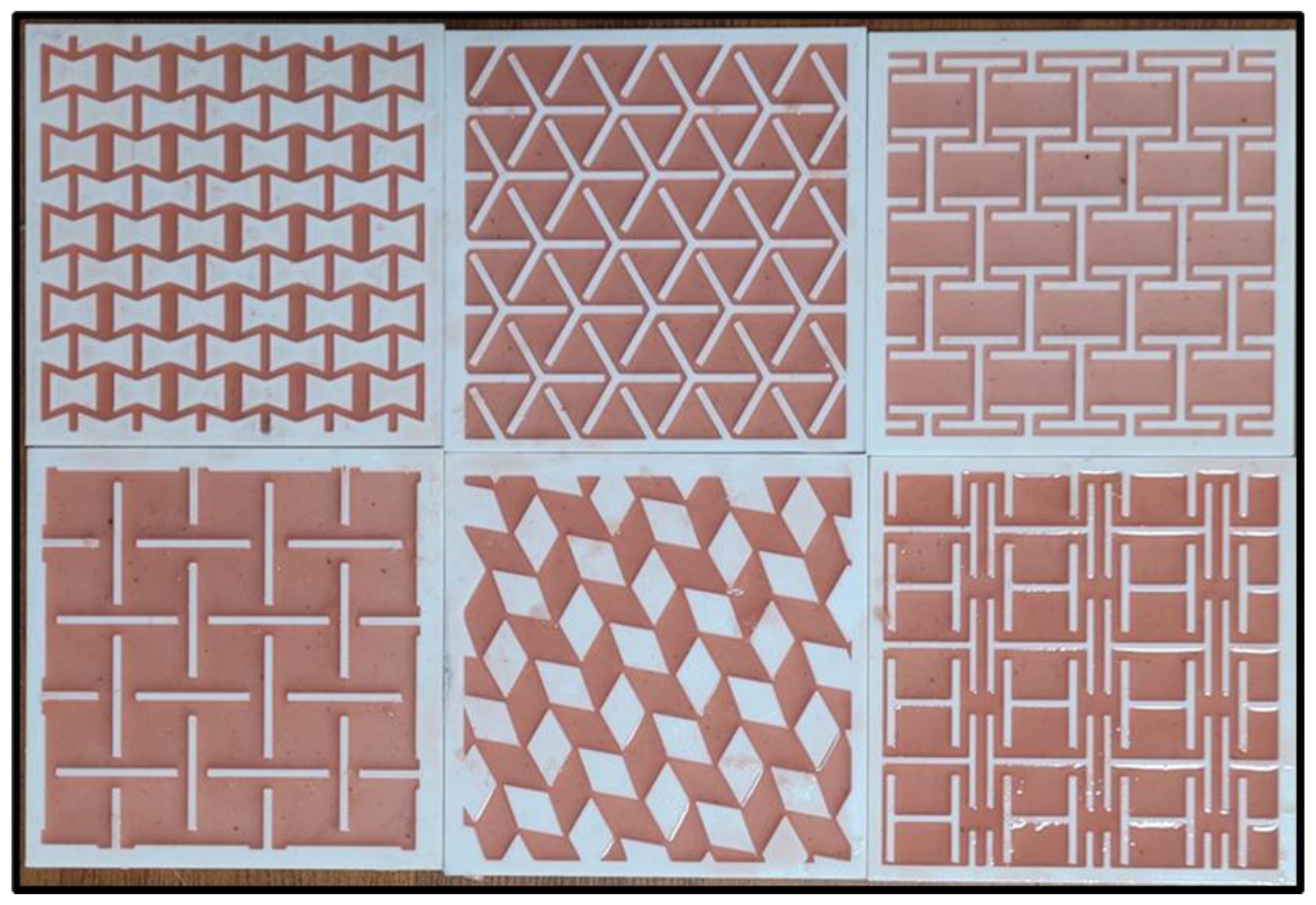
2.1. Design and Fabrication of Auxetic Skin Graft Simulants
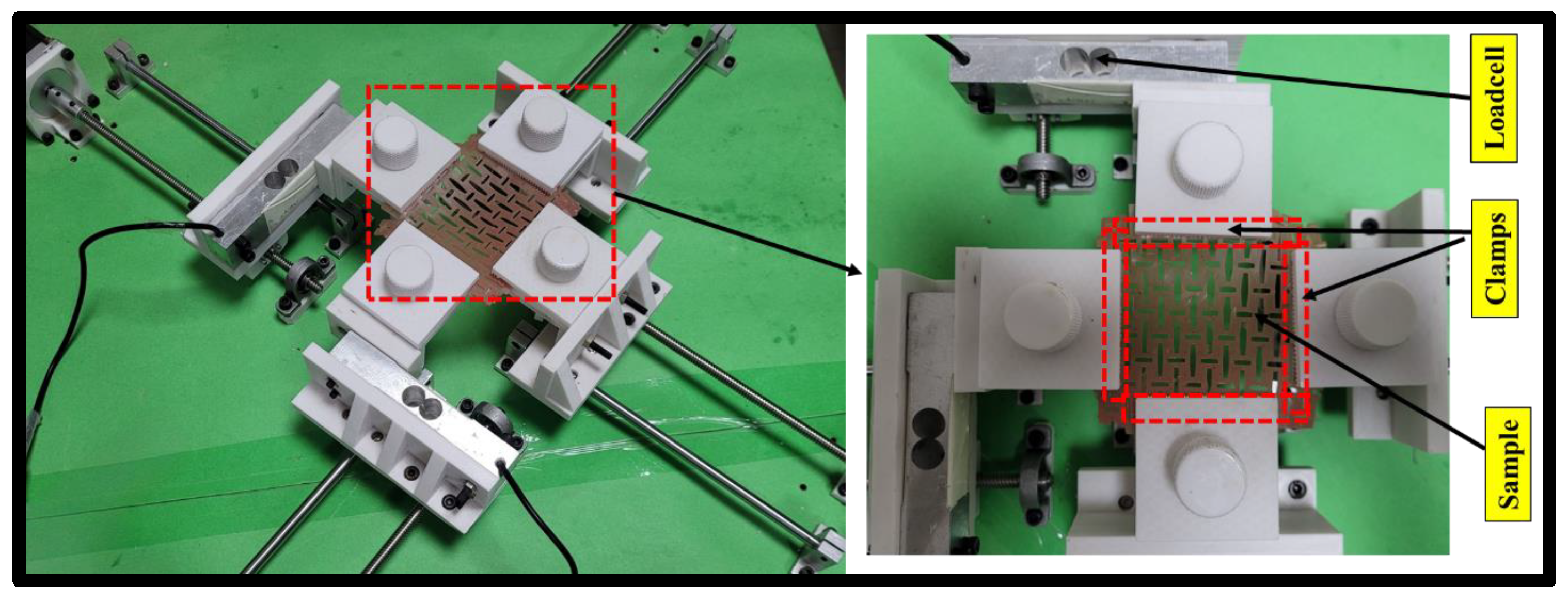
2.2. Design and Development of Planar Biaxial Tester
2.3. Material Modelling
3. Results and Discussions
3.1. Deformation Analysis of Auxetic Skin Graft Simulants
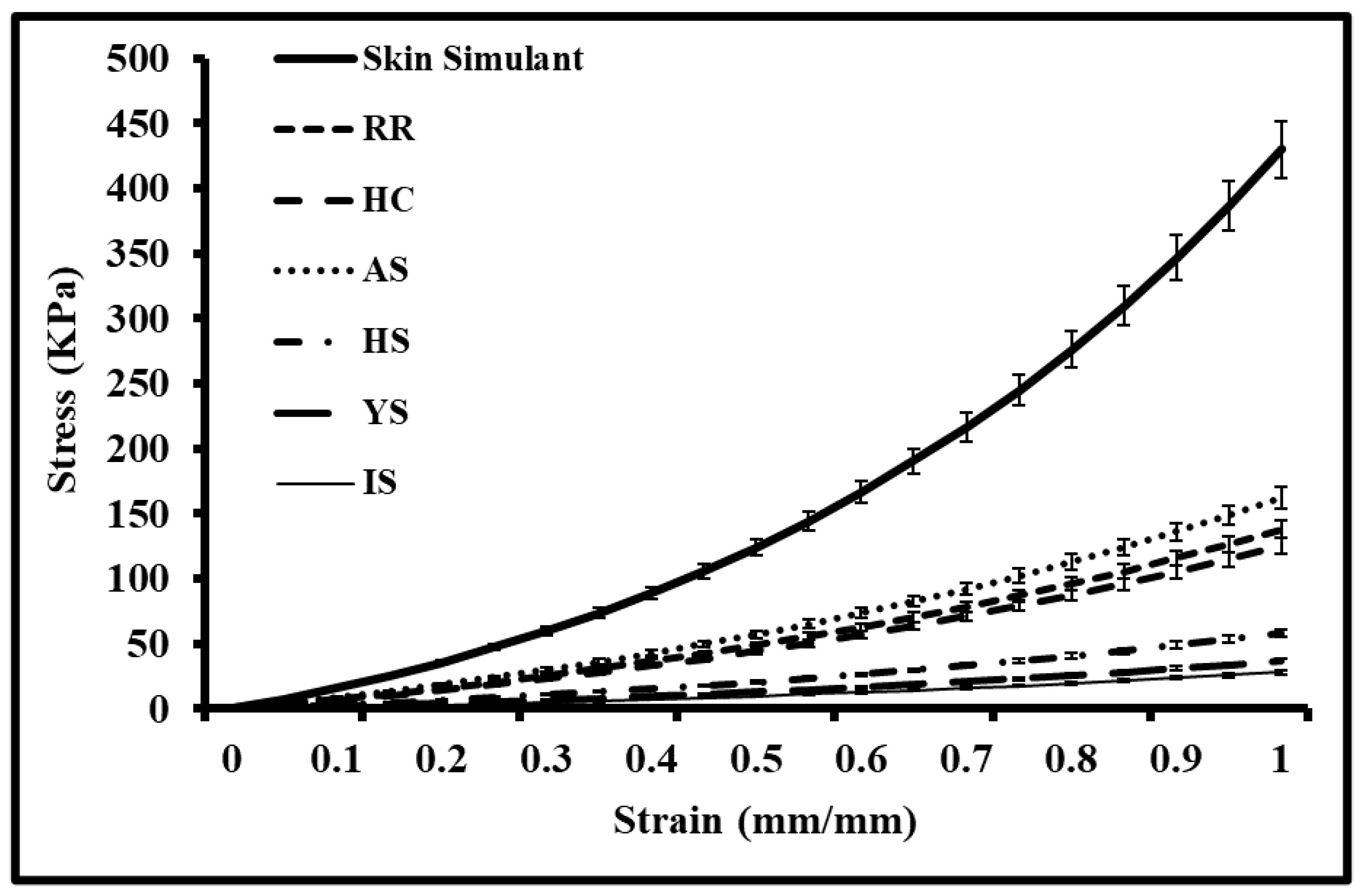
3.2. Stress Analysis of Auxetic Skin Graft Simulants
3.3. Meshing Ratio Analysis of Auxetic Skin Graft Simulants
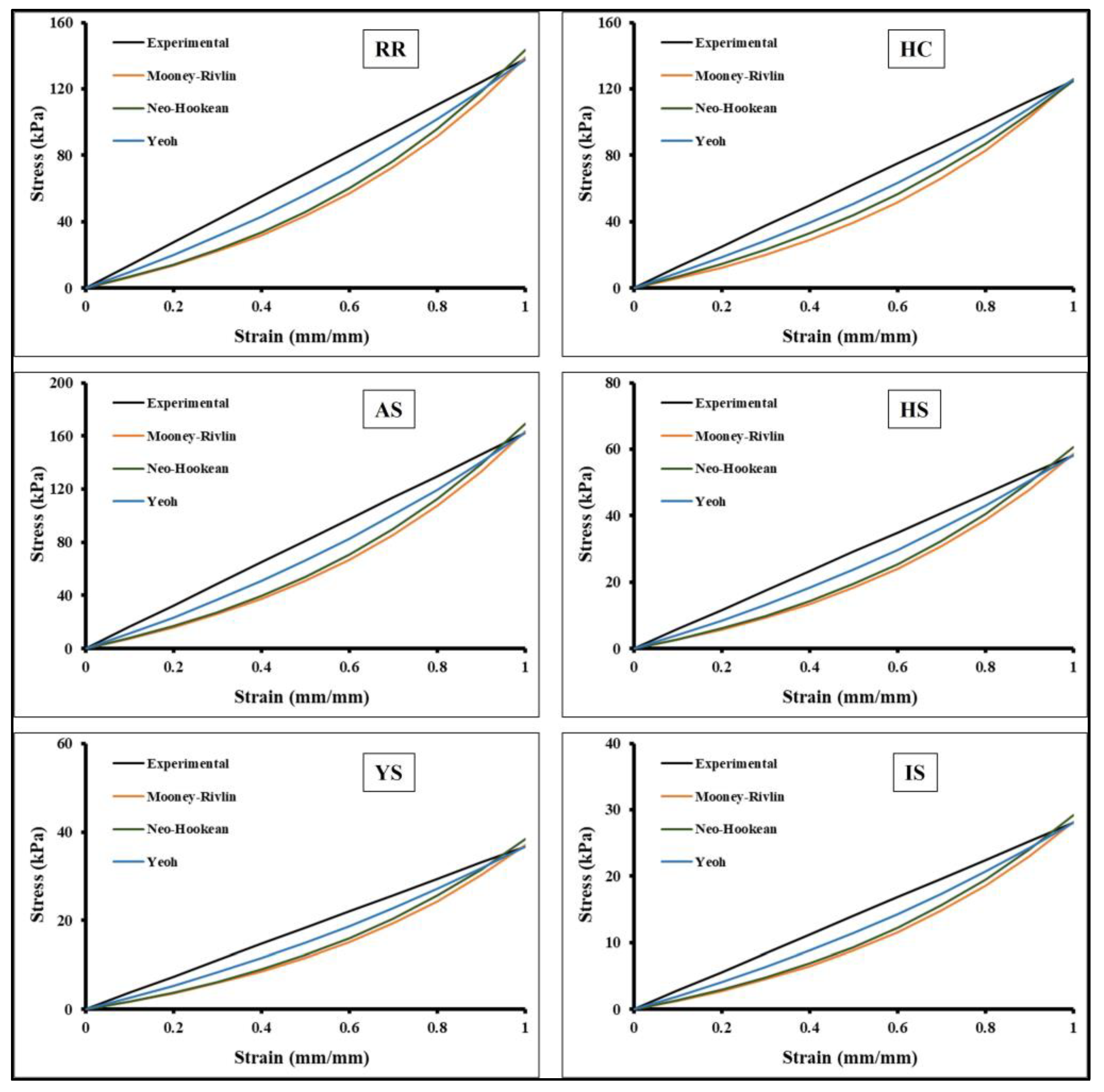
3.4. Hyperelastic Non-linear Curve Fits of Auxetic Skin Graft Simulants
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- WHO—World Health Organization. Burns. Available online: https://www.who.int/ (accessed on 1 July 2022).
- Wong, L.; Rajandram, R.; Allorto, N. Systematic review of excision and grafting in burns: Comparing outcomes of early and late surgery in low and high-income countries. Burns 2021, 47, 1705–1713. [Google Scholar] [CrossRef]
- Gupta, V.; Chanda, A. Expansion potential of skin grafts with novel I-shaped auxetic incisions. Biomed. Phys. Eng. Express 2022, 8, 100071. [Google Scholar] [CrossRef] [PubMed]
- Capek, L.; Flynn, C.; Molitor, M.; Chong, S.; Henys, P. Graft orientation influences meshing ratio. Burns 2018, 44, 1439–1445. [Google Scholar] [CrossRef] [PubMed]
- Bogdanov, S.B.; Gilevich, I.V.; Melkonyan, K.I.; Sotnichenko, A.S.; Alekseenko, S.N.; Porhanov, V.A. Total full-thickness skin grafting for treating patients with extensive facial burn injury: A 10-year experience. Burns 2021, 47, 1389–1398. [Google Scholar] [CrossRef]
- Oh, S.J. A systematic review of the scalp donor site for splitthickness skin grafting. Arch. Plast. Surg. 2020, 47, 528–534. [Google Scholar] [CrossRef]
- Pripotnev, S.; Papp, A. Split thickness skin graft meshing ratio indications and common practices. Burns 2017, 43, 1775–1781. [Google Scholar] [CrossRef] [PubMed]
- Stan, D.; Tanase, C.; Avram, M.; Apetrei, R.; Mincu, N.B.; Mateescu, A.L.; Stan, D. Wound healing applications of creams and “smart” hydrogels. Exp. Dermatol. 2021, 30, 1218–1232. [Google Scholar] [CrossRef]
- Gupta, V.; Chanda, A. Biomechanics of skin grafts: Effect of pattern size, spacing and orientation. Eng. Res. Express 2022, 4, 015006. [Google Scholar] [CrossRef]
- Peeters, R.; Hubens, A. The mesh skin graft-true expansion rate. Burns 1988, 14, 239–240. [Google Scholar] [CrossRef]
- Sarvazyan, A.P.; Skovoroda, A.R.; Emelianov, S.Y.; Fowlkes, J.B.; Pipe, J.G.; Adler, R.S.; Buxton, R.B.; Carson, P.L. Biophysical Bases of Elasticity Imaging. Acoust. Imaging 1995, 06, 223–240. [Google Scholar] [CrossRef]
- Singh, G.; Chanda, A. Mechanical properties of whole-body soft human tissues: A review. Biomed. Mater. 2021, 16, 062004. [Google Scholar] [CrossRef] [PubMed]
- Grgić, I.; Wertheimer, V.; Karakašić, M.; Ivandić, Ž. 3D printed clamps for in vitro tensile tests of human gracilis and the superficial third of quadriceps tendons. Appl. Sci. 2021, 11, 2563. [Google Scholar] [CrossRef]
- Zemánek, M.; Burša, J.; Děták, M. Biaxial tension tests with soft tissues of arterial wall. Eng. Mech. 2009, 16, 3–11. [Google Scholar]
- Chanda, A. Biomechanical modeling of human skin tissue surrogates. Biomimetics 2018, 3, 18. [Google Scholar] [CrossRef] [PubMed]
- Bhatnagar, N.; Bhardwaj, R.; Selvakumar, P.; Brieu, M. Development of a biaxial tensile test fixture for reinforced thermoplastic composites. Polym. Test. 2007, 26, 154–161. [Google Scholar] [CrossRef]
- Xue, Y.; Ravishankar, P.; Zeballos, M.A.; Sant, V.; Balachandran, K.; Sant, S. Valve leaflet-inspired elastomeric scaffolds with tunable and anisotropic mechanical properties. Polym. Adv. Technol. 2020, 31, 94–106. [Google Scholar] [CrossRef]
- Androulidakis, C.; Koukaras, E.N.; Parthenios, J.; Kalosakas, G.; Papagelis, K.; Galiotis, C. Graphene flakes under controlled biaxial deformation. Sci. Rep. 2015, 5, 18219. [Google Scholar] [CrossRef]
- Billiar, K.L.; Sacks, M.S. Biaxial mechanical properties of the natural and glutaraldehyde treated aortic valve cusp—Part I: Experimental results. J. Biomech. Eng. 2000, 122, 23–30. [Google Scholar] [CrossRef]
- Ravishankar, P.; Ozkizilcik, A.; Husain, A.; Balachandran, K. Anisotropic Fiber-Reinforced Glycosaminoglycan Hydrogels for Heart Valve Tissue Engineering. Tissue Eng.–Part A 2021, 27, 513–525. [Google Scholar] [CrossRef] [PubMed]
- Sutula, D.; Henyš, P.; Čapek, L. Optimal structural pattern for maximal compliance using topology optimization based on phasefields: Application to improve skin graft meshing efficiency. Int. J. Numer. Methods Biomed. Eng. 2020, 36, e3405. [Google Scholar] [CrossRef] [PubMed]
- Sacks, M.S. Biaxial mechanical evaluation of planar biological materials. J. Elast. 2000, 61, 199–246. [Google Scholar] [CrossRef]
- Ní Annaidh, A.; Bruyère, K.; Destrade, M.; Gilchrist, M.D.; Otténio, M. Characterization of the anisotropic mechanical properties of excised human skin. J. Mech. Behav. Biomed. Mater. 2012, 5, 139–148. [Google Scholar] [CrossRef] [PubMed]
- Vandeput, J.; Nelissen, M.; Tanner, J.C.; Boswick, J. A review of skin meshers. Burns 1995, 21, 364–370. [Google Scholar] [CrossRef] [PubMed]
- Egro, F.M.; Roy, E.; Konanur, A.; Murphy, C.; Corcos, A.C.; Ziembicki, J.A. Split-thickness Skin Graft Meshing: The True Mesh Ratio. J. Burn Care Res. 2020, 41, S229–S230. [Google Scholar] [CrossRef]
- Singh, G.; Gupta, V.; Chanda, A. Mechanical Characterization of Rotating Triangle Shaped Auxetic Skin Graft Simulants. Facta Univ. Ser. Mech. Eng. 2022, 1–16. [Google Scholar] [CrossRef]
- Novak, N.; Vesenjak, M.; Ren, Z. Auxetic cellular materials—A review. Stroj. Vestnik/J. Mech. Eng. 2016, 62, 485–493. [Google Scholar] [CrossRef]
- Scholze, M.; Singh, A.; Lozano, P.F.; Ondruschka, B.; Ramezani, M.; Werner, M.; Hammer, N. Utilization of 3D printing technology to facilitate and standardize soft tissue testing. Sci. Rep. 2018, 8, 11340. [Google Scholar] [CrossRef]
- Shi, D.F.; Wang, D.M.; Wang, C.T.; Liu, A. A novel, inexpensive and easy to use tendon clamp for in vitro biomechanical testing. Med. Eng. Phys. 2012, 34, 516–520. [Google Scholar] [CrossRef]
- Vella Wood, M.; Casha, A.; Gatt, A.; Formosa, C.; Chockalingam, N.; Grima, J.N.; Gatt, R. 3D Printed Clamps to Study the Mechanical Properties of Tendons at Low Strains. Phys. Status Solidi Basic Res. 2019, 256, 1800159. [Google Scholar] [CrossRef]
- Wahlsten, A.; Rütsche, D.; Nanni, M.; Giampietro, C.; Biedermann, T.; Reichmann, E.; Mazza, E. Mechanical stimulation induces rapid fibroblast proliferation and accelerates the early maturation of human skin substitutes. Biomaterials 2021, 273, 120779. [Google Scholar] [CrossRef]
- Hosseini, M.; Shafiee, A. Engineering Bioactive Scaffolds for Skin Regeneration. Small 2021, 17, 2101384. [Google Scholar] [CrossRef] [PubMed]
- Critchley, R.; Corni, I.; Wharton, J.A.; Walsh, F.C.; Wood, R.J.K.; Stokes, K.R. A review of the manufacture, mechanical properties and potential applications of auxetic foams. Phys. Status Solidi Basic Res. 2013, 250, 1963–1982. [Google Scholar] [CrossRef]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Veerabagu, U.; Palza, H.; Quero, F. Review: Auxetic Polymer-Based Mechanical Metamaterials for Biomedical Applications. ACS Biomater. Sci. Eng. 2022, 8, 2798–2824. [Google Scholar] [CrossRef]
- Kan, T.; Takahagi, S.; Kawai, M.; Ashizawa, S.; Matsubara, D.; Mizuno, H.; Tanaka, A.; Hide, M. Calculation of practical skin donor area for meshed skin grafting in real-world surgery. Dermatol. Ther. 2020, 33, 3–6. [Google Scholar] [CrossRef]
- Duncan, O.; Shepherd, T.; Moroney, C.; Foster, L.; Venkatraman, P.D.; Winwood, K.; Allen, T.; Alderson, A. Review of auxetic materials for sports applications: Expanding options in comfort and protection. Appl. Sci. 2018, 8, 941. [Google Scholar] [CrossRef]


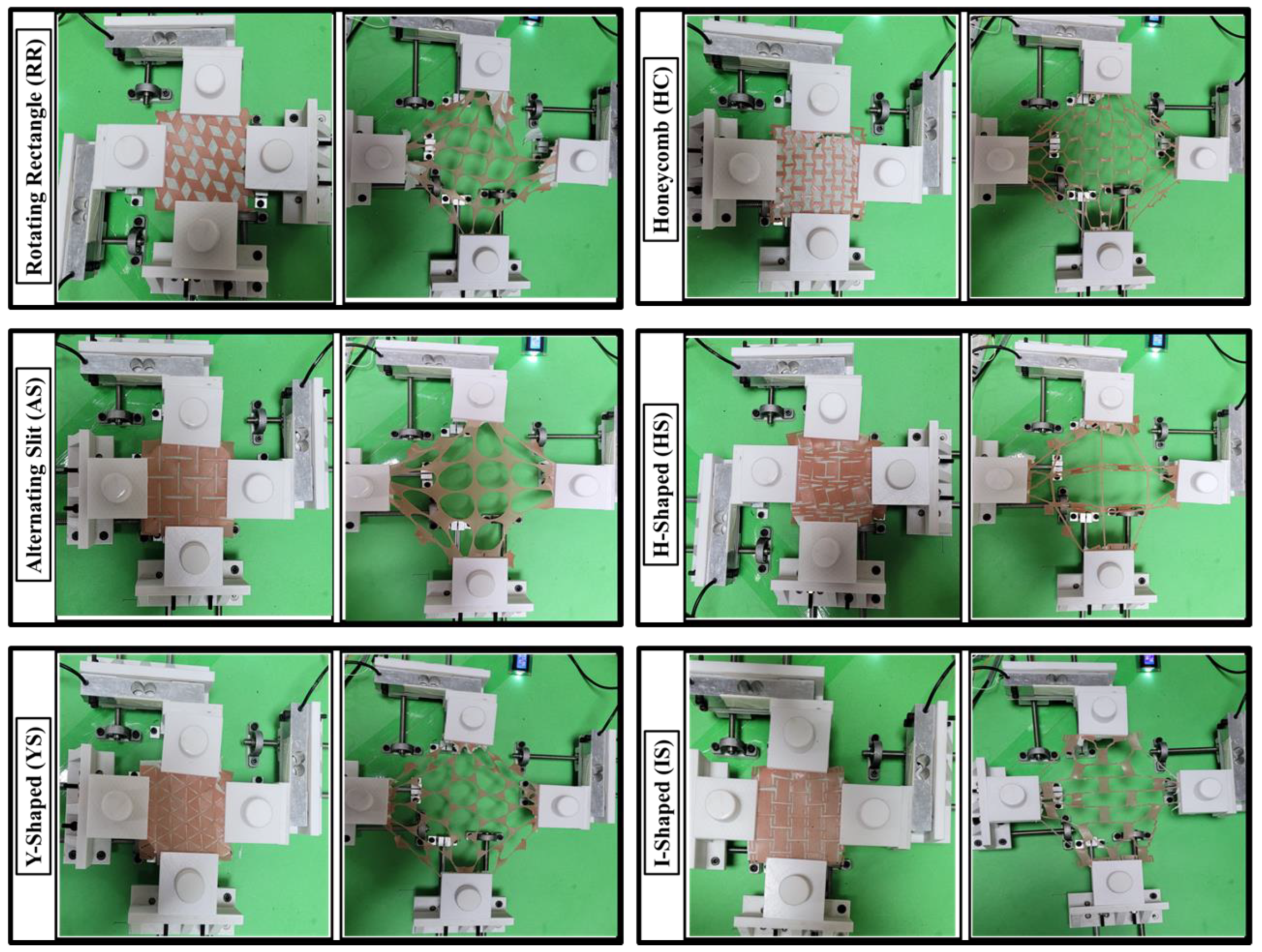

| Design | Initial Void Area (mm2) | Final Void Area (mm2) | % Void Area Increase |
|---|---|---|---|
| AS | 90 | 867 | 863.0 |
| HC | 111.4 | 409.63 | 267.7 |
| HS | 142.49 | 1613.18 | 1032.1 |
| IS | 135.29 | 750.27 | 454.6 |
| RR | 116.36 | 500.62 | 330.2 |
| YS | 122.15 | 785.86 | 543.3 |
| Hyperelastic Model | Curve Fit Coefficient | RR | HC | AS | HS | YS | IS |
|---|---|---|---|---|---|---|---|
| Mooney–Rivlin | c1 | 14.34949 | 13.01418 | 16.87916 | 6.065835 | 3.825152 | 2.917043 |
| c2 | 10.98502 | 09.96286 | 12.92231 | 4.644426 | 2.928595 | 2.233293 | |
| Neo-Hookean | c1 | 20.53203 | 18.62142 | 24.15204 | 8.679786 | 5.473409 | 4.173975 |
| Yeoh | c1 | 23.38589 | 21.22468 | 27.50868 | 9.885044 | 6.233806 | 4.753983 |
| c2 | −0.22559 | −0.20745 | −0.26527 | −0.09521 | −0.06008 | −0.04584 | |
| c3 | 0.001536 | 0.001551 | 0.001802 | 0.000641 | 0.000407 | 0.000311 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gupta, V.; Singh, G.; Chanda, A. High Expansion Auxetic Skin Graft Simulants for Severe Burn Injury Mitigation. Eur. Burn J. 2023, 4, 108-120. https://doi.org/10.3390/ebj4010011
Gupta V, Singh G, Chanda A. High Expansion Auxetic Skin Graft Simulants for Severe Burn Injury Mitigation. European Burn Journal. 2023; 4(1):108-120. https://doi.org/10.3390/ebj4010011
Chicago/Turabian StyleGupta, Vivek, Gurpreet Singh, and Arnab Chanda. 2023. "High Expansion Auxetic Skin Graft Simulants for Severe Burn Injury Mitigation" European Burn Journal 4, no. 1: 108-120. https://doi.org/10.3390/ebj4010011
APA StyleGupta, V., Singh, G., & Chanda, A. (2023). High Expansion Auxetic Skin Graft Simulants for Severe Burn Injury Mitigation. European Burn Journal, 4(1), 108-120. https://doi.org/10.3390/ebj4010011









