Evaluation of Fatigue Life for Dental Implants Using FEM Analysis
Abstract
:1. Introduction
2. Material and Methods
2.1. Preliminary Modeling
2.2. Material Properties
2.3. Finite Element Modeling
3. Result
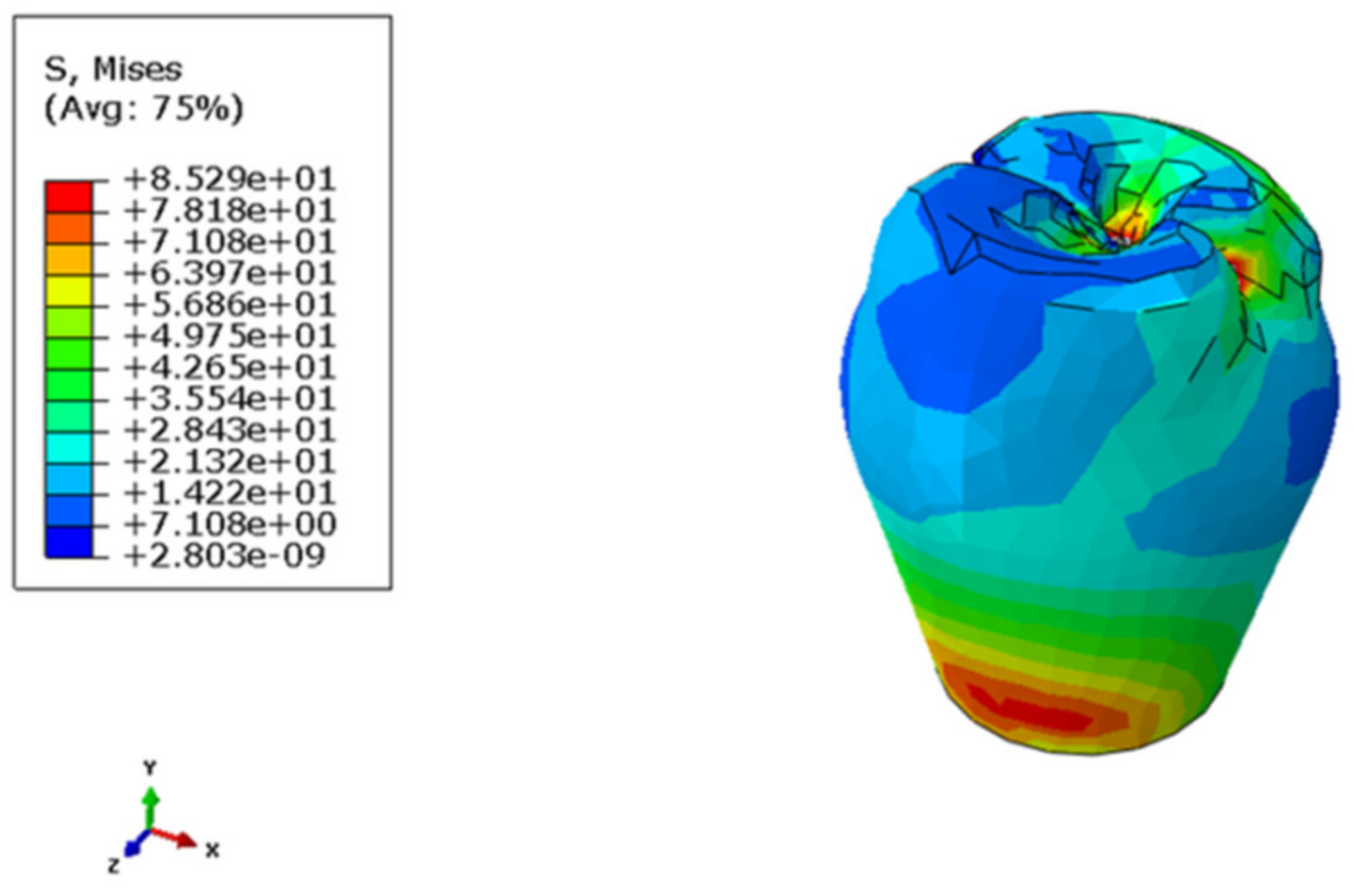
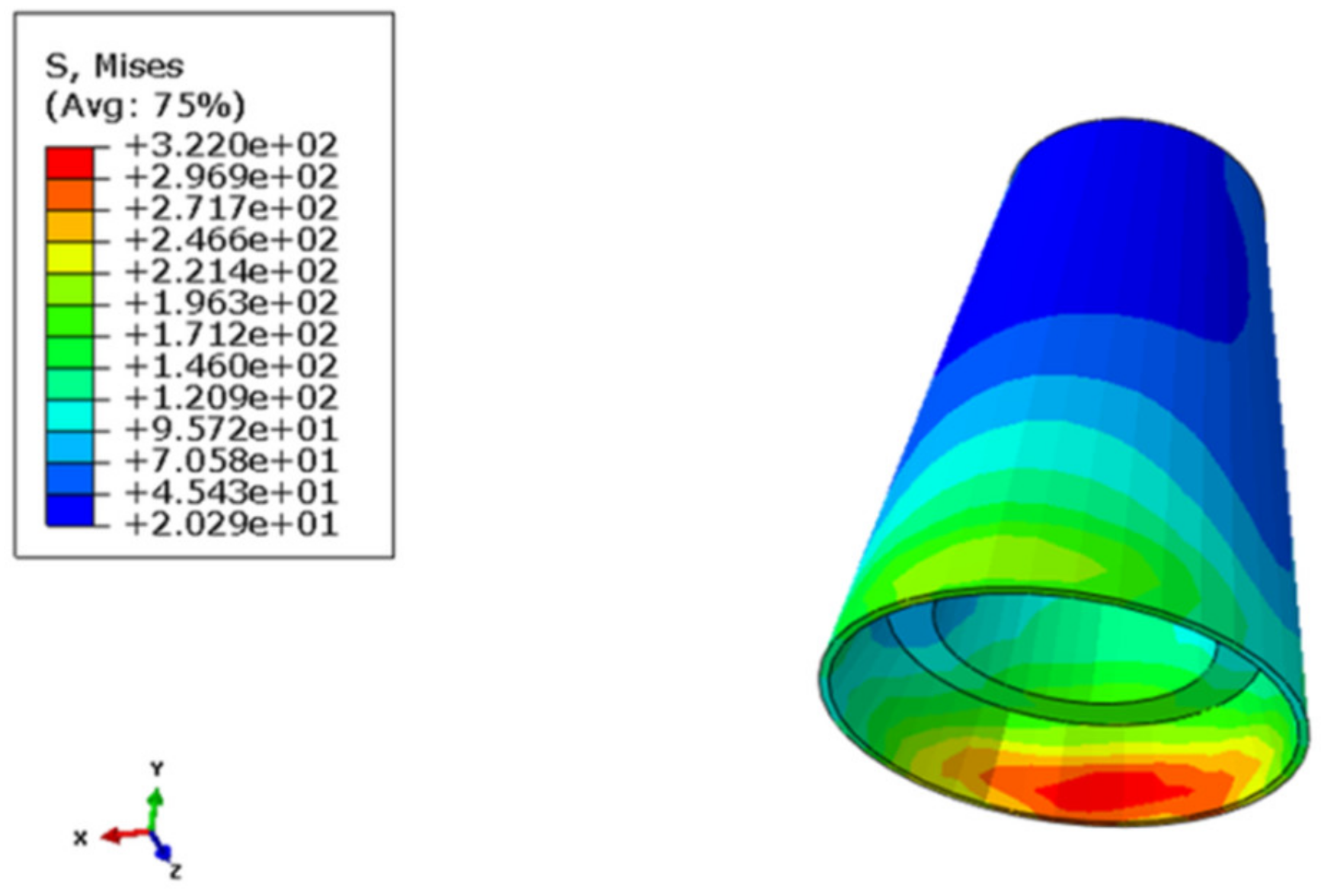
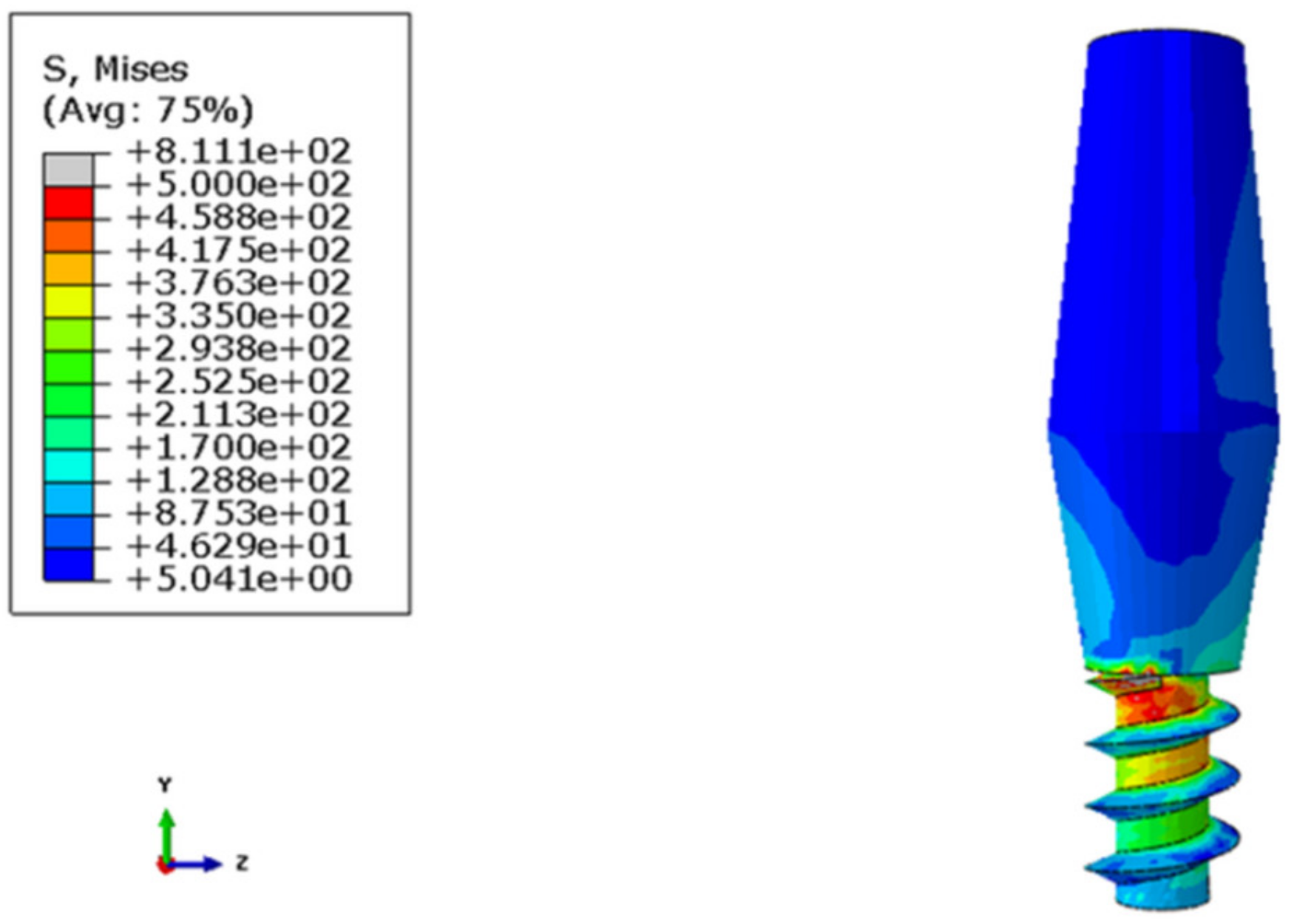
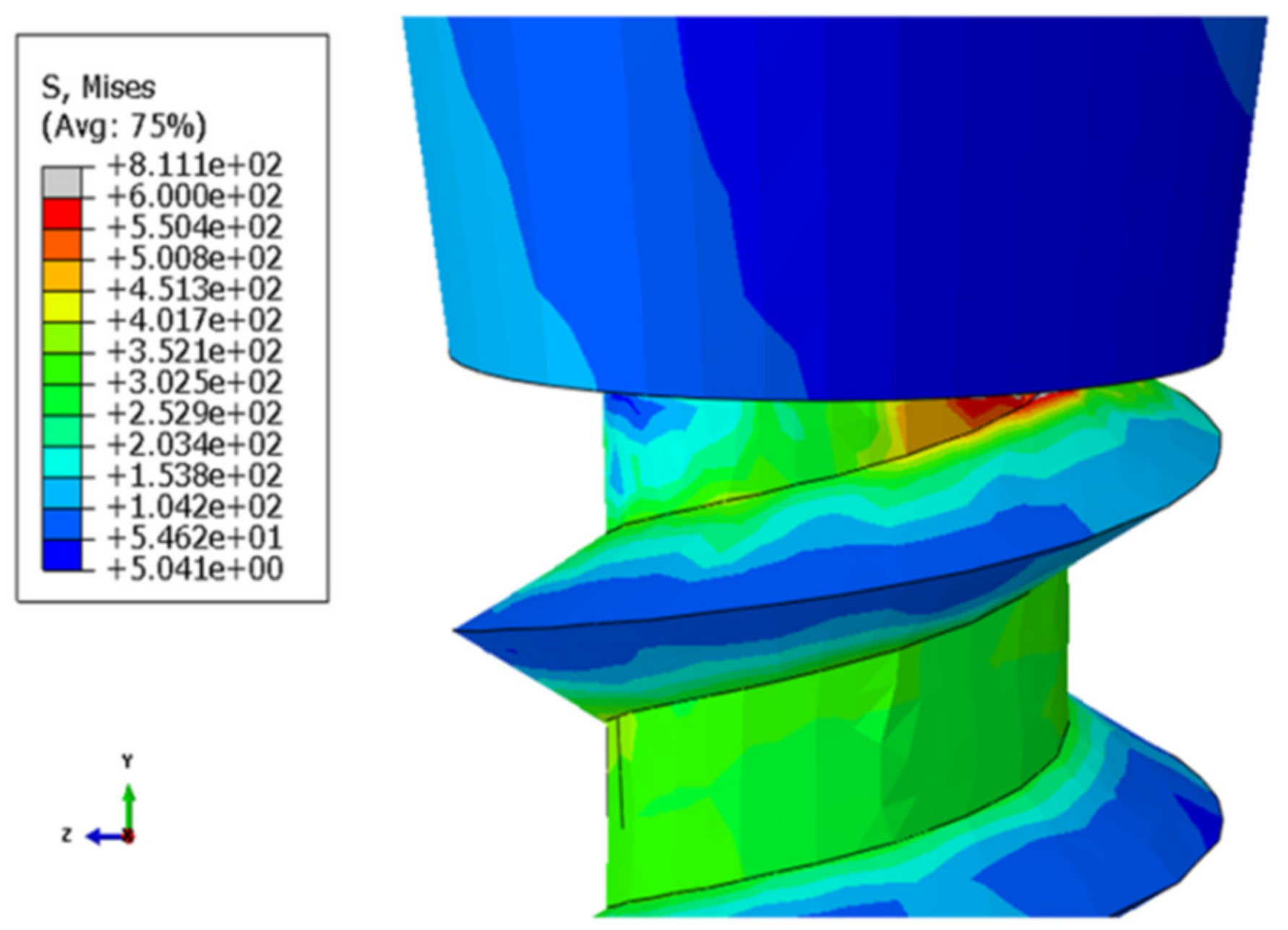
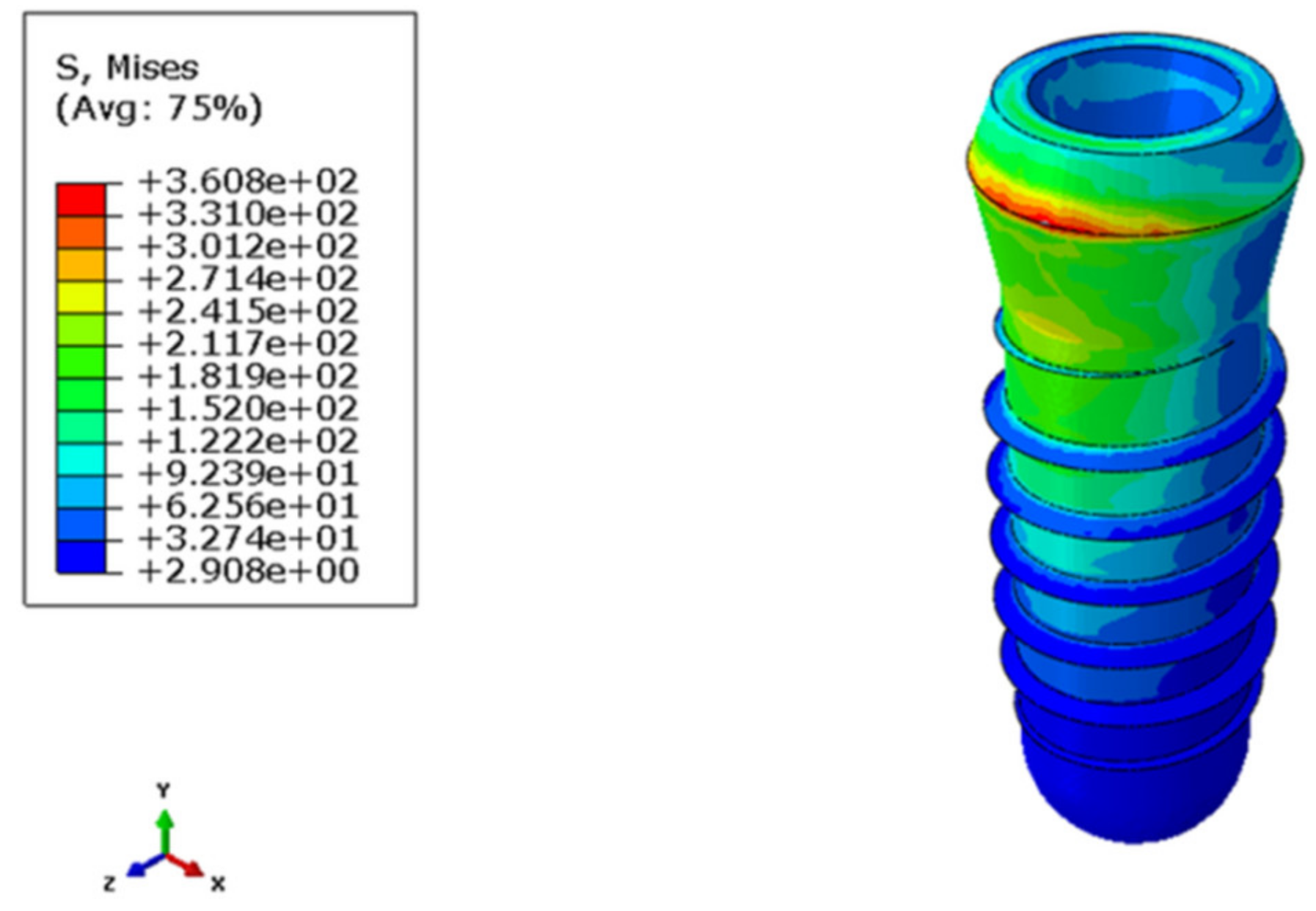
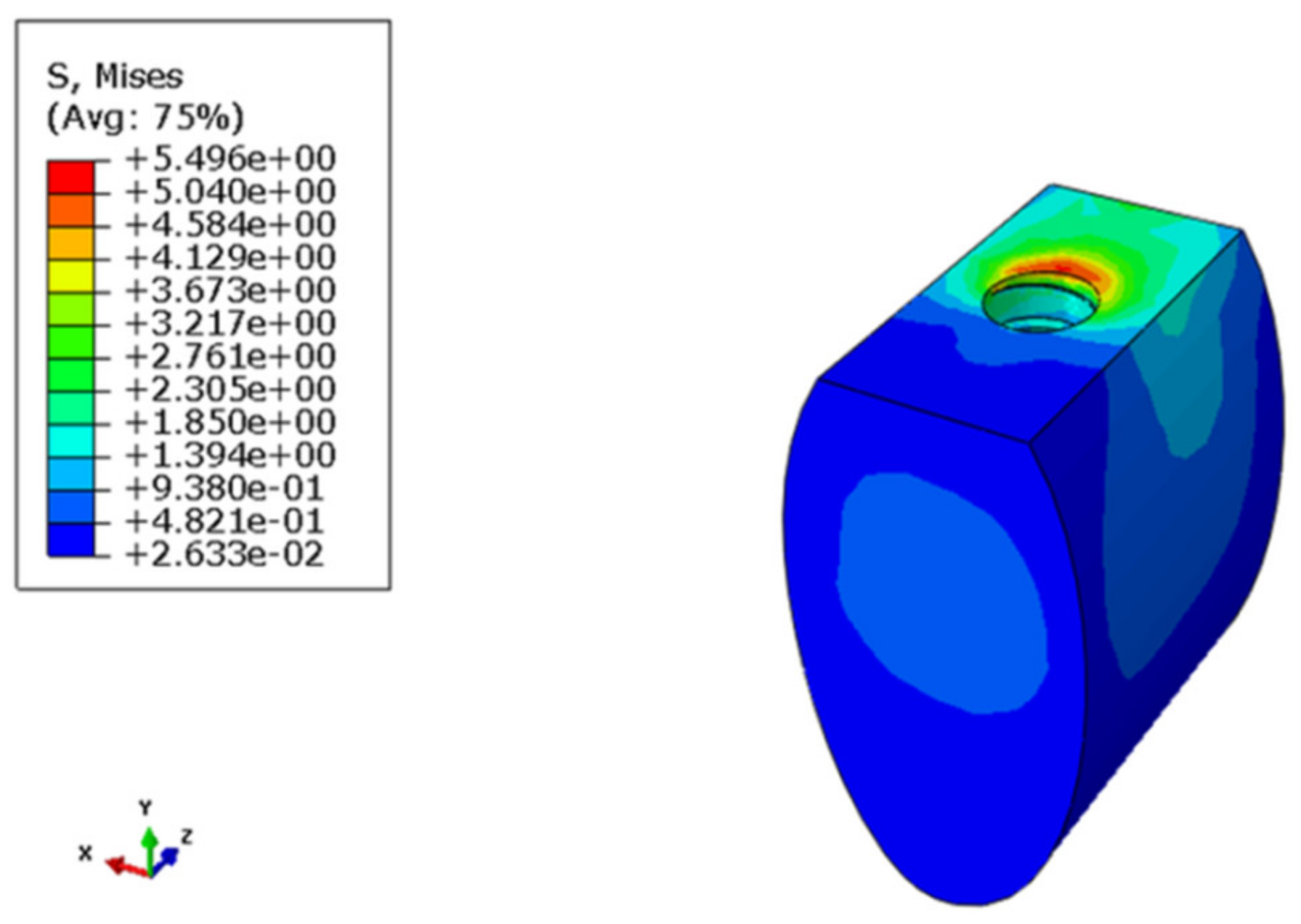
3.1. Stress Analysis
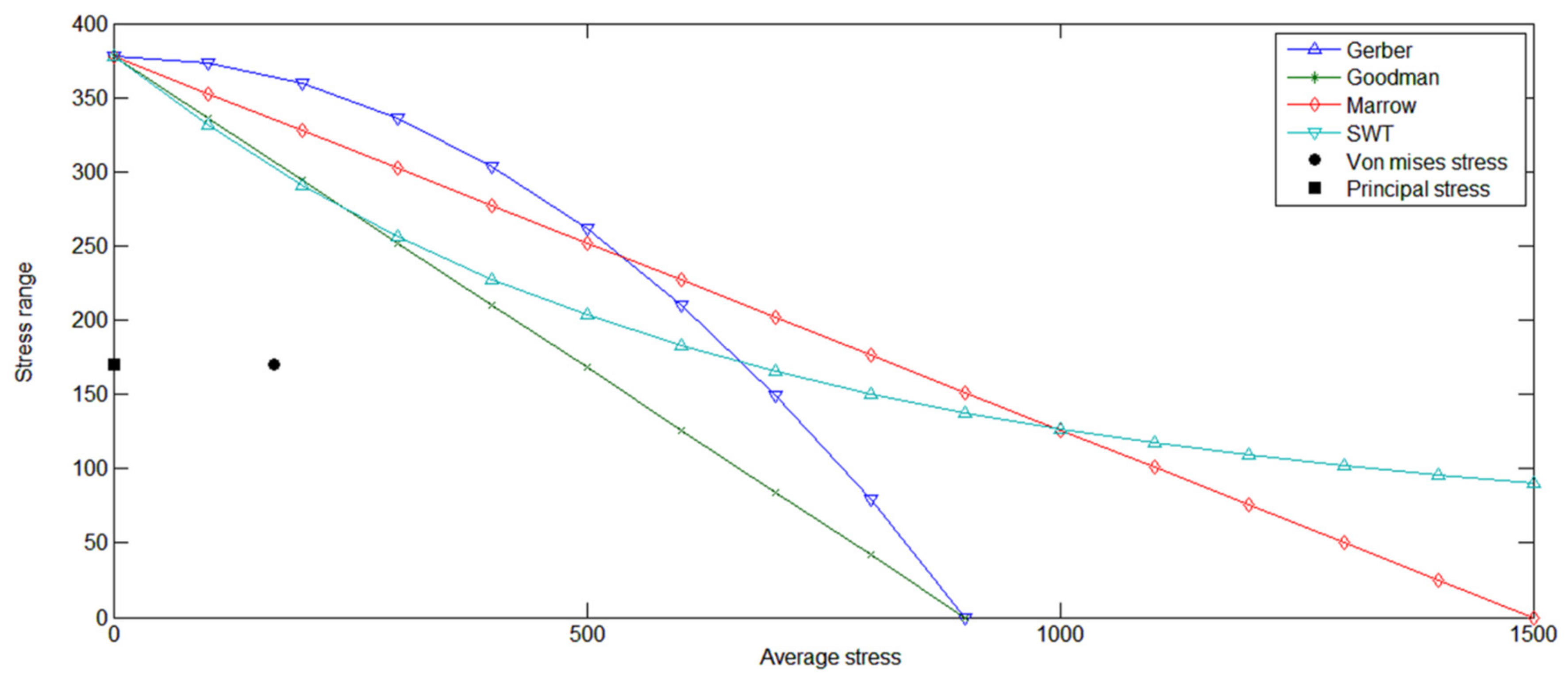
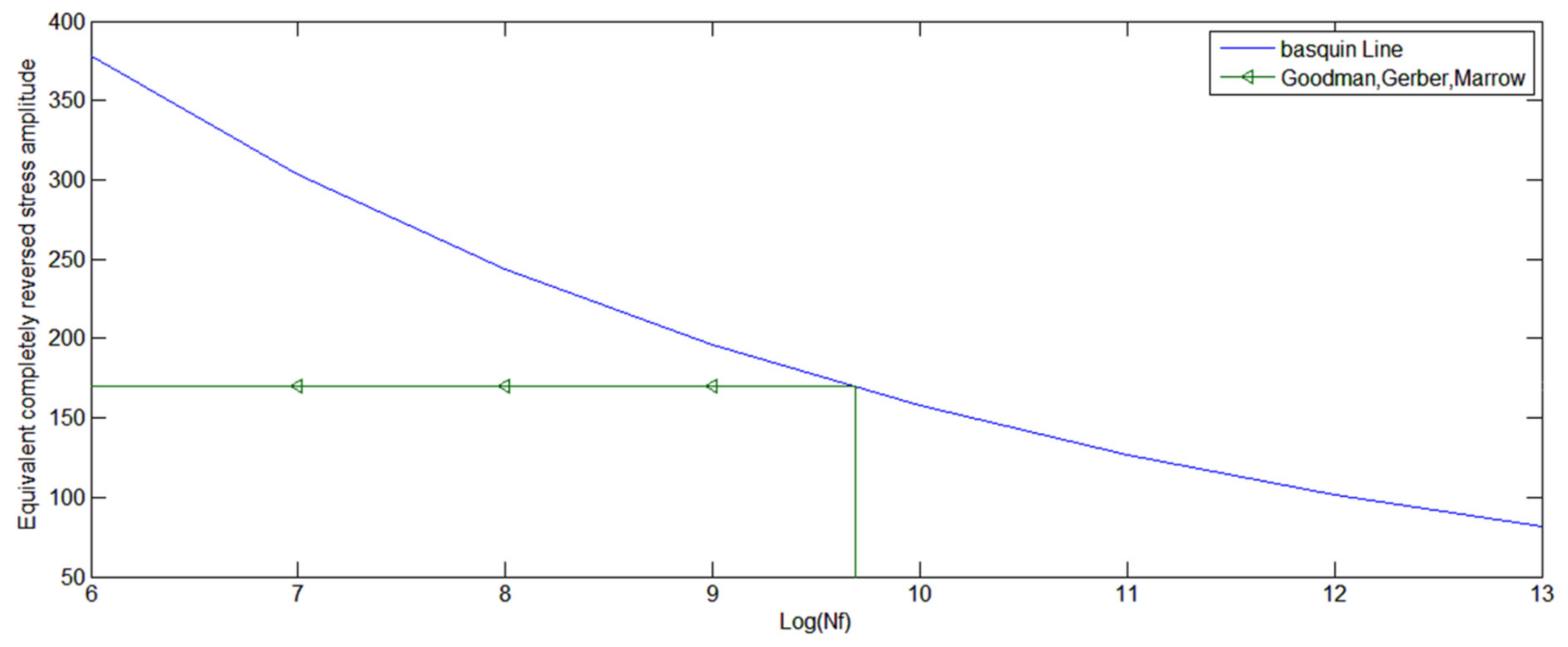
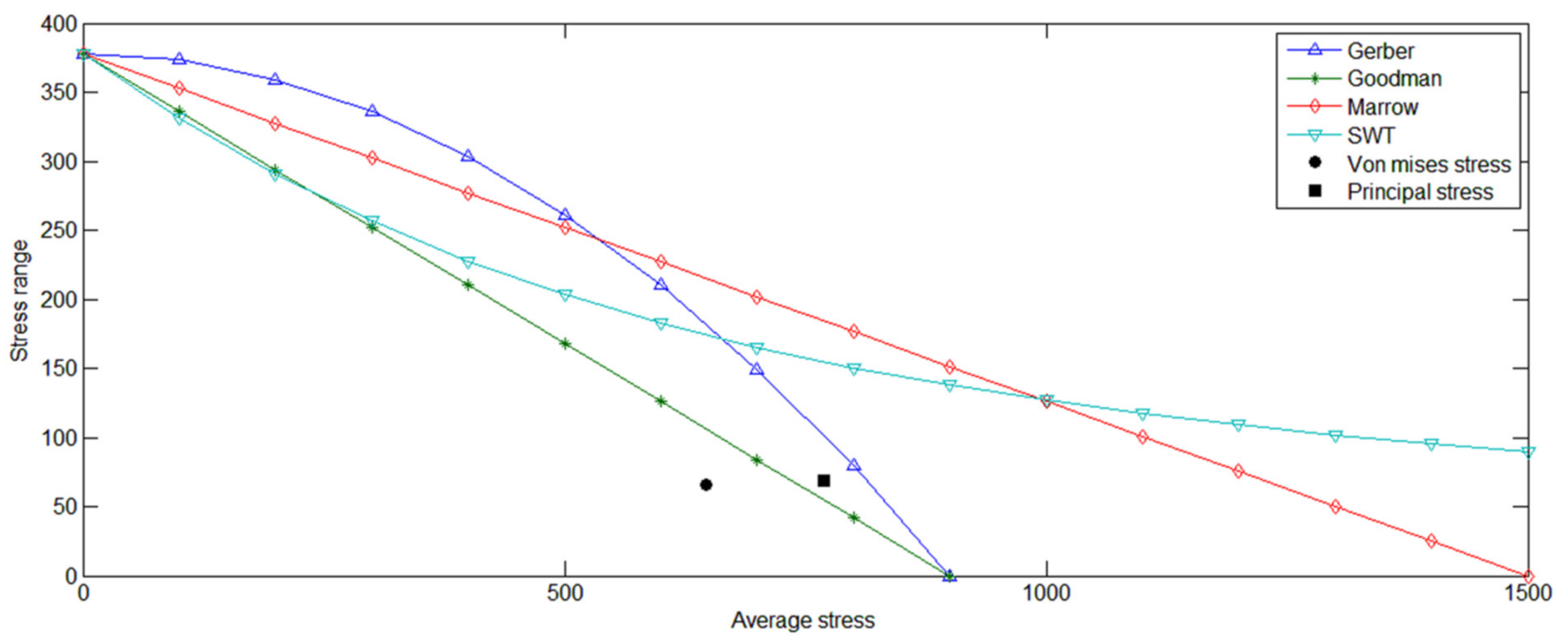
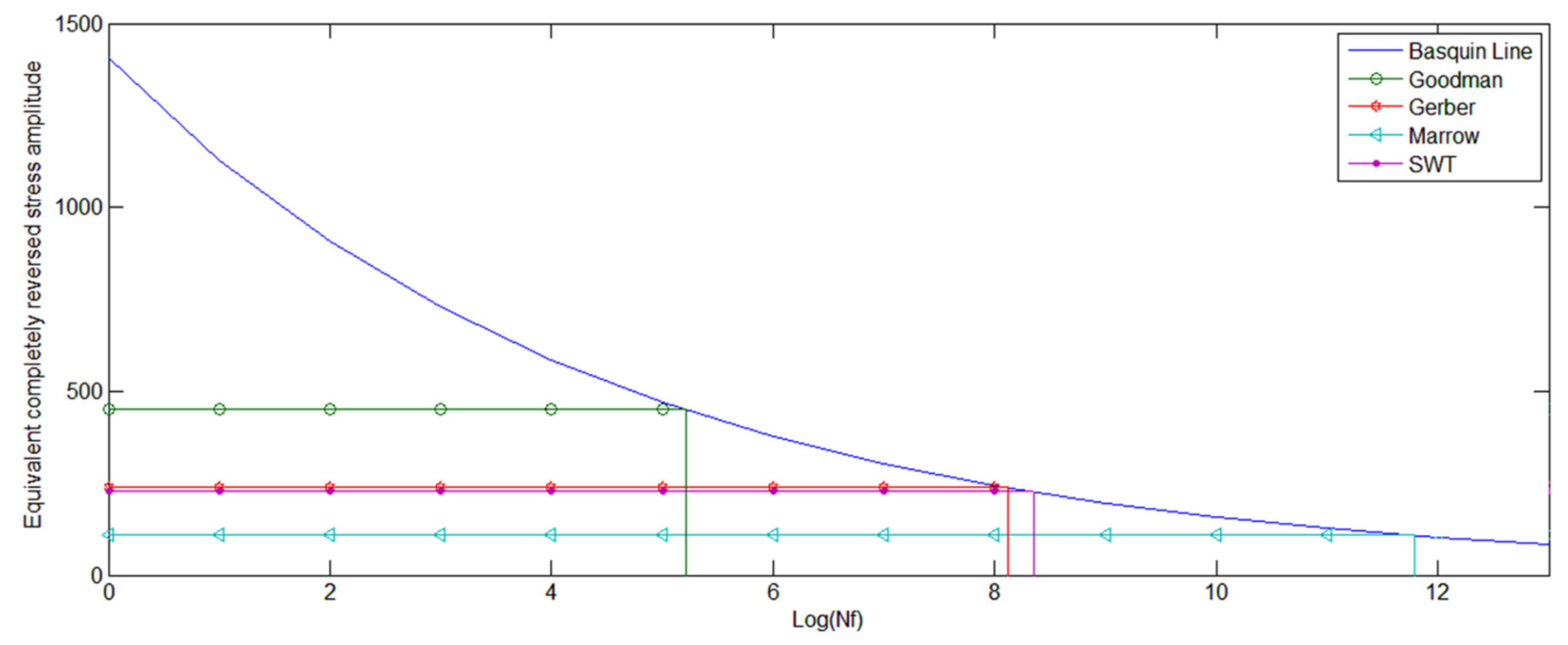
3.2. Fatigue Life Estimation
4. Discussion
5. Conclusions
- Among the entire assembly, the abutment is a critical component which is prone to the fatigue failure due to the tensile stresses resulted from the fastening torque.
- For the bones, the maximum stress is concentrated on the region around the top of implant neck. The maximum stress in the cortical bone is higher than that in the cancellous bone.
- Generally speaking, mechanical stresses developed within an implant are compression, which although contribute to a better fatigue life, they also increase the probability of detachment of upper parts of the implant when mastication forces and corresponding compression stresses are present.
- According to the results of the fatigue life prediction, the abutment screw may fail after about 3 × 105 cycles. Such a failure is likely to occur at the first thread. These results are obtained from the Goodman theory and are at a very good agreement with the clinical data.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Branemark, P.I.; Hansson, B.O.; Adell, R.; Breine, U.; Lindström, J.; Hallén, O.; Ohman, A. Osseointegrated implants in the treatment of the edentulous jaw. Experience from a 10-year period. Scand. J. Plastreconstr. Surg. Suppl. 1997, 16, 1–132. [Google Scholar]
- Akpinar, I.; Demirel, F.; Parnas, L.; Sahin, S. A comparison of stress and strain distribution characteristics of two different rigid implant designs for distal-extension fixed prostheses. Quintessence Int. 1996, 27, 11–17. [Google Scholar] [PubMed]
- Holmgren, E.P.; Seckinger, R.J.; Kilgren, L.M.; Mante, F. Evaluating parameters of osseointegrated dental implants using finite element analysis—A two dimensional comparative study examining the effects of implant diameter, implant shape, and load direction. J. Oral Implantol. 1998, 24, 80–88. [Google Scholar] [CrossRef]
- Nagasao, T.; Kobayashi, M.; Tsuchiya, Y.; Kaneko, T.; Nakajima, T. Finite element analysis of the stresses around fixtures in various reconstructed mandibular models. J. Craniomaxillofac. Surg. 2003, 31, 168–175. [Google Scholar] [CrossRef]
- Van Staden, R.C.; Guan, H.; Loo, Y.-C. Application of the finite element method in dental implant research. Comput. Methods Biomech. Biomed. Eng. 2006, 9, 257–270. [Google Scholar] [CrossRef] [Green Version]
- Edwards Rezende, C.E.; Chase-Diaz, M.; Costa, M.D.; Albarracin, M.L.; Paschoeto, G.; Capello Sousa, E.A.; Rubo, J.H.; Sanches Borges, A.F. Stress distribution in single dental implant system: Three-Dimensional finite element analysis based on an in vitro experimental model. J. Craniofac. Surg. 2015, 26, 2196–2200. [Google Scholar] [CrossRef] [PubMed]
- Kanneganti, K.C.; Vinnakota, D.N.; Pottem, S.R.; Pulagam, M. Comparative effect of implant abutment connections, abutment angulations, and screw lengths on preloaded abutment screw using three dimensional finite element analysis: An in vitro stud. J. Indian Prosthodont. Soc. 2018, 18, 161–167. [Google Scholar] [PubMed]
- Oh, J.-H.; Kim, Y.-S.; Lim, J.Y.; Choi, B.-H. Stress Distribution on the Prosthetic Screws in the All-on-4 Concept: A Three-Dimensional Finite Element Analysis. J. Oral Implant. 2020, 46, 3–12. [Google Scholar] [CrossRef]
- Chikr, Y.C.; Merdji, A.; Gouasmi, S. Effects of the geometric parameters on the interfacial stresses bone/implant. Nat. Technol. 2020, 22, 20–29. [Google Scholar]
- Himmlová, L.; Dostálová, T.; Kácovský, A.; Konvicčková, S. Influence of implant length and diameter on stress distribution: A finite element analysis. J. Prosthet. Dent. 2004, 91, 20–25. [Google Scholar] [CrossRef]
- Paracchini, L.; Barbieri, C.; Redaelli, M.; Di Croce, D.; Vincenzi, C.; Guarnieri, R. Finite Element Analysis of a New Dental Implant Design Optimized for the Desirable Stress Distribution in the Surrounding Bone Region. Prosthesis 2020, 2, 19. [Google Scholar] [CrossRef]
- Burgoa-la-Forcada, S.M.; Edwards Rezende, C.; Castiglia Gonzaga, C.; Cesar Zielak, J.; Yoshio Furuse, A. Photoelastic analysis of dynamic stress distribution around short implants restored with different materials. J. Osseointegr. 2018, 10, 44–49. [Google Scholar]
- Arinc, H. Effects of Prosthetic Material and Framework Design on Stress Distribution. Med. Sci. Monit. 2018, 24, 4279–4287. [Google Scholar] [CrossRef]
- Kayabaşı, O.; Yuzbasioglu, E.; Erzincanlı, F. Static, dynamic and fatigue behaviors of dental implant using finite element method. Adv. Eng. Softw. 2006, 37, 649–658. [Google Scholar] [CrossRef]
- Kong, L.; Zhao, Y.; Hu, K.; Li, D.; Zhou, H.; Wu, Z.; Liu, B. Selection of the implant thread pitch for optimal biomechanical properties: A three-dimensional finite element analysis. Adv. Eng. Softw. 2009, 40, 474–478. [Google Scholar] [CrossRef]
- Ao, J.; Li, T.; Liu, Y.; Ding, Y.; Wu, G.; Hu, K.; Kong, L. Optimal design of thread height and width on an immediately loaded cylinder implant: A finite element analysis. Comput. Biol. Med. 2009, 40, 681–686. [Google Scholar] [CrossRef]
- Oliveira, H.; Brizuela Velasco, A.; Ríos-Santos, J.V.; Sánchez Lasheras, F.; Lemos, B.F.; Gil, F.J.; Carvalho, A.; Herre-ro-Climent, M. Effect of Different Implant Designs on Strain and Stress Distribution under Non-Axial Loading: A Three-Dimensional Finite Element Analysis. Int. J. Environ. Res. Public Health 2020, 17, 4738. [Google Scholar] [CrossRef] [PubMed]
- Sevimay, M.; Turhan, F.; Kılıçarslan, M.A.; Eskitascioglu, G. Three-dimensional finite element analysis of the effect of different bone quality on stress distribution in an implant-supported crown. J. Prosthet. Dent. 2005, 93, 227–234. [Google Scholar] [CrossRef]
- Nesappan, T.; Ariga, P. Comparison of Stresses around Dental Implants Placed in Normal and Fibula Reconstructed Mandibular Models using Finite Element Analysis. J. Clin. Diagn. Res. 2014, 8, ZC45–ZC50. [Google Scholar] [CrossRef]
- Huang, H.L.; Hsu, J.T.; Fuh, L.J.; Lin, D.J.; Chen, M.Y.C. Biomechanical simulation of various surface rough-nesses and geometric designs on an immediately loaded dental implant. Comput. Biol. Med. 2010, 40, 525–532. [Google Scholar] [CrossRef]
- Merdji, A.; BachirBouiadjra, B.; Achour, T.; Serier, B.; OuldChikh, B.; Feng, Z.O. Stress analysis in dental prosthesis. Comput. Mater. Sci. 2010, 49, 126–133. [Google Scholar] [CrossRef]
- Ilieş, H.T.; Flanagan, D.; McCullough, P.T.; McQuoid, S. Determining the Fatigue Life of Dental Implants. J. Med. Devices 2008, 2, 011003. [Google Scholar] [CrossRef] [Green Version]
- Gibbs, C.H.; Anusavice, K.J.; Young, H.M.; Jones, J.S.; Esquivel Upshaw, J.F. Maximum Clenching Force of Patients with Moderate Loss of Posterior Tooth Support: A Pilot Study. J. Prosthet Dent. 2002, 88, 498–502. [Google Scholar] [CrossRef]
- Wierszycki, M.; Kakail, W.; Lodygowski, T. Fatigue algorithm for dental implant. Found. Civ. Environ. Eng. 2006, 7, 363–380. [Google Scholar]
- Nergiz, I.; Schmage, P.; Shahin, R. Removal of a fractured implant abutment screw: A clinical report. J. Prosthet. Dent. 2004, 91, 513–517. [Google Scholar] [CrossRef]
- Theoharidou, A.; Petridis, H.P.; Tzannas, K.; Garefis, P. Abutment screw loosening in single-implant restorations: A systematic review. Int. J. Oral Maxillofac. Implant. 2008, 23, 681–690. [Google Scholar]
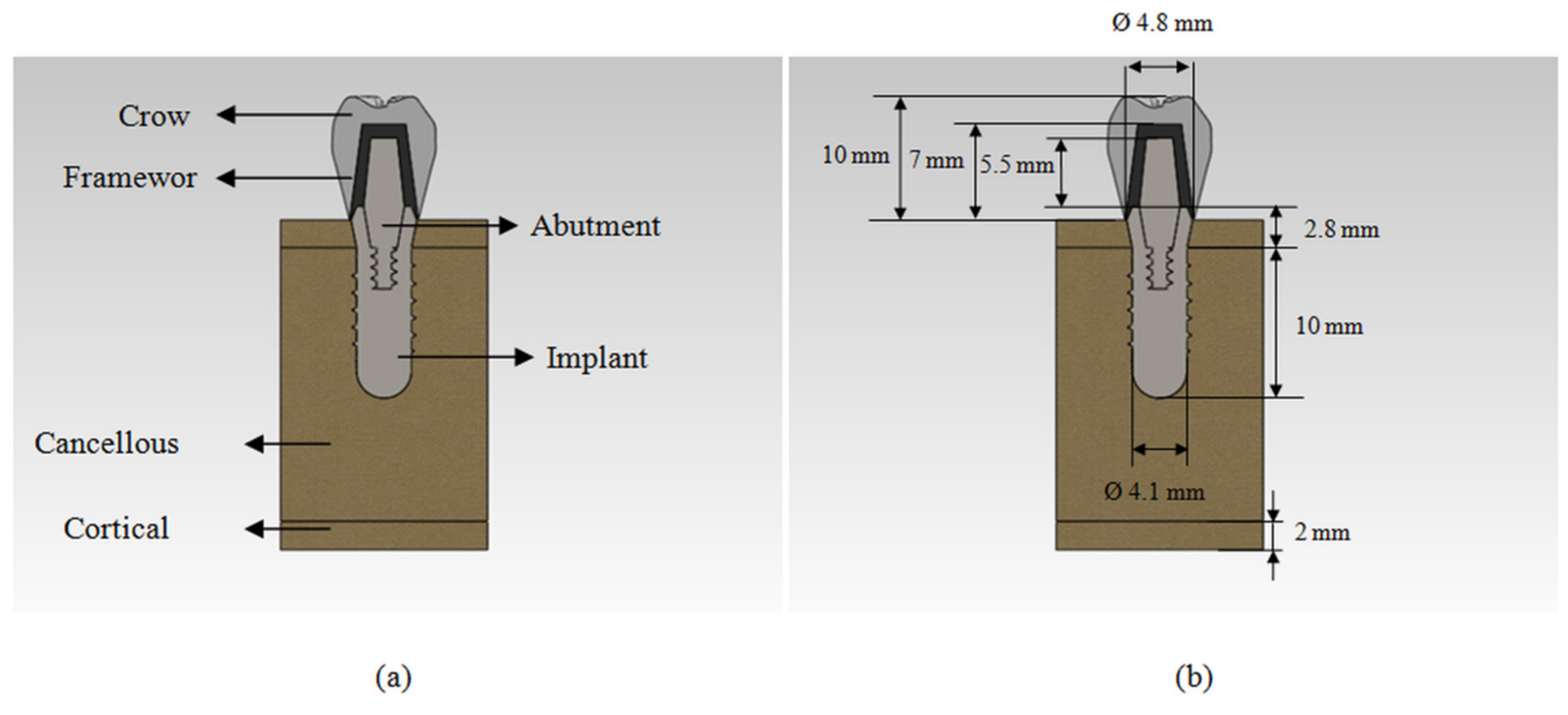
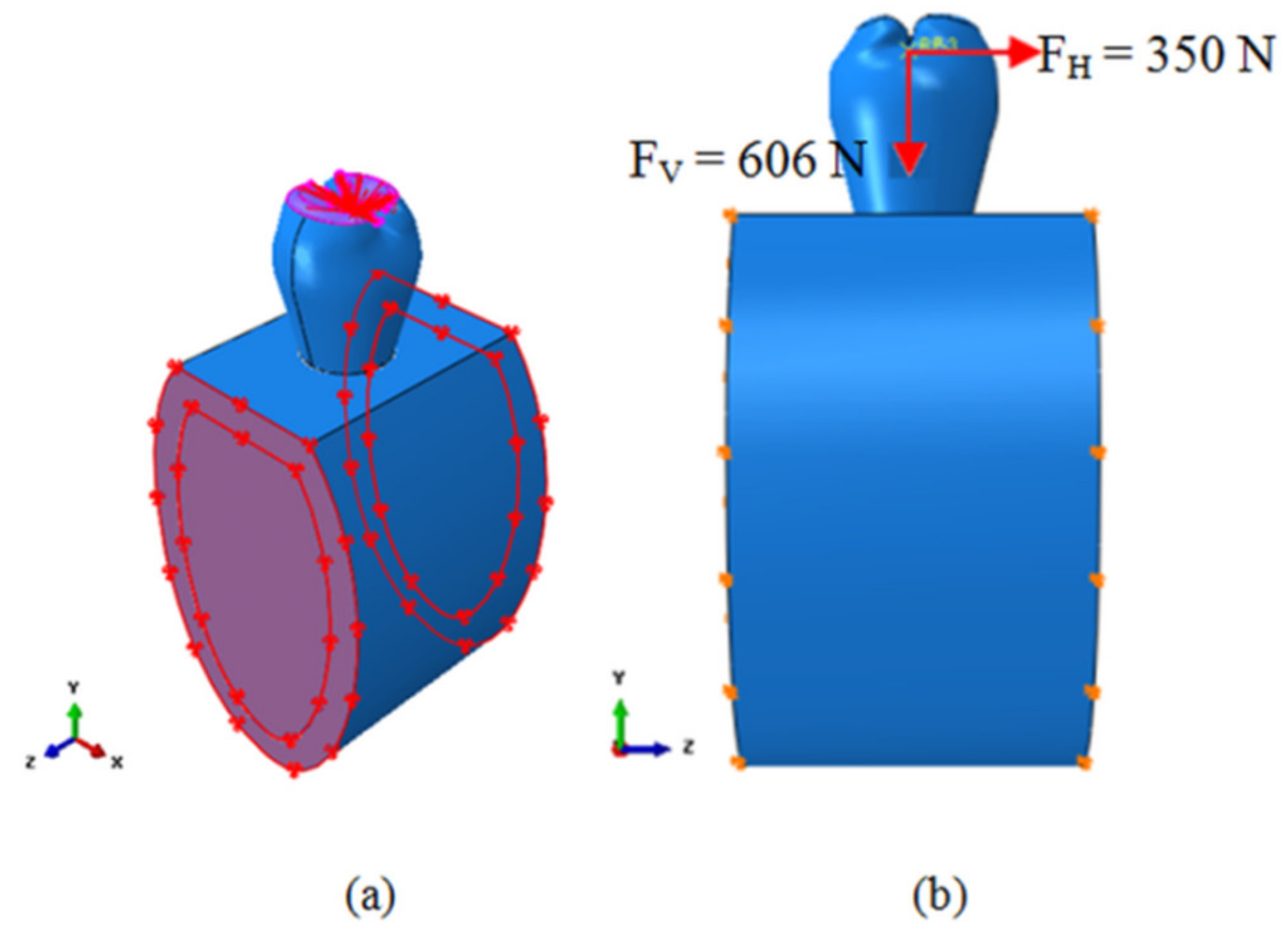





| Component | Material | Density, ρ (kg/m3) | Young’s Modulus, E (GPa) | Poisson Ratio, υ | Yield Strength, Sy (MPa) |
|---|---|---|---|---|---|
| Crown | porcelain | 2450 | 70 | 0.19 | 500 |
| Framework | Co-Cr-Alloy | 8300 | 220 | 0.3 | 770 |
| Implant | Ti-6Al-4V | 4500 | 110 | 0.35 | 800 |
| Abutment | Ti-6Al-4V | 4500 | 110 | 0.35 | 800 |
| Material | Density, ρ (kg/m3) | Young’s Modulus, E (GPa) | Poisson Ratio, υ | Shear Modulus, (MPa) |
|---|---|---|---|---|
| Cortical bone | 1700 | Ex = 12,600 | υxy = 0.3 | Gxy = 4850 |
| υyx = 0.3 | ||||
| Ey = 12,600 | υyz = 0.253 | Gyz = 5700 | ||
| υzy = 0.39 | ||||
| Ez = 19,400 | υxz = 0.253 | Gxz = 5700 | ||
| υzx = 0.39 | ||||
| Cancellous bone | 270 | Ex = 1148 | υxy = 0.055 | Gxy = 68 |
| υyx = 0.01 | ||||
| Ey = 270 | υyz = 0.01 | Gyz = 68 | ||
| υzy =0.055 | ||||
| Ez = 1148 | υxz = 0.322 | Gxz = 434 |
| Component | Max Von Mises Stress (MPa) | Yield Strength, Sy(MPa) |
|---|---|---|
| Crown | 82.29 | 500 |
| Framework | 322 | 770 |
| Abutment | 680 | 800 |
| Implant | 360.8 | 800 |
| Cortical bone | 168.9 | - |
| Cancellous bone | 5.496 | - |
| Ultimate tensile stress, Sut | 900 MPa |
| Fatigue strength coefficient, σf’ | 1500 MPa |
| Fatigue strength exponent, b | −0.095 |
| Endurance limit, S | 380 MPa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ziaie, B.; Khalili, S.M.R. Evaluation of Fatigue Life for Dental Implants Using FEM Analysis. Prosthesis 2021, 3, 300-313. https://doi.org/10.3390/prosthesis3040028
Ziaie B, Khalili SMR. Evaluation of Fatigue Life for Dental Implants Using FEM Analysis. Prosthesis. 2021; 3(4):300-313. https://doi.org/10.3390/prosthesis3040028
Chicago/Turabian StyleZiaie, Babak, and S. Mohammad Reza Khalili. 2021. "Evaluation of Fatigue Life for Dental Implants Using FEM Analysis" Prosthesis 3, no. 4: 300-313. https://doi.org/10.3390/prosthesis3040028
APA StyleZiaie, B., & Khalili, S. M. R. (2021). Evaluation of Fatigue Life for Dental Implants Using FEM Analysis. Prosthesis, 3(4), 300-313. https://doi.org/10.3390/prosthesis3040028







