Pre-Operative Modeling of Transcatheter Mitral Valve Replacement in a Surgical Heart Valve Bioprosthesis
Abstract
:1. Introduction
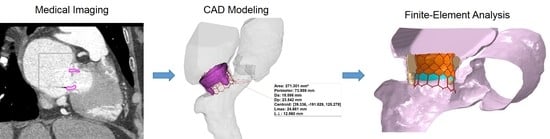
2. Material and Methods
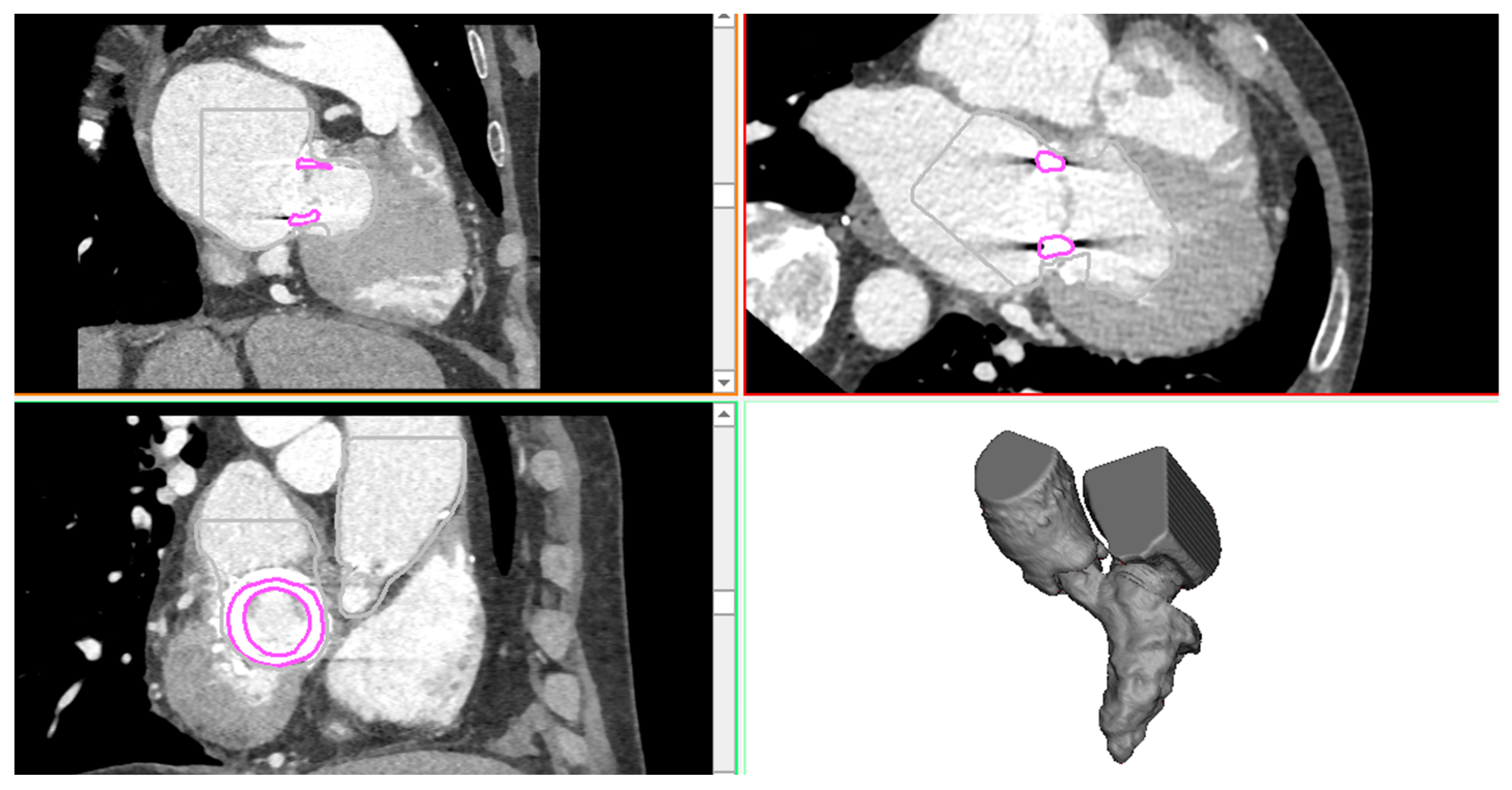
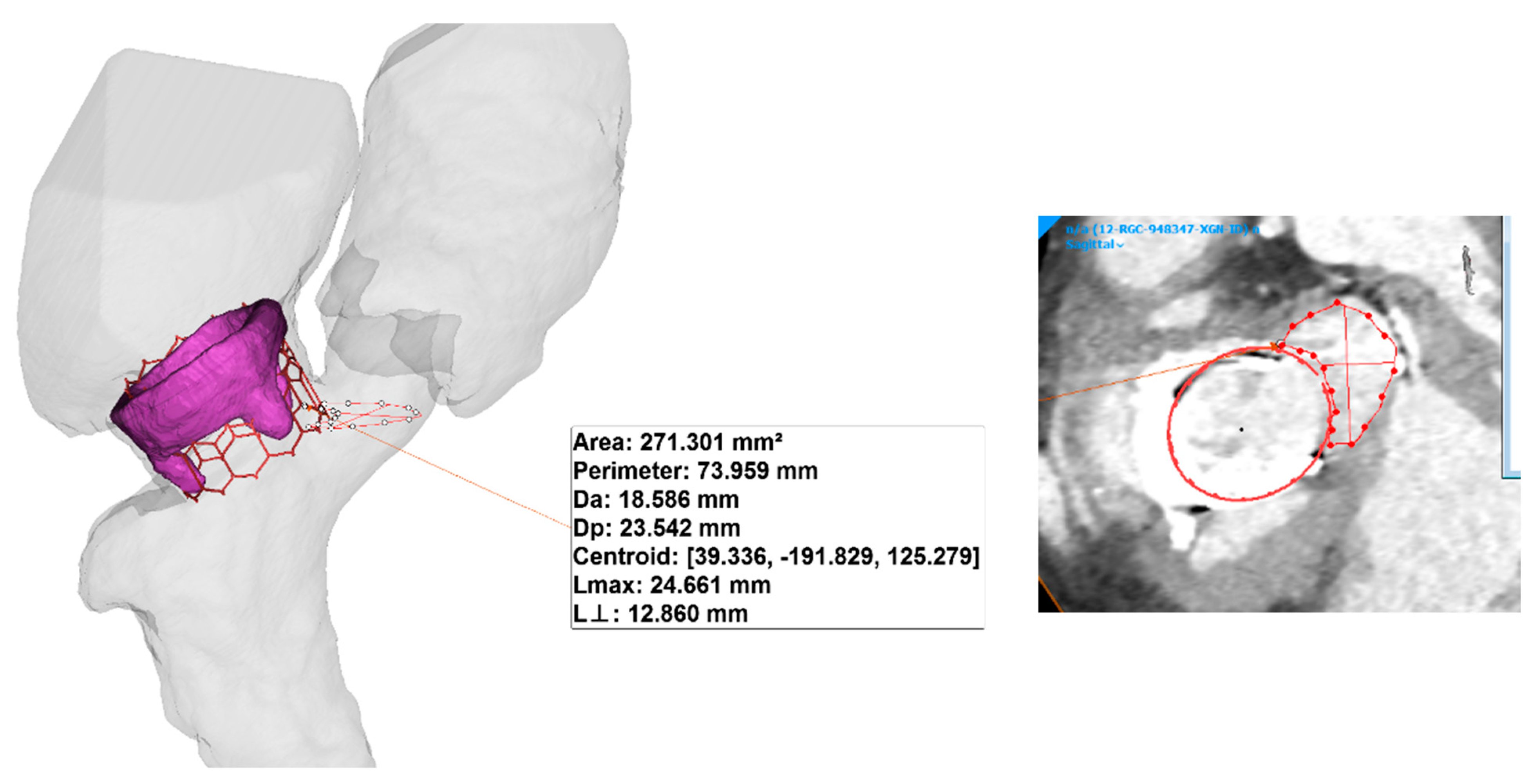
2.1. Anatomic Reconstruction
2.2. Computational Modeling
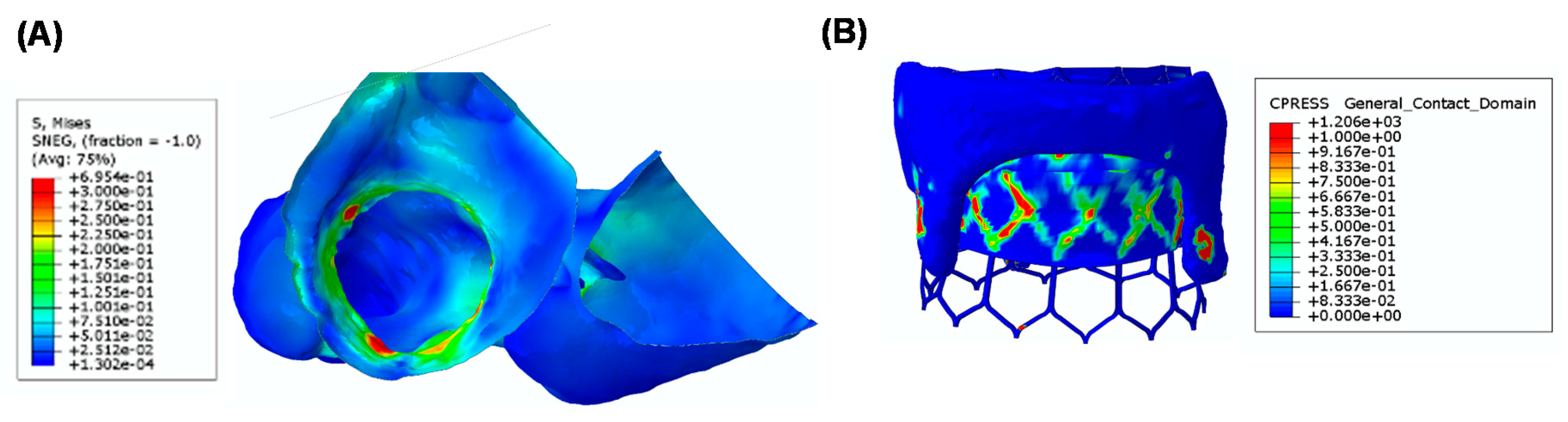
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yoon, S.H.; Bleiziffer, S.; Latib, A.; Eschenbach, L.; Ancona, M.; Vincent, F.; Kim, W.K.; Unbehaum, A.; Asami, M.; Dhoble, A.; et al. Predictors of Left Ventricular Outflow Tract Obstruction After Transcatheter Mitral Valve Replacement. JACC Cardiovasc. Interv. 2019, 12, 182–193. [Google Scholar] [CrossRef] [PubMed]
- Alsidawi, S.; Eleid, M.F.; Rihal, C.S.; Nkomo, V.T.; Pislaru, S. Significant LVOT obstruction after mitral valve in ring procedure. Eur. Heart J. Cardiovasc. Imaging 2015, 16, 1389. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yoon, S.H.; Whisenant, B.K.; Bleiziffer, S.; Delgado, V.; Schofer, N.; Eschenbach, L.; Fujita, B.; Sharma, R.; Ancona, M.; Yzeiraj, E.; et al. Transcatheter Mitral Valve Replacement for Degenerated Bioprosthetic Valves and Failed Annuloplasty Rings. J. Am. Coll. Cardiol. 2017, 70, 1121–1131. [Google Scholar] [CrossRef] [PubMed]
- Leipsic, J.; Blanke, P. Predicting Left Ventricular Outflow Tract Obstruction After Transcatheter Mitral Valve Replacement: From Theory to Evidence. JACC Cardiovasc. Interv. 2019, 12, 194–195. [Google Scholar] [CrossRef]
- Wang, D.D.; Eng, M.; Greenbaum, A.; Myers, E.; Forbes, M.; Pantelic, M.; Song, T.; Nelson, C.; Divine, G.; Taylor, A.; et al. Predicting LVOT Obstruction After TMVR. JACC Cardiovasc. Imaging 2016, 9, 1349–1352. [Google Scholar] [CrossRef]
- Blanke, P.; Naoum, C.; Dvir, D.; Bapat, V.; Ong, K.; Muller, D.; Cheung, A.; Ye, J.; Min, J.K.; Piazza, N.; et al. Predicting LVOT Obstruction in Transcatheter Mitral Valve Implantation: Concept of the Neo-LVOT. JACC Cardiovasc. Imaging 2017, 10, 482–485. [Google Scholar] [CrossRef]
- Chandran, K.B. Role of Computational Simulations in Heart Valve Dynamics and Design of Valvular Prostheses. Cardiovasc. Eng. Technol. 2010, 1, 18–38. [Google Scholar] [CrossRef] [Green Version]
- Aggarwal, A.; Aguilar, V.S.; Lee, C.H.; Ferrari, G.; Gorman, J.H.; Gorman, R.C.; Sacks, M.S. Patient-Specific Modeling of Heart Valves: From Image to Simulation. Funct. Imaging Model. Heart Int. Workshop FIMH Proc. 2013, 7945, 141–149. [Google Scholar] [CrossRef]
- Sacks, M.S.; Yoganathan, A.P. Heart valve function: A biomechanical perspective. Philos. Trans. R. Soc. Lond. Ser. B Biol. Sci. 2007, 362, 1369–1391. [Google Scholar] [CrossRef] [Green Version]
- Rabbah, J.P.; Saikrishnan, N.; Siefert, A.W.; Santhanakrishnan, A.; Yoganathan, A.P. Mechanics of healthy and functionally diseased mitral valves: A critical review. J. Biomech. Eng. 2013, 135, 021007. [Google Scholar] [CrossRef]
- Sacks, M.; Drach, A.; Lee, C.H.; Khalighi, A.; Rego, B.; Zhang, W.; Ayoub, S.; Yoganathan, A.; Gorman, R.C.; Gorman Iii, J.H. On the simulation of mitral valve function in health, disease, and treatment. J. Biomech. Eng. 2019. [Google Scholar] [CrossRef] [PubMed]
- Zakerzadeh, R.; Hsu, M.C.; Sacks, M.S. Computational methods for the aortic heart valve and its replacements. Expert Rev. Med. Devices 2017, 14, 849–866. [Google Scholar] [CrossRef] [PubMed]
- Khalighi, A.H.; Rego, B.V.; Drach, A.; Gorman, R.C.; Gorman, J.H., 3rd; Sacks, M.S. Development of a Functionally Equivalent Model of the Mitral Valve Chordae Tendineae Through Topology Optimization. Ann. Biomed. Eng. 2019, 47, 60–74. [Google Scholar] [CrossRef] [PubMed]
- D’Ancona, G.; Amaducci, A.; Rinaudo, A.; Pasta, S.; Follis, F.; Pilato, M.; Baglini, R. Hemodynamic Predictors of a Penetrating Atherosclerotic Ulcer Rupture using Fluid-Structure Interaction Analysis. ICVTS 2013, 17, 576–578. [Google Scholar]
- Pasta, S.; Cannata, S.; Gentile, G.; Di Giuseppe, M.; Cosentino, F.; Pasta, F.; Agnese, V.; Bellavia, D.; Raffa, G.M.; Pilato, M.; et al. Simulation study of transcatheter heart valve implantation in patients with stenotic bicuspid aortic valve. Med. Biol. Eng. Comput. 2020. [Google Scholar] [CrossRef]
- Pasta, S.; Gentile, G.; Raffa, G.M.; Bellavia, D.; Chiarello, G.; Liotta, R.; Luca, A.; Scardulla, C.; Pilato, M. In Silico Shear and Intramural Stresses are Linked to Aortic Valve Morphology in Dilated Ascending Aorta. Eur. J. Vasc. Endovasc. Surg. 2017, in press. [Google Scholar] [CrossRef]
- Rinaudo, A.; D’Ancona, G.; Lee, J.J.; Pilato, G.; Amaducci, A.; Baglini, R.; Folli, C.; Pilato, M.; Pasta, S. Predicting Outcome of Aortic Dissection with Patent False Lumen by Computational Flow Analysis. Cardiovasc. Eng. Technol. 2014, 5, 176–188. [Google Scholar] [CrossRef]
- Pasta, S.; Agnese, V.; Di Giuseppe, M.; Gentile, G.; Raffa, G.M.; Bellavia, D.; Pilato, M. In Vivo Strain Analysis of Dilated Ascending Thoracic Aorta by ECG-Gated CT Angiographic Imaging. Ann. Biomed. Eng. 2017. [Google Scholar] [CrossRef]
- Shen, X.; Wang, T.; Cao, X.; Cai, L. The geometric model of the human mitral valve. PLoS ONE 2017, 12, e0183362. [Google Scholar] [CrossRef]
- Morganti, S.; Conti, M.; Aiello, M.; Valentini, A.; Mazzola, A.; Reali, A.; Auricchio, F. Simulation of transcatheter aortic valve implantation through patient-specific finite element analysis: Two clinical cases. J. Biomech. 2014, 47, 2547–2555. [Google Scholar] [CrossRef]
- Kleinstreuer, C.; Li, Z.; Basciano, C.A.; Seelecke, S.; Farber, M.A. Computational mechanics of Nitinol stent grafts. J. Biomech. 2008, 41, 2370–2378. [Google Scholar] [CrossRef] [PubMed]
- Rinaudo, A.; Raffa, G.M.; Scardulla, F.; Pilato, M.; Scardulla, C.; Pasta, S. Biomechanical implications of excessive endograft protrusion into the aortic arch after thoracic endovascular repair. Comput. Biol. Med. 2015, 66, 235–241. [Google Scholar] [CrossRef] [PubMed]
- Morlacchi, S.; Chiastra, C.; Gastaldi, D.; Pennati, G.; Dubini, G.; Migliavacca, F. Sequential structural and fluid dynamic numerical simulations of a stented bifurcated coronary artery. J. Biomech. Eng. 2011, 133, 121010. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.J.; D’Ancona, G.; Amaducci, A.; Follis, F.; Pilato, M.; Pasta, S. Role of computational modeling in thoracic aortic pathology: A review. J. Card. Surg. 2014, 29, 653–662. [Google Scholar] [CrossRef]
- Lavon, K.; Marom, G.; Bianchi, M.; Halevi, R.; Hamdan, A.; Morany, A.; Raanani, E.; Bluestein, D.; Haj-Ali, R. Biomechanical modeling of transcatheter aortic valve replacement in a stenotic bicuspid aortic valve: Deployments and paravalvular leakage. Med. Biol. Eng. Comput. 2019, 57, 2129–2143. [Google Scholar] [CrossRef]
- Luraghi, G.; Migliavacca, F.; Garcia-Gonzalez, A.; Chiastra, C.; Rossi, A.; Cao, D.; Stefanini, G.; Rodriguez Matas, J.F. On the Modeling of Patient-Specific Transcatheter Aortic Valve Replacement: A Fluid-Structure Interaction Approach. Cardiovasc. Eng. Technol. 2019, 10, 437–455. [Google Scholar] [CrossRef]
- Bianchi, M.; Marom, G.; Ghosh, R.P.; Rotman, O.M.; Parikh, P.; Gruberg, L.; Bluestein, D. Patient-specific simulation of transcatheter aortic valve replacement: Impact of deployment options on paravalvular leakage. Biomech. Model. Mechanobiol. 2019, 18, 435–451. [Google Scholar] [CrossRef]
- Auricchio, F.; Conti, M.; Morganti, S.; Reali, A. Simulation of transcatheter aortic valve implantation: A patient-specific finite element approach. Comput. Methods Biomech. Biomed. Eng. 2014, 17, 1347–1357. [Google Scholar] [CrossRef]
- Finotello, A.; Morganti, S.; Auricchio, F. Finite element analysis of TAVI: Impact of native aortic root computational modeling strategies on simulation outcomes. Med. Eng. Phys. 2017, 47, 2–12. [Google Scholar] [CrossRef]


| E(MPa) | ν | σ y (MPa) | σult (MPa) | εp | μ (Pa s) | D (kg/m3) | Element Number (thousand) | |
|---|---|---|---|---|---|---|---|---|
| Heart | 4 | 0.49 | 1,060 | 65.6–68.5 | ||||
| S3 Ultra | 233 x103 | 0.35 | 414 | 930 | 0.45 | 8,000 | 59.2 | |
| Sealing Skirt | 55 | 0.49 | 6.6 | 6.6 | 0.6 | 8,000 | 3.5–3.7 | |
| Bioprosthesis | 8 | 0.45 | 1,060 | 10.5–12.5 | ||||
| Balloon | 600 | 0.3 | 1,060 | 62.8 | ||||
| Fluid | 3.7 × 10−3 | 1,060 | 301.1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pasta, S.; Gandolfo, C. Pre-Operative Modeling of Transcatheter Mitral Valve Replacement in a Surgical Heart Valve Bioprosthesis. Prosthesis 2020, 2, 39-45. https://doi.org/10.3390/prosthesis2010004
Pasta S, Gandolfo C. Pre-Operative Modeling of Transcatheter Mitral Valve Replacement in a Surgical Heart Valve Bioprosthesis. Prosthesis. 2020; 2(1):39-45. https://doi.org/10.3390/prosthesis2010004
Chicago/Turabian StylePasta, Salvatore, and Caterina Gandolfo. 2020. "Pre-Operative Modeling of Transcatheter Mitral Valve Replacement in a Surgical Heart Valve Bioprosthesis" Prosthesis 2, no. 1: 39-45. https://doi.org/10.3390/prosthesis2010004
APA StylePasta, S., & Gandolfo, C. (2020). Pre-Operative Modeling of Transcatheter Mitral Valve Replacement in a Surgical Heart Valve Bioprosthesis. Prosthesis, 2(1), 39-45. https://doi.org/10.3390/prosthesis2010004