Improved Mathematical Approach for Modeling Sport Differential Mechanism
Abstract
1. Introduction
2. Background on Sport DM Technology
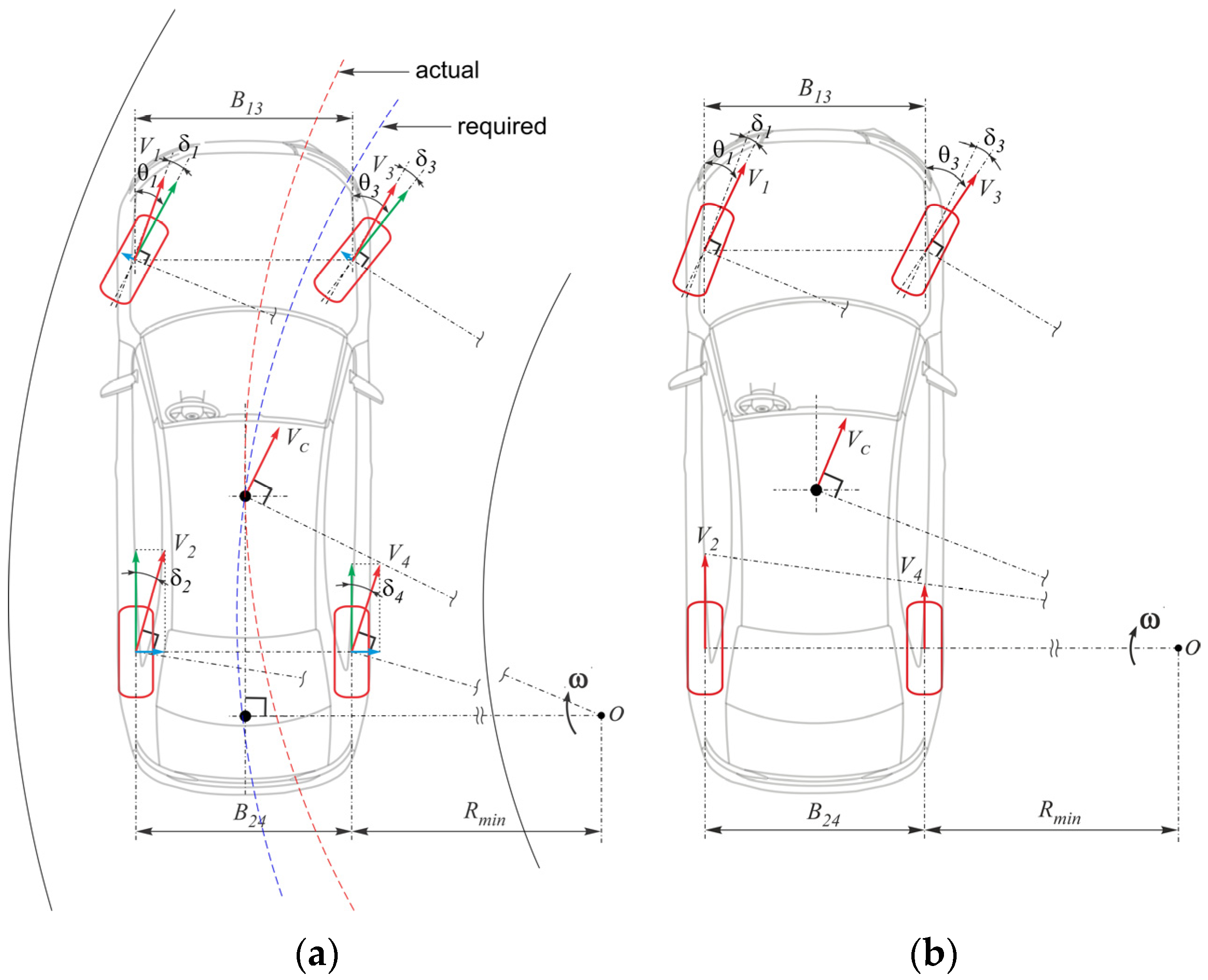
2.1. Steerability Issues
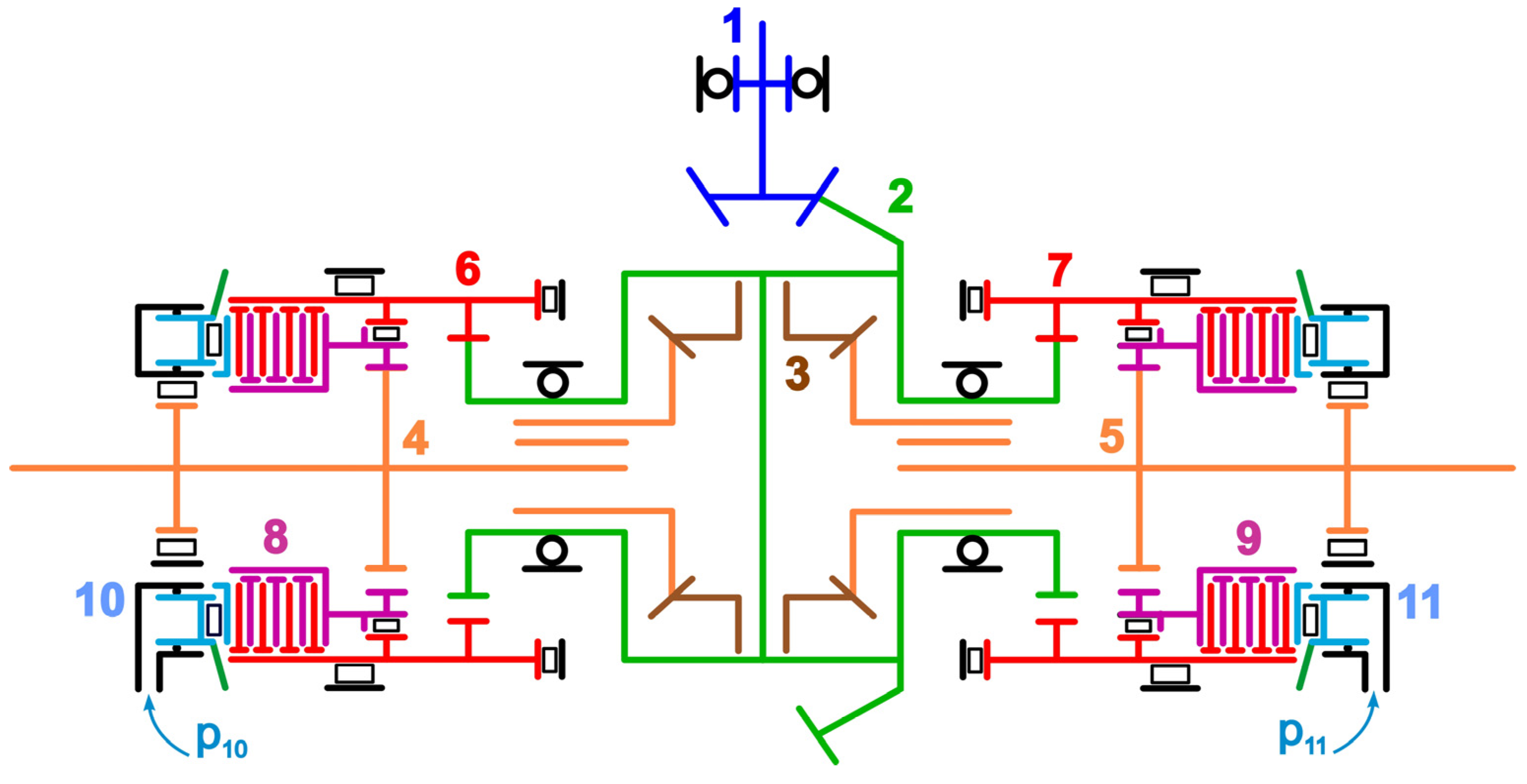
2.2. Design of Audi DM
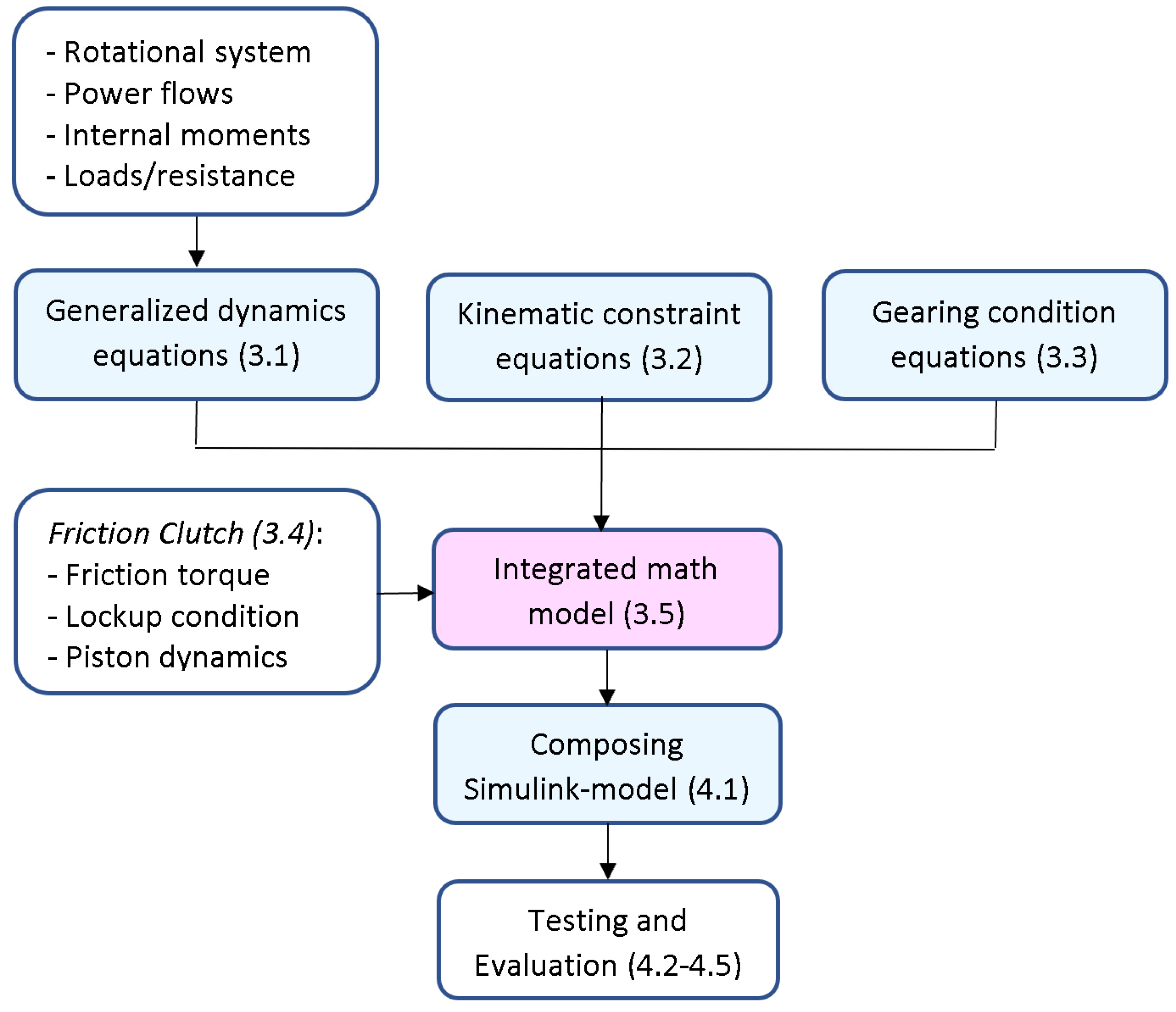
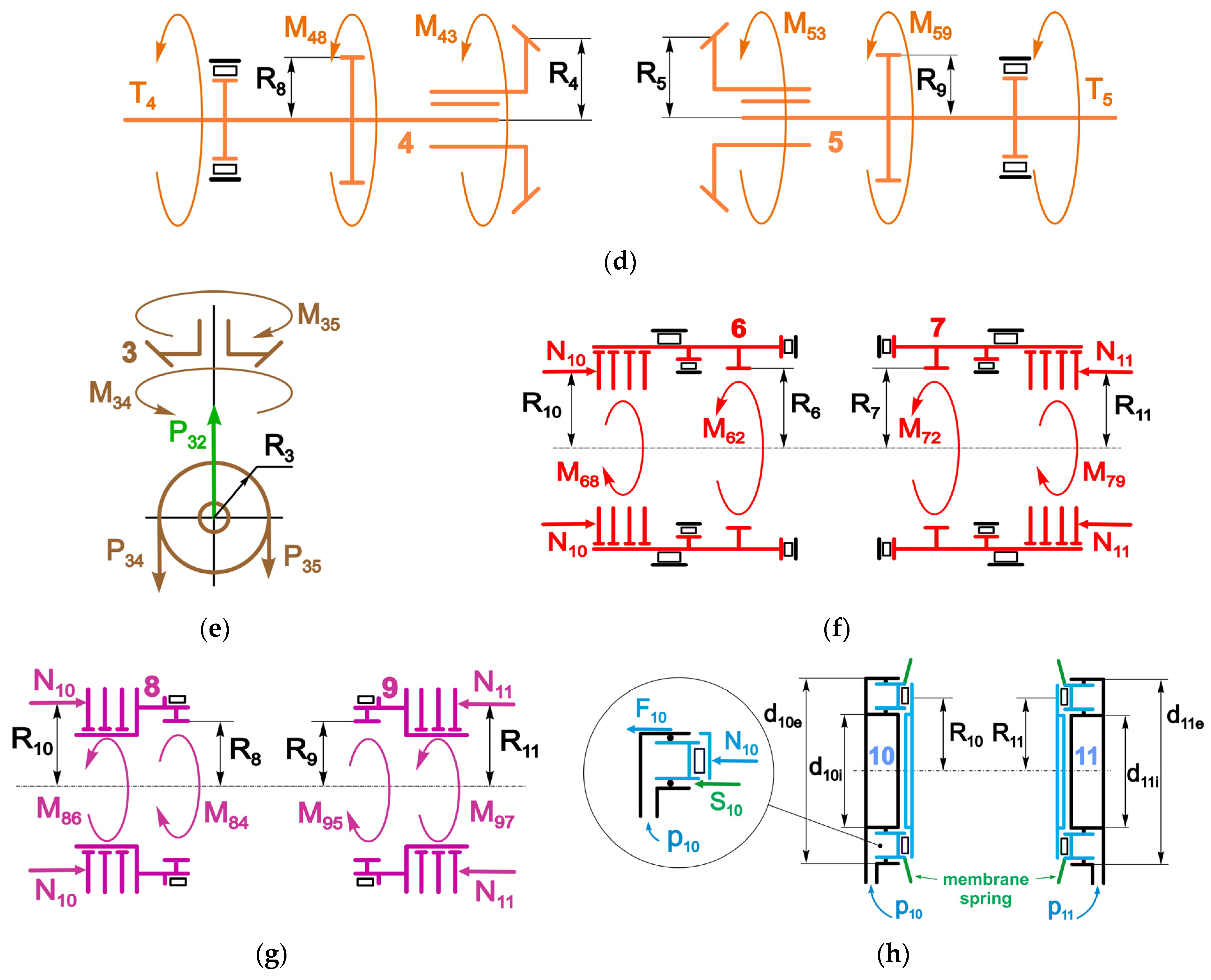
3. Integrated Mathematical Model
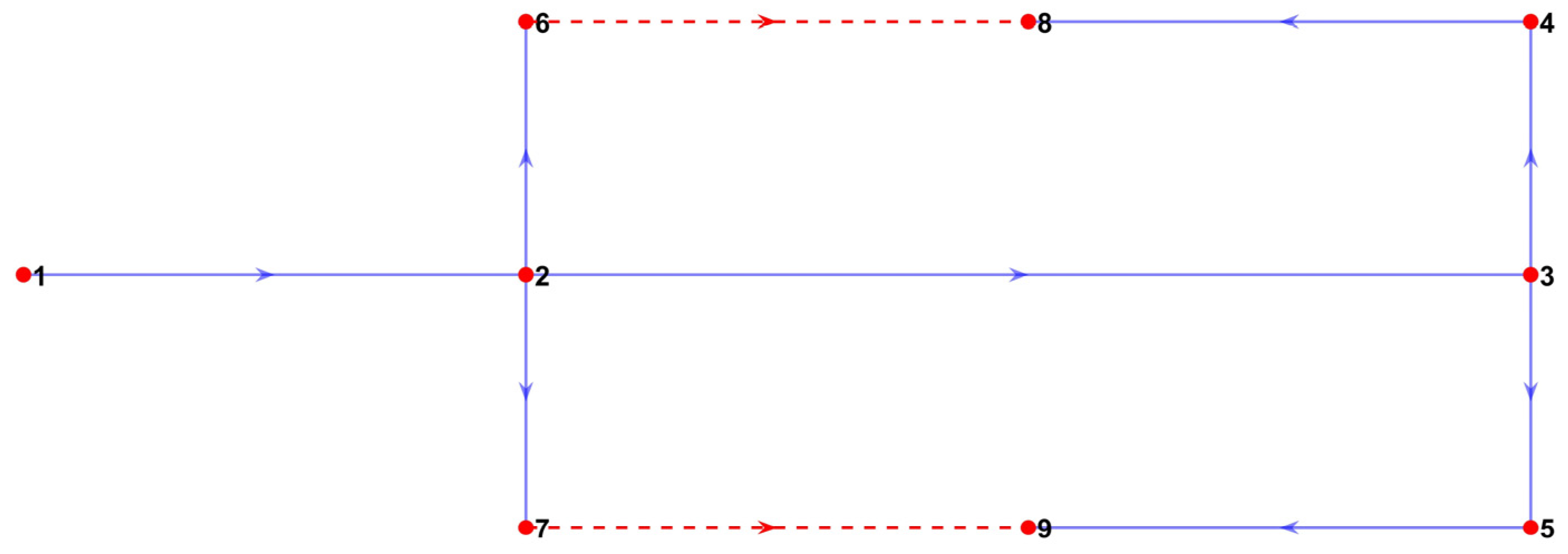
3.1. Generalization of Dynamics Equations
3.1.1. Rotational System
3.1.2. Power Flows and Efficiencies
3.1.3. Internal Moments
3.1.4. Loads and Resistance
3.2. Kinematic Constraints
3.3. Gearing Conditions
3.4. Friction Clutch
3.4.1. Friction Torque
3.4.2. Lockup Condition
3.4.3. Piston Dynamics
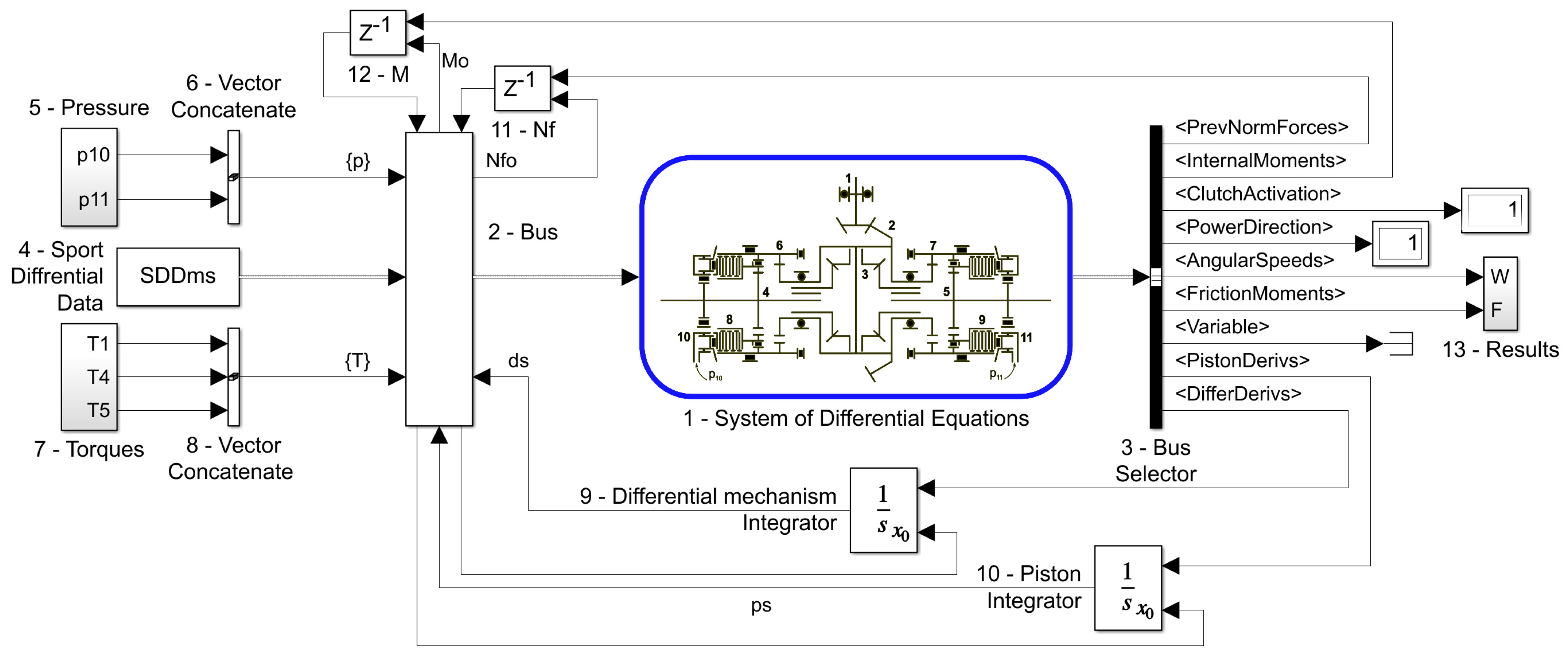
3.5. Matrix Form
4. Simulation
4.1. Simulink Model
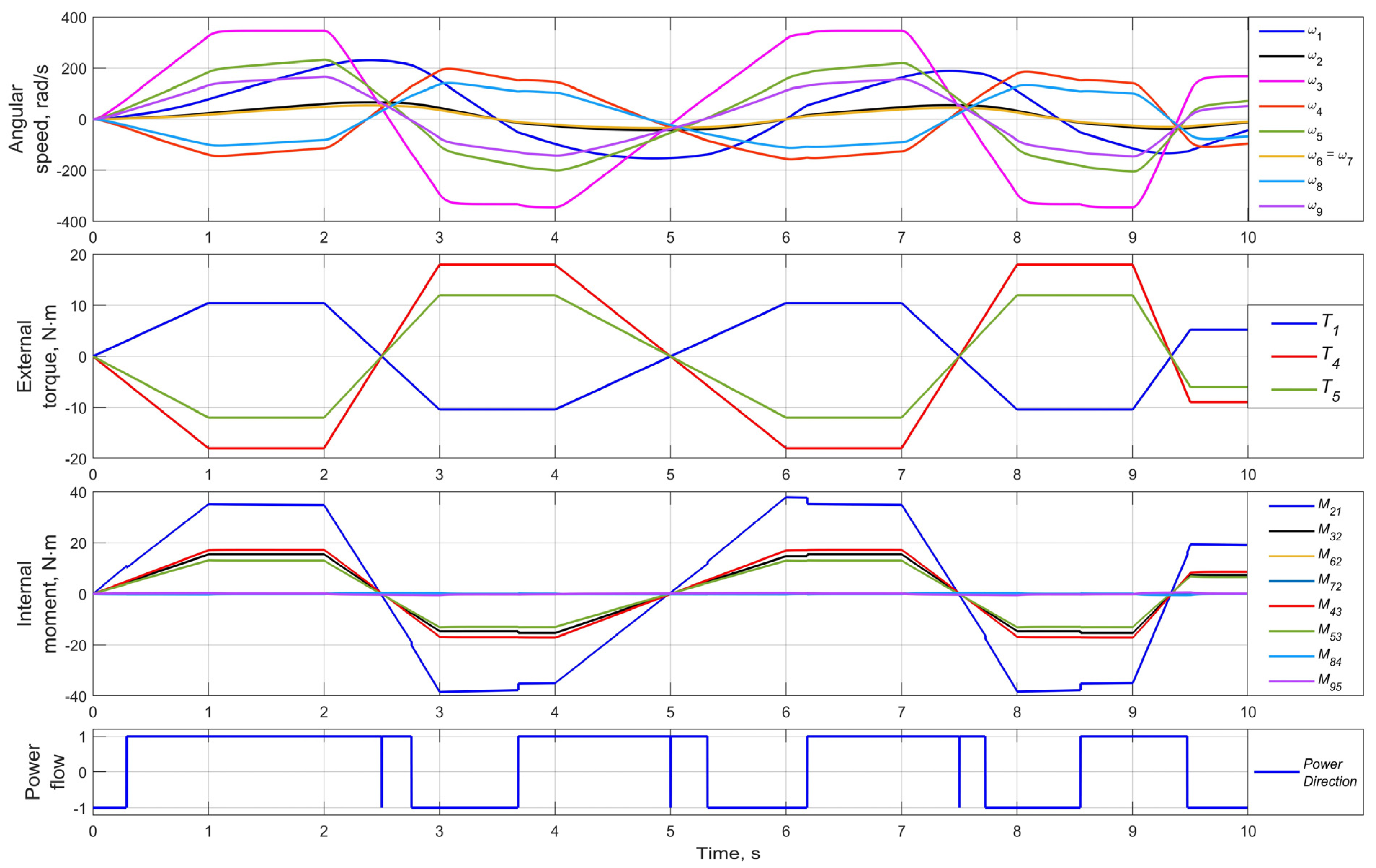
4.2. Testing Differential Model Operability
4.3. Zero-Resistance Case
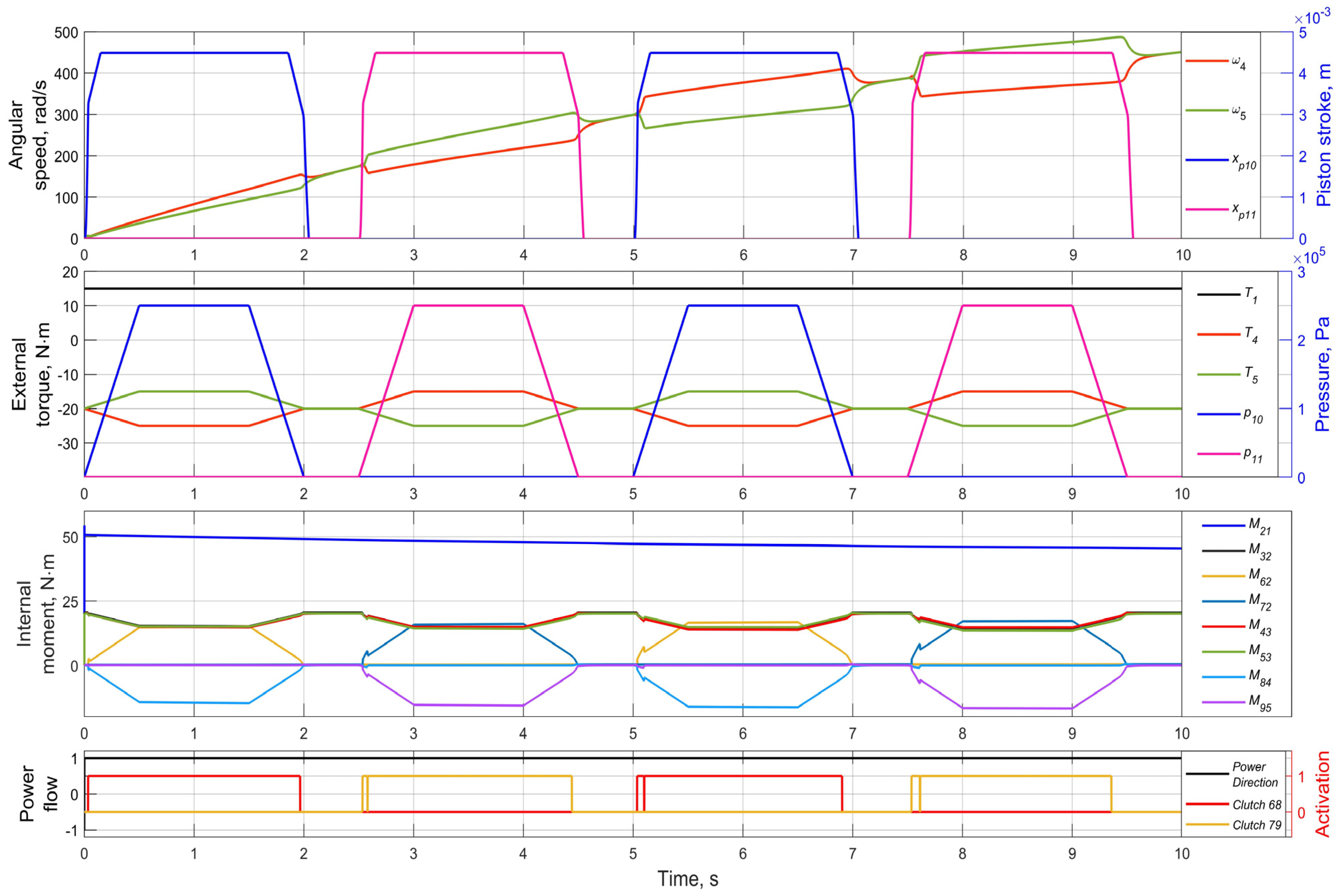
4.4. Alternating Activation
4.5. Analysis of Results
5. Conclusions
- All the simulations carried out with different sets of initial conditions confirm the model’s efficiency in transmitting a larger torque to an axle with higher resistance and equalizing the angular speeds of the output shafts. This is unlike the working principle of functioning the conventional (open) and passive limited-slip differentials. The angular speed growth on a shaft with higher resistance leads to an increase in slip (up to a critical) and, as a rule, to a rise in traction force on a wheel, which contributes to the appearance of an additional turning moment relative to the vertical vehicle axis (Figure 1). At the same time, the friction clutch usage can be practical not only in the traction mode but also in the driven one, when due to the activation of the outer wheel clutch, the negative longitudinal reaction decreases. This fosters the wide use of various algorithms for controlling the sport differential to stabilize/align the vehicle trajectory. In addition, the possibility of transmitting all the torque to one of the output axles was demonstrated to maintain the vehicle’s passability in the conditions of limited road-tire adhesion. Thus, the proposed sport differential model can be used for simulating the active control vehicle transmissions.
- The paper has proposed an alternative method for obtaining differential equations that describe the dynamics of rotational mechanical systems. As demonstrated, the main idea consisted of decomposing a mechanical system onto elementary components with the independent formation of three types of equations: dynamics, kinematic constraints, and force interactions. All the internal efforts’ signs are determined automatically. The developed mathematical apparatus effectively reduces the total number of equations for compactness and lowers the simulation time. Thus, the approach corresponds to the modern trend of multibody modeling and provides a field for developing a technique to automate the composition of motion equations for mechanical systems. The proposed method should be further improved in the complex modeling of all-wheel-drive transmissions with several DMs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gadola, M.; Chindamo, D. The Mechanical Limited-Slip Differential Revisited: High-Performance and Racing Car Applications. Int. J. Appl. Eng. Res. 2018, 13, 1478–1495. [Google Scholar]
- Morselli, R.; Zanasi, R.; Sandoni, G. Detailed and reduced dynamic models of passive and active limited-slip car differentials. Math. Comput. Model. Dyn. Syst. 2006, 12, 347–362. [Google Scholar] [CrossRef]
- Deur, J.; Ivanovic, V.; Hancock, M.; Assadian, F. Modeling and Analysis of Active Differential Dynamics. J. Dyn. Syst. Meas. Control-Trans. ASME 2010, 132, 061501. [Google Scholar] [CrossRef]
- Ji, J.; Li, Y.W.; Peng, H. Effects of Driveline and Tire Model on the Performance of Active Differential: Modeling and Simulation. In Applied Mechanics and Materials; Trans Tech Publications, Ltd.: Baech, Switzerland, 2011; Volume 97–98, pp. 771–776. Available online: https://doi.org/10.4028/www.scientific.net/amm.97-98.771 (accessed on 8 November 2021).
- Assadian, F.; Hancock, M.; Best, M. Development of a Control Algorithm for an Active Limited Slip Differential; Loughborough University: Loughborough, UK; p. 2008. Available online: https://hdl.handle.net/2134/8324 (accessed on 6 November 2021).
- Annicchiarico, C.; Rinchi, M.; Pellari, S.; Capitani, R. Design of a Semi Active Differential to Improve the Vehicle Dynamics. In Proceedings of the ASME 2014 12th Biennial Conference on Engineering Systems Design and Analysis, Copenhagen, Denmark, 25–27 July 2014; American Society of Mechanical Engineers, 2014; Volume 1, p. V001T02A006. [Google Scholar] [CrossRef]
- Jaafari, S.M.M.; Shirazi, K.H. Integrated Vehicle Dynamics Control Via Torque Vectoring Differential and Electronic Stability Control to Improve Vehicle Handling and Stability Performance. ASME. J. Dyn. Sys. Meas. Control 2018, 140, 071003. [Google Scholar] [CrossRef]
- Virlez, G.; Brüls, O.; Tromme, E.; Duysinx, P. Modeling joints with clearance and friction in multibody dynamic simulation of automotive differentials. Theor. Appl. Mech. Lett. 2013, 3, 013003. [Google Scholar] [CrossRef][Green Version]
- Brumercik, F.; Lukac, M.; Nieoczym, A. Mechanical differential mathematical model. Komunikacie 2015, 17, 88–91. [Google Scholar]
- Chen, Y.-F.; Chen, I.-M.; Chang, J.; Liu, T. Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications. Energies 2017, 10, 2157. [Google Scholar] [CrossRef]
- Chen, Y.-F.; Hsu, H.-C.; Yang, C.-P.; Liu, T. Design and Modeling of a Novel Torque Vectoring Differential System. MATEC Web Conf. 2017, 108, 07004. [Google Scholar] [CrossRef]
- Forstinger, M.; Bauer, R.; Hofer, A. Modelling and Simulation of Passive Limited-slip Differentials. IFAC-Pap. 2015, 48, 502–507. [Google Scholar] [CrossRef]
- Virlez, G.; Brüls, O.; Duysinx, P.; Poulet, N. Simulation of Differentials in Four-Wheel Drive Vehicles Using Multibody Dynamics. In Proceedings of the ASME Design Engineering Technical Conference, Washington, DC, USA, 28–31 August 2011; Volume 4. [Google Scholar] [CrossRef]
- Russo, R.; Strano, S.; Terzo, M. Enhancement of vehicle dynamics via an innovative magnetorheological fluid limited slip differential. Mech. Syst. Signal Process. 2016, 70–71, 1193–1208. [Google Scholar] [CrossRef]
- Litvinov, A.S.; Farobin, Y.E. Automobile: Theory of Operational Properties: Textbook for High Schools; Mashinostroenie: Moscow, Russia, 1989. [Google Scholar]
- Audi Sport Differential. 2021. Available online: https://www.audi-mediacenter.com/en/40-years-of-quattro-the-all-conquering-technology-from-audi-12598/the-mechanical-quattro-systems-12602 (accessed on 7 August 2021).
- Audi Sport Differential Technology. 2021. Available online: https://www.youtube.com/watch?v=FwhrmFuov-4 (accessed on 12 August 2021).
- Grishkevich, A.I. Automobiles: Theory: Textbook for High Schools; High Sch.: Minsk, Belarus, 1986; Volume 208, p. ill. [Google Scholar]
- MATLAB R2021b. Available online: https://www.mathworks.com/ (accessed on 24 October 2021).



| Reference | Topic | Features |
|---|---|---|
| [1] | Limited slip, self-locking TORSEN DM | Actualize issues of differentials and their influence on vehicle dynamics. Formed cornering moment in conditions of different slips. Compared DM to the difference in angular speeds loading torques. |
| [2] | Convectional, LSD, controlled LSD | Introduced torsional stiffness and lash of mechanical gearing. Matrix approach and simulation schemes. Developed detailed and simplified DM models. Combined DM and vehicle modeling for testing the cornering effect. |
| [3] | Active DM, Torque Vectoring | Developed a unified math model for active differential dynamics. Various DM designs and levels of model complexity are used. Restricted applicability as estimated time response is needed. |
| [4] | Active LSD (ALSD) | Investigated driveline and tire model effects on the ALSD performance. ALSD design includes friction clutches for transmitting the torque. Energy losses math models and Simulink tools are included. |
| [5] | Active LSD | Developed a control algorithm for a rear-wheel-drive sport vehicle. Compared ALSD impact on vehicle model behavior. Assessed ALSD influence on driver workload. |
| [6] | Active LSD | TV differential mechanism with electrohydraulic actuation. Race car model with 7 DOFs and low ground effect. Implemented nested control loop for the actuation system. |
| [7] | TV differential, electronic stability control | Nonlinear vehicle model based on CarSim software. TV Differential with two series of planetary gears. Electronic stability model with three-layer Integrated control system. Unscented Kalman filter and controller based on BA optimization. |
| [8] | TORSEN DM | Three-dimensional cylindrical joint model with clearance, misalignment, and friction. Matrix dynamics system including holonomic bi-lateral constraints. |
| [9] | Inter-wheel differential | DM with power balance and kinematic relations among three shafts. Three differential equations; no efficiencies or changes in power flows. |
| [10] | TV differential | New TV differential based on a Ravigneaux gear set. Two different speed ratios with only one pair of gear sets. SimulationX software is used to test maneuverability and steerability. |
| [11] | Original DM design | DM for TV concept; design combines inner gears. Math model includes dynamics and kinematic constraints equations. Losses, efficiencies, and power flow direction are neglected. |
| [12] | Asymmetric differential | Developed two DM variants (conic and planetary gear). Dynamics and constraints; static friction and limited-slip functionality. Overcomes simulation problems of discontinuity at zero angular speed. |
| [13] | TORSEN DM | Multibody simulation using nonlinear FEM. Flexible gear pair joints and contact conditions are used as constraints. AWD model for estimating torque redistribution. |
| [14] | Controllable DM | DM based on the magnetorheological fluid to realize the locking state. Torque, power balance, and kinematic constraint equations. Double-controller scheme including extended Kalman filter. SIL and HIL modeling using experimental prototypes. |
| 1.551 | 5.8 | 0.887 | 33 | 41 | 1.242 | 25 | 35 | 0.714 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diachuk, M.; Easa, S.M. Improved Mathematical Approach for Modeling Sport Differential Mechanism. Vehicles 2022, 4, 74-99. https://doi.org/10.3390/vehicles4010005
Diachuk M, Easa SM. Improved Mathematical Approach for Modeling Sport Differential Mechanism. Vehicles. 2022; 4(1):74-99. https://doi.org/10.3390/vehicles4010005
Chicago/Turabian StyleDiachuk, Maksym, and Said M. Easa. 2022. "Improved Mathematical Approach for Modeling Sport Differential Mechanism" Vehicles 4, no. 1: 74-99. https://doi.org/10.3390/vehicles4010005
APA StyleDiachuk, M., & Easa, S. M. (2022). Improved Mathematical Approach for Modeling Sport Differential Mechanism. Vehicles, 4(1), 74-99. https://doi.org/10.3390/vehicles4010005







