Next Article in Journal
Surface Thermodynamic Properties of Styrene–Divinylbenzene Copolymer Modified by Supramolecular Structure of Melamine Using Inverse Gas Chromatography
Next Article in Special Issue
Diazenium Betaines Derived from the Stable Free Radical DPPH with Diradicaloid Behavior
Previous Article in Journal
Hydrothermal Generation, Crystal Structures, and Catalytic Performance of Mn(II), Cu(II), and Ni(II) Coordination Polymers Based on a Pyridine–Tricarboxylate Ligand
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Chemistry
Volume 6
Issue 5
10.3390/chemistry6050049
chemistry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

The Magnetic Properties of Fluorenyl and tert-Butyl-nitroxyl Acene-Based Derivatives: A Quantum Chemical Insight

by
Alyona A. Starikova
*,
Maxim G. Chegerev
,
Andrey G. Starikov
and
Vladimir I. Minkin
Institute of Physical and Organic Chemistry, Southern Federal University, Stachka Avenue 194/2, 344090 Rostov-on-Don, Russia
*
Author to whom correspondence should be addressed.
Chemistry 2024, 6(5), 816-829; https://doi.org/10.3390/chemistry6050049
Submission received: 22 July 2024 / Revised: 17 August 2024 / Accepted: 19 August 2024 / Published: 23 August 2024
(This article belongs to the Special Issue Open-Shell Systems—a Memorial Issue Dedicated to Professor Masayoshi Nakano)
Browse Figures
Versions Notes

Abstract

:
Acenes, as a class of polycyclic aromatic hydrocarbons, attract considerable attention due to their remarkable nonlinear optical and magnetic properties. The aim of this work was the elucidation of the capability of radical-substituted acene derivatives to undergo spin-state-switching rearrangements. For this purpose, a series of acene-based (anthracene, pentacene, heptacene) molecules bearing fluorenyl and tert-butyl-nitroxyl radicals were investigated through comprehensive quantum chemical modeling of their electronic structures, isomerization and magnetic properties. A possible mechanism of the transformation of the closed-shell folded isomer into the biradical twisted structure of the bis-fluorenyl anthracene has been ascertained by applying the procedure of searching for the Minimum Energy Crossing Point. The conditions favoring the occurrence of spin-state-switching in such classes of polycyclic aromatic hydrocarbon derivatives have been formulated. By varying the size of an acene core and the type of radical substituent, the compounds capable of changing their magnetic properties have been revealed. Considering the unique features of radical-bearing acene-based derivatives, the proposed molecules can be used as functional materials in photonics and electronics.

Graphical Abstract

1. Introduction

Open-shell acene-based polycyclic aromatic hydrocarbon (PAH) derivatives demonstrate a range of unusual optical and magnetic properties, owing to which these compounds can be used in photonics and electronics [1,2,3,4,5,6,7,8,9,10,11,12]. For example, acenes with phenalenyl groups possess increased second hyperpolarizability and are able of manifesting singlet fission [13,14,15,16,17,18]. The insertion of nitroxyl radicals has been shown to promote the stability of the photoexcited states of acenes [19,20,21,22,23,24]. Another promising application of the radical derivatives of this type of PAH is in the field of organic spintronics [25,26,27,28,29]. An actively developed approach to the design of organic molecules with spin-switchable properties is the search for compounds capable of existing in two forms, one of which (quinoid) is closed-shell, while the other (biradical) contains two unpaired electrons [30,31,32,33]. Such compounds frequently represent resonant systems in which fixing their electronic states is difficult [34,35,36]. In this regard, the greatest interest is drawn to the molecules in which the quinoid and biradical forms have appreciably different geometries, as well as the mutual transformations between them that occur under the influence of external stimuli (temperature variation, light irradiation, etc.) and require the overcoming of a sufficiently high energy barrier. A review surveying the carbon-based biradicals [37] contains examples of compounds in which isomerization between sterically hindered diamagnetic quinoid and biradical forms favors the switching of their magnetic properties.
Recently, closed-shell anthracene, tetracene and pentacene derivatives with fluorenyl radicals characterized by a folded conformation have been obtained [32]. It was found that bis-fluorenyl anthracene 1, upon heating its solution in dichlorobenzene, converts to a biradical twisted form detected by EPR (Scheme 1). At the same time, the investigation of analogous compounds on the basis of tetracene and pentacene indicated the absence of paramagnetic particles with temperature variation.
In order to shed more light on the correlation between the structure and the magnetic properties of the aforementioned class of carbon-based biradical molecules, we have performed a comprehensive quantum chemical study of the derivatives with anthracene, pentacene and heptacene central cores (1–3). In addition, similarly constructed acene-based biradicals (4–6) in which fluorenyl groups were exchanged for tert-butyl-nitroxyl radical substituents have been also studied (Scheme 2).

2. Computational Details

The density functional theory (DFT) calculations were performed using the Gaussian 16 program package [38] with the UB3LYP [39] functional and 6-311++G(d,p) basis set. This methodology has been shown to provide good reproduction of the energies and magnetic properties of polycyclic organic compounds [40,41,42,43,44]. The discussed structures, to which minima and transition states on the potential energy surface (PES) and states with “broken symmetry” (BS) [45] correspond, are found by means of full geometry optimization in the gas phase without imposing symmetric constraints, followed by checking the stability of the DFT wave function. The character of each stationary point found was ascertained by calculating the force constant matrix. The localization of transition states (TS) was performed using the Gaussian program’s standard technique (opt = (ts, calcfc)). The affiliation of the TS with the discussed mechanisms was ascertained by employing gradient descent along the positive and negative directions of the transition vector, which has an imaginary eigenvalue (frequency) [46]. The search for the minimum energy crossing points (MECPs) was performed by applying the procedure proposed by Harvey et al. [47]. The estimation of exchange spin coupling parameters J (in cm−1) was carried out using the framework of “broken symmetry” formalism [45] and with the use of the generalized spin projection method developed by Yamaguchi [48]. For the search of the BS states, the Gaussian 16 procedure (Stable = opt) was employed. To verify the results obtained by the DFT method, the geometry optimization calculations obtained through the CASSCF(8,8) methods (combined with Def2-SVP basis) were carried out using the ORCA program package [49,50] for the isomers of the compound 1. The structural visualizations presented in Figures 1 and 3–6 were prepared using the ChemCraft software (Version 1.8) [51] with the calculated atomic coordinates as input parameters.

3. Results and Discussion

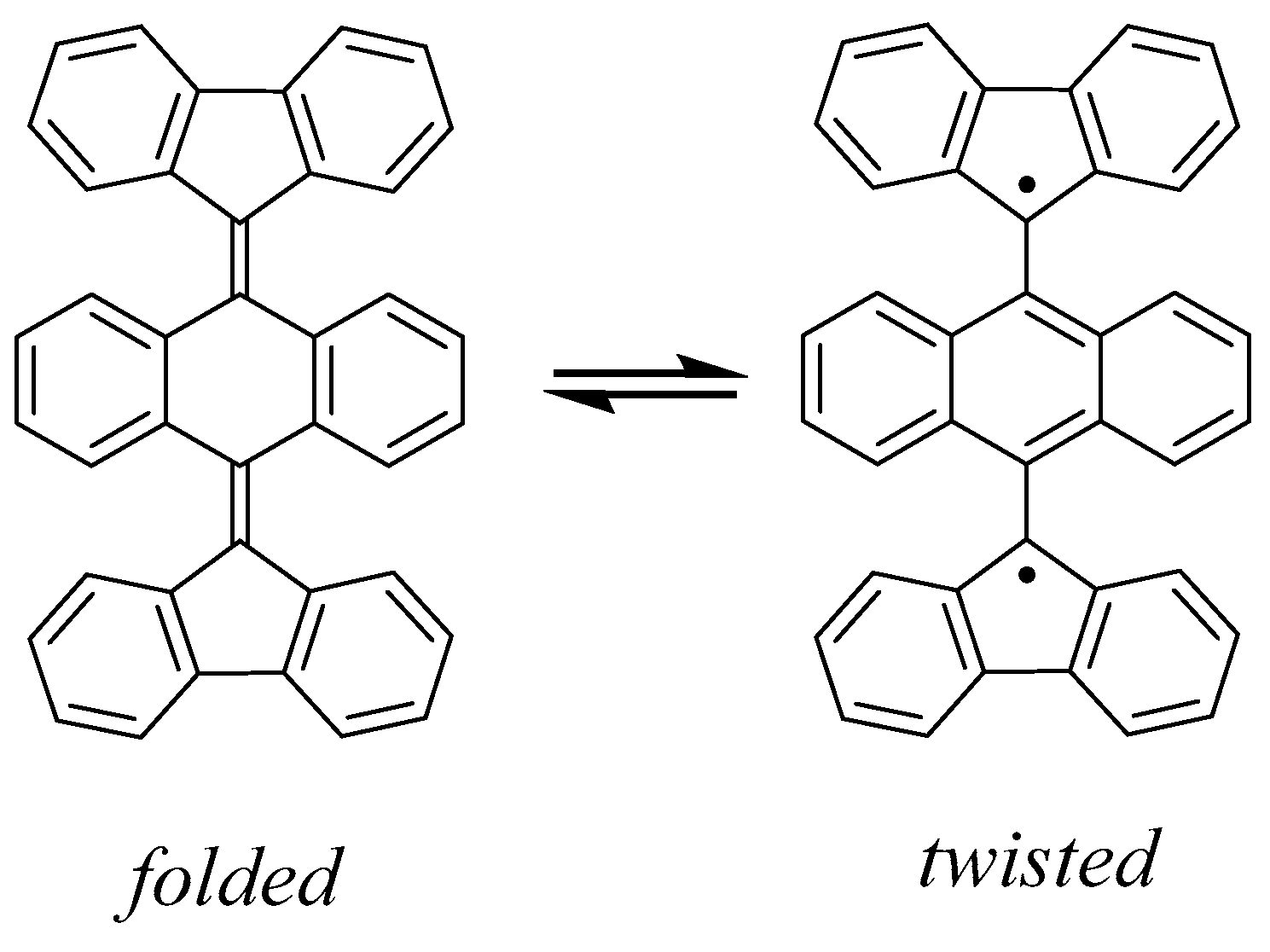
3.1. Compounds 1–3

In the above-mentioned work [32], a mechanism describing the transition of the compound 1 from a closed-shell isomer bFA (folded) to a biradical structure bFAtwist (twisted) (Scheme 1) was studied. The calculated (B3LYP/6-31G(d,p)) energy characteristics of this process are mainly consistent with the experimental data, according to which the transition between the two isomers proceeds by overcoming the energy barrier of 17.3 kcal mol−1. However, the proposed explanation for the appearance of the biradical isomer bFAtwist seems to be questionable, since this reaction occurs as a non-adiabatic process through a transition between states with different multiplicities. To ascertain the mechanisms of such processes, a search is necessary for the Minimum Energy Crossing Point (MECP), the point with minimum energy on the seam lying over the crossing of two potential energy surfaces (PESs), which allows one to correctly estimate the energy barrier between species located on PESs of different multiplicities.
Reproducing the computational results for the compound 1 obtained in [32] using the DFT B3LYP/6-31G(d,p) approximations showed that the proposed pathway of the isomerization reaction between the folded bFA and twisted bFAtwist structures is unfeasible. The structure of the bFAtwist isomer on the singlet PES has an unstable DFT wave function and does not conform to a stationary point on the PES. Analogous results were obtained by applying the extended 6-311++G(d,p) basis set (Figure S1, Table S1).
Assuming that the instability of the wave function is caused by the choice of the B3LYP functional, we performed calculations using other approximations, the results of which also led to the conclusion of the unstable wave function of the closed-shell twisted structure bFAtwist, characterized by the shortened (almost aromatic, in the range of 1.38–1.42 Å) bond lengths between the anthracene and fluorenyl carbon atoms (Table S1, Figure S1). The search for a stable solution and the subsequent geometry optimization in all the considered approximations led to a singlet biradical 1b(S), in which anthracene had a planar structure, with equalized C-C bonds (1.42–1.44 Å) of the central ring (Figure 1). At the same time, the distance between the carbon atoms of anthracene and fluorenyl increased to 1.46–1.48 Å. In order to verify this result, a calculation on a singlet PES was performed, using the multi-configurational CASSCF method (combined with the Def2-SVP basis) on the twisted bFAtwist isomer in the geometry obtained from the B3LYP/6-31G(d,p) approximation [32] and the (8,8) active space (the shapes of the orbitals included in the active space are shown in Figure S2). The optimization brought into the structure geometry close to that predicted by the DFT method for the singlet biradical 1b(S) (Figure S2). A significant contribution to the structure of two configurations with the active space occupation patterns 22220000 and 22202000 (51% and 37%, respectively) was found, which points to the biradical nature of the twisted isomer, in line with the above described DFT data. Since the CASSCF calculated geometry of 1b(S) is in good agreement with that obtained based on the UB3LYP/6-311++G(d,p) approximation, this combination of functional and basis set was applied for the subsequent calculations.
According to the spin density distribution (Figure 1) and the expected value of the spin-squared operator (Table S2), the open-shell structure 1b(S) found on the singlet PES represents a broken symmetry state (BS, a state with antiparallel orientation of electron spins) of the isomer 1b(T) corresponding to a local minimum on the triplet PES. With this in mind, we performed a search for MECP between the folded 1a(S) and biradical 1b(T) isomers with twisted geometry, enabling us to localize the structure 1MECPa–c, destabilized as compared to the ground state by 25.0 kcal mol−1 (Table 1). Gradient descent from the MECP along the singlet PES led to the initial isomer 1a(S), while the same procedure on the triplet PES resulted in the intermediate structure 1c(T), in which one of the fluorenyl groups is rotated relative to the anthracene skeleton and is spaced across it for 1.475 Å (Figure 1 and Figure S3). Further, the found intermediate 1c(T) relaxes into the twisted isomer 1b(T) via the transition state 1TSc–b(T). It should be noted that on the triplet PES, in addition to the twisted isomer 1b(T) with parallel orientation of the fluorenyl fragments, the conformer 1d(T) was found, in which the angle between the planes of the radical groups is equal to 33° (Figure S4). The energy barrier of the transition between these structures does not exceed 1 kcal mol−1 (Table S2).
Thus, a possible mechanism of the transformation of the closed-shell folded isomer 1a(S) into the biradical twisted structure 1b(T), presented schematically in Figure 2, includes two stages. First, rotation of one of the fluorenyl groups occurs, accompanied by a transition of the system via 1MECPa–c to the structure 1c(T) on the triplet PES. After that, the system passes into the isomer 1b(T) via the transition state 1TSc–b(T). Although the computed energy barrier exceeds the experimental value [32], it is comparable to that obtained using the B3LYP functional in another paper [52], which reports on the experimental and theoretical study of the mechanisms of spin transitions of anthracene derivatives with similar structures. The application of other approximations (the long-range corrected functionals wB97XD and LC-wPBE with the 6-311++G(d,p) basis set) predicts a greater discrepancy between the calculated data and the experimental data (Table 1), which confirms the validity of choosing the B3LYP functional for the calculations of such organic systems. Additional attention should be paid to the large spin contamination predicted by means of LC-wPBE (Table S3). For this reason, one should be careful in applying this functional for the computational study of open-shell compounds. The exchange spin coupling parameter in 1b(T), calculated using the Yamaguchi formula [48], indicates the average-strength antiferromagnetic exchange between the spins of unpaired electrons localized on the fluorenyl fragments (J = −77 cm−1). Therefore, the observed decay of the signal in the EPR spectrum with lowering temperatures is conditioned not only by depopulation of the paramagnetic biradical state (due to the 1b(T) → 1a(S) transition), but also by the antiferromagnetic exchange interactions between the fluorenyl radicals.
In agreement with the experimental data (and the previously performed B3LYP/6-31G(d,p) calculations [32]), a computational study of the compound 2 with a pentacene central core showed that its ground state is presented by the folded diamagnetic isomer 2a(S). According to the calculations, this species can be transformed into the singlet twisted structure 2b(S) via the transition state 2TSa–b(S) (Figure 3, Table 2). However, in opposition to the data obtained for complex 1, the twisted isomer 2b(S) is characterized by a stable DFT wavefunction on the singlet PES and exhibits sufficiently short interatomic C-C bond distances between the fluorenyl and pentacene moieties (1.405 Å). These findings indicate the possibility of isomerization between the folded and twisted forms of the compound 2 without spin-state-switching. The predicted energy barrier of this reaction, equal to 18.0 kcal mol−1, is consistent with the ΔG≠ value determined by the NMR method [32].
On the triplet PES, the structure 2c(T), destabilized relative to the ground state 2a(S) by 17.5 kcal mol−1, was localized (Table 2). In 2c(T), an elongation of the C-C bonds between the fluorenyl and acene moieties to 1.475 Å and an equalization of the bond lengths in the central ring of the pentacene are predicted; spin density is concentrated on the carbon atoms of the fluorenyl groups (Figure 3 and Figure S5). The relative energy of the discussed species is comparable to the value of the 2a(S) → 2b(S) transition barrier, which suggests the possibility of the existence of a biradical isomer with a twisted geometry. However, the search for the BS state on the singlet PES, carried out to clarify the nature and strength of the exchange interactions between the spins of unpaired electrons, led to the twisted closed-shell isomer 2b(S). Hence, the found 2c(T) triplet structure is not an isomer, but corresponds to a metastable excited state from which the system relaxes into the 2b(S) isomer without a barrier.
The earlier theoretical investigations of linear acenes have shown that increasing the size of an acene core results in considerable reduction of the HOMO-LUMO energy gap and promotes the energy preference of the singlet biradicals over the closed-shell structures [53,54,55,56,57,58,59,60,61]. It has recently been predicted that after reaching 13 condensed rings, additional paramagnetic centers appear [40]. To ascertain the effect of unpaired electrons of the central core on the magnetic properties of acene derivatives with fluorenyl radicals, we have studied the heptacene-based compound 3, which is potentially capable of having both closed- and open-shell isomers.
As follows from the computational results, the closed-shell folded structure 3a(S) corresponds to the ground state (Figure 4 and Figure S6). The search for the transition state allowed us to find the structure 3TSa–b(S), which has relative energy equal to 17.8 kcal mol−1 (Table 2). Movement along the transition vector in positive and negative directions led to the most stable isomer, 3a(S), as well as a twisted structure 3b(S) destabilized by 14.4 kcal mol−1. As in the case of pentacene derivative 2, analysis of the DFT wavefunction of the given stationary point indicates the stability of the found solution in a closed electron shell. The result obtained indicates the possibility of thermally induced 3a(S) → 3b(S) isomerization.
The stationary point 3c(Q) found on the quintet PES comprises four unpaired electrons localized at fluorenyl radical substituents (with one on each moiety) and heptacene cores (the other two electrons) (Figure 4). The significant destabilization of this structure relative to the isomer 3a(S) with a folded geometry (29.6 kcal mol−1) indicates a low probability of its observation in thermal conditions. The calculation on the triplet PES led to a structure, 3d(T) (Figure S7), which is 19.7 kcal mol−1 less energetically preferable than the ground state 3a(S). Therefore, despite the presence of additional paramagnetic centers, the compound 3 is not capable of spin-state-switching, since the predicted tetraradical 3c(Q) and biradical 3d(T) structures are significantly destabilized relative to the most stable isomer 3a(S) and correspond to metastable excited states.

3.2. Compounds 4–6

As noted earlier, experimental and theoretical studies of acene compounds with nitronylnitroxyl radicals have shown their ability to stabilize the excited states of polycyclic cores [19,20,21,22,23,24]. At the same time, the existence of closed-shell isomers of such systems has not yet been reported. The electronic structures and magnetic properties of acenes with other radicals, calculated by the DFT UB3LYP/6-311++G(d,p) method [41,62], indicate that depending on the number of condensed rings, these compounds represent bi- or tetraradical species. Here, we report on the quantum chemical modeling of anthracene, pentacene and heptacene derivatives functionalized with tert-butyl-nitroxyl radicals 4–6 (Scheme 2), aiming to elucidate their capability to switch magnetic properties.
According to the results obtained, the ground state of the compound 4 is presented by the twisted structure 4b(T), corresponding to a minimum on the triplet PES (Figure 5 and Figure S8, Table 3). The computed geometry characteristics are consistent with those found for nitronylnitroxyl derivatives [41], with the exception of a slight bend (at 7°) in the central ring caused by the steric effect of tert-butyl groups, which was previously predicted for acenes bearing two TEMPO radicals [41]. Calculations using the open-shell singlet PES led to the 4b(S) BS state. A slight energy preference towards the BS state over the 4b(T) isomer evidences the presence of weak antiferromagnetic exchange between the nitroxyl radicals (J = −15 cm−1).
The search for the closed-shell isomer resulted in the structure 4a(S), possessing folded geometry and short C-N bonds (1.33 Å). The destabilization of this isomer relative to the ground state structure is only 1.5 kcal mol−1 (Table 3), which makes it possible to expect the simultaneous presence of both isomeric forms. To ascertain the energy barrier of interconversion between the biradical (4b(T)) and singlet (4a(S)) isomers, the search for MECP was performed, which led to a 4MECPb–a structure (ΔE = 10.4 kcal mol−1). Gradient descent from 4MECPb–a along the triplet and singlet PESs confirmed its relation to the discussed transformation. Therefore, a thermally initiated transition between the biradical and diamagnetic states is possible in the compound 4, which makes it possible to consider it as a spin switch.
The computational study of pentacene derivative 5 has shown that increasing the size of a polycyclic core promotes stabilization of the closed-shell folded isomer 5a(S) on the singlet PES (Figure 6 and Figure S9, Table 3). The search for a biradical state of 5 on the triplet PES led to the structure 5b(T), the BS state for which (5b(S) BS) features antiferromagnetic exchange interactions between the radical substituents (J = −130 cm−1). The estimated energy difference between the 5a(S) and 5b(T) species (ΔE = 11 kcal mol−1) allows us to assume the possibility of their interconversion by varying the temperature. The predicted relative energy of the corresponding 5MECPa–b structure, equal to 16.8 kcal mol−1, is close to the experimentally determined energy barrier for switching between two spin states of 1 [32], which allows us to expect switching of spin states in the compound 5.
As in the case of 5, the diamagnetic structure 6a(S) with folded geometry corresponds to the ground state of the compound 6 (Figure 6, Table 3). The stationary point 6b(Q) on the quintet PES is higher in energy by 22.6 kcal mol−1 and exhibits a twisted orientation of its nitroxyl groups. To elucidate the nature of the exchange interactions in 6b(Q), attempts were made to find BS states with different orientations of spins. It appears that the search for BS states on the singlet PES invariably led to the closed-shell ground state 6a(S). On the triplet PES, a local minimum 6b(T) corresponding to a metastable excited state was found (Figure S10). Therefore, the compound 6 possesses a closed-shell folded structure. This finding contrasts with the data obtained for a pyrene derivative comprising two tert-butyl-nitroxyl groups [63]: this compound is stabilized as a biradical manifesting strong antiferromagnetic exchange interactions and the presence of a semi-quinoid structure is revealed only under certain conditions. This observation is probably conditioned by the significant rigidity of the polycyclic system, which inhibits the stabilization of structures with a folded (quinoid) form.
To clarify the reasons for the absence of paramagnetic isomers in the substituted pentacene 2 and heptacene (3, 6) species, the HOMA aromaticity indices [64,65] were calculated for the planar structures of acenes and their derivatives. As indicated by the values of this parameter, shown in Table 4, increasing the number of condensed rings results in a significant decrease in aromaticity, which may be a reason for the destabilization of the bi- and tetraradical isomers of the compounds, including 5 and 7 condensed rings.

4. Conclusions

To sum up, the performed calculations of acenes functionalized with fluorenyl radicals have shed light on the magnetic properties of the compounds with anthracene and pentacene cores [32]. It was found that the observed transition between the folded diamagnetic and twisted biradical forms in anthracene derivative 1 occurs in two stages: at the first stage, the transition to the triplet PES is realized by the rotation of one of the fluorenyl substituents around a Canthracene-Cfluorenyl bond, then the twisted structure transforms into its triplet electronic state, destabilized relative to the ground state by 6 kcal mol−1. Increasing the number of the condensed rings in the acene core of bis(fluorenyl)acenes results in substantial stabilization of the closed-shell folded and twisted isomers in the compounds 2 and 3, while the localized structures on the triplet and quintet PESs represent excited states.
Computational modeling of the acene derivatives with tert-butyl-nitroxyl radicals made it possible to identify new organic molecules with the potential of spin switches. In the case of the compound 4 with an anthracene core, twisted and folded forms that are close in energy are expected to be capable of triplet ⇄ singlet isomerization, overcoming an energy barrier not exceeding 12 kcal mol−1. The most stable diamagnetic isomer of 5 can be thermally transferred to the biradical state. It should be noted that the possibility of spin-state-switching in polycyclic organic compounds with tert-butyl-nitroxyl groups due to the formation of C=N double bonds has been reported only once [63]. The calculations predict that further elongation of the acene chain in heptacene derivative 6 gives rise to its single closed-shell isomeric form characterized by a folded structure.
Analysis of the computational results obtained and comparisons between them and those found for acenes with other radicals [20,21,22,23,24,41,62] have shown that one of the conditions for the formation of folded forms of such compounds is the localization of most of the spin density of the radical groups on the atoms directly bonded with the polycyclic system. This favors the possible formation of multiple carbon–carbon bonds between the acene core and the radical substituent. To introduce the possibility of spin-state-switching in PAHs, a biradical isomer with a planar polycyclic core (i.e., a twisted isomer) must be stabilized. The probability of the formation of a given isomer is inversely proportional to the aromaticity of rings bound to radical groups. Thus, to create rational designs of PAH derivatives with radical substituents capable of spin-state-switching, it is necessary to take into account such factors as the place of attachment of the substituent to the acene chain, the distribution of the spin density of the radical groups, the degree of aromaticity of the hydrocarbon rings and the steric rigidity of the hydrocarbon skeleton.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/chemistry6050049/s1, Table S1: Total energies (E), expectation values of the spin-squared operator (Ŝ2) and C-C bond lengths between the anthracene and fluorenyl carbon atoms, as calculated on the compound 1 on the closed-shell (twisted bFAtwist isomer) and open-shell (singlet biradical 1b(S) BS) singlet PESs by using various functionals and the 6-311++G(d,p) basis set; Figure S1: Optimized geometries, total energies and relative energies of the folded bFA isomer, twisted bFAtwist isomer and TS corresponding to the transition between them, as calculated on the compound 1 on the closed-shell singlet PES by the DFT B3LYP/6-311++G(d,p) method. Hereafter, hydrogen atoms are not shown, bond lengths are given in Å, angles are given in degrees; Figure S2: Optimized structure of the twisted bFAtwist isomer of the compound 1, shapes and occupation numbers of the natural orbitals included in the active space, as calculated by the CASSCF(8,8)/Def2-SVP method (contour value = 0.03 e Å−3); Table S2: Spin (S), total energies without (E) and with (EZPE) taking into account zero-point harmonic vibrations, total enthalpies (H298) and expectation values of the spin-squared operator (Ŝ2) of the possible states of the compounds 1–6, MECPs and TSs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S3: Spatial structure of the possible states of the compound 1, MECP and TS corresponding to the transitions between them in another projection, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S4: Spatial structure and geometry characteristics of the conformer 1d(T) (in two projections) and TS corresponding to the transitions between 1d(T) and 1b(T), as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S5: Spatial structure of the possible states of the compound 2 and TS corresponding to the transitions between them in another projection, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S6: Spatial structure of the possible states of the compound 3 and TS corresponding to the transitions between them in another projection, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S7: Spatial structure, geometry characteristics and spin density distribution of 3d(T), as calculated by the DFT UB3LYP/6-311++G(d,p) method (contour value = 0.02 e Å−3); Figure S8: Spatial structure of the possible states of the compound 4 and MECP corresponding to the transitions between them in another projection, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S9: Spatial structure of the possible states of the compounds 5 and 6 and MECP corresponding to the transitions between them in another projection, as calculated by the DFT UB3LYP/6-311++G(d,p) method; Figure S10: Spatial structure, geometry characteristics and spin density distribution of 6c(T), as calculated by the DFT UB3LYP/6-311++G(d,p) method (contour value = 0.02 e Å−3); Table S3: Spin (S), total energies without (E) and with (EZPE) taking into account zero-point harmonic vibrations, total enthalpies (H298) and expectation values of the spin-squared operator (Ŝ2) of the possible states of the compound 1, MECP and TS corresponding to the transitions between them, as calculated by the DFT wB97XD/6-311++G(d,p) and LC-wPBE/6-311++G(d,p) methods; Cartesian coordinates of the possible states of the compounds 1–6, MECPs and TSs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method.

Author Contributions

Conceptualization, A.G.S.; methodology, A.A.S.; validation, A.A.S., M.G.C. and A.G.S.; formal analysis, A.A.S. and M.G.C.; investigation, A.A.S., M.G.C. and A.G.S.; writing—original draft preparation, A.G.S.; writing—review and editing, A.A.S., M.G.C. and V.I.M.; visualization, M.G.C.; supervision, A.G.S.; project administration, A.G.S.; funding acquisition, A.A.S., M.G.C. and A.G.S. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the Russian Science Foundation (project no. 24-23-00417, https://rscf.ru/project/24-23-00417/, accessed on 18 August 2024).

Data Availability Statement

Data are contained within the article or the Supplementary Materials.

Conflicts of Interest

The authors declare no conflicts of interest. The funders had no role in the design of the study; in the collection, analysis, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.

References

  1. Anthony, J.E. Functionalized acenes and heteroacenes for organic electronics. Chem. Rev. 2006, 106, 5028–5048. [Google Scholar] [CrossRef] [PubMed]
  2. Hicks, R.G. Stable Radicals: Fundamentals and Applied Aspects of Odd-Electron Compounds; John Wiley & Sons Ltd.: Chichester, UK, 2010. [Google Scholar] [CrossRef]
  3. Sun, Z.; Wu, J. Open-Shell Polycyclic Aromatic Hydrocarbons. J. Mater. Chem. 2012, 22, 4151–4160. [Google Scholar] [CrossRef]
  4. Abe, M. Diradicals. Chem. Rev. 2013, 113, 7011–7088. [Google Scholar] [CrossRef] [PubMed]
  5. Miller, J.S. Organic- and Molecule-Based Magnets. Mater. Today 2014, 17, 224–235. [Google Scholar] [CrossRef]
  6. Kubo, T. Recent progress in quinoidal singlet biradical molecules. Chem. Lett. 2014, 44, 111–122. [Google Scholar] [CrossRef]
  7. Liu, J.; Xia, J.; Song, P.; Ding, Y.; Cui, Y.; Liu, X.; Dai, Y.; Ma, F. Organic Nonlinear Optical Materials: The Mechanism of Intermolecular Covalent Bonding Interactions of Kekulé Hydrocarbons with Significant Singlet Biradical Character. ChemPhysChem 2014, 15, 2626–2633. [Google Scholar] [CrossRef]
  8. Kumar, S.; Kumar, Y.; Kumar Keshri, S.; Mukhopadhyay, P. Recent Advances in Organic Radicals and Their Magnetism. Magnetochemistry 2016, 2, 42. [Google Scholar] [CrossRef]
  9. Hu, X.; Wang, W.; Wang, D.; Zheng, Y. The Electronic Applications of Stable Diradicaloids: Present and Future. J. Mater. Chem. C 2018, 6, 11232–11242. [Google Scholar] [CrossRef]
  10. Stuyver, T.; Chen, B.; Zeng, T.; Geerlings, P.; De Proft, F.; Hoffmann, R. Do Diradicals Behave like Radicals? Chem. Rev. 2019, 119, 11291–11351. [Google Scholar] [CrossRef]
  11. Jousselin-Oba, T.; Mamada, M.; Marrot, J.; Maignan, A.; Adachi, C.; Yassar, A.; Frigoli, M. Excellent Semiconductors Based on Tetracenotetracene and Pentacenopentacene: From Stable Closed-Shell to Singlet Open-Shell. J. Am. Chem. Soc. 2019, 141, 9373–9381. [Google Scholar] [CrossRef]
  12. Dressler, J.J.; Haley, M.M. Learning How to Fine-tune Diradical Properties by Structure Refinement. J. Phys. Org. Chem. 2020, 33, e4114. [Google Scholar] [CrossRef]
  13. Kamada, K.; Ohta, K.; Kubo, T.; Shimizu, A.; Morita, Y.; Nakasuji, K.; Kishi, R.; Ohta, S.; Furukawa, S.; Takahashi, H.; et al. Strong Two-Photon Absorption of Singlet Diradical Hydrocarbons. Angew. Chem. Int. Ed. 2007, 46, 3544–3546. [Google Scholar] [CrossRef] [PubMed]
  14. Lukman, S.; Richter, J.M.; Yang, L.; Hu, P.; Wu, J.; Greenham, N.C.; Musser, A.J. Efficient Singlet Fission and Triplet-Pair Emission in a Family of Zethrene Diradicaloids. J. Am. Chem. Soc. 2017, 139, 18376–18385. [Google Scholar] [CrossRef]
  15. Casanova, D. Theoretical Modeling of Singlet Fission. Chem. Rev. 2018, 118, 7164–7207. [Google Scholar] [CrossRef] [PubMed]
  16. Liu, X.; Tom, R.; Gao, S.; Marom, N. Assessing Zethrene Derivatives as Singlet Fission Candidates Based on Multiple Descriptors. J. Phys. Chem. C 2020, 124, 26134–26143. [Google Scholar] [CrossRef]
  17. Tonami, T.; Nagami, T.; Okada, K.; Yoshida, W.; Miyamoto, H.; Nakano, M. Quantum design for singlet-fission-induced nonlinear optical systems: Effects of π-conjugation length and molecular packing of butterfly-shaped acenes. J. Chem. Phys. 2020, 153, 084304. [Google Scholar] [CrossRef] [PubMed]
  18. Nakano, M. Open-Shell-Character-Based Molecular Design Principles: Applications to Nonlinear Optics and Singlet Fission. Chem. Rec. 2017, 17, 27–62. [Google Scholar] [CrossRef]
  19. Teki, Y.; Toichi, T.; Nakajima, S. π Topology and Spin Alignment in Unique Photoexcited Triplet and Quintet States Arising from Four Unpaired Electrons of an Organic Spin System. Chem. Eur. J. 2006, 12, 2329–2336. [Google Scholar] [CrossRef]
  20. Kawanaka, Y.; Shimizu, A.; Shinada, T.; Tanaka, R.; Teki, Y. Using Stable Radicals To Protect Pentacene Derivatives from Photodegradation. Angew. Chem. Int. Ed. 2013, 52, 6643–6647. [Google Scholar] [CrossRef]
  21. Shimizu, A.; Ito, A.; Teki, Y. Photostability enhancement of the pentacene derivative having two nitronyl nitroxide radical substituents. Chem. Commun. 2016, 52, 2889–2892. [Google Scholar] [CrossRef]
  22. Minami, N.; Yoshida, K.; Maeguchi, K.; Kato, K.; Shimizu, A.; Kashima, G.; Fujiwara, M.; Uragami, C.; Hashimoto, H.; Teki, Y. p-Topology and ultrafast excited-state dynamics of remarkably photochemically stabilized pentacene derivatives with radical substituents. Phys. Chem. Chem. Phys. 2022, 24, 13514–13518. [Google Scholar] [CrossRef] [PubMed]
  23. Kato, K.; Teki, Y. Theoretical investigation of multi-spin excited states of anthracene radical-linked p-conjugated spin systems by computational chemistry. Phys. Chem. Chem. Phys. 2024, 26, 8106–8114. [Google Scholar] [CrossRef]
  24. Shinozuka, T.; Shimizu, D.; Matsuda, K. Theoretical investigation of the effect of radical substituents on the open-shell character of polycyclic aromatic hydrocarbons. New J. Chem. 2024, 48, 8683–8689. [Google Scholar] [CrossRef]
  25. Sanvito, S. Molecular spintronics. Chem. Soc. Rev. 2011, 40, 3336–3355. [Google Scholar] [CrossRef]
  26. Pilevarshahri, R.; Rungger, I.; Archer, T.; Sanvito, S.; Shahtahmassebi, N. Spin transport in higher n-acene molecules. Phys. Rev. B 2011, 84, 174437. [Google Scholar] [CrossRef]
  27. Ratera, I.; Veciana, J. Playing with organic radicals as building blocks for functional molecular material. Chem. Soc. Rev. 2012, 41, 303–349. [Google Scholar] [CrossRef]
  28. Cano, J.; Lloret, F.; Julve, M. Theoretical design of magnetic wires from acene and nanocorone derivatives. Dalton Trans. 2016, 45, 16700–16708. [Google Scholar] [CrossRef]
  29. Zhang, H.; Miao, F.; Liu, X.; Wang, D.; Zheng, Y. Recent Advances of Stable Phenoxyl Diradicals. Chem. Res. Chin. Univ. 2023, 39, 170–175. [Google Scholar] [CrossRef]
  30. Kurata, H.; Tanaka, T.; Oda, M. Dibenzoannulated 3,5,3″,5″-Tetra(t-butyl)-p-terphenoquinone. A Reversible, Photochemical-Thermal Switching System Involving Restricted Conformational Change. Chem. Lett. 1999, 28, 749–750. [Google Scholar] [CrossRef]
  31. Wentrup, C.; Regimbald-Krnel, M.J.; Müller, D.; Comba, P.A. Thermally Populated, Perpendicularly Twisted Alkene Triplet Diradical. Angew. Chem. Int. Ed. 2016, 55, 14600–14605. [Google Scholar] [CrossRef]
  32. Yin, X.; Low, J.Z.; Fallon, K.J.; Paley, D.W.; Campos, L.M. The Butterfly Effect in Bisfluorenylidene-Based Dihydroacenes: Aggregation Induced Emission and Spin Switching. Chem. Sci. 2019, 10, 10733–10739. [Google Scholar] [CrossRef]
  33. Hamamoto, Y.; Hirao, Y.; Kubo, T. Biradicaloid Behavior of a Twisted Double Bond. J. Phys. Chem. Lett. 2021, 12, 4729–4734. [Google Scholar] [CrossRef] [PubMed]
  34. Ravat, P.; Baumgarten, M. “Tschitschibabin type Biradicals”: Benzenoid or Quinoid? Phys. Chem. Chem. Phys. 2015, 17, 983–991. [Google Scholar] [CrossRef]
  35. Ten, Y.A.; Troshkova, N.M.; Tretyakov, E.V. From spin-labelled fused polyaromatic compounds to magnetically active graphene nanostructures. Russ. Chem. Rev. 2020, 89, 693–712. [Google Scholar] [CrossRef]
  36. Tretyakov, E.V.; Ovcharenko, V.I.; Terent’ev, A.O.; Krylov, I.B.; Magdesieva, T.V.; Mazhukin, D.G.; Gritsan, N.P. Conjugated nitroxides. Russ. Chem. Rev. 2022, 91, RCR5025. [Google Scholar] [CrossRef]
  37. Ishigaki, Y.; Harimoto, T.; Shimajiri, T.; Suzuki, T. Carbon-based Biradicals: Structural and Magnetic Switching. Chem. Rev. 2023, 123, 13952–13965. [Google Scholar] [CrossRef]
  38. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16 (Revision C.01); Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
  39. Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
  40. Starikova, A.A.; Chegerev, M.G.; Starikov, A.G.; Metelitsa, A.V.; Minkin, V.I.; Aldoshin, S.M. Size matters: Computational insight into magnetic properties of extended acenes. Chem. Phys. Lett. 2023, 833, 140965. [Google Scholar] [CrossRef]
  41. Starikov, A.G.; Chegerev, M.G.; Starikova, A.A.; Minkin, V.I. Organic Polyradicals Based on Acenes. Computational Modeling. Dokl. Chem. 2022, 503, 51–55. [Google Scholar] [CrossRef]
  42. Starikova, A.A.; Starikov, A.G.; Minyaev, R.M.; Boldyrev, A.I.; Minkin, V.I. Magnetic Properties of Acenes and Their o-Quinone Derivatives: Computer Simulation. Dokl. Chem. 2018, 478, 21–25. [Google Scholar] [CrossRef]
  43. Minkin, V.I.; Starikov, A.G.; Starikova, A.A.; Gapurenko, O.A.; Minyaev, R.M.; Boldyrev, A.I. Electronic structure and magnetic properties of the triangular nanographenes with radical substituents: A DFT study. Phys. Chem. Chem. Phys. 2020, 22, 1288–1298. [Google Scholar] [CrossRef]
  44. Minkin, V.I.; Starikov, A.G.; Starikova, A.A. Acene-Linked Zethrenes and Bisphenalenyls: A DFT Search for Organic Tetraradicals. J. Phys. Chem. A 2021, 125, 6562–6570. [Google Scholar] [CrossRef]
  45. Noodleman, L. Valence Bond Description of Antiferromagnetic Coupling in Transition Metal Dimers. J. Chem. Phys. 1981, 74, 5737–5743. [Google Scholar] [CrossRef]
  46. Minyaev, R.M. Gradient lines on multidimensional potential energy surfaces and chemical reaction mechanisms. Russ. Chem. Rev. 1994, 63, 883–903. [Google Scholar] [CrossRef]
  47. Harvey, J.N.; Aschi, M.; Schwarz, H.; Koch, W. The singlet and triplet states of phenyl cation. A hybrid approach for locating minimum energy crossing points between non-interacting potential energy surfaces. Theor. Chem. Acc. 1998, 99, 95–99. [Google Scholar] [CrossRef]
  48. Shoji, M.; Koizumi, K.; Kitagawa, Y.; Kawakami, T.; Yamanaka, S.; Okumura, M.; Yamaguchi, K. A general algorithm for calculation of Heisenberg exchange integrals J in multispin systems. Chem. Phys. Lett. 2006, 432, 343–347. [Google Scholar] [CrossRef]
  49. Neese, F. The ORCA Program System. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
  50. Neese, F. Software update: The ORCA program system, version 4.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2017, 8, e1327. [Google Scholar] [CrossRef]
  51. Chemcraft, Version 1.8. 2014. Available online: http://www.chemcraftprog.com (accessed on 5 July 2021).
  52. Nishiuchi, T.; Ito, R.; Stratmann, E.; Kubo, T. Switchable Conformational Isomerization of an Overcrowded Tristricyclic Aromatic Ene. J. Org. Chem. 2020, 85, 179–186. [Google Scholar] [CrossRef] [PubMed]
  53. Bendikov, M.; Duong, H.M.; Starkey, K.; Houk, K.N.; Carter, E.A.; Wudl, F. Oligoacenes: Theoretical Prediction of Open-Shell Singlet Diradical Ground States. J. Am. Chem. Soc. 2004, 126, 7416–7417. [Google Scholar] [CrossRef] [PubMed]
  54. Jiang, D.; Dai, S. Electronic ground state of higher acenes. J. Phys. Chem. A 2008, 112, 332–335. [Google Scholar] [CrossRef] [PubMed]
  55. Zade, S.S.; Bendikov, M. Heptacene and Beyond: The Longest Characterized Acenes. Angew. Chem. Int. Ed. 2010, 49, 4012–4015. [Google Scholar] [CrossRef] [PubMed]
  56. Gao, X.; Hodgson, J.L.; Jiang, D.; Zhang, S.B.; Nagase, S.; Miller, G.P.; Chen, Z. Open-shell singlet character of stable derivatives of nonacene, hexacene and teranthene. Org. Lett. 2011, 13, 3316–3319. [Google Scholar] [CrossRef]
  57. Rivero, P.; Jiménez-Hoyos, C.A.; Scuseria, G.E. Entanglement and Polyradical Character of Polycyclic Aromatic Hydrocarbons Predicted by Projected Hartree-Fock Theory. J. Phys. Chem. B 2013, 117, 12750–12758. [Google Scholar] [CrossRef]
  58. Trinquier, G.; David, G.; Malrieu, J.-P. Qualitative Views on the Polyradical Character of Long Acenes. J. Phys. Chem. A 2018, 122, 6926–6933. [Google Scholar] [CrossRef]
  59. Hachmann, J.; Dorando, J.J.; Aviĺs, M.; Chan, G.K.-L. The Radical Character of the Acenes: A Density Matrix Renormalization Group Study. J. Chem. Phys. 2007, 127, 134309. [Google Scholar] [CrossRef]
  60. Bhattacharya, D.; Panda, A.; Misra, A.; Klein, D.J. Clar Theory Extended for Polyacenes and Beyond. J. Phys. Chem. A 2014, 118, 4325–4338. [Google Scholar] [CrossRef]
  61. Tönshoff, C.; Bettinger, H.F. Pushing the Limits of Acene Chemistry: The Recent Surge of Large Acenes. Chem. Eur. J. 2021, 27, 3193–3212. [Google Scholar] [CrossRef]
  62. Ali, M.d.E.; Datta, S.N. Polyacene Spacers in Intramolecular Magnetic Coupling. J. Phys. Chem. A 2006, 110, 13232–13237. [Google Scholar] [CrossRef]
  63. Ravat, P.; Teki, Y.; Ito, Y.; Gorelik, E.; Baumgarten, M. Breaking the semi-quinoid structure: Spin-switching from strongly coupled singlet to polarized triplet state. Chem. Eur. J. 2014, 20, 12041–12045. [Google Scholar] [CrossRef]
  64. Kruszewski, J.; Krygowski, T.M. Definition of aromaticity basing on the harmonic oscillator model. Tetrahedron Lett. 1972, 13, 3839–3842. [Google Scholar] [CrossRef]
  65. Krygowski, T.M. Crystallographic studies of inter- and intramolecular interactions reflected in aromatic character of.pi.-electron systems. J. Chem. Inf. Comput. Sci. 1993, 33, 70–78. [Google Scholar] [CrossRef]
Chemistry 06 00049 sch001
Scheme 1. Isomerization of bis-fluorenyl anthracene 1.
Scheme 1. Isomerization of bis-fluorenyl anthracene 1.
Chemistry 06 00049 sch001
Chemistry 06 00049 sch002
Scheme 2. Acene-based derivatives 1–6.
Scheme 2. Acene-based derivatives 1–6.
Chemistry 06 00049 sch002
Chemistry 06 00049 g001
Figure 1. The spatial structures and geometry characteristics of the possible states of the compound 1, MECP and TS corresponding to the transitions between them and the spin density distribution in 1b(S) BS, as calculated by the DFT UB3LYP/6-311++G(d,p) method. Hereafter, hydrogen atoms are not shown, bond lengths are given in Å and contour value = 0.02 e Å−3.
Figure 1. The spatial structures and geometry characteristics of the possible states of the compound 1, MECP and TS corresponding to the transitions between them and the spin density distribution in 1b(S) BS, as calculated by the DFT UB3LYP/6-311++G(d,p) method. Hereafter, hydrogen atoms are not shown, bond lengths are given in Å and contour value = 0.02 e Å−3.
Chemistry 06 00049 g001
Chemistry 06 00049 g002
Figure 2. The energy profile of the isomerization transformation between the folded (1a(S)) and twisted (1b(T)) isomers of the compound 1, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Figure 2. The energy profile of the isomerization transformation between the folded (1a(S)) and twisted (1b(T)) isomers of the compound 1, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Chemistry 06 00049 g002
Chemistry 06 00049 g003
Figure 3. The spatial structures and geometry characteristics of the possible states of the compound 2 and TS, corresponding to the transitions between them, as well as the spin density distribution in the 2c(T), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Figure 3. The spatial structures and geometry characteristics of the possible states of the compound 2 and TS, corresponding to the transitions between them, as well as the spin density distribution in the 2c(T), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Chemistry 06 00049 g003
Chemistry 06 00049 g004
Figure 4. The spatial structures and geometry characteristics of the possible states of the compound 3 and the TS corresponding to the transitions between them, along with the spin density distribution in the 3c(Q), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Figure 4. The spatial structures and geometry characteristics of the possible states of the compound 3 and the TS corresponding to the transitions between them, along with the spin density distribution in the 3c(Q), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Chemistry 06 00049 g004
Chemistry 06 00049 g005
Figure 5. The spatial structures and geometry characteristics of the possible states of the compound 4, as well as the MECP corresponding to the transitions between them, plus the spin density distribution in the 4b(S) BS, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Figure 5. The spatial structures and geometry characteristics of the possible states of the compound 4, as well as the MECP corresponding to the transitions between them, plus the spin density distribution in the 4b(S) BS, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Chemistry 06 00049 g005
Chemistry 06 00049 g006
Figure 6. The spatial structures and geometry characteristics of the possible states of the compounds 5 and 6, as well as the MECP corresponding to the transitions between them, plus the spin density distribution in the 6b(Q), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Figure 6. The spatial structures and geometry characteristics of the possible states of the compounds 5 and 6, as well as the MECP corresponding to the transitions between them, plus the spin density distribution in the 6b(Q), as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Chemistry 06 00049 g006
Chemistry 06 00049 sch003
Scheme 3. The numbering of benzene rings in the acene chain.
Scheme 3. The numbering of benzene rings in the acene chain.
Chemistry 06 00049 sch003
Table 1. The spin (S) and relative energies (ΔE) of the possible states of the compound 1, as well as the MECP and TS corresponding to the transitions between them, as calculated by the DFT UB3LYP/wB97XD/LC-wPBE/6-311++G(d,p) methods.
Table 1. The spin (S) and relative energies (ΔE) of the possible states of the compound 1, as well as the MECP and TS corresponding to the transitions between them, as calculated by the DFT UB3LYP/wB97XD/LC-wPBE/6-311++G(d,p) methods.
StructureSΔE, kcal mol−1
UB3LYPwB97XDLC-wPBE
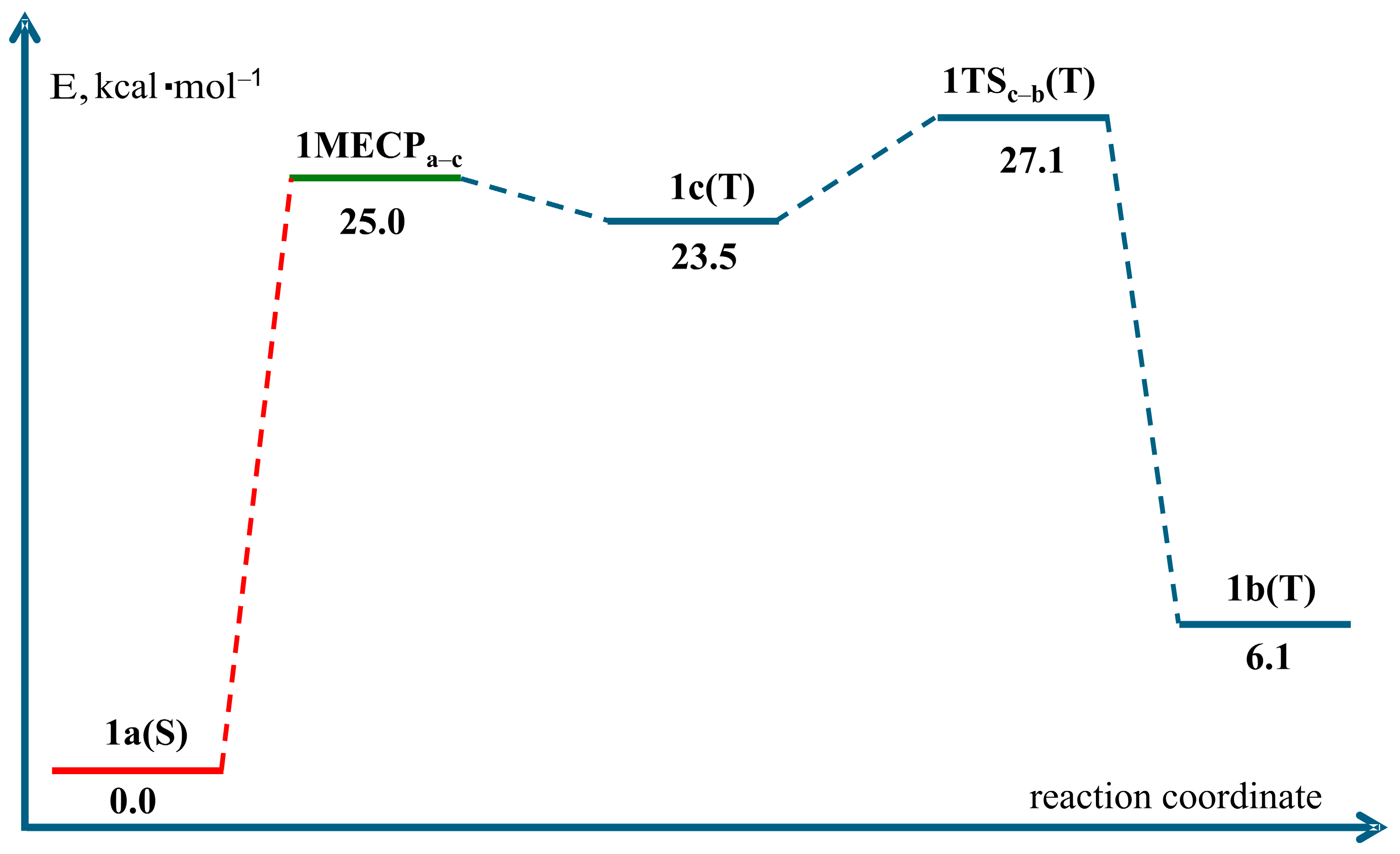
1a(S) 1 folded00.00.00.0
1b(T) twisted16.110.78.3
1b(S) BS05.910.48.2
1MECPa–c–25.028.728.1
1c(T)123.526.423.3
1TSc–b(T)127.131.829.6
1 (S)—singlet, (T)—triplet.
Table 2. The spin (S) and relative energies (ΔE) of the possible states of the compounds 2 and 3, as well as the TSs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Table 2. The spin (S) and relative energies (ΔE) of the possible states of the compounds 2 and 3, as well as the TSs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
StructureSΔE, kcal mol−1
2a(S)1 folded00.0
2TSa–b(S)018.0
2b(S) twisted013.5
2c(T) twisted117.5
3a(S) folded00.0
3TSa–b(S)017.8
3b(S) twisted014.4
3c(Q)229.6
1 (S)—singlet, (T)—triplet, (Q)—quintet.
Table 3. The spin (S) and relative energies (ΔE) of the possible states of the compounds 4–6 and the MECPs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Table 3. The spin (S) and relative energies (ΔE) of the possible states of the compounds 4–6 and the MECPs corresponding to the transitions between them, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
StructureSΔE, kcal mol−1
4a(S) 1 folded00.0
4b(T)twisted1−1.5
4b(S) BS0−1.5
4MECPb–a-10.4
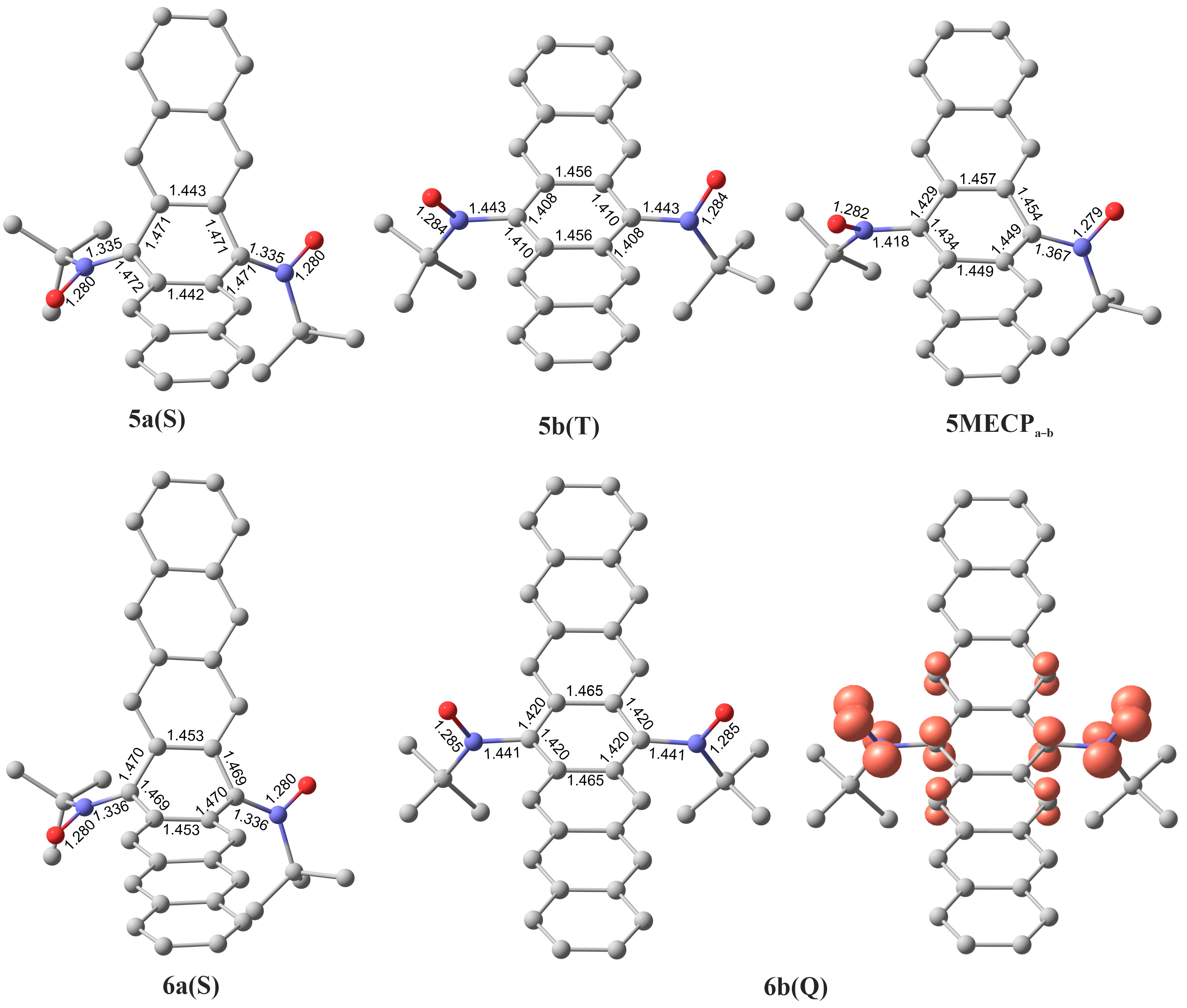
5a(S)folded00.0
5b(T)twisted111.0
5b(S) BS010.7
5MECPa–b-16.8
6a(S) folded00.0
6b(Q)222.6
1 (S)—singlet, (T)—triplet, (Q)—quintet.
Table 4. The HOMA aromaticity indices for acenes and their derivatives with fluorenyl and tert-butyl-nitroxyl radicals 1–6, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
Table 4. The HOMA aromaticity indices for acenes and their derivatives with fluorenyl and tert-butyl-nitroxyl radicals 1–6, as calculated by the DFT UB3LYP/6-311++G(d,p) method.
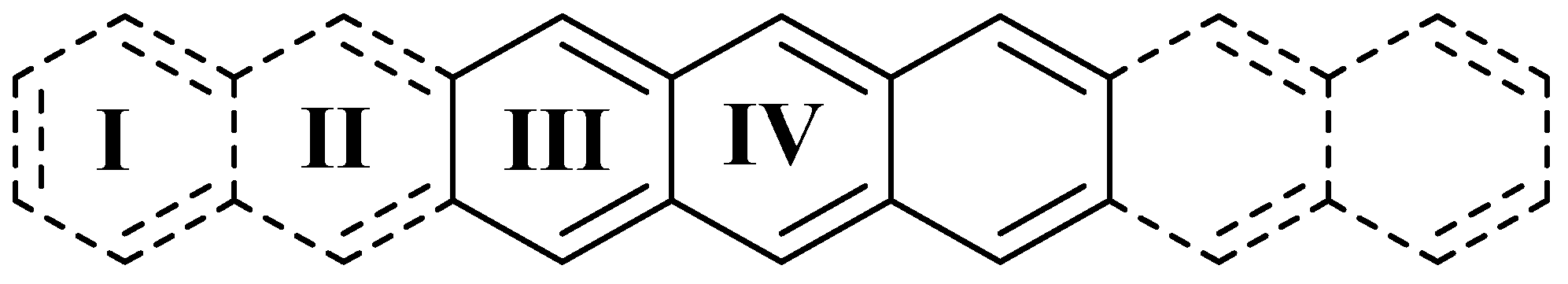
CompoundI 1IIIIIIV
Anthracene--0.6290.720
Pentacene-0.4730.5750.597
Heptacene0.7310.6820.4840.411
1--0.6230.628
2-0.5270.5860.458
30.7280.7020.5040.268
4--0.6350.680
5-0.5160.5870.530
60.7270.7040.5060.316
1 Numbering of rings is in accordance with Scheme 3.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Starikova, A.A.; Chegerev, M.G.; Starikov, A.G.; Minkin, V.I. The Magnetic Properties of Fluorenyl and tert-Butyl-nitroxyl Acene-Based Derivatives: A Quantum Chemical Insight. Chemistry 2024, 6, 816-829. https://doi.org/10.3390/chemistry6050049

AMA Style

Starikova AA, Chegerev MG, Starikov AG, Minkin VI. The Magnetic Properties of Fluorenyl and tert-Butyl-nitroxyl Acene-Based Derivatives: A Quantum Chemical Insight. Chemistry. 2024; 6(5):816-829. https://doi.org/10.3390/chemistry6050049

Chicago/Turabian Style

Starikova, Alyona A., Maxim G. Chegerev, Andrey G. Starikov, and Vladimir I. Minkin. 2024. "The Magnetic Properties of Fluorenyl and tert-Butyl-nitroxyl Acene-Based Derivatives: A Quantum Chemical Insight" Chemistry 6, no. 5: 816-829. https://doi.org/10.3390/chemistry6050049

APA Style

Starikova, A. A., Chegerev, M. G., Starikov, A. G., & Minkin, V. I. (2024). The Magnetic Properties of Fluorenyl and tert-Butyl-nitroxyl Acene-Based Derivatives: A Quantum Chemical Insight. Chemistry, 6(5), 816-829. https://doi.org/10.3390/chemistry6050049

Article Metrics

Back to TopTop