Topological Analysis of Magnetically Induced Current Densities in Strong Magnetic Fields Using Stagnation Graphs
Abstract
:1. Introduction
2. Theoretical Background
2.1. Magnetically Induced Current Densities
2.2. Topological Characteristics
3. Computational Methods
3.1. Selecting an Appropriate Objective Function

3.2. Optimization Algorithm
| Algorithm 1 Trust Region optimization |
|
3.3. Initial Point Selection
4. Results
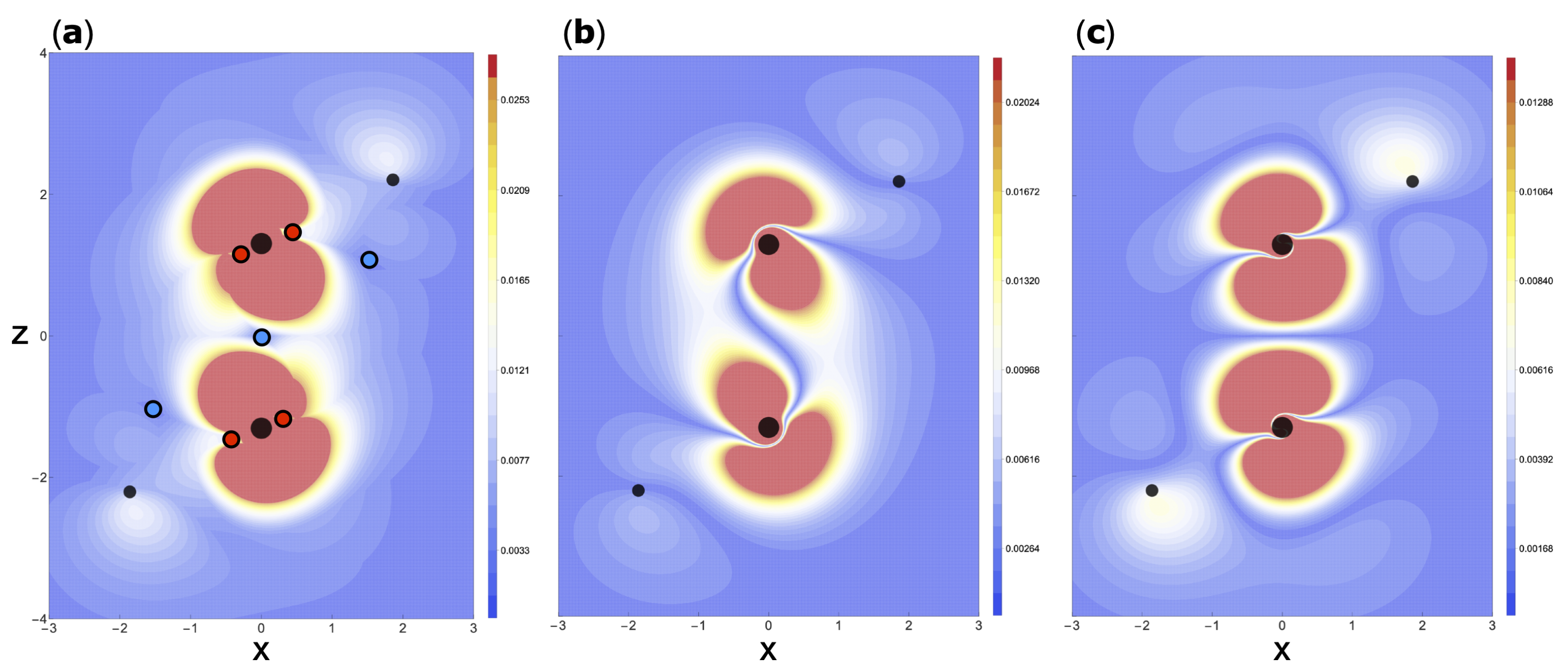
4.1. CH
4.2. CH
4.3. CH
5. Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Laws, E.A.; Stevens, R.M.; Lipscomb, W.N. Magnetic Properties of AlH and N2 from Coupled Hartree–Fock Theory. J. Chem. Phys. 1971, 54, 4269–4278. [Google Scholar] [CrossRef]
- Ditchfield, R. Self-consistent perturbation theory of diamagnetism. Mol. Phys. 1974, 27, 789–807. [Google Scholar] [CrossRef]
- Lazzeretti, P.; Rossi, E.; Zanasi, R. Magnetic properties and induced current density in acetylene. Int. J. Quantum Chem. 1984, 25, 1123–1134. [Google Scholar] [CrossRef]
- Wolinski, K.; Hinton, J.F.; Pulay, P. Efficient implementation of the gauge-independent atomic orbital method for NMR chemical shift calculations. J. Am. Chem. Soc. 1990, 112, 8251–8260. [Google Scholar] [CrossRef]
- Gauss, J. Calculation of NMR chemical shifts at second-order many-body perturbation theory using gauge-including atomic orbitals. Chem. Phys. Lett. 1992, 191, 614–620. [Google Scholar] [CrossRef]
- Keith, T.A.; Bader, R.F. Calculation of magnetic response properties using a continuous set of gauge transformations. Chem. Phys. Lett. 1993, 210, 223–231. [Google Scholar] [CrossRef]
- Ruud, K.; Helgaker, T.; Kobayashi, R.; Jørgensen, P.; Bak, K.L.; Jensen, H.J.A. Multiconfigurational self-consistent field calculations of nuclear shieldings using London atomic orbitals. J. Chem. Phys. 1994, 100, 8178–8185. [Google Scholar] [CrossRef] [Green Version]
- Coriani, S.; Lazzeretti, P.; Malagoli, M.; Zanasi, R. On CHF calculations of second-order magnetic properties using the method of continuous transformation of origin of the current density. Theor. Chim. Acta 1994, 89, 181–192. [Google Scholar] [CrossRef]
- Gauss, J.; Stanton, J.F. Coupled-cluster calculations of nuclear magnetic resonance chemical shifts. J. Chem. Phys. 1995, 103, 3561–3577. [Google Scholar] [CrossRef]
- Zanasi, R.; Lazzeretti, P.; Malagoli, M.; Piccinini, F. Molecular magnetic properties within continuous transformations of origin of the current density. J. Chem. Phys. 1995, 102, 7150–7157. [Google Scholar] [CrossRef]
- Lee, A.M.; Handy, N.C.; Colwell, S.M. The density functional calculation of nuclear shielding constants using London atomic orbitals. J. Chem. Phys. 1995, 103, 10095–10109. [Google Scholar] [CrossRef]
- Cheeseman, J.R.; Trucks, G.W.; Keith, T.A.; Frisch, M.J. A comparison of models for calculating nuclear magnetic resonance shielding tensors. J. Chem. Phys. 1996, 104, 5497–5509. [Google Scholar] [CrossRef]
- Rauhut, G.; Puyear, S.; Wolinski, K.; Pulay, P. Comparison of NMR Shieldings Calculated from Hartree-Fock and Density Functional Wave Functions Using Gauge-Including Atomic Orbitals. J. Phys. Chem. 1996, 100, 6310–6316. [Google Scholar] [CrossRef]
- Zanasi, R. Coupled Hartree–Fock calculations of molecular magnetic properties annihilating the transverse paramagnetic current density. J. Chem. Phys. 1996, 105, 1460–1469. [Google Scholar] [CrossRef]
- Ligabue, A.; Pincelli, U.; Lazzeretti, P.; Zanasi, R. Current Density Maps, Magnetizability, and Nuclear Magnetic Shielding Tensors for Anthracene, Phenanthrene, and Triphenylene. J. Am. Chem. Soc. 1999, 121, 5513–5518. [Google Scholar] [CrossRef]
- Jusélius, J.; Sundholm, D. Ab initio determination of the induced ring current in aromatic molecules. Phys. Chem. Chem. Phys. 1999, 1, 3429–3435. [Google Scholar] [CrossRef]
- Lazzeretti, P. Assessment of aromaticity via molecular response properties. Phys. Chem. Chem. Phys. 2004, 6, 217–223. [Google Scholar] [CrossRef]
- Jusélius, J.; Sundholm, D.; Gauss, J. Calculation of current densities using gauge-including atomic orbitals. J. Chem. Phys. 2004, 121, 3952–3963. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.C.; Jusélius, J.; Sundholm, D.; Gauss, J. Magnetically induced current densities in Al42- and Al44- species studied at the coupled-cluster level. J. Chem. Phys. 2005, 122, 214308. [Google Scholar] [CrossRef] [PubMed]
- Fliegl, H.; Taubert, S.; Lehtonen, O.; Sundholm, D. The gauge including magnetically induced current method. Phys. Chem. Chem. Phys. 2011, 13, 20500. [Google Scholar] [CrossRef] [PubMed]
- Sundholm, D.; Fliegl, H.; Berger, R.J. Calculations of magnetically induced current densities: Theory and applications. WIREs Comput. Mol. Sci. 2016, 6, 639–678. [Google Scholar] [CrossRef]
- Monaco, G.; Summa, F.F.; Zanasi, R. Program Package for the Calculation of Origin-Independent Electron Current Density and Derived Magnetic Properties in Molecular Systems. J. Chem. Inf. Model. 2020, 61, 270–283. [Google Scholar] [CrossRef]
- QUEST. A Rapid Development Platform for Quantum Electronic Structure Techniques. 2017. Available online: quest.codes (accessed on 23 August 2021).
- Kutzelnigg, W.; Wüllen, C.; Fleischer, U.; Franke, R.; Mourik, T. The IGLO method. Recent developments. In Nuclear Magnetic Shieldings and Molecular Structure; Springer: Dordrecht, The Netherlands, 1993; pp. 141–161. [Google Scholar] [CrossRef]
- Keith, T.; Bader, R. Calculation of magnetic response properties using atoms in molecules. Chem. Phys. Lett. 1992, 194, 1–8. [Google Scholar] [CrossRef]
- Keith, T.A.; Bader, R.F.W. Topological analysis of magnetically induced molecular current distributions. J. Chem. Phys. 1993, 99, 3669–3682. [Google Scholar] [CrossRef]
- Lazzeretti, P.; Malagoli, M.; Zanasi, R. Coupled Hartree–Fock calculations of origin-independent magnetic properties of benzene molecule. J. Chem. Phys. 1995, 102, 9619–9625. [Google Scholar] [CrossRef]
- Soncini, A.; Teale, A.M.; Helgaker, T.; Proft, F.D.; Tozer, D.J. Maps of current density using density-functional methods. J. Chem. Phys. 2008, 129, 074101. [Google Scholar] [CrossRef]
- Soncini, A. Charge and Spin Currents in Open-Shell Molecules: A Unified Description of NMR and EPR Observables. J. Chem. Theory Comput. 2007, 3, 2243–2257. [Google Scholar] [CrossRef] [PubMed]
- Lazzeretti, P. Methods of continuous translation of the origin of the current density revisited. In Marco Antonio Chaer Nascimento; Springer: Berlin/Heidelberg, Germany, 2012; pp. 103–115. [Google Scholar] [CrossRef]
- London, F. Théorie quantique des courants interatomiques dans les combinaisons aromatiques. J. Phys. Radium 1937, 8, 397–409. [Google Scholar] [CrossRef] [Green Version]
- Lazzeretti, P. Ring currents. Prog. Nucl. Magn. Reson. Spectrosc. 2000, 36, 1–88. [Google Scholar] [CrossRef]
- Pople, J. Molecular orbital theory of aromatic ring currents. Mol. Phys. 1958, 1, 175–180. [Google Scholar] [CrossRef]
- McWeeny, R. Ring currents and proton magnetic resonance in aromatic molecules. Mol. Phys. 1958, 1, 311–321. [Google Scholar] [CrossRef]
- Hegstrom, R.A.; Lipscomb, W.N. Magnetic Properties of the BH Molecule. J. Chem. Phys. 1966, 45, 2378–2383. [Google Scholar] [CrossRef]
- Gomes, J.A.N.F. Topological elements of the magnetically induced orbital current densities. J. Chem. Phys. 1983, 78, 4585–4591. [Google Scholar] [CrossRef]
- Gomes, J.A.N.F. Topology of the electronic current density in molecules. Phys. Rev. A 1983, 28, 559–566. [Google Scholar] [CrossRef]
- Fliegl, H.; Sundholm, D.; Taubert, S.; Jusélius, J.; Klopper, W. Magnetically Induced Current Densities in Aromatic, Antiaromatic, Homoaromatic, and Nonaromatic Hydrocarbons. J. Phys. Chem. A 2009, 113, 8668–8676. [Google Scholar] [CrossRef]
- Taubert, S.; Sundholm, D.; Pichierri, F. Magnetically Induced Currents in Bianthraquinodimethane-Stabilized Möbius and Hückel [16]Annulenes. J. Org. Chem. 2009, 74, 6495–6502. [Google Scholar] [CrossRef]
- Fliegl, H.; Sundholm, D.; Taubert, S.; Pichierri, F. Aromatic Pathways in Twisted Hexaphyrins. J. Phys. Chem. A 2010, 114, 7153–7161. [Google Scholar] [CrossRef]
- Fliegl, H.; Sundholm, D.; Pichierri, F. Aromatic pathways in mono- and bisphosphorous singly Möbius twisted [28] and [30]hexaphyrins. Phys. Chem. Chem. Phys. 2011, 13, 20659. [Google Scholar] [CrossRef]
- Dickens, T.K.; Mallion, R.B.; Radenković, S. Assessing the Extent of π-Electron Delocalization in Naphtho-Annelated Fluoranthenes by Means of Topological Ring-Currents. J. Phys. Chem. A 2019, 123, 1445–1450. [Google Scholar] [CrossRef] [PubMed]
- Monaco, G.; Porta, P.D.; Jabłoński, M.; Zanasi, R. Topology of the magnetically induced current density and proton magnetic shielding in hydrogen bonded systems. Phys. Chem. Chem. Phys. 2015, 17, 5966–5972. [Google Scholar] [CrossRef] [PubMed]
- Fliegl, H.; Lehtonen, O.; Sundholm, D.; Kaila, V.R.I. Hydrogen-bond strengths by magnetically induced currents. Phys. Chem. Chem. Phys. 2011, 13, 434–437. [Google Scholar] [CrossRef]
- Porta, P.D.; Zanasi, R.; Monaco, G. Hydrogen-hydrogen bonding: The current density perspective. J. Comput. Chem. 2015, 36, 707–716. [Google Scholar] [CrossRef]
- Cao, J.; London, G.; Dumele, O.; von Wantoch Rekowski, M.; Trapp, N.; Ruhlmann, L.; Boudon, C.; Stanger, A.; Diederich, F. The Impact of Antiaromatic Subunits in [4n+2] π-Systems: Bispentalenes with [4n+2] π-Electron Perimeters and Antiaromatic Character. J. Am. Chem. Soc. 2015, 137, 7178–7188. [Google Scholar] [CrossRef] [PubMed]
- Baryshnikov, G.V.; Karaush, N.N.; Valiev, R.R.; Minaev, B.F. Aromaticity of the completely annelated tetraphenylenes: NICS and GIMIC characterization. J. Mol. Model. 2015, 21. [Google Scholar] [CrossRef] [PubMed]
- Baryshnikov, G.V.; Valiev, R.R.; Karaush, N.N.; Sundholm, D.; Minaev, B.F. Aromaticity of the doubly charged [8]circulenes. Phys. Chem. Chem. Phys. 2016, 18, 8980–8992. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Haigh, C.; Mallion, R. Ring current theories in nuclear magnetic resonance. Prog. Nucl. Magn. Reson. Spectrosc. 1979, 13, 303–344. [Google Scholar] [CrossRef]
- Irons, T.J.P.; Spence, L.; David, G.; Speake, B.T.; Helgaker, T.; Teale, A.M. Analyzing Magnetically Induced Currents in Molecular Systems Using Current-Density-Functional Theory. J. Phys. Chem. A 2020, 124, 1321–1333. [Google Scholar] [CrossRef]
- Reyn, J.W. Classification and description of the singular points of a system of three linear differential equations. Z. Angew. Math. Phys. 1964, 15, 540–557. [Google Scholar] [CrossRef]
- Pelloni, S.; Faglioni, F.; Zanasi, R.; Lazzeretti, P. Topology of magnetic-field-induced current-density field in diatropic monocyclic molecules. Phys. Rev. A 2006, 74, 012506. [Google Scholar] [CrossRef] [Green Version]
- Pelloni, S.; Lazzeretti, P.; Zanasi, R. Topological models of magnetic field induced current density field in small molecules. Theor. Chem. Acc. 2009, 123, 353–364. [Google Scholar] [CrossRef]
- Pelloni, S.; Lazzeretti, P. Stagnation graphs and topological models of magnetic-field induced electron current density for some small molecules in connection with their magnetic symmetry. Int. J. Quantum Chem. 2010, 111, 356–367. [Google Scholar] [CrossRef]
- Lazzeretti, P. Topological definition of ring currents. Phys. Chem. Chem. Phys. 2016, 18, 11765–11771. [Google Scholar] [CrossRef] [PubMed]
- Irons, T.J.P.; David, G.; Teale, A.M. Optimizing Molecular Geometries in Strong Magnetic Fields. J. Chem. Theory Comput. 2021, 17, 2166–2185. [Google Scholar] [CrossRef] [PubMed]
- Ditchfield, R. On molecular orbital theories of NMR chemical shifts. Chem. Phys. Lett. 1972, 15, 203–206. [Google Scholar] [CrossRef]
- Tellgren, E.I.; Soncini, A.; Helgaker, T. Nonperturbative ab initio calculations in strong magnetic fields using London orbitals. J. Chem. Phys. 2008, 129, 154114. [Google Scholar] [CrossRef]
- Tellgren, E.I.; Teale, A.M.; Furness, J.W.; Lange, K.K.; Ekström, U.; Helgaker, T. Non-perturbative calculation of molecular magnetic properties within current-density functional theory. J. Chem. Phys. 2014, 140, 034101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vignale, G.; Rasolt, M. Density-functional theory in strong magnetic fields. Phys. Rev. Lett. 1987, 59, 2360–2363. [Google Scholar] [CrossRef]
- Furness, J.W.; Verbeke, J.; Tellgren, E.I.; Stopkowicz, S.; Ekström, U.; Helgaker, T.; Teale, A.M. Current Density Functional Theory Using Meta-Generalized Gradient Exchange-Correlation Functionals. J. Chem. Theory Comput. 2015, 11, 4169–4181. [Google Scholar] [CrossRef] [PubMed]
- Lazzeretti, P.; Rossi, E.; Zanasi, R. Singularities of magnetic-field induced electron current density: A study of the ethylene molecule. Int. J. Quantum Chem. 1984, 25, 929–940. [Google Scholar] [CrossRef]
- Lazzeretti, P. Continuity equations for electron charge densities and current densities induced in molecules by electric and magnetic fields. J. Chem. Phys. 2019, 151, 114108. [Google Scholar] [CrossRef]
- Lazzeretti, P. Stagnation graphs and separatrices of local bifurcations in velocity and current density planar vector fields. Rend. Lincei. Sci. Fis. Nat. 2019, 30, 515–535. [Google Scholar] [CrossRef]
- Monaco, G.; Zanasi, R. Magnetically Induced Current Density Spatial Domains. J. Phys. Chem. A 2019, 123, 1558–1569. [Google Scholar] [CrossRef]
- Pelloni, S.; Lazzeretti, P. Magnetotropicity of five-membered heterocyclic molecules. Theor. Chem. Acc. 2006, 117, 903–913. [Google Scholar] [CrossRef]
- Pelloni, S.; Lazzeretti, P.; Zanasi, R. Induced Orbital Paramagnetism and Paratropism in Closed-Shell Molecules. J. Phys. Chem. A 2009, 113, 14465–14479. [Google Scholar] [CrossRef] [PubMed]
- Steihaug, T. The Conjugate Gradient Method and Trust Regions in Large Scale Optimization. SIAM J. Sci. Comput. 1983, 20, 626–637. [Google Scholar] [CrossRef] [Green Version]
- Lebedev, V. Quadratures on a sphere. USSR Comput. Math. Math. Phys. 1976, 16, 10–24. [Google Scholar] [CrossRef]
- Lindh, R.; Malmqvist, P.Å.; Gagliardi, L. Molecular integrals by numerical quadrature. I. Radial integration. Theor. Chem. Acc. 2001, 106, 178–187. [Google Scholar] [CrossRef] [Green Version]
- Stoychev, G.L.; Auer, A.A.; Neese, F. Automatic Generation of Auxiliary Basis Sets. J. Chem. Theory Comput. 2017, 13, 554–562. [Google Scholar] [CrossRef]
- Lehtola, S.; Dimitrova, M.; Sundholm, D. Fully numerical electronic structure calculations on diatomic molecules in weak to strong magnetic fields. Mol. Phys. 2019, 118, e1597989. [Google Scholar] [CrossRef] [Green Version]
- Plotly. Plotly Python Graphing Library. 2021. Available online: plotly.com/python (accessed on 23 August 2021).
- Ceulemans, A.J. Group Theory Applied to Chemistry; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar] [CrossRef]
- Wetmore, R.W.; Schaefer, H.F. Triplet electronic states of acetylene: Cis and trans structures and energetics. J. Chem. Phys. 1978, 69, 1648–1654. [Google Scholar] [CrossRef]
- Pelloni, S.; Lazzeretti, P. Ring current models for acetylene and ethylene molecules. Chem. Phys. 2009, 356, 153–163. [Google Scholar] [CrossRef]
- Tellgren, E.I.; Fliegl, H. Non-perturbative treatment of molecules in linear magnetic fields: Calculation of anapole susceptibilities. J. Chem. Phys. 2013, 139, 164118. [Google Scholar] [CrossRef] [PubMed]
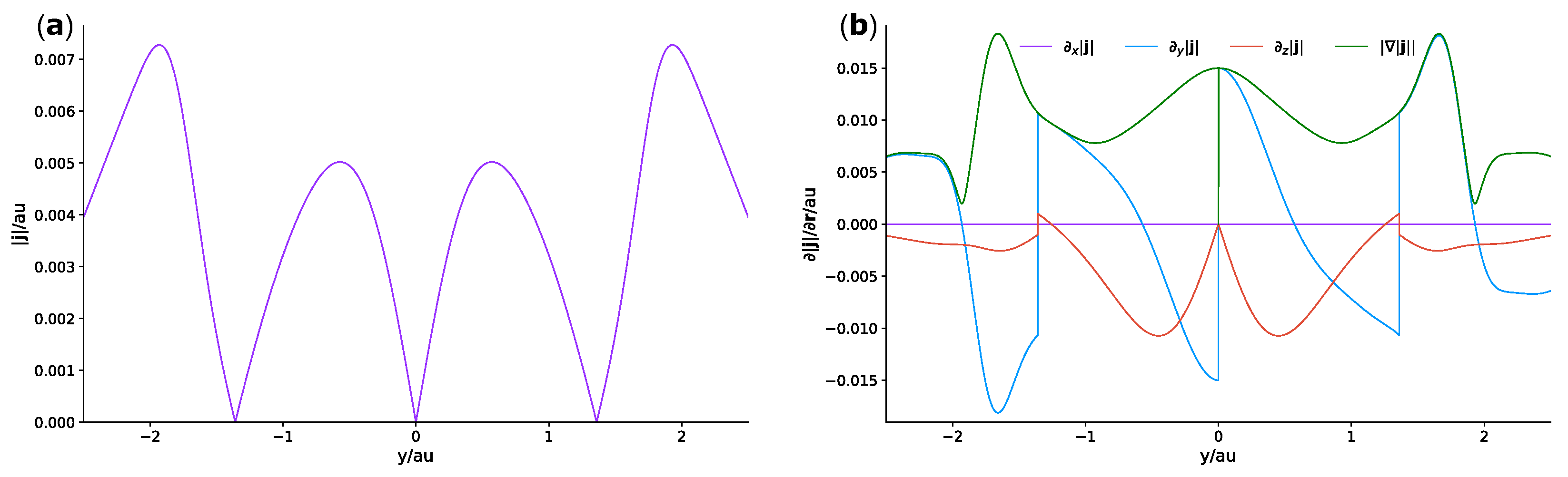

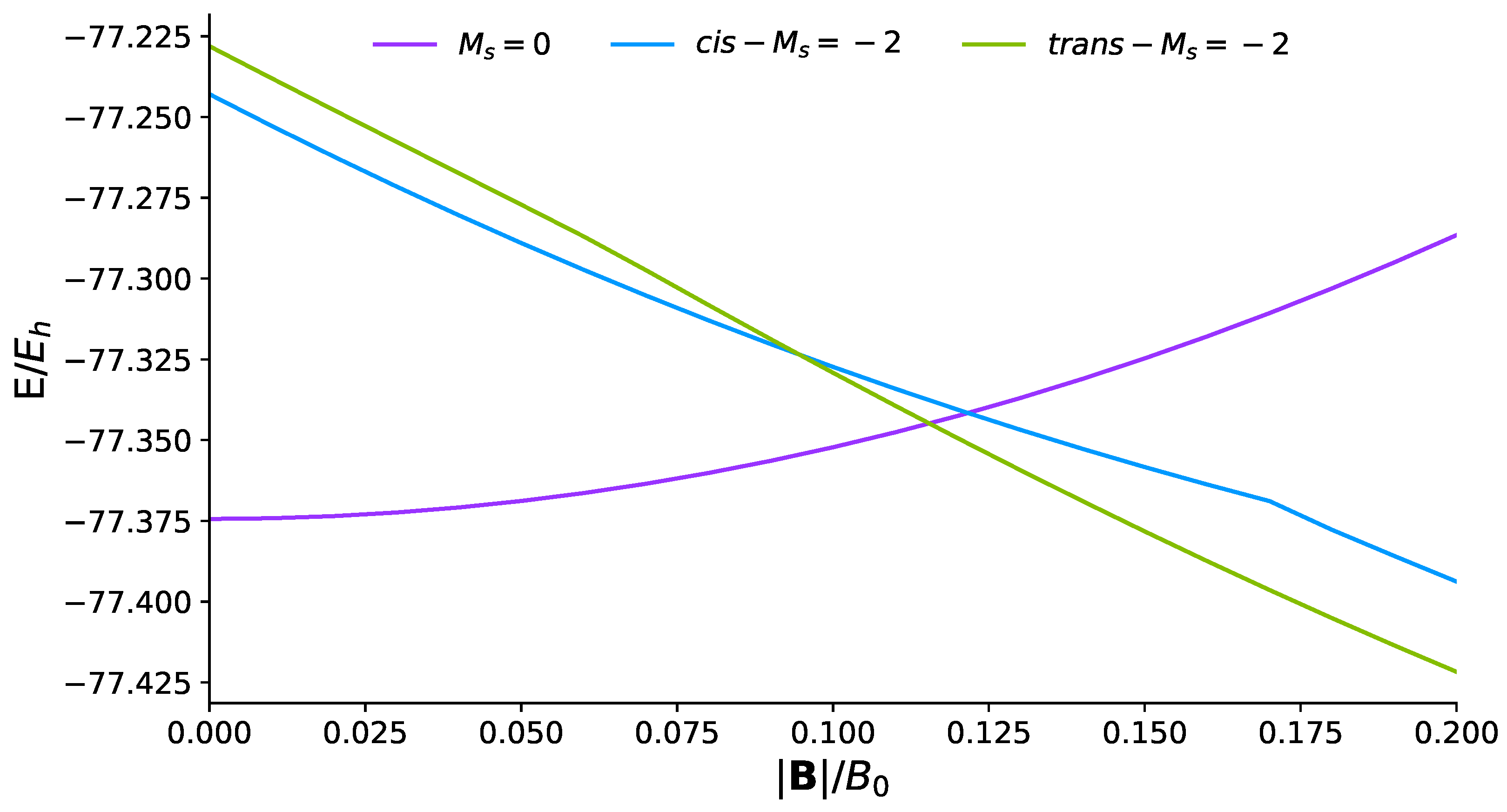
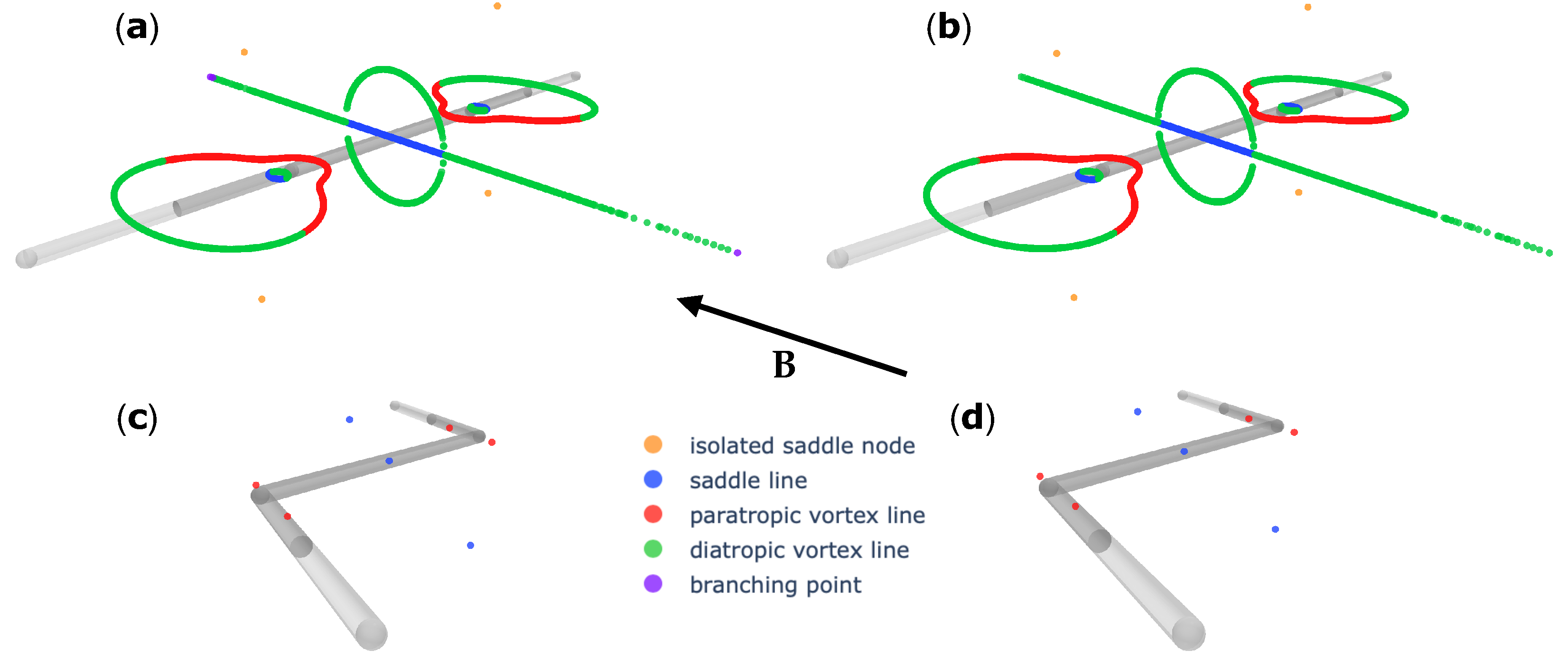
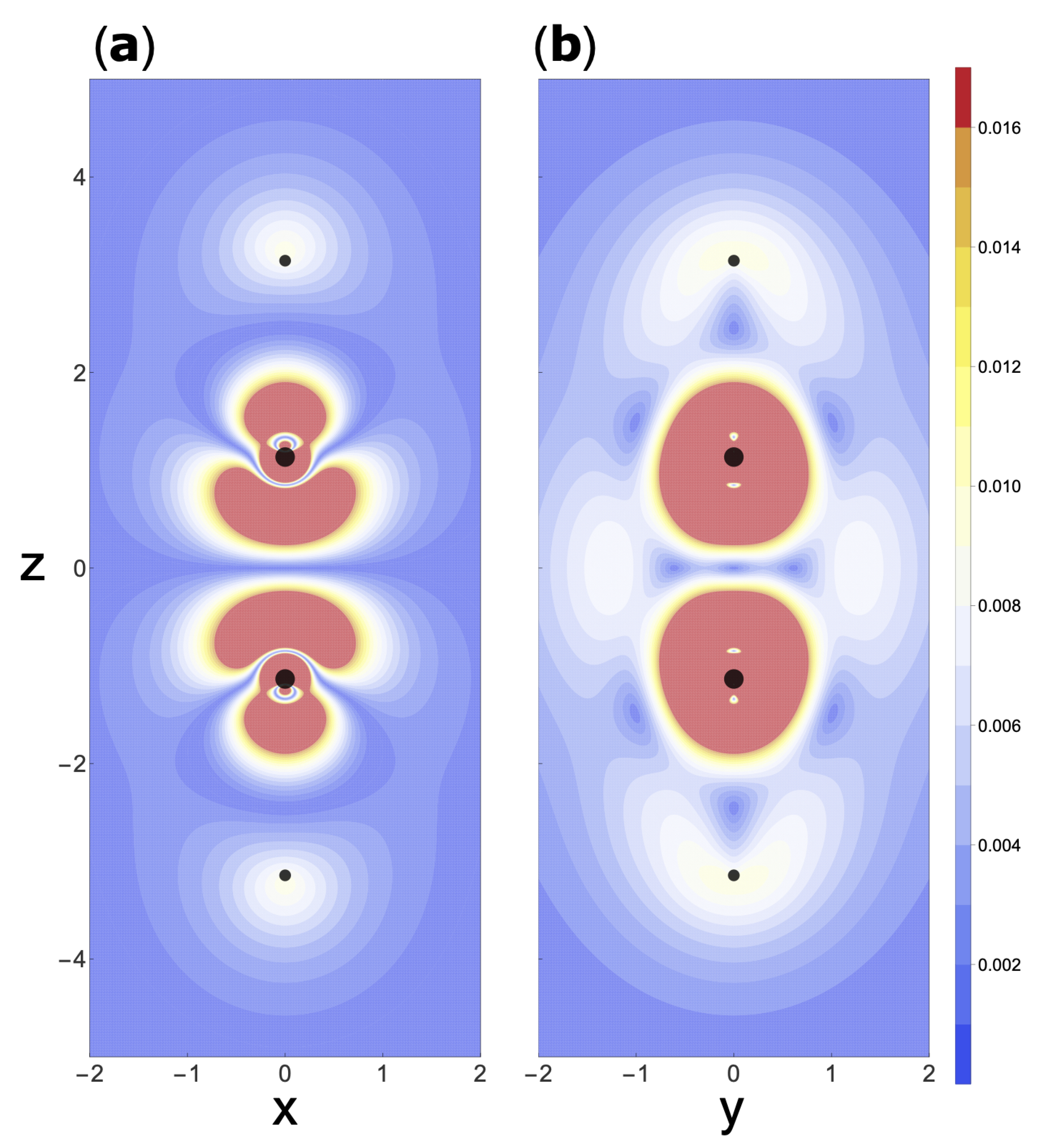


| i | Conditions | Magnetotropicity | Classification | |
|---|---|---|---|---|
| isolated singularity: saddle | ||||
| isolated singularity: paramagnetic focus | ||||
| isolated singularity: diamagnetic focus | ||||
| stagnation line: saddle | ||||
| stagnation line: paramagnetic vortex | ||||
| stagnation line: diamagnetic vortex | ||||
| branching point |
| / | Energy / | R / bohr | ∢ H–C–H / Degree | Point Group |
|---|---|---|---|---|
| 0.00 | −40.541554 | 2.0638 | 109.5 | T |
| 0.05 | −40.536608 | 2.0631, 2.0632 | 109.4, 109.5 | C |
| 0.10 | −40.522124 | 2.0614, 2.0615 | 109.3, 109.6 | C |
| 0.15 | −40.499129 | 2.0585, 2.0604 | 109.2, 109.8 | C |
| 0.20 | −40.469243 | 2.0543, 2.0620 | 108.9, 110.0 | C |
| / | Energy / | R / bohr | R / bohr | ∢ H–C–C / Degree | Point Group |
|---|---|---|---|---|---|
| 0.00 | −77.374420 | 2.2727 | 2.0136 | 180.0 | D |
| 0.05 | −77.368853 | 2.2722 | 2.0123 | 180.0 | C |
| 0.10 | −77.352224 | 2.2703 | 2.0085 | 180.0 | C |
| 0.15 | −77.378285 | 2.5974 | 2.0679 | 118.5 | C |
| 0.20 | −77.421742 | 2.6106 | 2.0658 | 115.8 | C |
| / | Energy / | R / bohr | R / bohr | ∢ H–C–H / Degree | Point Group |
|---|---|---|---|---|---|
| 0.00 | −78.633815 | 2.5150 | 2.0532 | 116.5 | D |
| 0.05 | −78.628954 | 2.5146 | 2.0523 | 116.2 | C |
| 0.10 | −78.614244 | 2.5133 | 2.0497 | 115.2 | C |
| 0.15 | −78.589425 | 2.5110 | 2.0457 | 113.7 | C |
| 0.20 | −78.554391 | 2.5084 | 2.0407 | 111.5 | C |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Irons, T.J.P.; Garner, A.; Teale, A.M. Topological Analysis of Magnetically Induced Current Densities in Strong Magnetic Fields Using Stagnation Graphs. Chemistry 2021, 3, 916-934. https://doi.org/10.3390/chemistry3030067
Irons TJP, Garner A, Teale AM. Topological Analysis of Magnetically Induced Current Densities in Strong Magnetic Fields Using Stagnation Graphs. Chemistry. 2021; 3(3):916-934. https://doi.org/10.3390/chemistry3030067
Chicago/Turabian StyleIrons, Tom J. P., Adam Garner, and Andrew M. Teale. 2021. "Topological Analysis of Magnetically Induced Current Densities in Strong Magnetic Fields Using Stagnation Graphs" Chemistry 3, no. 3: 916-934. https://doi.org/10.3390/chemistry3030067
APA StyleIrons, T. J. P., Garner, A., & Teale, A. M. (2021). Topological Analysis of Magnetically Induced Current Densities in Strong Magnetic Fields Using Stagnation Graphs. Chemistry, 3(3), 916-934. https://doi.org/10.3390/chemistry3030067






