The Crystal Chemistry of Inorganic Hydroborates †
Abstract
1. Introduction
2. Anions Packing
3. Controlling Anions Packing
4. Preferred Cation Sites
5. Anions and Cations Dynamics
6. Anions Chemistry
7. Classification of Inorganic Hydroborates
8. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References and Note
- Adams, R.M. Nomenclature of inorganic boron compounds. Pure Appl. Chem. 1972, 30, 681–710. [Google Scholar] [CrossRef]
- Lipscomb, W.N. Boron Hydrides; W.A. Benjamin Inc.: New York, NY, USA, 1963. [Google Scholar]
- Wade, K. The structural significance of the number of skeletal bonding electron-pairs in carboranes, the higher boranes and borane anions, and various transition-metal carbonyl cluster compounds. J. Chem. Soc. D 1971, 792–793. [Google Scholar] [CrossRef]
- Mingos, D.M.P. A General Theory for Cluster and Ring Compounds of the Main Group and Transition Elements. Nat. Phys. Sci. 1972, 236, 99–102. [Google Scholar] [CrossRef]
- Clark, J.D. Ignition!: An Informal History of Liquid Rocket Propellants; Rutgers University Press: New Brunswick, NJ, USA, 1972. [Google Scholar]
- Brown, H.C. Hydroboration; W.A. Benjamin Inc.: New York, NY, USA, 1962. [Google Scholar]
- Douvris, C.; Ozerov, O.V. Hydrodefluorination of Perfluoroalkyl Groups Using Silylium-Carborane Catalysts. Science 2008, 321, 1188–1190. [Google Scholar] [CrossRef] [PubMed]
- Shao, B.; Bagdasarian, A.L.; Popov, S.; Nelson, H.M. Arylation of Hydrocarbons Enabled by Organosilicon Reagents and Weakly Coordinating Anions. Science 2017, 355, 1403–1407. [Google Scholar] [CrossRef]
- Barfh, R.F.; Soloway, A.H.; Fairchild, R.G.; Brugger, R.M. Boron Neutron Capture Therapy for Cancer. Realities and Prospects. Cancer 1992, 70, 2995–3007. [Google Scholar] [CrossRef]
- Bondarev, O.; Khan, A.A.; Tu, X.; Sevryugina, Y.V.; Jalisatgi, S.S.; Hawthorne, M.F. Synthesis of [closo-B12(OH)11NH3]−: A New Heterobifunctional Dodecaborane Scaffold for Drug Delivery Applications. J. Am. Chem. Soc. 2013, 135, 13204–13211. [Google Scholar] [CrossRef]
- Goswami, L.N.; Ma, L.; Chakravarty, S.; Cai, Q.; Jalisatgi, S.S.; Hawthorne, M.F. Discrete Nanomolecular Polyhedral Borane Scaffold Supporting Multiple Gadolinium(III) Complexes as a High Performance MRI Contrast Agent. Inorg. Chem. 2013, 52, 1694–1700. [Google Scholar] [CrossRef]
- Johnson, J.W.; Brody, J.F. Lithium Closoborane Electrolytes: III. Preparation and Characterization. J. Electrochem. Soc. 1982, 129, 2213–2219. [Google Scholar] [CrossRef]
- Udovic, T.J.; Matsuo, M.; Unemoto, A.; Verdal, N.; Stavila, V.; Skripov, A.V.; Rush, J.J.; Takamura, H.; Orimo, S. Sodium superionic conduction in Na2B12H12. Chem. Commun. 2014, 50, 3750–3752. [Google Scholar] [CrossRef]
- Udovic, T.J.; Matsuo, M.; Tang, W.S.; Wu, H.; Stavila, V.; Soloninin, A.V.; Skoryunov, R.V.; Babanova, O.A.; Skripov, A.V.; Rush, J.J.; et al. Exceptional Superionic Conductivity in Disordered Sodium Decahydro-closo-decaborate. Adv. Mater. 2014, 26, 7622–7626. [Google Scholar] [CrossRef] [PubMed]
- Černý, R.; Schouwink, P. The crystal chemistry of inorganic metal borohydrides and their relation to metal oxides. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2015, 71, 619–640. [Google Scholar] [CrossRef] [PubMed]
- Pauling, L. The Principles Determining the Structure of Complex Ionic Crystals. J. Am. Chem. Soc. 1929, 51, 1010–1026. [Google Scholar] [CrossRef]
- Larsen, P.M.; Schmidt, S.; Schiøtz, J. Robust structural identification via polyhedral template matching. Model. Simul. Mater. Sci. Eng. 2016, 24, 055007. [Google Scholar] [CrossRef]
- Brighi, M.; Murgia, F.; Černý, R. Closo-hydroborate sodium salts: An emerging class of room-temperature solid electrolytes. Cell Rep. Phys. Sci. 2020, 1, 100217d. [Google Scholar]
- Kweon, K.E.; Varley, J.B.; Shea, P.; Adelstein, N.; Mehta, P.; Heo, T.W.; Udovic, T.J.; Stavila, V.; Wood, B.C. Structural, Chemical, and Dynamical Frustration: Origins of Superionic Conductivity in closo-Borate Solid Electrolytes. Chem. Mater. 2017, 29, 9142–9153. [Google Scholar] [CrossRef]
- Bradley, J.N.; Green, P.D. Relationship of structure and ionic mobility in solid MAg4I5. Trans. Faraday Soc. 1967, 63, 2516–2521. [Google Scholar] [CrossRef]
- Sadikin, Y.; Schouwink, P.; Brighi, M.; Łodziana, Z.; Černý, R. Modified Anion Packing of Na2B12H12 in Close to Room Temperature Superionic Conductors. Inorg. Chem. 2017, 56, 5006–5016. [Google Scholar] [CrossRef]
- Brighi, M.; Murgia, F.; Łodziana, Z.; Schouwink, P.; Wolczyk, A.; Černý, R. A mixed anion borane/carborane as a room temperature Na-ion solid electrolyte. J. Power Sour. 2018, 404, 7–12. [Google Scholar] [CrossRef]
- Sadikin, Y.; Brighi, M.; Schouwink, P.; Černý, R. Superionic Conduction of Sodium and Lithium in Anion-Mixed Hydroborates Na3BH4B12H12 and (Li0.7Na0.3)3BH4B12H12. Adv. Energy Mater. 2015, 5, 1501016. [Google Scholar] [CrossRef]
- Hansen, B.R.S.; Tumanov, N.; Santoru, A.; Pistidda, C.; Bednarcik, J.; Klassen, T.; Dornheim, M.; Filinchuk, Y.; Jensen, T.R. Synthesis, structures and thermal decomposition of ammine MxB12H12 complexes (M = Li, Na, Ca). Dalton Trans. 2017, 46, 7770–7781. [Google Scholar] [CrossRef] [PubMed]
- Jørgensen, M.; Hansen, B.R.S.; Lee, Y.-S.; Cho, Y.-W.; Jensen, T.R. Crystal Structures and Energy Storage Properties of Ammine Sodium Decahydro-closo-decaboranes (Na2B10H10·nNH3, n = 1,2). J. Phys. Chem. C 2019, 123, 20160–20166. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. Sect. A Cryst. Phys. Diffr. Theor. Gen. Crystallogr. 1976, 32, 751–767. [Google Scholar] [CrossRef]
- Hansen, B.R.S.; Paskevicius, M.; Li, H.W.; Akiba, E.; Jensen, T.R. Metal boranes: Progress and applications. Coord. Chem. Rev. 2016, 323, 60–70. [Google Scholar] [CrossRef]
- George, J.; Waroquiers, D.; Di Stefano, D.; Petretto, G.; Rignanese, G.-M.; Hautier, G. The Limited Predictive Power of the Pauling Rules. Angew. Chem. Int. Ed. 2020, 59, 7569–7575. [Google Scholar] [CrossRef]
- Wu, H.; Tang, W.S.; Stavila, V.; Zhou, W.; Rush, J.J.; Udovic, T.J. Structural Behavior of Li2B10H10. J. Phys. Chem. C 2015, 119, 6481–6487. [Google Scholar] [CrossRef]
- Verdal, N.; Her, J.-H.; Stavila, V.; Soloninin, A.V.; Babanova, O.A.; Skripov, A.V.; Udovic, T.J.; Rush, J.J. Complex high-temperature phase transitions in Li2B12H12 and Na2B12H12. J. Solid State Chem. 2014, 212, 81–91. [Google Scholar] [CrossRef]
- Hansen, B.R.S.; Paskevicius, M.; Jørgensen, M.; Jensen, T.R. Halogenated Sodium-closo-Dodecaboranes as Solid-State Ion Conductors. Chem. Mater. 2017, 29, 3423–3430. [Google Scholar] [CrossRef]
- Skripov, A.V.; Soloninin, A.V.; Babanova, O.A.; Skoryunov, R.V. Nuclear Magnetic Resonance Studies of Atomic Motion in Borohydride-Based Materials: Fast Anion Reorientations and Cation Diffusion. J. Alloys Compd. 2015, 645, S428–S433. [Google Scholar] [CrossRef]
- Varley, J.B.; Kweon, K.; Mehta, P.; Shea, P.; Heo, T.W.; Udovic, T.J.; Stavila, V.; Wood, B.C. Understanding Ionic Conductivity Trends in Polyborane Solid Electrolytes from Ab Initio Molecular Dynamics. ACS Energy Lett. 2017, 2, 250–255. [Google Scholar] [CrossRef]
- Skripov, A.V.; Soloninin, A.V.; Babanova, O.A.; Skoryunov, R.V. Anion and Cation Dynamics in Polyhydroborate Salts: NMR Studies. Molecules 2020, 25, 2940. [Google Scholar] [CrossRef]
- Verdal, N.; Udovic, T.J.; Stavila, V.; Tang, W.S.; Rush, J.J.; Skripov, A.V. Anion Reorientations in the Superionic Conducting Phase of Na2B12H12. J. Phys. Chem. C 2014, 118, 17483–17489. [Google Scholar] [CrossRef]
- Callear, S.K.; Nickels, E.A.; Jones, M.O.; Matauo, M.; Orimo, S.-I.; Edwards, P.; David, W.I.F. Order and disorder in lithium tetrahydroborate. J. Mater. Sci. 2011, 46, 566–569. [Google Scholar] [CrossRef]
- Heere, M.; Hansen, A.L.; Payandeh, S.H.; Aslan, N.; Gizer, G.; Sørby, M.H.; Hauback, B.C.; Ristidda, C.; Dornheim, M.; Lohstroh, W. Dynamics of porous and amorphous magnesium borohydride to understand solid state Mg-ionconductors. Sci. Rep. 2020, 10, 9080. [Google Scholar] [CrossRef] [PubMed]
- Tiritiris, I.; Schleid, T.; Müller, K. Solid-State NMR Studies on Ionic closo-Dodecaborates. Appl. Magn. Reson. 2007, 32, 459–481. [Google Scholar] [CrossRef]
- Verdal, N.; Udovic, T.J.; Rush, J.J.; Cappelletti, R.L.; Zhou, W. Reorientational Dynamics of the Dodecahydro-closo-dodecaborate Anion in Cs2B12H12. J. Phys. Chem. A 2011, 115, 2933–2938. [Google Scholar] [CrossRef]
- Skripov, A.V.; Babanova, O.A.; Soloninin, A.V.; Stavila, V.; Verdal, N.; Udovic, T.J.; Rush, J.J. Nuclear Magnetic Resonance Study of Atomic Motion in A2B12H12 (A = Na, K, Rb, Cs): Anion Reorientations and Na+ Mobility. J. Phys. Chem. C 2013, 117, 25961–25968. [Google Scholar] [CrossRef]
- Bürgi, H.B.; Restori, R.; Schwarzenbach, D. Structure of C60: Partial orientational order in the room-temperature modification of C60. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 1993, 49, 832–838. [Google Scholar] [CrossRef]
- Williams, R.E. The polyborane, carborane, carbocation continuum: Architectural patterns. Chem. Rev. 1992, 92, 177–207. [Google Scholar] [CrossRef]
- Aihara, J. Three-dimensional aromaticity of polyhedral boranes. J. Am. Chem. Soc. 1978, 100, 3339–3342. [Google Scholar] [CrossRef]
- Von Ragué Schleyer, P.; Najafian, K. Stability and Three-Dimensional Aromaticity of closo-Monocarbaborane Anions, CBn-1Hn-, and closo-Dicarboranes, C2Bn-2Hn. Inorg. Chem. 1998, 37, 3454–3470. [Google Scholar] [CrossRef] [PubMed]
- Douvris, C.; Michl, J. Update 1 of: Chemistry of the Carba-closo-dodecaborate(−) Anion, CB11H12–. Chem. Rev. 2013, 113, PR179–PR233. [Google Scholar] [CrossRef] [PubMed]
- Hofmann, M.; Fox, M.A.; Greatrex, R.; von Ragué Schleyer, P.; Williams, R.E. Empirical and ab Initio Energy/Architectural Patterns for 73 nido-6〈V〉-Carborane Isomers, from B6H9− to C4B2H6. Inorg. Chem. 2001, 40, 1790–1801. [Google Scholar] [CrossRef] [PubMed]
- Payandeh, S.H.; Asakura, R.; Avramidou, P.; Rentsch, D.; Łodziana, Z.; Černý, R.; Remhof, A.; Battaglia, C. Nido-Borate/Closo-Borate Mixed-Anion Electrolytes for All-Solid-State Batteries. Chem. Mater. 2020, 32, 1101–1110. [Google Scholar]
- Tang, W.S.; Matsuo, M.; Wu, H.; Stavila, V.; Zhou, W.; Talin, A.A.; Soloninin, A.V.; Skoryunov, R.V.; Babanova, O.A.; Skripov, A.V.; et al. Liquid-Like Ionic Conduction in Solid Lithium and Sodium Monocarba—closo—Decaborates Near or at Room Temperature. Adv. Energy Mater. 2016, 6, 1502237. [Google Scholar] [CrossRef]
- Bergerhoff, G.; Brown, I.D.; Allen, F. Crystallographic Databases; (Hrsg.) International Union of Crystallography: Chester, UK, 1987; Available online: http://www.fiz-karlsruhe.de/icsd_home.html.
- Villars, P.; Cenzual, K. Pearson’s Crystal Data-Crystal Structure Database for Inorganic Compounds; ASM International Materials Park: Russel Township, OH, USA, 2015. [Google Scholar]
- Villars, P.; Cenzual, K.; Gladyshevskii, R.; Iwata, S. Pauling File—Towards a holistic view. Chem. Met. Alloys 2018, 11, 43–76. [Google Scholar] [CrossRef]
- Paskevicius, M.; Jepsen, L.H.; Schouwink, P.; Černý, R.; Ravnsbaeck, D.; Filinchuk, Y.; Dornheim, M.; Besenbacher, F.; Jensen, T.R. Metal borohydrides and derivatives—Synthesis, structure and properties. Chem. Soc. Rev. 2017, 46, 1565–1634. [Google Scholar] [CrossRef]
- Axtell, J.C.; Saleh, L.M.A.; Qian, E.A.; Wixtrom, A.I.; Spokoyny, A.M. Synthesis and Applications of Perfunctionalized Boron Clusters. Inorg. Chem. 2018, 57, 2333–2350. [Google Scholar] [CrossRef]
- Muetterties, E.L.; Balthis, J.H.; Chia, Y.T.; Knoth, W.H.; Miller, H.C. Chemistry of Boranes. VIII. Salts and Acids of B10H10−2 and B12H12−2. Inorg. Chem. 1964, 3, 444–451. [Google Scholar] [CrossRef]
- Sivaev, I.B.; Prikaznov, A.V.; Naoufal, D. Fifty years of the closo-decaborate anion chemistry. Collect. Czechoslov. Chem. Commun. 2010, 75, 1149–1199. [Google Scholar] [CrossRef]
- Sivaev, I.B.; Bregadze, V.I.; Sjöberg, S. Chemistry of closo-Dodecaborate Anion [B12H12]2−: A Review. Collect. Czechoslov. Chem. Commun. 2002, 67, 679–727. [Google Scholar] [CrossRef]
- Housecroft, C.E. Carboranes as guests, counterions and linkers in coordination polymers and networks. J. Organomet. Chem. 2015, 798, 218–228. [Google Scholar] [CrossRef]
- Malinina, E.A.; Avdeeva, V.V.; Goeva, L.V.; Kuznetsov, N.T. Coordination Compounds of Electron-Deficient Boron Cluster Anions BnHn2− (n = 6, 10, 12). Russ. J. Inorg. Chem. 2010, 55, 2148–2202. [Google Scholar] [CrossRef]
- Müller, U. Symmetry Relationships between Crystal Structures; Oxford University Press: Oxford, UK, 2013. [Google Scholar]
- Blatov, V.A. Multipurpose christalochemical analysis with the program package TOPOS. IUCr Comp. Comm. Newsl. 2006, 7, 4–38. [Google Scholar]
- Topospro. Available online: https://topospro.com/databases/ttd/ (accessed on 29 September 2020).
- Her, J.-H.; Yousufuddin, M.; Zhou, W.; Jalisatgi, S.S.; Kulleck, J.G.; Zan, J.A.; Hwang, S.-J.; Bowman, R.C.; Udovic, T.J. Crystal structure of Li2B12H12: A possible intermediate species in the decomposition of LiBH4. Inorg. Chem. 2008, 47, 9757–9759. [Google Scholar] [CrossRef]
- Paskevicius, M.; Pitt, M.P.; Brown, D.H.; Sheppard, D.A.; Chumphongphan, S.; Buckley, C.E. First-order phase transition in the Li2B12H12 system. Phys. Chem. Chem. Phys. 2013, 15, 15825–15828. [Google Scholar] [CrossRef]
- This work. The symmetry of Pa-3 from ref. [63] was corrected to Fm-3.
- Tang, W.S.; Unemoto, A.; Zhou, W.; Stavila, V.; Matsuo, M.; Wu, H.; Orimo, S.-I.; Udovic, T.J. Unparalleled lithium and sodium superionic conduction in solid electrolytes with large monovalent cage-like anions. Energy Environ. Sci. 2015, 8, 3637–3645. [Google Scholar] [CrossRef]
- Jørgensen, M.; Shea, P.T.; Tomich, A.; Varley, J.B.; Bercx, M.; Lovera, S.; Černý, R.; Zhou, W.; Udovic, T.J.; Lavallo, V.; et al. Understanding Superionic Conductivity in Lithium and Sodium Salts of Weakly Coordinating Closo-Hexahalocarbaborate Anions. Chem. Mater. 2020, 32, 1475–1487. [Google Scholar] [CrossRef]
- Payandeh, S.H.; Rentsch, D.; Asakura, R.; Łodziana, Z.; Bigler, L.; Černý, R.; Remhof, A.; Battaglia, C. Nido-Hydroborate based Electrolytes for All-Solid-State Lithium Batteries. 2020; in preparation. [Google Scholar]
- Tang, W.S.; Yoshida, K.; Soloninin, A.V.; Skoryunov, R.V.; Babanova, O.A.; Skripov, A.V.; Dimitrievska, M.; Stavila, V.; Orimo, S.-I.; Udovic, T.J. Stabilizing Superionic-Conducting Structures via Mixed-Anion Solid Solutions of Monocarba-closo-borate Salts. ACS Energy Lett. 2016, 1, 659–664. [Google Scholar] [CrossRef]
- Moury, R.; Łodziana, Z.; Remhof, A.; Duchêne, L.; Roedern, E.; Gigante, A.; Hagemann, H. Pressure-induced phase transitions in Na2B12H12, structural investigation on a candidate for solid-state electrolyte. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2019, 75, 406–413. [Google Scholar] [CrossRef]
- Wu, H.; Tang, W.S.; Zhou, W.; Stavila, V.; Rush, J.J.; Udovic, T.J. The structure of monoclinic Na2B10H10: A combined diffraction, spectroscopy, and theoretical approach. CrystEngComm 2015, 17, 3533–3540. [Google Scholar] [CrossRef]
- Dunbar, A.C.; Macor, J.A.; Girolami, G.S. Synthesis and single crystal structure of sodium octahydrotriborate, NaB3H8. Inorg. Chem. 2014, 53, 822–826. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Tang, W.S.; Zhou, W.; Tarver, J.D.; Stavila, V.; Brown, C.M.; Udovic, T.J. The low-temperature structural behavior of sodium 1-carba-closo-decaborate: NaCB9H10. J. Solid State Chem. 2016, 243, 162–167. [Google Scholar] [CrossRef]
- Tang, W.S.; Dimitrievska, M.; Stavila, V.; Zhou, W.; Wu, H.; Talin, A.A.; Udovic, T.J. Order−Disorder Transitions and Superionic Conductivity in the Sodium nido—Undeca(carba)borates. Chem. Mater. 2017, 29, 10496–10509. [Google Scholar] [CrossRef]
- Yoshida, K.; Sato, T.; Unemoto, A.; Matsuo, M.; Ikeshoji, T.; Udovic, T.J.; Orimo, S.-I. Fast sodium ionic conduction in Na2B10H10-Na2B12H12 pseudo-binary complex hydride and application to a bulk-type all-solid-state battery. Appl. Phys. Lett. 2017, 110, 103901. [Google Scholar] [CrossRef]
- Duchêne, L.; Kuhnel, R.S.; Rentsch, D.; Remhof, A.; Hagemann, H.; Battaglia, C. A highly stable sodium solid-state electrolyte based on a dodeca/deca-borate equimolar mixture. Chem. Commun. 2017, 53, 4195–4198. [Google Scholar] [CrossRef] [PubMed]
- Wunderlich, J.A.; Lipscomb, W.N. Structure of B12H12−2 ion. J. Am. Chem. Soc. 1960, 82, 4427–4428. [Google Scholar] [CrossRef]
- Hofmann, K.; Albert, B. Crystal structures of M2[B10H10] (M = Na, K, Rb) via real space simulated annealing powder techniques. Z. Kristall. 2005, 220, 142–146. [Google Scholar] [CrossRef]
- Grinderslev, J.B.; Møller, K.T.; Yan, Y.; Chen, X.-M.; Li, Y.; Li, H.-W.; Zhou, W.; Skibsted, J.; Chen, X.; Jensen, T.R. Potassium octahydridotriborate: Diverse polymorphism in a potential hydrogen storage material and potassium ion conductor. Dalton Trans. 2019, 48, 8872–8881. [Google Scholar] [CrossRef]
- Kuznetsov, I.Y.; Vinitskii, D.M.; Solntsev, K.A.; Kuznetsov, N.T. The Crystal Structure of K2B6H6 and Cs2B6H6. Russ. J. Inorg. Chem. 1987, 32, 1803–1804. [Google Scholar]
- Dimitrievska, M.; Wu, H.; Stavila, V.; Babanova, O.A.; Skoryunov, R.V.; Soloninin, A.V.; Zhou, W.; Trump, B.A.; Andersson, M.S.; Skripov, A.V.; et al. Structural and Dynamical Properties of Potassium Dodecahydro-monocarba-closo-dodecaborate: KCB11H12. J. Phys. Chem. C 2020, 124, 17992–18002. [Google Scholar] [CrossRef]
- Bernhardt, E.; Brauer, D.; Finze, M.; Willner, H. Closo-[B21H18]−: A Face-Fused Diicosahedral Borate Ion. Angew. Chem. Int. Ed. 2007, 46, 2927–2930. [Google Scholar] [CrossRef] [PubMed]
- Sadikin, Y.; Skoryunov, R.V.; Babanova, O.A.; Soloninin, A.V.; Lodziana, Z.; Brighi, M.; Skripov, A.V.; Černý, R. Anion disorder in K3BH4B12H12 and its effect on cation mobility. J. Phys. Chem. C 2017, 121, 5503–5514. [Google Scholar] [CrossRef]
- Tiritiris, I.; Schleid, T. The Dodecahydro-closo-Dodecaborates M2[B12H12] of the Heavy Alkali Metals (M = K+, Rb+, NH4+, Cs+) and their Formal Iodide Adducts M3I[B12H12] (= MI·M2[B12H12]). Z. Anorg. Allg. Chem. 2003, 629, 1390–1402. [Google Scholar] [CrossRef]
- Dimitrievska, M.; Stavila, V.; Soloninin, A.V.; Skoryunov, R.V.; Babanova, O.A.; Wu, H.; Zhou, W.; Tang, W.S.; Faraone, A.; Tarver, J.D.; et al. Nature of Decahydro-Closo-Decaborate Anion Reorientations in an Ordered Alkali-Metal Salt: Rb2B10H10. J. Phys. Chem. C 2018, 122, 15198–15207. [Google Scholar] [CrossRef]
- Guggenberger, L.J. Chemistry of boranes. XXXIII. The crystal structure of Rb2B9H9. Inorg. Chem. 1968, 7, 2260–2264. [Google Scholar] [CrossRef]
- DeBoer, B.G.; Zalkin, A.; Templeton, D.H. The crystal structure of the rubidium salt of an octa-decahydroeicosaborate(2-) photoisomer. Inorg. Chem. 1968, 7, 1085–1090. [Google Scholar] [CrossRef]
- Schouwink, P.; Sadikin, Y.; van Beek, W.; Černý, R. Experimental observation of polymerization from BH4 to B12H12 in mixed-anion A3BH4B12H12 (A = Rb, Cs). Int. J. Hydrogen Energy 2015, 40, 10902–10907. [Google Scholar] [CrossRef]
- Tiritiris, I.; Schleid, T.; Müuller, K.; Preetz, W. Structural Investigations on Cs2[B12H12]. Z. Anorg. Allg. Chem. 2000, 626, 323–325. [Google Scholar] [CrossRef]
- Verdal, N.; Wu, H.; Udovic, T.J.; Stavila, V.; Zhou, W.; Rush, J.J. Evidence of a transition to reorientational disorder in the cubic alkali-metal dodecahydro-closo-dodecaborates. J. Solid State Chem. 2011, 184, 3110–3116. [Google Scholar] [CrossRef]
- Deiseroth, H.J.; Sommer, O.; Binder, H.; Wolfer, K.; Frei, B. CsB3H8: Crystal Structure and Upgrading of the Synthesis. Z. Anorg. Allg. Chem. 1989, 571, 21–28. [Google Scholar] [CrossRef]
- Schlüter, F.; Bernhardt, E. Syntheses and Crystal Structures of the closo-Borate M[B8H9] (M = [PPh4]+ and [N(n-Bu4)]+). Inorg. Chem. 2012, 51, 511–517. [Google Scholar] [CrossRef] [PubMed]
- Greenwood, N.N.; McGinnety, J.A.; Owen, J.D. Crystal structure of caesium tetradecahydrononaborate(1–), CsB9H14. J. Chem. Soc. Dalton Trans. 1972, 986–989. [Google Scholar] [CrossRef]
- Schlüter, F.; Bernhardt, E. Fluorination of closo-[B21H18]– with aHF and F2 to yield closo-[B21H18–xFx]– (x = 1–3) and closo-[B21F18]–. Z. Anorg. Allg. Chem. 2012, 638, 594–601. [Google Scholar] [CrossRef]
- Černý, R.; Brighi, M.; Dimitrievska, M.; Udovic, T.J. Dodeca-carbahydroborates of Caesium and Rubidium, crystal structure and anions dynamic. 2020; in preparation. [Google Scholar]
- Franken, A.; Bullen, N.J.; Jelínek, T.; Thornton-Pett, M.; Teat, S.J.; Clegg, W.; Kennedy, J.D.; Hardie, M.J. Structural chemistry of halogenated monocarbaboranes: The extended structures of Cs[1-HCB9H4Br5], Cs[1-HCB11H5Cl6] and Cs[1-HCB11H5Br6]. New J. Chem. 2004, 28, 1499–1505. [Google Scholar] [CrossRef]
- Jelínek, T.; Baldwin, P.; Scheldt, W.R.; Reed, C.A. New Weakly Coordinating Anions. 2. Derivatization of the Carborane Anion CB11H12−. Inorg. Chem. 1993, 32, 1982–1990. [Google Scholar] [CrossRef]
- Rius, J.; Romerosa, A.; Teixidor, F.; Casabo, J.; Miravitlles, C. Phase transitions in cesium 7,8-dicarbaundecaborate(12): A new one-dimensional cesium solid electrolyte at 210 °C. Inorg. Chem. 1991, 30, 1376–1379. [Google Scholar] [CrossRef]
- Tiritiris, I. Untersuchungen zu Reaktivität, Aufbau und Struktureller Dynamik von Salzartigen Closo Dodekaboraten. Ph.D. Thesis, Universität Stuttgart Fakultät Chemie, Stuttgart, Germany, 2003. [Google Scholar]
- Calabrese, J.C.; Gaines, D.F.; Hildebrandt, S.J.; Morris, J.H. The Low Temperature Crystal and Molecular Structure of Beryllium Bis(octahydrotriborate), Be(B3H8)2. J. Am. Chem. Soc. 1976, 98, 5489–5492. [Google Scholar] [CrossRef]
- Gaines, D.F.; Walsh, J.L.; Calabrese, J.C. Low-Temperature Crystal and Molecular Structures of 2-Tetrahydroborato-2- berylla-nido-hexaborane(11) and 2,2′-commo-Bis[2-berylla-nido-hexaborane1(1)]. Inorg. Chem. 1978, 17, 1242–1248. [Google Scholar] [CrossRef]
- Stavila, V.; Her, J.H.; Zhou, W.; Hwang, S.J.; Kim, C.; Ottley, L.A.M.; Udovic, T.J. Probing the structure, stability and hydrogen storage properties of calcium dodecahydro-closo-dodecaborate. J. Solid State Chem. 2010, 183, 1133–1140. [Google Scholar] [CrossRef]
- Jørgensen, M.; Zhou, W.; Wu, H.; Udovic, T.J.; Jensen, T.R.; Paskevicius, M.; Černý, R. Polymorphism of CaB10H10 and Its Hydrates. 2020; in preparation. [Google Scholar]
- Her, J.H.; Wu, H.; Verdal, N.; Zhou, W.; Stavila, V.; Udovic, T.J. Structures of the strontium and barium dodecahydro-closo-dodecaborates. J. Alloys Compd. 2012, 514, 71–75. [Google Scholar] [CrossRef]
- Goedde, D.M.; Windler, G.K.; Girolami, G.S. Synthesis and Characterization of the Homoleptic Octahydrotriborate Complex Cr(B3H8)2 and Its Lewis Base Adducts. Inorg. Chem. 2007, 46, 2814–2823. [Google Scholar] [CrossRef] [PubMed]
- Didelot, E.; Łodziana, Z.; Murgia, F.; Černý, R. Ethanol—and methanol—Coordinated and solvent—free dodecahydro closo-dodecaborates of 3d transition metals and of magnesium. Crystals 2019, 9, 372. [Google Scholar] [CrossRef]
- Didelot, E.; Sadikin, Y.; Łodziana, Z.; Černý, R. Hydrated and anhydrous dodecahydro closo-dodecaborates of 3d transition metals and of magnesium. Solid State Sci. 2019, 90, 86–94. [Google Scholar] [CrossRef]
- Sadikin, Y.; Didelot, E.; Łodziana, Z.; Černý, R. Synthesis and crystal structure of solvent-free dodecahydro closo-dodecaborate of nickel, NiB12H12. Dalton Trans. 2018, 47, 5843–5849. [Google Scholar] [CrossRef]
- Paskevicius, M.; Hansen, B.R.S.; Jørgensen, M.; Richter, B.; Jensen, T.R. Multifunctionality of Silver closo-Boranes. Nat. Commun. 2017, 8, 15136. [Google Scholar] [CrossRef]
- Xie, Z.; Jelinek, T.; Bau, R.; Reed, C.A. New weakly coordinating anions. 3. Useful silver and trityl salt reagents of carborane anions. J. Am. Chem. Soc. 1994, 116, 1907–1913. [Google Scholar] [CrossRef]
- Xie, Z.; Wu, B.; Mak, T.C.W.; Reed, C.A. Structural Diversity in Silver Salts of Hexahalogenocarborane Anions, Ag(CB11H6X6) (X = Cl, Br or I). J. Chem. Soc. Dalton Trans. 1997, 640, 1213–1217. [Google Scholar] [CrossRef]
- Nguyen-Duc, V. New Salt-Like Dodecahydro-Closo-Dodecaborates and Efforts for the Partial Hydroxylation of [B12H12]2– Anions. Ph.D. Thesis, Universität Stuttgart Fakultät Chemie, Stuttgart, Germany, 2009. [Google Scholar]
- Tiritiris, I.; Van, N.D.; Schleid, T. Two Dodecahydro-closo-Dodecaborates with Lone-Pair Cations of the 6th Period in Comparison: Tl2[B12H12] and Pb(H2O)3[B12H12]·3H2O. Z. Anorg. Allg. Chem. 2011, 637, 682–688. [Google Scholar] [CrossRef]
- Kleeberg, F.M.; Zimmermann, L.W.; Schleid, T. Synthesis and Crystal Structure of anhydrous Pb2[B12H12]. Z. Anorg. Allg. Chem. 2014, 640, 2352. [Google Scholar]
- Tang, W.S.; Udovic, T.J.; Stavila, V. Altering the structural properties of A2B12H12 compounds via cation and anion modifications. J. Alloys Compd. 2015, 645, S200–S204. [Google Scholar] [CrossRef]
- He, L.Q.; Li, H.W.; Nakajima, H.; Tumanov, N.; Filinchuk, Y.; Hwang, S.J.; Sharma, M.; Hagemann, H.; Akiba, E. Synthesis of a Bimetallic Dodecaborate LiNaB12H12 with Outstanding Superionic Conductivity. Chem. Mater. 2015, 27, 5483–5486. [Google Scholar] [CrossRef]
- Tumanov, N.; He, L.; Sadikin, Y.; Brighi, M.; Li, H.W.; Akiba, E.; Černý, R.; Filinchuk, Y. Structural study of a bimetallic dodecaborate LiKB12H12 synthesized from decaborane B10H14. in preparation.
- Polyakova, I.N.; Malinina, E.A.; Kuznetsov, N.T. Crystal Structures of Cesium and Dimethylammonium Cupradecaborates, Cs[CuB10H10] and (CH3)2NH2[CuB10H10]. Crystallogr. Rep. 2003, 48, 84–91. [Google Scholar] [CrossRef]
- Malinina, E.A.; Zhizhin, K.Y.; Polyakova, I.N.; Lisovskii, M.V.; Kuznetsov, N.T. Silver(I) and Copper(I) Complexes with the closo-Decaborate Anion B10H10− as a Ligand. Russ. J. Inorg. Chem. 2002, 47, 1158–1167. [Google Scholar]
- Yisgedu, T.B.; Huang, Z.; Chen, X.; Lingam, H.K.; King, G.; Highley, A.; Maharrey, S.; Woodward, P.M.; Behrens, R.; Shore, S.G.; et al. The structural characterization of (NH4)2B10H10 and thermal decomposition studies of (NH4)2B10H10 and (NH4)2B12H12. Int. J. Hydrogen Energy 2012, 37, 4267–4273. [Google Scholar] [CrossRef]
- Huang, Z.; Chen, X.; Yisgedu, T.; Meyers, E.A.; Shore, S.G.; Zhao, J.C. Ammonium Octahydrotriborate (NH4B3H8): New Synthesis, Structure, and Hydrolytic Hydrogen Release. Inorg. Chem. 2011, 50, 3738–3742. [Google Scholar] [CrossRef]
- Derdziuk, J.; Malinowski, P.J.; Jaron, T. The synthesis, structural characterization and thermal decomposition studies of (N2H5)2B12H12 and its solvates. Int. J. Hydrogen Energy 2019, 44, 27030–27038. [Google Scholar] [CrossRef]
- Schlüter, F.; Bernhardt, E. Syntheses and Crystal Structures of the closo-Borates M2[B7H7] and M[B7H8] (M = PPh4, PNP, and N(n-Bu4)): The Missing Crystal Structure in the Series [BnHn]2- (n = 6–12). Inorg. Chem. 2011, 50, 2580–2589. [Google Scholar] [CrossRef]
- McGrath, T.D.; Welch, A.J. [Ph3PH][nido-B11H14]. Acta Crystallogr. Sect. C Struct. Chem. 1997, 53, 229–231. [Google Scholar] [CrossRef]
- Polyakova, I.N.; Malinina, E.A.; Drozdova, V.V.; Kuznetsov, N.T. The Isomorphous Substitution of 2H+ for the Cu2+ Cation in the Complex of Bis(aminoguanidine) copper (II): Crystal Structures of (Cu0.61H0.78Agu2)B12H12 and (HAgu)2B12H12. Crystallogr. Rep. 2009, 54, 831–836. [Google Scholar] [CrossRef]
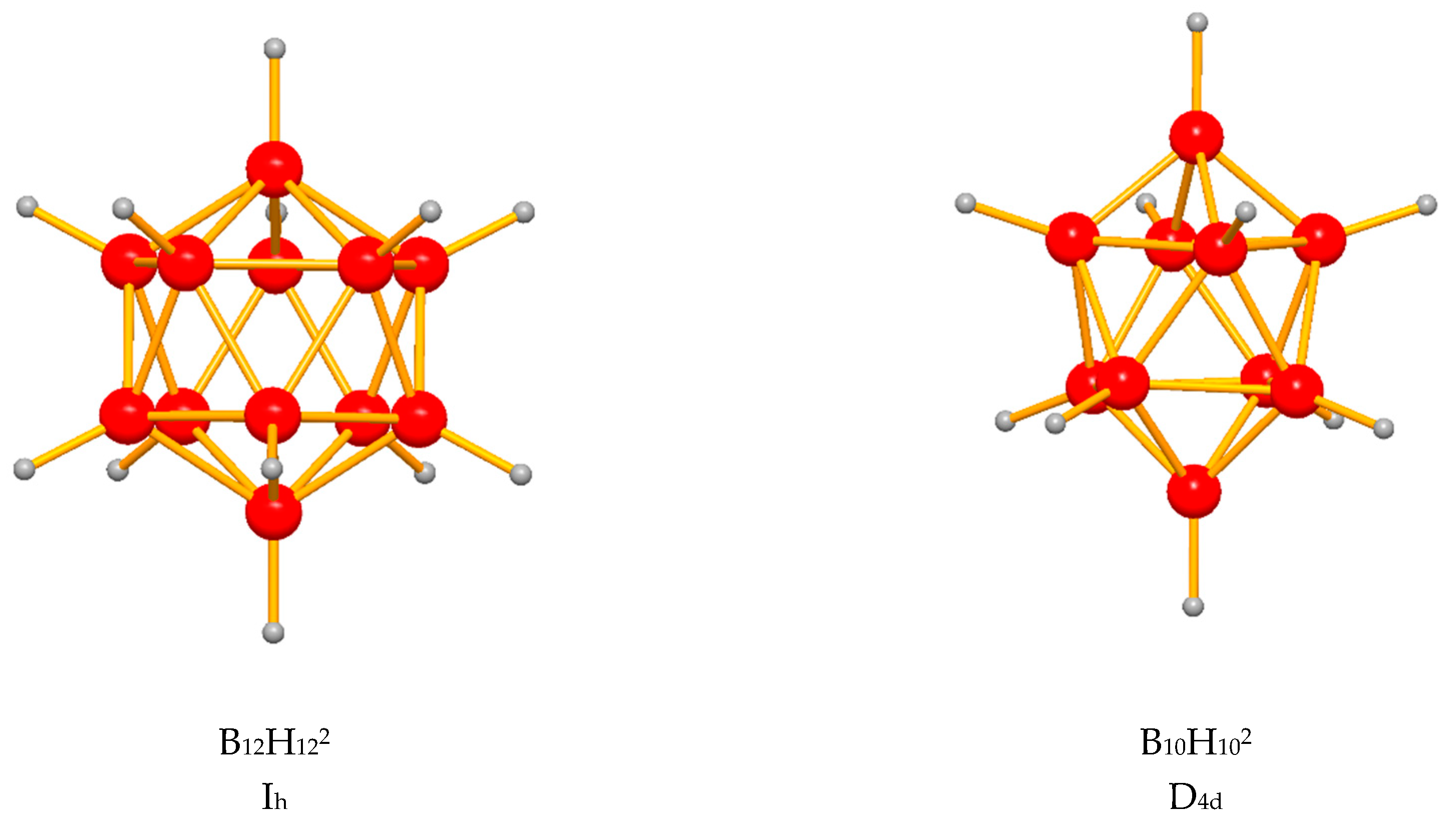
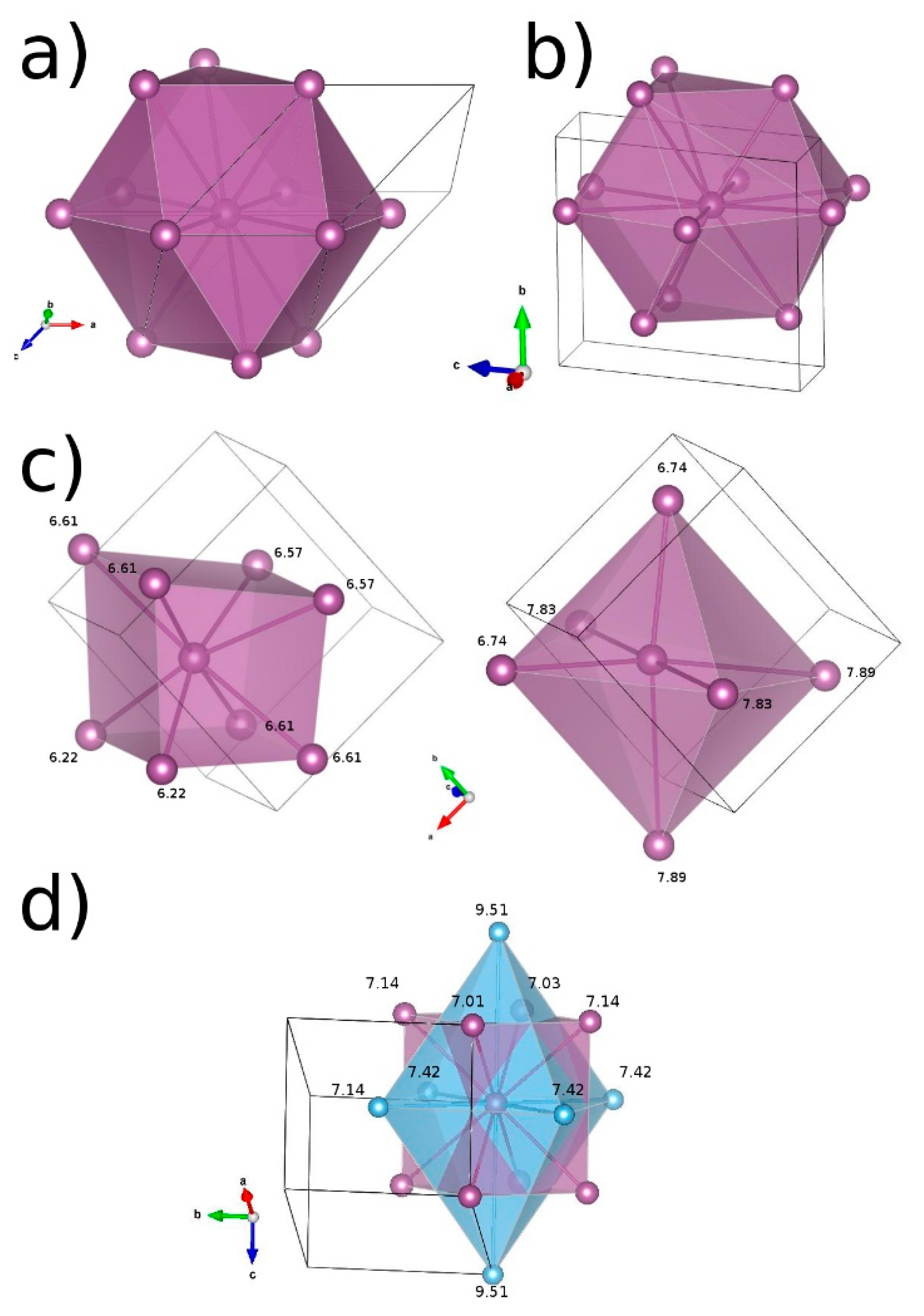

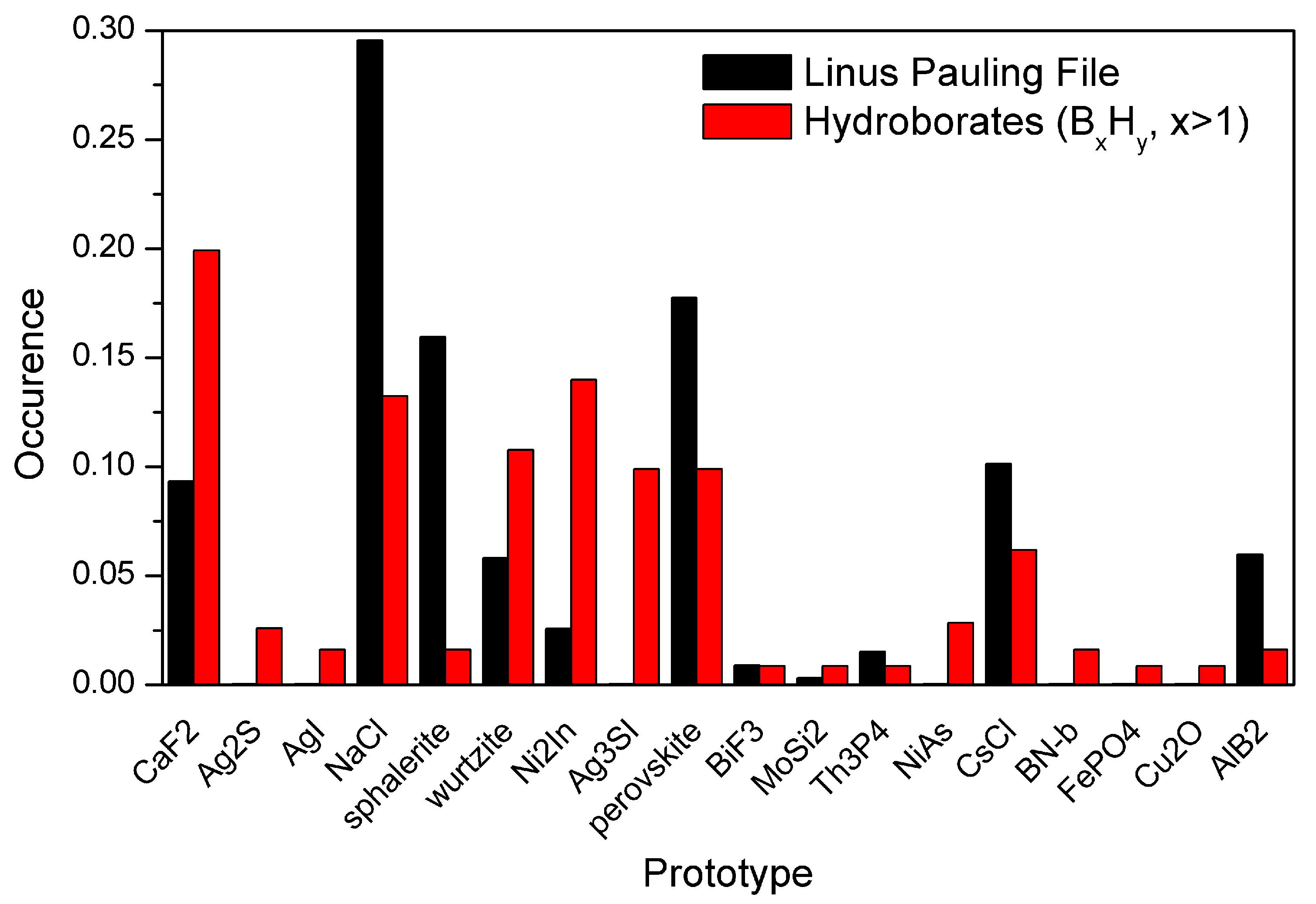
| Cation | Compound | Space Group | Structural Prototype | Cation Coordination by Anions | Cation Coordination by Hydrogen and Halogen | Anion Coordination | Aristotype of Anion Packing | Ref. |
|---|---|---|---|---|---|---|---|---|
| Li+closo- | rt-α-Li2B12H12 | Pa | anti-CaF2, cF12, 225 | Tri-bi | Oct | Oct | ccp | [62] |
| closo- | ht-β-Li2B12H12 | Fm | anti-CaF2, cF12, 225 | Tri | ccp | [63,64] | ||
| closo- | rt-Li2B10H10 | P6222 | anti-CaF2, cF12, 225 | Tri-bi | Oct | 6-fold | {336.446.59} 14-c net; tcc-x | [29] |
| closo- | ht-Li2B10H10 | [29] | ||||||
| closo- | rt-LiCB11H12 | Pca21 | NaCl, cF8, 225 | Tri-bi | Oct | Tri | ccp | [65] |
| closo- | ht-LiCB11H12 | Fmm | ccp | [65] | ||||
| closo- | rt-α-LiCB11H6Cl6 | Pnma | NaCl, cF8, 225 | Lin-tri | Oct | NLin | ccp | [66] |
| closo- | ht-γ-LiCB11H6Cl6 | P63/mmc | Lin, Tri | hcp | [66] | |||
| closo- | rt-α-LiCB11H6Br6 | Pnma | NaCl, cF8, 225 | Lin-tri | Oct | NLin | ccp | [66] |
| closo- | ht-γ-LiCB11H6Br6 | P63/mmc | Lin, Tri | hcp | [66] | |||
| closo- | rt-LiCB9H10 | [48] | ||||||
| closo- | ht1-LiCB9H10 | Cmc21 | wurtzite, hP4, 186 | hcp | [48] | |||
| closo- | ht2-LiCB9H10 | P31c | wurtzite, hP4, 186 | hcp | [48] | |||
| nido- | rt-LiB11H14 | Pbca | sphalerite, cF8, 216 | Tri-mon | Oct | NTri | ccp | [67] |
| nido- | ht-LiB11H14 | Fm | sphalerite, cF8, 216 | Tri | ccp | [67] | ||
| closo- | rt1-Li2(CB9H10) (CB11H12) | P31c | wurtzite, hP4, 186 | hcp | [68] | |||
| closo- | rt2-Li2(CB9H10) (CB11H12) | Fmm | ccp | [68] | ||||
| nido, closo- | Li(B11H14)x (CB11H12)1−x (x = 1/2, 1/3, 2/3) | Pca21 | NaCl, cF8, 225 | Tri | Tri | ccp | [67] | |
| nido, closo- | Li2-x(B11H14)x(B12H12)1−x (x = 1/2) | Pa | anti-CaF2, cF12, 225 | Tri | Tri | ccp | [67] | |
| nido, closo- | Li(B11H14)x (CB9H10)1−x (x = 1/2) | P31c | wurtzite, hP4, 186 | Tet | Tet | hcp | [67] | |
| Na+closo- | α-rt-Na2B12H12 | P21/n (P21/c) | anti-CaF2, cF12, 225 | Tet-mon, bi, tri | 10-fold | Cub | ccp | [21,30] |
| closo- | β-ht1-Na2B12H12 | Pmn | Ag2S, cI20, 229 | Tet | bcc | [21,30] | ||
| closo- | γ-ht2-Na2B12H12 | Imm | Ag2S, cI20, 229 | Tet | bcc | [21,30] | ||
| closo- | δ-ht3-Na2B12H12 | Fm | anti-CaF2, cF12, 225 | Tet | Cub | ccp | [21] | |
| closo- | ε-hp1-Na2B12H12 | Pbca | anti-CaF2, cF12, 225 | Tri-bi, tri | 7-fold | Oct | ccp | [69] |
| closo- | ζ-hp2-Na2B12H12 | Pnnm | anti-CaF2, cF12, 225 | Tet-mon, bi, tri | 9-fold | Cub | ccp | [69] |
| closo- | m-Na2B12H12−xIx | Pc | Ni2In (Co1.75Ge), hP6, 194 | Tet, Tri | 6-fold | hcp | [21] | |
| closo- | h-Na2B12H12−xIx | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Tet, Tri | Tet | hcp | [21] | |
| closo- | rt-Na2B10H10 | P21/c | anti-CaF2, cF12, 225 | Tet-bi, Tet-mon, bi, tri | 8-fold, 7-fold | Cub | ccp | [70] |
| closo- | ht-Na2B10H10 | Fmm | anti-CaF2, cF12, 225 | Tet, Oct | ccp | [70] | ||
| arachno- | NaB3H8 | Pmn21 | NaCl, cF8, 225 | Oct-mon, bi | 11-fold | Oct | ccp | [71] |
| closo- | rt-NaCB11H12 | Pca21 | NaCl, cF8, 225 | Tri-bi | Oct | Tri | ccp | [65] |
| closo- | ht-NaCB11H12 | Fmm | ccp | [65] | ||||
| closo- | rt-α-NaCB11H6Cl6 | Pbca | sphalerite, cF8, 216 | Tri-bi, tri | PentBiPyr | NTri | ccp | [66] |
| closo- | ht-γ-NaCB11H6Cl6 | P63/mmc | Lin, Tri | hcp | [66] | |||
| closo- | ht-γ-NaCB11H6Br6 | P63/mmc | Lin, Tri | hcp | [66] | |||
| closo- | lt-NaCB9H10 | P21/c | AgI, cI38, 229 | Tet-bi | 8-fold | Tet | bcc | [72] |
| closo- | rt-NaCB9H10 | Pna21 | AgI, cI38, 229 | Tet-bi | 8-fold | Tet | bcc | [72] |
| closo- | ht-NaCB9H10 | P31c | wurtzite, hP4, 186 | hcp | [48] | |||
| nido- | rt-NaB11H14 | Pna21 | wurtzite, hP4, 186 | Tet, Oct | Tet | hcp | [47,73] | |
| nido- | lt-NaB11H14 | Pna21 | wurtzite, hP4, 186 | Tet-bi, tri | 9-fold | Tet | hcp | [47] |
| nido- | ht-NaB11H14 | I3d | bcc | [73] | ||||
| nido- | rt-Na-7-CB10H13 | Pna21 | wurtzite, hP4, 186 | Tet-mon, bi, tri | SqAntPris | 4-fold | hcp | [73] |
| nido- | ht-Na-7-CB10H13 | Fmm | ccp | [73] | ||||
| nido- | rt-Na-7,8-C2B9H12 | P21 | [73] | |||||
| nido- | ht-Na-7,8-C2B9H12 | P31c | hcp | [73] | ||||
| nido- | rt-Na-7,9-C2B9H12 | Pna21 | wurtzite, hP4, 186 | Tet-mon, bi, tri | 1cap-SqAntPris | 4-fold | hcp | [73] |
| nido- | ht-Na-7,9-C2B9H12 | Fmm | ccp | [73] | ||||
| closo- | Na2(B12H12)0.75(B10H10)0.25 | bcc | [74] | |||||
| closo- | Na2(B12H12)0.50(B10H10)0.50 | Fmm | anti-CaF2, cF12, 225 | Tet, Oct | ccp | [75] | ||
| closo- | rt-Na2-x(CB11H12)x(B12H12)1−x (x = 1/2, 1/3, 2/3) | I23 | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [22] | ||
| closo- | ht1-Na2-x(CB11H12)x(B12H12)1−x (x = 1/3) | Pmn | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [22] | ||
| closo- | ht2-Na2-x(CB11H12)x(B12H12)1−x (x = 1/3) | Imm | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [22] | ||
| closo- | Na3(CB9H10) (B12H12) | P21c | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [18] | ||
| closo- | Na3(CB11H12) (B10H10) | Pnnm | anti-CaF2, cF12, 225 | Tet, Tri | ccp | [18] | ||
| closo- | rt1-Na2(CB9H10) (CB11H12) | P31c | wurtzite, hP4, 186 | Tri | hcp | [18,68] | ||
| closo- | rt2-Na2(CB9H10) (CB11H12) | Fmm | ccp | [68] | ||||
| nido, closo- | Na2-x(B11H14)x(B12H12)1−x (x = 1/2, 2/3) | Imm | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [47] | ||
| nido, closo- | rt-Na2-x(B11H14)x(B12H12)1−x (x = 1/3) | Pmn | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [47] | ||
| nido, closo- | ht-Na2-x(B11H14)x(B12H12)1−x (x = 1/3) | Imm | Ag3SI, (anti-perovskite) cP5, 221 | Tet | bcc | [47] | ||
| nido, closo- | Na3BH4B12H12 | Cmc21 | novel | Tet | CrB | [23] | ||
| K+closo- | K2B12H12 | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [76] |
| closo- | K2B10H10 | P21/c | Ni2In (Co1.75Ge), hP6, 194 | Tet-tri, Oct-mon, bi, tri, tetra | 12-fold, 14-fold | 2cap-SqAntPris | hcp | [77] |
| arachno- | α-KB3H8 | P21/m | NaCl, cF8, 225 | Oct-tri, tetra, penta | 24-fold | Oct | ccp | [78] |
| arachno- | α’-KB3H8 | Cmcm | NaCl, cF8, 225 | Oct-bi, tri, tetra | 18-fold | Oct | ccp | [78] |
| arachno- | β-KB3H8 | Fmm | NaCl, cF8, 225 | Oct | Oct | ccp | [78] | |
| closo- | K2B6H6 | Fmm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [79] |
| closo- | rt-KCB11H12 | P21/c | NaCl, cF8, 225 | Oct-mon, bi, tri | 12-fold, 10-fold | Oct | ccp | [80] |
| closo- | ht-KCB11H12 | Fmm | NaCl, cF8, 225 | Oct | Oct | ccp | [80] | |
| closo, closo- | KB21H18 | C2 | NiAs, hP4, 194 | TriAntPris-mon, tri | 14-fold | TriPris | hcp | [81] |
| nido, closo- | m-K3BH4B12H12 | P2/c | Ag3SI, (anti-perovskite) cP5, 221 | Oct-bi, tri | 14-fold | BH4-Oct, B12H12-Cuboct | bcc | [82] |
| nido, closo- | r-K3BH4B12H12 | Rm | Ag3SI, (anti-perovskite) cP5, 221 | Oct | BH4-Oct, B12H12-Cuboct | bcc | [82] | |
| nido, closo- | c-K3BH4B12H12 | P23 | Ag3SI, (anti-perovskite) cP5, 221 | Oct | BH4-Oct, B12H12-Cuboct | bcc | [82] | |
| Rb+closo- | Rb2B12H12 | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [83] |
| closo- | rt-Rb2B10H10 | P21/c | Ni2In (Co1.75Ge), hP6, 194 | Tet-bi, tri, tetra Oct-mon, bi, tri, tetra | 12-fold, 17-fold | 3cap-SqAntPris | hcp | [77,84] |
| closo- | lt-Rb2B10H10 | P | Ni2In (Co1.75Ge), hP6, 194 | Tet-bi, tri, tetra Oct-mon, bi, tri, tetra | 12-fold, 17-fold | 3cap-SqAntPris | hcp | [84] |
| closo- | Rb2B9H9 | P4/nmm | anti-BiF3, cF16, 225 | Tet-tri, SqPyr-bi, tri | Cuboct, 14-fold | 4cap-TriPris | ccp | [85] |
| closo, closo- | Rb2B20H18 | Pna21 | anti-MoSi2, tI6, 139 | SqPyr-mon, tri | 13-fold | 2cap-Cub | Mo in MoSi2 {348.466.56} 16-c net | [86] |
| nido, closo- | Rb3BH4B12H12 | P23 | Ag3SI, (anti-perovskite) cP5, 221 | Oct | BH4-Oct, B12H12-Cuboct | bcc | [87] | |
| Cs+closo- | rt-Cs2B12H12 | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [88] |
| closo- | ht-Cs2B12H12 | Fmm | anti-CaF2, cF12, 225 | Tet | Cub | ccp | [89] | |
| closo- | Cs2B6H6 | Fmm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [90] |
| arachno- | CsB3H8 | Ama2 | NaCl, cF8, 225 | Oct-bi, tri, tetra | 16-fold | Oct | ccp | [90] |
| closo- | Cs2B8H8 | Pnma | Ni2In (Co1.75Ge), hP6, 194 | Tet-tri, SqPyr-bi, tri | 12-fold | 1cap-SqAntPris | hcp | [91] |
| nido- | CsB9H14 | P | CsCl, cP2, 221 | Cub | Cub | aa | [92] | |
| closo, closo- | CsB21H18 | P21/c | NaCl, cF8, 225 | TriAntPris-mon, bi, tri | 15-fold | TriAntPris | ccp | [93] |
| closo- | rt-CsCB11H12 | R3 (R3/c above 323 K) | novel | Oct-bi, tri | 15-fold | 6-fold | hcp | [94] |
| closo- | ht-CsCB11H12 | I3d | Th3P4, cI28, 220 | Oct-bi, tri | 14-fold | 8-fold | hcp | [94] |
| closo- | CsCB11H6Cl6 | P2/c | CsCl, cP2, 221 | Cub-bi, tri | 6cap-Cub, 16-fold | Cub | aa | [95] |
| closo- | CsCB11H11Br1 | P21/n | NaCl, cF8, 225 | Oct-mon, bi, tri | 12-fold | Oct | ccp | [96] |
| closo- | CsCB11H6Br6 | P2/c | CsCl, cP2, 221 | Cub-bi, tri | 6cap-Cub, 16-fold | Cub | aa | [95] |
| closo- | CsCB9H5Br5 | P4/nmm | sphalerite, cF8, 216 | Tet-tri | Cuboct | Tet | ccp | [95] |
| nido- | α-CsC2B9H12 | I4 | AgI, cI38, 229 | Tet | SqPris | bcc | [97] | |
| nido- | β-CsC2B9H12 | P21/c | NiAs, hP4, 194—CsCl, cP2, 221 | 8-fold | 8-fold | hcp | [97] | |
| nido- | γ-CsC2B9H12 | P21/c | NiAs, hP4, 194 | Oct | TriPris | hcp | [97] | |
| closo, closo- | Cs(B21H18–xFx) (x = 2.55–2.85) | P21/n (P21/c) | NiAs, hP4, 194 | TriAntPris-bi, tri, tetra | 18-fold | TriPris | hcp | [93] |
| closo, closo- | Cs(B21H18–xFx) (x = 2.85–3) | P212121 | NiAs, hP4, 194 | TriAntPris-bi, tri, tetra | 18-fold | TriPris | hcp | [93] |
| nido, closo- | Cs3BH4B12H12 | P23 | Ag3SI, (anti-perovskite) cP5, 221 | Oct-bi | Cuboct | BH4-Oct, B12H12-Cuboct | bcc | [98] |
| Be2+arachno- | Be(B3H8)2 | P21/c | Lin-bi | Tet | ccp of Be(B3H8)2 molecules | [99] | ||
| arachno- nido- | Be(B5H10)BH4 | P21/c | Lin-bi | Tet | ccp of Be(B5H10)BH4 molecules | [100] | ||
| Ca2+closo- | CaB12H12 | C2/c | BN-b, hP4, 194 | TriBiPyr-mon, bi | 8-fold | TriBiPyr | hcp | [101] |
| closo- | α-CaB10H10 | Cc | wurtzite, hP4, 186 | Tet-mon, tri | 10-fold | Tet | hcp | [102] |
| closo- | β-CaB10H10 | Pbca | FePO4, oP48, 61 | Tet-bi, tri | 10-fold | Tet | hcp | [102] |
| Sr2+closo- | SrB12H12 | P31c | wurtzite, hP4, 186 | Tet-tri | Cuboct | Tet | hcp | [103] |
| Ba2+closo- | BaB12H12 | P31c | wurtzite, hP4, 186 | Tet-tri | Cuboct | Tet | hcp | [103] |
| Cr2+arachno- | Cr(B3H8)2 | P | Lin-bi | Sq | aa of Cr(B3H8)2 molecules | [104] | ||
| Mn2+closo- | MnB12H12 | P31c | BN-b, hP4, 194 | Lin-tri | Oct | Lin | hcp | [105] |
| Fe2+closo- | FeB12H12 | R | Lin-tri | Oct | Tri | bcc | [105] | |
| Co2+closo- | CoB12H12 | R | Lin-tri | Oct | Tri | bcc | [106] | |
| Ni2+closo- | NiB12H12 | R | Lin-tri | Oct | Tri | bcc | [106,107] | |
| Cu+closo- | Cu2B12H12 | Pn | Cu2O, cP6, 224 | Lin-bi | 4-fold | Tet | bcc | [106] |
| Ag+closo- | α-Ag2B12H12 | Pa | anti-CaF2, cF12, 225 | Tri-bi | TriAntPris | TriAntPris | ccp | [108] |
| closo- | β-Ag2B12H12 | Fmm | anti-CaF2, cF12, 225 | Tet | Cub | ccp | [108] | |
| closo- | α-Ag2B10H10 | P4/nnc | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [108] |
| closo- | β-Ag2B10H10 | Fmm | anti-CaF2, cF12, 225 | Tet | Cub | ccp | [108] | |
| closo- | AgCB9H10 | P21/m | NaCl, cF8, 225 | TriAntPris-mon, tri | 10-fold | TriPris | ccp | [109] |
| closo- | AgCB11H6Br6 | Pnma | NaCl, cF8, 225 | Lin-tri | Oct | NLin | ccp | [110] |
| Hg2+closo- | HgB12H12 | P | CsCl, cP2, 221 | Cub | Cub | ccp | [111] | |
| Tl+closo- | Tl2B10H10 | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [112] |
| Pb2+closo- | PbB12H12 | P31c | wurtzite, hP4, 186 | Tet-tri | Cuboct | Tet | hcp | [113] |
| Cation | Compound | Space Group | Structural Prototype | Cation Coordination by Anions | Cation Coordination by Hydrogen | Anion Coordination | Anion Packing | Ref. |
|---|---|---|---|---|---|---|---|---|
| Li+, Na+closo- | rt-(Lix,Na1−x)2B12H12 (x = 0.33–0.67) | Pa | anti-CaF2, cF12, 225 | Tri-bi | Oct | Oct | ccp | [114] |
| closo- | ht-(Lix,Na1−x)2B12H12 (x = 0.377) | Fmm | anti-CaF2, cF12, 225 | Tri | ccp | [115] | ||
| nido-, closo- | (Li0.7Na0.3)3BH4B12H12 | Pna21 | novel | Tet | FeB | [23] | ||
| Li+, K+closo- | rt-LiKB12H12 | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Li-Tri-bi, K-Oct-bi, tri | Li-TriPris K-15-fold | 3cap-TriPris | hcp | [116] |
| closo- | ht-(Lix,K1−x)2B12H12 (x = 0.8) | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Li-Tri, (K,Li)-Oct | 3cap-TriPris | hcp | [21] | |
| Li+, Cs+closo- | rt-LiCsB12H12 | Pn21a | Ni2In (Co1.75Ge), hP6, 194 | Li-Tri, Cs-Oct | 3cap-TriPris | hcp | [21] | |
| closo- | ht-LiCsB12H12 | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Li-Tri, Cs-Oct | 3cap-TriPris | hcp | [21] | |
| closo- | meta-(Lix,Cs1−x)2B12H12 (x = 0.11) | P63mc | Ni2In (Co1.75Ge), hP6, 194 | (Cs,Li)-Tri, Tet Cs-Oct | 10-fold | hcp | [21] | |
| Na+, K+closo- | rt-(Nax,K1−x)2B12H12 (x = 0.5) | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [21] |
| closo- | ht-(Nax,K1−x)2B12H12 (x = 0.5) | Pmn | Ag2S, cI20, 229 | Tet | bcc | [21] | ||
| closo- | rt-NaKB12H12 | P1 | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, K-Oct | 4cap-TriPris | hcp | [21] | |
| closo- | ht-NaKB12H12 | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, K-Oct | 4cap-TriPris | hcp | [21] | |
| Na+, Cs+closo- | rt-NaCsB12H12 | P | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, Cs-Oct | 4cap-TriPris | hcp | [21] | |
| closo- | ht1-NaCsB12H12 | P21/c | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, Cs-Oct | 4cap-TriPris | hcp | [21] | |
| closo- | ht2-NaCsB12H12 | Pn21a | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, Cs-Oct | 4cap-TriPris | hcp | [21] | |
| closo- | ht3-NaCsB12H12 | P63mc | Ni2In (Co1.75Ge), hP6, 194 | Na-Tet, Cs-Oct | 4cap-TriPris | hcp | [21] | |
| Cs+,Cu+closo- | Cs(CuB10H10) | Pbcn | novel | Cs- Oct-mon, bi, tri Cu-Lin-bi | Cs-15-fold Cu-Tet | 8-fold | ccp | [117] |
| Cs+,Ag+closo- | Cs(AgB10H10) | Pbcm | anti-BiF3, cF16, 225 | Cs-Oct-mon, bi, tri Ag-Tet-bi | Cs-15-fold Ag-8-fold | 2cap-SqAntPris | ccp | [118] |
| Cation | Compound | Space Group | Structural Prototype | Cation Coordination by Anions | Cation Coordination by Hydrogen | Anion Coordination | Anion Packing | Ref. |
|---|---|---|---|---|---|---|---|---|
| NH4+ closo- | (NH4)2B12H12 | Fm | anti-CaF2, cF12, 225 | Tet-tri | Cuboct | Cub | ccp | [83] |
| closo- | (NH4)2B10H10 | P21/c | Ni2In (Co1.75Ge), hP6, 194 | Tet, Oct | 8-fold | hcp | [119] | |
| arachno- | NH4B3H8 | Cmcm | NaCl, cF8, 225 | Oct-bi, tri, tetra | 18-fold | Oct | ccp | [120] |
| N2H5+ closo- | (N2H5)2B12H12 | C2/c | anti-AlB2, hP3, 191 | TriPris | HexPris | aa | [121] | |
| N(n-Bu4)+ closo- | N(n-Bu4)B8H9 | P41 | LiSe subnet in NH4LiSeO4, oP28, 33 | Tet | Sq | aa | [91] | |
| closo- | N(n-Bu4)2B7H7 | P21/n (P21/c) | anti-NiP subnet in Ba2Ni(PO4)2, mP52, 14 | Tri, Lin | TriBiPyr | hcp | [122] | |
| closo- | N(n-Bu4)B7H8 | P41 | LiSe subnet in NH4LiSeO4, oP28, 33 | Sq | Sq | aa | [122] | |
| Ph4P+ closo- | lt-(Ph4P)B8H9 | P2/c | CsCl, cP2, 221 | SqAntPris | Cub | aa | [91] | |
| closo- | rt-(Ph4P)B8H9 | P4/n | CsCl, cP2, 221 | SqAntPris | Cub | aa | [91] | |
| Ph3P+ nido- | (Ph3P)B11H14 | P21/m | BN-b, hP4, 194 | TriBiPyr | TriBiPyr | aa | [123] | |
| PNP+ closo- | (PNP)B7H8 | P21/c | novel | 9-fold | 9-fold | aa | [122] | |
| HAgu+ closo- | (HAgu)2B12H12 | P | anti-AlB2, hP3, 191 | TriPris | HexPris | aa | [124] | |
| HAgu+ Cu2+ closo- | (Cu0.61H0.78Agu2)B12H12 | P | CsCl, cP2, 221 | RhoPris | RhoPris | aa | [124] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Černý, R.; Brighi, M.; Murgia, F. The Crystal Chemistry of Inorganic Hydroborates. Chemistry 2020, 2, 805-826. https://doi.org/10.3390/chemistry2040053
Černý R, Brighi M, Murgia F. The Crystal Chemistry of Inorganic Hydroborates. Chemistry. 2020; 2(4):805-826. https://doi.org/10.3390/chemistry2040053
Chicago/Turabian StyleČerný, Radovan, Matteo Brighi, and Fabrizio Murgia. 2020. "The Crystal Chemistry of Inorganic Hydroborates" Chemistry 2, no. 4: 805-826. https://doi.org/10.3390/chemistry2040053
APA StyleČerný, R., Brighi, M., & Murgia, F. (2020). The Crystal Chemistry of Inorganic Hydroborates. Chemistry, 2(4), 805-826. https://doi.org/10.3390/chemistry2040053






