Casimir Interaction of Chern–Simons Layers on Substrates via Vacuum Stress Tensor
Abstract
1. Introduction
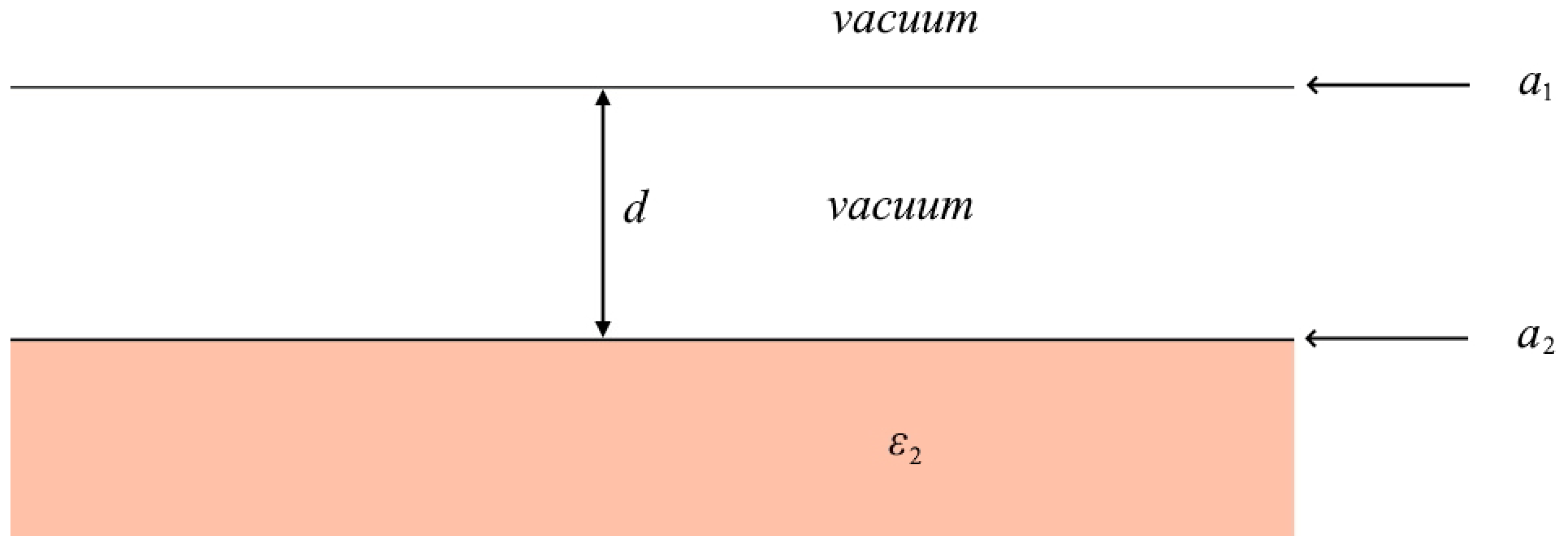
2. Casimir Pressure in the System of Two Dielectric Half-Spaces with Chern–Simons Boundary Layers
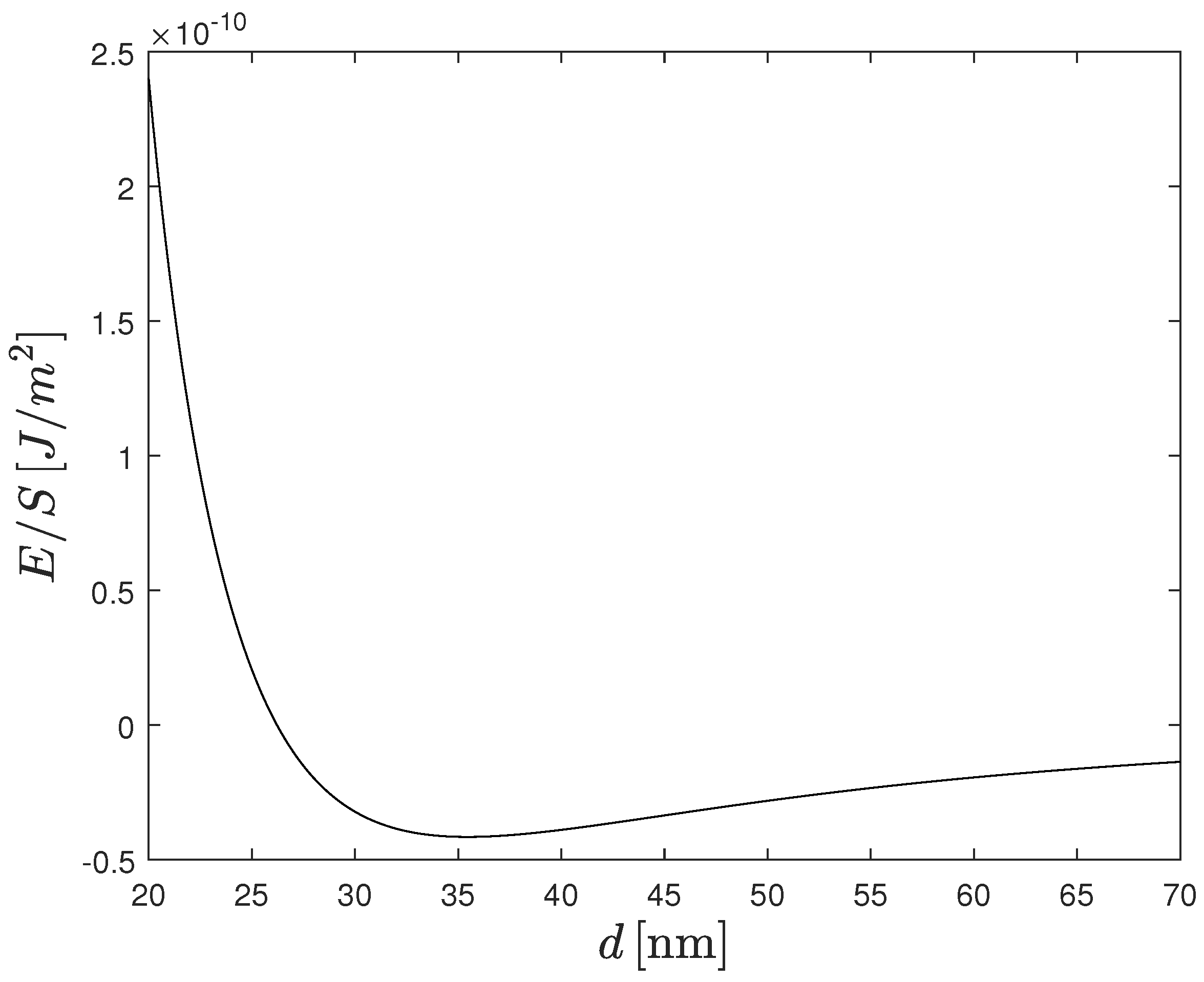
3. Casimir Interaction in Systems with Chern–Simons Layers on Realistic Substrates
4. Discussion and Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Representations of the Casimir Energy in Two Bases
References
- Casimir, H.B.G.; Polder, D. The influence of retardation on the London–van der Waals forces. Phys. Rev. 1948, 73, 360–372. [Google Scholar] [CrossRef]
- Casimir, H.B.G. On the attraction between two perfectly conducting plates. Proc. Kon. Ned. Akad. Wetensch. B 1948, 51, 793–795. Available online: https://dwc.knaw.nl/DL/publications/PU00018547.pdf (accessed on 1 February 2024).
- Marachevsky, V.N.; Sidelnikov, A.A. Green functions scattering in the Casimir effect. Universe 2021, 7, 195. [Google Scholar] [CrossRef]
- Lifshitz, E.M.; Pitaevskii, L.P. Statistical Physics. Part 2: Theory of Condensed States; Butterworh–Heinemann Ltd/Elsevier Ltd.: Oxford, UK, 1980. [Google Scholar] [CrossRef]
- Barash, Y.S.; Ginzburg, V.L. Electromagnetic fluctuations in matter and molecular (Van-der-Waals) forces between them. Sov. Phys. Usp. 1975, 18, 305–322. [Google Scholar] [CrossRef]
- Barash, Y.S.; Ginzburg, V.L. Some problems in the theory of van der Waals forces. Sov. Phys. Usp. 1984, 27, 467–491. [Google Scholar] [CrossRef]
- Plunien, G.; Müller, B.; Greiner, W. The Casimir effect. Phys. Rep. 1986, 134, 87–193. [Google Scholar] [CrossRef]
- Bordag, M.; Mohideen, U.; Mostepanenko, V.M. New developments in the Casimir effect. Phys. Rep. 2001, 353, 1–205. [Google Scholar] [CrossRef]
- Santangelo, E.M. Evaluation of Casimir energies through spectral functions. Theor. Math. Phys. 2002, 131, 527–542. [Google Scholar] [CrossRef]
- Milton, K.A. The Casimir effect: Recent controversies and progress. J. Phys. A Math. Gen. 2004, 37, R209–R277. [Google Scholar] [CrossRef]
- Jaffe, R.L. Casimir effect and the quantum vacuum. Phys. Rev. D 2005, 72, 021301(R). [Google Scholar] [CrossRef]
- Scheel, S.; Buhmann, S.Y. Macroscopic quantum electrodynamics—Concepts and applications. Acta Phys. Slovaca 2008, 58, 675–809. Available online: http://www.physics.sk/aps/pub.php?y=2008&pub=aps-08-05 (accessed on 1 February 2024). [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827–1885. [Google Scholar] [CrossRef]
- Rodriguez, A.; Capasso, F.; Johnson, S. The Casimir effect in microstructured geometries. Nat. Photon. 2011, 5, 211–221. [Google Scholar] [CrossRef]
- Marachevsky, V.N. The Casimir effect: Medium and geometry. J. Phys. A Math. Theor. 2012, 45, 374021. [Google Scholar] [CrossRef]
- Woods, L.M.; Dalvit, D.A.R.; Tkatchenko, A.; Rodriguez-Lopez, P.; Rodriguez, A.W.; Podgornik, R. Materials perspective on Casimir and van der Waals interactions. Rev. Mod. Phys. 2016, 88, 045003. [Google Scholar] [CrossRef]
- Woods, L.M.; Krüger, M.; Dodonov, V.V. Perspective on some recent and future developments in Casimir interactions. Appl. Sci. 2021, 11, 293. [Google Scholar] [CrossRef]
- Lu, B.-S. The Casimir effect in topological matter. Universe 2021, 7, 237. [Google Scholar] [CrossRef]
- Elizalde, E. Ten Physical Applications of Spectral Zeta Functions; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Kirsten, K. Spectral Functions in Mathematics and Physics; Chapman & Hall/CRC Press/Taylor & Francis Group: Boca Raton, FL, USA, 2002. [Google Scholar] [CrossRef]
- Fursaev, D.; Vassilevich, D. Operators, Geometry and Quanta: Methods of Spectral Geometry in Quantum Field Theory; Springer Science+Business Media B.V.: Dordrecht, The Netherlands, 2011. [Google Scholar]
- Buhmann, S.Y. Dispersion Forces I. Macroscopic Quantum Electrodynamics and Ground-State Casimir, Casimir–Polder and van der Waals Forces; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Buhmann, S.Y. Dispersion Forces II. Many-Body Effects, Excited Atoms, Finite Temperature and Quantum Friction; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in the Casimir Effect; Oxford University Press: Oxford, UK, 2015. [Google Scholar] [CrossRef]
- Lifshitz, E.M. The theory of molecular attractive forces between solids. Zh. Eksp. Teor. Fiz. 1955, 29, 94–110. (In Russian) English translation: Sov. Phys. JETP 1956, 2, 73–83. Available online: http://jetp.ras.ru/cgi-bin/e/index/e/2/1/p73?a=list (accessed on 1 February 2024).
- Renne, M.J. Microscopic theory of retarded van der Waals forces between macroscopic dielectric bodies. Physica 1971, 56, 125–137. [Google Scholar] [CrossRef]
- Dzyaloshinskii, I.E.; Lifshitz, E.M.; Pitaevskii, L.P. General theory of van der Waals’ forces. Sov. Phys. Usp. 1961, 4, 153–176. [Google Scholar] [CrossRef]
- Munday, J.N.; Capasso, F.; Parsegian, V.A. Measured long-range repulsive Casimir–Lifshitz forces. Nature 2009, 457, 170–173. [Google Scholar] [CrossRef] [PubMed]
- Pirozhenko, I.; Lambrecht, A. Repulsive Casimir forces and the role of surface modes. Phys. Rev. A 2009, 80, 042510. [Google Scholar] [CrossRef]
- Schmidt, F.; Callegari, A.; Daddi-Moussa-Ider, A.; Munkhbat, B.; Verre, R.; Shegai, T.; Käll, M.; Löwen, H.; Gambassi, A.; Volpe, G. Tunable critical Casimir forces counteract Casimir–Lifshitz attraction. Nat. Phys. 2023, 19, 271–278. [Google Scholar] [CrossRef]
- Tomaš, M.S. Casimir force between dispersive magnetodielectrics. Phys. Lett. A 2005, 342, 381–388. [Google Scholar] [CrossRef][Green Version]
- Geyer, B.; Klimchitskaya, G.L.; Mostepanenko, V.M. Thermal Casimir interaction between two magnetodielectric plates. Phys. Rev. B 2010, 81, 104101. [Google Scholar] [CrossRef]
- Inui, N. Thickness dependence of the Casimir force between a magnetodielectric plate and a diamagnetic plate. Phys. Rev. A 2011, 84, 052505. [Google Scholar] [CrossRef]
- Brevik, I.; Parashar, P.; Shajesh, K.V. Casimir force for magnetodielectric media. Phys. Rev. A 2018, 98, 032509. [Google Scholar] [CrossRef]
- Shelden, C.; Spreng, B.; Munday, J.N. Enhanced repulsive Casimir forces between gold and thin magnetodielectric plates. Phys. Rev. A 2023, 108, 032817. [Google Scholar] [CrossRef]
- Boyer, T.H. Van der Waals forces and zero-point energy for dielectric and permeable materials. Phys. Rev. A 1974, 9, 2078–2084. [Google Scholar] [CrossRef]
- Rosa, F.S.S.; Dalvit, D.A.R.; Milonni, P.W. Casimir–Lifshitz theory and metamaterials. Phys. Rev. Lett. 2008, 100, 183602. [Google Scholar] [CrossRef]
- Rosa, F.S.S.; Dalvit, D.A.R.; Milonni, P.W. Casimir interactions for anisotropic magnetodielectric metamaterials. Phys. Rev. A 2008, 78, 032117. [Google Scholar] [CrossRef]
- Yannopapas, V.; Vitanov, N.V. First-principles study of Casimir repulsion in metamaterials. Phys. Rev. Lett. 2009, 103, 120401. [Google Scholar] [CrossRef] [PubMed]
- Zhao, R.; Zhou, J.; Koschny, T.; Economou, E.N.; Soukoulis, C.M. Repulsive Casimir force in chiral metamaterials. Phys. Rev. Lett. 2009, 103, 103602. [Google Scholar] [CrossRef] [PubMed]
- Markov, V.N.; Pis’mak, Y.M. Casimir effect for thin films in QED. J. Phys. A Math. Gen. 2006, 39, 6525–6532. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Casimir effect for Chern–Simons layers in the vacuum. Theor. Math. Phys. 2017, 190, 315–320. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Casimir interaction of two dielectric half spaces with Chern–Simons boundary layers. Phys. Rev. B 2019, 99, 075420. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Chern–Simons boundary layers in the Casimir effect. Mod. Phys. Lett. A 2020, 35, 2040015. [Google Scholar] [CrossRef]
- Pavlovsky, O.; Ulybyshev, M. Casimir energy calculations within the formalism of noncompact lattice QED. Int. J. Mod. Phys. A 2010, 25, 2457–2473. [Google Scholar] [CrossRef]
- Pavlovsky, O.V.; Ulybyshev, M.V. Casimir energy in noncompact lattice electrodynamics. Theor. Math. Phys. 2010, 164, 1051–1063. [Google Scholar] [CrossRef]
- Milton, K.A.; Ng, Y.J. Maxwell–Chern–Simons Casimir effect. Phys. Rev. D 1990, 42, 2875–2880. [Google Scholar] [CrossRef]
- Elizalde, E.; Vassilevich, D.V. Heat kernel coefficients for Chern–Simons boundary conditions in QED. Class. Quantum Gravity 1999, 16, 813–822. [Google Scholar] [CrossRef][Green Version]
- Bordag, M.; Vassilevich, D.V. Casimir force between Chern–Simons surfaces. Phys. Lett. A 2000, 268, 75–80. [Google Scholar] [CrossRef][Green Version]
- Qi, X.-L.; Li, R.; Zang, J.; Zhang, S.-C. Inducing a magnetic monopole with topological surface states. Science 2009, 323, 1184–1187. [Google Scholar] [CrossRef] [PubMed]
- Grushin, A.G.; Cortijo, A. Tunable Casimir repulsion with three-dimensional topological insulators. Phys. Rev. Lett. 2011, 106, 020403. [Google Scholar] [CrossRef] [PubMed]
- Grushin, A.G.; Rodriguez-Lopez, P.; Cortijo, A. Effect of finite temperature and uniaxial anisotropy on the Casimir effect with three-dimensional topological insulators. Phys. Rev. B 2011, 84, 045119. [Google Scholar] [CrossRef]
- Chen, L.; Wan, S. Casimir interaction between topological insulators with finite surface band gap. Phys. Rev. B 2011, 84, 075149. [Google Scholar] [CrossRef]
- Chen, L.; Wan, S. Critical surface band gap of repulsive Casimir interaction between three-dimensional topological insulators at finite temperature. Phys. Rev. B 2012, 85, 115102. [Google Scholar] [CrossRef]
- Martín-Ruiz, A.; Cambiaso, M.; Urrutia, L.F. A Green’s function approach to the Casimir effect on topological insulators with planar symmetry. Europhys. Lett. 2016, 113, 60005. [Google Scholar] [CrossRef]
- Fialkovsky, I.; Khusnutdinov, N.; Vassilevich, D. Quest for Casimir repulsion between Chern–Simons surfaces. Phys. Rev. B 2018, 97, 165432. [Google Scholar] [CrossRef]
- Weng, H.; Yu, R.; Hu, X.; Dai, X.; Fang, Z. Quantum anomalous Hall effect and related topological electronic states. Adv. Phys. 2015, 64, 227–282. [Google Scholar] [CrossRef]
- Liu, C.-X.; Zhang, S.-C.; Qi, X.-L. The quantum anomalous Hall effect: Theory and experiment. Annu. Rev. Cond. Matter. Phys. 2016, 7, 301–321. [Google Scholar] [CrossRef]
- Ren, Y.; Qiao, Z.; Niu, Q. Topological phases in two-dimensional materials: A review. Rep. Prog. Phys. 2016, 79, 066501. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez-Lopez, P.; Grushin, A.G. Repulsive Casimir effect with Chern insulators. Phys. Rev. Lett. 2014, 112, 056804. [Google Scholar] [CrossRef] [PubMed]
- Muniz, Y.; Farina, C.; Kort-Kamp, W.J.M. Casimir forces in the flatland: Interplay between photoinduced phase transitions and quantum Hall physics. Phys. Rev. Res. 2021, 3, 023061. [Google Scholar] [CrossRef]
- Tse, W.-K.; MacDonald, A.H. Quantized Casimir force. Phys. Rev. Lett. 2012, 109, 236806. [Google Scholar] [CrossRef] [PubMed]
- Ezawa, Z.F. Quantum Hall Effects: Field Theoretical Approach and Related Topics; World Scientific: Singapore, 2008. [Google Scholar] [CrossRef]
- Marachevsky, V.N.; Sidelnikov, A.A. Gauge-invariant derivation of the Casimir–Lifshitz pressure. Phys. Part. Nucl. Lett. 2023, 20, 1114–1116. [Google Scholar] [CrossRef]
- Barton, G. Quantum-electrodynamic level shifts between parallel mirrors: Analysis. Proc. R. Soc. Lond. A 1987, 410, 141–174. [Google Scholar]
- Brevik, I.; Lygren, M.; Marachevsky, V.N. Casimir–Polder effect for a perfectly conducting wedge. Ann. Phys. 1998, 267, 134–142. [Google Scholar] [CrossRef]
- Messina, R.; Dalvit, D.A.R.; Maia Neto, P.A.; Lambrecht, A.; Reynaud, S. Dispersive interactions between atoms and nonplanar surfaces. Phys. Rev. A 2009, 80, 022119. [Google Scholar] [CrossRef]
- Bender, H.; Stehle, C.; Zimmermann, C.; Slama, S.; Fiedler, J.; Scheel, S.; Buhmann, S.Y.; Marachevsky, V.N. Probing atom-surface interactions by diffraction of Bose-Einstein condensates. Phys. Rev. X 2014, 4, 011029. [Google Scholar] [CrossRef]
- Levin, M.; McCauley, A.P.; Rodriguez, A.W.; Homer Reid, M.T.; Johnson, S.G. Casimir repulsion between metallic objects in vacuum. Phys. Rev. Lett. 2010, 105, 090403. [Google Scholar] [CrossRef] [PubMed]
- Eberlein, C.; Zietal, R. Casimir–Polder interaction between a polarizable particle and a plate with a hole. Phys. Rev. A 2011, 83, 052514. [Google Scholar] [CrossRef]
- Buhmann, S.Y.; Marachevsky, V.N.; Scheel, S. Impact of anisotropy on the interaction of an atom with a one-dimensional nano-grating. Int. J. Mod. Phys. A 2016, 31, 1641029. [Google Scholar] [CrossRef]
- Milton, K.A.; Abalo, E.K.; Parashar, P.; Pourtolami, N.; Brevik, I.; Ellingsen, S.A. Casimir–Polder repulsion near edges: Wedge apex and a screen with an aperture. Phys. Rev. A 2011, 83, 062507. [Google Scholar] [CrossRef]
- Milton, K.A.; Parashar, P.; Pourtolami, N.; Brevik, I. Casimir–Polder repulsion: Polarizable atoms, cylinders, spheres, and ellipsoids. Phys. Rev. D 2012, 85, 025008. [Google Scholar] [CrossRef]
- Milton, K.A.; Abalo, E.K.; Parashar, P.; Pourtolami, N.; Brevik, I.; Ellingsen, S.A. Repulsive Casimir and Casimir–Polder forces. J. Phys. A Math. Theor. 2012, 45, 374006. [Google Scholar] [CrossRef]
- Shajesh, K.V.; Schaden, M. Repulsive long-range forces between anisotropic atoms and dielectrics. Phys. Rev. A 2012, 85, 012523. [Google Scholar] [CrossRef]
- Marchetta, J.J.; Parashar, P.; Shajesh, K.V. Geometrical dependence in Casimir–Polder repulsion. Phys. Rev. A 2021, 104, 032209. [Google Scholar] [CrossRef]
- Savin, V.P.; Koksharov, Y.A. Electrostatic repulsion between an uncharged or slightly charged conductor and a point charge. J. Electrost. 2022, 120, 103769. [Google Scholar] [CrossRef]
- Nogueira, E.C.M.; Queiroz, L.; Alves, D.T. Sign inversion in the lateral van der Waals force. Phys. Rev. A 2022, 105, 062816. [Google Scholar] [CrossRef]
- Queiroz, L.; Nogueira, E.C.M.; Alves, D.T. Sign inversion in the lateral van der Waals force between an anisotropic particle and a plane with a hemispherical protuberance: An exact calculation. J. Phys. A Math. Theor. 2023, 56, 115301. [Google Scholar] [CrossRef]
- Alves, D.T.; Queiroz, L.; Nogueira, E.C.M.; Peres, N.M.R. Curvature-induced repulsive effect on the lateral Casimir–Polder–van der Waals force. Phys. Rev. A 2023, 107, 062821. [Google Scholar] [CrossRef]
- Venkataram, P.S.; Molesky, S.; Chao, P.; Rodriguez, A.W. Fundamental limits to attractive and repulsive Casimir–Polder forces. Phys. Rev. A 2020, 101, 052115. [Google Scholar] [CrossRef]
- Marachevsky, V.N.; Pis’mak, Y.M. Casimir–Polder effect for a plane with Chern–Simons interaction. Phys. Rev. D 2010, 81, 065005. [Google Scholar] [CrossRef]
- Jiang, Q.-D.; Wilczek, F. Chiral Casimir forces: Repulsive, enhanced, tunable. Phys. Rev. B 2019, 99, 125403. [Google Scholar] [CrossRef]
- Høye, J.S.; Brevik, I. Casimir force between ideal metal plates in a chiral vacuum. Eur. Phys. J. Plus 2020, 135, 271. [Google Scholar] [CrossRef]
- Butcher, D.T.; Buhmann, S.Y.; Scheel, S. Casimir–Polder forces between chiral objects. New J. Phys. 2012, 14, 113013. [Google Scholar] [CrossRef]
- Khriplovich, I.B. Parity Nonconservation in Atomic Phenomena; Gordon and Breach Science Publishers S.A: Philadelphia, PA, USA, 1991. [Google Scholar]
- Buhmann, S.Y.; Marachevsky, V.N.; Scheel, S. Charge-parity-violating effects in Casimir–Polder potentials. Phys. Rev. A 2018, 98, 022510. [Google Scholar] [CrossRef]
- Marachevsky, V.N.; Sidelnikov, A.A. Casimir–Polder interaction with Chern–Simons boundary layers. Phys. Rev. D 2023, 107, 105019. [Google Scholar] [CrossRef]
- Brown, L.S.; Maclay, G.J. Vacuum stress between conducting plates: An image solution. Phys. Rev. 1969, 184, 1272–1279. [Google Scholar] [CrossRef]
- Weyl, H. Ausbreitung elektromagnetischer Wellen uber einen Leiter. Ann. Der Phys. 1919, 365, 481–500. [Google Scholar] [CrossRef]
- Obukhov, Y.N.; Hehl, F.W. Measuring a piecewise constant axion field in classical electrodynamics. Phys. Lett. A 2005, 341, 357–365. [Google Scholar] [CrossRef]
- Pis’mak, D.Y.; Pis’mak, Y.M.; Wegner, F.J. Electromagnetic waves in a model with Chern–Simons potential. Phys. Rev. E 2015, 92, 013204. [Google Scholar] [CrossRef] [PubMed]
- Lambrecht, A.; Marachevsky, V.N. Casimir interaction of dielectric gratings. Phys. Rev. Lett. 2008, 101, 160403. [Google Scholar] [CrossRef] [PubMed]
- Lambrecht, A.; Marachevsky, V.N. Theory of the Casimir effect in one-dimensional periodic dielectric systems. Int. J. Mod. Phys. A 2009, 24, 1789–1795. [Google Scholar] [CrossRef]
- Antezza, M.; Chan, H.B.; Guizal, B.; Marachevsky, V.N.; Messina, R.; Wang, M. Giant Casimir torque between rotated gratings and the θ = 0 anomaly. Phys. Rev. Lett. 2020, 124, 013903. [Google Scholar] [CrossRef]
- Marachevsky, V.N. The Casimir effect for diffraction gratings, symmetry breaking and geometric transitions. Phys. Part. Nucl. Lett. 2023, 20, 255–258. [Google Scholar] [CrossRef]
- Emig, T.; Graham, N.; Jaffe, R.L.; Kardar, M. Casimir forces between arbitrary compact objects. Phys. Rev. Lett. 2007, 99, 170403. [Google Scholar] [CrossRef]
- Rahi, S.J.; Emig, T.; Graham, N.; Jaffe, R.L.; Kardar, M. Scattering theory approach to electromagnetic Casimir forces. Phys. Rev. D 2009, 80, 085021. [Google Scholar] [CrossRef]
- Emig, T.; Jaffe, R.L.; Kardar, M.; Scardicchio, A. Casimir interaction between a plate and a cylinder. Phys. Rev. Lett. 2006, 96, 080403. [Google Scholar] [CrossRef]
- Canaguier-Durand, A.; Maia Neto, P.A.; Cavero-Pelaez, I.; Lambrecht, A.; Reynaud, S. Casimir interaction between plane and spherical metallic surfaces. Phys. Rev. Lett. 2009, 102, 230404. [Google Scholar] [CrossRef] [PubMed]
- Canaguier-Durand, A.; Gérardin, A.; Guérout, R.; Maia Neto, P.A.; Nesvizhevsky, V.V.; Voronin, A.Y.; Lambrecht, A.; Reynaud, S. Casimir interaction between a dielectric nanosphere and a metallic plane. Phys. Rev. A 2011, 83, 032508. [Google Scholar] [CrossRef]
- Bordag, M.; Nikolaev, V. Casimir force for a sphere in front of a plane beyond proximity force approximation. J. Phys. A Math. Theor. 2008, 41, 164002. [Google Scholar] [CrossRef]
- Bordag, M.; Pirozhenko, I. Vacuum energy between a sphere and a plane at finite temperature. Phys. Rev. D 2010, 81, 085023. [Google Scholar] [CrossRef]
- Bordag, M.; Pirozhenko, I.G. On the Casimir entropy for a ball in front of a plane. Phys. Rev. D 2010, 82, 125016. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Rahi, S.J.; Emig, T. Three-body Casimir effects and nonmonotonic forces. Phys. Rev. A 2009, 80, 022519. [Google Scholar] [CrossRef]
- Graham, N.; Shpunt, A.; Emig, T.; Rahi, S.J.; Jaffe, R.L.; Kardar, M. Electromagnetic Casimir forces of parabolic cylinder and knife-edge geometries. Phys. Rev. D 2011, 83, 125007. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Emig, T.; Noruzifar, E.; Zandi, R. Effect of curvature and confinement on the Casimir–Polder interaction. Phys. Rev. A 2015, 91, 012516. [Google Scholar] [CrossRef]
- Fialkovsky, I.V.; Marachevsky, V.N.; Vassilevich, D.V. Finite-temperature Casimir effect for graphene. Phys. Rev. B 2011, 84, 035446. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir and Casimir–Polder forces in graphene systems: Quantum field theoretical description and thermodynamics. Universe 2020, 6, 150. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Casimir–Polder effect for a stack of conductive planes. Phys. Rev. A 2016, 94, 012513. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Thermal Casimir and Casimir–Polder interactions in N parallel 2D Dirac materials. 2D Mater. 2018, 5, 035032. [Google Scholar] [CrossRef]
- Antezza, M.; Fialkovsky, I.; Khusnutdinov, N. Casimir–Polder force and torque for anisotropic molecules close to conducting planes and their effect on CO2. Phys. Rev. B 2020, 102, 195422. [Google Scholar] [CrossRef]
- Liu, M.; Zhang, Y.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Demonstration of an unusual thermal effect in the Casimir force from graphene. Phys. Rev. Lett. 2021, 126, 206802. [Google Scholar] [CrossRef]
- Lambrecht, A.; Pirozhenko, I.; Duraffourg, L.; Andreucci, P. The Casimir effect for silicon and gold slabs. Europhys. Lett. 2007, 77, 44006. [Google Scholar] [CrossRef]
- Palik, E.D. (Ed.) Handbook of Optical Constants of Solids; Academic Press/Elsevier Inc.: Cambridge, MA, USA, 1997; Available online: https://www.sciencedirect.com/book/9780125444156/handbook-of-optical-constants-of-solids (accessed on 1 February 2024).


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marachevsky, V.N.; Sidelnikov, A.A. Casimir Interaction of Chern–Simons Layers on Substrates via Vacuum Stress Tensor. Physics 2024, 6, 496-514. https://doi.org/10.3390/physics6020033
Marachevsky VN, Sidelnikov AA. Casimir Interaction of Chern–Simons Layers on Substrates via Vacuum Stress Tensor. Physics. 2024; 6(2):496-514. https://doi.org/10.3390/physics6020033
Chicago/Turabian StyleMarachevsky, Valery N., and Arseny A. Sidelnikov. 2024. "Casimir Interaction of Chern–Simons Layers on Substrates via Vacuum Stress Tensor" Physics 6, no. 2: 496-514. https://doi.org/10.3390/physics6020033
APA StyleMarachevsky, V. N., & Sidelnikov, A. A. (2024). Casimir Interaction of Chern–Simons Layers on Substrates via Vacuum Stress Tensor. Physics, 6(2), 496-514. https://doi.org/10.3390/physics6020033




