Alternative Origin of Galactic Positrons Generated by Ultraperipheral Collisions of Cosmic Rays
Abstract
1. Introduction
2. Ultraperipheral Electron–Positron Pairs from Collisions
2.1. General Review
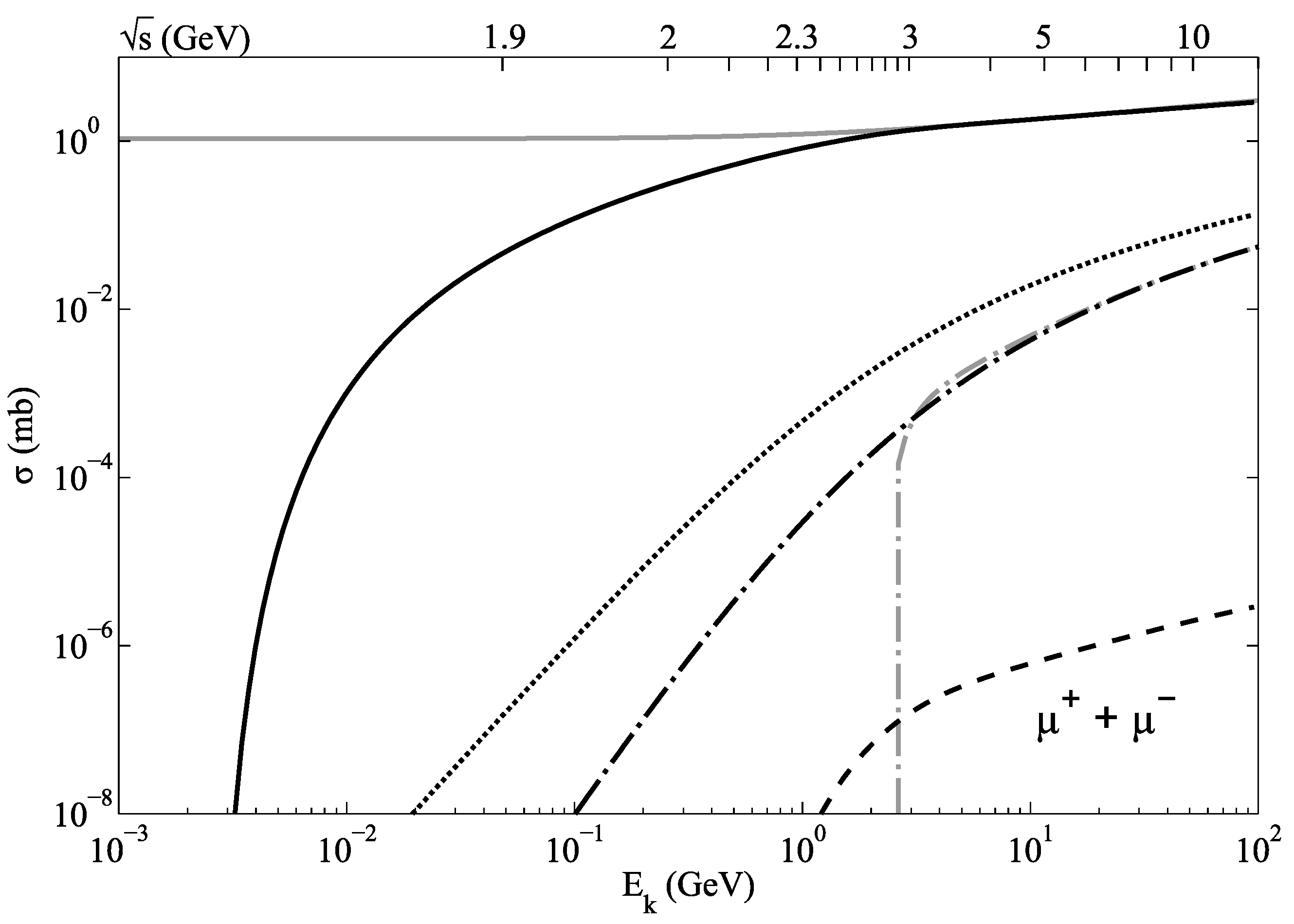
2.2. The Born Approximation
2.3. The Equivalent Photon Approximation
3. Positron Production in the Galaxy
- What power sources of fast particles need to be supplied in order to produce sufficient amounts of positrons?
- How does injecting this many fast particles affect the background gas?
- What spectrum of byproduct emission is expected?
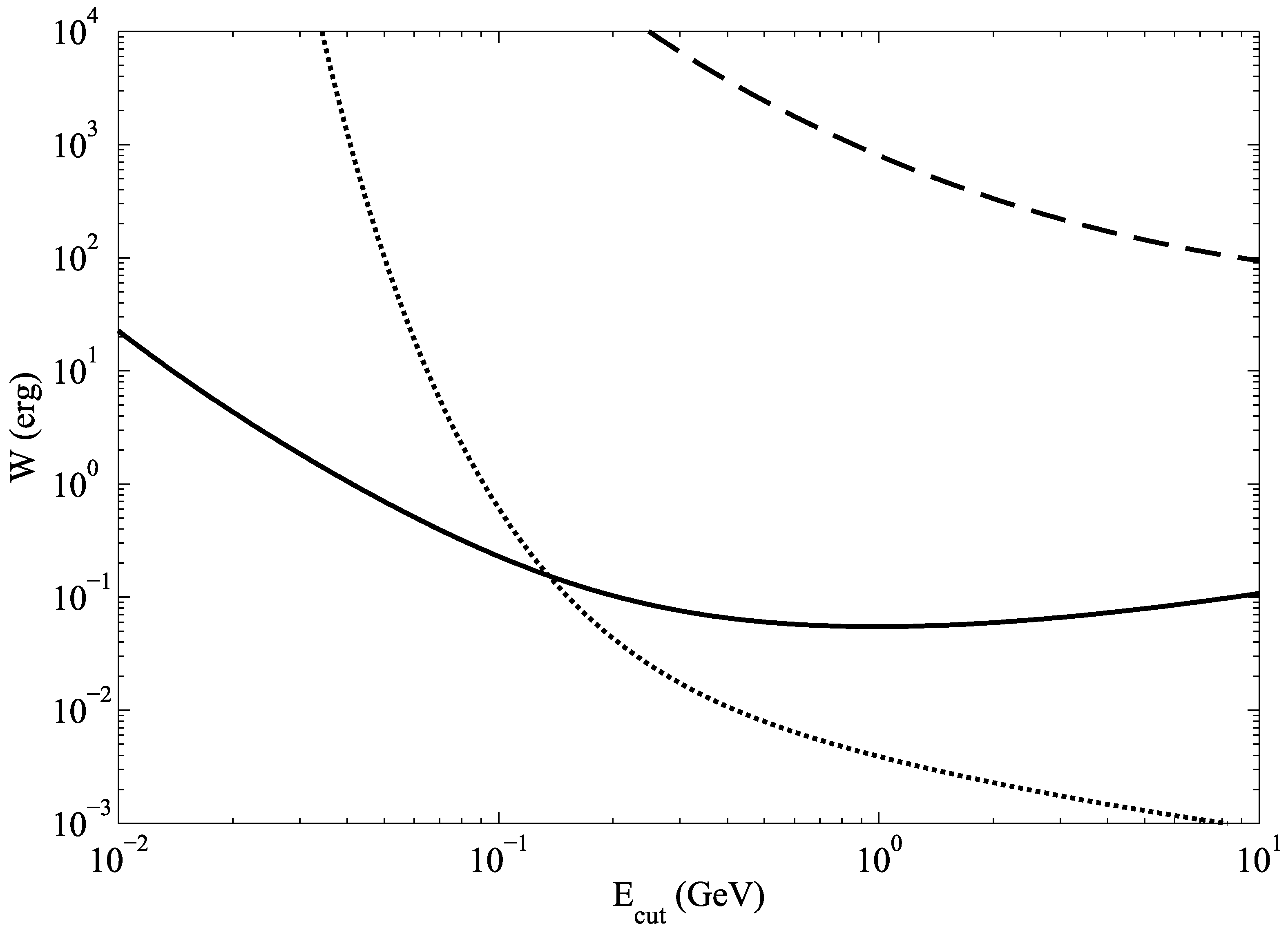
3.1. Power of Sources of Primary Particles
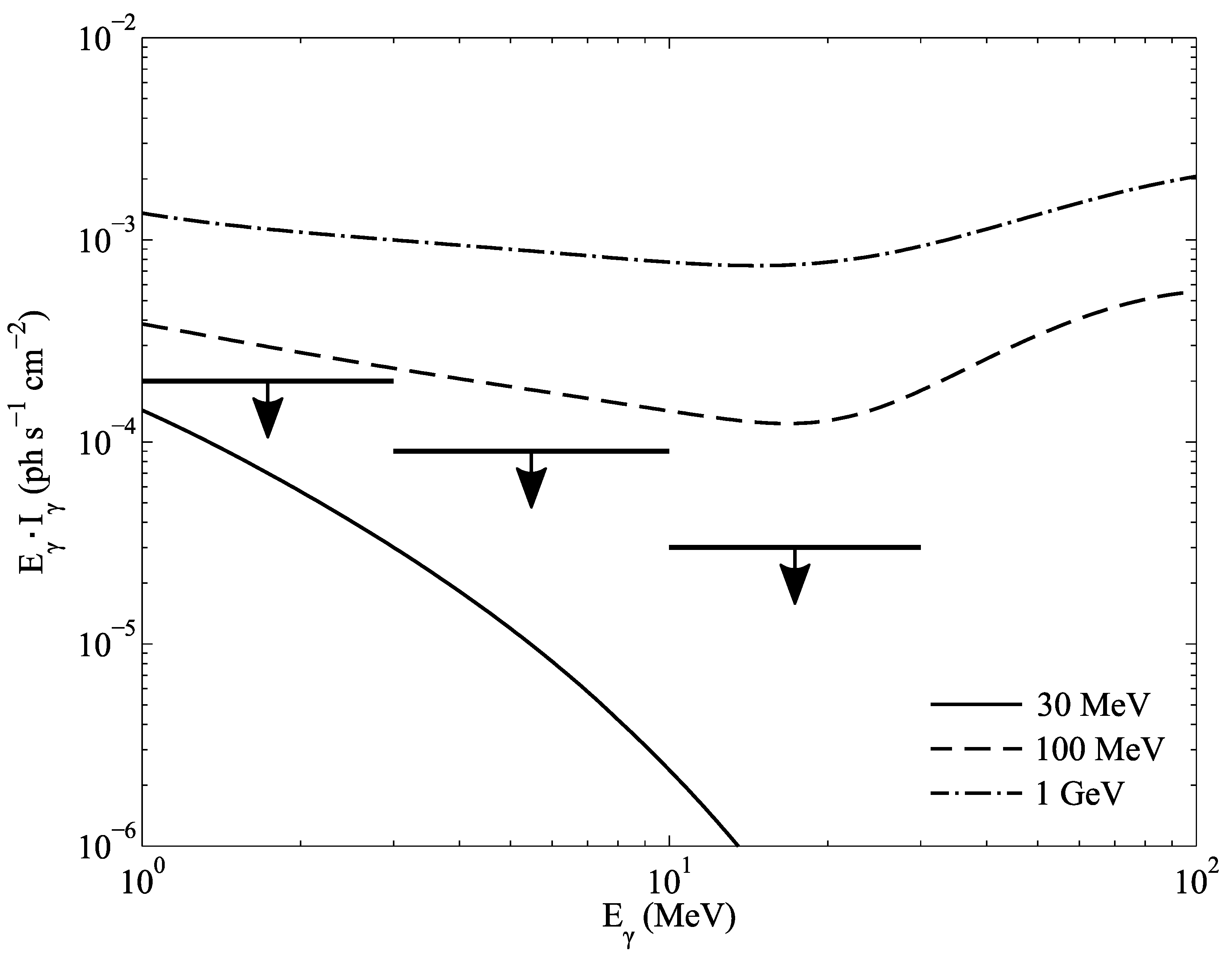
3.2. Byproduct -Ray Emission
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Churazov, E.; Bouchet, L.; Jean, P.; Jourdain, E.; Knödlseder, J.; Krivonos, R.; Roques, J.P.; Sazonov, S.; Siegert, T.; Strong, A.; et al. INTEGRAL results on the electron-positron annihilation radiation and X-ray & Gamma-ray diffuse emission of the Milky Way. New Astron. Rev. 2020, 90, 101548. [Google Scholar] [CrossRef]
- Frontera, F.; Virgilli, E.; Guidorzi, C.; Rosati, P.; Diehl, R.; Siegert, T.; Fryer, C.; Amati, L.; Auricchio, N.; Campana, R.; et al. Understanding the origin of the positron annihilation line and the physics of supernova explosions. Exp. Astron. 2021, 51, 1175–1202. [Google Scholar] [CrossRef]
- Siegert, T. The Positron puzzle. Astrophys. Space Sci. 2023, 368, 27. [Google Scholar] [CrossRef]
- Wang, W.; Pun, C.S.J.; Cheng, K.S. Could electron-positron annihilation lines in the Galactic center result from pulsar winds? Astron. Astrophys. 2006, 446, 943–948. [Google Scholar] [CrossRef]
- Istomin, Y.N.; Chernyshov, D.O.; Sob’yanin, D.N. Extinct radio pulsars as a source of subrelativistic positrons. Mon. Not. R. Astron. Soc. 2020, 498, 2089–2094. [Google Scholar] [CrossRef]
- Liang, E.P.; Dermer, C.D. Interpretation of the gamma-ray bump from Cygnus X-1. Astrophys. J. 1988, 325, L39–L42. [Google Scholar] [CrossRef]
- Bartels, R.; Calore, F.; Storm, E.; Weniger, C. Galactic binaries can explain the Fermi Galactic centre excess and 511 keV emission. Mon. Not. R. Astron. Soc. 2018, 480, 3826–3841. [Google Scholar] [CrossRef]
- Pshirkov, M.S. Positron excess in the center of the Milky Way from short-lived β+ emitting isotopes. Phys. Rev. D 2016, 94, 103002. [Google Scholar] [CrossRef]
- Prantzos, N.; Boehm, C.; Bykov, A.M.; Diehl, R.; Ferrière, K.; Guessoum, N.; Jean, P.; Knoedlseder, J.; Marcowith, A.; Moskalenko, I.V.; et al. The 511 keV emission from positron annihilation in the Galaxy. Rev. Mod. Phys. 2011, 83, 1001–1056. [Google Scholar] [CrossRef]
- Crocker, R.M.; Ruiter, A.J.; Seitenzahl, I.R.; Panther, F.H.; Sim, S.; Baumgardt, H.; Möller, A.; Nataf, D.M.; Ferrario, L.; Eldridge, J.J.; et al. Diffuse Galactic antimatter from faint thermonuclear supernovae in old stellar populations. Nat. Astron. 2017, 1, 0135. [Google Scholar] [CrossRef]
- Atoyan, A.M. Relativistic neutrons in active galactic nuclei. II. Gamma-rays of high and very high energies. Astron. Astrophys. 1992, 257, 476–488. [Google Scholar]
- Cheng, K.S.; Chernyshov, D.O.; Dogiel, V.A. Annihilation emission from the Galactic black hole. Astrophys. J. 2006, 645, 1138–1151. [Google Scholar] [CrossRef][Green Version]
- Cheng, K.S.; Chernyshov, D.O.; Dogiel, V.A. Diffuse gamma-ray emission from the Galactic center—A multiple energy injection model. Astron. Astrophys. 2007, 473, 351–356. [Google Scholar] [CrossRef][Green Version]
- Totani, T. A RIAF Interpretation for the past higher activity of the Galactic center black hole and the 511 keV annihilation emission. Publ. Astron. Soc. Jpn. 2006, 58, 965–977. [Google Scholar] [CrossRef]
- Aharonian, F.A.; Atoyan, A.M. On the Origin of the Galactic Annihilation Radiation. Astron. Lett. 1981, 7, 395–398. [Google Scholar]
- Beacom, J.F.; Yüksel, H. Stringent constraint on Galactic positron production. Phys. Rev. Lett. 2006, 97, 071102. [Google Scholar] [CrossRef]
- Sizun, P.; Cassé, M.; Schanne, S. Continuum γ-ray emission from light dark matter positrons and electrons. Phys. Rev. D 2006, 74, 063514. [Google Scholar] [CrossRef]
- Budnev, V.M.; Ginzburg, I.F.; Meledin, G.V.; Serbo, V.G. The two-photon particle production mechanism. Physical problems. Applications. Equivalent photon approximation. Phys. Rep. 1975, 15, 181–282. [Google Scholar] [CrossRef]
- Dremin, I.M. Ultraperipheral nuclear interactions. Phys. Uspekhi 2020, 63, 758–765. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. On the production of electrons and positrons by a collision of two particles. Phys. Z. Sowjet. 1934, 6, 244–257, Reprinted in Perspectives in Theoretical Physics. The Collected Papers of E.M. Lifshitz; Pitaevskii, L.P., Ed.; Elsevier: Amsterdam, The Netherlands, 1992; pp. 27–38. [Google Scholar] [CrossRef]
- Berestetskii, V.B.; Lifshits, E.M.; Pitaevskii, L.P. Quantum Electrodynamics; Pergamon Press Ltd.: Oxford, UK, 1982. [Google Scholar] [CrossRef]
- Lee, R.N.; Mingulov, K.T. Total Born cross section of e+e−-pair production in relativistic ion collisions from differential equations. Phys. Lett. B 2016, 757, 207–210. [Google Scholar] [CrossRef][Green Version]
- Racah, G. Sulla nascita di coppie per urti di particelle elettrizzate. Nuovo Cim. 1937, 14, 93–113. [Google Scholar] [CrossRef]
- Dremin, I.M. Ultraperipheral production of lepton pairs (perturbative and nonperturbative effects). Int. J. Mod. Phys. A 2022, 37, 2250098. [Google Scholar] [CrossRef]
- Froissart, M. Asymptotic behavior and subtractions in the Mandelstam representation. Phys. Rev. 1961, 123, 1053–1057. [Google Scholar] [CrossRef]
- Vysotsky, M.I.; Zhemchugov, E.V. Equivalent photons in proton-proton and ion-ion collisions at the Large Hadron Collider. Phys. Uspekhi 2019, 62, 910–919. [Google Scholar] [CrossRef]
- Godunov, S.I.; Karkaryan, E.K.; Novikov, V.A.; Rozanov, A.N.; Vysotsky, M.I.; Zhemchugov, E.V. pp scattering at the LHC with the lepton pair production and one proton tagging. Eur. Phys. J. C 2022, 82, 1055. [Google Scholar] [CrossRef]
- Dremin, I.M.; Gevorkyan, S.R.; Madigozhin, D.T. Enhancement of low-mass dileptons in ultraperipheral collisions. Eur. Phys. J. C 2021, 81, 276. [Google Scholar] [CrossRef]
- Bertulani, C.A.; Baur, G. Electromagnetic processes in relativistic heavy ion collisions. Phys. Rep. 1988, 163, 299–408. [Google Scholar] [CrossRef]
- Obraztsov, I.V.; Milstein, A.I. Quadrupole radiation and e+e- pair production in the collision of nonrelativistic nuclei. Phys. Lett. B 2021, 820, 136514. [Google Scholar] [CrossRef]
- Fermi, E. Sull’equilibrio termico di ionizzazione. Nuovo Cim. 1924, 1, 153–158. [Google Scholar] [CrossRef]
- Fermi, E. Über die Theorie des Stoßes zwischen Atomen und elektrisch geladenen Teilchen. Z. Phys. 1924, 29, 315–327. [Google Scholar] [CrossRef]
- Weizsäcker, C.F.V. Ausstrahlung bei Stößen sehr schneller Elektronen. Z. Phys. 1934, 88, 612–625. [Google Scholar] [CrossRef]
- Williams, E.J. Nature of the high energy particles of penetrating radiation and status of ionization and radiation formulae. Phys. Rev. 1934, 45, 729–730. [Google Scholar] [CrossRef]
- Siegert, T.; Diehl, R.; Khachatryan, G.; Krause, M.G.H.; Guglielmetti, F.; Greiner, J.; Strong, A.W.; Zhang, X. Gamma-ray spectroscopy of positron annihilation in the Milky Way. Astron. Astrophys. 2016, 586, A84. [Google Scholar] [CrossRef]
- Kamae, T.; Karlsson, N.; Mizuno, T.; Abe, T.; Koi, T. Parameterization of γ, e±, and neutrino spectra produced by p-p interaction in astronomical environments. Astrophys. J. 2006, 647, 692–708. [Google Scholar] [CrossRef]
- Dai, L.; McKinney, J.C.; Roth, N.; Ramirez-Ruiz, E.; Miller, M.C. A Unified model for tidal disruption events. Astrophys. J. Lett. 2018, 859, L20. [Google Scholar] [CrossRef]
- Geballe, T.R.; McCall, B.J.; Hinkle, K.H.; Oka, T. Detection of in the diffuse interstellar medium: The Galactic center and Cygnus OB2 Number 12. Astrophys. J. 1999, 510, 251–257. [Google Scholar] [CrossRef]
- Ferrière, K.; Gillard, W.; Jean, P. Spatial distribution of interstellar gas in the innermost 3 kpc of our galaxy. Astron. Astrophys. 2007, 467, 611–627. [Google Scholar] [CrossRef]
- Ivlev, A.V.; Padovani, M.; Galli, D.; Caselli, P. Interstellar dust charging in dense molecular clouds: Cosmic ray effects. Astrophys. J. 2015, 812, 135. [Google Scholar] [CrossRef]
- Stone, E.C.; Cummings, A.C.; McDonald, F.B.; Heikkila, B.C.; Lal, N.; Webber, W.R. Voyager 1 observes low-energy Galactic cosmic rays in a region depleted of heliospheric ions. Science 2013, 341, 150–153. [Google Scholar] [CrossRef]
- Dirac, P.A.M. On the annihilation of electrons and protons. Proc. Camb. Philos. Soc. 1930, 26, 361–375. [Google Scholar] [CrossRef]
- Kafexhiu, E.; Aharonian, F.; Taylor, A.M.; Vila, G.S. Parametrization of gamma-ray production cross sections for pp interactions in a broad proton energy range from the kinematic threshold to PeV energies. Phys. Rev. D 2014, 90, 123014. [Google Scholar] [CrossRef]
- Strong, A.W.; Bloemen, H.; Diehl, R.; Hermsen, W.; Schönfelder, V. COMPTEL Skymapping: A new approach using parallel computing. Astrophys. Lett. Commun. 1999, 39, 209–212. Available online: https://ui.adsabs.harvard.edu/abs/1999ApL%26C..39..209S%2F/ (accessed on 9 January 2024).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chernyshov, D.; Dogiel, V.; Dremin, I. Alternative Origin of Galactic Positrons Generated by Ultraperipheral Collisions of Cosmic Rays. Physics 2024, 6, 251-263. https://doi.org/10.3390/physics6010018
Chernyshov D, Dogiel V, Dremin I. Alternative Origin of Galactic Positrons Generated by Ultraperipheral Collisions of Cosmic Rays. Physics. 2024; 6(1):251-263. https://doi.org/10.3390/physics6010018
Chicago/Turabian StyleChernyshov, Dmitry, Vladimir Dogiel, and Igor Dremin. 2024. "Alternative Origin of Galactic Positrons Generated by Ultraperipheral Collisions of Cosmic Rays" Physics 6, no. 1: 251-263. https://doi.org/10.3390/physics6010018
APA StyleChernyshov, D., Dogiel, V., & Dremin, I. (2024). Alternative Origin of Galactic Positrons Generated by Ultraperipheral Collisions of Cosmic Rays. Physics, 6(1), 251-263. https://doi.org/10.3390/physics6010018





