Quantum Theory without the Axiom of Choice, and Lefschetz Quantum Physics
Abstract
:1. Introduction
2. Axiom of Choice: Mathematical Discussion
2.1. Russel’s Socks
2.2. Choice Functions and Cartesian Products
2.3. Vector Spaces
3. København Interpretations beyond
- (HS) A physical quantum system is represented by a Hilbert space , with the standard inner product and allowed to be nonfinite.
- (IP) The standard inner product sends to (or ), where is the complex conjugate of ; complex conjugation is an involutory automorphism of the field .
- (PS) Up to complex scalars, pure states (wave functions) are represented by nonzero vectors in ; usually, one considers normalized vectors.
- (TE) Time evolution operators are represented by linear operators of that preserve , that is, unitary operators. If is finite, unitary operators correspond to nonsingular complex -matrices U such that , where is the identity operator.
- (OB) Measuring an observable A in a system described by the wave function amounts to collapsing into one of the orthogonal eigenvectors of the Hermitian operator A, yielding as measurement the corresponding eigenvalue .
- (TP) Composite product states correspond to tensor products ; if a state in is not a product state, it is entangled.
- (BR) One follows Born’s rule, which says that if an observable A with discrete spectrum is measured, then the measurement will be one of the eigenvalues of A (this is OB), and the probability of measuring an eigenvalue is , where is the normalized wave function of the system, and is the projection onto the eigenspace of A corresponding to . If the eigenspace is 1-dimensional and is the normalized eigenvector which spans the eigenspace, one has that is the probability that the measurement will be made.
- (⋯).
3.1. The General Setting
3.2. Standard -Hermitian Forms
From now on, we propose to depict a physical quantum system in a general Hilbert space with k a division ring with involution .
3.3. Algebraically Closed Fields
3.4. Extension of Quantum Theories
Is embeddable in a complex-like theory (or in any other GQT, for that matter)?
3.4.1. Comparison Theory
3.4.2. Schnor’s Result on Involutions
3.5. The Minimal Model:
3.6. Finite Fields
- Let q be any prime power; then, up to isomorphism has a unique extension of degree 2, namely . The mapsends each element of to itself, while being an involutory automorphism of .
- Let n be any positive integer different from 0; then, if is the n-dimensional vector space over , define for and in V,
- For , one finds that
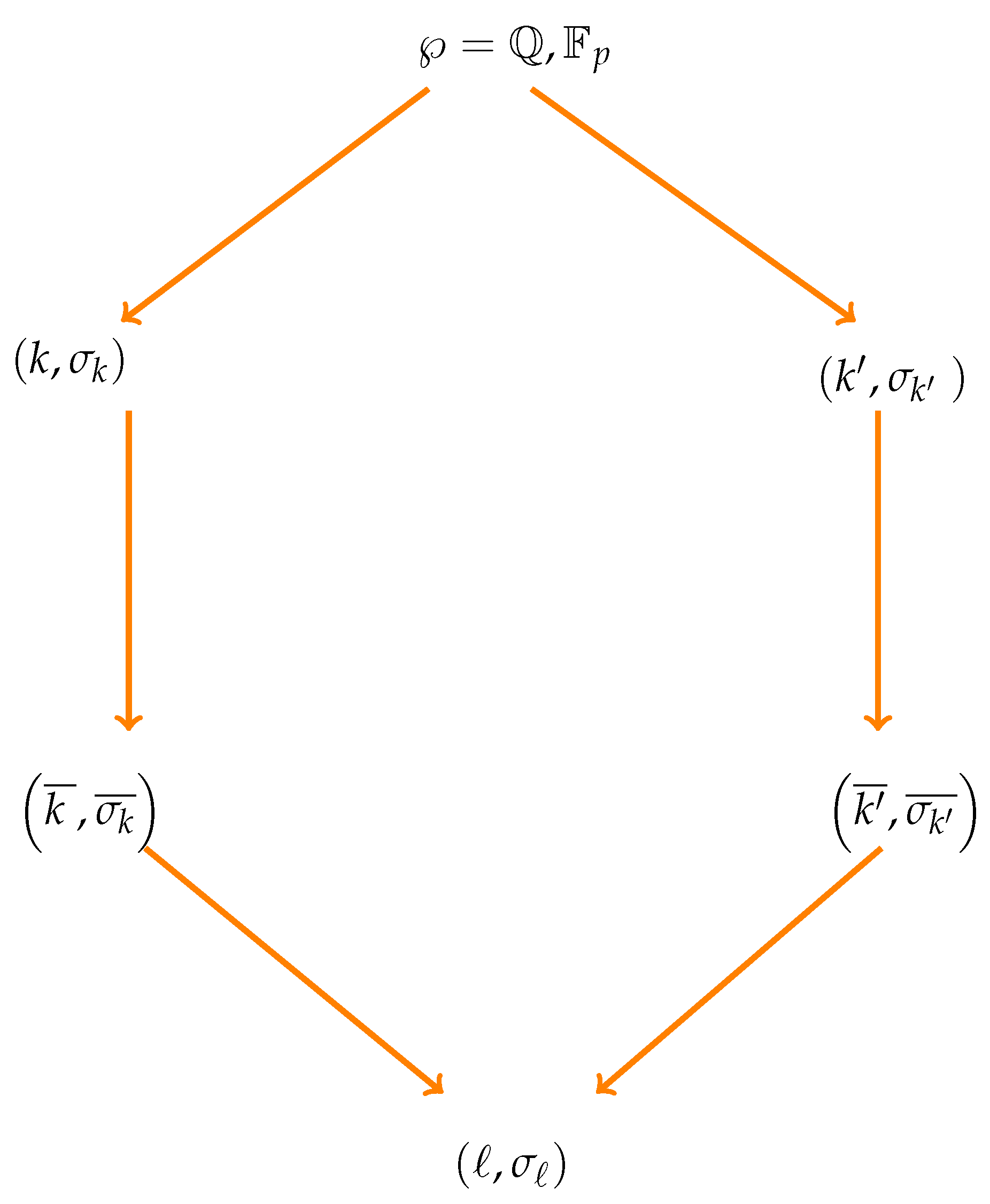
3.7. The Base Field in Fixed Characteristic: Quantum Lefschetz Formalism
The very question as of which base field is needed in the Københaving interpretation has a long history, and has been considered in many papers. In Appendix A, a short overview of some papers that consider the “base field question” is given and those are compared to the GQT-approach proposed in Ref. [2] by the author of this paper. It is instructive as well to refer to Refs. [9,10,11,12] (considered in Ref. [2]) to list other relevant studies.
4. Quantum Lefschetz Principle A
4.1. Lefschetz Principle
- Φ is true over every algebraically closed field in characteristic 0;
- Φ is true over some algebraically closed field in characteristic 0;
- Φ is true over algebraically closed fields in characteristic for arbitrarily large primes p;
- Φ is true over algebraically closed fields in characteristic for sufficiently large primes p.
4.2. Algebraically Closed Fields in Quantum Theory
5. Eigenvalues, Eigenvectors, and Probabilities
5.1. A Particular Example [19]
5.1.1. Identical Particles
5.1.2. Thought Experiment
- Both spaces do not admit an infinite orthonormal (Schauder, see Section 5.2) eigenbase, so there is no way to choose a mode of observation (in the sense of Bohr’s complementarity interpretation); there is no Hamel base either;
- As the vector space duals of and are different from and , there is no notion of a self-adjoint operator in both and .
5.2. Schauder Bases
5.3. Projector Operators
5.4. Double Slit Experiments: A Variation in Quantum Theory without AC
Thought Experiment: AC Black Box Measurements
5.5. New Born Formalism and Higher Schauder Bases
5.6. Blass’s Theorem and Ineffective Observables
6. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Comparing Theories
Appendix A.1. Barret/Hardy Approaches
- Both Hardy [24] and Barret [25] see states as probability vectors in some vector space V, and as the probability entries are real numbers (contained in the interval ), V is assumed to be a real vector space. This means that the underlying algebraic structure is assumed to contain the field of real numbers.In the unifying viewpoint of GQTs [2], probabilities are manifestations of the Hermitian form (through the generalized Born rule, e.g.), and the field or division ring one uses as underlying algebraic structure (whatever it is).
- In Ref. [24], two integer parameters K and N emerge, for which the identity holds. If one considers underlying algebraic structures such as the real numbers , the complex numbers or the quaternions , only confirms the aforementioned identity. Hardy concludes that this—at least intuitively—points towards the complex numbers, without providing a formal proof [26]. However, the identity was not considered in the entire realm of fields and division rings in characteristic 0—only over the set . (On the other hand, assuming the probabilities to be rational numbers in would also yield more flexibility for V.) Barret’s generalized probabilistic theories are based on Hardy’s axiomatic approach, so Barret ends up with the complex numbers as well.In our approach of GQTs [2], we also work with vector spaces, but any division ring (with involution) is allowed to be the coordinatizing agent, so as to find unifying behavior in this universe of quantum theories. The no-cloning result of Ref. [2], for instance, solely follows from the concept of linearity/superposition and is applicable for all division rings, hence also fields and algebraically closed fields, such as in particular (we refer to Refs. [27,28] for the initial no-cloning results over the complex numbers). The study in Ref. [2] shows that no-cloning is not a particular instance at all of quantum theory represented in the framework of complex numbers.
Appendix A.2. Cassinelli–Lahti Approach
References
- Braeckman, J.; Thas, K. De Invloed van Esthetiek op ons “Onafhankelijk” Denken; Ghent University: Ghent, Belgium, 2023. [Google Scholar]
- Thas, K. General quantum theory—unification of classical and modal quantum theories. J. Phys. A Math. Gen. 2020, 53, 395304. [Google Scholar] [CrossRef]
- Wootters, W.K. Entanglement sharing in real-vector-space quantum theory. Found. Phys. 2012, 42, 19–28. [Google Scholar] [CrossRef]
- Wootters, W.K. Optimal information transfer and real-vector-space quantum theory. In Quantum Theory: Informational Foundations and Foils; Chiribella, G., Spekkens, R.W., Eds.; Springer Science+Business Media B.V.: Dordrecht, The Netherlands, 2016; pp. 21–43. [Google Scholar] [CrossRef]
- Artin, E.; Schreier, O. Algebraische Konstruktion reeller Körper. Abh. Math. Semin. Univ. Hambg. 1927, 5, 85–99. [Google Scholar] [CrossRef]
- Schnor, B. Involutions in the group of automorphisms of an algebraically closed field. J. Algebra 1992, 152, 520–524. [Google Scholar] [CrossRef]
- Schumacher, B.; Westmoreland, M.D. Modal quantum theory. Found. Phys. 2012, 42, 918–925. [Google Scholar] [CrossRef]
- Lev, F.M. Introduction to a quantum theory over a Galois field. Symmetry 2010, 2, 1810–1845. [Google Scholar] [CrossRef]
- Aerts, D.; Daubechies, I. Physical justification for using the tensor product to describe two quantum systems as one joint system. Helv. Phys. Acta 1978, 51, 661–675. [Google Scholar] [CrossRef]
- Baez, J.C. Division algebras and quantum mechanics. Found. Phys. 2012, 42, 819–855. [Google Scholar] [CrossRef]
- Cassinelli, G.; Lahti, P. A theorem of Solér, the theory of symmetry and quantum mechanics. Int. J. Geom. Methods Mod. Phys. 2012, 9, 1260005. [Google Scholar] [CrossRef]
- Solér, P.M. Characterization of Hilbert spaces by orthomodular spaces. Commun. Algebra 1995, 23, 219–243. [Google Scholar] [CrossRef]
- Lefschetz, S. Algebraic Geometry; Princeton University Press: Princeton, NJ, USA, 1953. [Google Scholar] [CrossRef]
- Seidenberg, A. Comments on Lefschetz’s principle. Am. Math. Month. 1958, 65, 685–690. [Google Scholar] [CrossRef]
- Tarski, A. A Decision Method for Elementary Algebra and Geometry; RAND Corporation: Santa Monica, CA, USA, 1957; Available online: https://www.rand.org/pubs/reports/R109.html (accessed on 14 August 2023).
- Wootters, W.K.; (Williams College, Williamstown, US). Personal communication, 2023.
- Thas, K. Involutory Quantum Codes (Working Title); Ghent University: Ghent, Belgium, 2023. [Google Scholar]
- Thas, K. Quantum Theory over Finite Fields Is Falsifiable through the Heisenberg Principle; Ghent University: Ghent, Belgium, 2023. [Google Scholar]
- Brunner, N.; Svozil, K.; Baaz, M. The axiom of choice in quantum theory. Math. Log. Quart. 1996, 42, 319–340. [Google Scholar] [CrossRef]
- Loaiza, M. A short introduction to Hilbert Space theory. J. Phys. Conf. Ser. 2017, 839, 012002. [Google Scholar] [CrossRef]
- Läuchli, H. Auswahlaxiom in der Algebra. Comment. Math. Helv. 1962, 37, 1–18. [Google Scholar] [CrossRef]
- MATHEMATICS. Uncountable Series without Axiom of Choice; Stack Exchange Inc.: New York, NY, USA, 2014; Available online: https://math.stackexchange.com/questions/1042030/uncountable-series-without-axiom-of-choice (accessed on 14 August 2023).
- Blass, A. Existence of bases implies the axiom of choice. Contemp. Math. 1984, 31, 31–33. [Google Scholar] [CrossRef]
- Hardy, L. Quantum theory from five reasonable axioms. arXiv 2021, arXiv:quant-ph/0101012. [Google Scholar] [CrossRef]
- Barret, J. Information processing in generalized probabilistic theories. Phys. Rev. A 2007, 75, 032304. [Google Scholar] [CrossRef]
- Hardy, L.; (Perimeter Institute for Theoretical Physics, Waterloo, ON, Canada). Personal communication, 2019.
- Wootters, W.K.; Zurek, W.H. A single quantum cannot be cloned. Nature 1982, 299, 802–803. [Google Scholar] [CrossRef]
- Wootters, W.K.; Zurek, W.H. The no-cloning theorem. Phys. Today 2009, 62, 76–77. [Google Scholar] [CrossRef]
- Cassinelli, G.; Lahti, P. Quantum mechanics: Why complex Hilbert space? Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2017, 375, 20160393. [Google Scholar] [CrossRef] [PubMed]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thas, K. Quantum Theory without the Axiom of Choice, and Lefschetz Quantum Physics. Physics 2023, 5, 1109-1125. https://doi.org/10.3390/physics5040072
Thas K. Quantum Theory without the Axiom of Choice, and Lefschetz Quantum Physics. Physics. 2023; 5(4):1109-1125. https://doi.org/10.3390/physics5040072
Chicago/Turabian StyleThas, Koen. 2023. "Quantum Theory without the Axiom of Choice, and Lefschetz Quantum Physics" Physics 5, no. 4: 1109-1125. https://doi.org/10.3390/physics5040072
APA StyleThas, K. (2023). Quantum Theory without the Axiom of Choice, and Lefschetz Quantum Physics. Physics, 5(4), 1109-1125. https://doi.org/10.3390/physics5040072



