Clustering in Oxygen Nuclei and Spectator Fragments in 16O–16O Collisions at the LHC
Abstract
1. Introduction
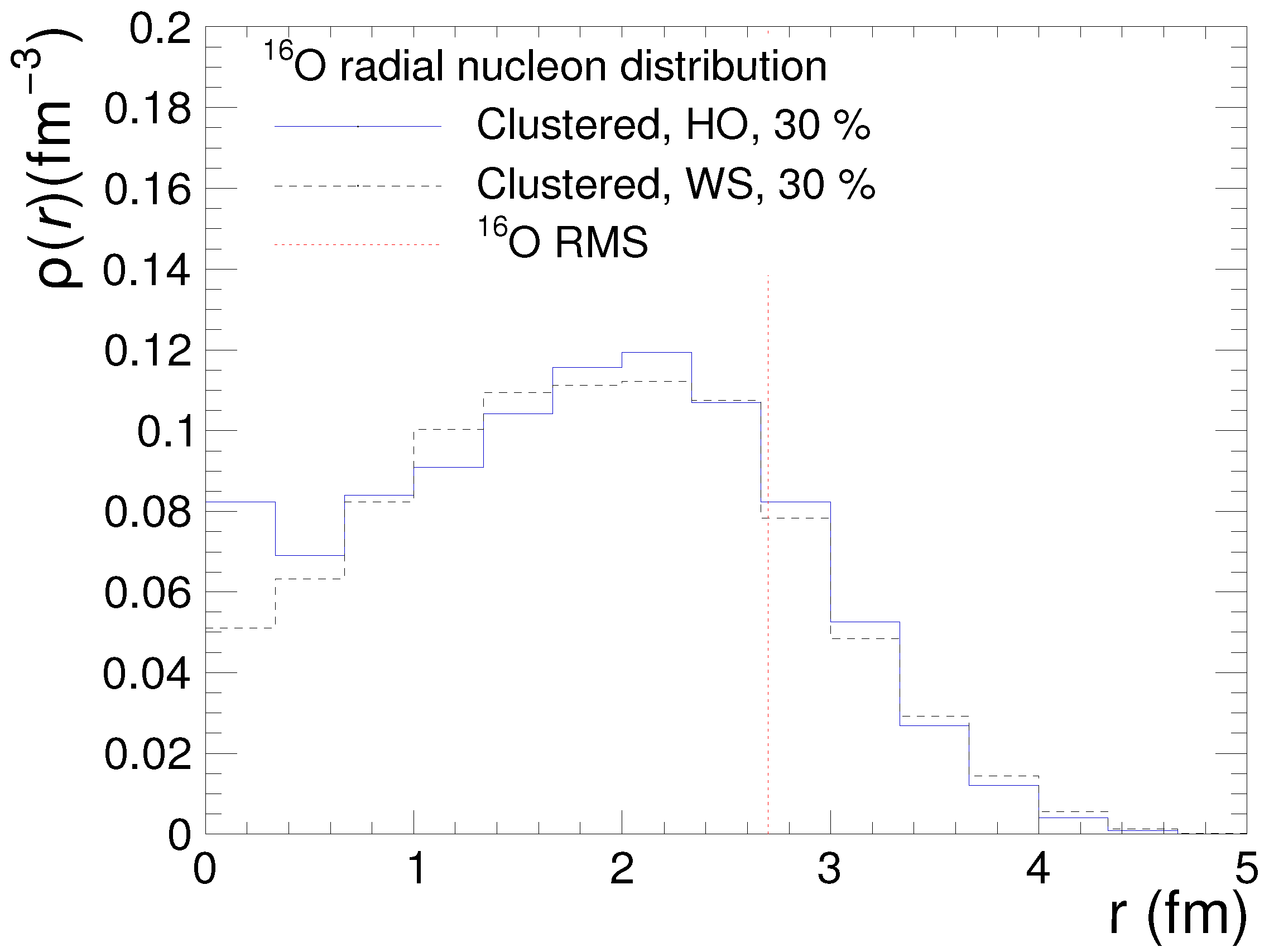
2. Modelling O–O Collisions in AAMCC-MST
3. Results
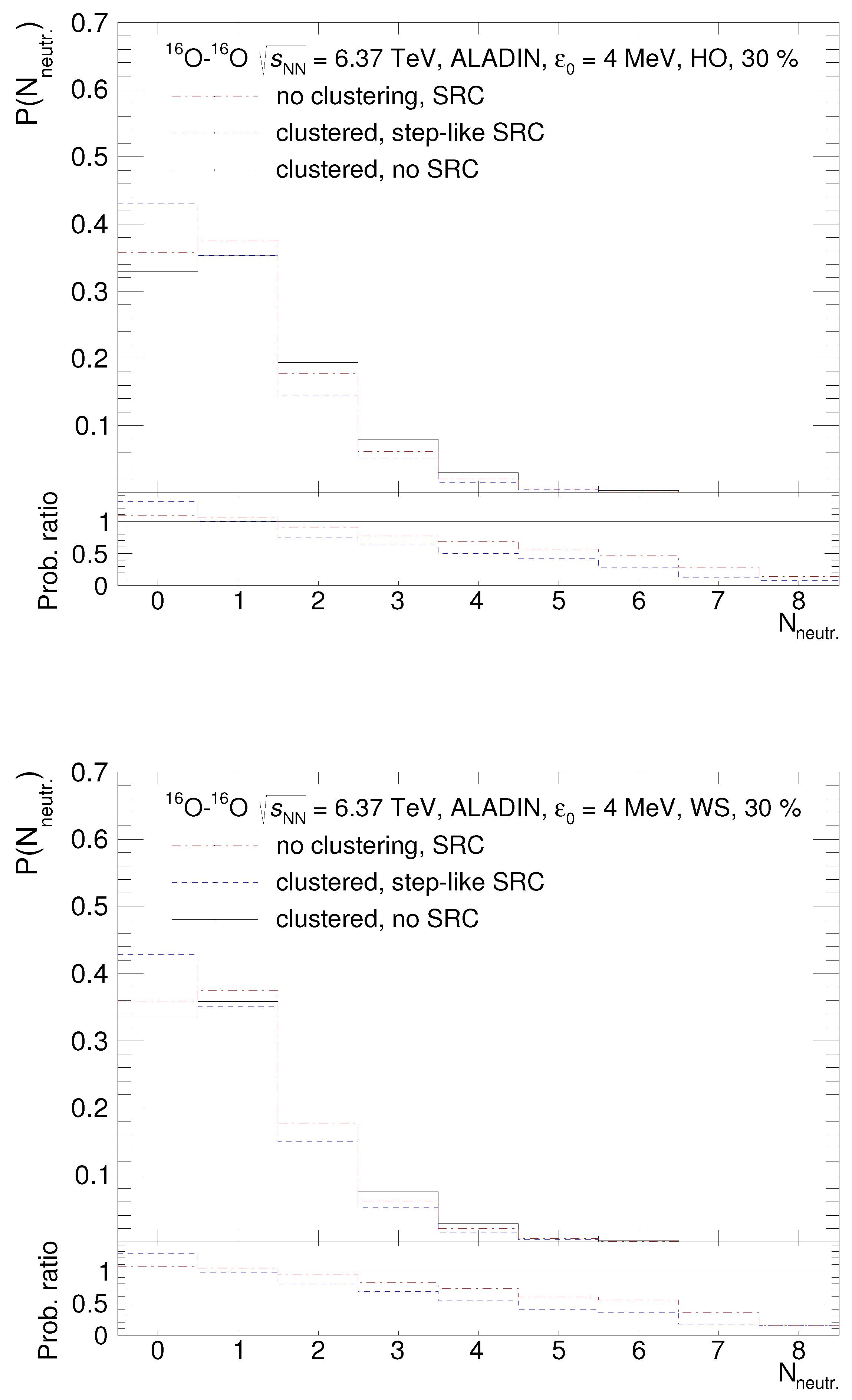
3.1. Production of Spectator Neutrons at the LHC
3.2. Production of Spectator Deuterons at the LHC
3.3. Production of Spectator Nuclei at the LHC
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AAMCC | Abrasion–Ablation Monte Carlo for Colliders |
| ALICE | A Large Ion Colider Experiment |
| AMPT | A Multi-Phase Transport model |
| CERN | European Organization for Nuclear Research |
| CNO | carbon, nitrogen, oxygen |
| GlauberMC | Glauber Monte Carlo |
| HO | harmonic oscillator |
| LHC | Large Hadron Collider |
| MST | Minimum Spanning Tree |
| QCD | Quantum Chromodynamics |
| RMS | root mean square |
| SPS | Super Proton Synchrotron |
| SRC | short-range correlations |
References
- ALICE Collaboration. The ALICE experiment—A journey through QCD. arXiv 2022, arXiv:2211.04384. [CrossRef]
- Report from Working Group 5: Future physics opportunities for high-density QCD at the LHC with heavy-ion and proton beams. In Physics at the HL-LHC and Perspectives for the HE-LHC; CERN Yellow Report, CERN-2019-007; Dainese, A., Mangano, M., Andreas, B., Meyer, A.B., Nisati, A., Salam, G., Vesterinen, M.A., Eds.; CERN: Geneva, Switzerland, 2019. [Google Scholar] [CrossRef]
- Brewer, J.; Mazeliauskas, A.; van der Schee, W. Opportunities of OO and pO collisions at the LHC. arXiv 2021, arXiv:2103.01939. [Google Scholar] [CrossRef]
- Bijker, R.; Iachello, F. Evidence for tetrahedral symmetry in 16O. Phys. Rev. Lett. 2014, 112, 152501. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.B.; Dong, G.X.; Gao, Z.C.; Chen, Y.S.; Shen, C.W. Tetrahedral symmetry in the ground state of 16O. Phys. Lett. B 2019, 790, 498–501. [Google Scholar] [CrossRef]
- Zuker, A.P.; Buck, B.; McGrory, J.B. Structure of 16O. Phys. Rev. Lett. 1968, 21, 39–43. [Google Scholar] [CrossRef]
- Behera, D.; Mallick, N.; Tripathy, S.; Prasad, S.; Mishra, A.N.; Sahoo, R. Predictions on global properties in O+O collisions at the Large Hadron Collider using a multi-phase transport model. Eur. Phys. J. A 2022, 58, 175. [Google Scholar] [CrossRef]
- Lim, S.H.; Carlson, J.; Loizides, C.; Lonardoni, D.; Lynn, J.E.; Nagle, J.L.; Orjuela Koop, J.D.; Ouellette, J. Exploring new small system geometries in heavy ion collisions. Phys. Rev. C 2019, 99, 044904. [Google Scholar] [CrossRef]
- Li, Y.-A.; Zhang, S.; Ma, Y.-G. Signatures of α-clustering in 16O by using a multiphase transport model. Phys. Rev. C 2020, 102, 054907. [Google Scholar] [CrossRef]
- Bally, B.; Brandenburg, J.D.; Giacalone, G.; Heinz, U.; Huang, S.; Jia, J.; Lee, D.; Lee, Y.-J.; Li, W.; Loizides, C.; et al. Imaging the initial condition of heavy-ion collisions and nuclear structure across the nuclide chart. arXiv 2022, arXiv:2209.11042. [Google Scholar] [CrossRef]
- Broniowski, W.; Bożek, P.; Rybczyński, M.; Ruiz Arriola, E. Flow in collisions of light nuclei. Nucl. Phys. A 2021, 1005, 121763. [Google Scholar] [CrossRef]
- Katz, R.; Prado, C.A.G.; Noronha-Hostler, J.; Suaide, A.A.P. System-size scan of D meson RAA and vn using PbPb, XeXe, ArAr, and OO collisions at energies available at the CERN Large Hadron Collider. Phys. Rev. C 2020, 102, 041901. [Google Scholar] [CrossRef]
- El-Nagdy, M.S.; Abdelsalam, A.; Badawy, B.M.; Zarubin, P.I.; Abdalla, A.M.; Nabil Yasin, M.; Saber, A.; Mohamed, M.M.; Ahmed, M.M. Channels of projectile fragmentation of 16O nucleus in nuclear emulsion. J. Phys. Commun. 2018, 2, 035010. [Google Scholar] [CrossRef]
- Nepeivoda, R.; Svetlichnyi, A.; Kozyrev, N.; Pshenichnov, I. Pre-equilibrium clustering in production of spectator fragments in collisions of relativistic nuclei. Particles 2022, 5, 40–51. [Google Scholar] [CrossRef]
- Svetlichnyi, A.; Nepeivoda, R.; Kozyrev, N.; Pshenichnov, I. Secondary nuclei from 16O fragmentation at the LHC. PoS 2022, EPS-HEP2021, 310. [Google Scholar] [CrossRef]
- Svetlichnyi, A.; Savenkov, S.; Nepeivoda, R.; Kozyrev, N.; Pshenichnov, I. Smoking gun of nuclear clusterization in collisions of light relativistic nuclei. Phys. At. Nucl. 2022, 85, 958–964. [Google Scholar] [CrossRef]
- Alvioli, M.; Drescher, H.J.; Strikman, M. A Monte Carlo generator of nucleon configurations in complex nuclei including nucleon–nucleon correlations. Phys. Lett. B 2009, 680, 225–230. [Google Scholar] [CrossRef]
- Alvioli, M.; Strikman, M. Beam fragmentation in heavy ion collisions with realistically correlated nuclear configurations. Phys. Rev. C 2011, 83, 044905. [Google Scholar] [CrossRef]
- Kozyrev, N.; Svetlichnyi, A.; Nepeivoda, R.; Pshenichnov, I. Peeling away neutron skin in ultracentral collisions of relativistic nuclei. Eur. Phys. J. A 2022, 58, 184. [Google Scholar] [CrossRef]
- Abrasion-Ablation Monte Carlo for Colliders Repository. Available online: https://github.com/Spectator-matter-group-INR-RAS/AAMCC (accessed on 15 March 2023).
- Dumbrajs, O. Analyticity and model-independent determination of the nuclear charge density. Phys. Rev. C 1980, 21, 1677–1679. [Google Scholar] [CrossRef]
- Angeli, I.; Marinova, K. Table of experimental nuclear ground state charge radii: An update. At. Data Nucl. Data Tables 2013, 99, 69–95. [Google Scholar] [CrossRef]
- Shukla, A.; Åberg, S.; Patra, S.K. Nuclear structure and reaction properties of even–even oxygen isotopes towards drip line. J. Phys. G 2011, 38, 095103. [Google Scholar] [CrossRef]
- Ozawa, A.; Suzuki, T.; Tanihata, I. Nuclear size and related topics. Nucl. Phys. A 2001, 693, 32–62. [Google Scholar] [CrossRef]
- Liatard, E.; Bruandet, J.F.; Glasser, F.; Kox, S.; Chan, T.U.; Costa, G.J.; Heitz, C.; Masri, Y.E.; Hanappe, F.; Bimbot, R.; et al. Matter distribution in neutron-rich light nuclei and total reaction cross-section. Europhys. Lett. (EPL) 1990, 13, 401–404. [Google Scholar] [CrossRef]
- Owen, A.B. Monte Carlo Theory, Methods and Examples. 2013. Available online: https://artowen.su.domains/mc (accessed on 15 March 2023).
- Loizides, C.; Nagle, J.; Steinberg, P. Improved version of the PHOBOS Glauber Monte Carlo. arXiv 2014, arXiv:1408.2549. [Google Scholar] [CrossRef]
- Loizides, C.; Kamin, J.; D’Enterria, D. Improved Monte Carlo Glauber predictions at present and future nuclear colliders. Phys. Rev. C 2018, 97, 054910. [Google Scholar] [CrossRef]
- Botvina, A.S.; Mishustin, I.N.; Begemann-Blaich, M.; Hubele, J.; Imme, G.; Iori, I.; Kreutz, P.; Kunde, G.J.; Kunze, W.D.; Lindenstruth, V.; et al. Multifragmentation of spectators in relativistic heavy-ion reactions. Nucl. Phys. A 1995, 584, 737–756. [Google Scholar] [CrossRef]
- Allison, J.; Amako, K.; Apostolakis, J.; Arce, P.; Asai, M.; Aso, T.; Bagli, E.; Bagulya, A.; Banerjee, S.; Barrand, G.; et al. Recent developments in Geant4. Nucl. Inst. Meth. A 2016, 835, 186–225. [Google Scholar] [CrossRef]
- Puddu, G.; Arnaldi, R.; Chiavassa, E.; Cicaló, C.; Cortese, P.; De Falco, A.; Dellacasa, G.; Ferretti, A.; Floris, M.; Gagliardi, M.; et al. The zero degree calorimeters for the ALICE experiment. Nucl. Inst. Meth. A 2007, 581, 397–401. [Google Scholar] [CrossRef]
- ALICE Collaboration. Centrality determination of Pb-Pb collisions at sNN = 2.76 TeV with ALICE. Phys. Rev. C 2013, 88, 044909. [Google Scholar] [CrossRef]
- ALICE Collaboration. ALICE luminosity determination for Pb-Pb collisions at sNN=5.02 TeV. arXiv 2022, arXiv:2204.10148. [CrossRef]
- Kondev, F.G.; Wang, M.; Huang, W.J.; Naimi, S.; Audi, G. The NUBASE2020 evaluation of nuclear physics properties. Chin. Phys. C 2021, 45, 030001. [Google Scholar] [CrossRef]
- Bruce, R.; Alemany-Fernández, R.; Bartosik, H.; Jebramcik, M.A.; Jowett, J.M.; Schaumann, M. Studies for an LHC pilot run with oxygen beams. In Proceedings of the 12th International Particle Accelerator Conference: IPAC2021, Campinas, Brazil, 24–28 May 2021; Lin, L., Byrd, J.M., Neuenschwander, R., Picoreti, R., Schaa, V.R.W., Eds.; JACoW Publishing: Geneva, Switzerland, 2021; pp. 53–56. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Svetlichnyi, A.; Savenkov, S.; Nepeivoda, R.; Pshenichnov, I. Clustering in Oxygen Nuclei and Spectator Fragments in 16O–16O Collisions at the LHC. Physics 2023, 5, 381-390. https://doi.org/10.3390/physics5020027
Svetlichnyi A, Savenkov S, Nepeivoda R, Pshenichnov I. Clustering in Oxygen Nuclei and Spectator Fragments in 16O–16O Collisions at the LHC. Physics. 2023; 5(2):381-390. https://doi.org/10.3390/physics5020027
Chicago/Turabian StyleSvetlichnyi, Aleksandr, Savva Savenkov, Roman Nepeivoda, and Igor Pshenichnov. 2023. "Clustering in Oxygen Nuclei and Spectator Fragments in 16O–16O Collisions at the LHC" Physics 5, no. 2: 381-390. https://doi.org/10.3390/physics5020027
APA StyleSvetlichnyi, A., Savenkov, S., Nepeivoda, R., & Pshenichnov, I. (2023). Clustering in Oxygen Nuclei and Spectator Fragments in 16O–16O Collisions at the LHC. Physics, 5(2), 381-390. https://doi.org/10.3390/physics5020027







