Parametrization of Deceleration Parameter in f(Q) Gravity
Abstract
:1. Introduction
2. Gravity Formalism
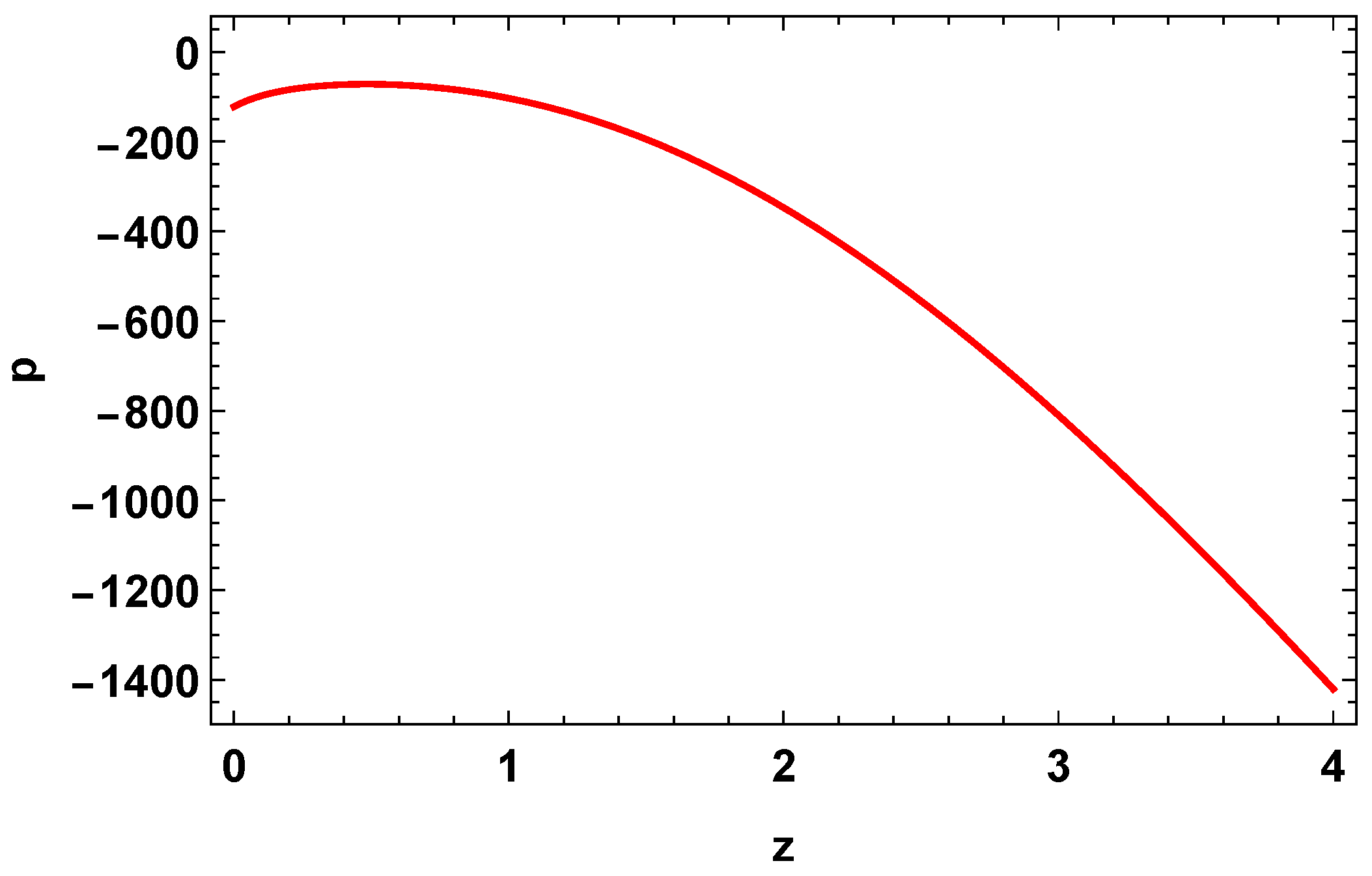
3. Parametrization of the Deceleration Parameter
4. Observational Constraints and Cosmological Applications
Cosmic Chronometer (CC) Sample
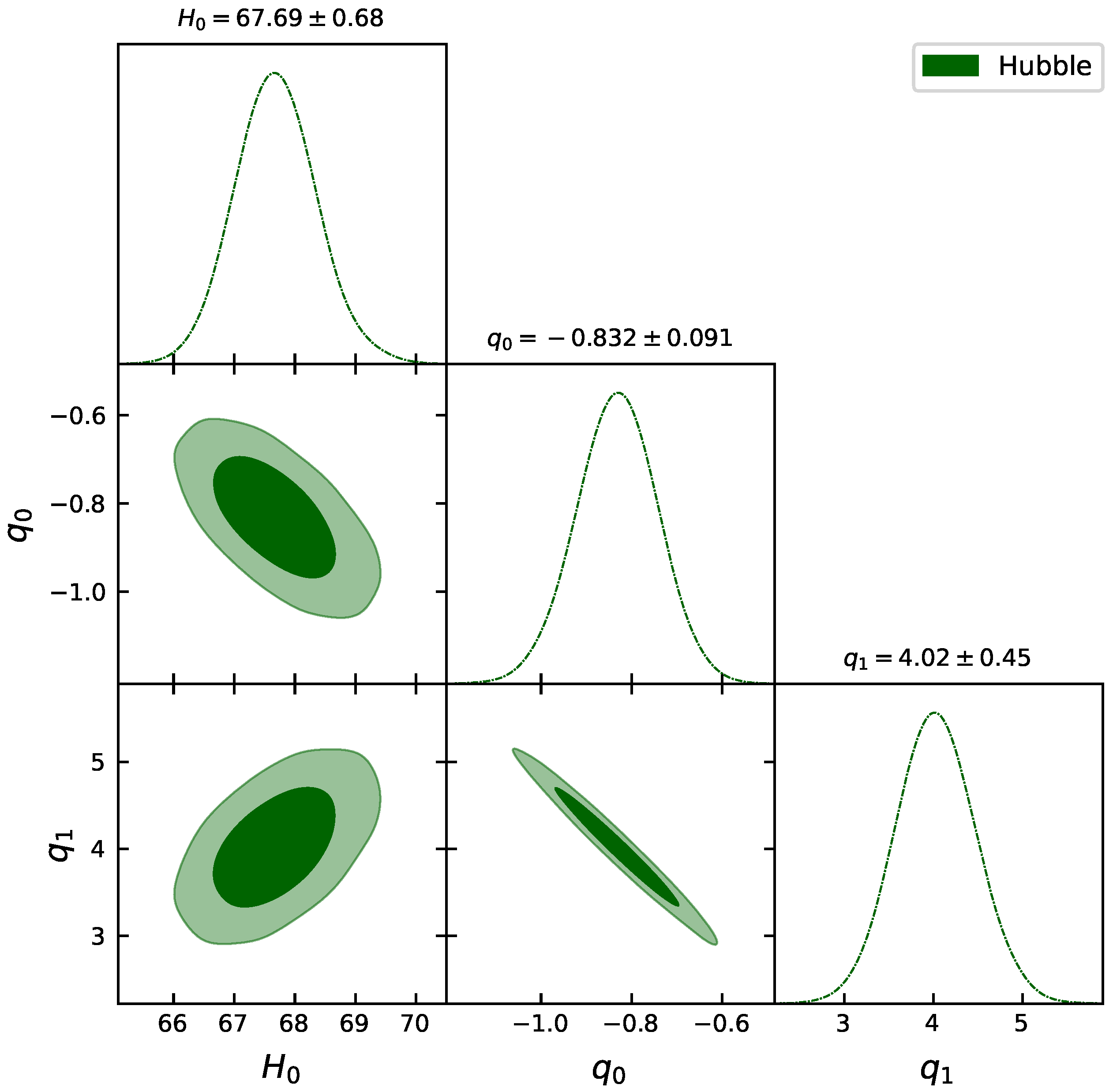
5. Cosmological Parameters
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E. et al. [The Supernova Cosmology Project] Measurements of Ω and Λ from 42 high-redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, J.C.; Jha, S.; Kirshner, P.R.; et al. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astrophys. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef] [Green Version]
- Riess, A.G.; Strolger, L.G.; Tonry, J.; Casertano, S.; Ferguson, H.C.; Mobasher, B.; Challis, P.; Filippenko, A.V.; Jha, S.; Li, W.; et al. Type Ia supernova discoveries at z > 1 from the Hubble Space Telescope: Evidence for past deceleration and constraints on dark energy evolution. Astrophys. J. 2004, 607, 665–687. [Google Scholar] [CrossRef] [Green Version]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First-year Wilkinson Microwave Anisotropy Probe (WMAP) observations: Determination of cosmological parameters. Astrophys. J. Suppl. 2003, 148, 175–194. [Google Scholar] [CrossRef] [Green Version]
- Koivisto, T.; Mota, D.F. Dark energy anisotropic stress and large scale structure formation. Phys. Rev. D 2006, 73, 083502. [Google Scholar] [CrossRef]
- Daniel, S.F.; Caldwell, R.R.; Cooray, A.; Melchiorri, A. Large scale structure as a probe of gravitational slip. Phys. Rev. D 2008, 77, 103513. [Google Scholar] [CrossRef] [Green Version]
- Corda, C. Interferometric detection of gravitational waves: The definitive test for general relativity. Int. J. Mod. Phys. D 2009, 18, 2275–2282. [Google Scholar] [CrossRef]
- Buchdahl, H.A. Non-linear Lagrangians and cosmological theory. Mon. Not. R. Astron. Soc. 1970, 150, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Ferraro, R.; Fiorini, F. Modified teleparallel gravity: Inflation without an inflaton. Phys. Rev. D 2007, 75, 084031. [Google Scholar] [CrossRef] [Green Version]
- Myrzakulov, R. Accelerating universe from F(T) gravity. Eur. Phys. J. C 2012, 71, 1752. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.; Nojiri, S.I.; Odintsov, S.D. f(R, T) gravity. Phys. Rev. D 2011, 84, 024020. [Google Scholar] [CrossRef] [Green Version]
- Sahoo, P.K.; Moraes, P.H.R.S.; Sahoo, P. Wormholes in R2-gravity within the f(R, T) formalism. Eur. Phys. J. C 2018, 78, 46. [Google Scholar] [CrossRef] [Green Version]
- Harko, T.; Lobo, F.S.N. f(R, Lm) gravity. Eur. Phys. J. C 2010, 70, 373–379. [Google Scholar] [CrossRef]
- Jaybhaye, L.V.; Solanki, R.; Mandal, S.; Sahoo, P.K. Cosmology in f(R, Lm) gravity. Phys. Lett. B 2022, 831, 137148. [Google Scholar] [CrossRef]
- Elizalde, E.; Myrzakulov, R.; Obukhov, V.V.; Sáez-Gómez, D. ΛCDM epoch reconstruction from F(R, G) and modified Gauss–Bonnet gravities. Class. Quantum Grav. 2010, 27, 095007. [Google Scholar] [CrossRef] [Green Version]
- Bamba, K.; Odintsov, S.D.; Sebastiani, L.; Zerbini, S. Finite-time future singularities in modified Gauss-Bonnet and F(R, G) gravity and singularity avoidance. Eur. Phys. J. C 2010, 67, 295–310. [Google Scholar] [CrossRef]
- Jimenez, J.B.; Heisenberg, L.; Koivisto, T. Coincident general relativity. Phys. Rev. D 2018, 98, 044048. [Google Scholar] [CrossRef] [Green Version]
- Mandal, S.; Wang, D.; Sahoo, P.K. Cosmography in f(Q) gravity. Phys. Rev. D 2020, 102, 124029. [Google Scholar] [CrossRef]
- Mandal, S.; Sahoo, P.K.; Santos, J.R.L. Energy conditions in f(Q) gravity. Phys. Rev. D 2020, 102, 024057. [Google Scholar] [CrossRef]
- Lazkoz, R.; Lobo, F.S.N.; Ortiz-Baños, M.; Salzano, V. Observational constraints of f(Q) gravity. Phys. Rev. D 2019, 100, 104027. [Google Scholar] [CrossRef]
- Solanki, R.; Mandal, S.; Sahoo, P.K. Cosmic acceleration with bulk viscosity in modified f(Q) gravity. Phys. Dark Univ. 2021, 32, 100820. [Google Scholar] [CrossRef]
- Esposito, F.; Carloni, S.; Cianci, R.; Vignolo, S. Reconstructing isotropic and anisotropic f(Q) cosmologies. Phys. Rev. D 2022, 105, 084061. [Google Scholar] [CrossRef]
- Anagnostopoulos, F.K.; Gakis, V.; Saridakis, E.N.; Basilakos, S. New models and Big Bang Nucleosynthesis constraints in f(Q) gravity. arXiv 2022, arXiv:2205.11445. [Google Scholar] [CrossRef]
- Harko, T.; Koivisto, T.S.; Lobo, F.S.; Olmo, G.J.; Rubiera-Garcia, D. Coupling matter Q in modified gravity. Phys. Rev. D 2018, 98, 084043. [Google Scholar] [CrossRef] [Green Version]
- Frusciante, N. Signatures of f(Q) gravity in cosmology. Phys. Rev. D 2021, 103, 044021. [Google Scholar] [CrossRef]
- Khyllep, W.; Paliathanasis, A.; Dutta, J. Cosmological solutions and growth index of matter perturbations in f(Q) gravity. Phys. Rev. D 2021, 103, 103521. [Google Scholar] [CrossRef]
- Ayuso, I.; Lazkoz, R.; Salzano, V. Observational constraints on cosmological solutions of f(Q) theories. Phys. Rev. D 2021, 103, 063505. [Google Scholar] [CrossRef]
- Gadbail, G.; Mandal, S.; Sahoo, P.K. Reconstruction of ΛCDM universe in f(Q) gravity. Phys. Lett. B 2022, 835, 137509. [Google Scholar] [CrossRef]
- Capozziello, S.; D’Agostino, R. Model-independent reconstruction of f(Q) non-metric gravity. Phys. Lett. B 2022, 832, 137229. [Google Scholar] [CrossRef]
- Mukherjee, A. Acceleration of the universe: A reconstruction of the effective equation of state. Mon. Not. R. Astron. Soc. 2016, 460, 273–282. [Google Scholar] [CrossRef]
- Arora, S.; Parida, A.; Sahoo, P.K. Constraining effective equation of state in f(Q,T) gravity. Eur. Phys. J. C 2021, 81, 555. [Google Scholar] [CrossRef]
- Gadbail, G.N.; Arora, S.; Kumar, P.; Sahoo, P.K. Interaction of divergence-free deceleration parameter in Weyl-type f(Q,T) gravity. Chin. J. Phys. 2022, 79, 246–255. [Google Scholar] [CrossRef]
- Banerjee, N.; Das, S. Acceleration of the universe with a simple trigonometric potential. Gen. Relativ. Gravit. 2005, 37, 1695–1703. [Google Scholar] [CrossRef] [Green Version]
- Cunha, J.V.; Lima, J.A.S. Transition redshift: New kinematic constraints from supernovae. Mon. Not. R. Astron. Soc. 2008, 390, 210–217. [Google Scholar] [CrossRef]
- Escamilla-Rivera, C.; Nájera, A. Dynamical dark energy models in the light of gravitational-wave transient catalogues. J. Cosmol. Astropart. Phys. 2022, 03, 060. [Google Scholar] [CrossRef]
- Wang, Y.-T.; Xu, L.-X.; Lu, J.-B.; Gui, Y.-X. Reconstructing dark energy potentials from parameterized deceleration parameters. Chin. Phys. B 2010, 19, 019801. [Google Scholar] [CrossRef] [Green Version]
- Gong, Y.; Wang, A. Observational constraints on the acceleration of the Universe. Phys. Rev. D 2006, 73, 083506. [Google Scholar] [CrossRef] [Green Version]
- Hobson, M.P.; Jaffe, A.H.; Liddle, A.R.; Mukherjee, P.; Parkison, D. (Eds.) Bayesian Methods in Cosmology; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar] [CrossRef] [Green Version]
- Singirikonda, H.; Desai, S. Model comparison of ΛCDM vs Rh=ct using cosmic chronometers. Eur. Phys. J. C 2020, 80, 694. [Google Scholar] [CrossRef]
- Simon, J.; Verde, L.; Jimenez, R. Constraints on the redshift dependence of the dark energy potential. Phys. Rev. D 2005, 71, 123001. [Google Scholar] [CrossRef]
- Stern, D.; Jimenez, R.; Verde, L.; Kamionkowski, M.; Stanford, S.A. Cosmic chronometers: Constraining the equation of state of dark energy. I: H(z) measurements. J. Cosmol. Astropart. Phys. 2010, 02, 008. [Google Scholar] [CrossRef] [Green Version]
- Moresco, M. Raising the bar: New constraints on the Hubble parameter with cosmic chronometers at z2. Mon. Not. R. Astron. Soc. 2015, 450, L16–L20. [Google Scholar] [CrossRef] [Green Version]
- Ratsimbazafy, A.L.; Loubser, S.I.; Crawford, S.M.; Cress, C.M.; Bassett, B.A.; Nichol, R.C.; Väisänen, P. Age-dating luminous red galaxies observed with the Southern African Large Telescope. Mon. Not. R. Astron. Soc. 2017, 467, 3239–3254. [Google Scholar] [CrossRef] [Green Version]
- Gao, L.-Y.; Zhao, Z.-W.; Xue, S.-S.; Zhang, X. Relieving the H0 tension with a new interacting dark energy model. J. Cosmol. Astropart. Phys. 2021, 07, 005. [Google Scholar] [CrossRef]
- Mamon, A.A.; Das, S. A divergence-free parametrization of deceleration parameter for scalar field dark energy. Int. J. Mod. Phys. D 2016, 25, 1650032. [Google Scholar] [CrossRef]
- Hanafy, W.E.; Nashed, G.G.L. Phenomenological reconstruction of f(T) teleparallel gravity. Phys. Rev. D 2019, 100, 083535. [Google Scholar] [CrossRef] [Green Version]
- Gong, Y.; Wang, A. Reconstruction of the deceleration parameter and the equation of state of dark energy. Phys. Rev. D 2007, 75, 043520. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gadbail, G.N.; Mandal, S.; Sahoo, P.K. Parametrization of Deceleration Parameter in f(Q) Gravity. Physics 2022, 4, 1403-1412. https://doi.org/10.3390/physics4040090
Gadbail GN, Mandal S, Sahoo PK. Parametrization of Deceleration Parameter in f(Q) Gravity. Physics. 2022; 4(4):1403-1412. https://doi.org/10.3390/physics4040090
Chicago/Turabian StyleGadbail, Gaurav N., Sanjay Mandal, and Pradyumn Kumar Sahoo. 2022. "Parametrization of Deceleration Parameter in f(Q) Gravity" Physics 4, no. 4: 1403-1412. https://doi.org/10.3390/physics4040090
APA StyleGadbail, G. N., Mandal, S., & Sahoo, P. K. (2022). Parametrization of Deceleration Parameter in f(Q) Gravity. Physics, 4(4), 1403-1412. https://doi.org/10.3390/physics4040090






