Seismic Fragility Curves of RC Buildings Subjected to Aging
Abstract
1. Introduction
2. Degradation of Reinforced Concrete Structures
2.1. Damage and Durability
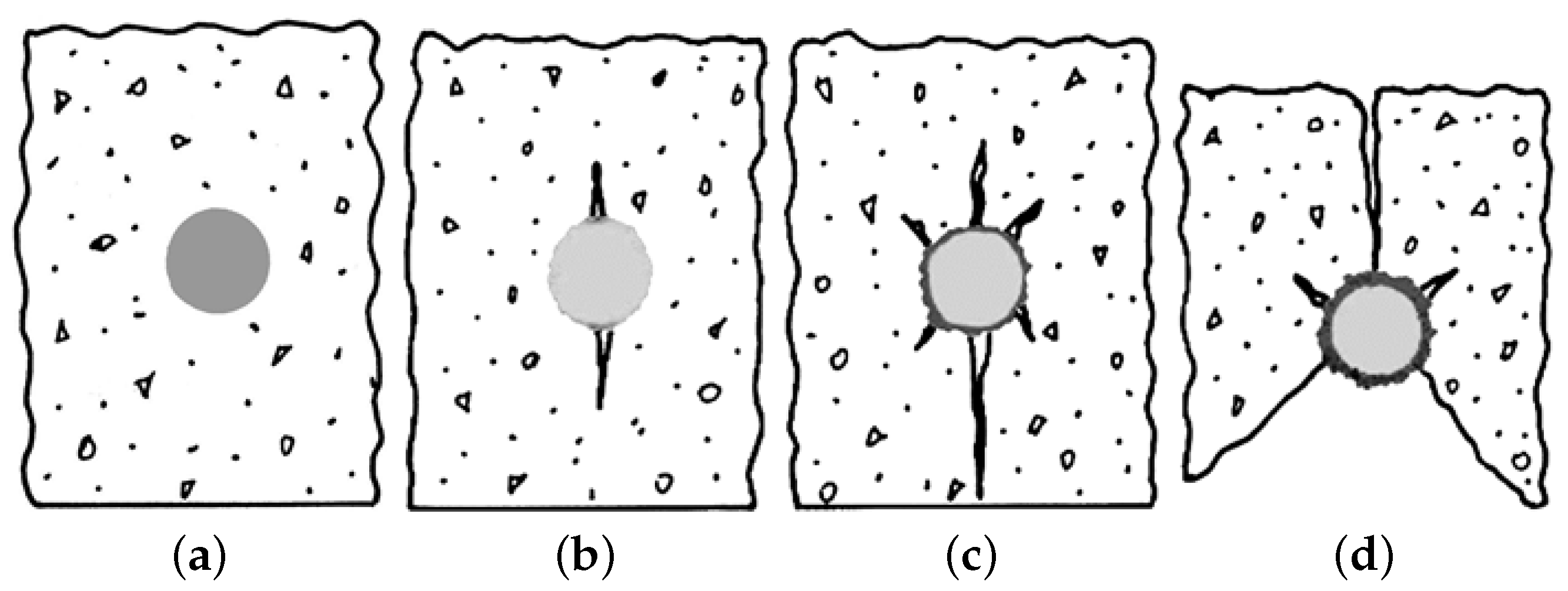
2.2. Corrosion Mechanism
3. Seismic Fragility Assessment of Structures
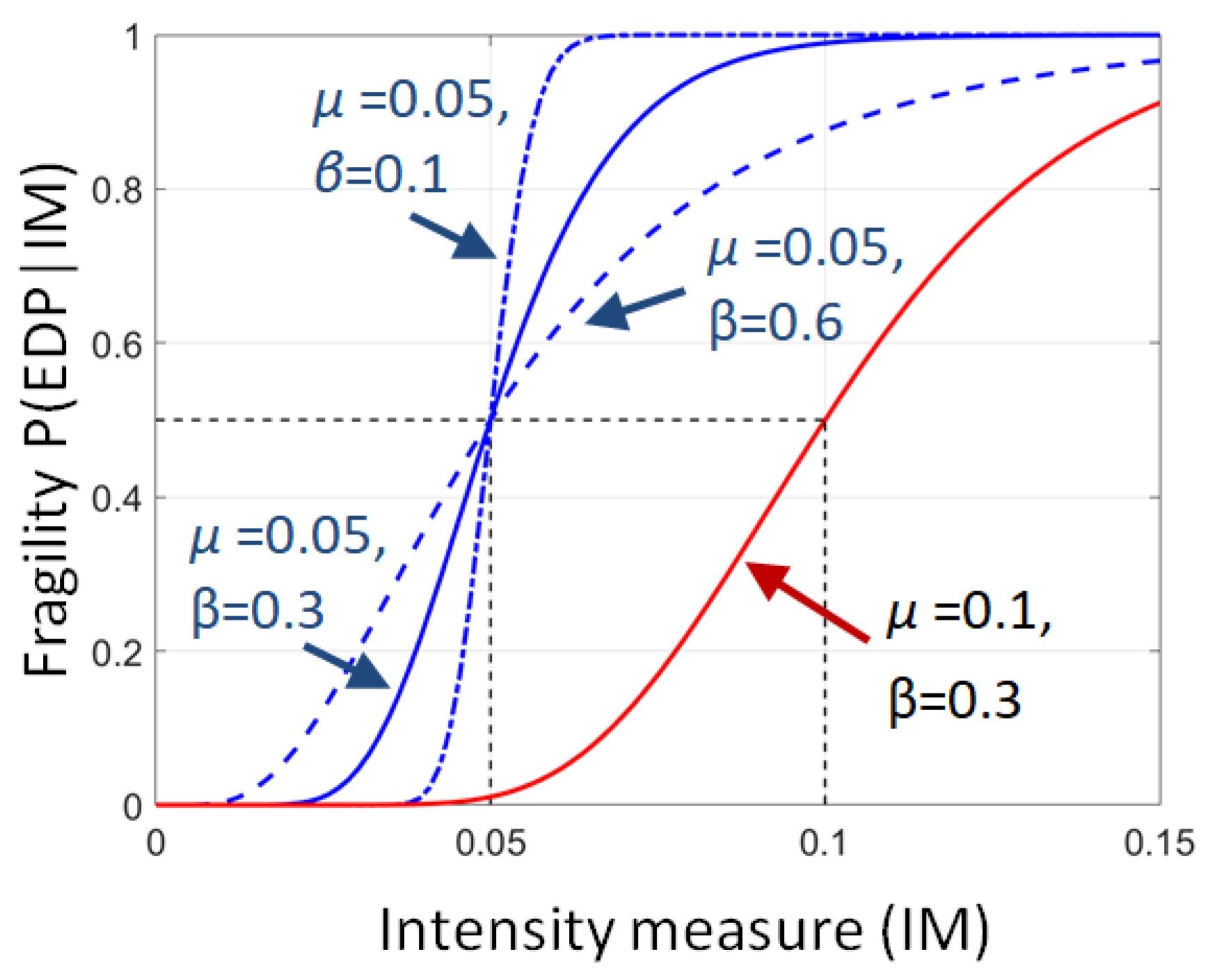
3.1. Fragility Analysis Methods
3.1.1. Multiple-Stripe Analysis
3.1.2. Cloud Analysis
3.1.3. Maximum Likelihood (MLE) Fitting
3.1.4. SPO2IDA Tool
4. Past Research on the Fragility Assessment of Corroded RC Structures
5. Corrosion Modelling
5.1. Initiation Time of Corrosion
5.2. Reduction of Rebar Diameter and Ductility
5.3. Reduction in the Concrete Cover Compressive Strength
6. Case Study
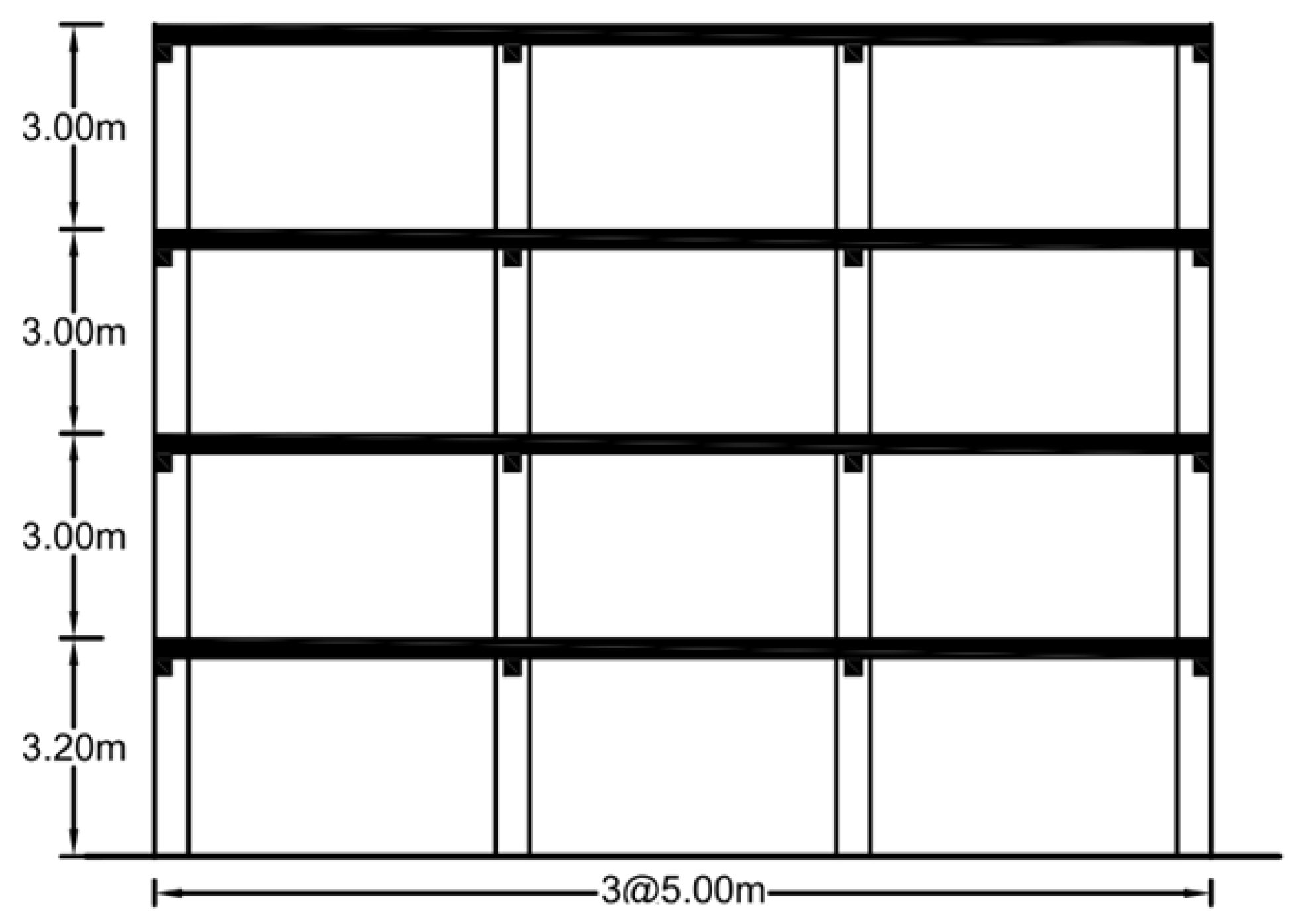
6.1. Four-Story RC Building under Corrosion
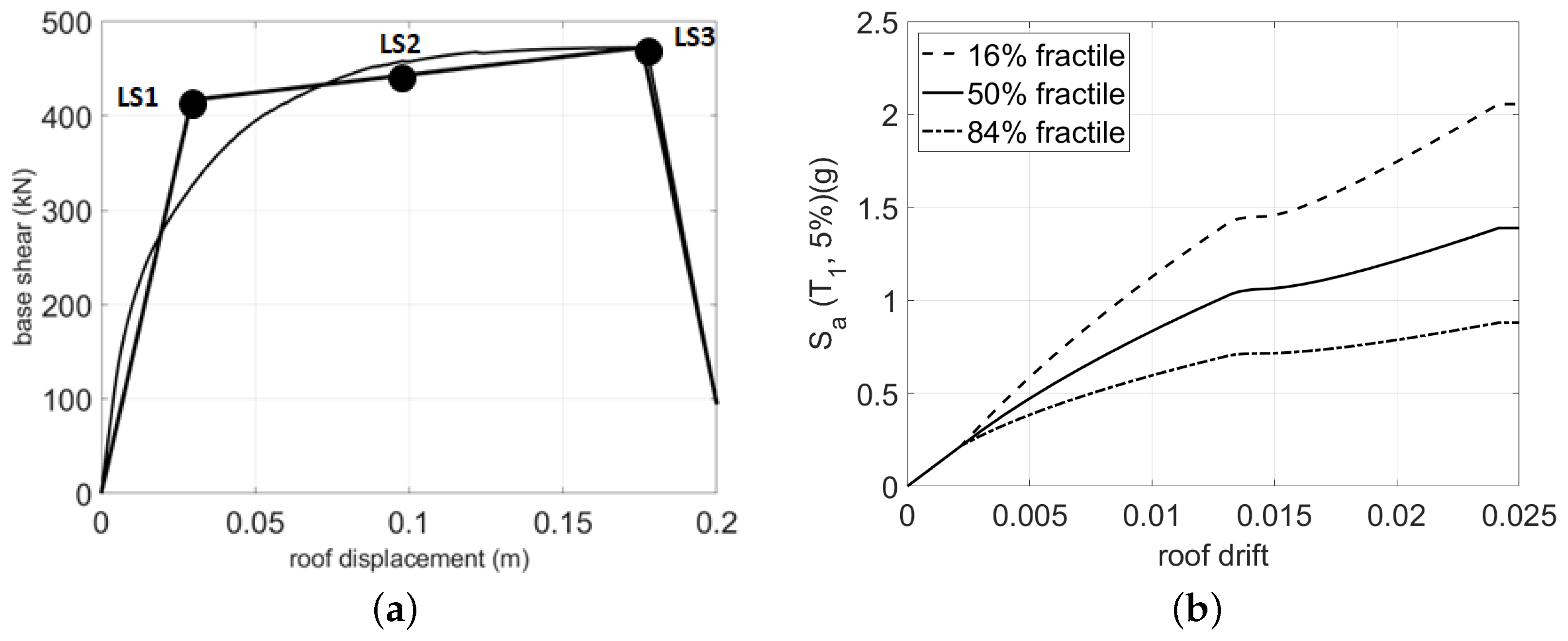
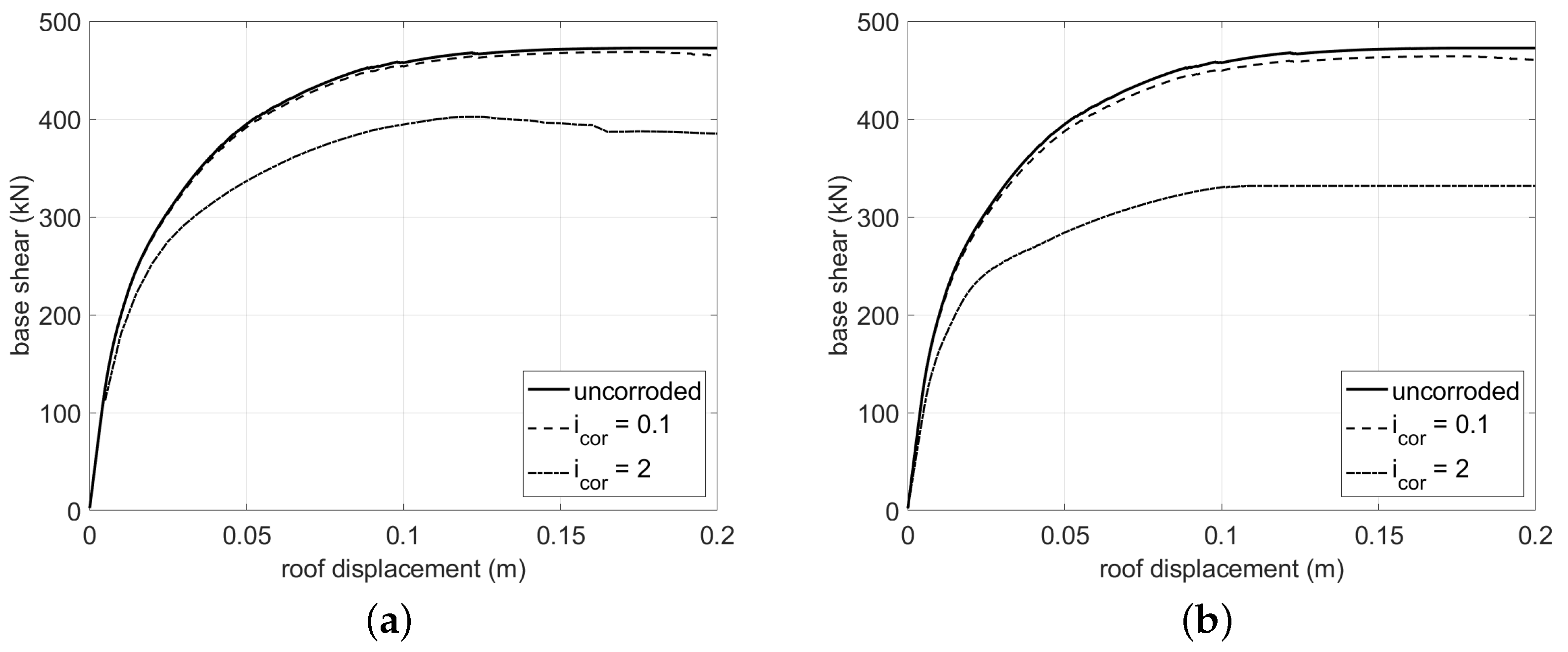
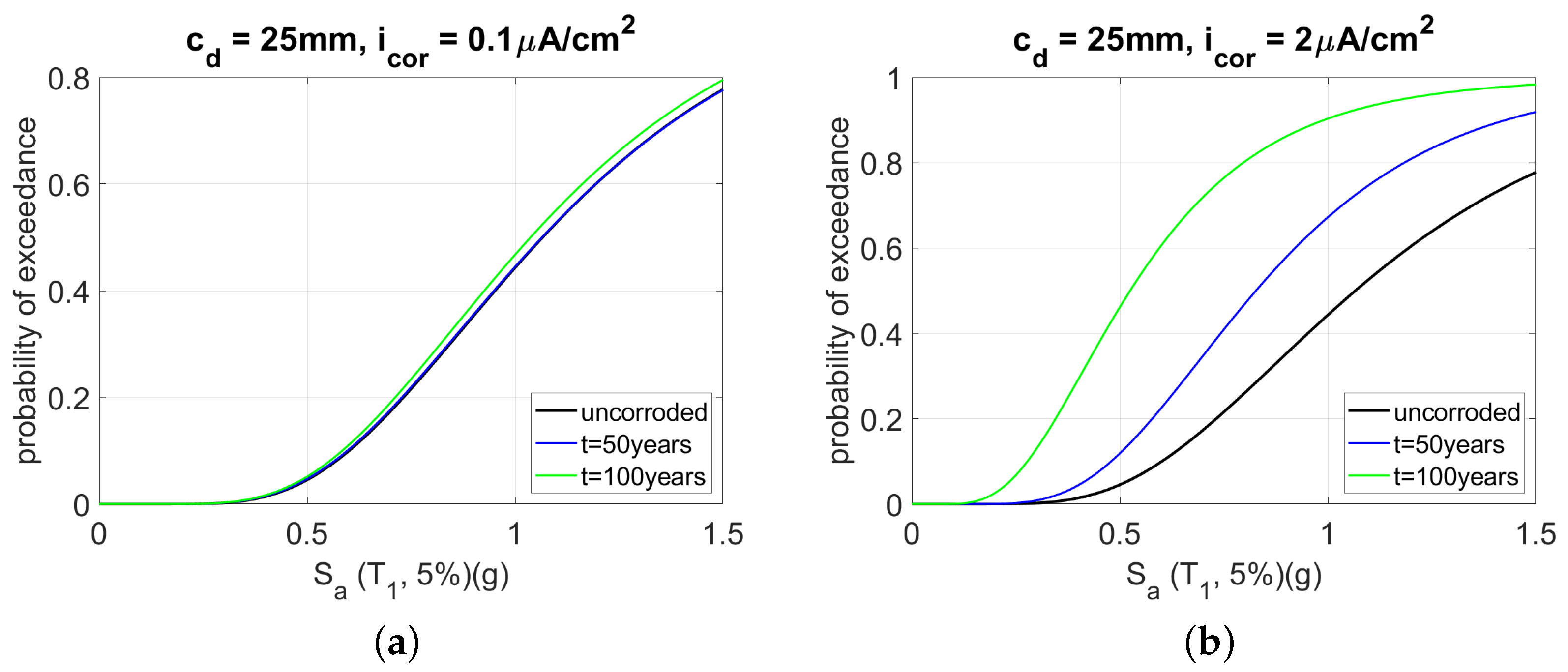
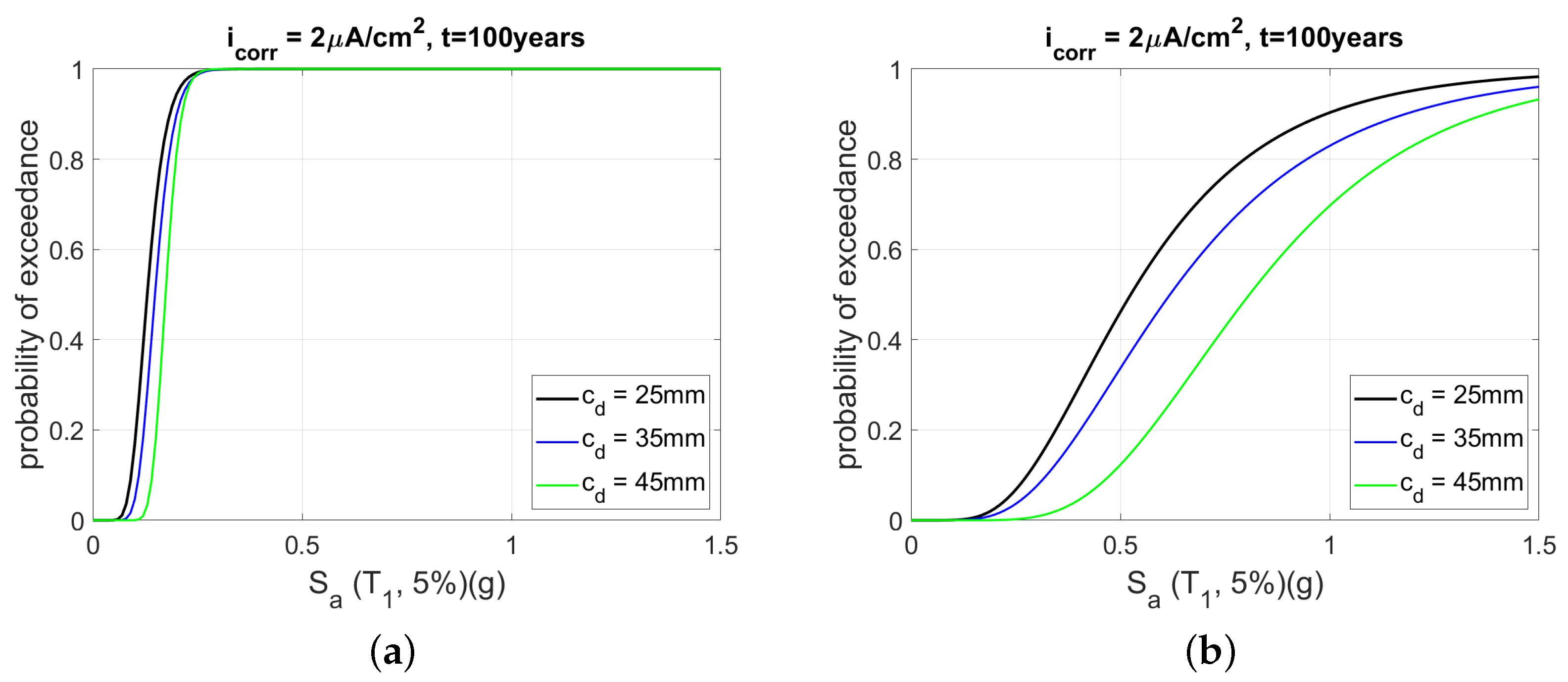
6.2. Numerical Results
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bertolini, L.; Elsener, B.; Pedeferri, P.; Polder, R.B. Corrosion of Steel in Concrete; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2003. [Google Scholar]
- Khan, M.; Deng, X.; Wang, Y. Service life prediction model for chloride-induced corrosion of concrete-steel tubular columns. J. Build. Eng. 2023, 74, 106761. [Google Scholar] [CrossRef]
- Qian, R.; Li, Q.; Fu, C.; Zhang, Y.; Wang, Y.; Jin, N.; Jin, X. Investigations on atmospheric carbonation corrosion of concrete structure beam exposed to real marine-environment for 7 years. J. Build. Eng. 2023, 71, 106517. [Google Scholar] [CrossRef]
- Zucca, M.; Crespi, P.; Stochino, F.; Puppio, M.L.; Coni, M. Maintenance interventions period of existing RC motorway viaducts located in moderate/high seismicity zones. Structures 2023, 47, 976–990. [Google Scholar] [CrossRef]
- Crespi, P.; Zucca, M.; Valente, M.; Longarini, N. Influence of corrosion effects on the seismic capacity of existing RC bridges. Eng. Fail. Anal. 2022, 140, 106546. [Google Scholar] [CrossRef]
- Böhni, H. Corrosion in Reinforced Concrete Structures; Woodhead Publishing Ltd.: Sawston, UK; CRC Press LLC: Boca Raton, FL, USA, 2005. [Google Scholar]
- Zhou, Y.; Gencturk, B.; Willam, K.; Attar, A. Carbonation-induced and chloride-induced corrosion in reinforced concrete structures. J. Mater. Civ. Eng. 2015, 27, 04014245. [Google Scholar] [CrossRef]
- Yin, D.; Jin, H.; Gu, X.; Yu, S.; Wang, Z. Influence of rubber geometrical characteristics on the corrosion behavior of rebar in rubberized concrete. J. Build. Eng. 2023, 77, 107535. [Google Scholar] [CrossRef]
- CP Technologies. Identifying and Treating Corrosion in Reinforced Concrete Structures. 2017. Available online: https://cp-tech.co.uk/identifying-and-treating-corrosion-in-reinforced-concrete-structures/ (accessed on 29 November 2022).
- Tuutti, K. Corrosion of Steel in Concrete; Swedish Cement and Concrete Research Institute: Stockholm, Sweden, 1982. [Google Scholar]
- Zheng, Y.; Zheng, S.-S.; Yang, L.; Dong, L.-G.; Ruan, S. Experimental study and numerical model of the seismic behavior of reinforced concrete beams in an artificial corrosion environment. J. Build. Eng. 2022, 46, 103705. [Google Scholar] [CrossRef]
- Schießl, P.; Bamforth, P.; Baroghel-Bouny, V.; Corley, G.; Faber, M.; Gehlen, C.; Helland, S.; Ishida, T.; Helene, P.; Markeset, G.; et al. Fib Bulletin 34: Model Code for Service Life Design; The International Federation for Structural Concrete: Lausanne, Switzerland, 2006. [Google Scholar]
- Jalayer, F. Direct Probabilistic Seismic Analysis: Implementing Non-Linear Dynamic Assessments. Ph.D. Thesis, Department of Civil and Environmental Engineering, Stanford University, Stanford, CA, USA, 2003. [Google Scholar]
- Fragiadakis, M.; Diamantopoulos, S. Fragility and risk assessment of freestanding building contents. Earthq. Eng. Struct. Dyn. 2020, 49, 1028–1048. [Google Scholar] [CrossRef]
- Fragiadakis, M.; Vamvatsikos, D. Fast performance uncertainty estimation via pushover and approximate IDA. Earthq. Eng. Struct. Dyn. 2010, 39, 683–703. [Google Scholar] [CrossRef]
- Karapetrou, S.; Fotopoulou, S.; Pitilakis, K. Seismic vulnerability of RC buildings under the effect of ageing. Procedia Environ. Sci. 2017, 38, 461–468. [Google Scholar] [CrossRef]
- Geng, F.; Wen, Z.; Xu, C.; Chau, K. Time-dependent seismic fragility for ageing RC frame structures in the inland atmosphere environment. J. Earthq. Eng. 2023, 27, 1440–1457. [Google Scholar] [CrossRef]
- Dizaj, E.A.; Madandoust, R.; Kashani, M.M. Probabilistic seismic vulnerability analysis of corroded reinforced concrete frames including spatial variability of pitting corrosion. Soil Dyn. Earthq. Eng. 2018, 114, 97–112. [Google Scholar] [CrossRef]
- Dizaj, E.A.; Salami, M.R.; Kashani, M.M. Seismic vulnerability assessment of ageing reinforced concrete structures under real mainshock-aftershock ground motions. Struct. Infrastruct. Eng. 2022, 18, 1674–1690. [Google Scholar] [CrossRef]
- Dizaj, E.A.; Kashani, M.M. Nonlinear structural performance and seismic fragility of corroded reinforced concrete structures: Modelling guidelines. Eur. J. Environ. Civ. Eng. 2022, 26, 5374–5403. [Google Scholar] [CrossRef]
- Yu, X.-H.; Dai, K.-Y.; Li, Y.-S. Variability in corrosion damage models and its effect on seismic collapse fragility of ageing reinforced concrete frames. Constr. Build. Mater. 2021, 295, 123654. [Google Scholar] [CrossRef]
- Yalciner, H.; Sensoy, S.; Eren, O. Seismic performance assessment of a corroded 50-year-old reinforced concrete building. J. Struct. Eng. 2015, 141, 05015001. [Google Scholar] [CrossRef]
- Karapetrou, S.; Manakou, M.; Bindi, D.; Petrovic, B.; Pitilakis, K. “Time-building specific” seismic vulnerability assessment of a hospital RC building using field monitoring data. Eng. Struct. 2016, 112, 114–132. [Google Scholar] [CrossRef]
- Couto, R.; Requena-García-Cruz, M.V.; Bento, R.; Morales-Esteban, A. Seismic capacity and vulnerability assessment considering ageing effects: Case study—Three local Portuguese RC buildings. Bull. Earthq. Eng. 2021, 19, 6591–6614. [Google Scholar] [CrossRef]
- Evangelisti, L.; Guattari, C.; Fontana, L.; De Lieto Vollaro, R.; Asdrubali, F. On the ageing and weathering effects in assembled modular facades: On-site experimental measurements in an Italian building of the 1960s. J. Build. Eng. 2022, 45, 103519. [Google Scholar] [CrossRef]
- Tomás, A.; Ródenas, J.L.; García-Ayllón, S. Proposal for new values of behaviour modifiers for seismic vulnerability evaluation of reinforced concrete buildings applied to Lorca (Spain) using damage data from the 2011 earthquake. Bull. Earthq. Eng. 2017, 15, 3943–3962. [Google Scholar] [CrossRef]
- Di Sarno, L.; Pugliese, F. Seismic fragility of existing RC buildings with corroded bars under earthquake sequences. Soil Dyn. Earthq. Eng. 2020, 134, 106169. [Google Scholar] [CrossRef]
- Di Sarno, L.; Pugliese, F. Effects of mainshock-aftershock sequences on fragility analysis of RC buildings with ageing. Eng. Struct. 2021, 232, 111837. [Google Scholar] [CrossRef]
- Pugliese, F.; Di Sarno, L. Probabilistic structural performance of RC frames with corroded smooth bars subjected to near- and far-field ground motions. J. Build. Eng. 2022, 49, 104008. [Google Scholar] [CrossRef]
- Lindvall, A. DuraCrete, Models for Environmental Actions on Concrete Structures: DuraCrete, Probabilistic Performance Based Durability Design of Concrete Structures; CUR: Gouda, The Netherlands, 1999. [Google Scholar]
- Edvardsen, C.; Engelund, S.; Mohr, L. DuraCrete, General Guidelines for Durability Design and Redesign: DuraCrete—Probabilistic Performance Based Durability Design of Concrete Structures (Contract BRPR-CT95-0132, Project BE95-1347); CUR: Gouda, The Netherlands, 2000. [Google Scholar]
- Visser, J. DuraCrete, Statistical Quantification of the Variables in the Limit State Functions: DuraCrete, Probabilistic Performance Based Durability Design of Concrete Structures; CUR: Gouda, The Netherlands, 2000. [Google Scholar]
- Sascha, L.; Schießl, P.; Cairns, J. Life Cycle Management of Concrete Infrastructures for Improved Sustainability, Technical Report No. D 3.2. Probabilistic Service Life Models for Reinforced Concrete Structures; Transportation Research Board: Washington, DC, USA, 2003. [Google Scholar]
- EN 1992-1-1; Eurocode 2: Design of Concrete Structures-Part 1-1: General Rules and Rules for Buildings. CEN: Brussels, Belgium, 2005.
- Ghosh, J.; Padgett, J.E. ageing considerations in the development of time-dependent seismic fragility curves. J. Struct. Eng. 2010, 136, 1497–1511. [Google Scholar] [CrossRef]
- Berto, L.; Vitaliani, R.; Saetta, A.; Simioni, P. Seismic assessment of existing RC structures affected by degradation phenomena. Struct. Saf. 2009, 31, 284–297. [Google Scholar] [CrossRef]
- Jesús, R.; Andrade, C. CONTECVET: A Validated Users Manual for Assessing the Residual Service Life of Concrete Structures; eC Innovation project IN30902I; Division of Building Materials, LTH, Lund University: Lund, Sweden, 2001; Volume 7161. [Google Scholar]
- Coronelli, D.; Gambarova, P. Structural assessment of corroded reinforced concrete beams: Modeling guidelines. J. Struct. Eng. 2004, 130, 1214–1224. [Google Scholar] [CrossRef]
- Simioni, P. Seismic Response of Reinforced Concrete Structures Affected by Reinforcement Corrosion. Ph.D. Thesis, Faculty of Engineering, University of Florence, Florence, Italy, 2009. [Google Scholar]
- McKenna, F.; Fenves, G.L.; Scott, M.H. Opensees (Open System for Earthquake Engineering Simulation); University of California: Berkeley, CA, USA, 2000. [Google Scholar]
- Ditommaso, R.; Iacovino, C.; Auletta, G.; Parolai, S.; Ponzo, F.C. Damage Detection and Localization on Real Structures Subjected to Strong Motion Earthquakes Using the Curvature Evolution Method: The Navelli (Italy) Case Study. Appl. Sci. 2021, 11, 6496. [Google Scholar] [CrossRef]
- Cataldo, A.; Roselli, I.; Fioriti, V.; Saitta, F.; Colucci, A.; Tatì, A.; Ponzo, F.C.; Ditommaso, R.; Mennuti, C.; Marzani, A. Advanced Video-Based Processing for Low-Cost Damage Assessment of Buildings under Seismic Loading in Shaking Table Tests. Sensors 2023, 23, 5303. [Google Scholar] [CrossRef]






| Paper | t | LS1 | LS2 | LS3 | |||
|---|---|---|---|---|---|---|---|
| Years | Min | Max | Min | Max | Min | Max | |
| Karapetrou et al. [16] | 50 | - | - | 0.17 | 0.24 | - | - |
| Dizaj et al. [20] | 40 | 0.15 | 0.17 | 0.49 | 0.52 | 0.44 | 0.49 |
| Yalciner et al. [22] | 50 | 0.25 | 0.25 | 0.15 | 0.15 | 0.41 | 0.41 |
| Couto et al. [24] | 40–60 | 0.11 | 0.17 | - | - | - | - |
| Karapetrou et al. [23] | 45 | 0.013 | 0.15 | 0.18 | 0.36 | - | - |
| Pugliese and DiSarno [29] | 50 | 0.25 | 0.63 | 0.28 | 0.61 | 0.28 | 0.55 |
| Paper | CL | LS1 | LS2 | LS3 | |||
|---|---|---|---|---|---|---|---|
| % | Min | Max | Min | Max | Min | Max | |
| Dizaj et al. [18] | 10 | - | - | 0.525 | 0.526 | - | - |
| DiSarno & Pugliese [27] | 10 | 0.44 | 0.448 | 0.4 | 0.412 | - | - |
| DiSarno & Pugliese [28] | 10 | 0.368 | 0.411 | 0.22 | 0.278 | 0 | 0 |
| Parameter | Mean | COV | Distribution |
|---|---|---|---|
| 0.676 | 0.17 | Gamma | |
| 1.25 | 0.28 | Normal | |
| (m2/s) | 1.58 × 10−11 | 0.2 | Normal |
| (years) | 0.0767 | - | Deterministic |
| n | 0.362 | 0.677 | Beta |
| (wt% cement) | 0.6 | 0.25 | Beta |
| (wt% cement) | 1.2825 | 0.35 | Normal |
| t | LS1 | LS2 | LS3 | ||||
|---|---|---|---|---|---|---|---|
| Years | mm | Min | Max | Min | Max | Min | Max |
| 50 | 25 | 0.00 | 0.21 | 0.00 | 0.22 | 0.00 | 0.22 |
| 35 | 0.00 | 0.09 | 0.00 | 0.03 | 0.00 | 0.04 | |
| 45 | 0.00 | 0.04 | 0.00 | 0.05 | 0.00 | 0.06 | |
| 100 | 25 | 0.00 | 0.40 | 0.00 | 0.49 | 0.00 | 0.51 |
| 35 | 0.00 | 0.31 | 0.00 | 0.35 | 0.00 | 0.37 | |
| 45 | 0.00 | 0.18 | 0.00 | 0.19 | 0.00 | 0.19 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diamantopoulos, S.; Achmet, Z.; Stefanidou, S.; Markogiannaki, O.; Fragiadakis, M. Seismic Fragility Curves of RC Buildings Subjected to Aging. GeoHazards 2024, 5, 192-208. https://doi.org/10.3390/geohazards5010010
Diamantopoulos S, Achmet Z, Stefanidou S, Markogiannaki O, Fragiadakis M. Seismic Fragility Curves of RC Buildings Subjected to Aging. GeoHazards. 2024; 5(1):192-208. https://doi.org/10.3390/geohazards5010010
Chicago/Turabian StyleDiamantopoulos, Spyridon, Zeinep Achmet, Sotiria Stefanidou, Olga Markogiannaki, and Michalis Fragiadakis. 2024. "Seismic Fragility Curves of RC Buildings Subjected to Aging" GeoHazards 5, no. 1: 192-208. https://doi.org/10.3390/geohazards5010010
APA StyleDiamantopoulos, S., Achmet, Z., Stefanidou, S., Markogiannaki, O., & Fragiadakis, M. (2024). Seismic Fragility Curves of RC Buildings Subjected to Aging. GeoHazards, 5(1), 192-208. https://doi.org/10.3390/geohazards5010010








