Analytical and Numerical Thermodynamic Equilibrium Simulations of Steam Methane Reforming: A Comparison Study
Abstract
1. Introduction
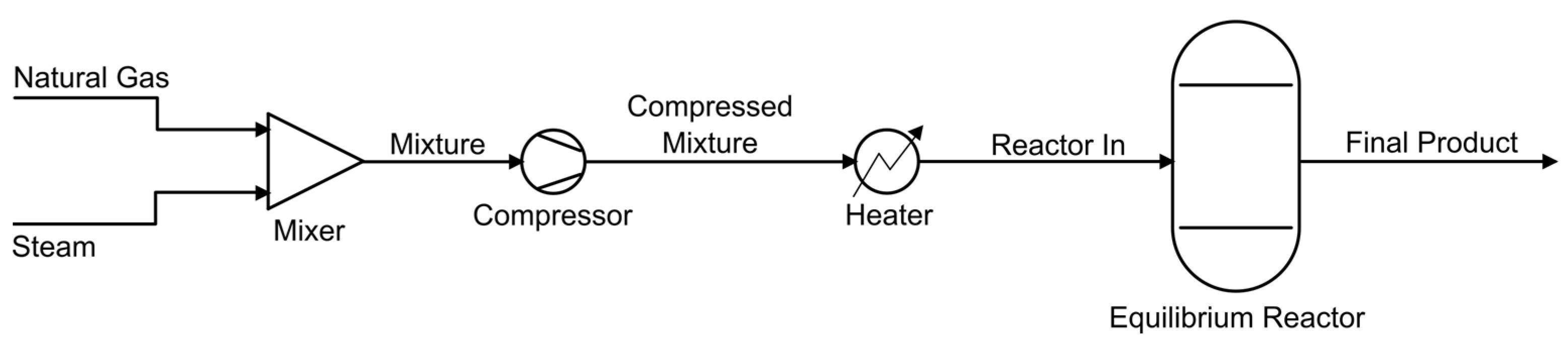
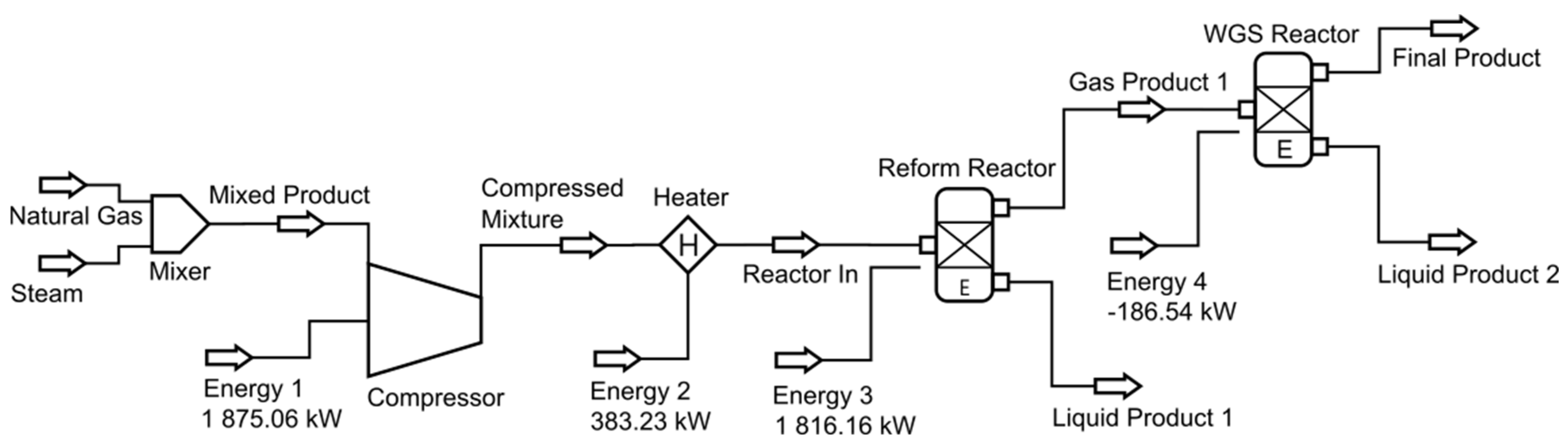
2. Materials and Methods
- Reform reaction:
- WGS reaction:
3. Results and Discussion
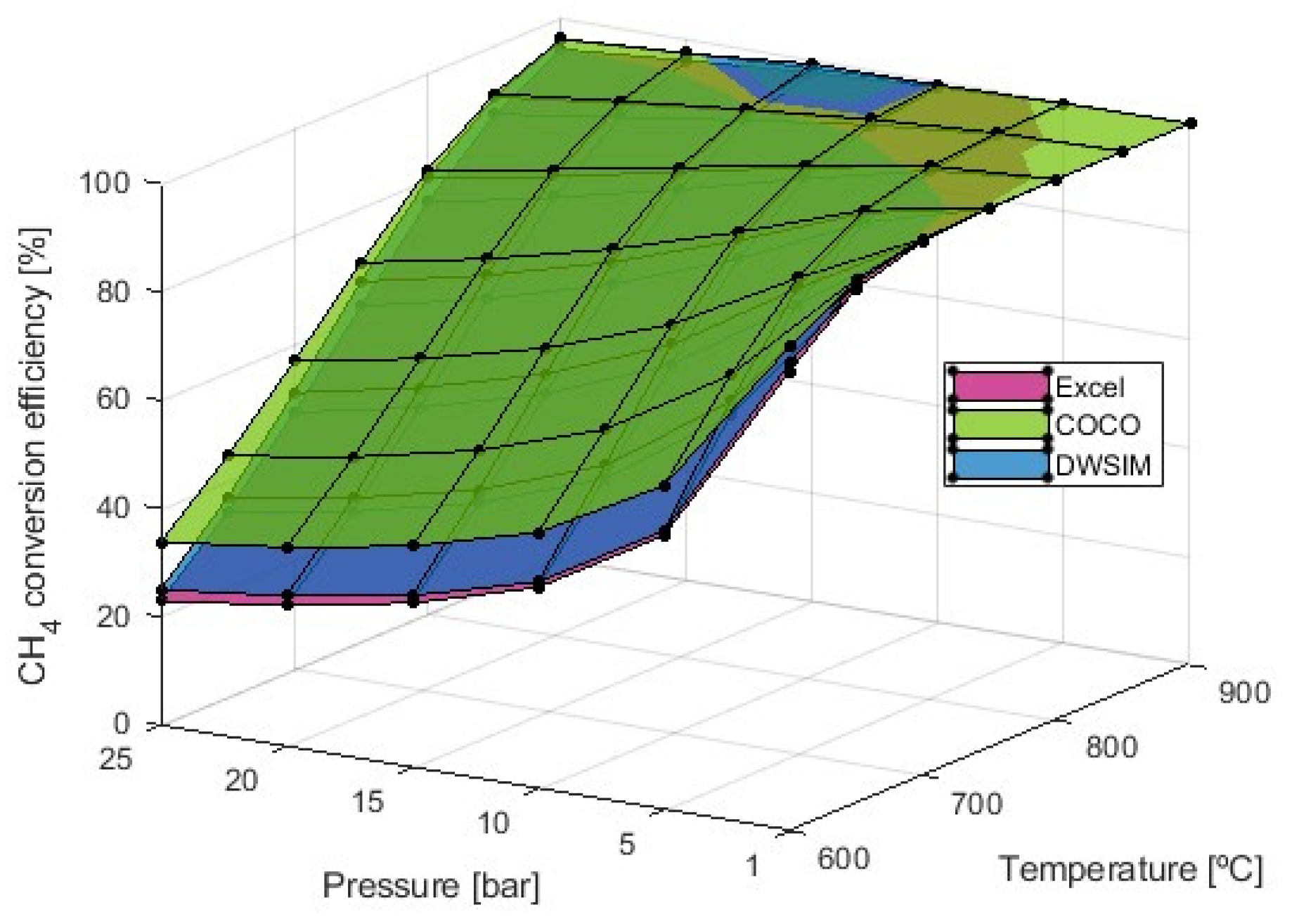
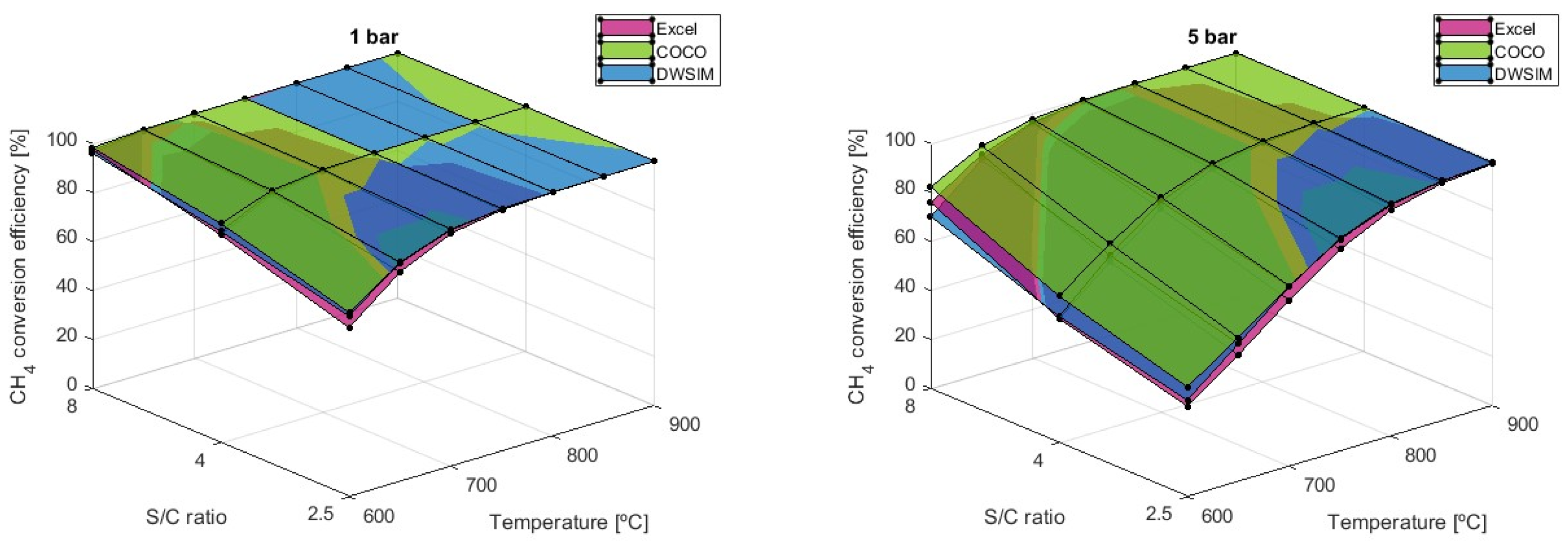
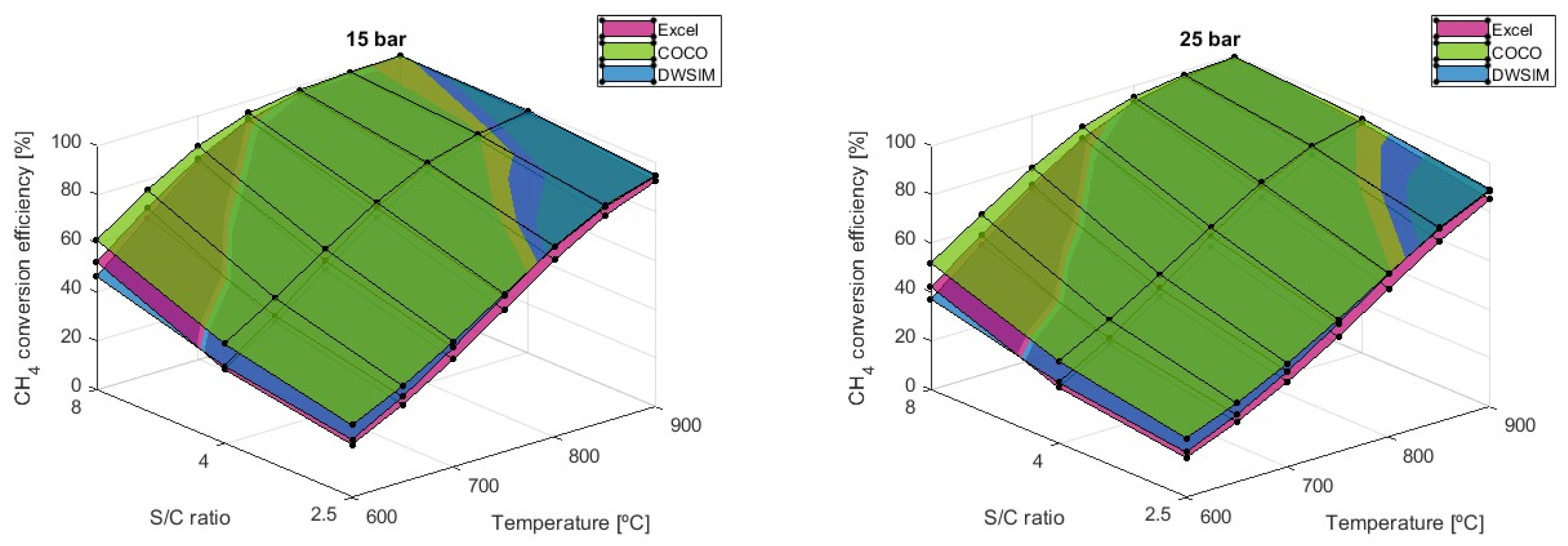
3.1. Hydrogen Production
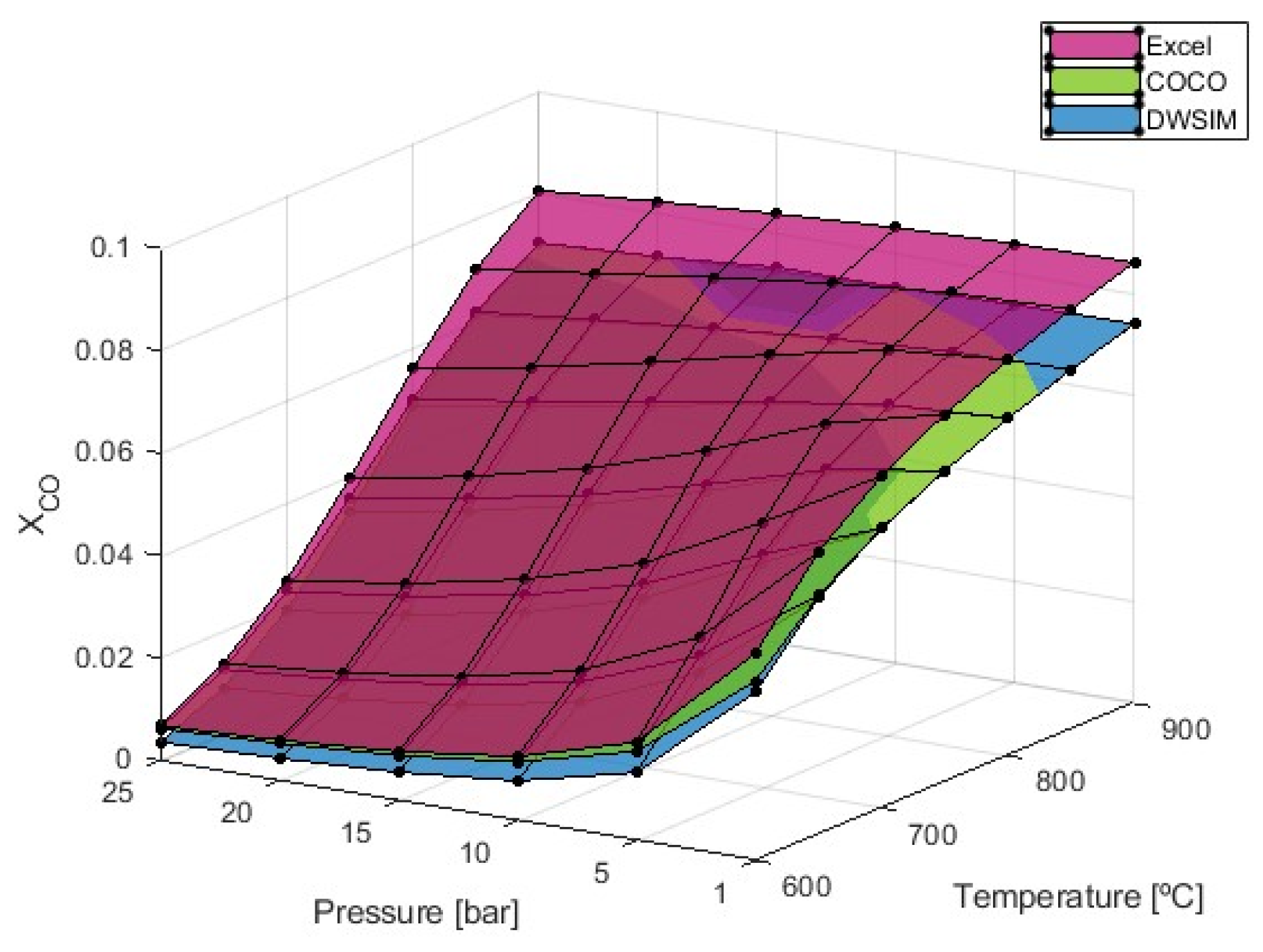
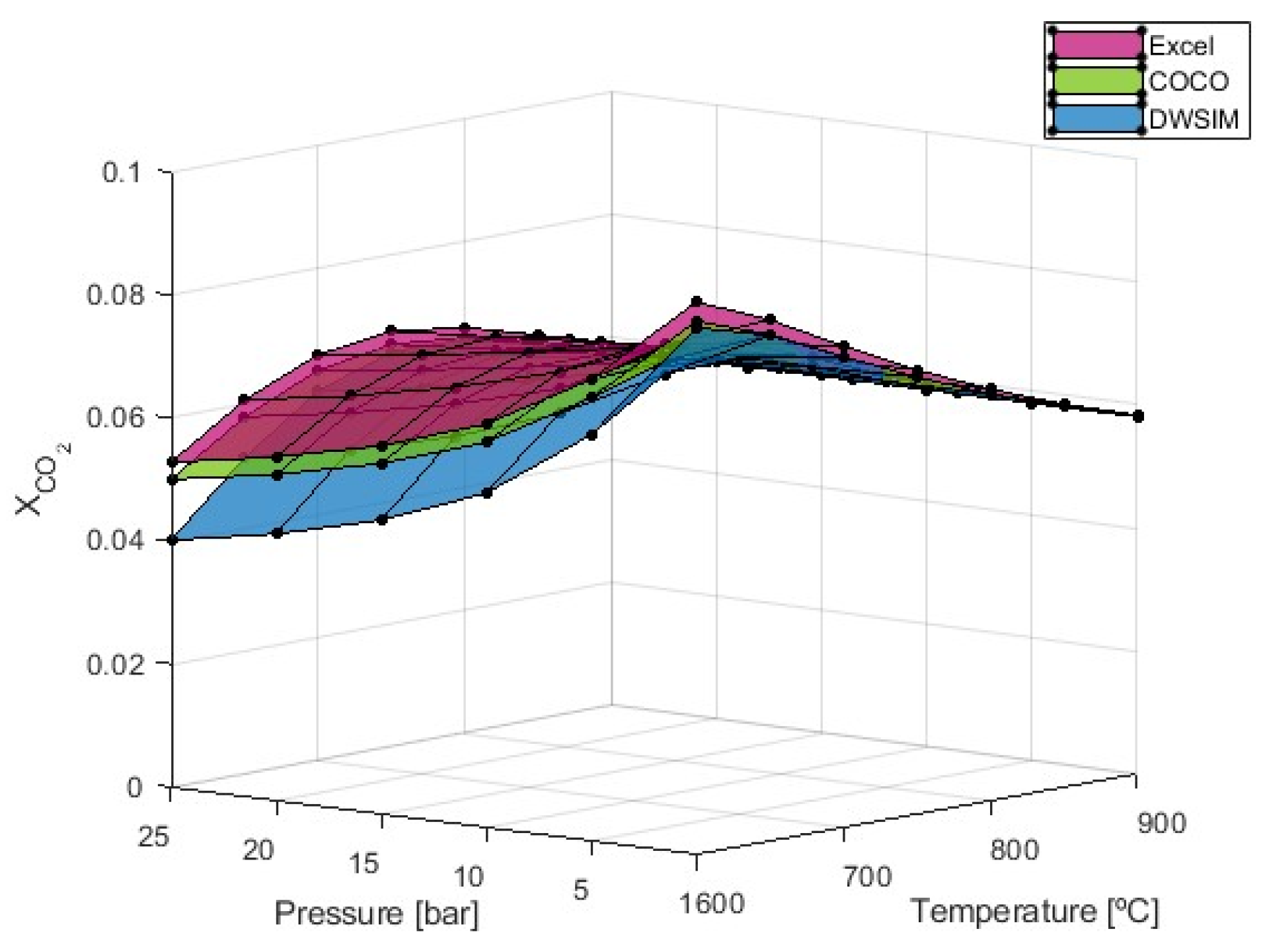
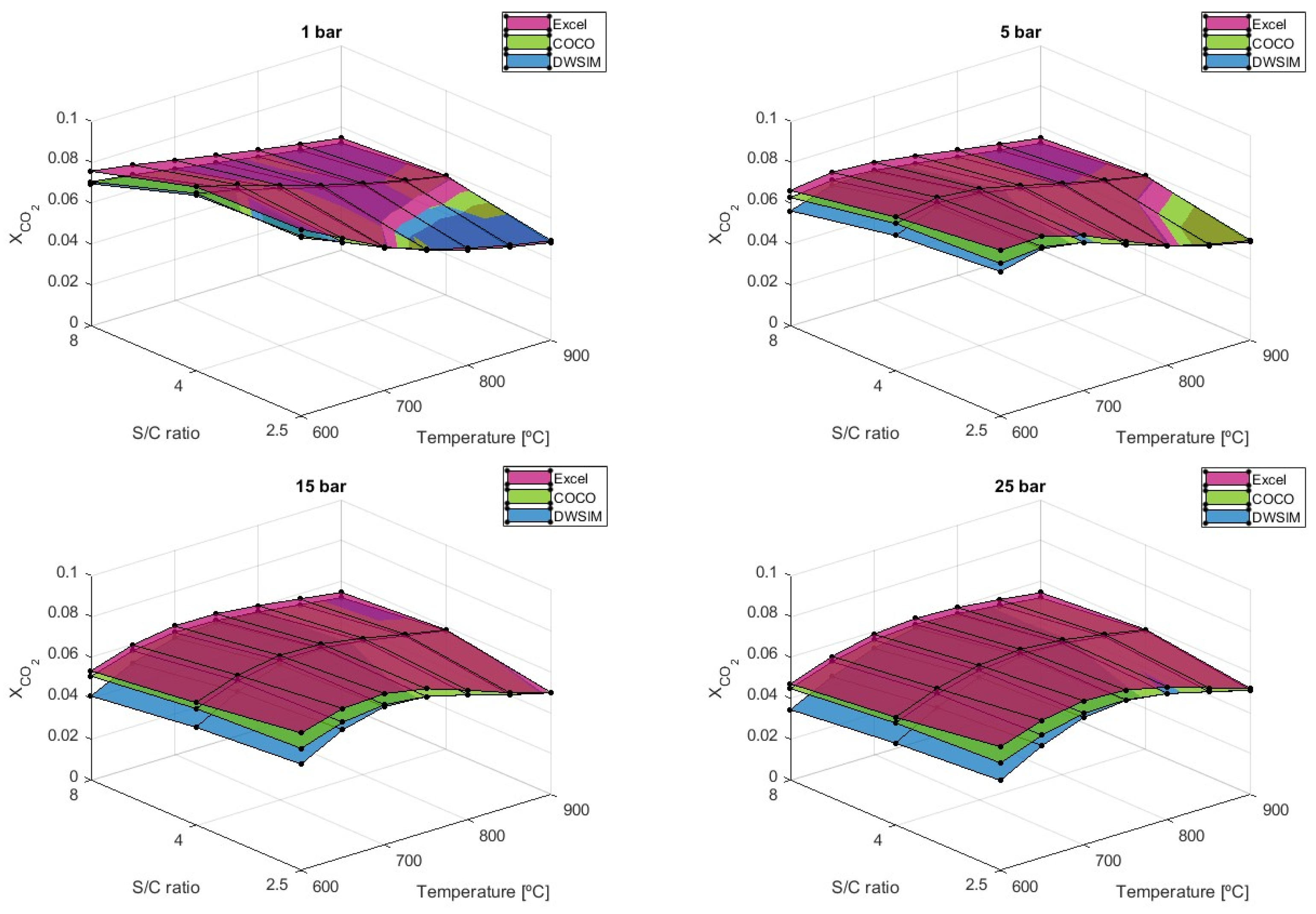
3.2. Production of Carbonaceous Products
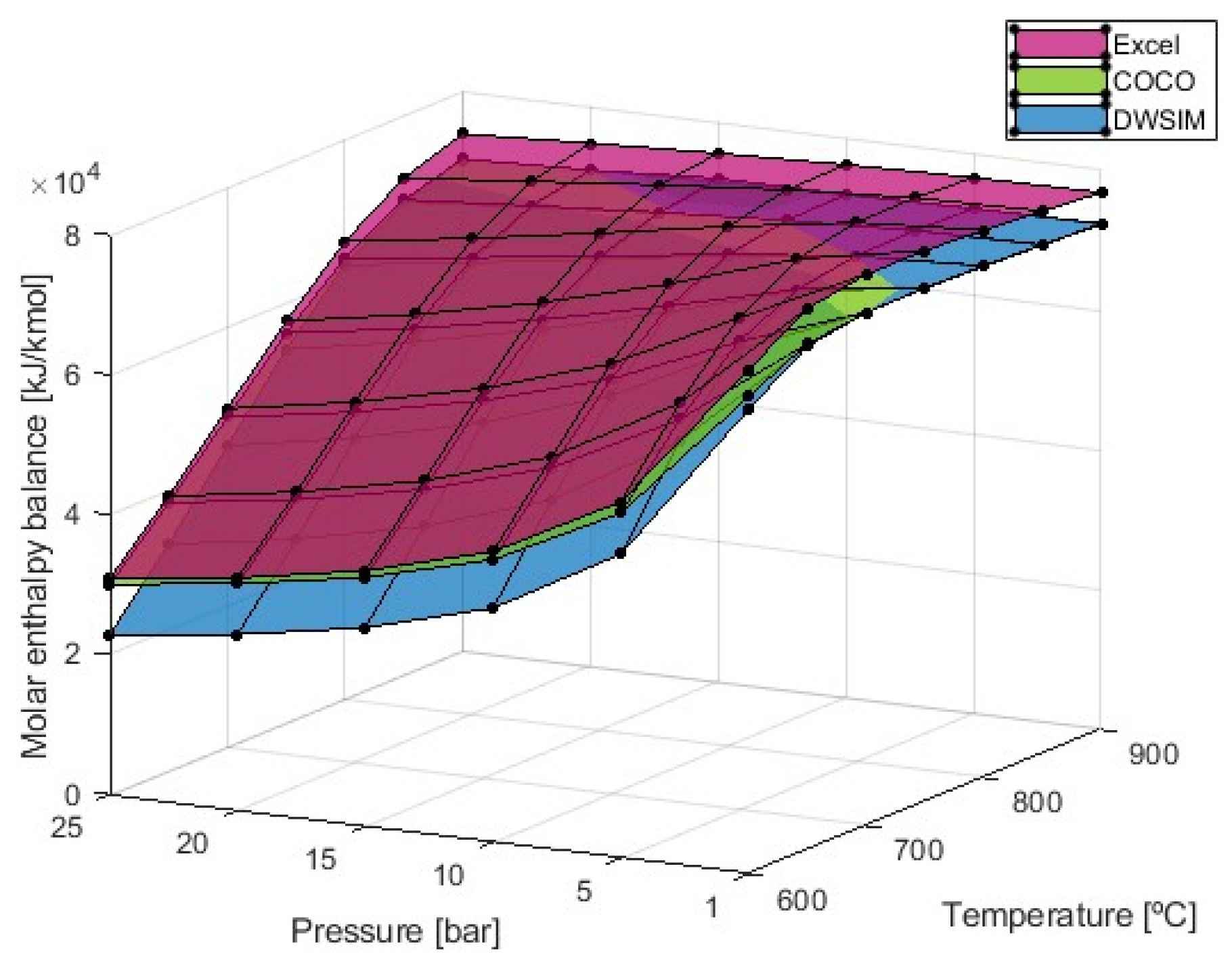
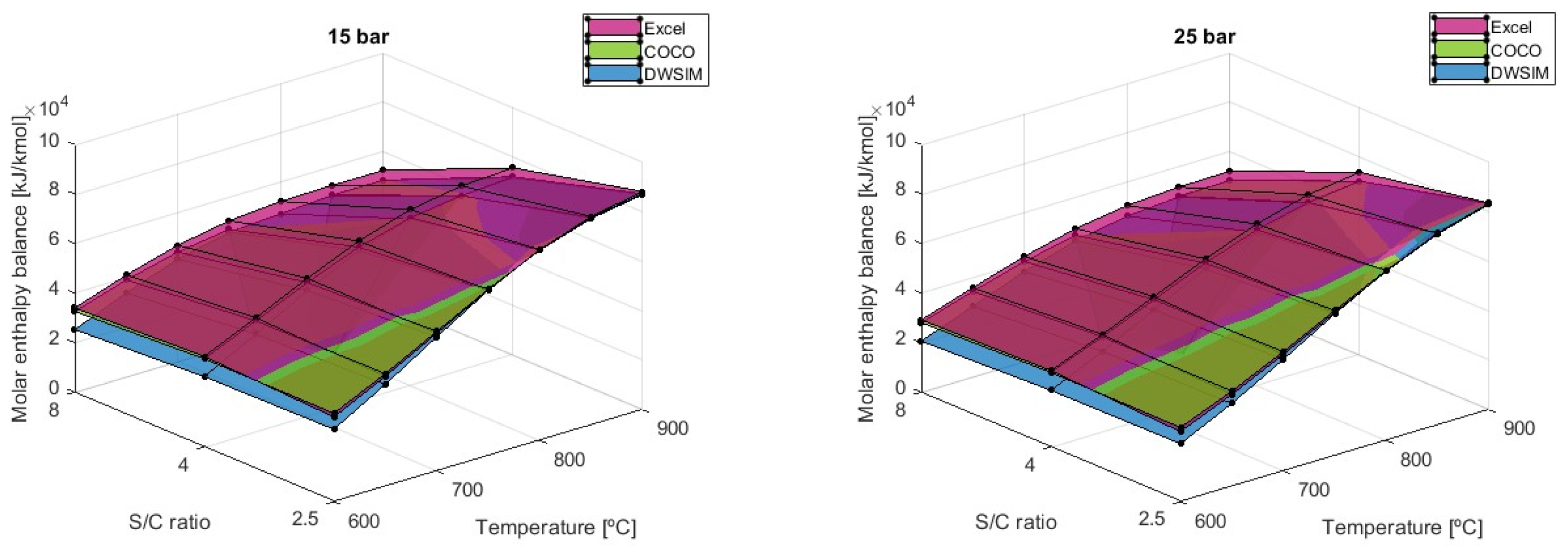
3.3. Heat Balance
3.4. Steam-to-Carbon Ratio Study (S/C)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- De Medeiros, F.G.M.; Lopes, F.W.B.; de Vasconcelos, B.R. Prospects and Technical Challenges in Hydrogen Production through Dry Reforming of Methane. Catalysts 2022, 12, 363. [Google Scholar] [CrossRef]
- Haryanto, A.; Fernando, S.; Murali, N.; Adhikari, S. Current Status of Hydrogen Production Techniques by Steam Reforming of Ethanol: A Review. Energy Fuels 2005, 19, 2098–2106. [Google Scholar] [CrossRef]
- Rosen, M.A.; Koohi-Fayegh, S. The prospects for hydrogen as an energy carrier: An overview of hydrogen energy and hydrogen energy systems. Energy Ecol. Environ. 2016, 1, 10–29. [Google Scholar] [CrossRef]
- Elam, C.C.; Padró, C.E.; Sandrock, G.; Luzzi, A.; Lindblad, P.; Hagen, E.F. Realizing the hydrogen future: The International Energy Agency’s efforts to advance hydrogen energy technologies. Int. J. Hydrogen Energy 2003, 28, 601–607. [Google Scholar] [CrossRef]
- Cipriani, G.; Di Dio, V.; Genduso, F.; La Cascia, D.; Liga, R.; Miceli, R.; Galluzzo, G.R. Perspective on hydrogen energy carrier and its automotive applications. Int. J. Hydrogen Energy 2014, 39, 8482–8494. [Google Scholar] [CrossRef]
- Simpson, A.P.; Lutz, A.E. Exergy analysis of hydrogen production via steam methane reforming. Int. J. Hydrogen Energy 2007, 32, 4811–4820. [Google Scholar] [CrossRef]
- Lee, S.; Kim, H.S.; Park, J.; Kang, B.M.; Cho, C.-H.; Lim, H.; Won, W. Scenario-Based Techno-Economic Analysis of Steam Methane Reforming Process for Hydrogen Production. Appl. Sci. 2021, 11, 6021. [Google Scholar] [CrossRef]
- Wang, J.; Liu, Z.; Ji, C.; Liu, L. Heat Transfer and Reaction Characteristics of Steam Methane Reforming in a Novel Composite Packed Bed Microreactor for Distributed Hydrogen Production. Energies 2023, 16, 4347. [Google Scholar] [CrossRef]
- Richter, J.; Rachow, F.; Israel, J.; Roth, N.; Charlafti, E.; Günther, V.; Flege, J.I.; Mauss, F. Reaction Mechanism Development for Methane Steam Reforming on a Ni/Al2O3 Catalyst. Catalysts 2023, 13, 884. [Google Scholar] [CrossRef]
- Shagdar, E.; Lougou, B.G.; Shuai, Y.; Ganbold, E.; Chinonso, O.P.; Tan, H. Process analysis of solar steam reforming of methane for producing low-carbon hydrogen. RSC Adv. 2020, 10, 12582–12597. [Google Scholar] [CrossRef] [PubMed]
- Van Beurden, P. On the Catalytic Aspects of Steam-Methane Reforming; Technical Report I04-003; Energy Research Centre of the Netherlands (ECN): Amsterdam, The Netherlands, 2004. [Google Scholar]
- Hou, K.; Hughes, R. The kinetics of methane steam reforming over a Ni/α-Al2O catalyst. Chem. Eng. J. 2001, 82, 311–328. [Google Scholar] [CrossRef]
- Barnoon, P.; Toghraie, D.; Mehmandoust, B.; Fazilati, M.A.; Eftekhari, S.A. Comprehensive study on hydrogen production via propane steam reforming inside a reactor. Energy Rep. 2021, 7, 929–941. [Google Scholar] [CrossRef]
- Ramantani, T.; Evangeliou, V.; Kormentzas, G.; Kondarides, D.I. Hydrogen production by steam reforming of propane and LPG over supported metal catalysts. Appl. Catal. B Environ. 2022, 306, 121129. [Google Scholar] [CrossRef]
- Stefanidis, G.D.; Vlachos, D.G. Intensification of steam reforming of natural gas: Choosing combustible fuel and reforming catalyst. Chem. Eng. Sci. 2010, 65, 398–404. [Google Scholar] [CrossRef]
- Jeong, H.; Kang, M. Hydrogen production from butane steam reforming over Ni/Ag loaded MgAl2O4 catalyst. Appl. Catal. B Environ. 2010, 95, 446–455. [Google Scholar] [CrossRef]
- Avcı, A.K.; Trimm, D.L.; Aksoylu, A.; Önsan, Z. Hydrogen production by steam reforming of n-butane over supported Ni and Pt-Ni catalysts. Appl. Catal. A Gen. 2004, 258, 235–240. [Google Scholar] [CrossRef]
- Sá, S.; Silva, H.; Brandão, L.; Sousa, J.M.; Mendes, A. Catalysts for methanol steam reforming—A review. Appl. Catal. B Environ. 2010, 99, 43–57. [Google Scholar] [CrossRef]
- Iulianelli, A.; Ribeirinha, P.; Mendes, A.; Basile, A. Methanol steam reforming for hydrogen generation via conventional and membrane reactors: A review. Renew. Sustain. Energy Rev. 2014, 29, 355–368. [Google Scholar] [CrossRef]
- Contreras, J.; Salmones, J.; Colín-Luna, J.; Nuño, L.; Quintana, B.; Córdova, I.; Zeifert, B.; Tapia, C.; Fuentes, G. Catalysts for H2 production using the ethanol steam reforming (a review). Int. J. Hydrogen Energy 2014, 39, 18835–18853. [Google Scholar] [CrossRef]
- Kim, T.W.; Park, J.C.; Lim, T.-H.; Jung, H.; Chun, D.H.; Lee, H.T.; Hong, S.; Yang, J.-I. The kinetics of steam methane reforming over a Ni/γ-Al2O3 catalyst for the development of small stationary reformers. Int. J. Hydrogen Energy 2015, 40, 4512–4518. [Google Scholar] [CrossRef]
- Kokka, A.; Petala, A.; Panagiotopoulou, P. Support Effects on the Activity of Ni Catalysts for the Propane Steam Reforming Reaction. Nanomaterials 2021, 11, 1948. [Google Scholar] [CrossRef]
- Christensen, K.; Chen, D.; Lødeng, R.; Holmen, A. Effect of supports and Ni crystal size on carbon formation and sintering during steam methane reforming. Appl. Catal. A Gen. 2006, 314, 9–22. [Google Scholar] [CrossRef]
- Zalazar-Garcia, D.; Fernandez, A.; Rodriguez-Ortiz, L.; Torres, E.; Reyes-Urrutia, A.; Echegaray, M.; Rodriguez, R.; Mazza, G. Exergo-ecological analysis and life cycle assessment of agro-wastes using a combined simulation approach based on Cape-Open to Cape-Open (COCO) and SimaPro free-software. Renew. Energy 2022, 201, 60–71. [Google Scholar] [CrossRef]
- Van Baten, J.M. An introduction to COCO. In Proceedings of the AIChE Annual Meeting, Salt Lake City, UT, USA, 4–9 November 2007. [Google Scholar]
- Jain, R.; Moudgalya, K.M.; Fritzson, P.; Pop, A. Development of a Thermodynamic Engine in OpenModelica. In Proceedings of the 12th International Modelica Conference, Prague, Czech Republic, 15–17 May 2017; pp. 89–99. [Google Scholar]
- Li, Y.; Wang, Y.; Zhang, X.; Mi, Z. Thermodynamic analysis of autothermal steam and CO2 reforming of methane. Int. J. Hydrogen Energy 2008, 33, 2507–2514. [Google Scholar] [CrossRef]
- Pashchenko, D. Thermodynamic equilibrium analysis of steam methane reforming based on a conjugate solution of material balance and law action mass equations with the detailed energy balance. Int. J. Energy Res. 2020, 44, 438–447. [Google Scholar] [CrossRef]
- Di Nardo, A.; Portarapillo, M.; Russo, D.; Di Benedetto, A. Hydrogen production via steam reforming of different fuels: Thermodynamic comparison. Int. J. Hydrogen Energy 2024, 55, 1143–1160. [Google Scholar] [CrossRef]
- Nieva, M.A.; Villaverde, M.M.; Monzón, A.; Garetto, T.F.; Marchi, A.J. Steam-methane reforming at low temperature on nickel-based catalysts. Chem. Eng. J. 2014, 235, 158–166. [Google Scholar] [CrossRef]
- Carapellucci, R.; Giordano, L. Steam, dry and autothermal methane reforming for hydrogen production: A thermodynamic equilibrium analysis. J. Power Sources 2020, 469, 228391. [Google Scholar] [CrossRef]

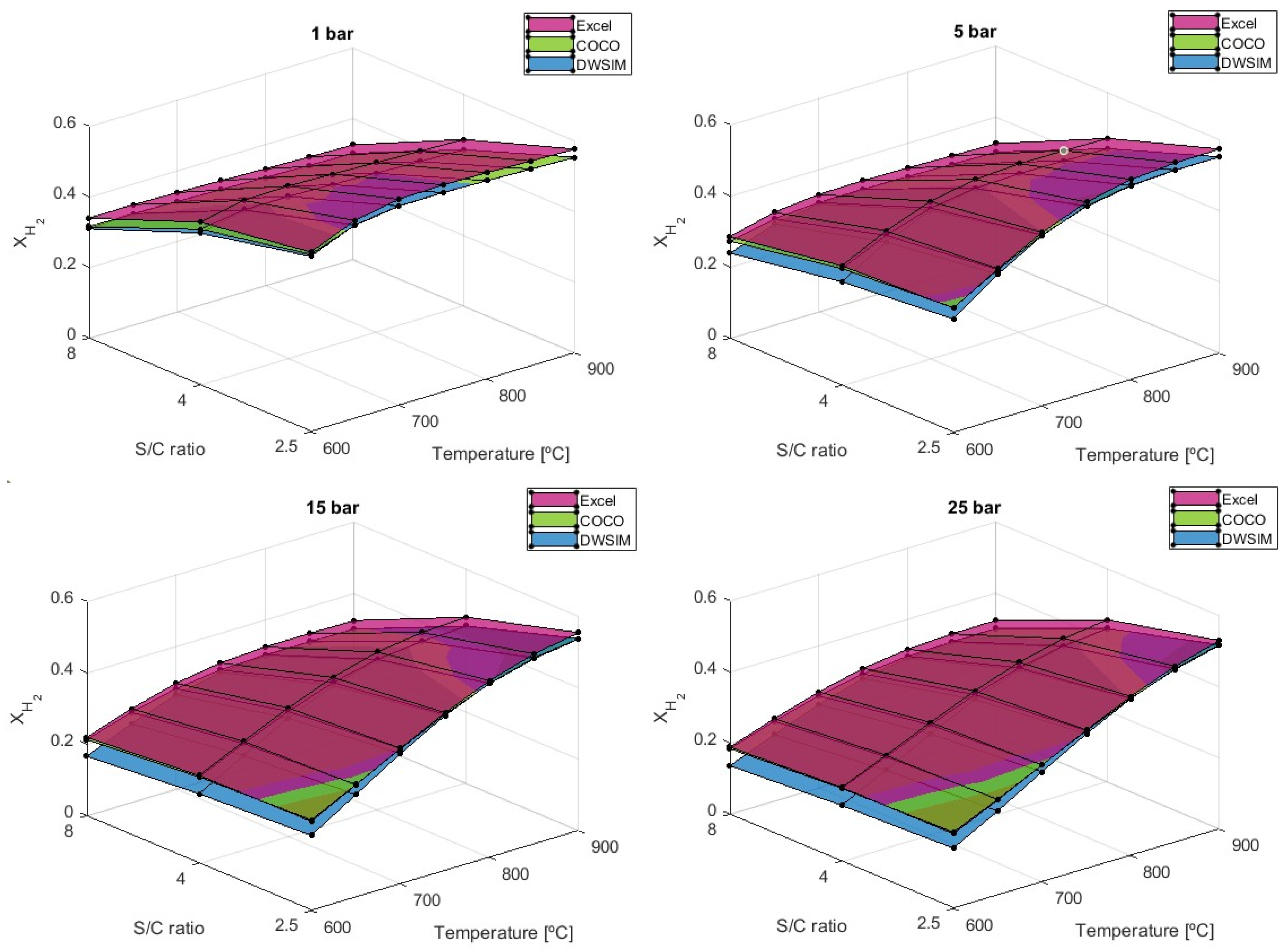


| Temperature | Software | 1 Bar |
|---|---|---|
| 600 °C | Excel | 0.461 |
| COCO | 0.441 | |
| DWSIM | 0.430 | |
| 700 °C | Excel | 0.489 |
| COCO | 0.459 | |
| DWSIM | 0.459 | |
| 800 °C | Excel | 0.481 |
| COCO | 0.451 | |
| DWSIM | 0.451 | |
| 900 °C | Excel | 0.472 |
| COCO | 0.443 | |
| DWSIM | 0.443 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Varandas, B.; Oliveira, M.; Borges, A. Analytical and Numerical Thermodynamic Equilibrium Simulations of Steam Methane Reforming: A Comparison Study. Reactions 2024, 5, 246-259. https://doi.org/10.3390/reactions5010011
Varandas B, Oliveira M, Borges A. Analytical and Numerical Thermodynamic Equilibrium Simulations of Steam Methane Reforming: A Comparison Study. Reactions. 2024; 5(1):246-259. https://doi.org/10.3390/reactions5010011
Chicago/Turabian StyleVarandas, Bruno, Miguel Oliveira, and Amadeu Borges. 2024. "Analytical and Numerical Thermodynamic Equilibrium Simulations of Steam Methane Reforming: A Comparison Study" Reactions 5, no. 1: 246-259. https://doi.org/10.3390/reactions5010011
APA StyleVarandas, B., Oliveira, M., & Borges, A. (2024). Analytical and Numerical Thermodynamic Equilibrium Simulations of Steam Methane Reforming: A Comparison Study. Reactions, 5(1), 246-259. https://doi.org/10.3390/reactions5010011






