Development of Vertical Vibration Model for Micro-Tiller by Smoothed Particle Hydrodynamics Method
Abstract
1. Introduction
2. Soil-Cutting Process Simulation with the SPH Method
2.1. Simulation Model
2.2. Simulation Results
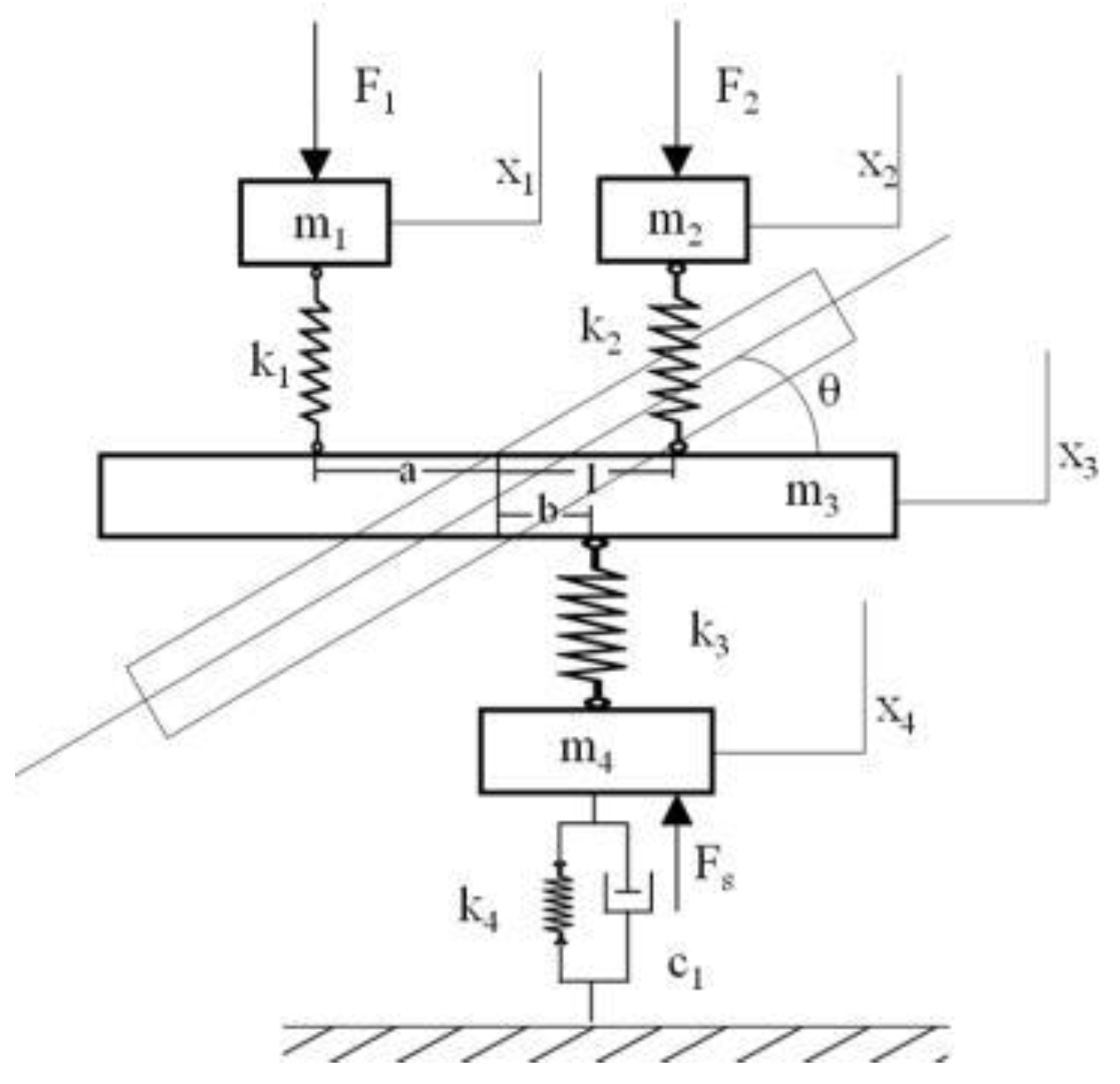
3. Vibration Model
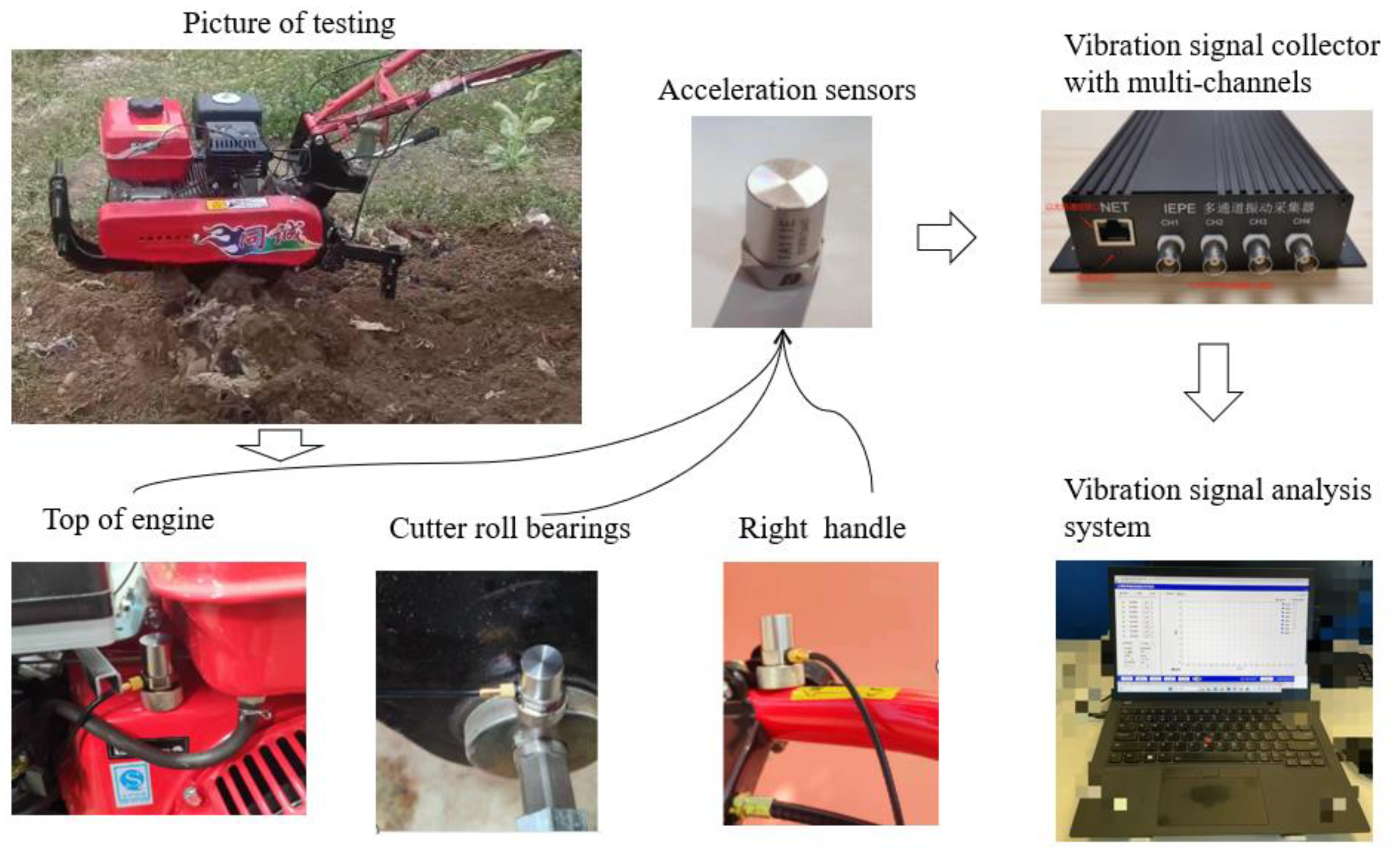
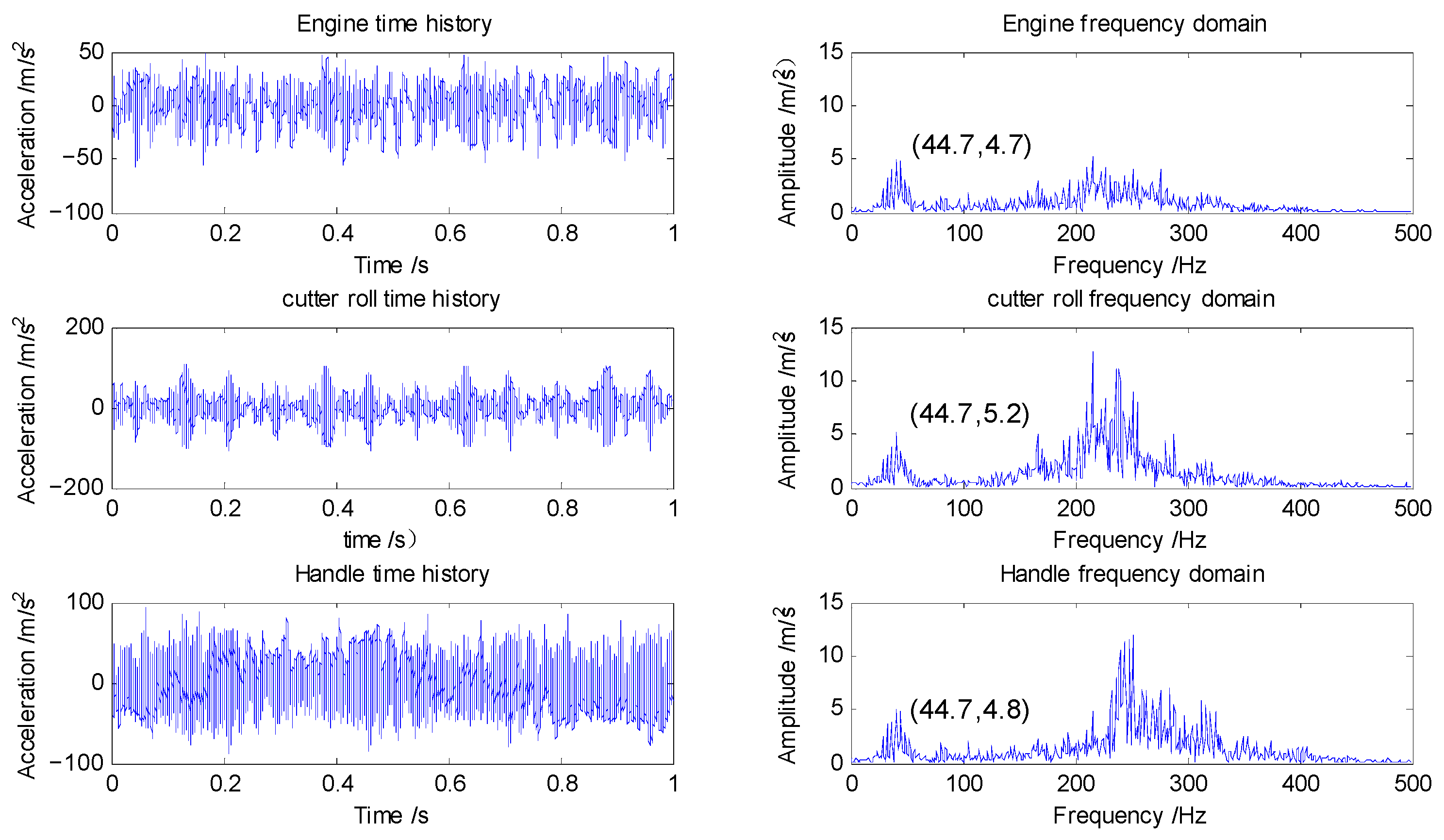
4. Vibration Tests
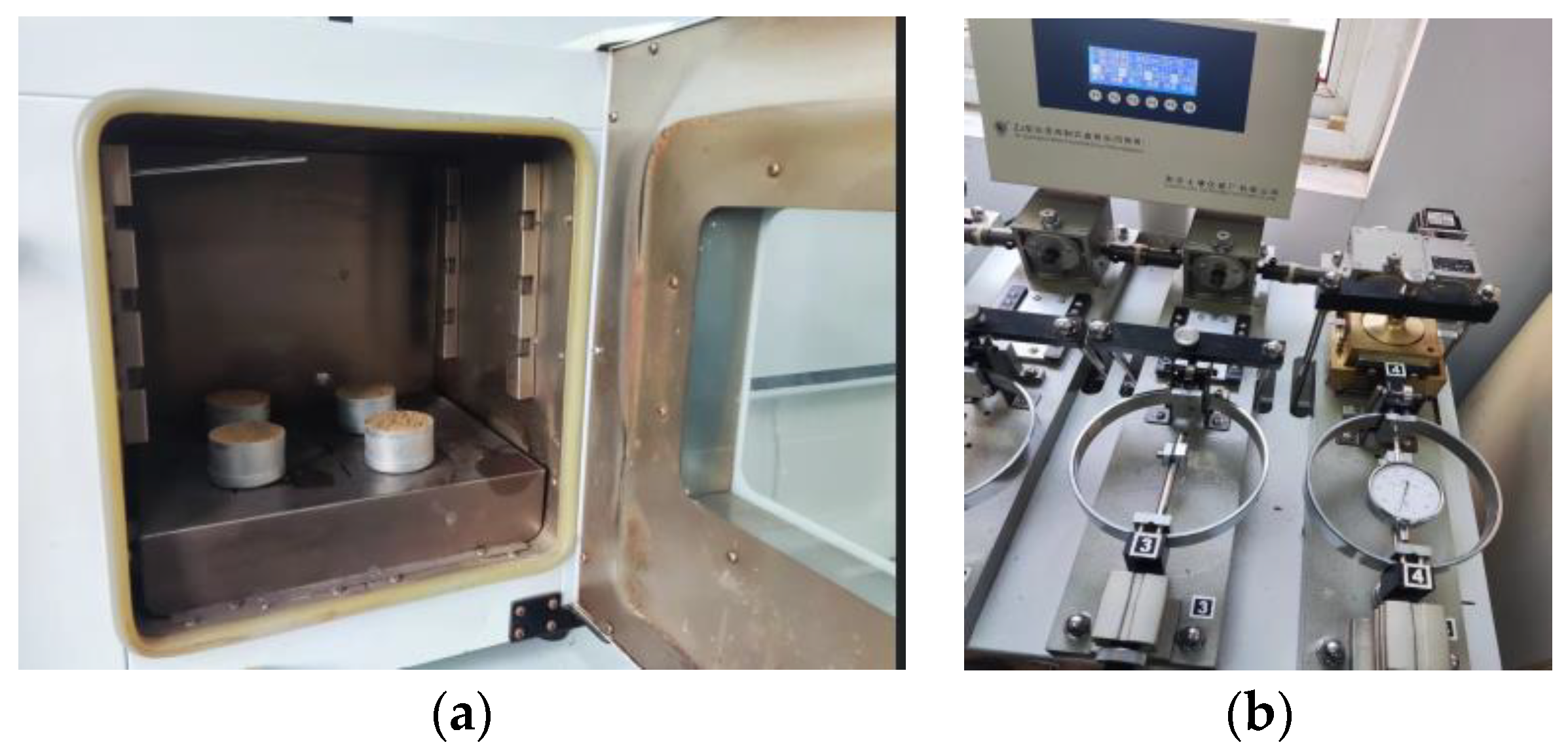
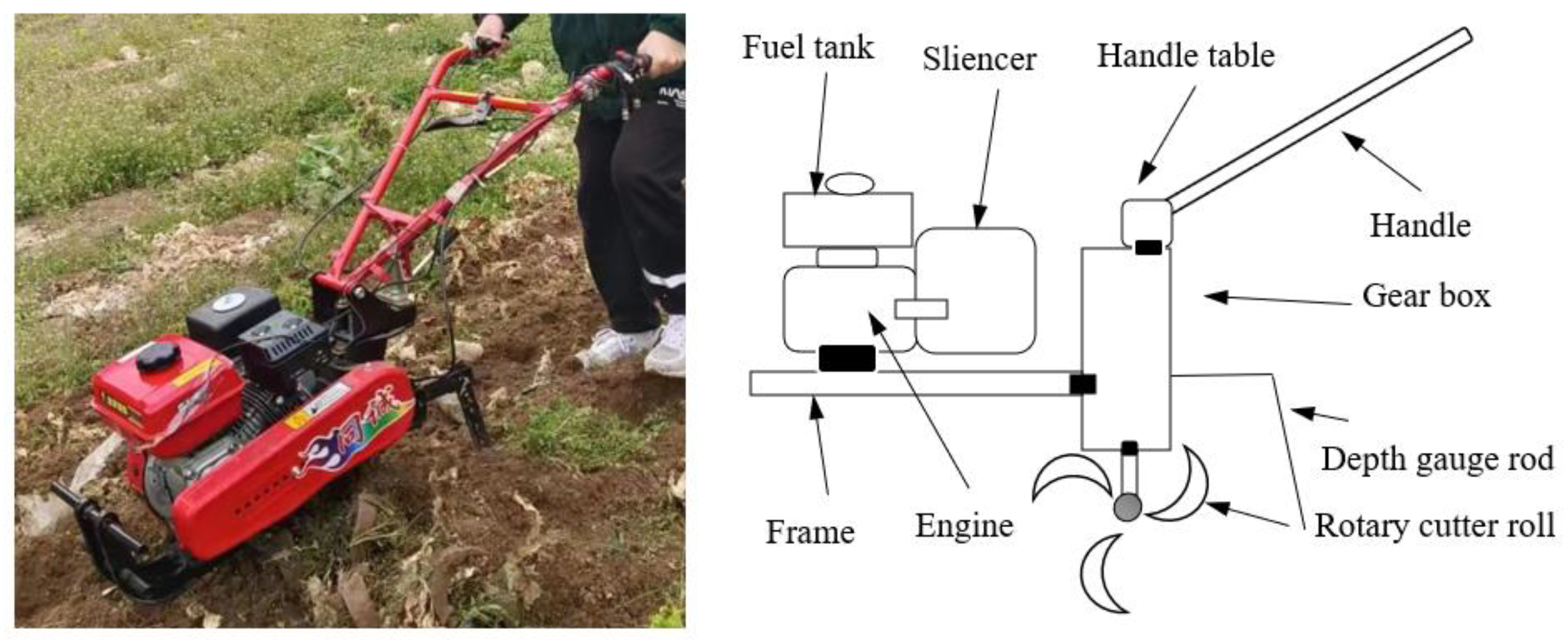
4.1. Experimental Design
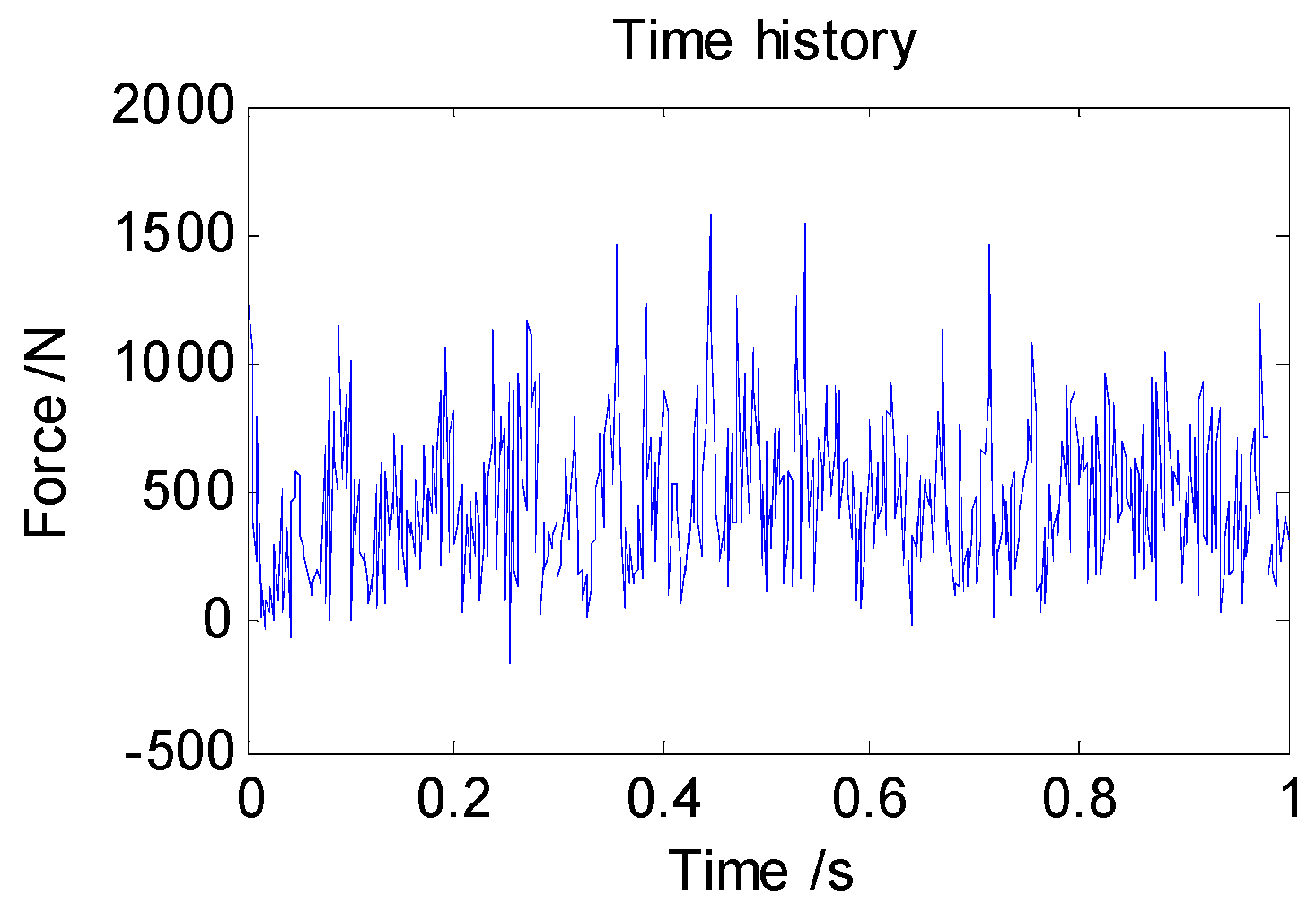
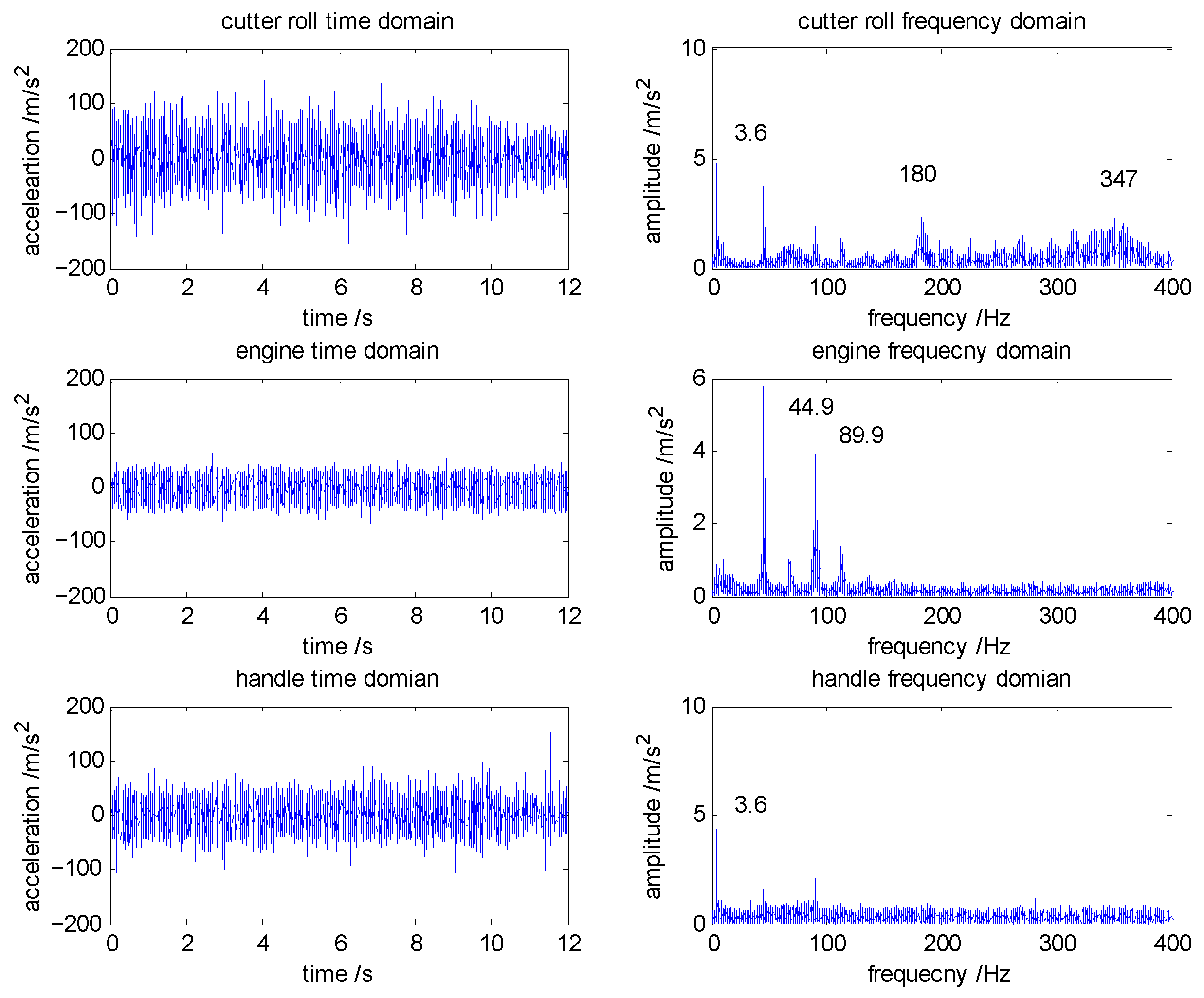
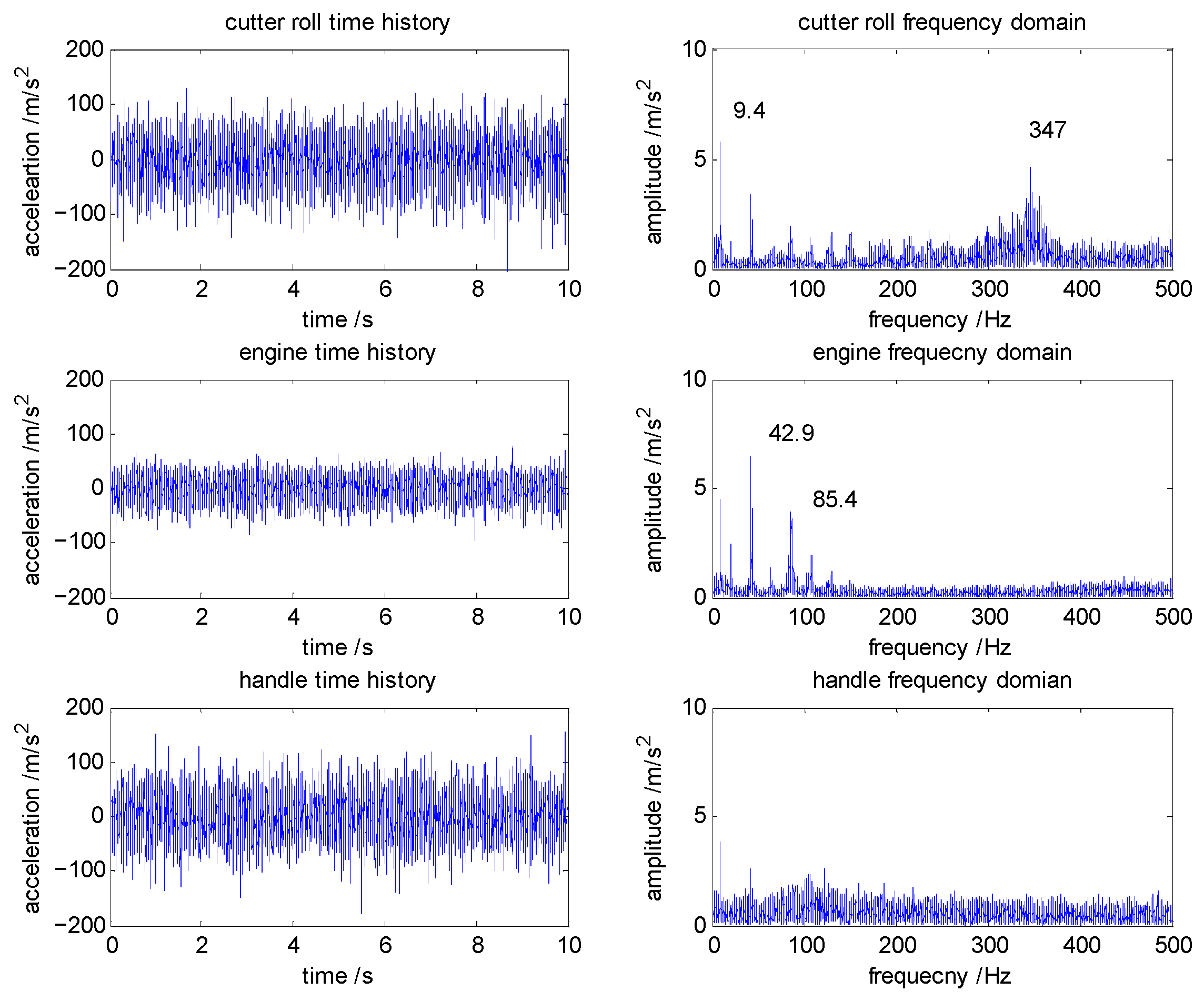
4.2. Test Results Analysis
5. Simulation and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, J.; Meng, X. Study on vibration mechanism and measures for vibration reducing to the handle of cultivator by virtual prototype technology. Nongye Jixie Xuebao Trans. Chin. Soc. Agric. Mach. 2005, 36, 39–42. [Google Scholar]
- Liu, Y. Analysis of vibration characteristics for power tillers based on a rigid-flexible coupling model. J. Vib. Shock. 2018, 37, 250–256. [Google Scholar]
- Dewangan, K.; Tewari, V. Characteristics of hand-transmitted vibration of a hand tractor used in three operational modes. Int. J. Ind. Ergon. 2009, 39, 239–245. [Google Scholar] [CrossRef]
- Chaturvedi, V.; Kumar, A.; Singh, J. Power tiller: Vibration magnitudes and intervention development for vibration reduction. Appl. Ergon. 2012, 43, 891–901. [Google Scholar] [CrossRef]
- Lu, S.; Xu, Z.; Jin, H.; He, M. Vibration Analysis and Vibration Damping Device Design of Power Tiller. IOP Conf. Series Mater. Sci. Eng. 2018, 452, 042180. [Google Scholar] [CrossRef]
- Sun, Y.; Ke, S.; Wang, G.; Liu, Z. Control Strategy Simulation Analysis of a New Micro-cultivator MR Elastomer Vibration Isolation System. Adv. Eng. Sci. Gongcheng Kexue Yu Jishu 2021, 53, 218. [Google Scholar]
- Ko, Y.H.; Ean, O.L.; Ripin, Z.M. The design and development of suspended handles for reducing hand-arm vibration in petrol driven grass trimmer. Int. J. Ind. Ergon. 2011, 41, 459–470. [Google Scholar] [CrossRef]
- Mouazen, A.; Neményi, M. A review of the finite element modelling techniques of soil tillage. Math. Comput. Simul. 1998, 48, 23–32. [Google Scholar] [CrossRef]
- Mouazen, A.M.; Neményi, M. Tillage Tool Design by the Finite Element Method: Part 1. Finite Element Modelling of Soil Plastic Behaviour. J. Agric. Eng. Res. 1999, 72, 37–51. [Google Scholar] [CrossRef]
- Mouazen, A.M.; Neményi, M.; Schwanghart, H.; Rempfer, M. Tillage Tool Design by the Finite Element Method: Part 2. Experimental Validation of the Finite Element Results with Soil Bin Test. J. Agric. Eng. Res. 1999, 72, 53–58. [Google Scholar] [CrossRef]
- Jafari, R.; Tavakoli, T.; Minaee, S.; Raoufat, M.H. Large deformation modeling in soil-tillage tool interaction using advanced 3D nonlinear finite element approach. In Proceedings of the 6th WSEAS International Conference on Simulation, Modelling and Optimization, Lisbon, Portugal, 22–24 September 2006; p. 6. [Google Scholar]
- Davoudi, S.; Alimardani, R.; Keyhani, A.; Atarnejad, R. A two dimensional finite element analysis of a plane tillage tool in soil using a non-linear elasto-plastic model. Am. Eurasian J. Agric. Environ. Sci 2008, 3, 498–505. [Google Scholar]
- Ibrahmi, A.; Bentaher, H.; Maalej, A. Soil-blade orientation effect on tillage forces determined by 3D finite element models. Span. J. Agric. Res. 2014, 12, 941–951. [Google Scholar] [CrossRef]
- Liu, X.H.; Yu, Y.; Qiu, L.C. An Overview of the Finite Element Method on the Tool-Soil Interacting Problem of Tillage. Appl. Mech. Mater. 2015, 707, 397–400. [Google Scholar] [CrossRef]
- Ucgul, M.; Saunders, C.; Fielke, J.M. Comparison of the discrete element and finite element methods to model the interaction of soil and tool cutting edge. Biosyst. Eng. 2018, 169, 199–208. [Google Scholar] [CrossRef]
- Zhou, W.; Ni, X.; Song, K.; Wen, N.; Wang, J.; Fu, Q.; Na, M.; Tang, H.; Wang, Q. Bionic Optimization Design and Discrete Element Experimental Design of Carrot Combine Harvester Ripping Shovel. Processes 2023, 11, 1526. [Google Scholar] [CrossRef]
- Cui, J.; Kui, L.; Zhang, W.; Zhao, D.; Chang, J. Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory. Aerospace 2023, 10, 368. [Google Scholar] [CrossRef]
- Sun, Y.; Guo, C.; Li, Q.; Yue, H.; Zhang, J.; Wang, C.; Hu, Z.; Bo, T. Optimization of rock cutting process parameters with disc cutter for wear and cutting energy reduction based on the discrete element method. J. Clean. Prod. 2023, 391, 136160. [Google Scholar] [CrossRef]
- Zhang, L.; Zhai, Y.; Chen, J.; Zhang, Z.; Huang, S. Optimization design and performance study of a subsoiler underlying the tea garden subsoiling mechanism based on bionics and EDEM. Soil Tillage Res. 2022, 220, 105375. [Google Scholar] [CrossRef]
- Wang, X.; Hu, H.; Wang, Q.; Li, H.; He, J.; Chen, W. Calibration method of soil contact characteristic parameters based on DEM theory. Nongye Jixie Xuebao Trans. Chin. Soc. Agric. Mach. 2017, 48, 78–85. [Google Scholar]
- Rui, Z.; Han, D.; Ji, Q.; He, Y.; Li, J. Calibration methods of sandy soil parameters in simulation of discrete element method. Nongye Jixie Xuebao Trans. Chin. Soc. Agric. Mach. 2017, 48, 49–56. [Google Scholar]
- Zhou, P.; Li, Y.; Liang, R.; Zhang, B.; Kan, Z. Calibration of Contact Parameters for Particulate Materials in Residual Film Mixture after Sieving Based on EDEM. Agriculture 2023, 13, 959. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G.R. Smoothed Particle Hydrodynamics (SPH): An Overview and Recent Developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Hu, M.; Gao, T.; Dong, X.; Tan, Q.; Yi, C.; Wu, F.; Bao, A. Simulation of soil-tool interaction using smoothed particle hydrodynamics (SPH). Soil Tillage Res. 2023, 229, 105671. [Google Scholar] [CrossRef]
- Major, T.; Csanády, V. Combined FEM-SPH Simulation Method for the Modeling of the Interaction of Tillage Tools and the Soil. Erdészettudományi Közlemények 2015, 5, 7–19. [Google Scholar] [CrossRef][Green Version]
- Li, S.; Chen, X.; Chen, W.; Zhu, S.; Li, Y.; Yang, L.; Xie, S.; Yang, M. Soil-cutting simulation and parameter optimization of handheld tiller’s rotary blade by Smoothed Particle Hydrodynamics modelling and Taguchi method. J. Clean. Prod. 2018, 179, 55–62. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Zhang, X.; Tian, H.; Yu, F. Handheld Micro tiller time-frequency characteristic and vibration isolation measures. J. Vibroeng. 2022, 24, 824–835. [Google Scholar] [CrossRef]


| Parameters | Values |
|---|---|
| Cutter roll material density | 7830 kg/m3 |
| Poisson’s ratio | 0.3 |
| Elastic modulus | 207 GPa |
| Rotation radius of the cutter roll | 210 mm |
| Soil density | 1750 kg/m3 |
| Soil bulk modulus | 3.5 × 107 Pa |
| Specific gravity of soil particle | 2.75 |
| Soil moisture content | 16.07% |
| Soil shear modulus | 2.1 × 107 Pa |
| Soil internal friction angle | 0.436 rad |
| Cutter Roll Speed (r/s) | Tillage Depth/m | Acting Forces of Soil on Cutter Roll | Error/% | |
|---|---|---|---|---|
| Calculated/N | Simulated/N | |||
| 4 | 0.10 | 537.12 | 480 | 11.87 |
| 4 | 0.11 | 547.38 | 485 | 12.86 |
| 4.4 | 0.10 | 549.39 | 498 | 10.32 |
| 4.8 | 0.10 | 550.40 | 506 | 8.77 |
| Gear | Test | RMS Values (m/s2) | ||
|---|---|---|---|---|
| Cutter Roll | Engine | Right Handle | ||
| low gear | Test 1 | 28.58 | 17.00 | 21.17 |
| Test 2 | 28.87 | 17.68 | 23.40 | |
| high gear | Test 3 | 31.63 | 19.20 | 36.56 |
| Test 4 | 32.78 | 19.26 | 32.62 | |
| Test | Prominent Frequency (Hz) and Amplitude(m/s2) | |||||
|---|---|---|---|---|---|---|
| Cutter Roll | Engine | Handle (Right) | ||||
| Frequency | Amplitude | Frequency | Amplitude | Frequency | Amplitude | |
| Test 1 2 r/s | 6.8 42.2 317.2 | 5.5 3.4 2.6 | 42.2 85.0 6.8 | 5.9 4.5 2.6 | 6.8 42.3 | 4.9 2.2 |
| Test 2 2 r/s | 7.1 44.9 3.6 348.1 90.0 | 7.1 6.1 3.2 3.2 3.0 | 44.9 89.5 7.1 | 10.3 6.3 3.1 | 7.1 3.6 44.7 | 5.7 2.7 2.5 |
| Test 3 3 r/s | 9.6 342.3 44.0 180.0 4.8 | 3.9 3.7 3.3 2.6 2.6 | 44.0 88.3 9.6 4.8 | 4.8 3.3 3.2 2.6 | 9.6 143.0 4.8 44.9 | 3.1 2.7 2.4 2.0 |
| Test 4 3 r/s | 9.4 347 43.0 85.6 | 5.8 4.7 3.4 1.8 | 43.0 9.4 85.5 | 65 4.5 3.9 | 9.4 43.0 122.2 | 3.9 2.6 2.6 |
| Main Parts | Tested RMS/(m/s2) | Simulated RMS/(m/s2) | Error (%) |
|---|---|---|---|
| Engine | 19.62 | 18.78 | 4.28 |
| Cutter roll | 32.78 | 34.43 | 5.03 |
| Right handle | 32.62 | 34.69 | 6.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Hao, W.; Chen, Y.; Hao, Q.; Zhang, X.; Sun, Z. Development of Vertical Vibration Model for Micro-Tiller by Smoothed Particle Hydrodynamics Method. AgriEngineering 2024, 6, 2481-2493. https://doi.org/10.3390/agriengineering6030145
Liu X, Hao W, Chen Y, Hao Q, Zhang X, Sun Z. Development of Vertical Vibration Model for Micro-Tiller by Smoothed Particle Hydrodynamics Method. AgriEngineering. 2024; 6(3):2481-2493. https://doi.org/10.3390/agriengineering6030145
Chicago/Turabian StyleLiu, Xiaochan, Wenchang Hao, Yong Chen, Qingle Hao, Xiuli Zhang, and Zhipeng Sun. 2024. "Development of Vertical Vibration Model for Micro-Tiller by Smoothed Particle Hydrodynamics Method" AgriEngineering 6, no. 3: 2481-2493. https://doi.org/10.3390/agriengineering6030145
APA StyleLiu, X., Hao, W., Chen, Y., Hao, Q., Zhang, X., & Sun, Z. (2024). Development of Vertical Vibration Model for Micro-Tiller by Smoothed Particle Hydrodynamics Method. AgriEngineering, 6(3), 2481-2493. https://doi.org/10.3390/agriengineering6030145








