Modeling and Assessing Heat Transfer of Piglet Microclimates
Abstract
1. Introduction
- Develop a steady-state, thermal balance model for piglet heat transfer,
- Expand the Effective Environment Temperature (EET) to include a conduction component with the mat and multiple piglets in the creep area (assuming a single layer, no piling), and
- Demonstrate differences in EET for two different microclimates provided by (1) a conventional heat lamp and (2) a heated semi-enclosed device.
2. Materials and Methods
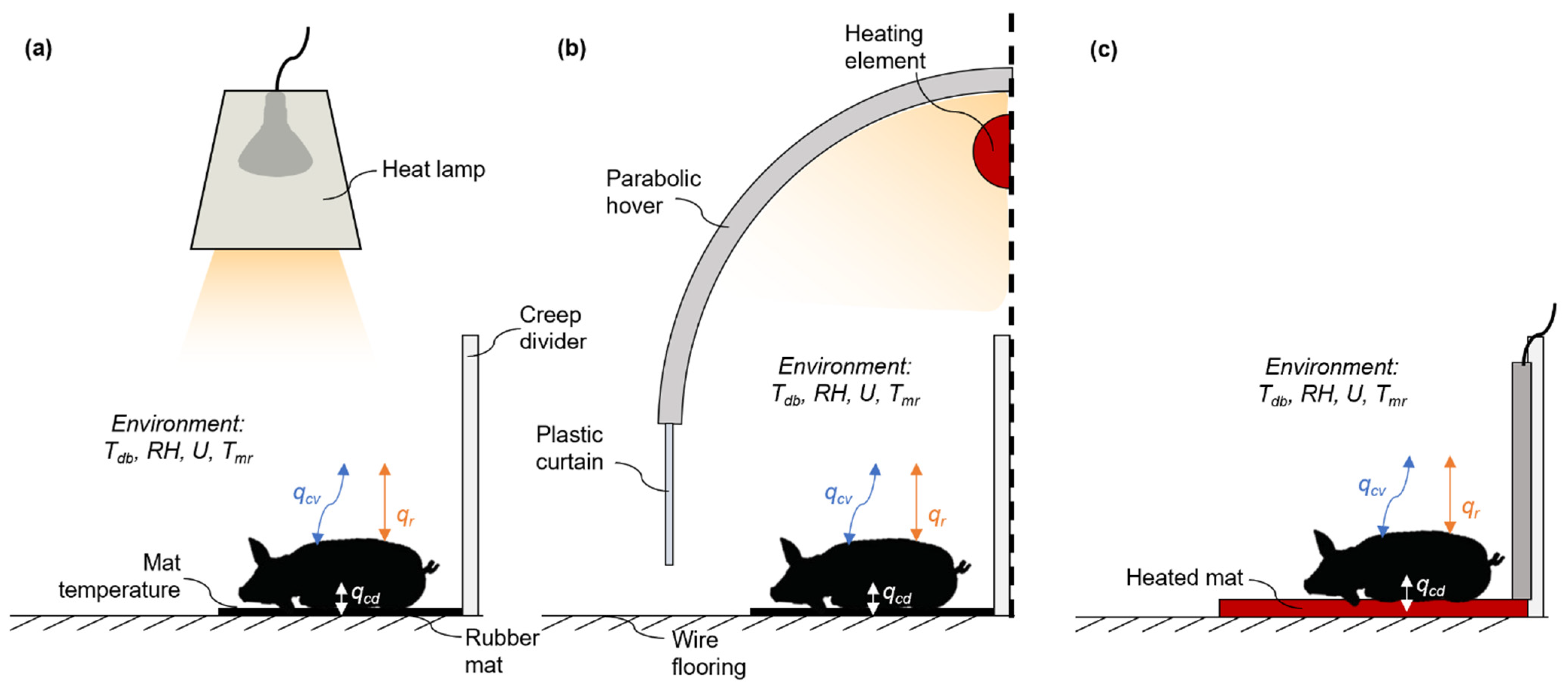
2.1. Model Development
- = piglet steady-state internal heat production (W)
- = conductive steady-state heat transfer (dissipation or gain, W)
- = convective steady-state heat dissipation (W)
- = radiative steady-state heat dissipation (W)
- = steady-state conductive heat transfer to rubber mat (W)
- = piglet surface area (m2)
- = surface area portion touching the mat
- = piglet core body temperature (39.5 °C)
- = mat underside temperature (°C)
- = piglet tissue resistance (m2 °C W−1)
- = mat thermal resistance (m2 °C W−1)
- = steady-state conductive heat gain from heat mat (W)
- = heat mat contact temperature with a piglet (°C)
- hcv = convective heat transfer coefficient (W m−2 K−1)
- kcr = surface area proportion interacting with mixed modes heat transfer (m2)
- Tsk = piglet skin surface temperature (K)
- Tdb = microclimate dry-bulb temperature (K)
- ε = emissivity (dimensionless)
- σ = Stefan‒Boltzmann constant (5.67 E-8 W m−2 K−4)
- TMR = mean radiant temperature (K)
- SA = surface area of a piglet (m2)
- BW = body weight of the piglet (kg)
2.2. EETcp Development
- EETcp = Effective Environment Temperature (°C)
- TMAT = Modified Ambient Temperature (°C, [21])
- kmm = surface area proportion interacting with mixed modes of heat transfer (0.80)
- kcd = surface area proportion interacting with conduction to the mat (0.20)
- Tmc = mat contact surface temperature (°C)
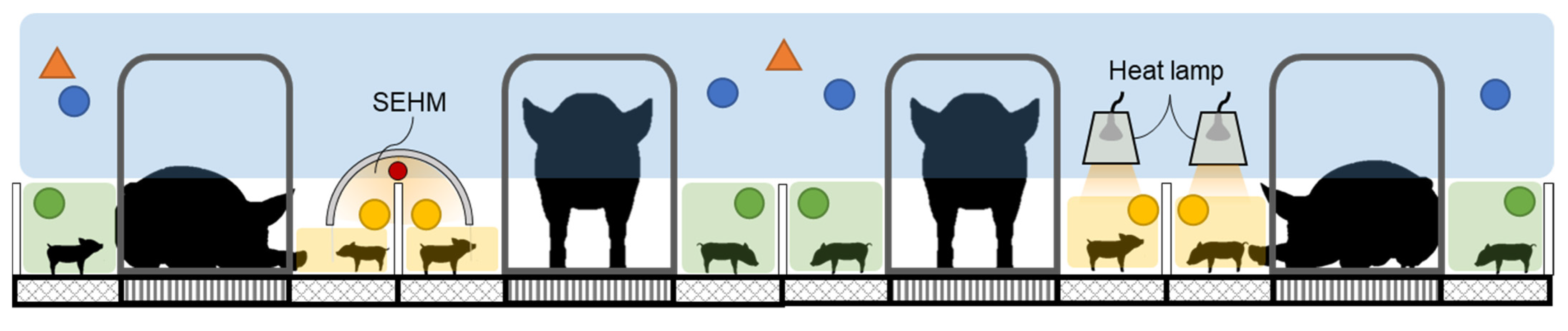
2.3. Model and EETcp Application
- THP = total heat production (W)
- FHP = fasting heat production (W)
- HI = heat increment (W)
3. Results and Discussion
3.1. Model and EETcp
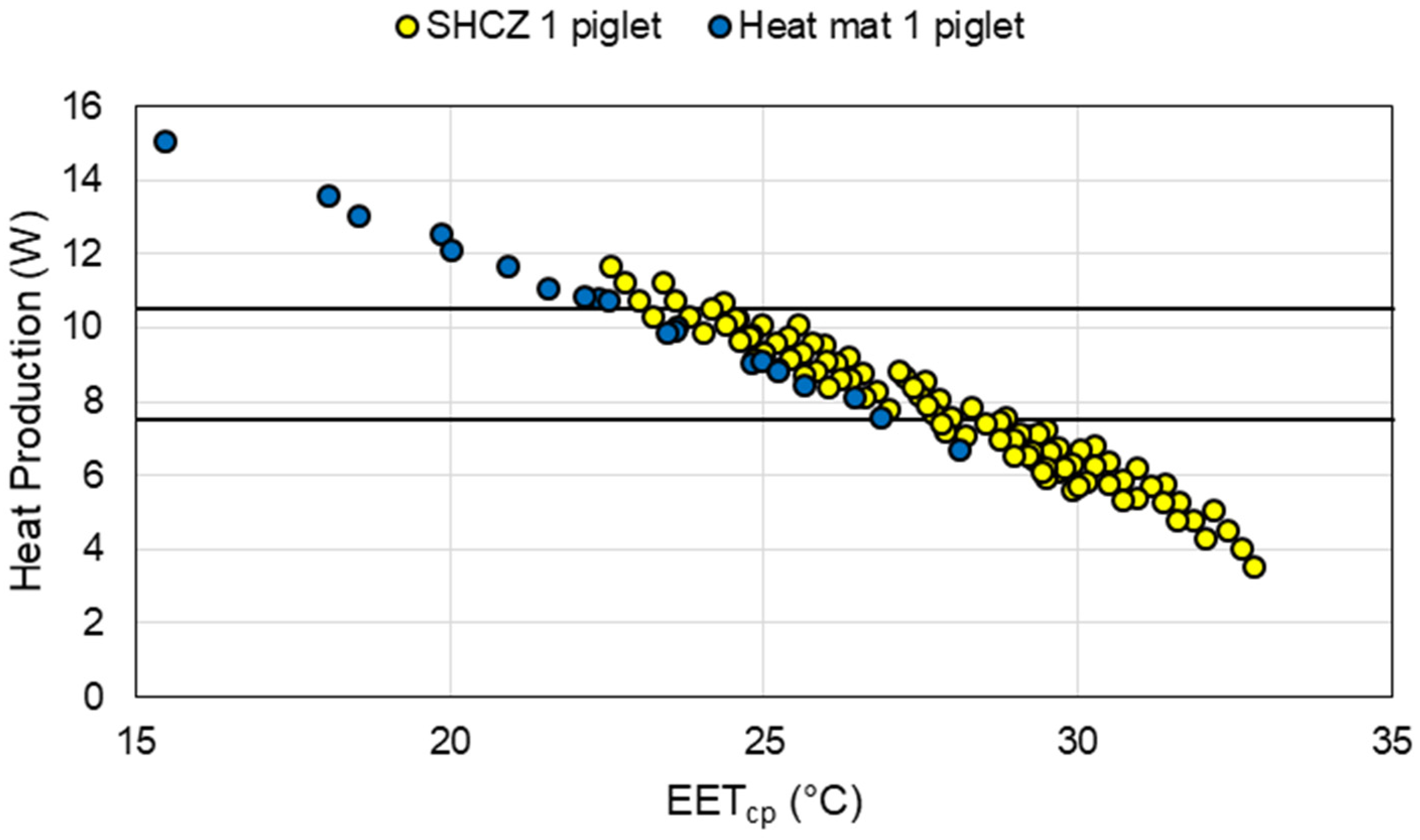
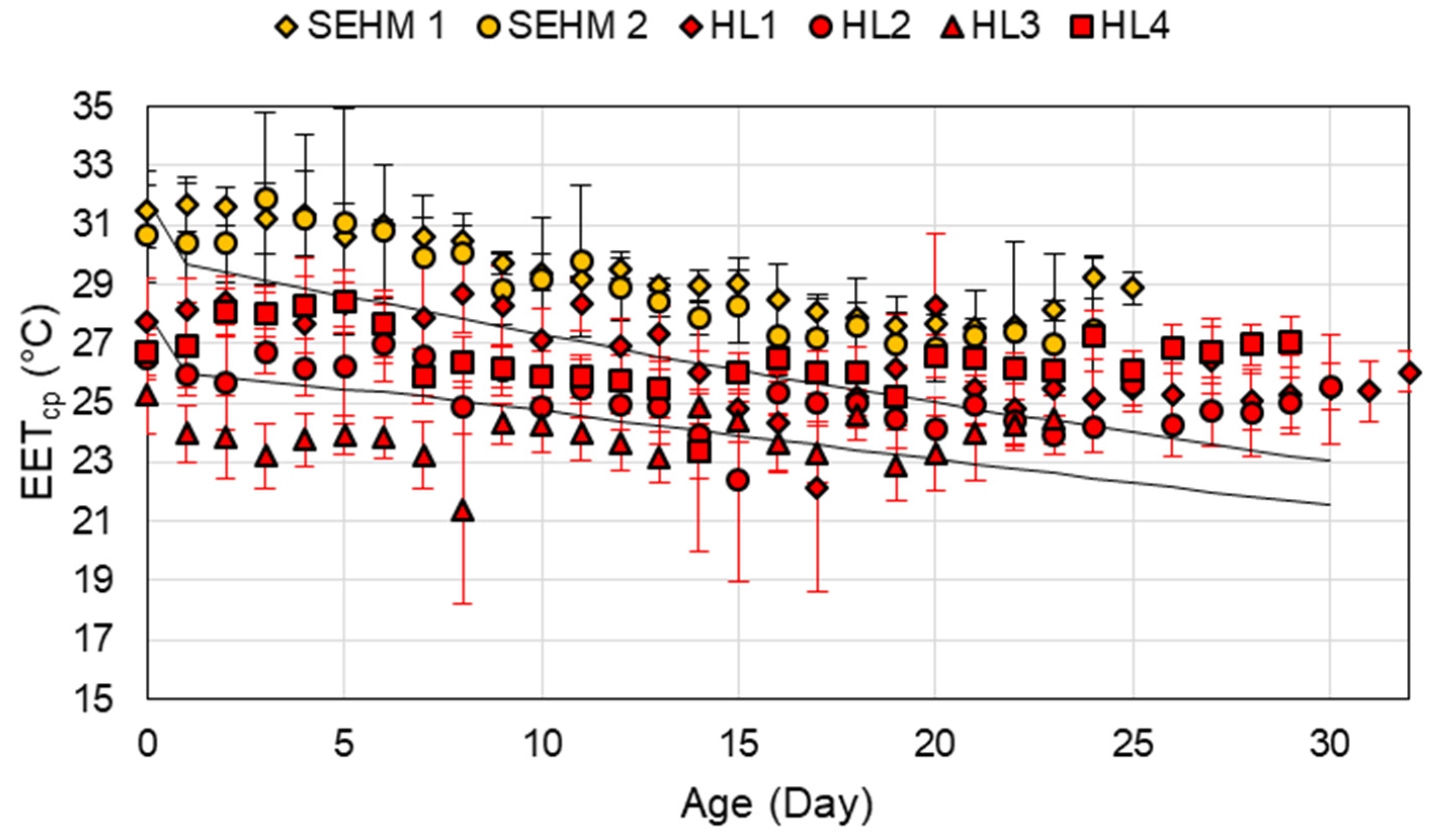
3.2. EETcp Application
4. Conclusions
- (1)
- The SEHM is capable of consistent microclimate environments across multiple units covering different creep areas.
- (2)
- Manual adjustment of HL height results in variable environments within a room.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- PIC. PIC Sow and Gilt Manual 2015; PIC: Henersonville, TN, USA, 2015. [Google Scholar]
- Varley, M.A. (Ed.) The Neonatal Pig Development and Survival; CAB International: Wallingford, UK, 1995; ISBN 0 85198 925 X. [Google Scholar]
- Mount, L.E. Adaptation to Thermal Environment; Edward Arnold Limited: London, UK, 1979; ISBN 0-7131-2739-2. [Google Scholar]
- Hoff, S.J.; Janni, K.A.; Jacobson, L.D. Modeling Newborn Piglet Thermal Interactions with a Surface Energy Balance Model. Trans. ASAE 1993, 36, 151–159. [Google Scholar] [CrossRef][Green Version]
- Stinn, J.P.; Xin, H. Heat Lamp vs. Heat Mat as Localized Heat Source in Swine Farrowing Crate; Iowa State University, Digital Repository: Ames, IA, USA, 2014. [Google Scholar]
- Smith, B.C.; Ramirez, B.C.; Hoff, S.J.; Greiner, L.L. Pilot-Scale Assessment of a Novel Farrowing Creep Area Supplementary Heat Source. Animals 2019, 9, 996. [Google Scholar] [CrossRef] [PubMed]
- Gorczyca, M.T.; Milan, H.F.M.; Maia, A.S.C.; Gebremedhin, K.G. Machine Learning Algorithms to Predict Core, Skin, and Hair-Coat Temperatures of Piglets. Comput. Electron. Agric. 2018, 151, 286–294. [Google Scholar] [CrossRef]
- Morello, G.M.; Lay, D.C., Jr.; Rodrigues, L.H.A.; Richert, B.T.; Marchant-Forde, J.N. Microenvironments in Swine Farrowing Rooms: The Thermal, Lighting, and Acoustic Environments of Sows and Piglets. Sci. Agric. 2018, 75, 1–11. [Google Scholar] [CrossRef]
- Berckmans, D. General Introduction to Precision Livestock Farming. Anim. Front. 2017, 7, 6–11. [Google Scholar] [CrossRef]
- Milan, H.F.M.; Campos Maia, A.S.; Gebremedhin, K.G. Prediction of Optimum Supplemental Heat for Piglets. Trans. ASABE 2019, 62, 321–342. [Google Scholar] [CrossRef]
- Mount, L.E. Radiant and Convective Heat Loss from the Newborn Pig. J. Physiol. 1964, 173, 96–113. [Google Scholar] [CrossRef]
- Mount, L.E. The Heat Loss from Newborn Pigs to the Floor. Res. Vet. Sci. 1967, 8, 175–186. [Google Scholar] [CrossRef]
- Andersen, I.L.; Haukvik, I.A.; Bøe, K.E. Drying and Warming Immediately after Birth May Reduce Piglet Mortality in Loose-Housed Sows. Animal 2009, 3, 592–597. [Google Scholar] [CrossRef]
- Vande Pol, K.D.; Tolosa, A.F.; Shull, C.M.; Brown, C.B.; Alencar, S.A.S.; Ellis, M. Effect of Drying and/or Warming Piglets at Birth on Rectal Temperature over the First 24 h after Birth. Transl. Anim. Sci. 2020, 4, txaa184. [Google Scholar] [CrossRef]
- Brown-Brandl, T.M.; Hayes, M.D.; Xin, H.; Nienaber, J.A.; Li, H.; Eigenberg, R.A.; Stinn, J.P.; Shepherd, T. Heat and Moisture Production of Modern Swine. ASHRAE Trans. 2014, 120, 469. [Google Scholar]
- Stinn, J.P.; Xin, H. Heat and Moisture Production Rates of a Modern U.S. Swine Breeding, Gestation, and Farrowing Facility. Trans. ASABE 2014, 1517–1528. [Google Scholar] [CrossRef]
- Hayes, M.D.; Brown-Brandl, T.; Stinn, J.P.; Li, H.; Xin, H.; Nienaber, J.A.; Shepherd, T.A. House-Level Moisture Production of Modern Swine by Age, Temperature and Source; ASABE: Kansas City, MI, USA, 2013. [Google Scholar]
- Johnson, J.S.; Zhang, S.; Morello, G.M.; Maskal, J.M.; Trottier, N.L. Technical Note: Development of an Indirect Calorimetry System to Determine Heat Production in Individual Lactating Sows. J. Anim. Sci. 2019, 97, 1609–1618. [Google Scholar] [CrossRef]
- DeShazer, J.A. Livestock Energetics and Thermal Environmental Management, 1st ed.; American Society of Agricultural and Biological Engineers: St. Joesph, MI, USA, 2009; ISBN 1-892769-74-3. [Google Scholar]
- Mostaco, G.M.; Miranda, K.O.S.; Condotta, I.C.F.S.; Salgado, D.D.A. Determination of Piglets’ Rectal Temperature and Respiratory Rate through Skin Surface Temperature under Climatic Chamber Conditions. J. Braz. Assoc. Agric. Eng. 2015, 35, 979–989. [Google Scholar] [CrossRef]
- Hoff, S.J.; Janni, K.A.; Jacobson, L.D. Defining the Newborn Piglet’s Thermal Environment with an Effective Environmental Temperature. Trans. ASAE 1993, 36, 143–150. [Google Scholar] [CrossRef]
- Mount, L.E. Evaporation Heat Loss in the Newborn Pig. J. Physiol. 1962, 164, 274–281. [Google Scholar] [CrossRef]
- Holman, J.P. Heat Transfer, 9th ed.; McGraw-Hill: New York, NY, USA, 2002; ISBN 0-07-240655-0. [Google Scholar]
- Zhang, Q.; Xin, H. Responses of Piglets to Creep Heat Type and Location in Farrowing Cage. Appl. Eng. Agric. 2001, 17, 515–519. [Google Scholar] [CrossRef]
- ASHRAE. ASHRAE Handbook; Volume Fundamentals; ASHRAE: Atlanta, GA, USA, 2013. [Google Scholar]
- Ramirez, B.C.; Gao, Y.; Hoff, S.J.; Harmon, J.D. Thermal Environment Sensor Array: Part I. Development and Field Performance Assessment. Biosyst. Eng. 2018, 174, 329–340. [Google Scholar] [CrossRef]
- Smith, B.C.; Ramirez, B.C. Dimensions of the Suckling Pig. Front. Anim. Sci. 2021, 2, 31. [Google Scholar] [CrossRef]
- Fialho, F.B.; Bucklin, R.A.; Zazueta, F.S.; Myer, R.O. Theoretical Model of Heat Balance in Pigs. Anim. Sci. 2004, 79, 121–134. [Google Scholar] [CrossRef]
- Zhang, Q.; Xin, H. Static and Dynamic Temperature Distribution of Heat Mats for Swine Farrowing Creep Heating. Appl. Eng. Agric. 2000, 16, 563–569. [Google Scholar] [CrossRef]
- Bruce, J.M.; Clark, J.J. Models of Heat Production and Critical Temperature for Growing Pigs. Anim. Sci. 1979, 28, 353–369. [Google Scholar] [CrossRef]
- PIC. Wean To Finish Manual; PIC: Hendersonville, TN, USA, 2014. [Google Scholar]
- Usry, J.L.; Turner, L.W.; Bridges, T.C.; Nienaber, J.A. Modeling the Physiological Growth of Swine Part III: Heat Production and Interaction With Environment. Trans. ASAE 1992, 35, 1035–1042. [Google Scholar] [CrossRef]
- Kluyver, T.; Ragan-Kelley, B.; Pérez, F.; Granger, B.E.; Bussonnier, M.; Frederic, J.; Kelley, K.; Hamrick, J.; Grout, J.; Corlay, S.; et al. Jupyter Notebooks—A Publishing Format for Reproducible Computational Workflows. In Positioning and Power in Academic Publishing: Players, Agents and Agendas; IOS Press: Amsterdam, The Netherlands, 2016; pp. 87–90. [Google Scholar] [CrossRef]
- Smith, B.C.; Weyer, S.E.; Ramirez, B.C.; Hoff, S.J.; Gates, R.S. Development and Validation of an Animal Thermal Environement Interaction Laboratory. Appl. Eng. Agric. 2021, in press. [Google Scholar] [CrossRef]
- Smith, B.C. Technology and Assessment Techniques for Swine Farrowing Facility Management. Master’s Thesis, Iowa State University, Ames, IA, USA, 2021. [Google Scholar]
- Brody, S. Bioenergetics and Growth; Reinhold: New York, NY, USA, 1945. [Google Scholar]
- Jurgens, M.; Bergendahl, K.; Coverdale, J.; Hansen, S. Animal Feeding and Nutrition, 11th ed.; Kendall Hunt: Dubuque, IA, USA, 2012; ISBN 978-0-7575-9113-6. [Google Scholar]
- Labussière, E.; van Milgen, J.; de Lange, C.F.M.; Noblet, J. Maintenance Energy Requirements of Growing Pigs and Calves Are Influenced by Feeding Level. J. Nutr. 2011, 141, 1855–1861. [Google Scholar] [CrossRef] [PubMed]
- Labussière, E.; Dubois, S.; van Milgen, J.; Noblet, J. Partitioning of Heat Production in Growing Pigs as a Tool to Improve the Determination of Efficiency of Energy Utilization. Front. Physiol. 2013, 4, 146. [Google Scholar] [CrossRef] [PubMed]
- Milgen, J.V.; Noblet, J.; Dubois, S.; Bernier, J.-F. Dynamic Aspects of Oxygen Consumption and Carbon Dioxide Production in Swine. Br. J. Nutr. 1997, 78, 397–410. [Google Scholar] [CrossRef] [PubMed]
- Mount, L.E. Environmental Temperature Preferred by the Young Pig. Nature 1963, 199, 1212–1213. [Google Scholar] [CrossRef]
- Zhang, Q.; Xin, H. Modeling Heat Mat Operation for Piglet Creep Heating. Trans. ASAE 2000, 43, 1261–1267. [Google Scholar] [CrossRef]





| Tdb (°C) | TMR (°C) | U (m s−1) | Tm (°C) | Predicted Piglet Heat Production (W) | Predicted Piglet TSK (°C) | EET (°C) | EETcp (°C) |
|---|---|---|---|---|---|---|---|
| 29 | 33 | 0.12 | 37.0 | 5.8 | 37.3 | 29.6 | 31.4 |
| 25 | 29 | 0.12 | 37.0 | 7.4 | 36.3 | 26.5 | 28.8 |
| 29 | 33 | 0.32 | 37.0 | 6.7 | 36.6 | 28.0 | 30.1 |
| 25 | 29 | 0.32 | 37.0 | 9.5 | 35.4 | 22.8 | 25.9 |
| 29 | 33 | 0.12 | 39.5 | 4.5 | 37.5 | 30.6 | 32.4 |
| 25 | 29 | 0.12 | 39.5 | 6.9 | 36.5 | 26.5 | 29.1 |
| 29 | 33 | 0.32 | 39.5 | 6.2 | 36.8 | 28.0 | 30.3 |
| 25 | 29 | 0.32 | 39.5 | 9.1 | 35.6 | 22.8 | 26.2 |
| 29 | 33 | 0.12 | 42.0 | 4.0 | 37.8 | 30.6 | 32.6 |
| 25 | 29 | 0.12 | 42.0 | 6.4 | 36.7 | 26.5 | 29.3 |
| 29 | 33 | 0.32 | 42.0 | 5.8 | 37.0 | 28.0 | 30.5 |
| 25 | 29 | 0.32 | 42.0 | 8.6 | 35.8 | 22.8 | 26.4 |
| 29 | 33 | 0.12 | 44.5 | 3.5 | 38.0 | 30.6 | 32.8 |
| 25 | 29 | 0.12 | 44.5 | 5.9 | 36.9 | 26.5 | 29.5 |
| 29 | 33 | 0.32 | 44.5 | 5.3 | 37.2 | 28.0 | 30.7 |
| 25 | 29 | 0.32 | 44.5 | 8.1 | 36.0 | 22.8 | 26.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smith, B.C.; Ramirez, B.C.; Hoff, S.J. Modeling and Assessing Heat Transfer of Piglet Microclimates. AgriEngineering 2021, 3, 768-782. https://doi.org/10.3390/agriengineering3040048
Smith BC, Ramirez BC, Hoff SJ. Modeling and Assessing Heat Transfer of Piglet Microclimates. AgriEngineering. 2021; 3(4):768-782. https://doi.org/10.3390/agriengineering3040048
Chicago/Turabian StyleSmith, Benjamin C., Brett C. Ramirez, and Steven J. Hoff. 2021. "Modeling and Assessing Heat Transfer of Piglet Microclimates" AgriEngineering 3, no. 4: 768-782. https://doi.org/10.3390/agriengineering3040048
APA StyleSmith, B. C., Ramirez, B. C., & Hoff, S. J. (2021). Modeling and Assessing Heat Transfer of Piglet Microclimates. AgriEngineering, 3(4), 768-782. https://doi.org/10.3390/agriengineering3040048







