Angle-Dependent Absorption of Sound on Porous Materials
Abstract
:1. Introduction
2. Materials and Methods
2.1. Studied Material Samples
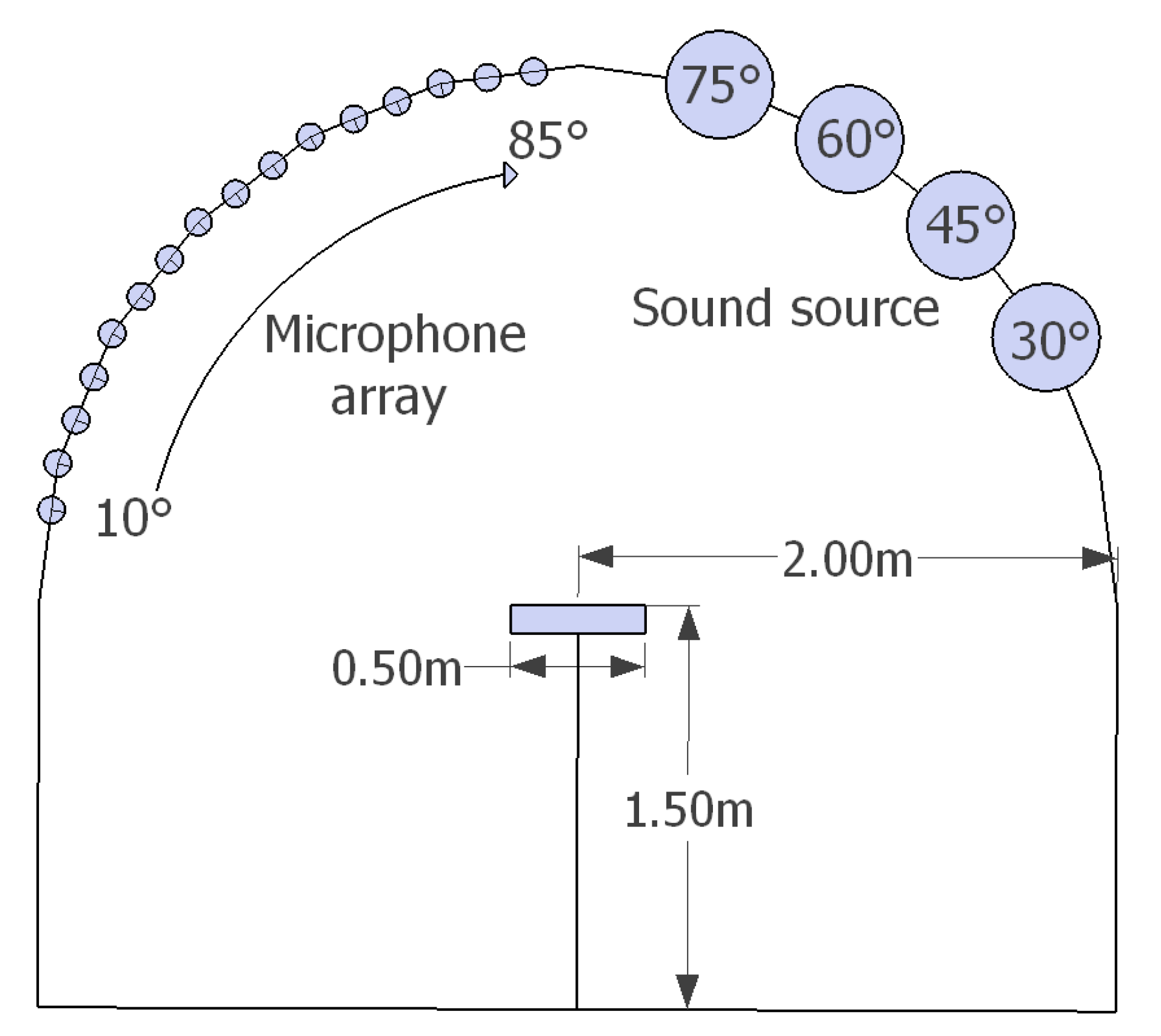
2.2. Experimental Setup
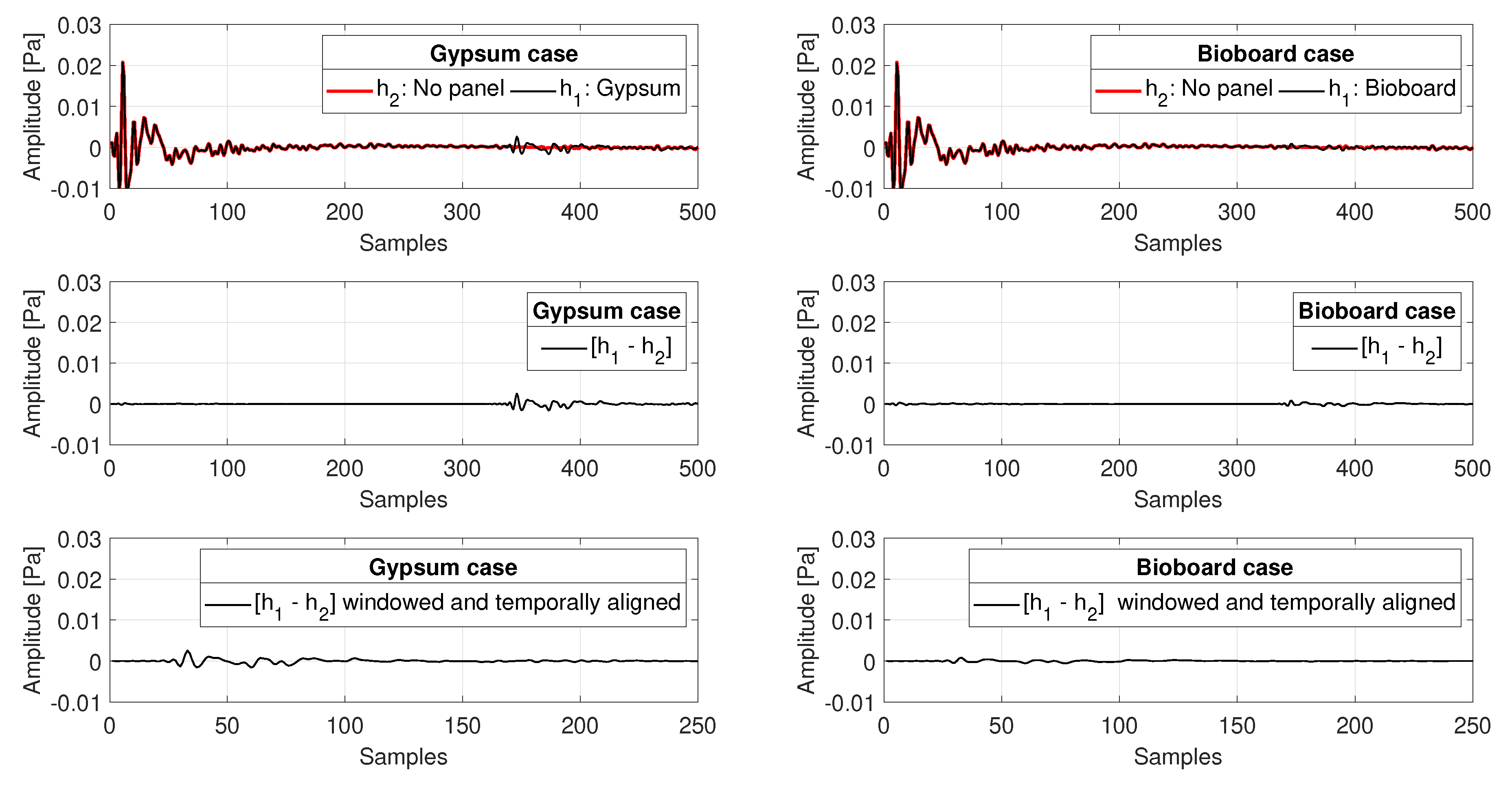
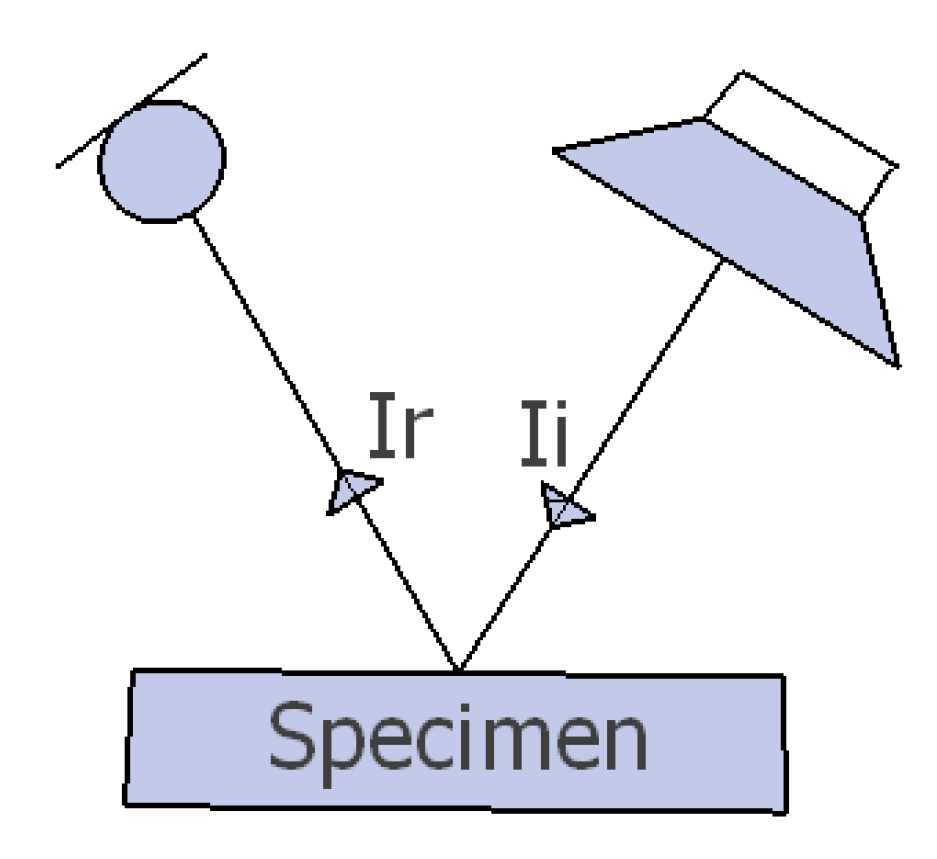
2.3. Compensation of the Measurement Device Responses and Generation of Polar Responses
2.4. Computation of the Angle-Dependent Absorption Coefficients
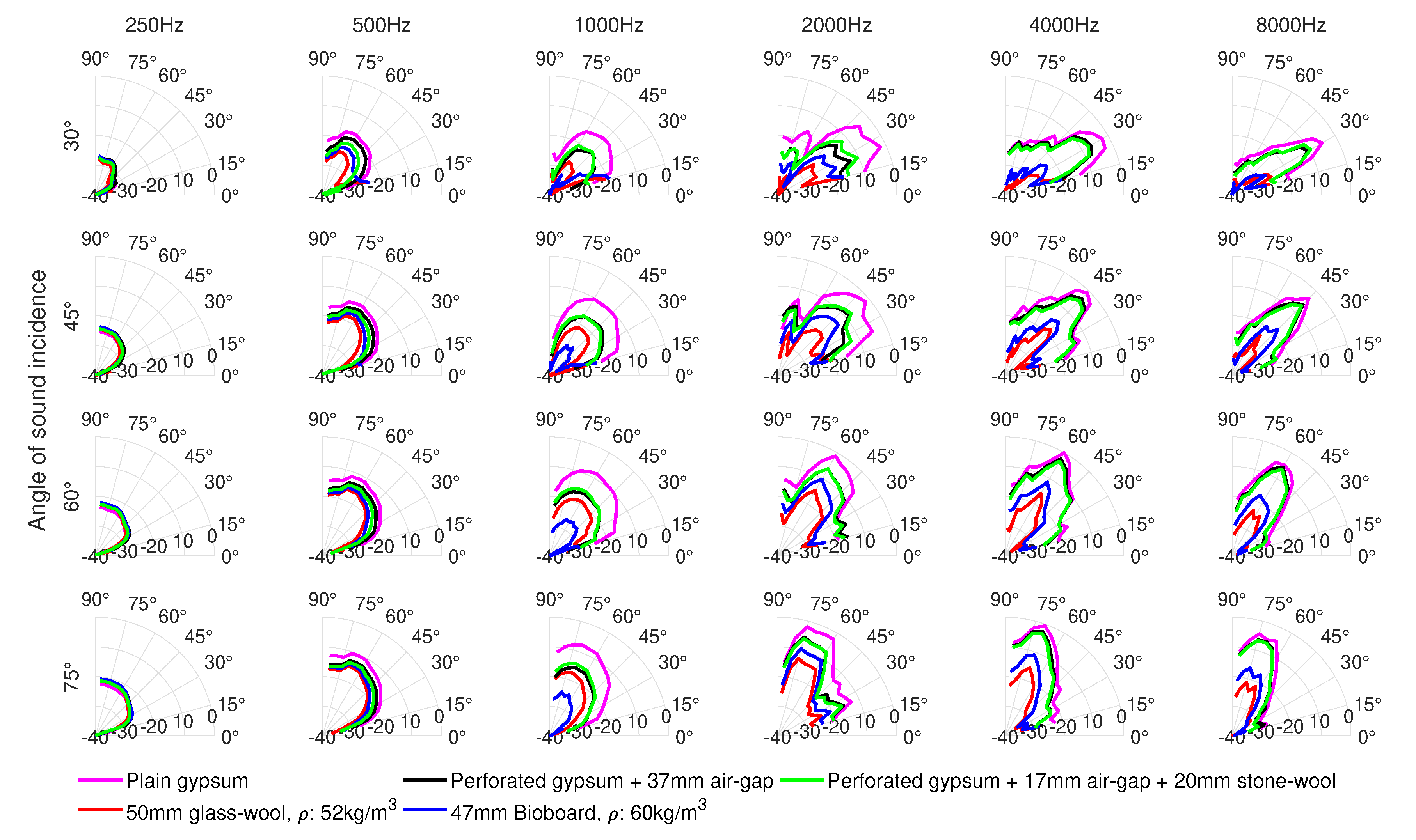
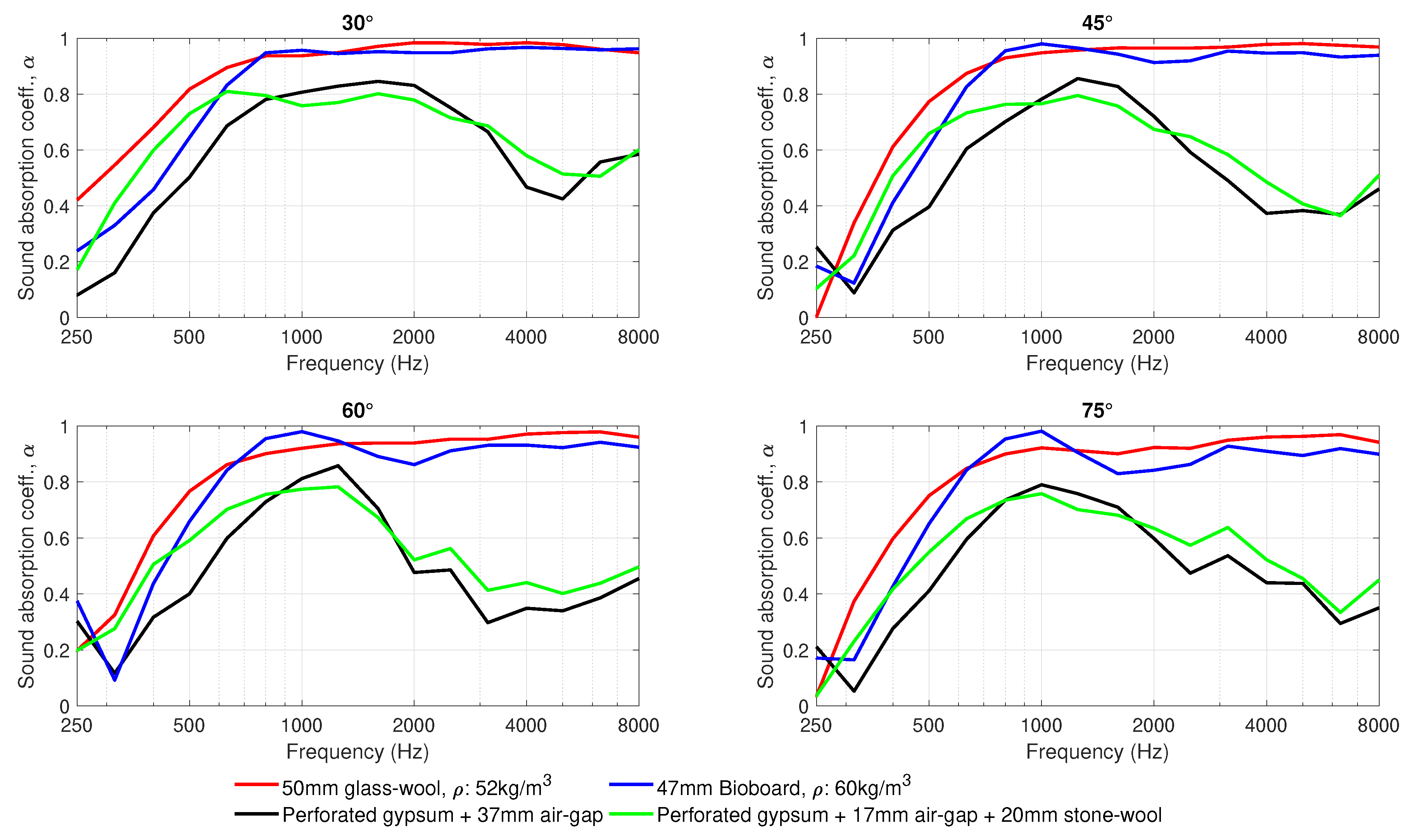
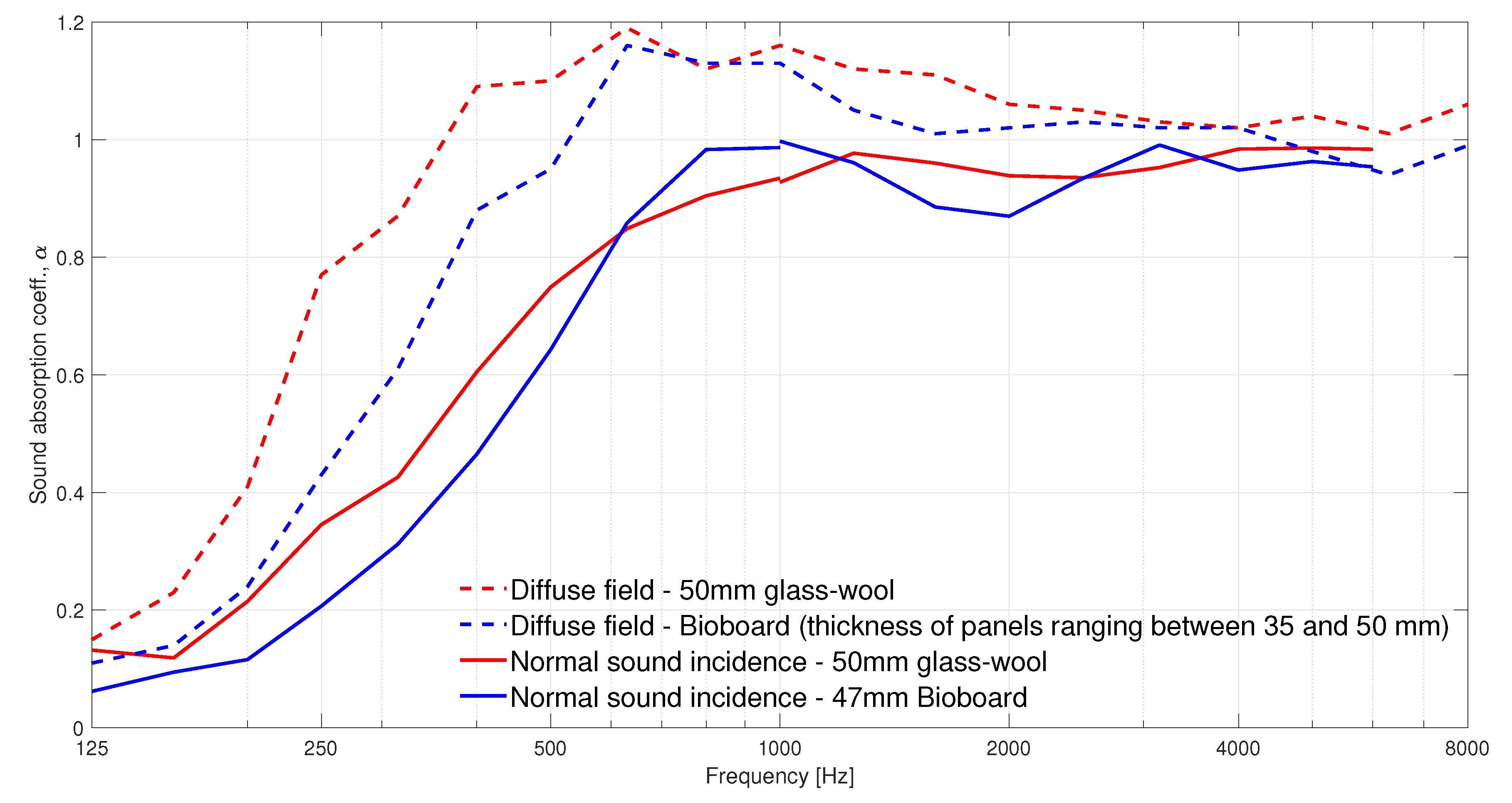
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| ISO | International Standard Organization |
| CO | Carbon dioxide |
| AES | Audio Engineering Society |
| ITA | Institute of Technical Acoustics, Aachen, Germany |
| O.d.s. | Overall depth of system |
References
- D’Antonio, P.; Cox, T. Acoustic Absorbers and Diffusers: Theory, Design and Application; Taylor & Francis: Abingdon, UK, 2004. [Google Scholar]
- Venegas, R.; Umnova, O. Influence of sorption on sound propagation in granular activated carbon. J. Acoust. Soc. Am. 2016, 140, 755–766. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Berardi, U.; Iannace, G. Acoustic characterization of natural fibers for sound absorption applications. Build. Environ. 2015, 94, 840–852. [Google Scholar] [CrossRef]
- Berardi, U.; Iannace, G. Predicting the sound absorption of natural materials: Best-fit inverse laws for the acoustic impedance and the propagation constant. Appl. Acoust. 2017, 115, 131–138. [Google Scholar] [CrossRef]
- Jiménez, N.; Romero-García, V.; Pagneux, V.; Groby, J.P. Rainbow-trapping absorbers: Broadband, perfect and asymmetric sound absorption by subwavelength panels for transmission problems. Sci. Rep. 2017, 7, 13595. [Google Scholar] [CrossRef] [PubMed]
- Tang, Y.; Ren, S.; Meng, H.; Xin, F.; Huang, L.; Chen, T.; Zhang, C.; Lu, T.J. Hybrid acoustic metamaterial as super absorber for broadband low-frequency sound. Sci. Rep. 2017, 7, 43340. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Arenas, J.P.; Asdrubali, F. Eco-materials with noise reduction properties. In Handbook of Ecomaterials; Martinez, L.M.T., Kharissova, O.V., Kharisov, B.I., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 3031–3056. [Google Scholar]
- Ciers, J.; Mandic, A.; Toth, L.D.; Op’t Veld, G. Carbon Footprint of Academic Air Travel: A Case Study in Switzerland. Sustainability 2019, 11, 80. [Google Scholar] [CrossRef] [Green Version]
- ISO 354. Acoustics—Measurements of Sound Absorption in a Reverberation Room; ISO: Geneva, Switzerland, 2003. [Google Scholar]
- ISO 10534-2. Acoustics—Determination of Sound Absorption Coefficient and Impedance in Impedance Tubes—Part 2: Transfer-Function Method; ISO: Geneva, Switzerland, 1998. [Google Scholar]
- Mommertz, E. Angle-dependent in-situ measurements of reflection coefficients using a subtraction technique. Appl. Acoust. 1995, 46, 251–263, Building Acoustics. [Google Scholar] [CrossRef]
- Nocke, C. In-situ acoustic impedance measurement using a free-field transfer function method. Appl. Acoust. 2000, 59, 253–264. [Google Scholar] [CrossRef]
- Garai, M. Measurement of the sound-absorption coefficient in situ: The reflection method using periodic pseudo-random sequences of maximum length. Appl. Acoust. 1993, 39, 119–139. [Google Scholar] [CrossRef]
- Nolan, M. Estimation of angle-dependent absorption coefficients from spatially distributed in situ measurements. J. Acoust. Soc. Am. 2020, 147, EL119–EL124. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Karjalainen, M.; Tikander, M. Reducing Artefacts of In-Situ Surface Impedance Measurements. Available online: http://pcfarina.eng.unipr.it/Public/Standing-Wave/ica01.pdf (accessed on 15 October 2020).
- Brandão, E.; Lenzi, A.; Paul, S. A Review of the In Situ Impedance and Sound Absorption Measurement Techniques. Acta Acust. United Acust. 2015, 101, 443–463. [Google Scholar] [CrossRef]
- Vorländer, M.; Mommertz, E. Definition and measurement of random-incidence scattering coefficients. Appl. Acoust. 2000, 60, 187–199. [Google Scholar] [CrossRef]
- ISO 17497-1. Acoustics—Sound-Scattering Properties of Surfaces—Part 2: Measurement of the Random-Incidence Scattering Coefficient in a Reverberation Room; ISO: Geneva, Switzerland, 2004. [Google Scholar]
- D’Antonio, P.; Cox, T. AES information document for room acoustics and sound reinforcement systems-characterization and measurement of surface scattering uniformity. J. Audio Eng. Soc. 2001, 49, 149–165. [Google Scholar]
- ISO 17497-2. Acoustics—Sound-Scattering Properties of Surfaces—Part 2: Measurement of the Directional Diffusion Coefficient in a Free Field; ISO: Geneva, Switzerland, 2012. [Google Scholar]
- Jahangiri, P.; Logawa, B.; Korehei, R.; Hodgson, M.; Martinez, D.M.; Olson, J.A. On acoustical properties of novel foam-formed cellulose-based material. Nord. Pulp Pap. Res. J. 2016, 31, 14–19. [Google Scholar] [CrossRef]
- Kerekes, R.J.; Schell, C.J. Effects of fiber length and coarseness on pulp flocculation. Tappi J.—(USA) 1995, 78, 133–139. [Google Scholar]
- Beghello, L.; Eklund, D. Some mechanisms that govern fiber flocculation. Nord. Pulp Pap. Res. J. 1997, 12, 119–123. [Google Scholar] [CrossRef]
- Lappalainen, T.; Lehmonen, J. Determinations of bubble size distribution of foam-fibre mixture using circular hough transform. Nord. Pulp Pap. Res. J. 2012, 27, 930–939. [Google Scholar] [CrossRef]
- Haimei, Z.; Ma, S.; Wu, Y. Building Materials in Civil Engineering; Elsevier: Amsterdam, The Netherlands, 2011; Volume 80, pp. 81–308. [Google Scholar]
- Berzborn, M.; Bomhardt, R.; Klein, J.; Richter, J.G.; Vorländer, M. The ITA-Toolbox: An open source MATLAB toolbox for acoustic measurements and signal processing. In Proceedings of the 43th Annual German Congress on Acoustics, Kiel, Germany, 6–9 March 2017; Volume 2017, pp. 6–9. [Google Scholar]
- Farina, A. Simultaneous measurement of impulse response and distortion with a swept-sine technique. In Audio Engineering Society Convention 108; Audio Engineering Society: New York, NY, USA, 2000. [Google Scholar]
- ISO 3382-1. Measurement of Room Acoustic Parameters—Part 1: Performance of Spaces; ISO: Geneva, Switzerland, 2009. [Google Scholar]
- Hald, J.; Song, W.; Haddad, K.; Jeong, C.H.; Richard, A. In-situ impedance and absorption coefficient measurements using a double-layer microphone array. Appl. Acoust. 2019, 143, 74–83. [Google Scholar] [CrossRef]
- Datasheet of Perforated Panels Provided by Their Manufacturer Knauf Danoline. Available online: https://knaufdanoline.com/wp-content/uploads/Data_sheet_Solopanel_UK.pdf (accessed on 31 August 2020).
- Yamaguchi, M.; Nakagawa, H.; Mizuno, T. Sound absorption mechanism of porous asphalt pavement. J. Acoust. Soc. Jpn. (E) 1999, 20, 29–43. [Google Scholar] [CrossRef] [Green Version]
- Swift, M.; Bris, P.; Horoshenkov, K. Acoustic absorption in re-cycled rubber granulate. Appl. Acoust. 1999, 57, 203–212. [Google Scholar] [CrossRef]
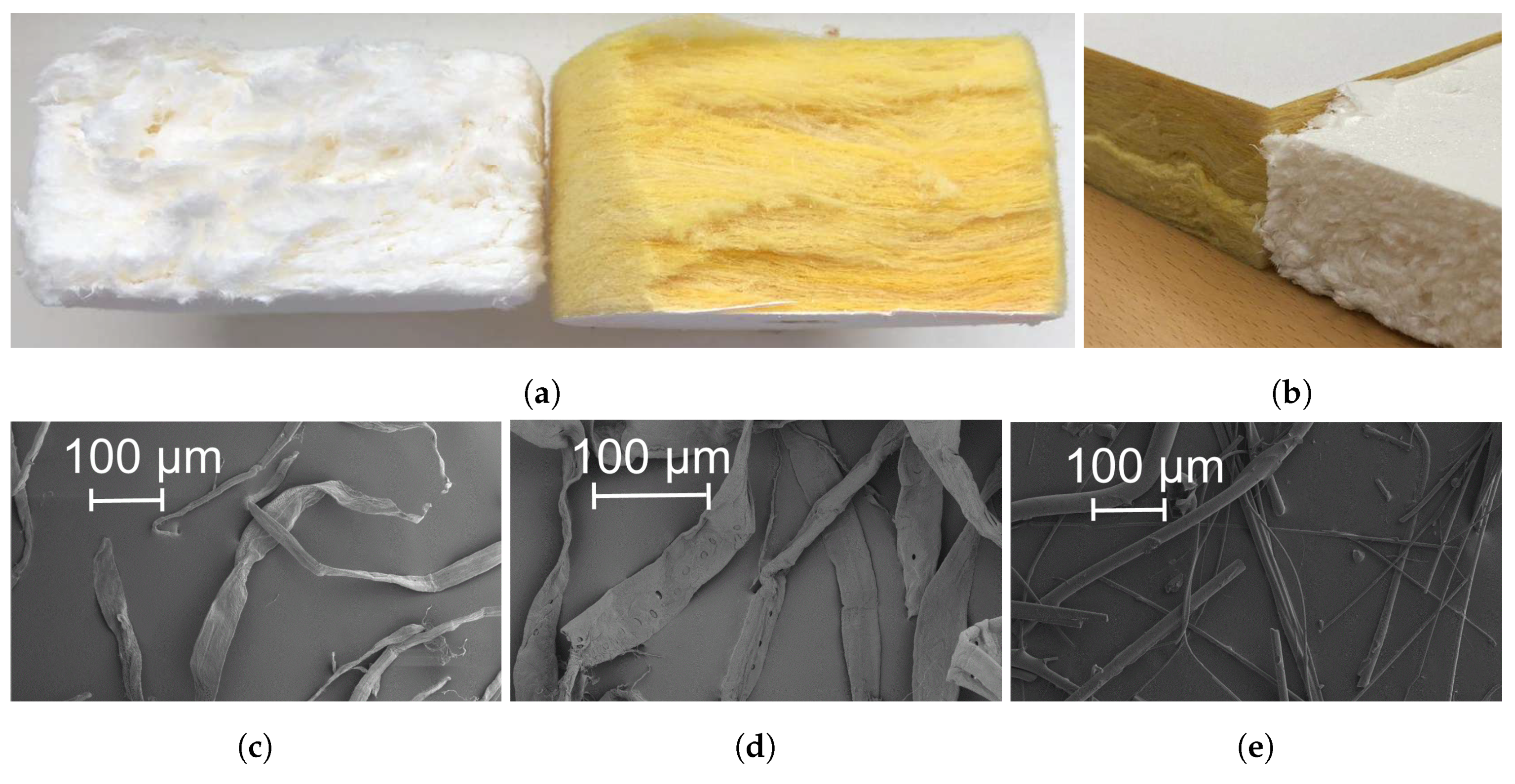


| Material | Manufacturer | o.d.s. | Density | % of Perforation |
|---|---|---|---|---|
| Plain gypsum | Knauf | 13 mm | ||
| Knauf | 13 mm + | Square 8 mm, 20% | ||
| 37 mm air-gap | ||||
| Perforated gypsum | Knauf | 13 mm + | Square 8 mm, 20% | |
| 17 mm air-gap + | ||||
| 20 mm stone wool | ||||
| Glass-wool | Ecophon | 50 mm | 52 kg/m | |
| Bioboard | Lumir | 47 mm | 60 kg/m |
| Fiber Type | Length (mm) | Width (m) | Curl (%) |
|---|---|---|---|
| 0.73 | 16.32 | 17.7 | |
| 1.97 | 25.36 | 15.4 | |
| 50–150 | 12 |
| Central Frequencies of the Octave Frequency Bands (Hz) | 125 | 250 | 500 | 1000 | 2000 | 4000 | 8000 |
|---|---|---|---|---|---|---|---|
| (dB) | 43.2 | 38.6 | 36.3 | 30.8 | 23.4 | 15.6 | 14.4 |
| 10, 15, 20 | 15 |
| 25, 30, 35 | 30 |
| 40, 45, 50 | 45 |
| 55, 60, 65 | 60 |
| 70, 75, 80 | 75 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cucharero, J.; Hänninen, T.; Lokki, T. Angle-Dependent Absorption of Sound on Porous Materials. Acoustics 2020, 2, 753-765. https://doi.org/10.3390/acoustics2040041
Cucharero J, Hänninen T, Lokki T. Angle-Dependent Absorption of Sound on Porous Materials. Acoustics. 2020; 2(4):753-765. https://doi.org/10.3390/acoustics2040041
Chicago/Turabian StyleCucharero, Jose, Tuomas Hänninen, and Tapio Lokki. 2020. "Angle-Dependent Absorption of Sound on Porous Materials" Acoustics 2, no. 4: 753-765. https://doi.org/10.3390/acoustics2040041
APA StyleCucharero, J., Hänninen, T., & Lokki, T. (2020). Angle-Dependent Absorption of Sound on Porous Materials. Acoustics, 2(4), 753-765. https://doi.org/10.3390/acoustics2040041





