Superdirective Robust Algorithms’ Comparison for Linear Arrays
Abstract
1. Introduction
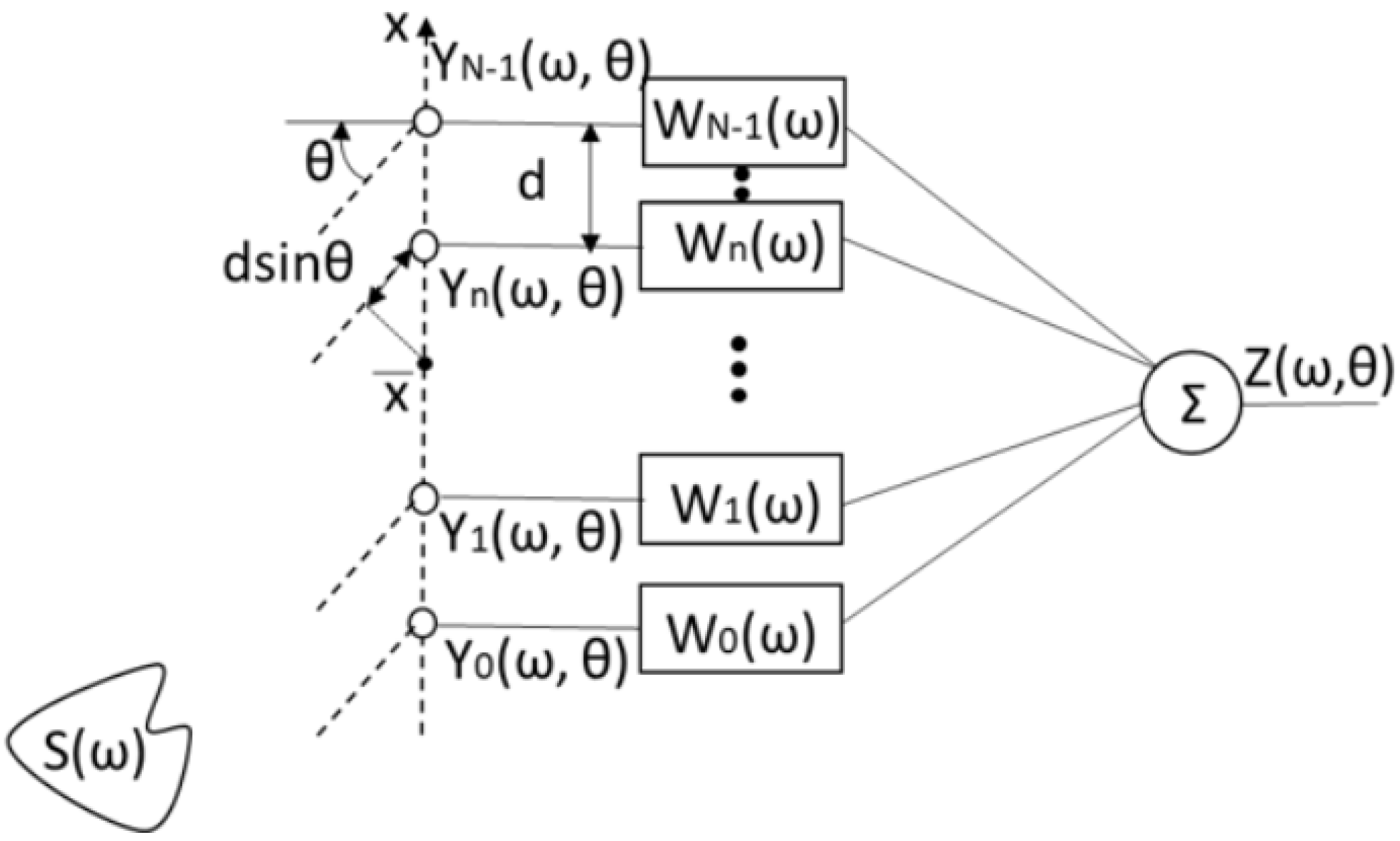
2. Materials and Methods
2.1. Proposed Methods
2.1.1. Robust Least-Squares Frequency-Invariant Beamformer Design (RLSFIB)
2.1.2. Frequency-Invariant Beam Pattern Design (FIBP)
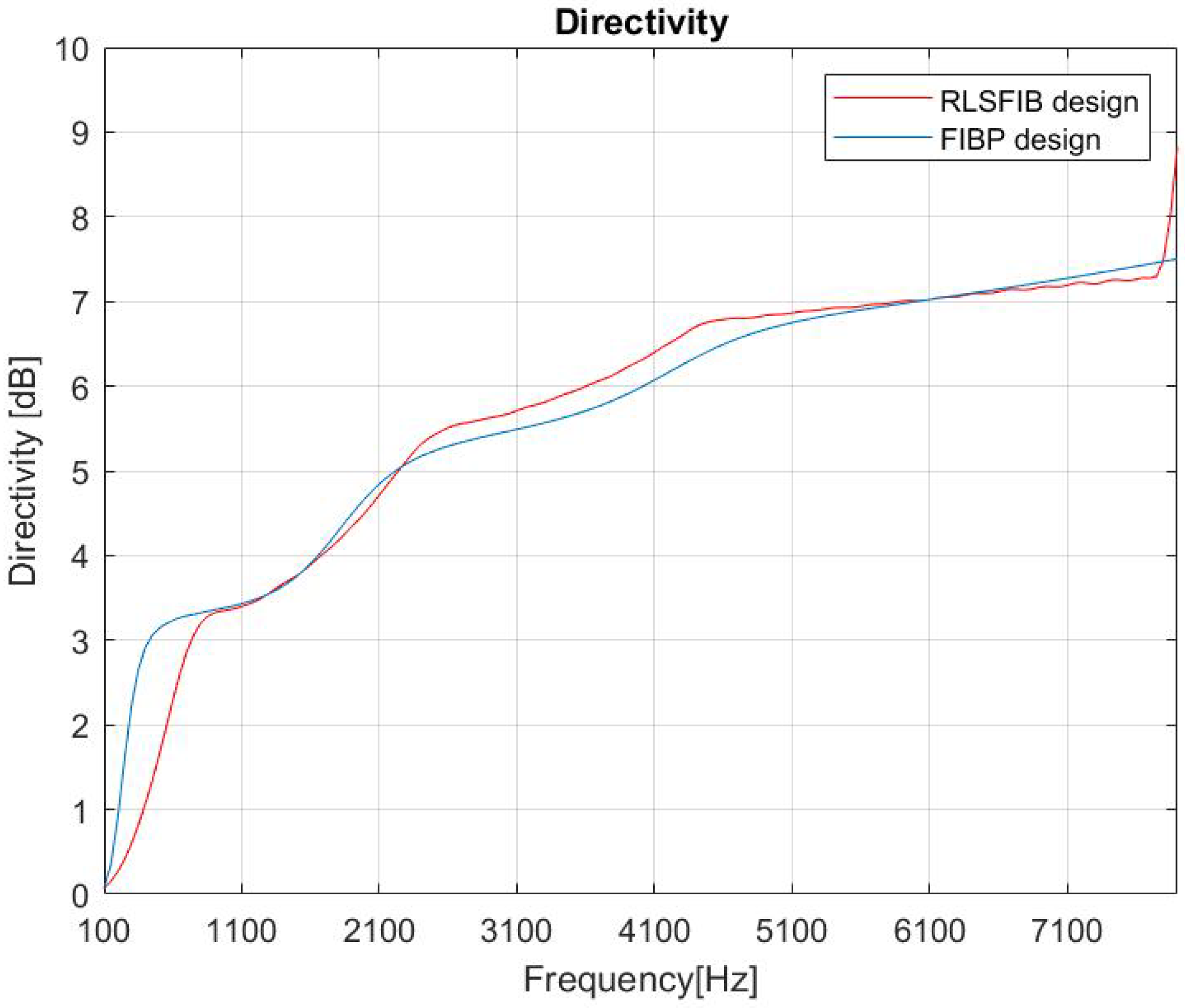
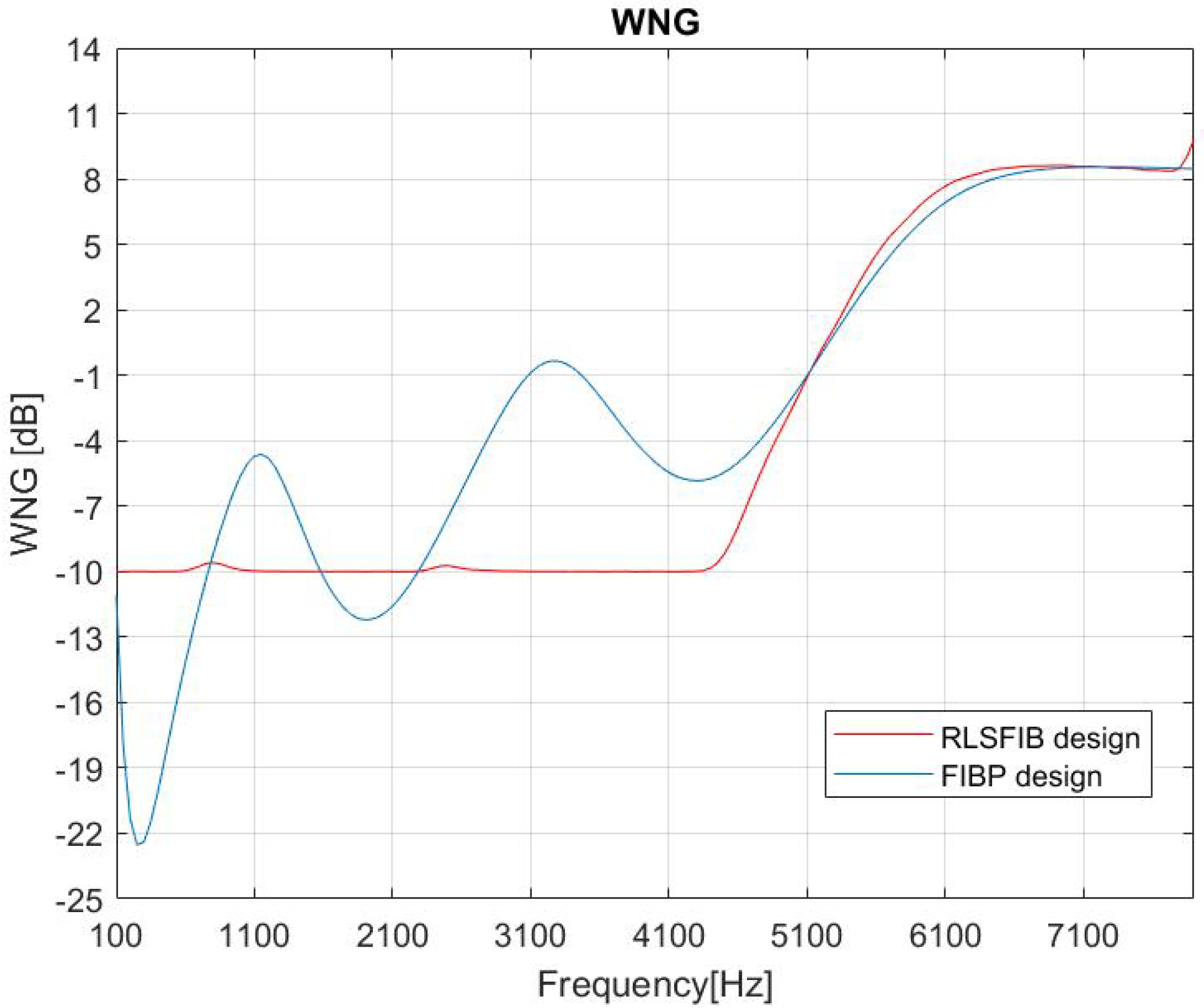
3. Results
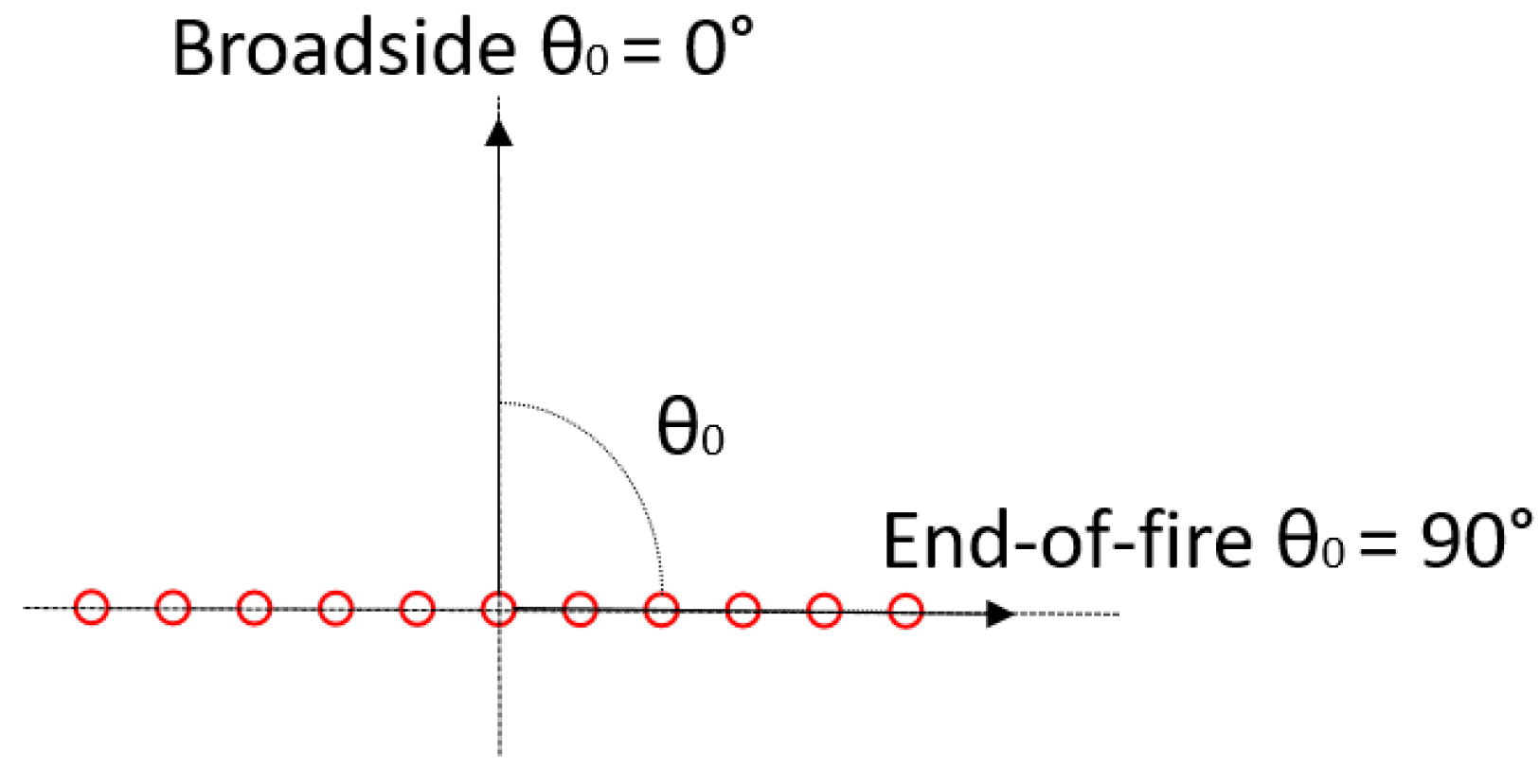
- Aperture of the array 12 cm (length for superdirective beamforming).
- 8 equi-spaced microphones (good compromise between number of microphones and final price of the array).
- Sampling frequency 16 kHz (twice the maximum frequency of the signals involved to respect the Nyquist’s theorem).
- L i.e., FIR’s filter length 128 taps.
- Frequency band of interest (100–8000) Hz (typical bandwidth for audio applications).
- Value of the speed of sound m/s.
- We used a grid of input data dividing the frequency bandwidth of interest Hz in values with a constant step of 50 Hz, and the range of the angles of the direction of arrival in values with a constant step of .
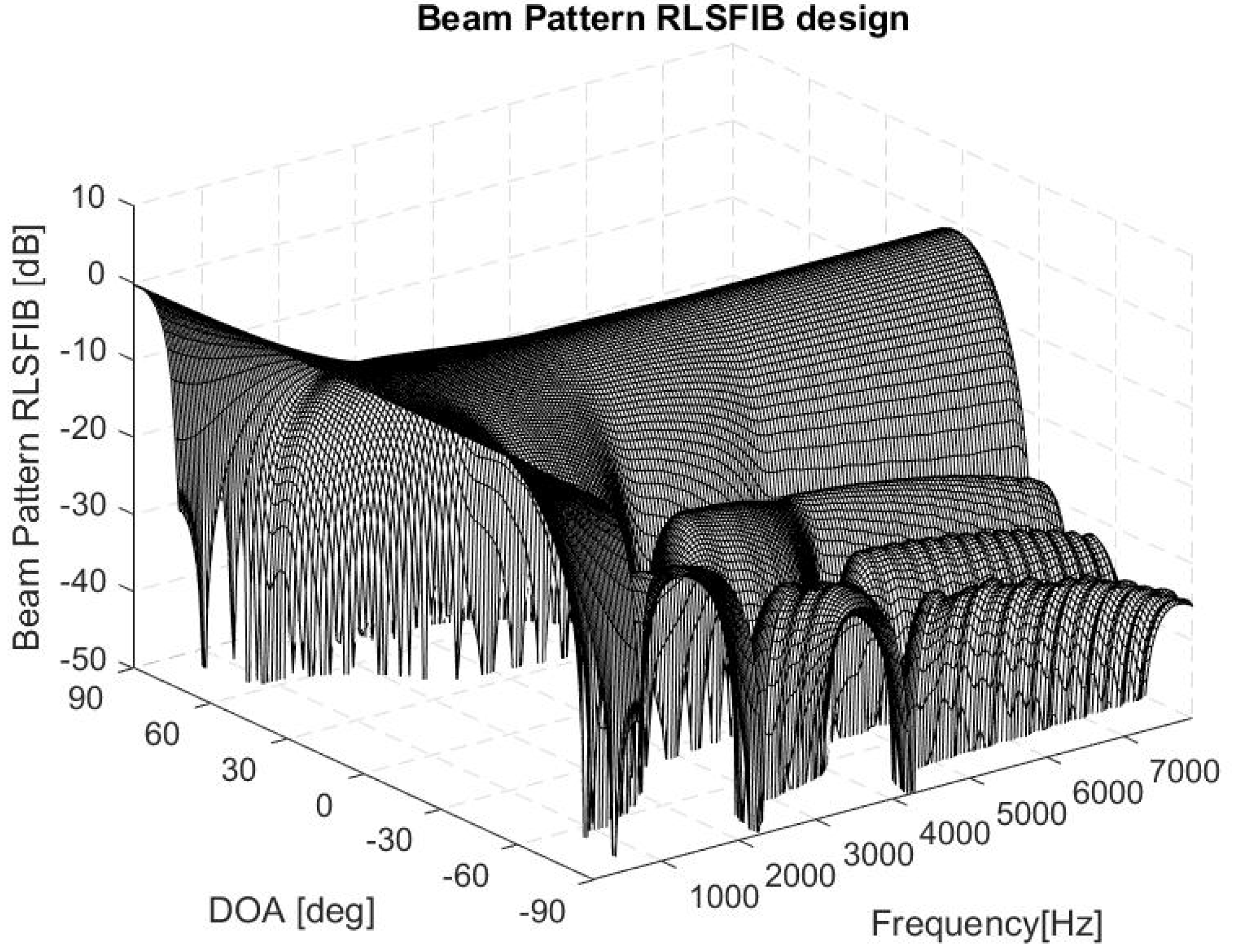
3.1. RLSFIB Algorithm
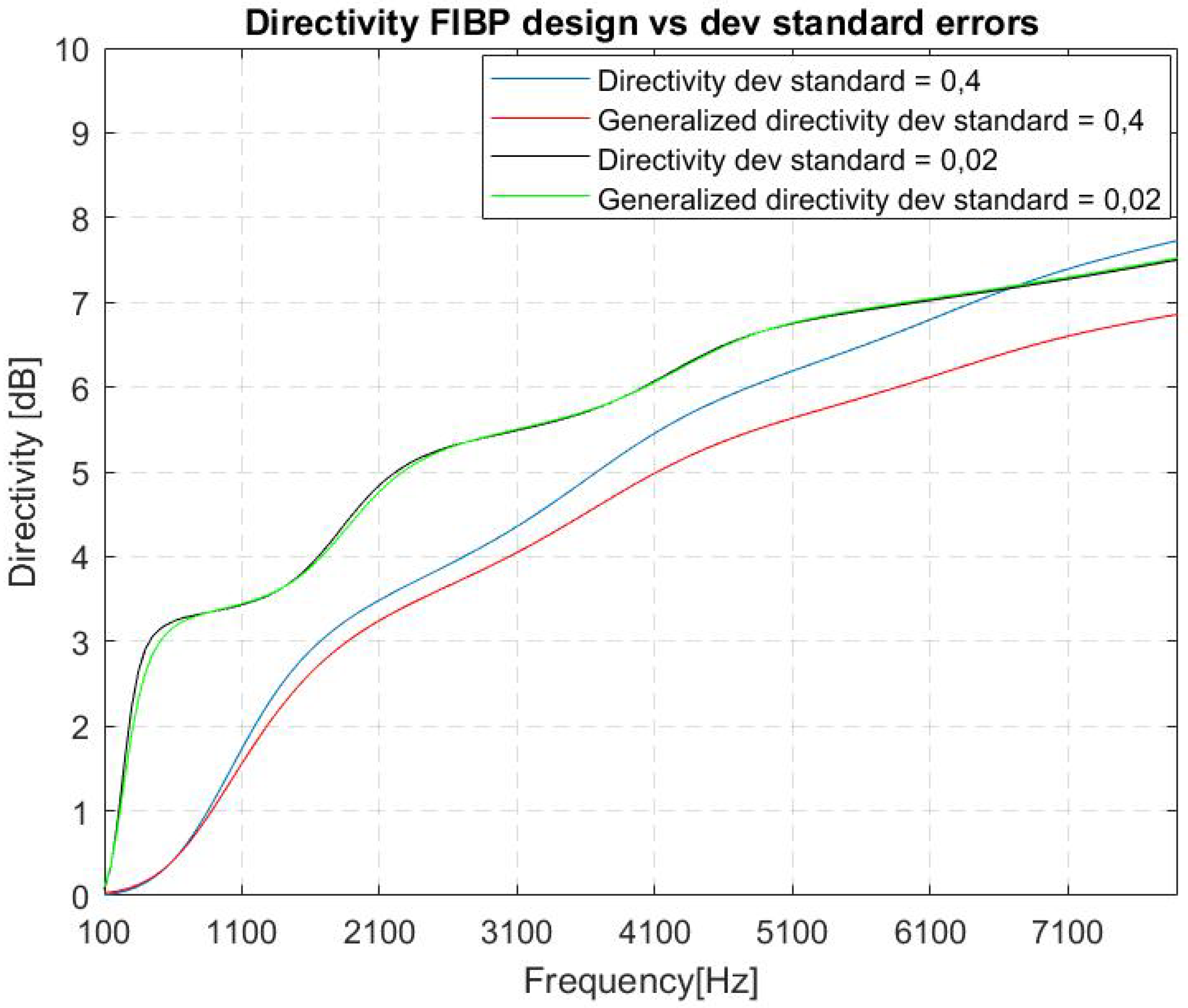
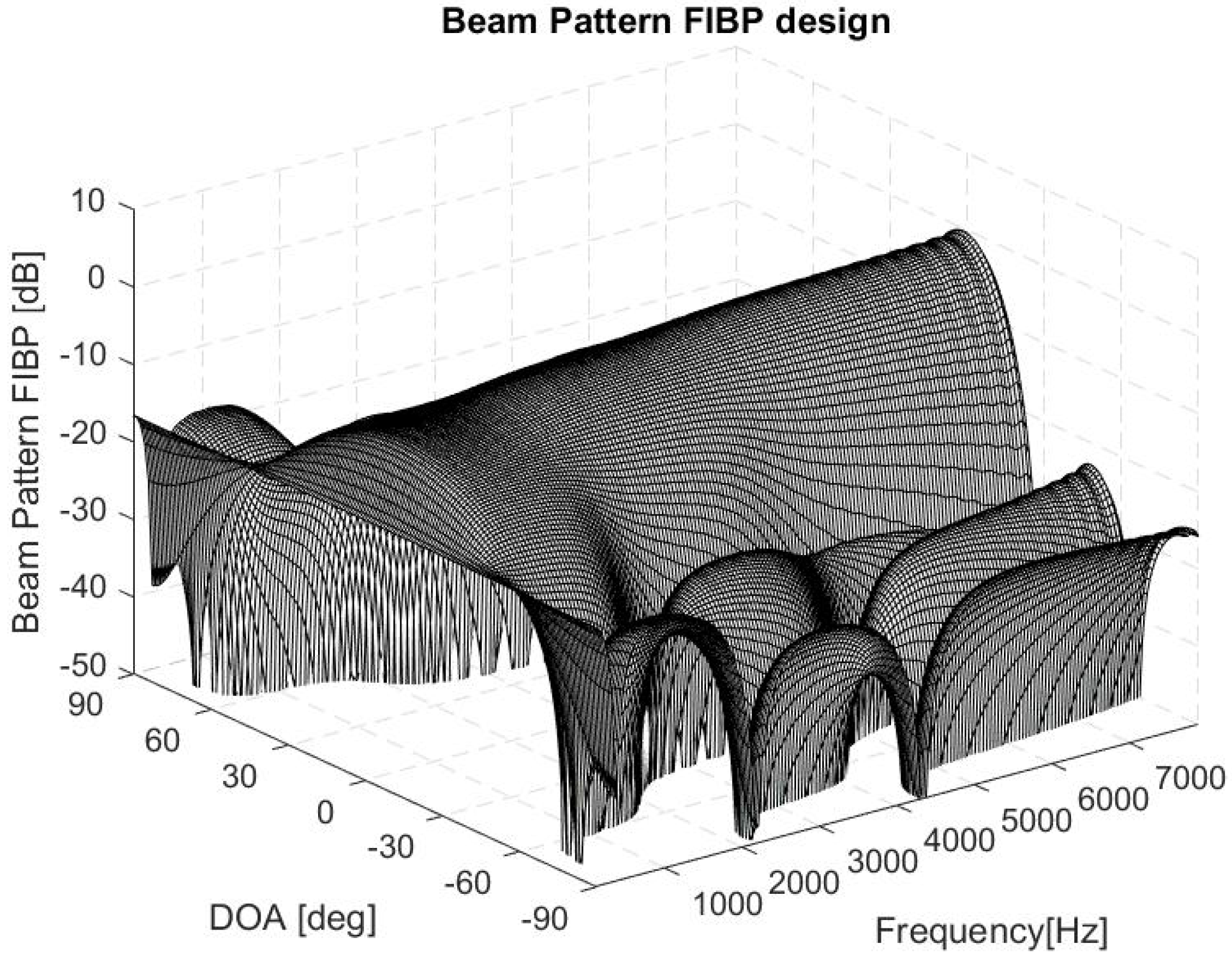
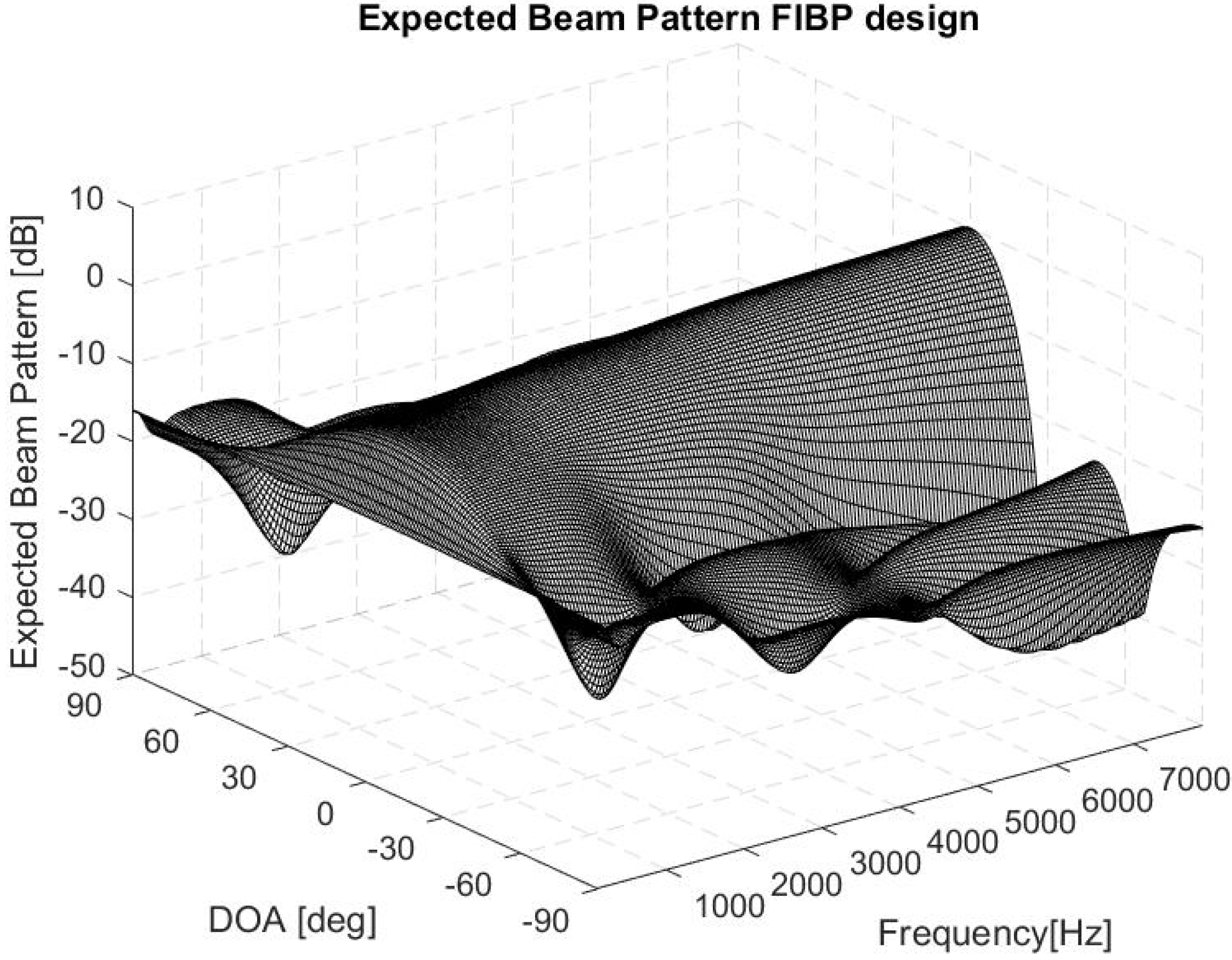
3.2. FIBP Algorithm
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Veen, B.D.V.; Buckley, K.M. Beamforming: A versatile approach to spatial filtering. IEEE Assp Mag. 1998, 5, 4–24. [Google Scholar] [CrossRef]
- Ward, D.B.; Kennedy, R.A.; Williamson, R.C. FIR filter design for frequency invariant beamformers. IEEE Signal Process. Lett. 1996, 3, 69–71. [Google Scholar] [CrossRef]
- Ward, D.B.; Kennedy, R.A.; Williamson, R.C.; Brandstein, M. Microphone Arrays Signal Processing Techniques and Applications (Digital Signal Processing); Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Trees, H.L.V. Optimum Array Processing: Part IV of Detection, Estimation, and Modulation Theory; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2002. [Google Scholar]
- Ward, D.B.; Kennedy, R.; Williamson, R. Theory and design of broadband sensor arrays with frequency invariant far-field beam patterns. J. Acoust. Soc. Am. 1999, 97, 1023–1034. [Google Scholar] [CrossRef]
- Mabande, E.; Schad, A.; Kellermann, W. Design of robust superdirective beamformers as a convex optimization problem. In Proceedings of the 2009 IEEE International Conference on Acoustics, Speech and Signal Processing, Taipei, Taiwan, 19–24 April 2009; pp. 77–80. [Google Scholar] [CrossRef]
- Crocco, M.; Trucco, A. Design of robust superdirective arrays with a tunable tradeoff between directivity and frequency-invariance. IEEE Trans. Signal Process. 2011, 59, 2169–2181. [Google Scholar] [CrossRef]
- Doclo, S.; Moonen, M. Superdirective Beamforming Robust Against Microphone Mismatch. IEEE Trans. Audio Speech Lang. Process. 2007, 15, 617–631. [Google Scholar] [CrossRef]
- Doclo, S. Multi-Microphone Noise Reduction and Dereverberation Techniques for Speech Applications. Ph.D. Thesis. Available online: ftp://ftp.esat.kuleuven.ac.be/stadius/doclo/phd/ (accessed on 23 May 2003).
- Crocco, M.; Trucco, A. The synthesis of robust broadband beamformers for equallyspaced array. J. Acoust. Soc. Am. 2010, 128, 691–701. [Google Scholar] [CrossRef] [PubMed]
- Crocco, M.; Trucco, A. Stochastic and Analytic Optimization of Sparse Aperiodic Arrays and Broadband Beamformers With Robust Superdirective Patterns. IEEE Trans. Audio Speech Lang. Process. 2012, 20, 2433–2447. [Google Scholar] [CrossRef]
- Trucco, A.; Crocco, M.; Traverso, F. Avoiding the imposition of a desired beam pattern in superdirective frequency-invariant beamformers. In Proceedings of the 26th Annual Review of Progress in Applied Computational Electromagnetics, Tampere, Finland, 26–29 April 2010; pp. 952–957. [Google Scholar]
- Doclo, S.; Moonen, M. Design of Broadband Beamformers Robust Against Gain and Phase Errors in the Microphone Array Characteristics. IEEE Trans. Signal Process. 2003, 51, 2511–2526. [Google Scholar] [CrossRef]
- Mabande, E. Robust Time-Invariant Broadband Beamforming as a Convex Optimization Problem. Ph.D. Thesis. Available online: https://opus4.kobv.de/opus4-fau/frontdoor/index/index/year/2015/docId/6138/ (accessed on 10 April 2015).
- Trucco, A.; Traverso, F.; Crocco, M. Robust Superdirective End-Fire Arrays. In Proceedings of the OCEANS’13 IEEE Bergen Conference, Bergen, Norway, 10–14 June 2013; pp. 1–6. [Google Scholar] [CrossRef]
- Traverso, F.; Crocco, M.; Trucco, A. Design of Frequency-Invariant Robust Beam Patterns by the Oversteering of End-Fire Arrays. Signal Process. 2014, 99, 129–135. [Google Scholar] [CrossRef]
- Trucco, A.; Crocco, M. Design of an Optimum Superdirective Beamformer Through Generalized Directivity Maximization. IEEE Trans. Signal Process. 2014, 62, 12. [Google Scholar] [CrossRef]
- Trucco, A.; Traverso, F.; Crocco, M. Broadband performance of superdirective delay-and-sum beamformers steered to end-fire. J. Acoust. Soc. Am. 2014, 135, EL331–EL337. [Google Scholar] [CrossRef] [PubMed]
- Crocco, M.; Trucco, A. A Synthesis Method for Robust Frequency-Invariant Very Large Bandwidth Beamforming. In Proceedings of the 18th European Signal Processing Conference (EUSIPCO 2010), Aalborg, Denmark, 23–27 August 2010; pp. 2096–2100. [Google Scholar]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Greco, D.; Trucco, A. Superdirective Robust Algorithms’ Comparison for Linear Arrays. Acoustics 2020, 2, 707-718. https://doi.org/10.3390/acoustics2030038
Greco D, Trucco A. Superdirective Robust Algorithms’ Comparison for Linear Arrays. Acoustics. 2020; 2(3):707-718. https://doi.org/10.3390/acoustics2030038
Chicago/Turabian StyleGreco, Danilo, and Andrea Trucco. 2020. "Superdirective Robust Algorithms’ Comparison for Linear Arrays" Acoustics 2, no. 3: 707-718. https://doi.org/10.3390/acoustics2030038
APA StyleGreco, D., & Trucco, A. (2020). Superdirective Robust Algorithms’ Comparison for Linear Arrays. Acoustics, 2(3), 707-718. https://doi.org/10.3390/acoustics2030038





