Self Noise Reduction and Aerodynamics of Airfoils with Porous Trailing Edges
Abstract
:1. Introduction
2. Materials and Methods
2.1. Airfoils
2.2. Wind Tunnel
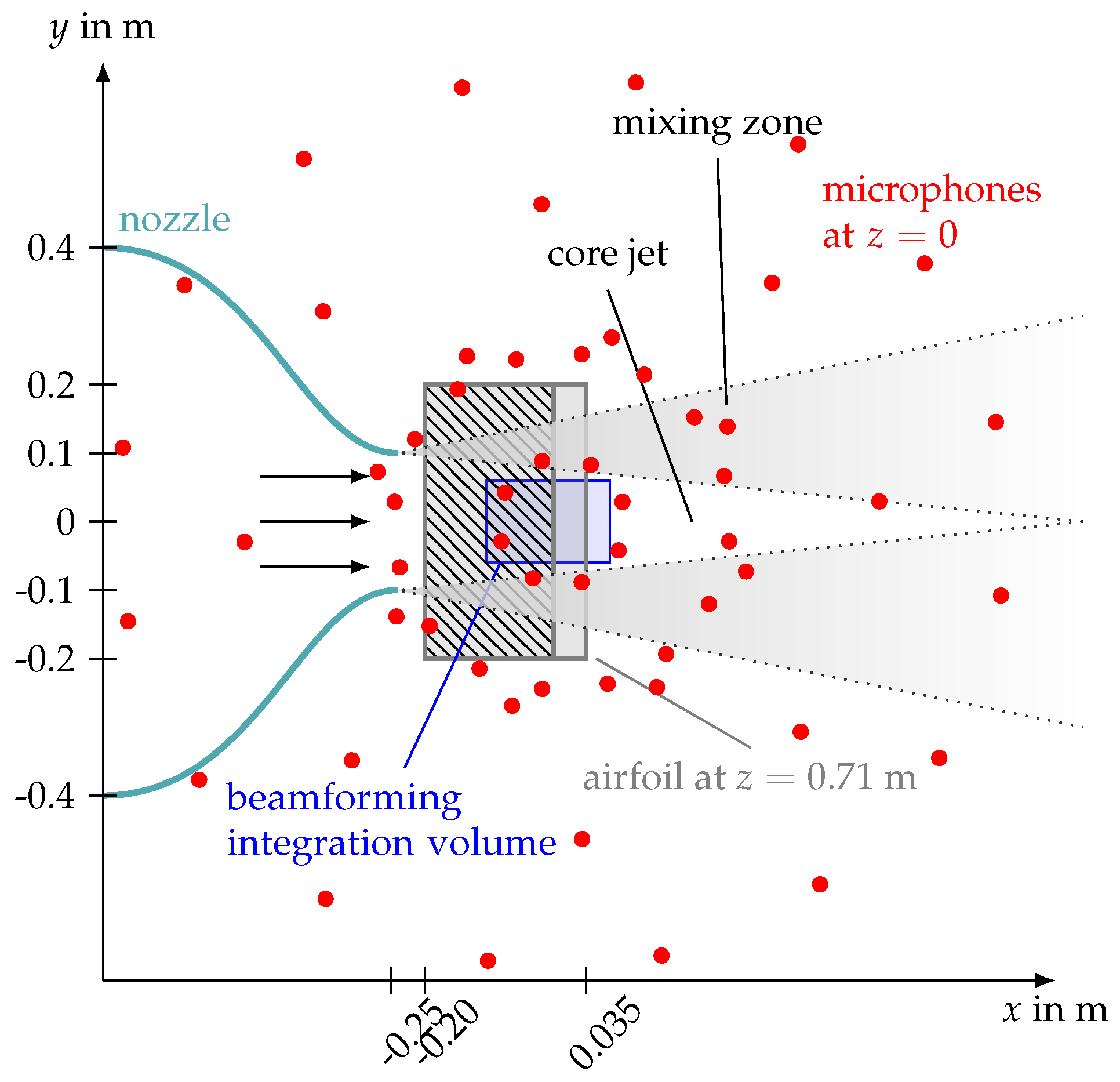
2.3. Microphone Array and Data Processing
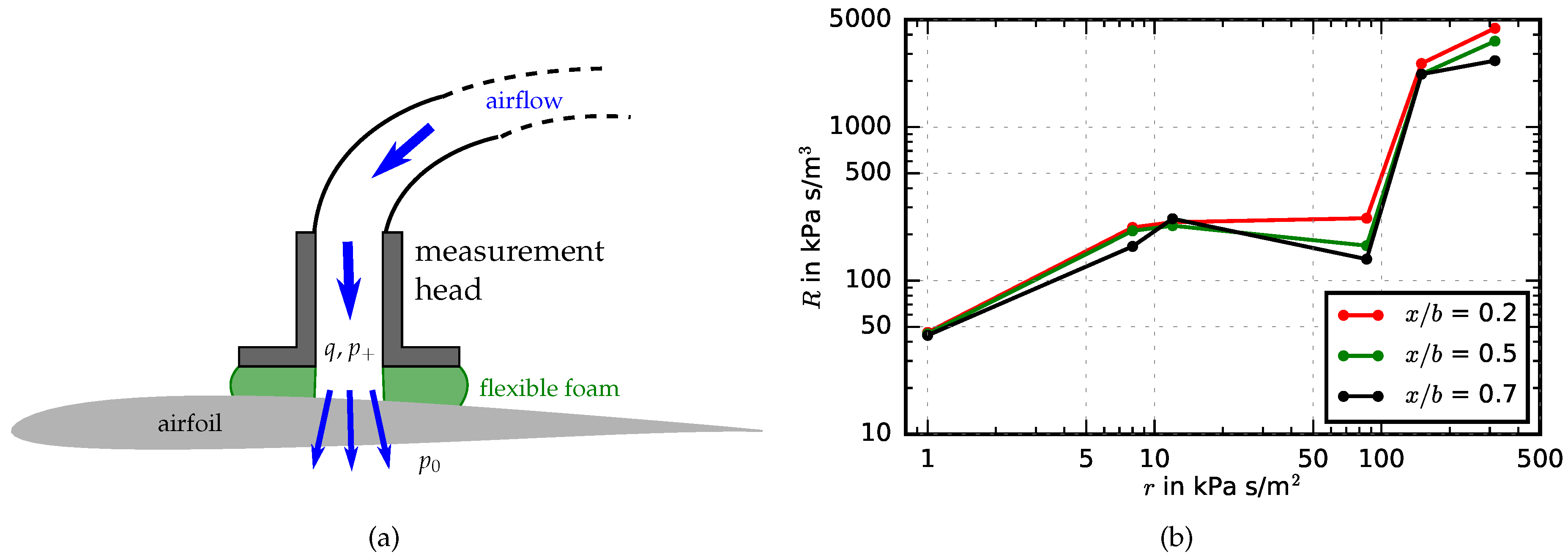
2.4. Aerodynamic Measurements
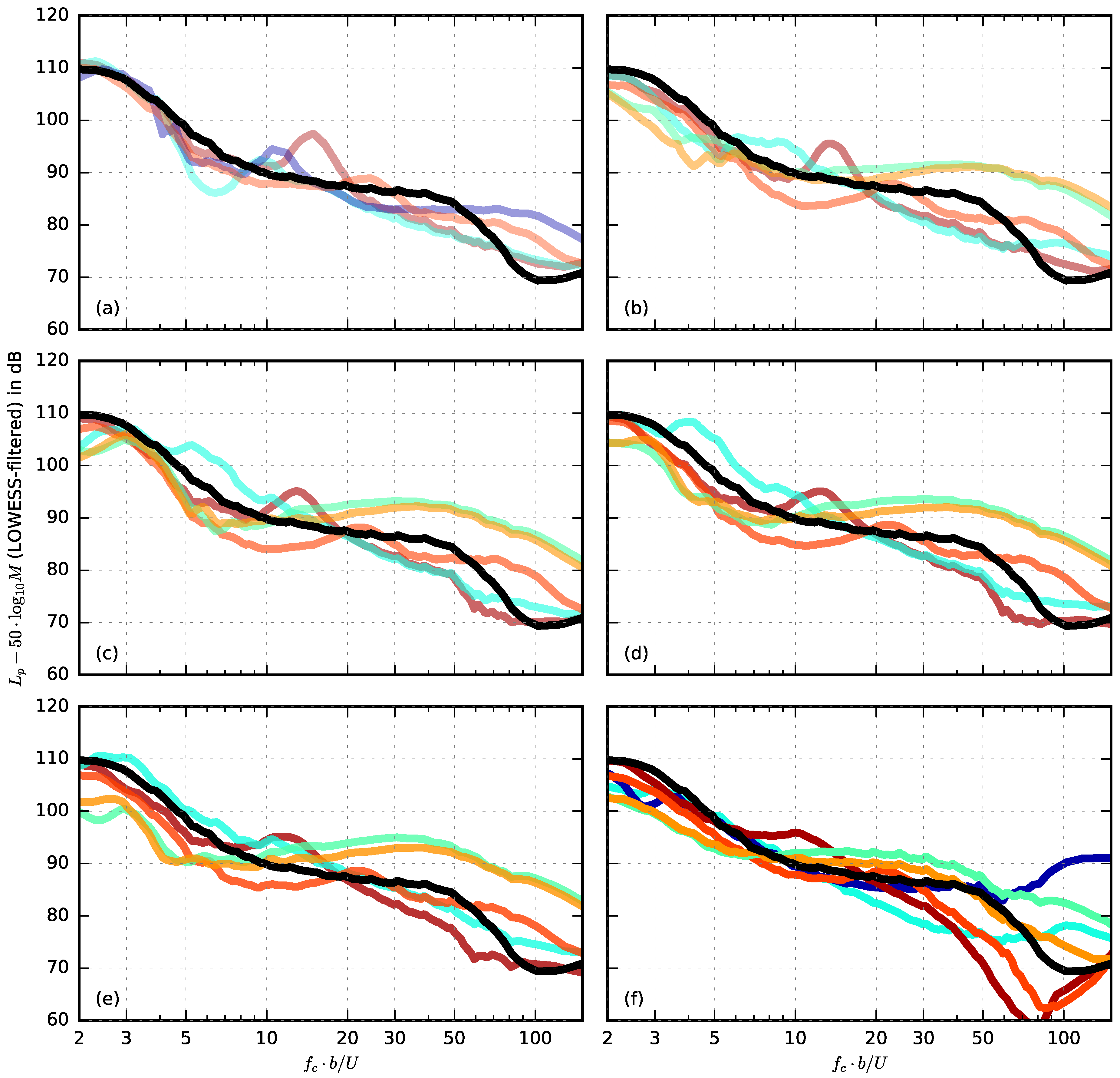
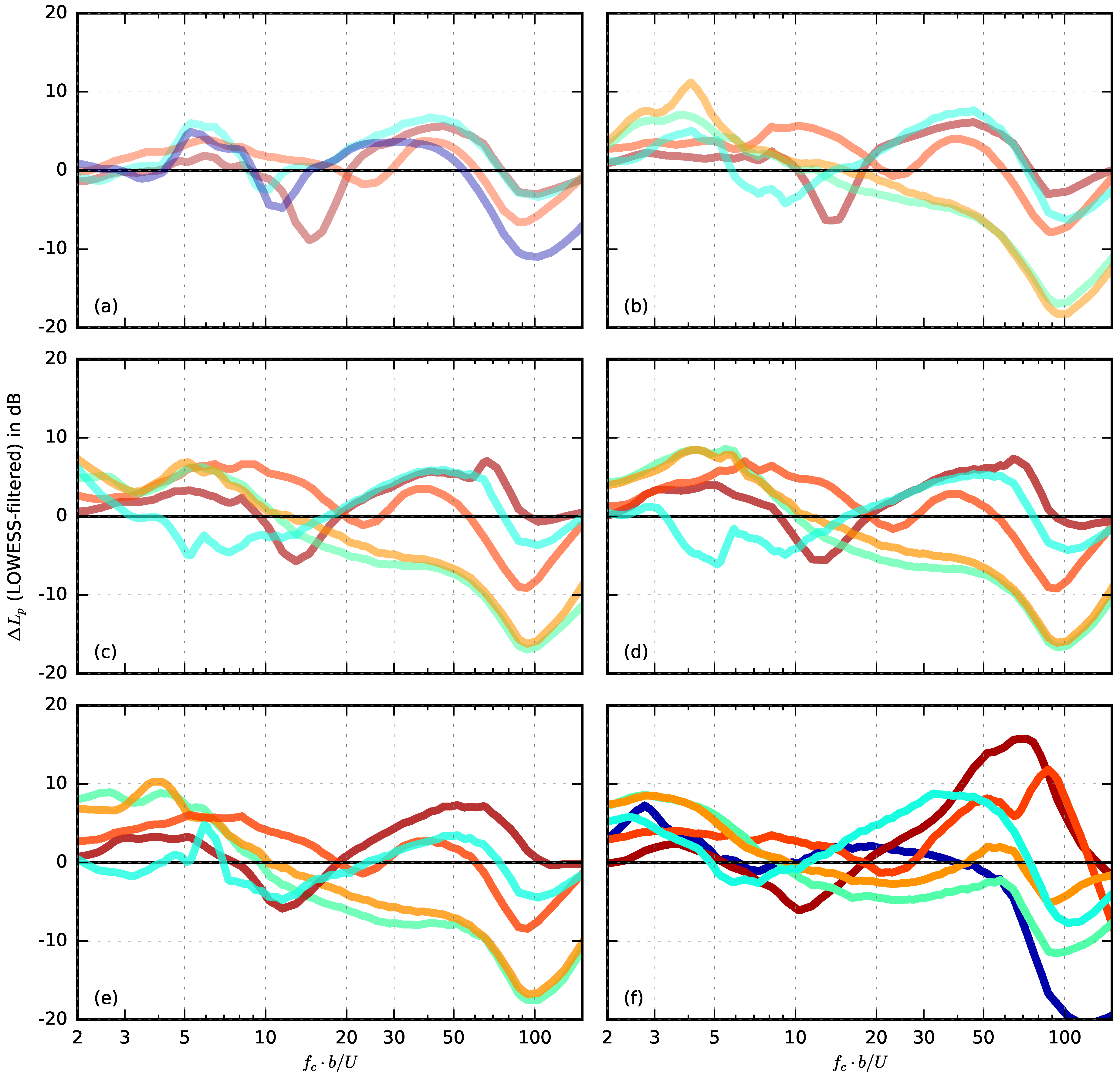
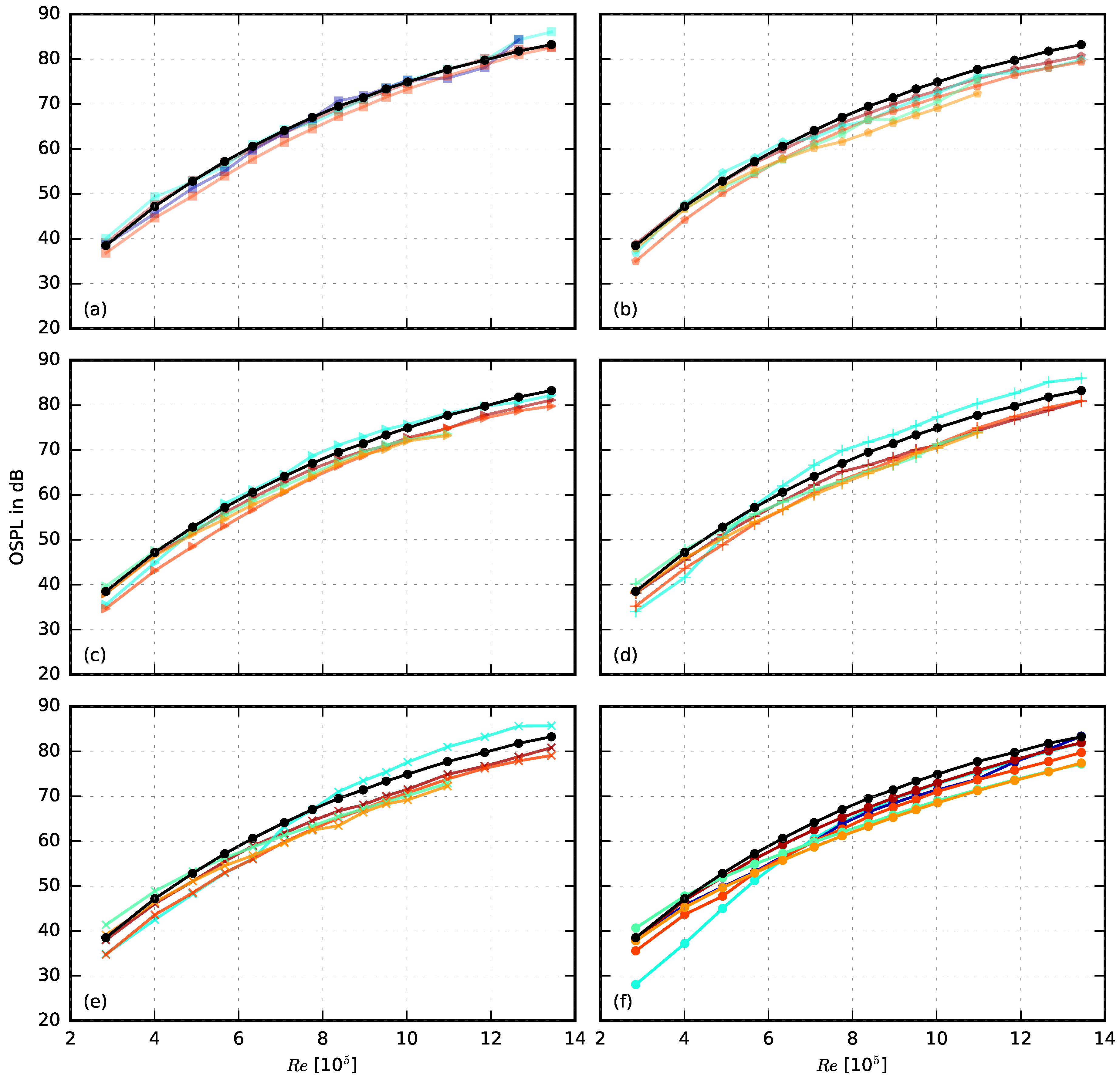
3. Results and Discussion
3.1. Aerodynamic Results
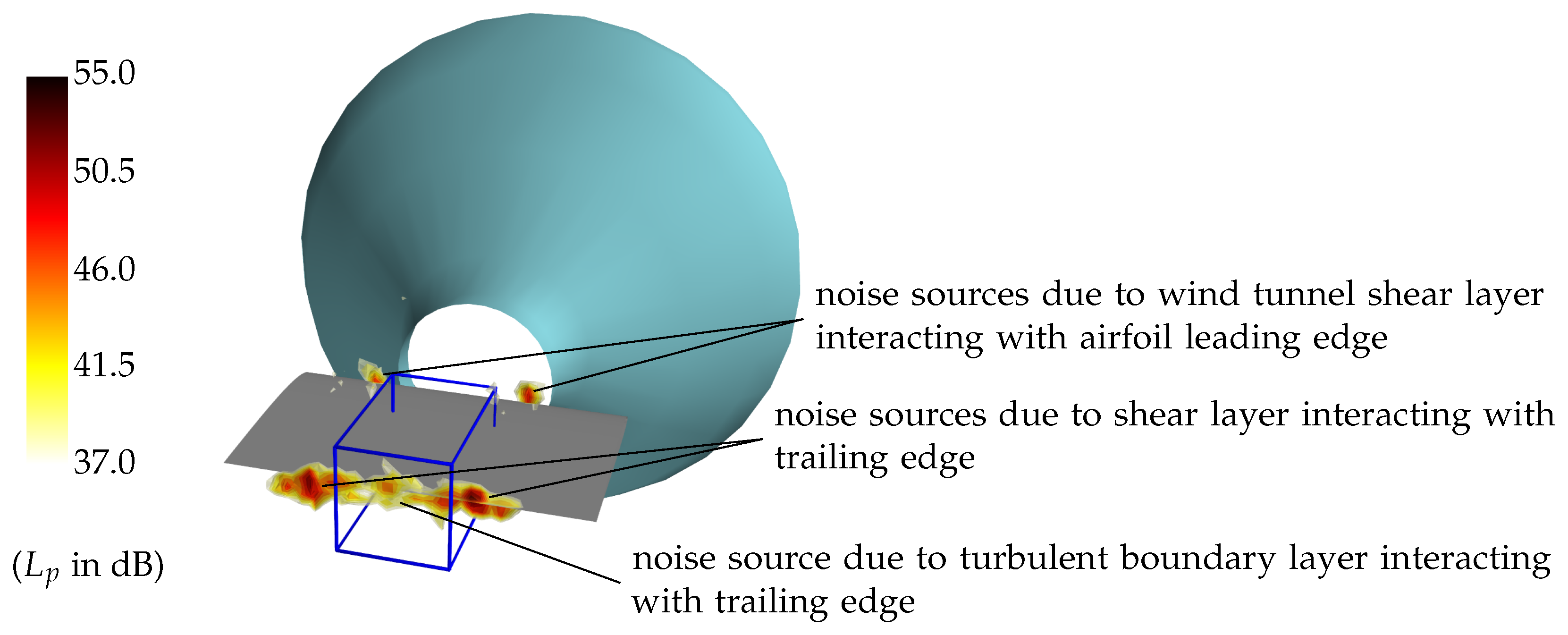
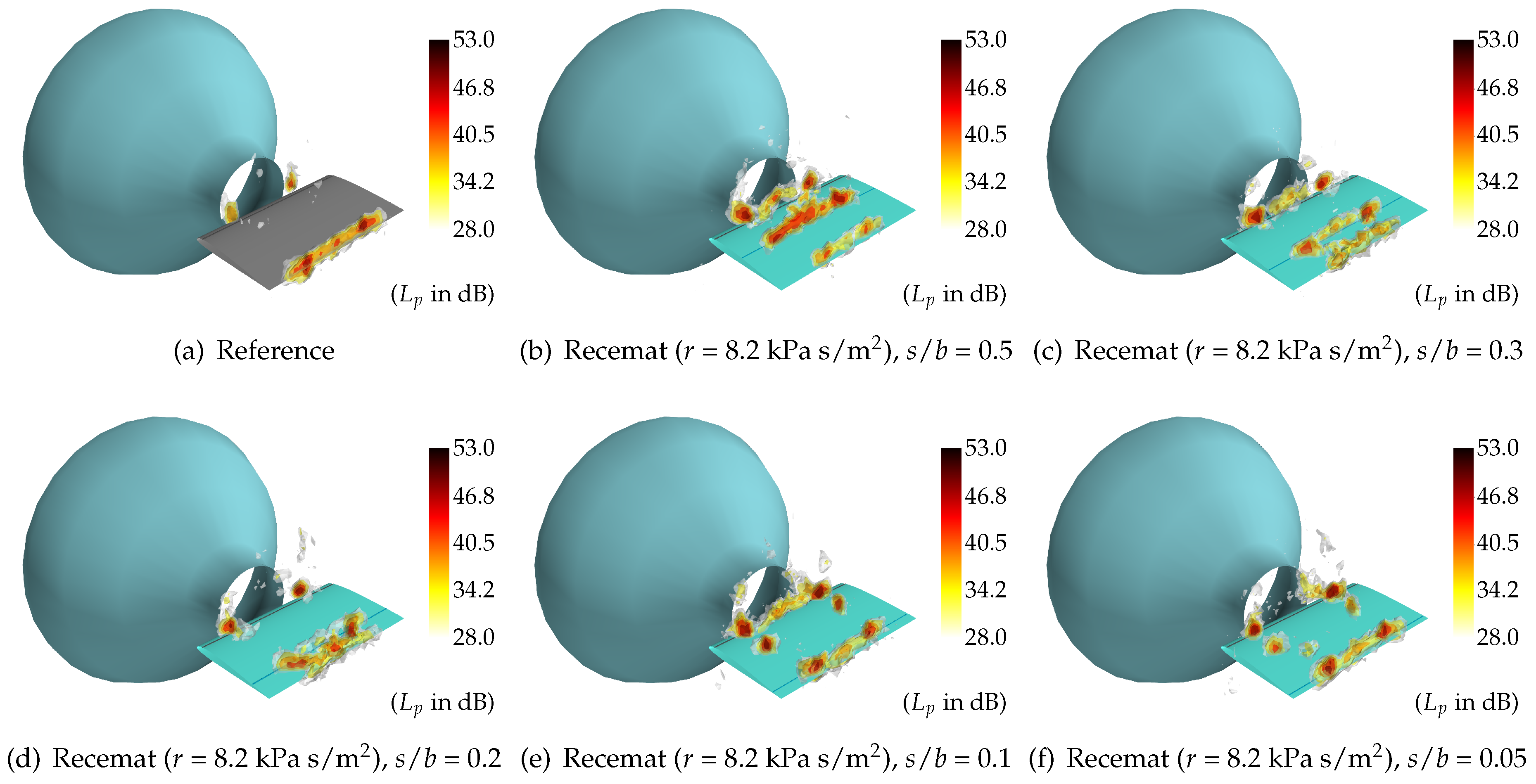
3.2. Acoustic Results
4. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | [m2] | cross sectional area of porous sample |
| b | [m] | airfoil chord length |
| c | [m/s] | speed of sound |
| [-] | drag coefficient | |
| [-] | lift coefficient | |
| [Hz] | (third octave band) center frequency | |
| [N] | drag force | |
| [N] | lift force | |
| h | [m] | thickness of a porous sample |
| M | [-] | Mach number |
| [dB] | sound pressure level | |
| [Pa] | ambient pressure | |
| q | [m3/s] | volume flow rate |
| r | [Pa·s/m2] | airflow resistivity |
| R | [Pa·s/m3] | airflow resistance |
| [-] | chord based Reynolds number | |
| s | [m] | chordwise extent of porous material |
| S | [m2] | “wetted” area of the airfoil |
| [-] | chord based Strouhal number | |
| U | [m/s] | free stream velocity (flow speed) |
| w | [m] | airfoil span width |
| x, y, z | [m] | cartesian coordinates |
| [] | geometric angle of attack | |
| [Pa] | pressure difference across porous sample | |
| [dB] | sound pressure level difference | |
| [m] | viscous characteristic length | |
| [m] | thermal characteristic length | |
| [m2/s] | kinematic viscosity of air | |
| [kg/m3] | density of air | |
| [kg/m3] | density of skeletal material | |
| [kg/m3] | total density | |
| [-] | porosity | |
| [-] | tortuosity |
References
- Herr, M. Design criteria for low-noise trailing edges. In Proceedings of the 13th AIAA/CEAS Aeroacoustics Conference, Rome, Italy, 21–23 May 2007. [Google Scholar]
- Finez, A.; Jondeau, E.; Roger, M.; Jacob, M.C. Broadband noise reduction with trailing edge brushes. In Proceedings of the 16th AIAA/CEAS Aeroacoustics Conference, Stockholm, Sweden, 7–9 June 2010. [Google Scholar]
- Sudhakaran, R.; Mimani, A.; Porteous, R.; Doolan, C.J. An experimental investigation of the flow-induced noise generated by a porous trailing edge of a flat plate. In Proceedings of the Acoustics, Australian Acoustical Society, Hunter Valley, Australia, 15–18 November 2015. [Google Scholar]
- Geyer, T.F.; Sarradj, E.; Fritzsche, C. Measurement of the noise generation at the trailing edge of porous airfoils. Exp. Fluids 2010, 48, 291–308. [Google Scholar] [CrossRef]
- Geyer, T.F.; Sarradj, E.; Fritzsche, C. Porous airfoils: noise reduction and boundary layer effects. Int. J. Aeroacoust. 2010, 9, 787–820. [Google Scholar] [CrossRef]
- Herr, M.; Reichenberger, J. In search of airworthy trailing-edge noise reduction means. In Proceedings of the 17th AIAA/CEAS Aeroacoustics Conference, Portland, OR, USA, 5–8 June 2011. [Google Scholar]
- Herr, M.; Rossignol, K.S.; Delfs, J.; Lippitz, N.; Mößner, M. Specification of porous materials for low-noise trailing-edge applications. In Proceedings of the 20th AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 16–20 June 2014. [Google Scholar]
- Carpio, A.R.; Martínez, R.M.; Avallone, F.; Ragni, D.; Snellen, M.; van der Zwaag, S. Experimental characterization of the turbulent boundary layer over a porous trailing edge for noise abatement. J. Sound Vib. 2019, 443, 537–558. [Google Scholar] [CrossRef]
- Ali, S.A.S.; Azarpeyvand, M.; da Silva, C.R.I. Trailing-edge flow and noise control using porous treatments. J. Fluid Mech. 2018, 850, 83–119. [Google Scholar]
- Jiang, C.; Moreau, D.J.; Yauwenas, Y.; Fischer, J.R.; Doolan, C.J.; Gao, J.; Kingan, M. Control of rotor trailing edge noise using porous additively manufactured blades. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Vancouver, BC, Canada, 5–7 May 2018. [Google Scholar]
- Vathylakis, A.; Chong, T.P.; Joseph, P.F. Poro-serrated trailing-edge devices for airfoil self-noise reduction. AIAA J. 2015, 53, 3379–3394. [Google Scholar] [CrossRef]
- Kisil, A.; Ayton, L.J. Aerodynamic noise from rigid trailing edges with finite porous extentions. J. Fluid Mech. 2018, 836, 117–144. [Google Scholar] [CrossRef]
- Bae, Y.; Moon, Y.J. Effect of passive porous surface on the trailing-edge noise. Phys. Fluids 2011, 23, 126101. [Google Scholar] [CrossRef]
- Faßmann, B.W.; Rautmann, C.; Ewert, R.; Delfs, J.W. Prediction of porous trailing edge noise reduction via acoustic perturbation equations and volume averaging. In Proceedings of the 21st AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 16–20 June 2015. [Google Scholar]
- Zhou, B.Y.; Gauger, N.R.; Koh, S.R.; Meinke, M.; Schröder, W. On the adjoint-based control of trailing-edge turbulence and noise minimization via porous material. In Proceedings of the 21st AIAA/CEAS Aeroacoustics Conference, Dallas, TX, USA, 22–26 June 2018. [Google Scholar]
- Koh, S.R.; Meinke, M.; Schröder, W. Numerical analysis of the impact of permeability on trailing-edge noise. J. Sound Vib. 2018, 421, 348–376. [Google Scholar] [CrossRef]
- Geyer, T.F.; Sarradj, E. Trailing edge noise of partially porous airfoils. In Proceedings of the 20th AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 16–20 June 2014. [Google Scholar]
- Selig, M.S.; Donovan, J.F.; Fraser, D.B. Airfoils at Low Speeds; Stokely, H.A., Ed.; SoarTech Publications: Virginia Beach, VA, USA, 1989; Volume 121. [Google Scholar]
- ISO 9053. Acoustics—Materials for Acoustical Applications—Determination of Airflow Resistance; International Organization for Standardization: Geneva, Switzerland, 1993. [Google Scholar]
- Jaouen, L.; Chevillotte, F. The acoustic characterization of porous media and its standards. In Proceedings of the INTER-NOISE and NOISE-CON Congress and Conference Proceedings, Hamburg, Germany, 21–24 August 2016; Volume 253, pp. 725–730. [Google Scholar]
- Sarradj, E.; Fritzsche, C.; Geyer, T.F.; Giesler, J. Acoustic and aerodynamic design and characterization of a small-scale aeroacoustic wind tunnel. Appl. Acoust. 2009, 70, 1073–1080. [Google Scholar] [CrossRef]
- Geyer, T.F. Trailing edge noise generation of porous airfoils. Ph.D. Thesis, Brandenburg University of Technology, Cottbus, Germany, 2011. [Google Scholar]
- Merino-Martinez, R.; et al. A review of acoustic imaging methods using phased microphone arrays. CEAS Aeronautical J. 2019. [Google Scholar] [CrossRef]
- Sijtsma, P. CLEAN based on spatial source coherence. Int.J. Aeroacoust. 2007, 6, 357–374. [Google Scholar] [CrossRef]
- Sarradj, E. Three-dimensional acoustic source mapping with different beamforming steering vector formulations. Adv. Acoust. Vib. 2012. [Google Scholar] [CrossRef]
- Sarradj, E. A fast ray casting method for sound refraction at shear layers. Int. J. Aeroacoust. 2016, 16, 65–77. [Google Scholar] [CrossRef]
- Von Schulz-Hausmann, F.K. Wechselwirkung ebener Freistrahlen mit der Umgebung. VDI Fortschr. Strömungstech. 1985, 52, 56. [Google Scholar] [CrossRef]
- Brooks, T.F.; Pope, D.S.; Marcolini, M.A. Airfoil Self-Noise and Prediction; National Aeronautics and Space Administration, Office of Management, Scientific and Technical Information Division: Washington, DC, USA, 1989. [Google Scholar]
- Gruber, M.; Joseph, P.; Chong, T.P. Experimental investigation of airfoil self noise and turbulent wake reduction by the use of trailing edge serrations. In Proceedings of the 16th AIAA/CEAS Aeroacoustics Conference, Stockholm, Sweden, 7–9 June 2010. [Google Scholar]
- Moreau, D.J.; Doolan, C.J. Noise-reduction mechanism of a flat-plate serrated trailing edge. AIAA J. 2013, 51, 2513–2522. [Google Scholar] [CrossRef]
- Blake, W. Noncavitating lifting sections. In Mechanics of Flow-Induced Sound and Vibration, Volume II: Complex Flow-Structure Interactions; Academic Press, Inc.: Cambridge, MA, USA, 1986; pp. 718–836. [Google Scholar]
- Cleveland, W.S. Robust locally weighted regression and smoothing scatterplots. J. Am. Stat. Assoc. 1979, 74, 829–836. [Google Scholar] [CrossRef]
- Cleveland, W.S.; Devlin, S.J. Locally weighted regression: An approach to regression analysis by local fitting. J. Am. Stat. Assoc. 1988, 83, 596–610. [Google Scholar] [CrossRef]



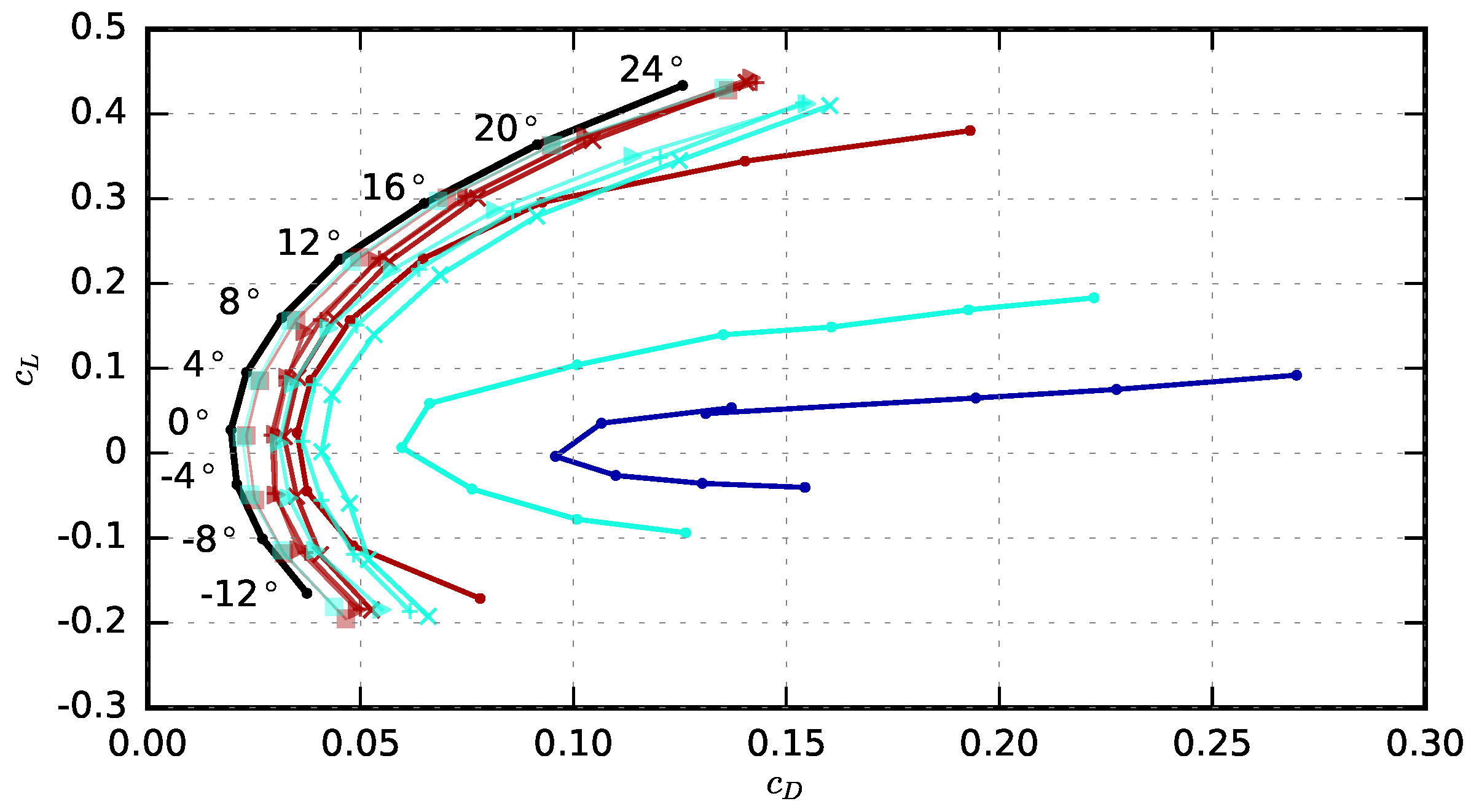
 non-porous reference airfoil, r = ∞,
non-porous reference airfoil, r = ∞,  Porex, r = 316,500 Pa·s/m2,
Porex, r = 316,500 Pa·s/m2,  Siperm, r = 150,000 Pa·s/m2,
Siperm, r = 150,000 Pa·s/m2,  Damtec estra, r = 86,100 Pa·s/m2,
Damtec estra, r = 86,100 Pa·s/m2,  Damtec SMB K 20, r = 12,900 Pa·s/m2,
Damtec SMB K 20, r = 12,900 Pa·s/m2,  Recemat, r = 8200 Pa·s/m2,
Recemat, r = 8200 Pa·s/m2,  M-Pore Al 45, r = 1000 Pa·s/m2). (a) Lift coefficient as a function of chord based Reynolds number; (b) Drag coefficient as a function of chord based Reynolds number.
M-Pore Al 45, r = 1000 Pa·s/m2). (a) Lift coefficient as a function of chord based Reynolds number; (b) Drag coefficient as a function of chord based Reynolds number.
 non-porous reference airfoil, r = ∞,
non-porous reference airfoil, r = ∞,  Porex, r = 316,500 Pa·s/m2,
Porex, r = 316,500 Pa·s/m2,  Siperm, r = 150,000 Pa·s/m2,
Siperm, r = 150,000 Pa·s/m2,  Damtec estra, r = 86,100 Pa·s/m2,
Damtec estra, r = 86,100 Pa·s/m2,  Damtec SMB K 20, r = 12,900 Pa·s/m2,
Damtec SMB K 20, r = 12,900 Pa·s/m2,  Recemat, r = 8200 Pa·s/m2,
Recemat, r = 8200 Pa·s/m2,  M-Pore Al 45, r = 1000 Pa·s/m2). (a) Lift coefficient as a function of chord based Reynolds number; (b) Drag coefficient as a function of chord based Reynolds number.
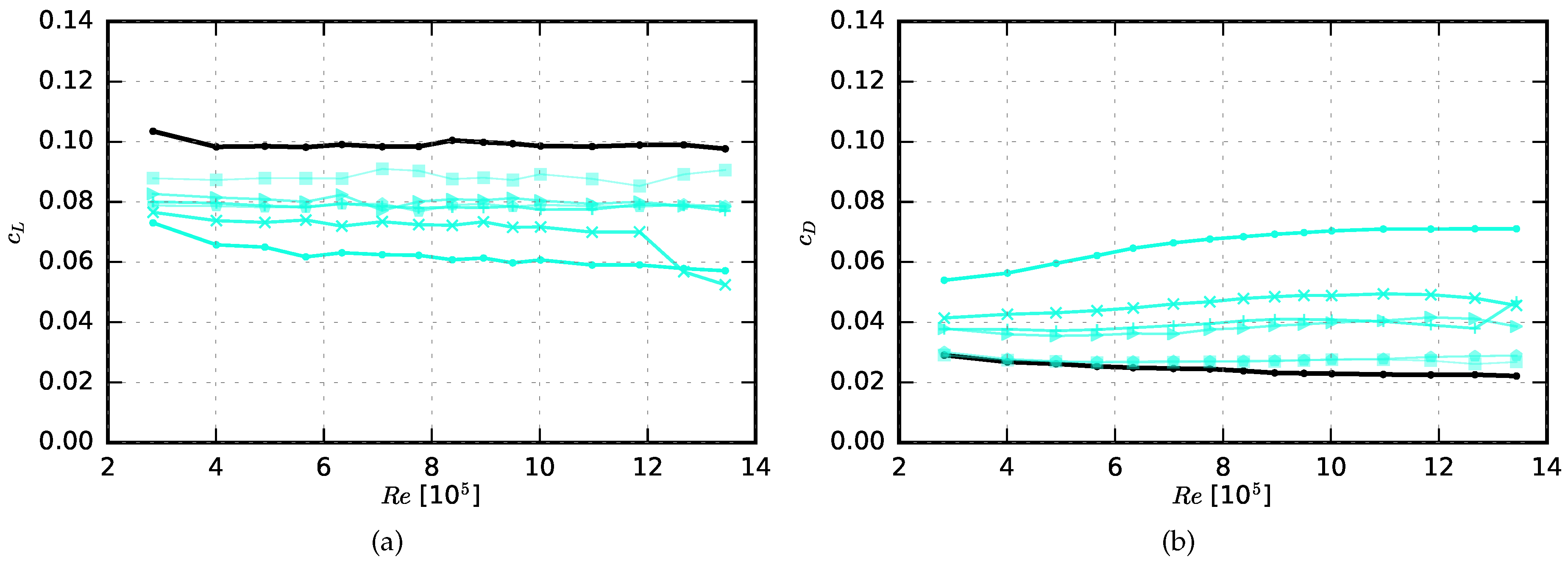
M-Pore Al 45, r = 1000 Pa·s/m2). (a) Lift coefficient as a function of chord based Reynolds number; (b) Drag coefficient as a function of chord based Reynolds number.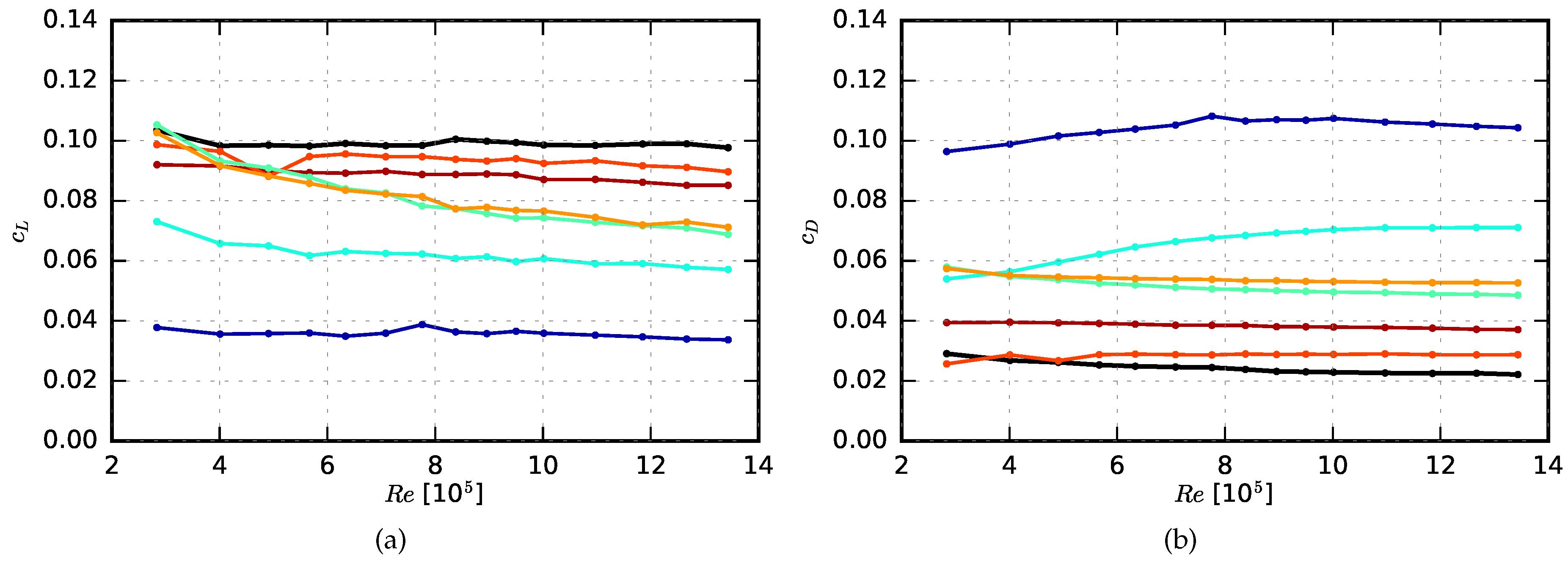


| Name | Material | Airflow Resistivity r (Pa·s/m2) | Porosity |
|---|---|---|---|
| Reference | non-porous | ∞ | 0 |
| Porex | polyethylene granulate | 316,500 | 0.40–0.46 |
| Siperm R200 | metal foam | 150,000 | 0.49–0.54 |
| Damtec estra | rubber granulate | 86,100 | 0.18–0.29 |
| Damtec SBM K 20 | rubber granulate | 12,900 | 0.29–0.32 |
| Recemat Ni-4753 | metal foam | 8200 | 0.95 |
| M-Pore Al 45 ppi | metal foam | 1000 | 0.90 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Geyer, T.F.; Sarradj, E. Self Noise Reduction and Aerodynamics of Airfoils with Porous Trailing Edges. Acoustics 2019, 1, 393-409. https://doi.org/10.3390/acoustics1020022
Geyer TF, Sarradj E. Self Noise Reduction and Aerodynamics of Airfoils with Porous Trailing Edges. Acoustics. 2019; 1(2):393-409. https://doi.org/10.3390/acoustics1020022
Chicago/Turabian StyleGeyer, Thomas Fritz, and Ennes Sarradj. 2019. "Self Noise Reduction and Aerodynamics of Airfoils with Porous Trailing Edges" Acoustics 1, no. 2: 393-409. https://doi.org/10.3390/acoustics1020022
APA StyleGeyer, T. F., & Sarradj, E. (2019). Self Noise Reduction and Aerodynamics of Airfoils with Porous Trailing Edges. Acoustics, 1(2), 393-409. https://doi.org/10.3390/acoustics1020022





