Bursting the Bubble: The Fluids Mechanics That Prove Godzilla Would Survive the Plan

Abstract

Cinematic and pop culture narratives offer powerful tools for engaging with science by contextualizing complex principles within familiar, imaginative stories. This paper investigates the scientific feasibility of a plan depicted in Godzilla Minus One to neutralize the iconic kaiju through buoyancy reduction, exposure to deep-sea pressure, and rapid decompression. Employing principles from fluid mechanics, thermodynamics, and biomechanics, the study critically examines the use of freon bubbles and additional weight to counteract buoyant forces, the effects of 1500-m oceanic pressure on Godzilla’s physiology, and the potential for barotrauma during rapid ascent. While theoretically plausible, the proposed strategies face insurmountable challenges, including logistical impracticality and Godzilla’s presumed biological adaptations. This interdisciplinary critique highlights the intersection of film and science, encouraging critical analysis of cinematic representations and fostering a deeper appreciation for the scientific principles they attempt to portray.

1. Introduction

Cinematic and pop culture [1] elements have become valuable tools in science education, bridging the gap between complex scientific concepts and student engagement. By integrating familiar narratives and characters from movies [2], television shows, comics, and even video games, educators can create relatable and memorable learning experiences. For example, Shakespeare’s plays [3] and Agatha Christie’s murder mysteries [4] have been used to illustrate science principles, such as the role of chemistry in crime-solving and forensic investigations. Similarly, the detective stories of Sherlock Holmes [5,6,7] showcase chemical analysis and deduction, bringing abstract scientific methods to life through gripping narratives. Incorporating science fiction and adventure films further enriches the educational landscape. The James Bond series [8], for instance, provides an opportunity to explore real-world applications of chemistry, such as sedatives, explosive compounds, and rocket fuels. The Harry Potter series inspires creative chemistry experiments [9], including the recreation of magical phenomena like invisible inks, color-changing flames, and other enchanting laboratory activities. Michael Crichton’s Jurassic Park delves into the defense mechanisms of plants and the role of biochemistry in animal communication [10], offering a springboard for discussions on evolutionary biology and synthetic chemistry. Movies grounded in historical or engineering themes, such as Apollo 13 [11], have been widely praised for their accurate depiction of scientific problem-solving under pressure. Similarly, films like Black Panther [12] explore the futuristic applications of materials science, introducing students to the possibilities of advanced technologies and their societal implications. These cinematic examples not only illustrate scientific principles but also inspire curiosity and creativity by linking theoretical knowledge to practical applications. Television series have also played a crucial role in making science accessible. Shows like The Big Bang Theory [13,14], CSI [15], and Bones [16] integrate chemistry, physics, and biology into their storylines, demonstrating their relevance to everyday life and modern challenges. Animated shows, comics, and graphic novels, such as Archie Comics [17], Marvel [18], and DC Comics [19], have introduced scientific concepts like microscale reactions and highlighted scientific inaccuracies in an engaging way. Even video games and interactive media are emerging as platforms to integrate science into pop culture. Escape room activities in classrooms, inspired by popular characters like Superman and his kryptonite, engage students in solving related puzzles [20,21,22]. Despite their potential, video games [1,23] are underexplored in scientific pedagogy, though they hold promise for illustrating complex chemical and engineering principles in an immersive environment.

Ultimately, these creative approaches highlight the benefits of using pop culture to contextualize science, making it more accessible, relevant, and enjoyable for students. However, educators must also navigate the limitations, such as ensuring scientific accuracy and balancing entertainment with educational objectives. Science fiction films have long been a powerful medium for exploring the intersection of creativity and science, pushing the boundaries of imagination while often venturing into the realm of the implausible. However, when a story’s scientific inaccuracies overshadow its narrative, the result can be an unintended distraction, diminishing the film’s impact. Critiquing movies from a scientific perspective allows to distinguish between creative liberties taken for storytelling and outright scientific missteps that undermine believability. This practice of analyzing science in movies is not merely about pointing out flaws but about fostering a deeper appreciation for how science can enhance storytelling. By understanding the principles of physics, biology, and engineering, audiences can engage with a film more critically, appreciating its merits while recognizing its limitations. It also highlights the importance of accuracy in bridging the gap between entertainment and education, especially in an era where media significantly shapes public understanding of science. This study contributes to the broader conversation on how pop culture and scientific pedagogy interact by examining how cinematic narratives influence public understanding of scientific concepts. By analyzing a well-known film through a scientific lens, we aim to highlight both the potential and the limitations of using fiction as an educational tool to develop critical thinking skills. In this paper, we take a closer look at a cinematic example where science takes center stage from the movie Godzilla minus one: a proposed plan to defeat Godzilla using freon tanks, deep-sea pressure, and rapid decompression. Through this analysis, we explore how such depictions align—or fail to align—with the laws of physics and fluid mechanics, offering insights into the broader relationship between science and film.

2. Materials

“Godzilla Minus One” is a 2023 Japanese epic kaiju film written, directed, and produced by Takashi Yamazaki, marking the 37th installment in the Godzilla franchise [24]. Set in a post-war Japan struggling to rebuild after World War II, the film explores the profound societal and psychological impact of devastation as Godzilla emerges as an unstoppable force of destruction. The title, “Minus One”, symbolizes a nation already at its lowest point, only to be thrust into an even deeper abyss with the arrival of the iconic monster. This study examines the feasibility of the plan to defeat Godzilla proposed in the movie, focusing on three critical phases, as depicted in Figure 1.

This figure illustrates the three-step plan proposed to defeat Godzilla using freon tanks, deep-sea pressure, and rapid decompression:

Step 1: Sinking with Freon Tanks attached to Godzilla’s body. The plan involves using bubble generated by the gas to increase Godzilla’s effective density, causing him to sink below the ocean surface. The downward arrow indicates the intended sinking motion as buoyancy is overcome by the added weight.

Step 2: Exposing to Deep-Sea Pressure at 1500 m. The second panel depicts Godzilla fully submerged at a depth of 1500 m. At this depth, the immense oceanic pressure is expected to exert extreme force on his body, potentially causing structural damage or collapse.

Step 3: Inducing Rapid Decompression During Ascent In the third panel, Godzilla is shown ascending rapidly toward the surface. This stage of the plan aims to cause barotrauma through rapid decompression, which would expand any gases within his body, potentially resulting in fatal damage.

The analysis requires several data points and assumptions, including physical characteristics of Godzilla, properties of freon tanks, and environmental conditions. Godzilla’s estimated mass is 20,000 metric tons, with a hypothesized density of 800 kg/m³ (like semi aquatic creature), making him less dense than water and capable of floating without additional weight. The density of liquid freon (R-22) is taken as 1174 kg/m³ and 5 kg/m³ for gas freon, with industrial freon tanks assumed to have a volume of 50 L (0.05 m³). Ocean water density is set at 1000 kg/m³, with gravitational acceleration g as 9.8 m/s². For rapid decompression analysis, gas expansion follows Boyle’s Law, considering the surface pressure of 0.1 MPa and deep-sea pressure of 14.7 MPa at 1500 m. Biomechanical comparisons use data on marine mammals and deep-sea creatures to evaluate the structural resilience of Godzilla to extreme pressures and decompression effects.

3. Results

The results of the analysis reveal significant challenges in implementing the proposed plan to defeat Godzilla using freon tanks, deep-sea pressure, and rapid decompression. Each phase of the plan was evaluated using established scientific principles, with the following findings.

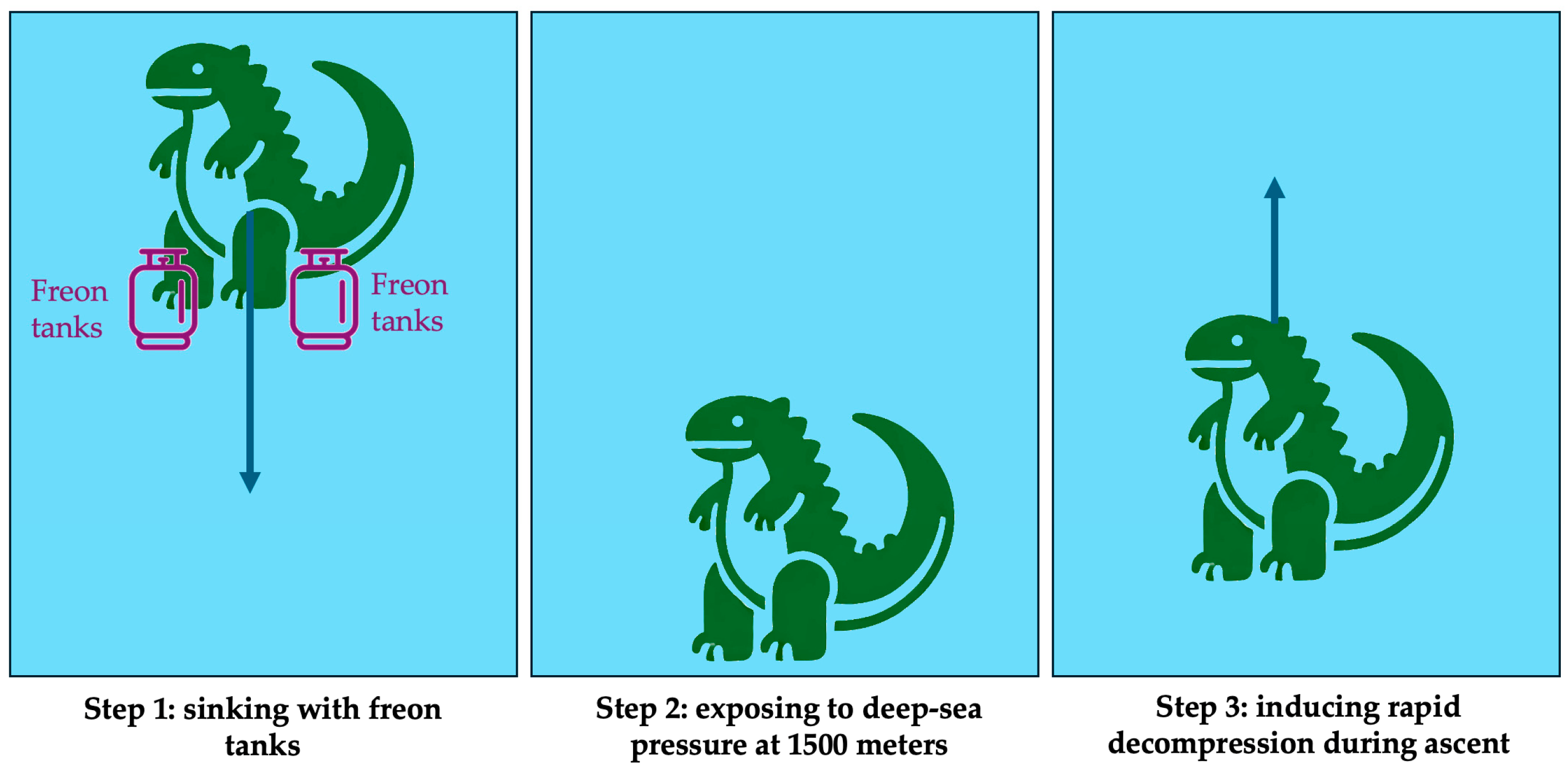
3.1. Freon Tanks and Sinking Feasibility

The rationale for using freon gas in the movie is unclear and not explained in the movie. The choice of freon over other gases may be due to its low density, ease of liquefaction, and historical use in industrial applications. Freon is a gas that can be stored as a liquid under pressure, making it convenient for controlled release in deep-sea environments. However, alternative gases such as helium or nitrogen might have been considered for similar buoyancy effects. It might be due to its ability to remain in gaseous form under specific conditions, but the exact reasoning is uncertain. The idea behind this strategy is to decrease the effective density of water by introducing bubbles of freon gas, which has a much lower density than water. The reduction in buoyant force is proportional to the reduction in the effective density of the fluid. Releasing freon bubbles beneath Godzilla offers a theoretical approach to reduce the buoyant force acting on him by altering the effective density of the surrounding water.

One fundamental challenge to this strategy is that releasing freon gas at the bottom of Godzilla is not practically feasible. At depths of 1500 m, the hydrostatic pressure is approximately 14.7 MPa, which would prevent the gas from forming stable bubbles. Instead, the gas would likely dissolve rapidly into the seawater due to the increased solubility of gases under high pressure. Furthermore, even if bubbles could be generated, they would burst as they ascend due to pressure differentials, leading to rapid mixing into the surrounding water rather than forming a continuous buoyancy-reducing layer beneath Godzilla. This issue significantly undermines the effectiveness of the approach, as the intended reduction in water density would be disrupted before it can have a meaningful impact on buoyancy.

Freon gas, with a density of approximately 5 kg/m³, is significantly less dense than water at 1000 kg/m³. By introducing bubbles into the water, the overall density of the fluid decreases proportionally to the volume fraction of the bubbles. For example, replacing 10% of the water’s volume with freon reduces the effective density to approximately 900.5 kg/m³. This decrease in density directly reduces the buoyant force acting on Godzilla’s body, as buoyancy is determined by the density of the displaced fluid.

$$F_b=\rho \times V\times g$$

(1)

Using Archimedes’ principle, the original buoyant F_b force acting on Godzilla, with a volume of 25,000 cubic meters, is approximately 2.45 × 10⁸ N. When 10% of the water is replaced with freon bubbles, the effective density of the fluid drops to 900.5 kg/m³, and the buoyant force decreases to 2.206 × 10⁸ N, resulting in a reduction of 2.44 × 10⁷ N, or about 10% of the original buoyancy. While this reduction may seem significant, it is unlikely to be sufficient to sink a massive creature like Godzilla without additional measures.

For greater reductions in buoyancy, the proportion of freon bubbles must increase substantially. For instance, if 50% of the water is replaced with freon bubbles, the effective density decreases further to 502.5 kg/m³, which reduces the buoyant force to 1.23 × 10⁸ N, nearly halving the original buoyant force. However, achieving such high proportions of freon bubbles in water would require an enormous volume of gas and an efficient deployment system to release the bubbles consistently and precisely beneath Godzilla. Given Godzilla’s estimated volume of 25,000 cubic meters, the volume of freon bubbles needed would be 12,500 cubic meters, replacing half of the water beneath him. With freon’s density of 5 kg/m³, this corresponds to a total mass of 62.5 tons of freon gas. To deploy this amount, approximately 3000 standard freon gas tanks, each with a capacity of 50 L. The effectiveness of this strategy depends also on the placement of the bubbles. Bubbles must be released directly beneath Godzilla to reduce the upward buoyant force, as this force arises from the pressure exerted by the water beneath the object. If the bubbles are placed to the sides or above Godzilla, they would reduce lateral or downward pressures, which do not contribute to buoyancy, rendering the approach ineffective. Achieving precise placement of freon bubbles beneath a massive and mobile creature like Godzilla presents a fundamental limitation to the plan, as the logistical and practical challenges of such a strategy are immense. The effectiveness of buoyancy reduction using freon bubbles is further limited by the short residence time of bubbles in water. Unlike solid objects, gas bubbles rise rapidly due to their low density and large buoyancy force. This means that the local reduction in effective water density only occurs for a brief moment before the bubbles ascend and dissipate. In high-pressure environments, turbulent mixing can further accelerate bubble dispersion, making it even more difficult to sustain a buoyancy-reducing effect around Godzilla.

After testing the buoyancy-reduction approach proposed in the movie, another strategy to sink Godzilla is proposed and involves directly adding sufficient weight to counteract the net upward buoyant force acting on him.

While the use of heavier freon tanks to increase the net density of Godzilla and force him downward might appear plausible, the key question is: to what depth would this strategy remain effective? The density and weight distribution of these tanks would need to counteract not only Godzilla’s natural buoyancy but also external forces such as ocean currents and turbulence. Furthermore, at greater depths, material constraints of standard freon tanks would become an issue, as high external pressures could cause structural deformation or rupture, rendering the tanks ineffective. A more realistic approach would involve the use of high-density materials designed to withstand extreme pressures, but deploying and securing such materials onto a moving kaiju presents additional logistical challenges.

With an estimated mass of 20,000 metric tons and a volume of 25,000 m³, Godzilla experiences a buoyant force of 2.45 × 10⁸ N, exceeding his weight of 1.96 × 10⁸ N This leaves an upward force of 4.9 × 10⁷ N to be neutralized by additional weight. To achieve this, an extra 5000 metric tons of mass is required, which could be delivered via freon tanks or other dense materials. Assuming each freon tank weighs 58.7 kg, 85,187 tanks would be needed, collectively occupying 4259 m³.

3.2. Pressure at Depth (1500 m)

At a depth of 1500 m, the pressure exerted on Godzilla arises from the weight of the overlying water column. This pressure, known as hydrostatic pressure, can be calculated using the relation:

$$P={\rho }_{water}\times g\times h$$

(2)

where P is the pressure, ${\rho }_{water}$ is the density of seawater (1000 kg/m³), g is the gravitational acceleration (9.8 m/s), and h is the depth (1500 m). Substituting these values gives P = 14.7 M Pa. This pressure corresponds to approximately 145 atmospheres, an extreme condition far beyond the survivability threshold of most terrestrial organisms.

For instance, human lungs and other air-filled cavities, such as the sinuses or inner ear, would collapse under pressures exceeding 0.2 MPa [25], causing catastrophic damage and rapid fatality. This inability to withstand high pressure arises from the compressibility of air, which is a significant limitation for terrestrial life forms. Despite the severity of this pressure, Godzilla’s cinematic biology suggests a high degree of adaptation akin to deep-sea organisms.

A crucial limitation to consider is whether the biological tissues of Godzilla could survive exposure to such high pressures. While deep-sea organisms demonstrate extreme adaptations to pressure, terrestrial and semi-aquatic creatures generally exhibit significant vulnerability. At 1500 m, the pressure would exceed the structural limits of most biological materials, potentially leading to tissue damage, protein denaturation, and cellular collapse. If Godzilla possesses air-filled cavities, such as lungs or sinuses, these would be highly susceptible to compression damage, unless adapted mechanisms exist to prevent collapse. Given that marine mammals such as sperm whales can dive to depths of approximately 1000 m, an upper survival threshold for Godzilla could reasonably be estimated in a similar range. However, without direct evidence of such adaptations, the assumption that Godzilla could endure prolonged exposure to 1500-m depths remains speculative

These creatures routinely survive pressures exceeding 40 MPa [26] in the hadal zones, where extreme conditions dominate. Their ability to thrive is attributed to the absence of compressible cavities such as lungs or swim bladders, which would otherwise collapse. Instead, their bodies are filled with incompressible fluids that equalize internal and external pressure, preventing the destructive mechanical stress that air-filled spaces would experience. If Godzilla possesses similar adaptations, his internal pressure would align with the external pressure at 1500 m, effectively neutralizing the impact of the water column’s weight on his physiology.

Additionally, the mechanical resilience of Godzilla’s tissues likely plays a pivotal role in his survival at such depths. Deep-sea organisms feature pressure-resistant tissues with specialized proteins, such as piezolytes, that stabilize cellular structures under high compressive forces. The assumptions about Godzilla’s physiology are largely derived from cinematic portrayals of its resilience rather than empirical data. While comparisons to deep-sea organisms provide a useful framework, alternative interpretations could consider adaptations similar to extremophiles or fictional biological structures that enable survival under extreme conditions. These tissues also lack the rigid skeletons that would otherwise deform under immense pressures. If Godzilla’s biological makeup includes such pressure-resistant proteins and highly elastic cellular matrices, his structural integrity would remain intact, even at depths generating 14.7 MPa. Furthermore, his larger size may contribute to increased structural robustness, dispersing pressure across a broader surface area compared to smaller organisms.

While the calculated pressure at 1500 m is formidable, these biomechanical adaptations render it unlikely to cause fatal damage to Godzilla. The assumption that exposure to deep-sea pressure would harm him does not account for his cinematic depiction, which aligns more closely with highly adapted deep-sea organisms. This analysis underscores the ineffectiveness of relying solely on environmental extremes to neutralize such a creature, highlighting the need for more nuanced and biologically informed strategies.

Despite the severity of this pressure, Godzilla’s cinematic biology suggests a high degree of adaptation, similar to deep-sea organisms. A useful comparison can be drawn with deep-sea species that have evolved to withstand extreme pressures. For example, the Mariana snailfish (Pseudoliparis swirei [27]) inhabits depths exceeding 8000 m and relies on high concentrations of osmolytes like trimethylamine N-oxide (TMAO) to stabilize proteins under high pressure. Similarly, amphipods in hadal trenches lack gas-filled cavities and rely on flexible, gelatinous tissues to avoid pressure-induced collapse. If Godzilla shares similar adaptations—such as reduced skeletal rigidity and pressure-resistant cellular structures—he could hypothetically withstand the 14.7 MPa pressure at 1500 m without significant physiological damage. Deep-sea creatures have evolved specific mechanisms to withstand pressures of this magnitude, including the absence of compressible cavities such as lungs or swim bladders. These organisms avoid structural collapse because their tissues are nearly incompressible, and their internal fluids provide pressure equalization. If Godzilla possesses analogous adaptations, his internal pressure would match the external environment, effectively mitigating the mechanical stresses imposed by the surrounding water.

3.3. Rapid Ascent and Decompression Effects

The final phase of the plan involves rapid ascent, which would expose Godzilla to a drastic pressure drop of 14.6 MPa between the depth and the surface. This rapid decompression would cause any compressible gases within Godzilla’s body to expand by a factor of 147, as calculated using Boyle’s Law. The factor of 147 is derived from Boyle’s Law, which states that the volume of a gas is inversely proportional to its pressure P₁V₁ = P₂V₂ where P₁ and P₂ are the pressures at two different depths, and V1 and V2 are the corresponding gas volumes. At 1500 m, the pressure is 14.7 MPa, while at the surface it is 0.1 MPa. This leads to a gas expansion factor of 17). At a depth of 1500 m, the pressure is approximately 14.7 MPa, while surface pressure is 0.1 MPa. The ratio of these pressures determines the expansion factor, leading to extreme internal stress and potential barotrauma under normal biological conditions. While this could cause severe barotrauma in organisms with gas-filled cavities, deep-sea organisms and marine mammals demonstrate physiological adaptations that mitigate these effects [28]. If Godzilla possesses similar adaptations, such as the absence of compressible air pockets, he would likely be resistant to rapid decompression.

4. Discussion

The analysis reveals that the plan to neutralize Godzilla, as depicted in Godzilla Minus One, is scientifically unfeasible due to overwhelming logistical challenges and the biological adaptations of the kaiju that likely render the strategy ineffective. Other strategies for countering Godzilla, such as controlled cavitation or the use of high-density materials, were likely omitted from the film’s narrative for storytelling purposes. These alternatives might have provided a more feasible approach but were beyond the scope of the cinematic premise. The proposed use of freon bubbles to reduce buoyancy, though theoretically plausible, would require the deployment of approximately 3000 freon tanks, each containing 50 L of pressurized gas, to replace 50% of the water beneath Godzilla’s body. Achieving this precise placement beneath a moving and massive creature presents insurmountable practical challenges. Similarly, the alternative strategy of adding sufficient weight to counteract the upward buoyant force would require 85,187 tanks of liquid freon, collectively weighing 5000 metric tons and occupying 4259 cubic meters of space. The logistics of attaching such a vast number of tanks to a resistant and dynamic target compound the impracticality of this approach. Additionally, Godzilla’s presumed physiological resilience to extreme conditions significantly undermines the effectiveness of this plan. At a depth of 1500 m, Godzilla would be exposed to a pressure of 14.7 MPa (approximately 145 atmospheres), far exceeding the tolerance of most terrestrial organisms, which typically collapse under pressures as low as 0.2 MPa [25]. However, cinematic depictions of Godzilla suggest adaptations similar to those of deep-sea organisms, which thrive under high pressures. These adaptations include the absence of compressible air pockets, such as lungs or swim bladders, and the presence of pressure-resistant tissues containing specialized proteins like piezolytes, which stabilize cellular structures under compressive forces [29]. Such mechanisms would likely allow Godzilla to withstand deep-sea pressures without structural damage. The final phase of the plan, involving rapid decompression during ascent, is equally ineffective. As Godzilla ascends from a depth of 1500 m, he would experience a pressure drop of 14.6 MPa. Using Boyle’s Law, this pressure difference would cause gas-filled cavities to expand by a factor of 147, potentially leading to severe barotrauma. However, deep-sea organisms and marine mammals, which lack significant gas-filled cavities and feature collapsible lungs [28], demonstrate biological adaptations that prevent such injuries. For instance, marine mammals redistribute air to non-compressible structures like the trachea during dives, a feature that likely parallels adaptations in Godzilla [30]. If Godzilla possesses similar adaptations, the rapid expansion of gases would be mitigated, further reducing the plan’s likelihood of success.

This analysis underscores the significant gap between cinematic creativity and scientific plausibility. While the concept of using freon tanks, deep-sea pressure, and decompression to neutralize Godzilla provides an engaging narrative, it falls short of scientific validity due to the logistical challenges and biological implausibility involved. By critically evaluating such inaccuracies, audiences are encouraged to engage more deeply with the science depicted in films, fostering an appreciation for the balance between storytelling and realism while promoting a better understanding of scientific principles. While Archimedes’ principle and Boyle’s Law provide a foundational understanding of buoyancy and gas expansion, their application to a fictional entity like Godzilla presents limitations. Without precise knowledge of Godzilla’s internal structure and gas composition, assumptions about buoyancy reduction and decompression effects remain speculative

5. Using Movies as an Educational Tool

Educators have the opportunity to significantly enhance student discussions by guiding learners towards authoritative scientific resources. One such resource is the seminal article by Mossa & Tolve (1998) [31] on flow visualization, which provides a rigorous experimental framework for understanding fluid dynamics through cinematic examples. Additionally, encouraging students to explore educational video platforms fosters autonomous learning and increases their engagement with the subject matter.

Cinematic narratives like Godzilla Minus One provide unique opportunities to inspire and engage students by integrating scientific analysis into education. This film, with its imaginative yet scientifically questionable strategies to neutralize Godzilla, is a rich medium for teaching fundamental principles in physics, biology, engineering, and environmental science. By dissecting the scientific elements of the movie, educators can encourage critical thinking, problem-solving, and creativity in their students [31,32,33,34]. One approach is to use the movie’s depiction of buoyancy manipulation, deep-sea pressure, and rapid decompression to teach fluid mechanics and thermodynamics. For example, analyzing the feasibility of sinking Godzilla with freon tanks introduces concepts such as Archimedes’ principle, hydrostatic pressure, and gas laws. Educators can guide students through buoyant force, water density, and gas expansion calculations using equations like Fb = ρVg and Boyle’s Law (P₁V₁ = P₂V₂). These exercises link abstract equations to a tangible and exciting scenario, making the subject matter more relatable and engaging. The discussion of Godzilla’s biological adaptations to extreme environments can also introduce students to the principles of biomechanics and evolutionary biology. By comparing Godzilla to deep-sea organisms and diving mammals, educators can highlight real-world adaptations such as pressure-resistant tissues, lack of air-filled cavities, and metabolic strategies to cope with extreme conditions. This interdisciplinary approach fosters curiosity about the natural world and helps students appreciate the ingenuity of biological systems. Moreover, the logistical challenges of the plan to neutralize Godzilla can be used to explore engineering concepts. Students can evaluate the feasibility of deploying thousands of freon tanks, consider alternative designs for underwater mechanisms, or propose innovative solutions to logistical constraints. This kind of activity encourages systems thinking and teamwork, key skills for budding engineers and problem-solvers. Finally, educators can use the movie to emphasize the importance of scientific accuracy in media. While films like Godzilla Minus One take creative liberties for entertainment, analyzing their scientific plausibility helps students develop media literacy, teaching them to differentiate between fact and fiction. Discussions can also address the broader implications of how science is portrayed in popular culture and its impact on public understanding. Educators can transform an action-packed kaiju film into a powerful teaching tool by incorporating Godzilla Minus One into the classroom. The combination of storytelling, science, and critical thinking not only enriches the learning experience but also inspires students to explore the connections between entertainment and real-world science.

6. Conclusions

The analysis of the plan proposed in Godzilla Minus One demonstrates significant scientific and logistical barriers to its feasibility, underscoring the gap between cinematic creativity and scientific reality. The proposed use of freon bubbles to reduce buoyancy, while theoretically plausible, requires an impractical deployment of approximately 3000 freon tanks with precise positioning beneath a mobile and massive target. Similarly, the alternative strategy of adding 85,187 tanks of liquid freon, weighing 5000 metric tons, faces insurmountable logistical challenges in both distribution and secure attachment to Godzilla. Further, Godzilla’s presumed adaptations to extreme environments, inspired by deep-sea organisms, render the physiological impact of deep-sea pressure and rapid decompression ineffective. At 14.7 MPa, the pressure at 1500 m exceeds terrestrial tolerances but aligns with the conditions deep-sea organisms endure. Biological features such as pressure-resistant tissues and the absence of compressible cavities likely allow Godzilla to withstand these conditions without structural damage. Similarly, the rapid decompression phase, which relies on gas expansion to induce barotrauma, is unlikely to succeed given Godzilla’s presumed adaptations, such as gas redistribution mechanisms and resilient tissue structures. By critiquing the scientific inaccuracies in Godzilla Minus One, this study illustrates the importance of bridging entertainment and scientific plausibility. While the narrative captivates audiences, its implausible science offers an opportunity for educational engagement, encouraging critical thinking and the application of scientific principles. Ultimately, this analysis highlights the potential of pop culture to inspire deeper exploration of science, fostering a better understanding of its complexities while appreciating the balance between fiction and reality.