A Building-Block Approach to State-Space Modeling of DC-DC Converter Systems
Abstract
1. Introduction
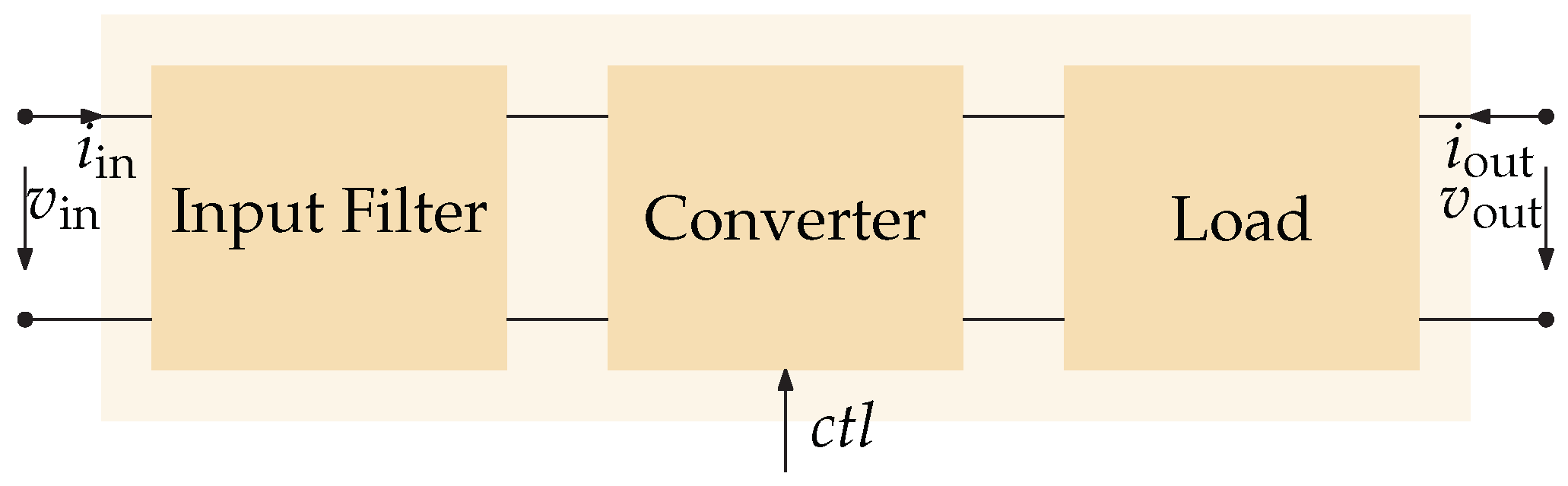
2. Modular Modeling Approach
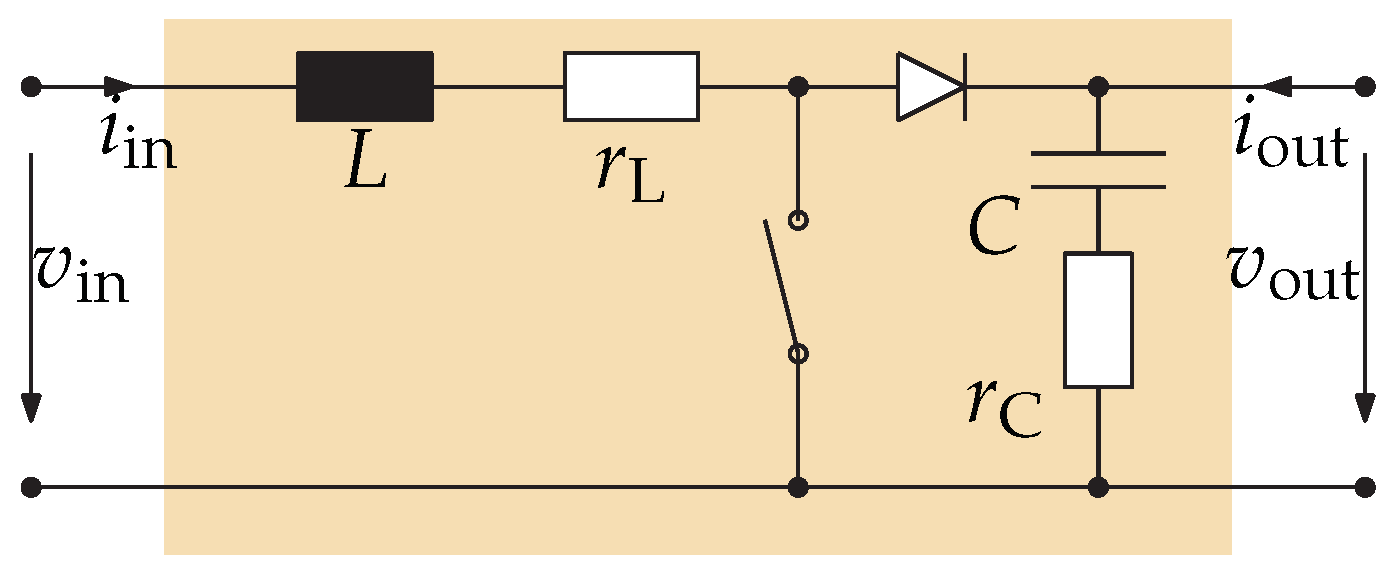
2.1. Model for Passive Components
2.2. Model for Controlled Converters and Converter Systems
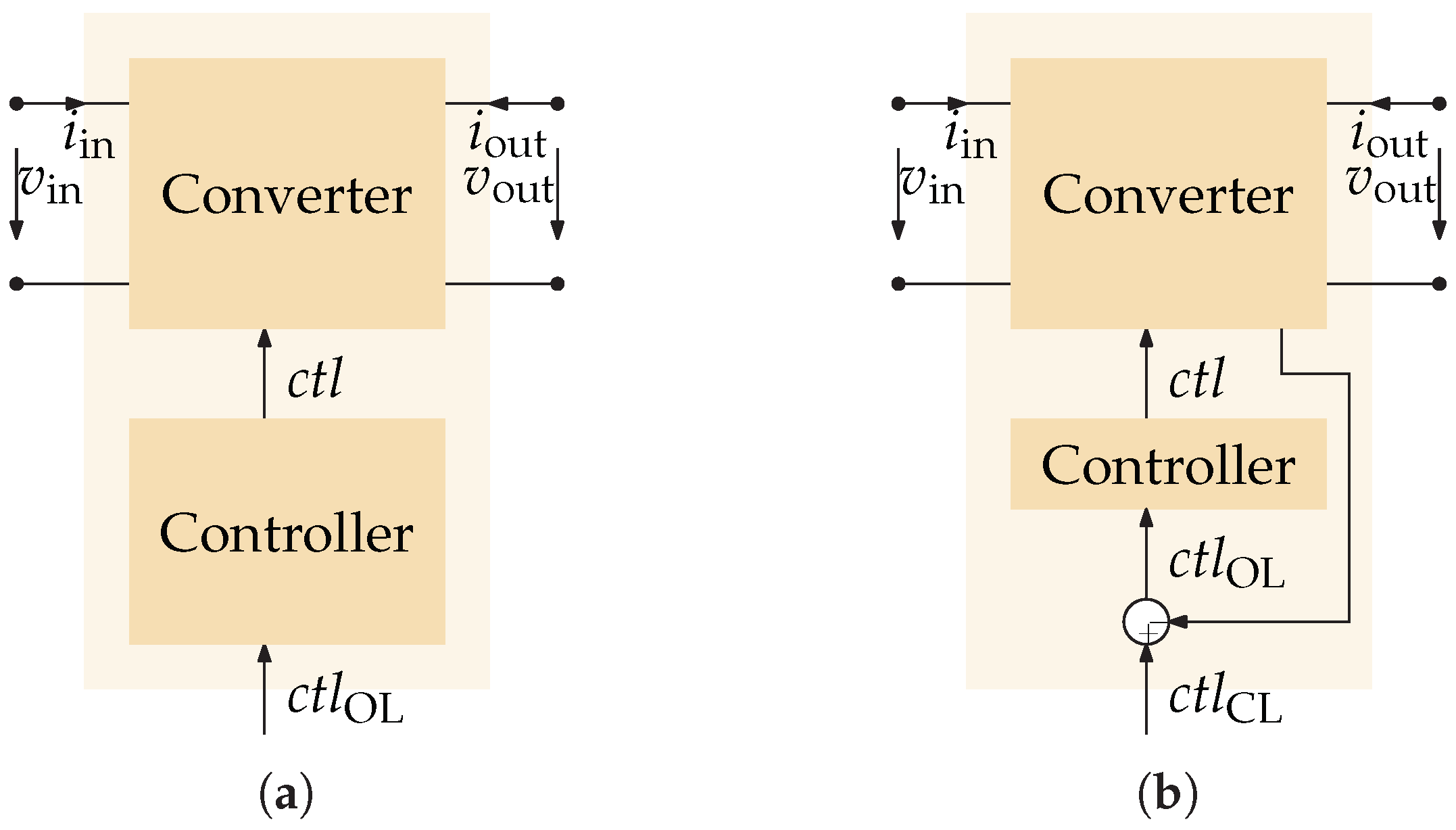
2.3. Model for Controllers
2.4. Making Connections
3. Adding Control Loops to Converter Models
3.1. Average Current Mode
3.2. Voltage Mode or Outer Voltage Loops
4. Connecting Converter Models
5. Summary of the Building-Block Modeling Approach
5.1. Building Block Types
5.2. Connecting Building Blocks
- Connecting converters with passive components or other converters. Since both building blocks for passive elements as given by (1) and building blocks for converters as given by (2) are two-port models, the same connection operation can be used for all these cases. The resulting model (10) is obtained by applying (13).
- Creating control loops. Connecting a controller, that is, a building block type as given by (3), to a converter is done by creating an open-loop model (4) using (5). For closing the control loop, the model’s matrix must be adapted using (6), with a feedback gain matrix chosen depending on the controlled variable, for example, one of (7) or (8) for current or voltage mode control.
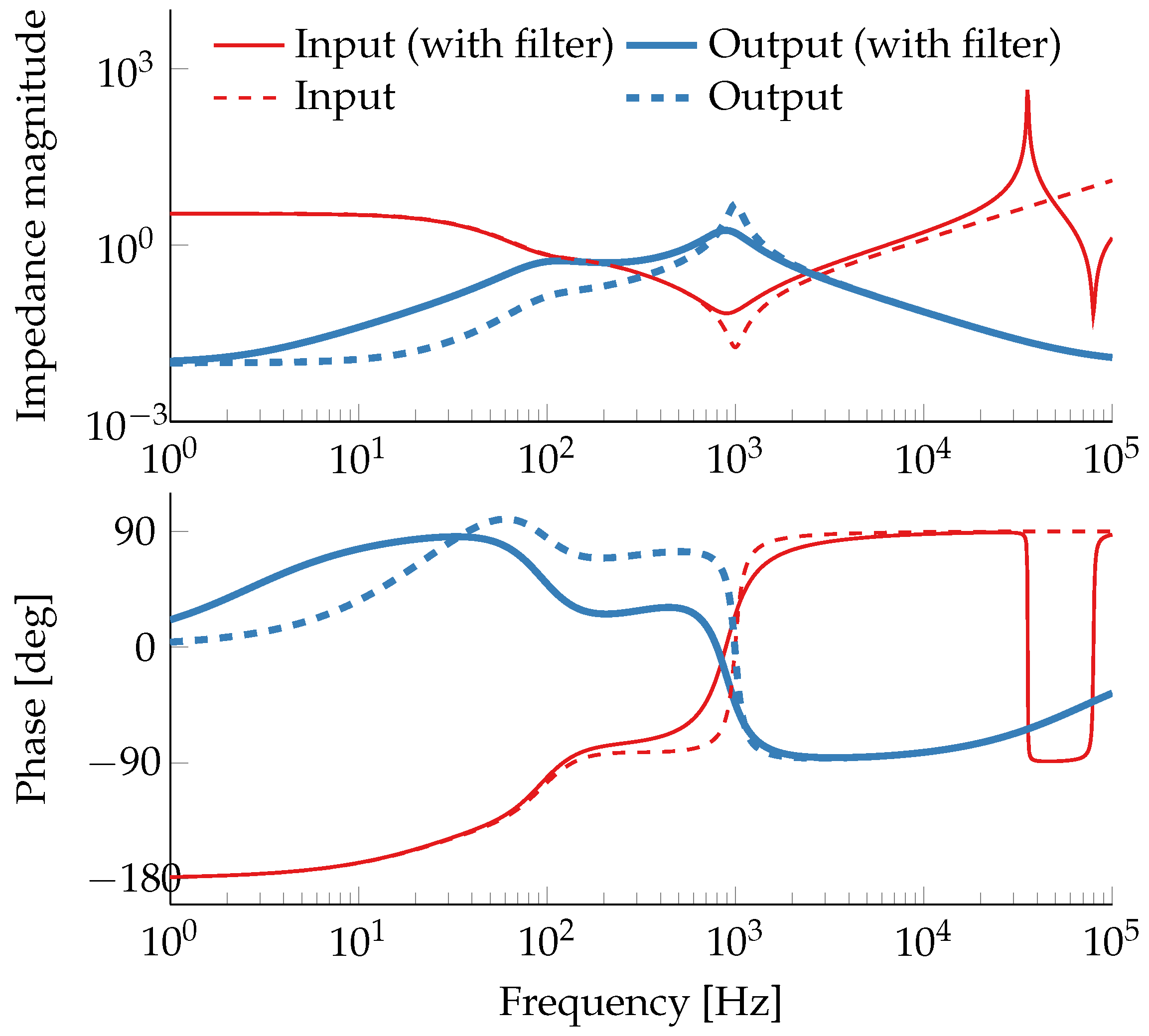
5.3. Frequency-Domain Analysis
6. Examples
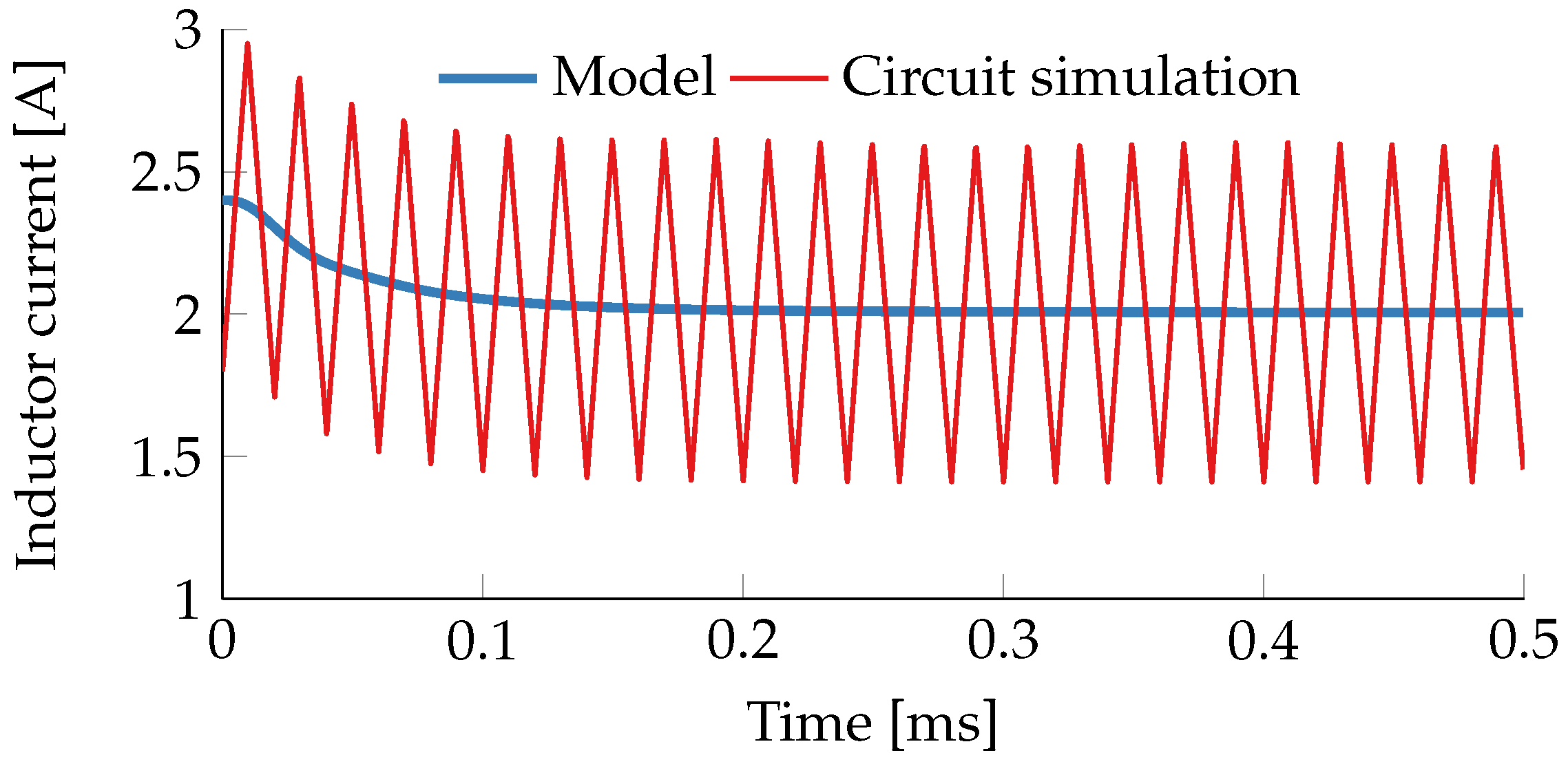
6.1. Buck Converter with Multiloop (I2) Control
- As the starting point to build up the complete model, the two-port state-space model for the PCM buck converter from Reference [34] is being used. The example is parameterized as given in the following. Operating point: , , . Power stage: , , . Peak current mode controller: external ramp of per switching cycle.
- For the I2 current loop, an additional controller for the average inductor current is being added. A Type 1 controller with ,000 is employed, cf. Appendix C, which is connected to the state-space model as described in Section 3.1.
- For the outer voltage control loop, a Type 2 controller with zero at , pole at and is being used, cf. Appendix C. It is connected to the model as described in Section 3.2.
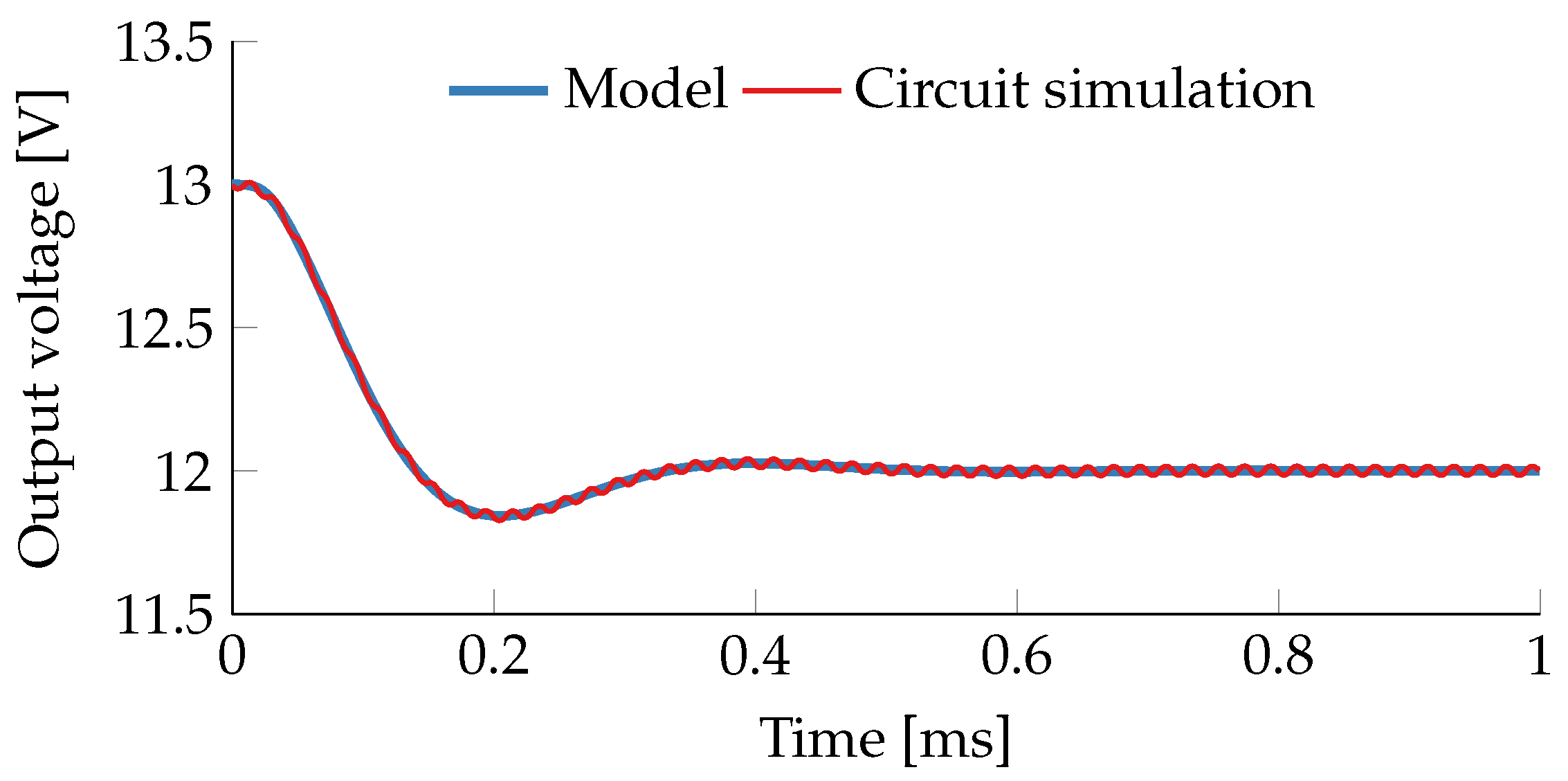
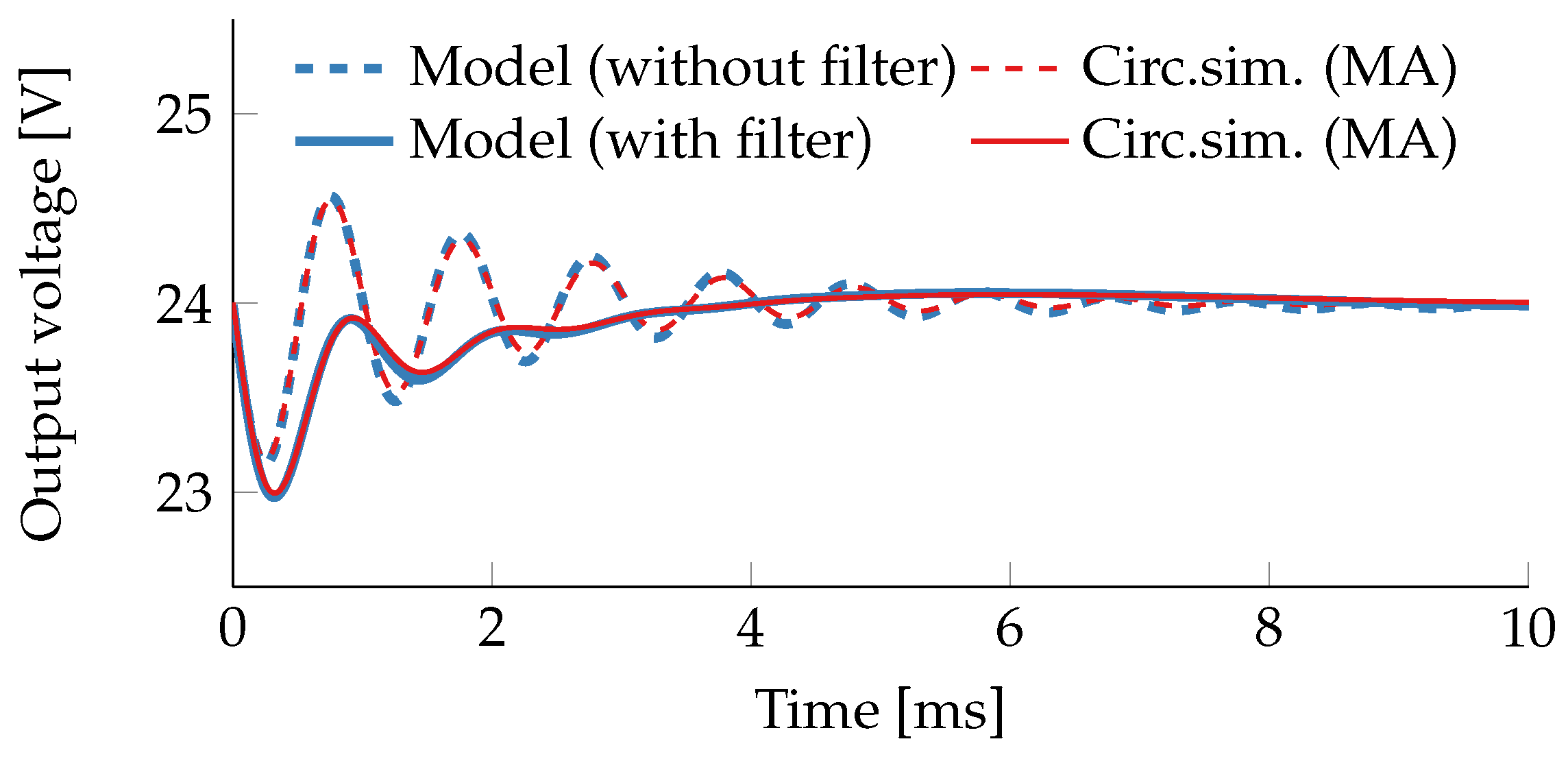
6.2. Boost Converter with Input Filter
- A linearized state-space model for a boost converter in CCM as given in Appendix B.2. The example is parameterized as given in the following. Operating point: , , . Power stage: , , .
- A load resistance (model from Appendix A.1) corresponding to the operating point is connected at the converter output using Section 4.
- A Type 3 controller (cf. Appendix C) is being employed for the voltage control loop, with zeros placed at , poles at and , and . It is connected to the model as described in Section 3.2.
- Finally, an LC filter model (cf. Appendix A.2) accounting for both an input filter and wiring is connected at the input of the converter model using Section 4, with , , and .
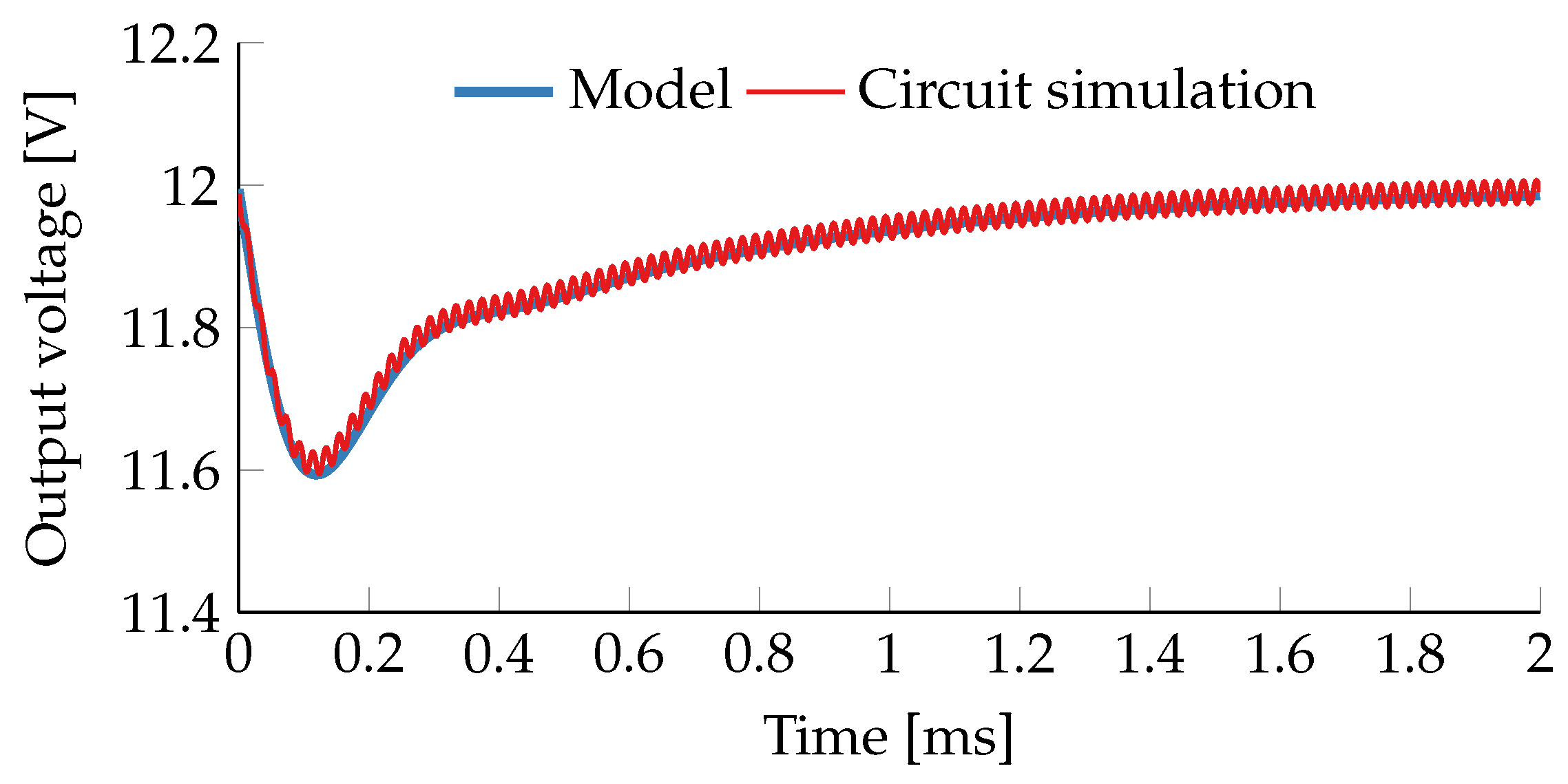
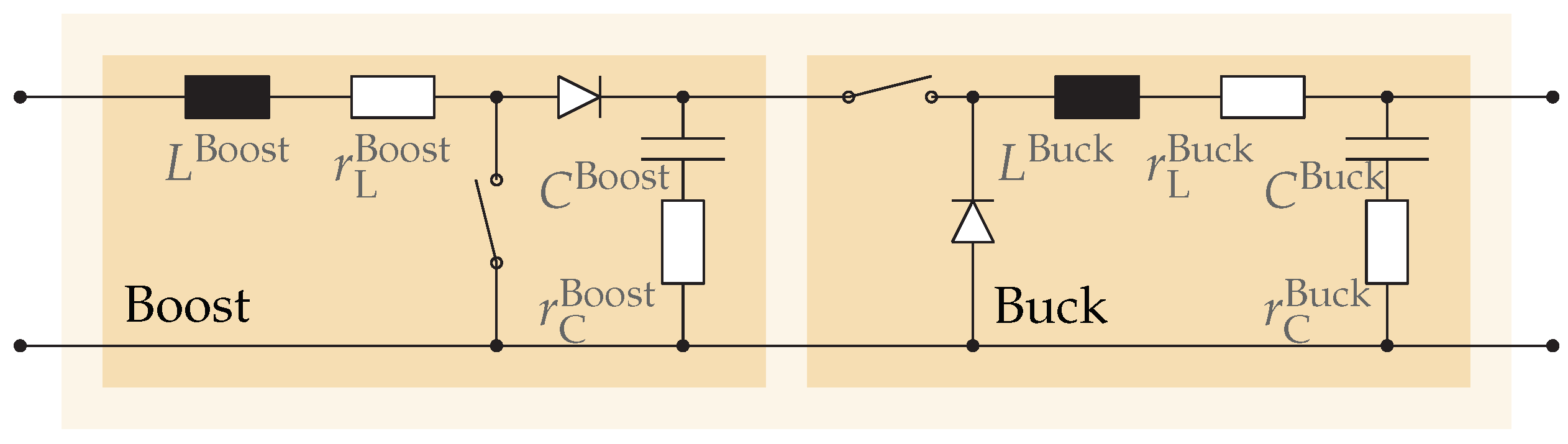
6.3. Series-Connected Boost and Buck Converter Stages
- Reuse the final model from Section 6.2 (voltage-controlled boost converter including input filter) but without the load resistance, since the buck converter will constitute the new load to the boost stage.
- Reuse the final model from Section 6.1 (voltage-controlled buck converter with underlying I2 current control).
- Connect the buck converter model at the output of the boost converter, as described in Section 4.
7. Conclusions
Funding
Conflicts of Interest
Appendix A. Building Blocks: Passive Component Models
Appendix A.1. Resistive Load
Appendix A.2. LC Filter

Appendix B. Building Blocks: Converter Models
Appendix B.1. Available Converter Models
Appendix B.2. Example: Boost Converter
Appendix C. Building Blocks: Controller Models
| Type 1 | Type 2 | Type 3 |
|---|---|---|
References
- Middlebrook, R.D.; Ćuk, S. A General Unified Approach to Modelling Switching-Converter Power Stages. In Proceedings of the 1976 IEEE Power Electronics Specialists Conference, Cleveland, OH, USA, 8–10 June 1976; pp. 18–34. [Google Scholar] [CrossRef]
- Vorpérian, V. Simplified Analysis of PWM Converters Using Model of PWM Switch. Part I: Continuous Conduction Mode. IEEE Trans. Aerosp. Electron. Syst. 1990, 26, 490–496. [Google Scholar] [CrossRef]
- Czarkowski, D.; Kazimierczuk, M.K. Energy-Conservation Approach to Modeling PWM DC-DC Converters. IEEE Trans. Aerosp. Electron. Syst. 1993, 29, 1059–1063. [Google Scholar] [CrossRef]
- Francés, A.; Asensi, R.; García, O.; Prieto, R.; Uceda, J. Modeling Electronic Power Converters in Smart DC Microgrids—An Overview. IEEE Trans. Smart Grid 2018, 9, 6274–6287. [Google Scholar] [CrossRef]
- Zhan, D.; Wei, L.; Zhang, Y.; Yao, Y. A Generalized Model of Nonisolated Multiphase DC-DC Converter Based on Novel Switching Period Averaging Method. IEEE Trans. Power Electron. 2015, 30, 5181–5191. [Google Scholar] [CrossRef]
- Davoudi, A.; Jatskevich, J.; De Rybel, T. Numerical State-Space Average-Value Modeling of PWM DC-DC Converters Operating in DCM and CCM. IEEE Trans. Power Electron. 2006, 21, 1003–1012. [Google Scholar] [CrossRef]
- Sun, J.; Mitchell, D.M.; Greuel, M.F.; Krein, P.T. Averaged Modeling of PWM Converters Operating in Discontinuous Conduction Mode. IEEE Trans. Power Electron. 2001, 16, 482–492. [Google Scholar] [CrossRef]
- Priewasser, R.; Agostinelli, M.; Unterrieder, C.; Marsili, S.; Huemer, M. Modeling, Control, and Implementation of DC-DC Converters for Variable Frequency Operation. IEEE Trans. Power Electron. 2014, 29, 287–301. [Google Scholar] [CrossRef]
- Meo, S.; Toscano, L. Some New Results on the Averaging Theory Approach for the Analysis of Power Electronic Converters. IEEE Trans. Ind. Electron. 2018, 65, 9367–9377. [Google Scholar] [CrossRef]
- Petrović, T.B.; Rakić, A.Ž. Linear Robust Approach to DC/DC Converter Modeling—I: Deterministic Switching. Electr. Eng. 2004, 86, 267–273. [Google Scholar] [CrossRef]
- Vesti, S.K.; Suntio, T.; Oliver, J.Á.; Prieto, R.; Cobos, J.A. Effect of Control Method on Impedance-Based Interactions in a Buck Converter. IEEE Trans. Power Electron. 2013, 28, 5311–5322. [Google Scholar] [CrossRef]
- Basso, C. Switch-Mode Power Supplies: SPICE Simulations and Practical Designs, 2nd ed.; McGraw-Hill: New York, NY, USA, 2014. [Google Scholar]
- Wu, T.F.; Chen, Y.K. Modeling PWM DC/DC Converters out of Basic Converter Units. IEEE Trans. Power Electron. 1998, 13, 870–881. [Google Scholar] [CrossRef]
- Francés, A.; Asensi, R.; García, O.; Prieto, R.; Uceda, J. A Black-box Modeling Approach for DC Nanogrids. In Proceedings of the 2016 IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 20–24 March 2016; pp. 1624–1631. [Google Scholar] [CrossRef]
- Francés, A.; Asensi, R.; García, O.; Prieto, R.; Uceda, J. How to Model a DC Microgrid: Towards an Automated Solution. In Proceedings of the 2017 IEEE Second International Conference on DC Microgrids (ICDCM), Nuremburg, Germany, 27–29 June 2017; pp. 609–616. [Google Scholar] [CrossRef]
- Haghmaram, R.; Sedaghati, F.; Ghafarpour, R. Power Exchange Among Microgrids using Modular-Isolated Bidirectional DC-DC Converter. Electr. Eng. 2017, 99, 441–454. [Google Scholar] [CrossRef]
- Haroun, R.; Cid-Pastor, A.; Aroudi, A.E.; Martíinez-Salamero, L. Synthesis of Canonical Elements for Power Processing in DC Distribution Systems Using Cascaded Converters and Sliding-Mode Control. IEEE Trans. Power Electron. 2014, 29, 1366–1381. [Google Scholar] [CrossRef]
- Li, H.; Shang, J.; You, X.; Zheng, T.; Zhang, B.; Lü, J. A Novel Stability Analysis Method Based on Floquet Theory for Cascaded DC-DC Converters System. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 2679–2683. [Google Scholar] [CrossRef]
- Mummadi, V.; Singamaneni, B.S. Stability Analysis of Cascaded DC-DC Power Electronic System. IEEJ Trans. Electr. Electron. Eng. 2009, 4, 763–770. [Google Scholar] [CrossRef]
- Ahmadi, R.; Ferdowsi, M. Modeling Closed-Loop Input and Output Impedances of DC-DC Power Converters Operating Inside DC Distribution Systems. In Proceedings of the 2014 IEEE Applied Power Electronics Conference and Exposition (APEC), Fort Worth, TX, USA, 16–20 March 2014; pp. 1131–1138. [Google Scholar] [CrossRef]
- Ali, H.; Zheng, X.; Zaman, H.; Liu, H.; Wu, X. Behavioral Modeling for Parallel- and Cascade-Connected DC-DC Converters. J. Circuits Syst. Comput. 2018. [Google Scholar] [CrossRef]
- Ahmadi, R.; Ferdowsi, M. Controller Design Method for a Cascaded Converter System Comprised of Two DC-DC Converters Considering the Effects of Mutual Interactions. In Proceedings of the 2012 Twenty-Seventh Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 5–9 February 2012; pp. 1838–1844. [Google Scholar] [CrossRef]
- Pidaparthy, S.K.; Choi, B. Control Design and Loop Gain Analysis of DC-to-DC Converters Intended for General Load Subsystems. Math. Probl. Eng. 2015. [Google Scholar] [CrossRef]
- Ahmadi, R.; Paschedag, D.; Ferdowsi, M. Analyzing Stability Issues in a Cascaded Converter System Comprised of Two Voltage-Mode Controlled DC-DC Converters. In Proceedings of the 2011 Twenty-Sixth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Fort Worth, TX, USA, 6–11 March 2011; pp. 1769–1775. [Google Scholar] [CrossRef]
- Cupelli, M.; Zhu, L.; Monti, A. Why Ideal Constant Power Loads Are Not the Worst Case Condition From a Control Standpoint. IEEE Trans. Smart Grid 2015, 6, 2596–2606. [Google Scholar] [CrossRef]
- Tehrani, H.M.; Francés, A.; Asensi, R.; Uceda, J. A Comparison of Stability Analysis of Constant Power Load with Detailed Model in DC Microgrids. In Proceedings of the 2018 7th International Conference on Renewable Energy Research and Applications (ICRERA), Paris, France, 14–17 October 2018; pp. 487–493. [Google Scholar] [CrossRef]
- Maranesi, P.G.; Tavazzi, V.; Varoli, V. Two-port Characterization of PWM Voltage Regulators at Low Frequencies. IEEE Trans. Ind. Electron. 1988, 35, 444–450. [Google Scholar] [CrossRef]
- Suntio, T.; Viinamäki, J.; Jokipii, J.; Messo, T.; Kuperman, A. Dynamic Characterization of Power Electronic Interfaces. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 949–961. [Google Scholar] [CrossRef]
- Zenger, K.; Altowati, A.; Suntio, T. Dynamic Properties of Interconnected Power Systems—A System Theoretic Approach. In Proceedings of the 2006 1st IEEE Conference on Industrial Electronics and Applications, Singapore, 24–26 May 2006; pp. 1–6. [Google Scholar] [CrossRef]
- Arnedo, L.; Burgos, R.; Boroyevich, D.; Wang, F. System-Level Black-Box DC-to-DC Converter Models. In Proceedings of the 2009 Twenty-Fourth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Washington, DC, USA, 15–19 February 2009; pp. 1476–1481. [Google Scholar] [CrossRef]
- Cvetkovic, I.; Boroyevich, D.; Mattavelli, P.; Lee, F.C.; Dong, D. Unterminated Small-Signal Behavioral Model of DC-DC Converters. IEEE Trans. Power Electron. 2013, 28, 1870–1879. [Google Scholar] [CrossRef]
- Suntio, T. On Dynamic Modeling of PCM-Controlled Converters—Buck Converter as an Example. IEEE Trans. Power Electron. 2018, 33, 5502–5518. [Google Scholar] [CrossRef]
- Yan, Y.; Lee, F.C.; Mattavelli, P.; Liu, P.H. I2 Average Current Mode Control for Switching Converters. IEEE Trans. Power Electron. 2014, 29, 2027–2036. [Google Scholar] [CrossRef]
- Smithson, S.C.; Williamson, S.S. A Unified State-Space Model of Constant-Frequency Current-Mode Controlled Power Converters in Continuous Conduction Mode. IEEE Trans. Ind. Electron. 2015, 62, 4514–4524. [Google Scholar] [CrossRef]
- He, S.; Hung, J.Y.; Nelms, R.M. Small-Signal Modeling of I2 Average Current Mode Control. IEEE Trans. Power Electron. 2016, 31, 3849–3858. [Google Scholar] [CrossRef]
- Li, R.; Seto, K.; Kiefer, J.; Li, S. Analysis, Simulation and Experimental Evaluation of Constant-Frequency Trailing-Edge-Modulated I2 Average Current-Mode Control. In Proceedings of the 2016 IEEE 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 2077–2084. [Google Scholar] [CrossRef]
- Suntio, T. Dynamic Modeling and Analysis of PCM-Controlled DCM-Operating Buck Converters—A Reexamination. Energies 2018, 11, 1267. [Google Scholar] [CrossRef]
- Suntio, T. Modeling and Analysis of a PCM-Controlled Boost Converter Designed to Operate in DCM. Energies 2019, 12, 4. [Google Scholar] [CrossRef]
- Suntio, T. Dynamic Profile of Switched-Mode Converter: Modeling, Analysis and Control; Wiley-VCH: Weinheim, Germany, 2009. [Google Scholar]
- Suntio, T.; Messo, T.; Puukko, J. Power Electronic Converters: Dynamics and Control in Conventional and Renewable Energy Applications; Wiley-VCH: Weinheim, Germany, 2017. [Google Scholar]
- Suntio, T. Unified Average and Small-Signal Modeling of Direct-On-Time Control. IEEE Trans. Ind. Electron. 2006, 53, 287–295. [Google Scholar] [CrossRef]
- Venable, H.D. Optimum Feedback Amplifier Design for Control Systems; Venable Technical Paper #3; Venable Industries: Austin, TX, USA, 1986. [Google Scholar]
- Basso, C. Designing Control Loops for Linear and Switching Power Supplies: A Tutorial Guide; Artech House: Boston, MA, USA, 2012. [Google Scholar]

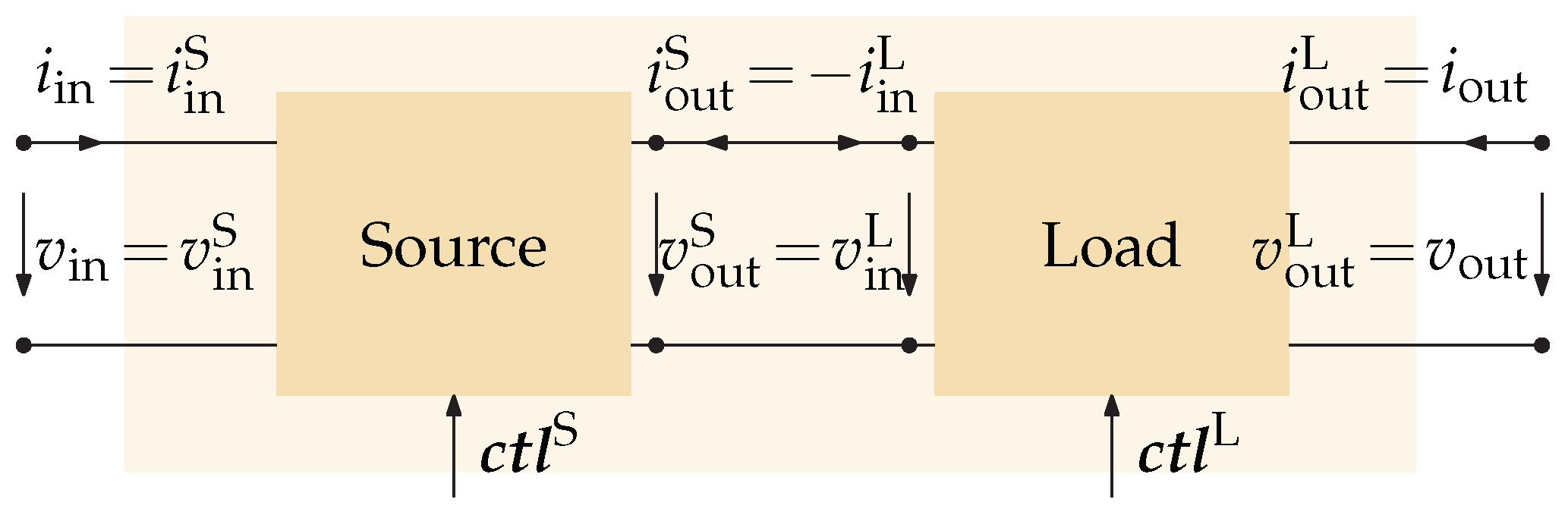

| Type | Equation | Definition |
|---|---|---|
| Passive components | (1) | |
| Controlled converters | (2) | |
| Controllers | (3) |
| Name | Definition | ||
|---|---|---|---|
| Control-to-output † | |||
| Output impedance | |||
| Input admittance | |||
| Forward voltage gain | |||
| Reverse current gain | |||
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Herbst, G. A Building-Block Approach to State-Space Modeling of DC-DC Converter Systems. J 2019, 2, 247-267. https://doi.org/10.3390/j2030018
Herbst G. A Building-Block Approach to State-Space Modeling of DC-DC Converter Systems. J. 2019; 2(3):247-267. https://doi.org/10.3390/j2030018
Chicago/Turabian StyleHerbst, Gernot. 2019. "A Building-Block Approach to State-Space Modeling of DC-DC Converter Systems" J 2, no. 3: 247-267. https://doi.org/10.3390/j2030018
APA StyleHerbst, G. (2019). A Building-Block Approach to State-Space Modeling of DC-DC Converter Systems. J, 2(3), 247-267. https://doi.org/10.3390/j2030018






