1. Introduction
Climate change has become a critical issue during the last decades and is attributed to the increased levels of greenhouse gases (GHG) in the atmosphere. Today, carbon dioxide (CO
2) is present in the atmosphere at a concentration over 415 ppmv, i.e., about 0.04 volume percent, equivalent to an atmospheric reservoir of about 3200 GtCO
2 [
1]. To address the increase in global CO
2 emissions to the atmosphere, GHG emission reduction targets and a wide range of greenhouse gas mitigation technologies are being considered, such as CO
2 capture from flue gases at power plants and other industrial sites followed by CO
2 transport and long-term geological storage [
2,
3]. The direct capture of CO
2 in the ambient air (DAC: Direct Air Capture) through a contactor is an alternative pathway among the negative emissions technologies to capture CO
2 directly from the atmosphere and should be deployed to achieve emission trajectories in line with the carbon neutrality objective and climate change mitigation [
4,
5]. Indeed, CO
2 removal is expected to play a key role in the transition to a net-zero system, in particular, to offset the emissions of industrial sectors that are difficult to decarbonize. In this regard, CO
2 removal technologies and changes in land-use sinks account for 450–1000 GtCO
2 of negative emissions to keep the global warming below 1.5 °C by 2100 [
6]. The CO
2 can be permanently stored in deep geological formations, resulting in negative emissions, or used in the production of building materials, chemical intermediates, or synthetic fuels to replace conventional fossil fuels. The DAC technique seems attractive given its potential to decarbonize the atmosphere: it can address distributed emissions such as aviation and transport and can be installed close to suitable storage sites and to low- or zero-carbon energy sources which are needed to run the plant, with little degradation performance in the case of low-pollutant air. However, it still presents multiple technical and economic uncertainties and barriers [
7]. Furthermore, the relatively high dilution of CO
2 in the atmosphere leads to higher energy needs (approximately three times more energy) and costs for DAC relative to other conventional CO
2 capture technologies and applications. Thus, DAC is energetically and economically challenging to be deployed at large scale.
DAC technologies have attracted new interest for several years [
8,
9] but the first developments of CO
2 capture in ambient air date back to the 1930s, with first applications in gas separation upstream of cryogenic air separation (N
2/O
2/Ar) in order to avoid CO
2 solidification, then for the control of air composition in confined systems (submarine, spacecraft) to keep the air breathable with no possibility of renewal [
10]. There are currently a number of small pilot and demonstration DAC plants operating worldwide [
11], mainly in Europe, the United States, and Canada, capturing around 10,000 tCO
2/year, with large-scale facilities (1 MtCO
2/year) in advanced development in the United Kingdom and in the United States, e.g., for use in enhanced oil recovery. After production is scaled up, the costs are expected to become competitive and fall to USD 200 per ton of CO
2 [
12]. However, the DAC sector is still in an early stage of commercial development, and research and development are still needed to overcome some challenges, e.g., to redesign and optimize the materials and processes to achieve low-cost and low-carbon performances [
13]. Several approaches to DAC are technically feasible but the development efforts are mainly focused on two reversible techniques for CO
2 capture in air: chemical absorption [
14], which relies on the property of a liquid basic solution to solubilize CO
2, such as sodium and potassium hydroxide, and adsorption, where CO
2 is fixed on the surface of a porous solid sorbent [
15,
16]. Both techniques require roughly 80% thermal energy (e.g., sorbent regeneration) and 20% electricity (e.g., contactor fans, vacuum pumps) for operation [
11], and have to be fueled by low-carbon energy sources [
17], in particular close to industrial sites where low-carbon heat can be recovered, to reduce lifecycle emissions [
18,
19].
The DAC technique based on low-temperature adsorption is developed by several companies among which are Climeworks in Switzerland and Global Thermostat in the United States. Such technologies have limited land and water footprints [
7]. However, the co-adsorption of H
2O in wet air treatment increases the thermal energy consumption for regeneration and the sorbent regeneration requires large amounts of low temperature heat [
15]. Compared to absorption that works continuously with solvent looping, the adsorption process works generally in batches with several beds filled in parallel. Processes have been developed to allow efficient contact of air with adsorbent and efficient regeneration of the material. The adsorption phenomena are schematically divided into two large families according to the nature of the bonds between the adsorbate and the solid: physisorption (weak interaction), e.g., on activated carbons, activated aluminas, silica gels or zeolites, and chemisorption (strong interaction), e.g., on chemical adsorbents based on amines immobilized on a solid support or alkali carbonates [
20,
21]. An optimal adsorbent would combine the following qualities: high adsorption capacity, high selectivity, easily regenerable, fast kinetics, high resistance (mechanical, chemical, and thermal) and lifetime, high availability, low pressure drop, low toxicity, and low cost. In practice, a major limitation of physical adsorbents is their low adsorption capacity at low CO
2 partial pressure, which leads to a preferable use of chemical adsorbents for CO
2 capture from air. On the other hand, while chemical adsorbents have a higher adsorption capacity compared to physical adsorbents, the energy consumption associated with their regeneration is higher for breaking the chemical bonds between the adsorbate and the adsorbent. The use of an amine bonded to a porous solid support, e.g., such as honeycomb monoliths, pellets, or other granular shapes, is therefore suitable [
15,
16,
22].
In cyclic VTSA (vacuum temperature swing adsorption), the air ventilated from the atmosphere may flow through a fixed bed of solid adsorbent, where the CO
2 (and H
2O) is adsorbed on a porous material at ambient temperature and pressure. The flow to be treated is depleted as it progresses through the solid bed, which gradually becomes saturated. After operation and when the adsorbent approaches saturation, the adsorbent is regenerated in situ by heating (TSA around 80–130 °C) and rough vacuum (VSA below 300 mbar
abs). The CO
2 and H
2O are then released in the gas phase, which allows, after gas drying, to recover a high-purity CO
2 stream that can be compressed for transport and storage. The adsorbent is finally cooled to room temperature for reuse in a new cycle (adsorption, purge, regeneration, repressurization). The VTSA proves to be a promising process from analysis of productivity and energy consumption [
23].
Despite their apparent simplicity, the design and development of adsorption processes is time consuming and requires cost reductions to be competitive at full scale. Process modularization is a strong feature in order to reduce investment costs through series and learning effects [
24,
25]. Compared to an all-in-one architecture, the modular design allows to realize and test a large number of modules in a first development phase, before completely setting the optimal module architecture which can then be standardized and produced in large series in order to lower the costs by learning rate. Modularity allows scale-up by increasing the number of components operating in parallel.
There have been a few studies on the modeling of a DAC fixed bed process [
16,
26,
27,
28]. Simulations are most commonly performed at the laboratory scale, i.e., with sorbent material masses in the range of grams to kilograms using amine-functionalized nano-fibrillated cellulose [
16], metal organic framework [
26,
28], or polystyrene [
27]. In this paper, we propose to model in Aspen Adsorption software a DAC modular process that employs VTSA on a packed bed using amine-functionalized material. The one-dimensional model accounts for adsorption isotherms, mass, energy, and momentum balances to simulate temperature and concentration dynamics along the bed. The simulations are performed for several adsorption–desorption cycles at two scales: first at the laboratory scale (2 kgCO
2/year), then at the larger scale of a pilot module (50 tCO
2/year). A parametric study on the main operating conditions, i.e., air velocity (superficial gas velocity lower to minimum fluidization velocity), air relative moisture (0–80%), air temperature (5–35 °C), and CO
2 capture rate (5–98%), is undertaken to assess the global performances and energy consumption. The results obtained in this work can guide future research on the design of a DAC modular process.
3. Results
The modeling approach was at first validated within experimental data of [
32] performed on a lab-scale pilot, around 2.8 kgCO
2/year. A parametric study was then performed on the lab-scale pilot before the latter was scaled-up to the module of around 50 tCO
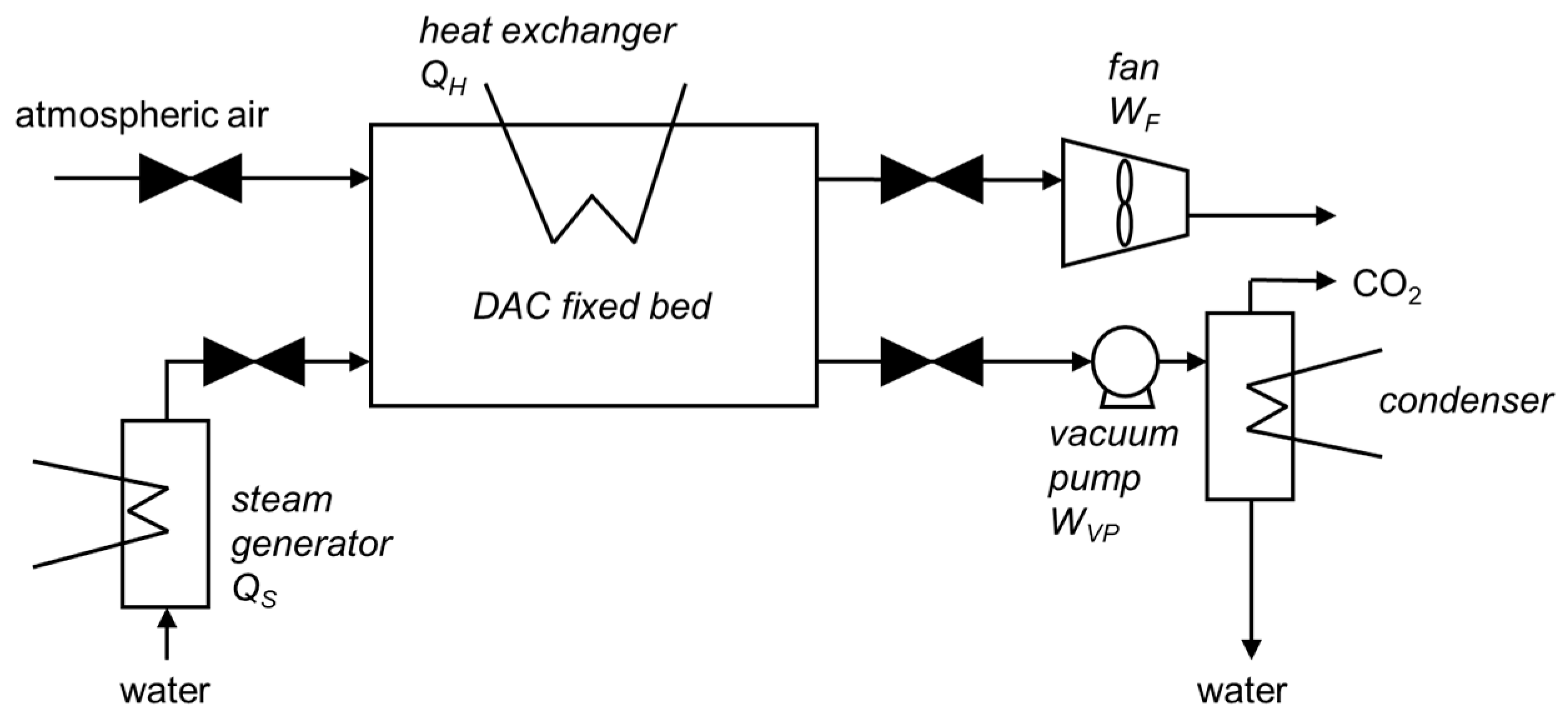
2/year. The module’s bed was equipped with a jacket indirect heat exchanger. Low-grade overheated steam was provided co-currently via a separate inlet. Two separate outlets were used, one rejected the bed gas into the atmosphere during adsorption and the purge steps, while the second one transported the desorbed gas during the desorption step. Both outlet streams were evacuated by dedicated vacuum pumps.
The CO2 was recovered from the gas exiting the bed during the desorption steps and then experiences a condensation and a phase separation step to fully eliminate the steam. The remaining gas was CO2 with a purity superior to 95%.
3.1. Lab-Scale Configuration
The lab-scale characteristics are given in
Table 4. Depending on initialization values provided to the cycle organizer block (
Figure 2), steady state cycles would not be reached within the first cycle but only starting from the second and successive following cycles.
Figure 3 shows established regime of the temperature during cycles.
The largest spatial temperature gradient was about 30 K (
Figure 3) meaning that the bed was globally homogeneous in temperature. A non-obvious behavior occurred at the end of the desorption step when the temperature decreased before the cooling step. This was linked to the endothermic nature of desorption during the steam-stripping step. During this latter step, a higher amount of CO
2 was desorbed in a relatively short time, therefore the temperature of the bed slightly decreased.
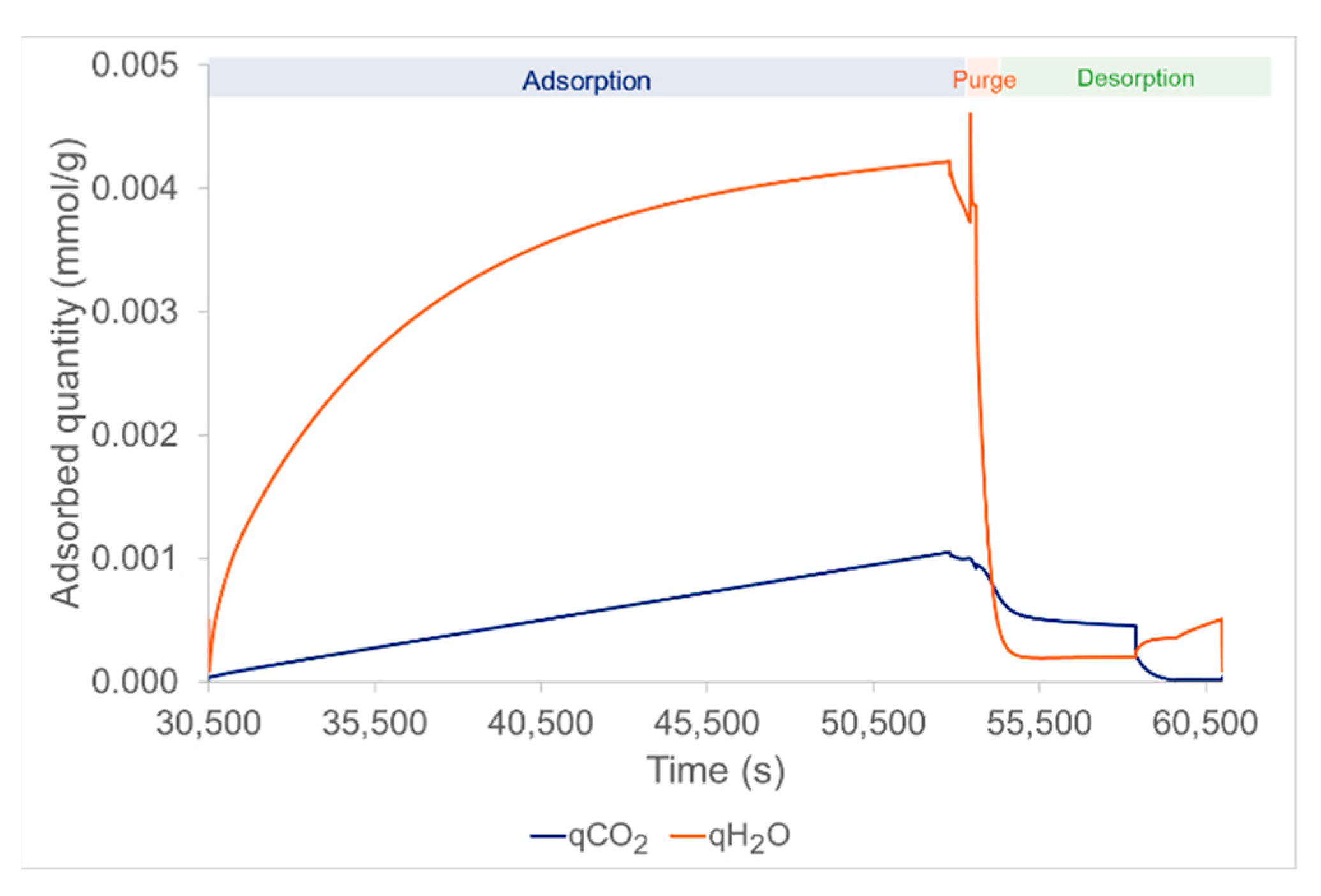
Figure 4 represents the adsorbed quantities of CO
2 and H
2O over time. The evolution during the adsorption phase was relatively different for the H
2O and CO
2. In fact, the adsorbed quantity of H
2O was more than four times larger than for CO
2. This is linked to the partial pressures of the substances and to the fact that the two substances have different isotherms with different mathematical expressions derived from the experimental measurement on the lab-scale pilot test facility. Moreover, H
2O adsorption seems to saturate over adsorption phase time while CO
2 adsorption continues to increase linearly. The H
2O adsorption peak, around
t = 52,500 s (
Figure 4), is linked to the pre-purge step using steam because there is a steep increase in the vapor partial pressure resulting in a quick increase in the adsorbed quantities. Finally, during the vapor stripping step, the vapor partial pressure increases compared to the previous step. Therefore, there is adsorption of H
2O and desorption of CO
2 because of high temperature and low CO
2 partial pressure.
The adsorption uptake was calculated via the results of the CO2 loading variable of adsorbent material over time during the adsorption step. The cycle’s CO2 cyclic capacity was calculated by time integration of the CO2 quantity at the outlet of the bed during desorption and was equal to around 0.83 mmol/g, i.e., 0.037 g/g. The capture rate, i.e., the quantity of CO2 recovered at the end of a whole cycle divided by the quantity of CO2 treated during the adsorption step, was around 77% at a purity larger than 99%.
The specific energy requirements are summarized in
Table 5 below.
Finally, the specific energy need given by the model was consistent with the energy need evaluated by Wurzbacher et al. [
15]. In fact, the data provided values around 9.3 GJ/tCO
2 (i.e., 2600 kWh/tCO
2) for the total thermal energy need and 100 kWh/tCO
2 for the electrical energy need. Furthermore, the significant difference from the minimum separation work, around 100 kWh/tCO
2 (25 times smaller factor in order of magnitude) must be pointed out, which shows the energetic improvement potential to improve this capture technique.
3.2. Parametric Study of the Lab-Scale Process
The objective of this section is to investigate the impact of ambient air parameters on the performance of the DAC process. This way, it is possible to determine the bed dimensions for the most stringent operating point with the objective to minimize energy requirements, while maintaining the highest possible cyclic capacity. Otherwise, it is necessary to increase the bed volume to achieve similar annual capture values.
The parameters of the study and the associated variations are summarized in
Table 6 and the reference points used in the different studies are presented in
Table 7.
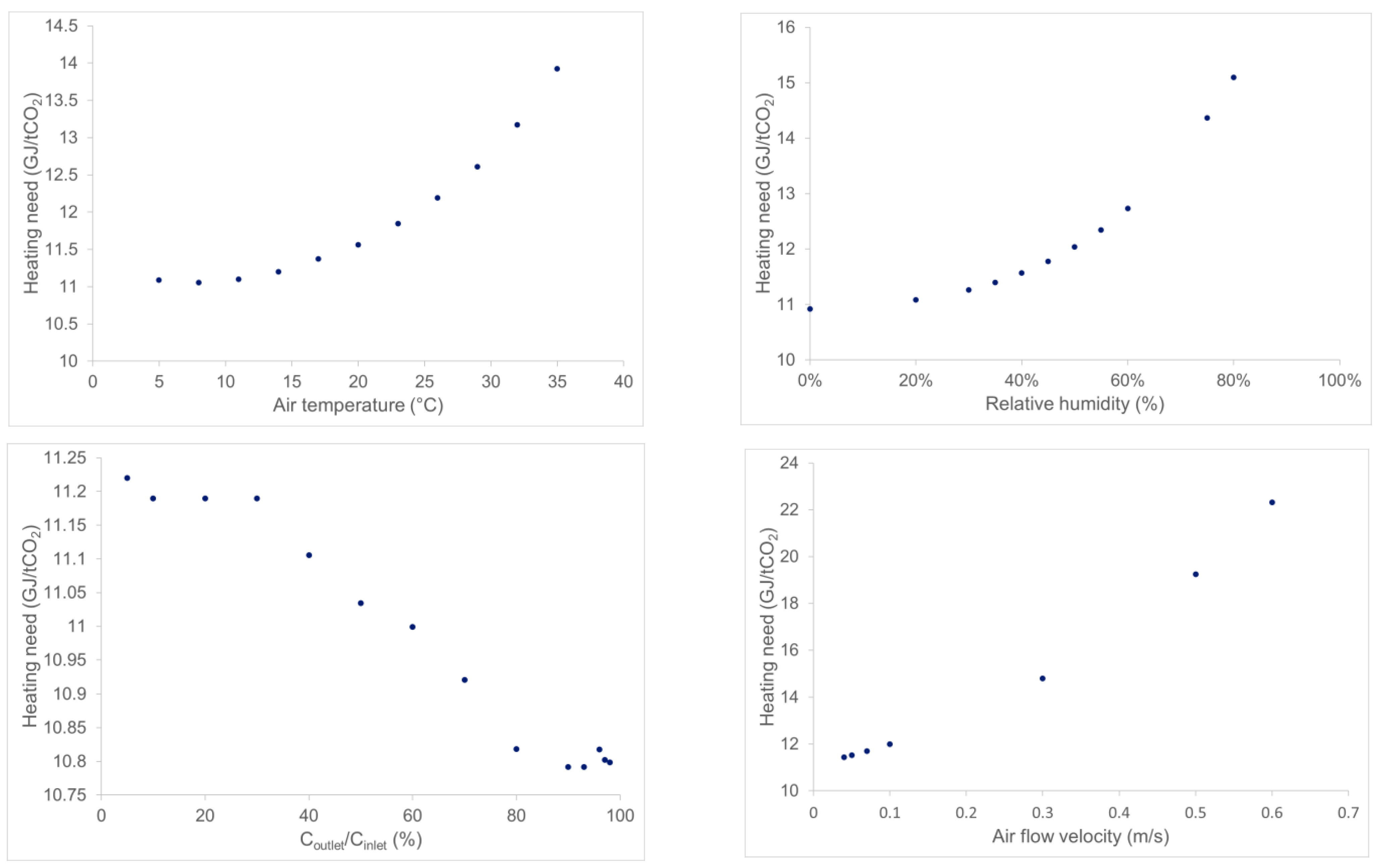
The results are presented in
Figure 5. The evolutions of the CO
2 cyclic capacity (
Figure 5, upper left) are shown to decrease with increasing ambient air temperature. This is directly linked to the isotherm equations presented before that indicate a better adsorption at lower temperature. Surprisingly, as shown in upper-right of
Figure 5, the increase in ambient air relative humidity is favorable for increasing CO
2 cyclic capacity. This is also explained by the expression of the humidity enhancement factor in the binary CO
2 isotherm (Equations (9) and (10)). Indeed, there is competition between increasing the relative humidity and temperature; the first trend increases the CO
2 capacity linearly while the latter is decreased with increasing temperature. These trends are coherent with VTSA desorption technology characteristics observed in laboratory-scale beds [
32].
Figure 5 (bottom-left and right) shows the evolution of the CO
2 cyclic capacity and the mass transfer coefficient (MTC) as functions of the air velocity in the bed. The condition stopping the adsorption remains the same for all simulations, i.e., when the CO
2 breakthrough reaches 10% of the inlet CO
2 concentration. Thus, increasing the air flow leads to an adsorption duration that is too short because of two competitive phenomena: the first one is that adsorption is kinetically faster because there is more CO
2 entering the bed in the same time period leading to higher CO
2 bed-volumetric mole concentration, and the second one is that by increasing air velocity, the residence time of the CO
2 inside the bed becomes closer and closer to the characteristic time of adsorption (~
) (see bottom-right of
Figure 5). Therefore, the thermodynamics of adsorption become less and less predominant over the kinetics. Some CO
2 cannot be adsorbed and goes directly to the outlet. The bed is consequently less charged than in the lower air velocity cases when applied to the CO
2 concentration, at the bed exit, a fixed breakthrough of 10% of the inlet CO
2 concentration.
Figure 5 (bottom-right) shows the sensitivity analysis of the mass transfer coefficients of CO
2 and H
2O on air velocity which was performed for air velocity variation. It shows the interest in working with high air velocity, because the higher the air velocity the lower the predominant diffusion and, consequently, the adsorption step of the cycle is quicker. However, if the increased air velocity is obtained via forced ventilation, then the energy penalty of the fan will increase with the flow velocity. Therefore, there is a tradeoff between the adsorption kinetics, the CO
2 cyclic capacity, and the energy need of the process.
Figure 6 summarizes the specific heating energy results of the parametric study. The results can be explained for ambient temperature and velocity impacts by the same arguments as above: CO
2 cyclic capacity decreases by increasing these parameters, but the cycle’s conditions are unchanged particularly for a fixed breakthrough cutoff level, this leads to a higher specific heating need because all the solid sorbent is heated during the stripping step, while it is not sufficiently loaded with CO
2.
When increasing the breakthrough level (
Figure 6, bottom-left), the cyclic capacity increases, which means that the solid sorbent is loaded with more CO
2 and the heating need decreases slightly. Finally, increasing the humidity rate also increases the partial pressure of H
2O during adsorption, therefore, there is more H
2O being adsorbed and more H
2O to desorb. As desorption is endothermic, an increased amount of H
2O in the air increases the heating need; indeed, increasing the cyclic capacity is not enough to maintain at least a constant specific heating need.
3.3. Modular-Scale Configuration
The lab-scale bed design is very similar to the design presented in [
32], apart from the shape of the bed. The scale-up methodology aims to define the size of the adsorbent bed and the flow rates so that the yearly capture of 50 tCO
2 could be reached.
Given that a large bed diameter allows to increase the volumetric rate of inlet air without increasing the superficial velocity, however, the bed diameter was limited to 1.8 m in order to avoid radial dispersion. In addition, the bed length is also dependent on the material characteristics such as the density and the amine content along with the superficial velocity. A bed with a length of 2 m and a density of 55.4 kg/m3 was considered at first. Increasing the density by a factor of four to reach 221.6 kg/m3, but conserving the adsorbent mass and the same adsorption duration implies having a shorter length of the bed of 0.50 m. Finally, starting from the latter configuration, increasing the amine content of the adsorbent material by 14% implies reducing the length of the bed more, to 0.44 m, to keep the adsorption time constant. Therefore, when increasing both the volume of the bed and the sorbent density or amine content, it allows to increase the superficial velocity while keeping the adsorption time constant.
The chosen design has a bed density of 221.6 kg/m3, a length of 1.78 m, to reach a total weight of bed of 1 ton of adsorbent material. The bulk air velocity during adsorption is about 1.97 m/s.
3.3.1. Up-Scaling Approach
At the lab scale, the global Reynolds number, used to determine whether the fluid flow is laminar or turbulent, reaches a value of 21 (i.e., 1 < Re < 100). It is in a transitory regime where both the inertia and viscous terms have an impact. This configuration allows to limit the pressure drop and still have high values for the mass transfer. For the chosen modular-scale design, the value of the Reynolds number is much higher, around 985. In this configuration, the fluid flow is much more turbulent; therefore, the viscous term becomes negligeable in comparison with inertia forces and, consequently, the pressure drop is around 1500 times higher than the lab-scale bed case.
For the selected design (
Table 8), the Sherwood number is around 64, which is roughly in the same order of magnitude as the lab-scale dimension, around 8. It has little impact on the MTC because the latter is only increased by 8% during the scale-up operation. In both configurations, the axial Péclet numbers are superior to 10
4. Those high values imply that the dispersion is weak.
3.3.2. Performances
The goal of the scaling-up is to design an adsorption bed that could achieve the capture of 50 tCO
2 yearly. The chosen design with high bed weight and short cycle duration (around 6 h 30 min) allows the module to perform more than three cycles per day. The cyclic capacity of this configuration was evaluated as 0.958 mmol of CO
2/g of sorbent, i.e., 0.042 g/g. In this configuration, the module can capture up to 56 tons of CO
2 yearly with a purity superior to 95%. The specific energy requirements are summarized in
Table 9 below.
The strong increase in the fan work (by 345%) is linked to the pressure loss which is much higher in the industrial bed comparatively to the lab-scale bed: −1395 Pa compared to −0.95 Pa for the lab-scale pilot.