Multiplicity Distribution and KNO Scaling of Charged Particles Production from pp Collisions at Different Energies
Abstract
1. Introduction
2. PYTHIA Event Generator
Event and Particle Selections
3. Results and Discussion
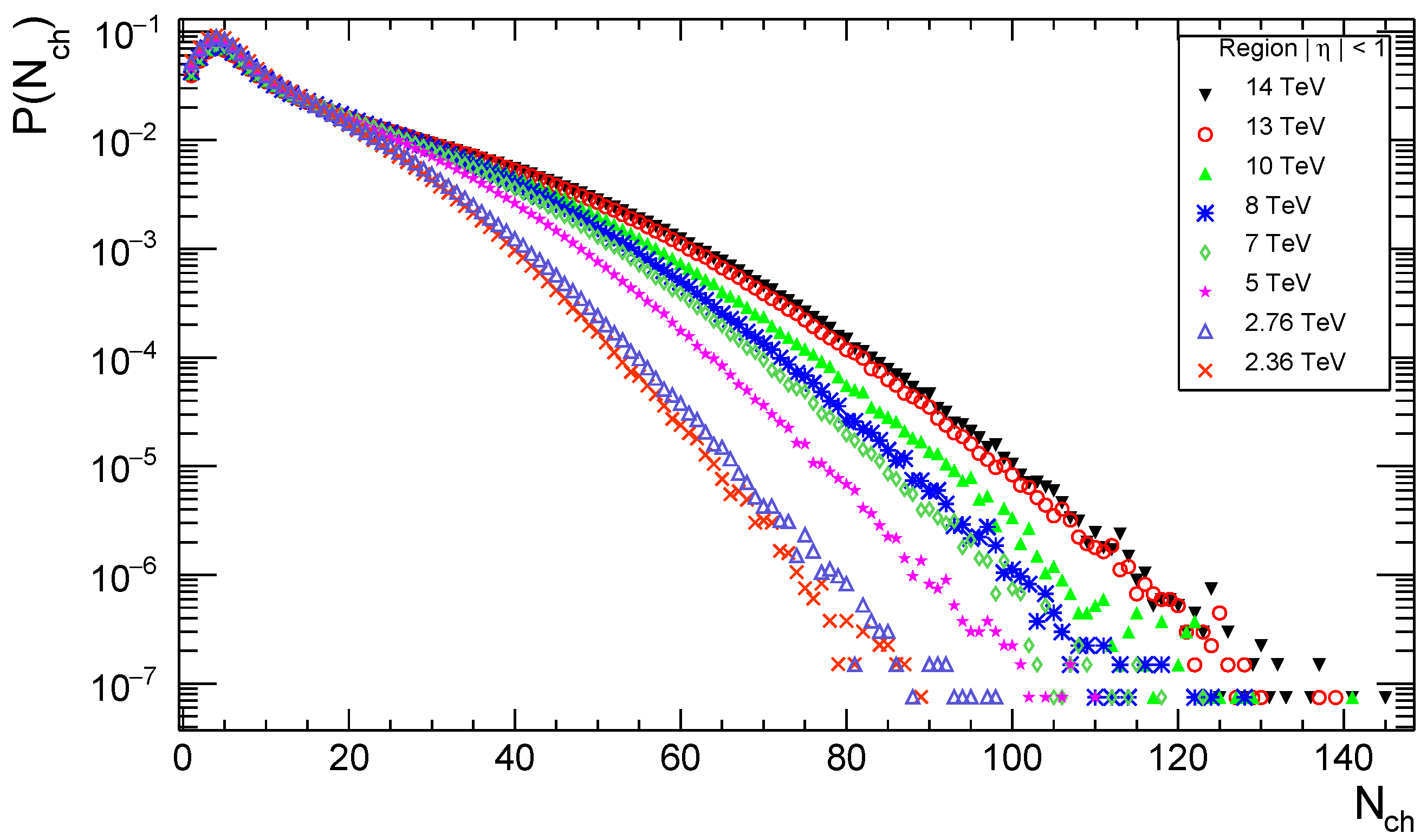
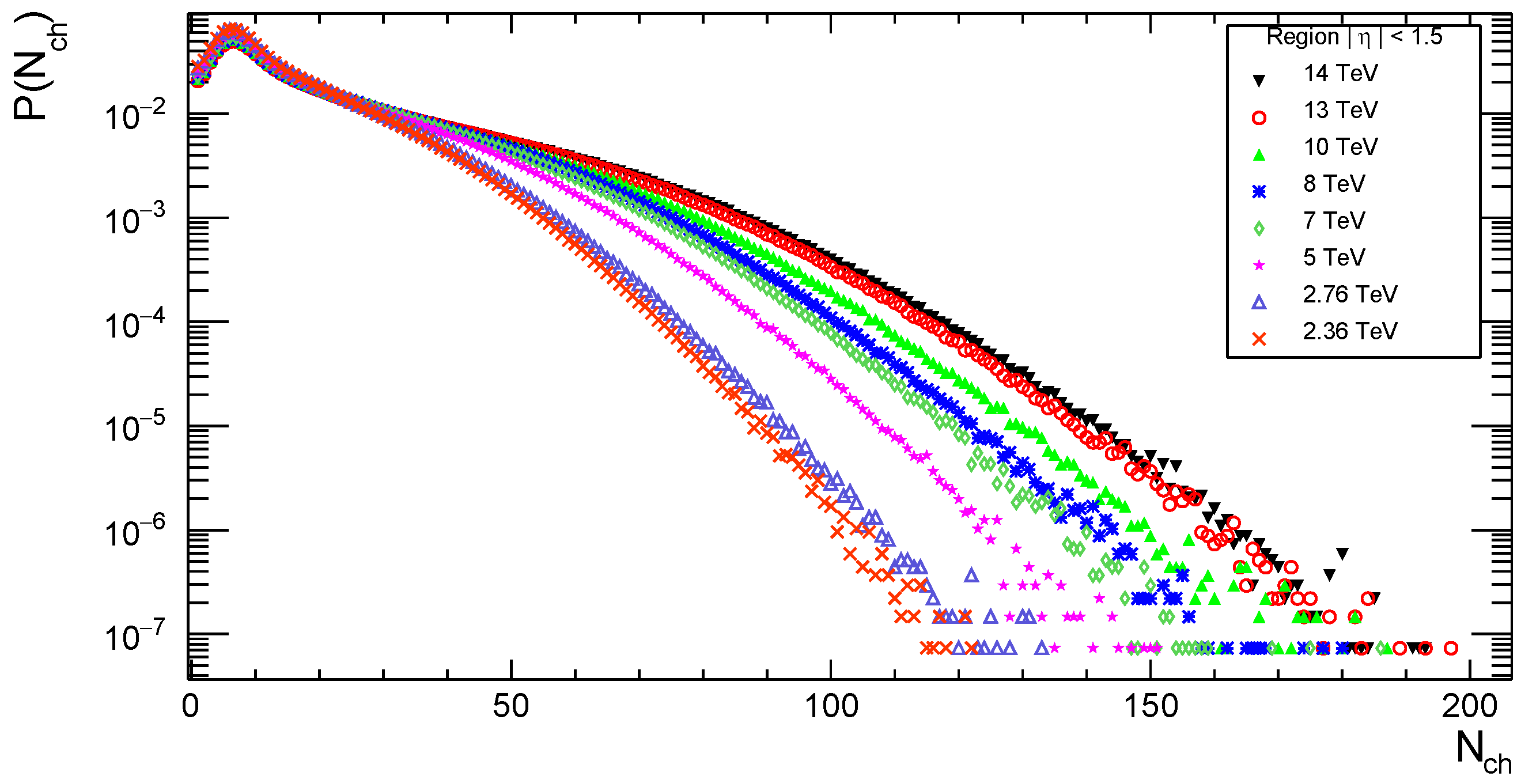
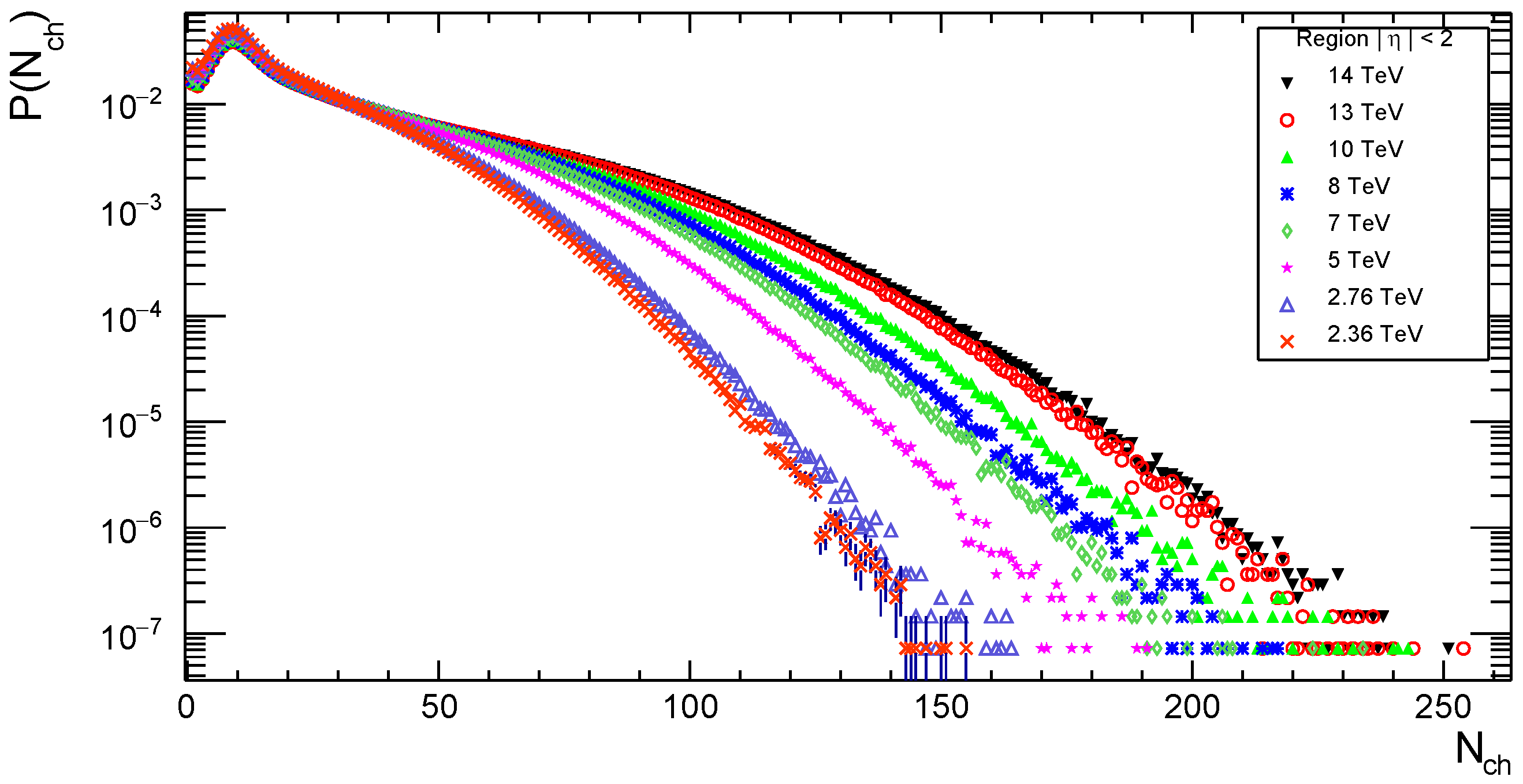
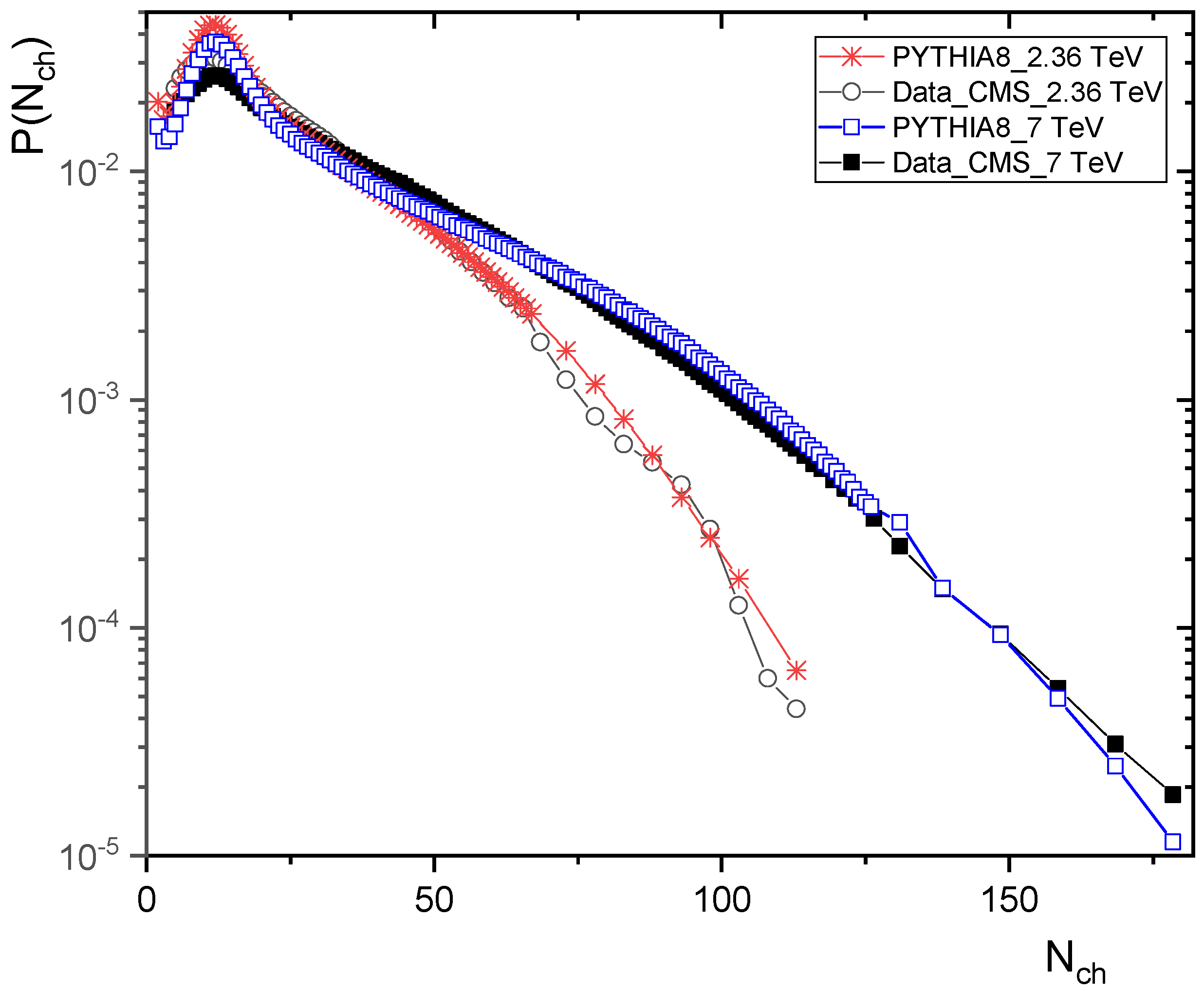
3.1. Multiplicity Distribution of Charged Particles
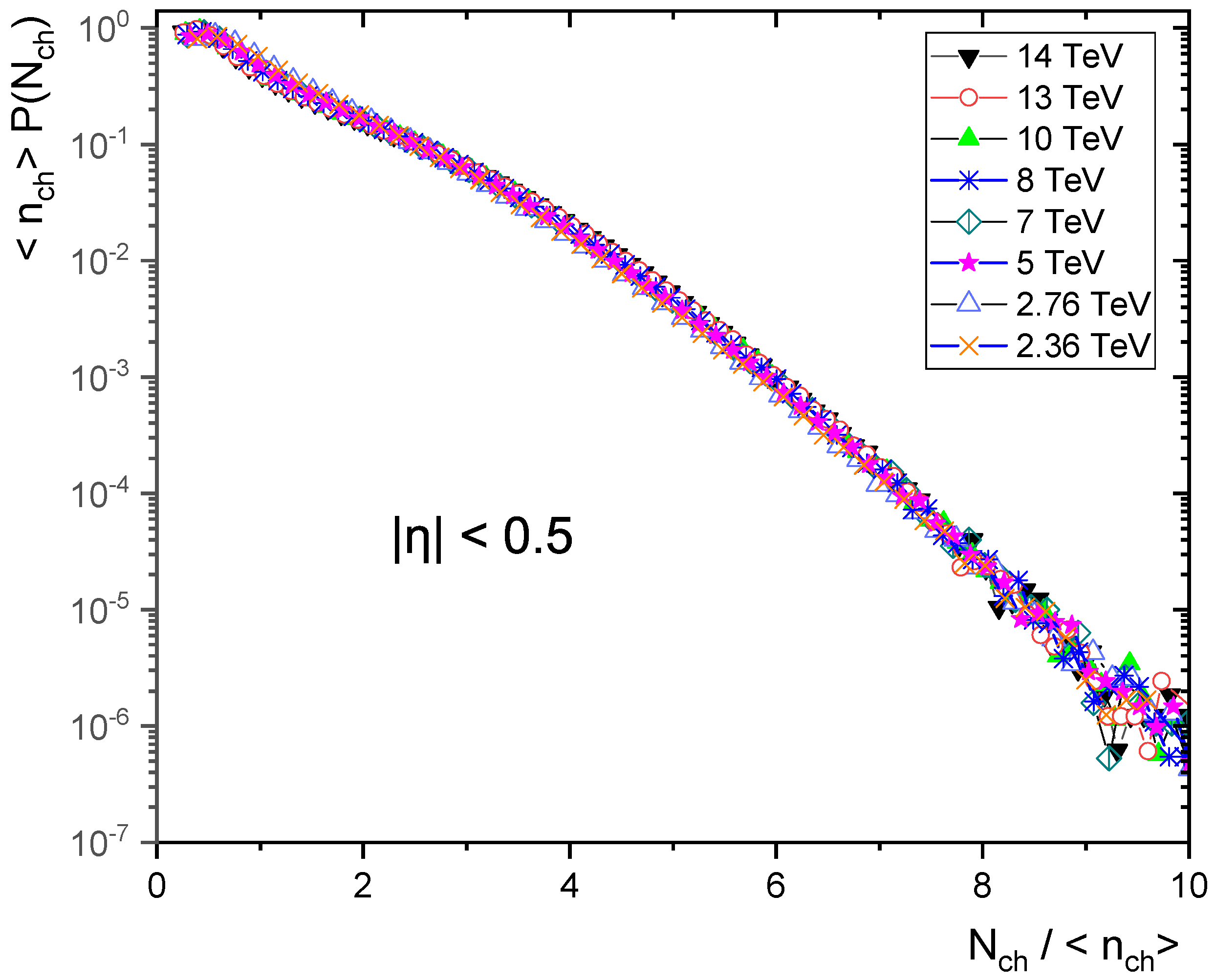
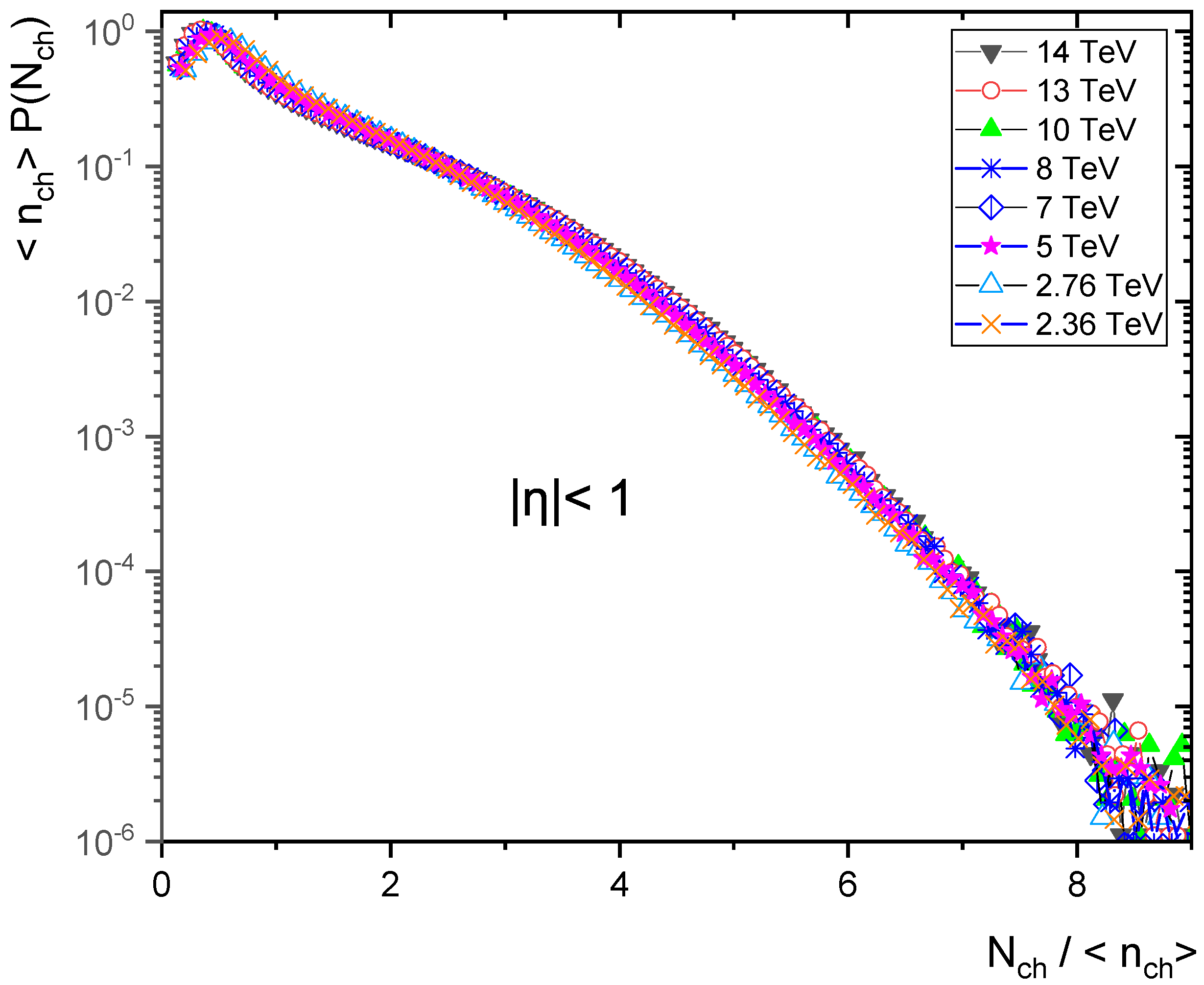
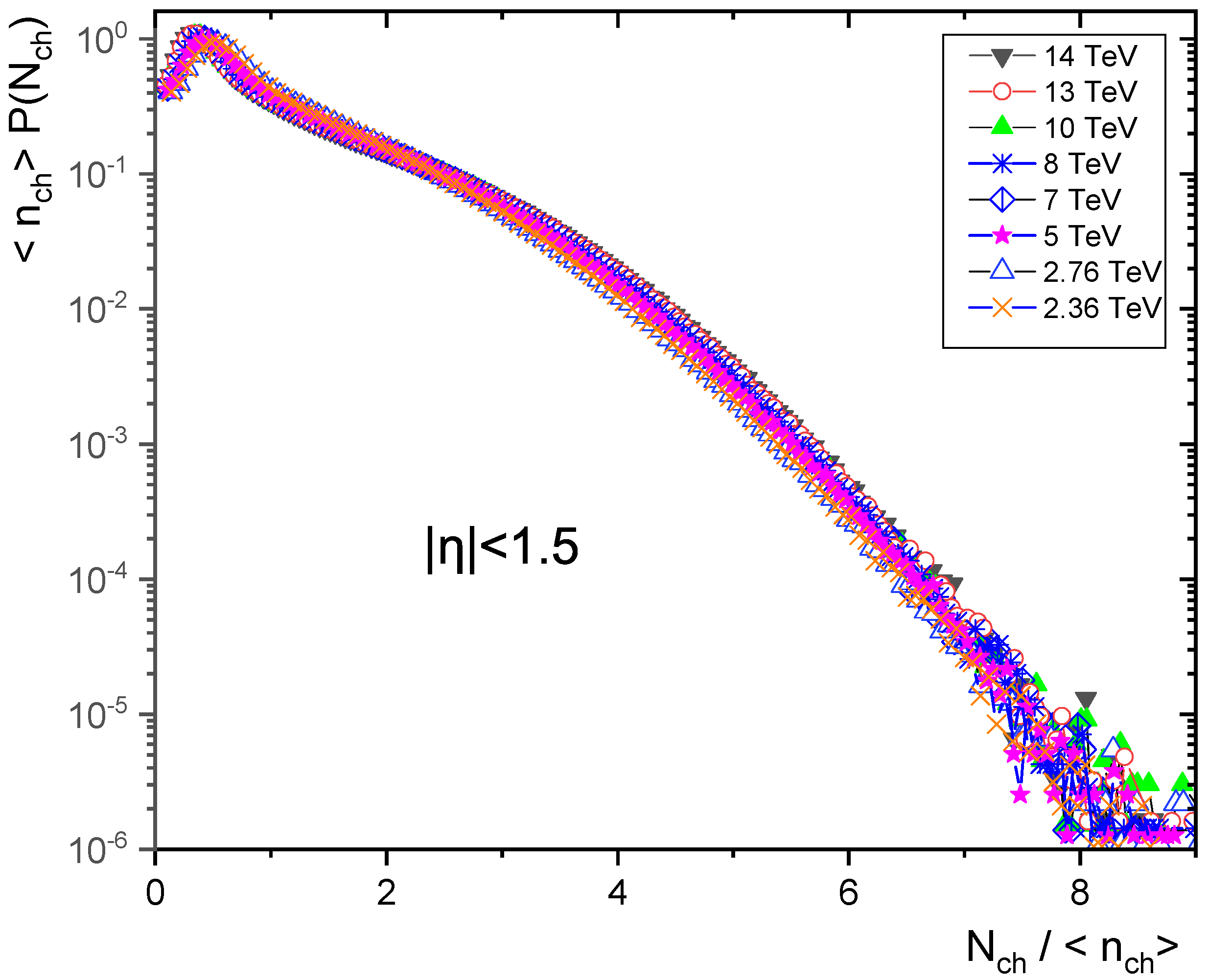
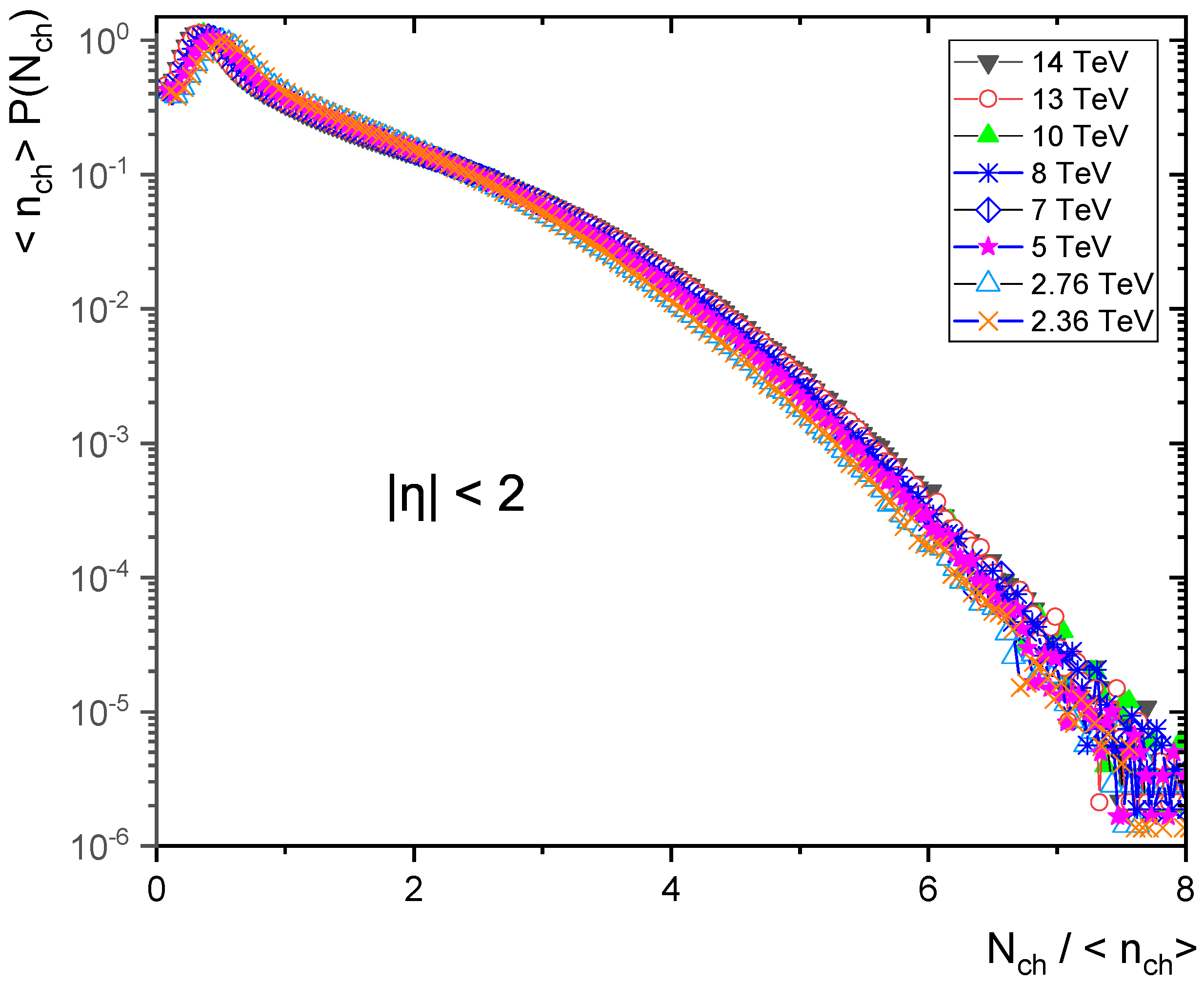
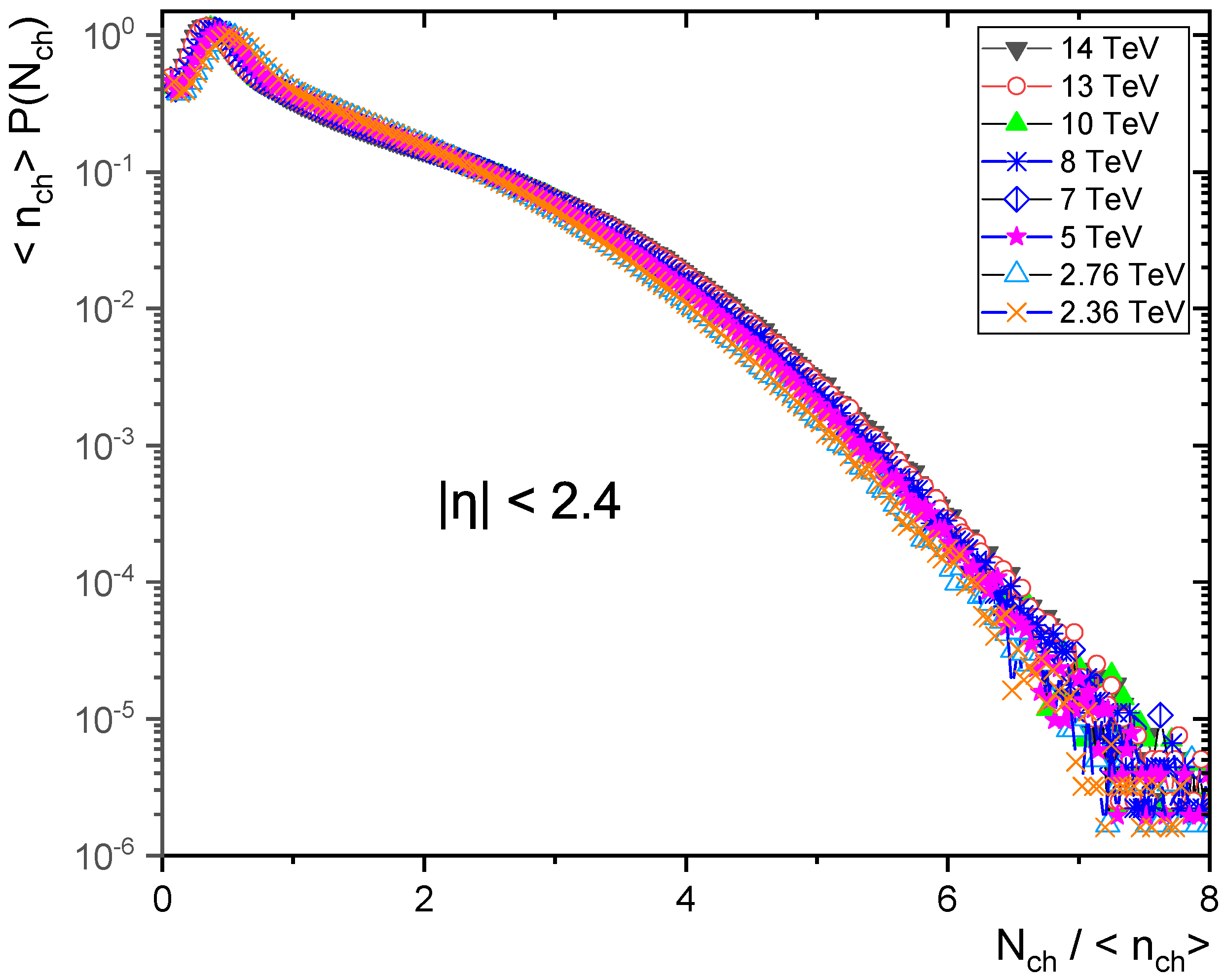
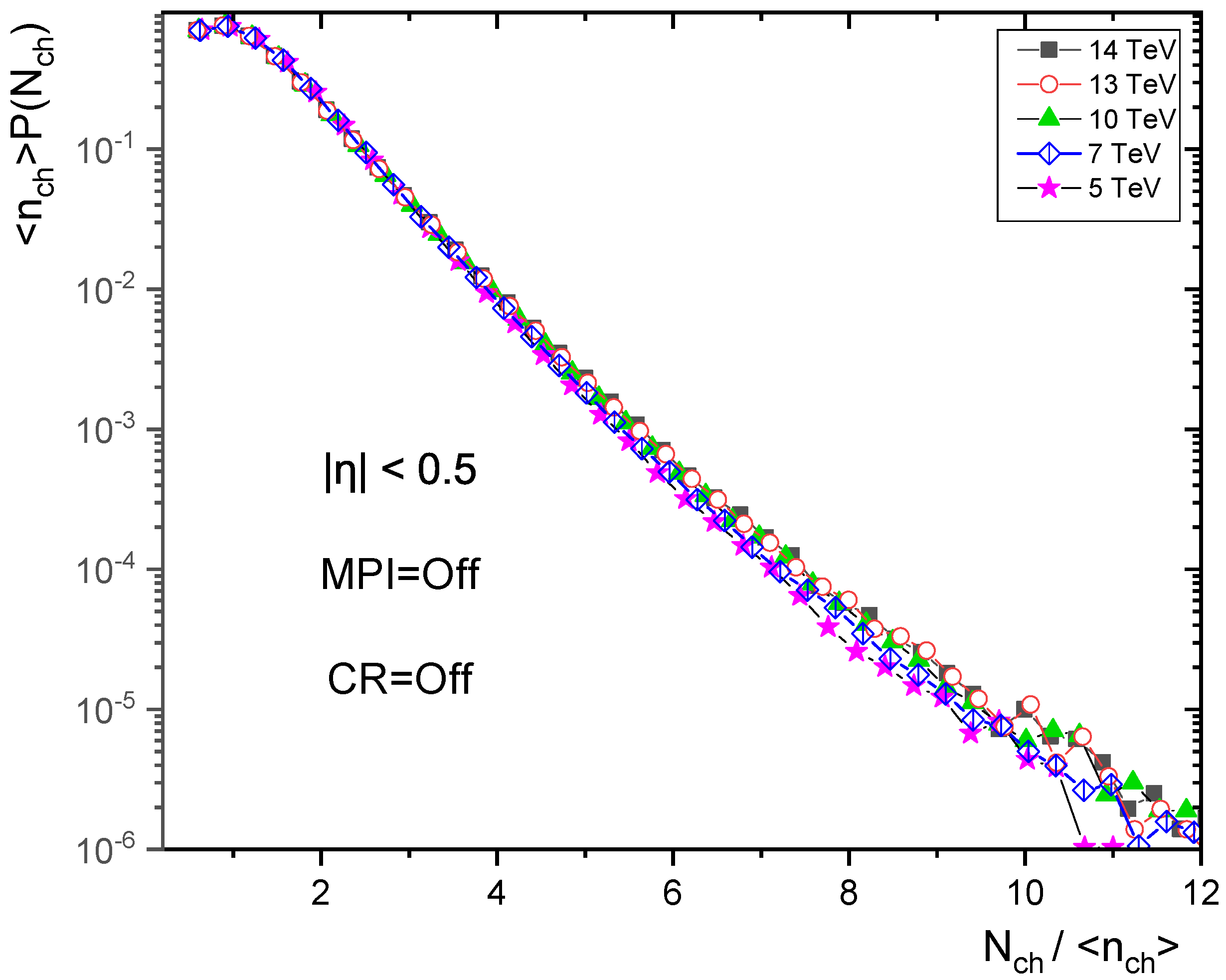
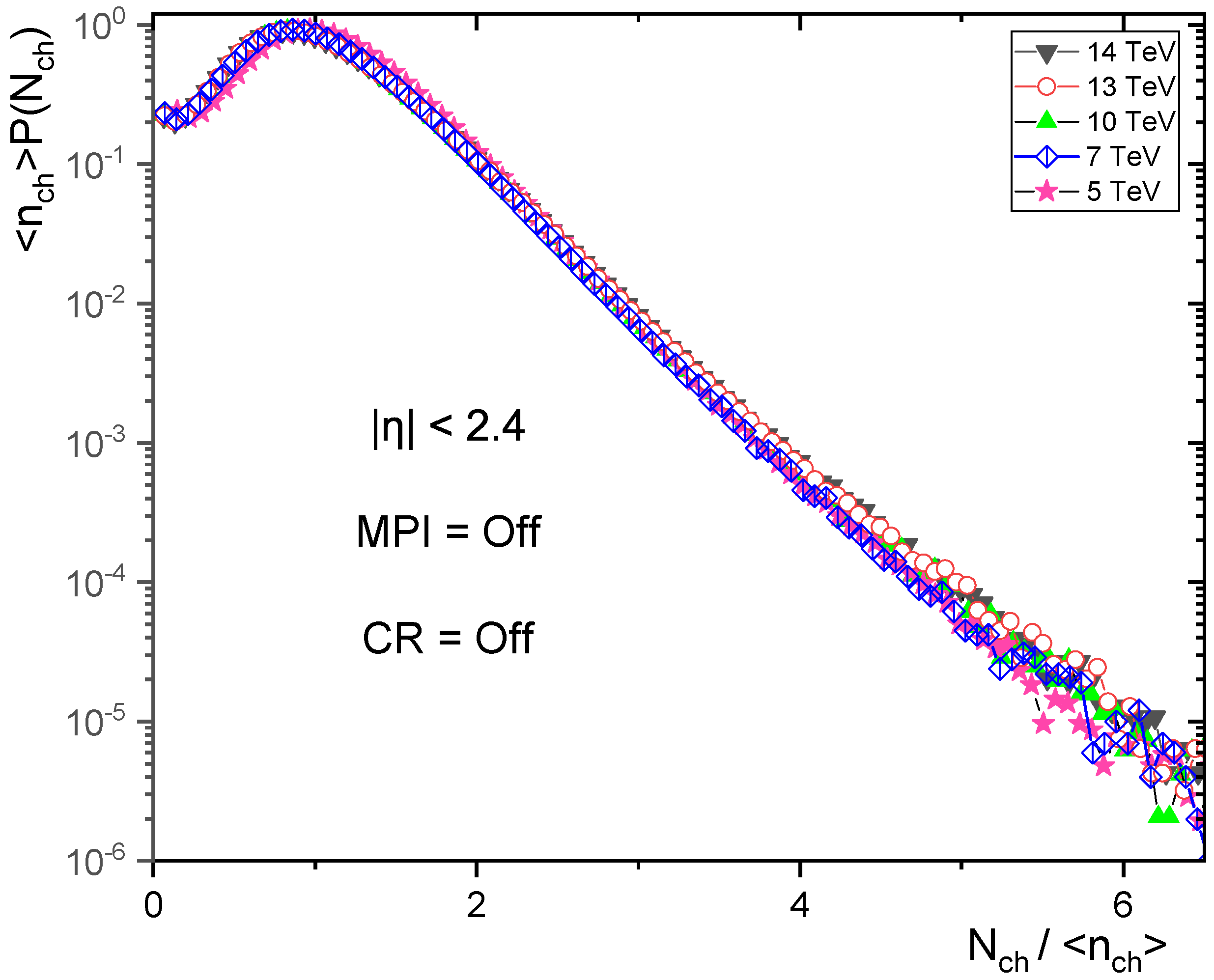
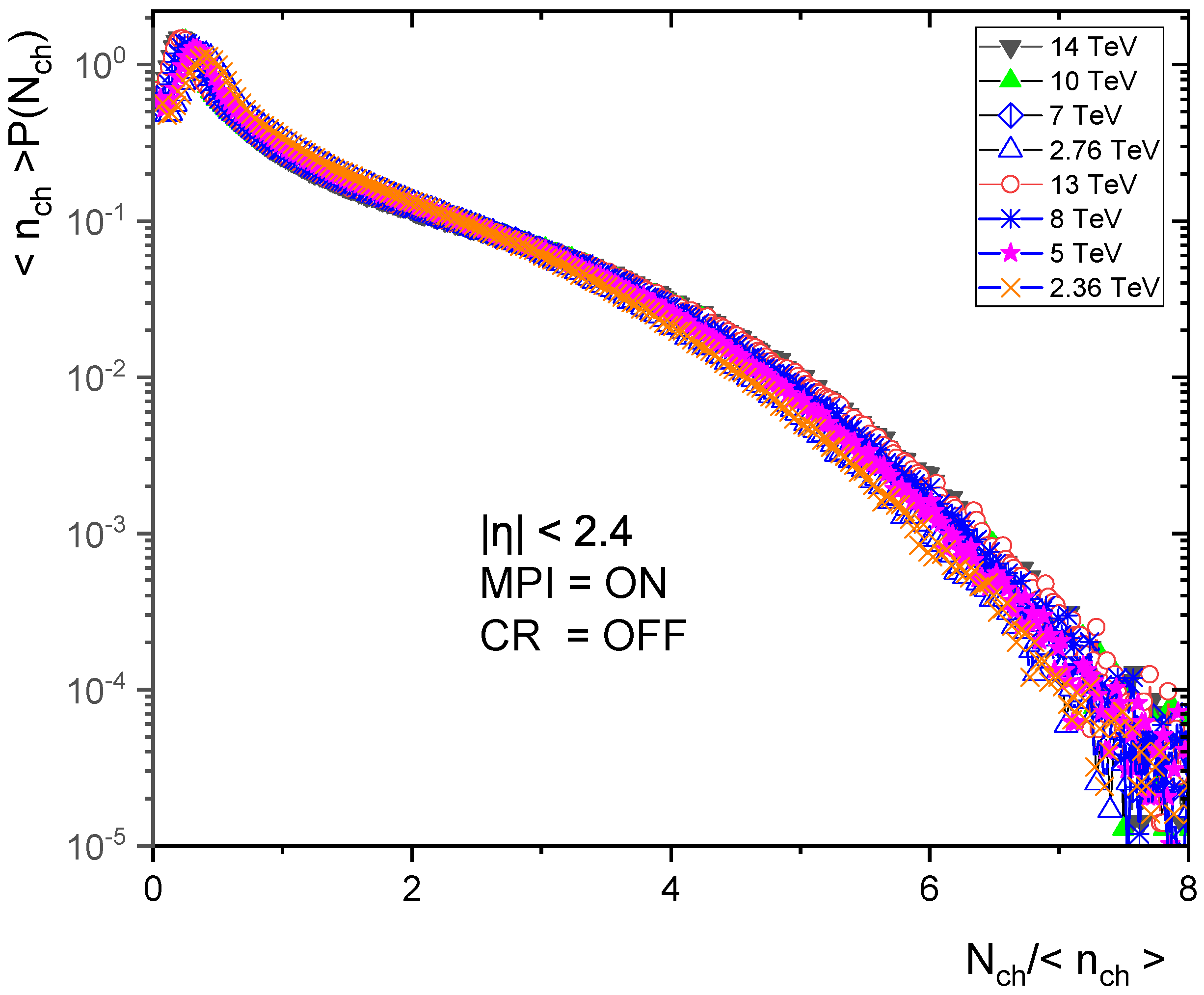
3.2. Testing of KNO Scaling at Different Collisions’ Energies
3.3. Average Multiplicity and Dependence of Energy
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aamodt, K.; Quintana, A.A.; Adamová, D.; Adare, A.M.; Aggarwal, M.M.; Rinella, G.A.; Agocs, A.G.; Salazar, S.A.; Ahammed, Z.; Ahmad, N.; et al. Centrality Dependence of the Charged-Particle Multiplicity Density at Midrapidity in Pb-Pb Collisions at = 2.76 TeV. Phys. Rev. Lett. 2011, 106, 032301. [Google Scholar] [CrossRef] [PubMed]
- CMS Collaboration; Chatrchyan, S.; Bäni, L.; Bortignon, P.; Buchmann, M.A.; Casal, B.; Chanon, N.; Deisher, A.; Dissertori, G.; Dittmar, M.; et al. Measurement of the Pseudorapidity and Centrality Dependence of the Transverse Energy Density in Pb-Pb Collisions at = 2.76 TeV. Phys. Rev. Lett. 2012, 109, 152303. [Google Scholar] [CrossRef] [PubMed]
- Adam, J.; Adamová, D.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahmad, S.; Ahn, S.U.; Aiola, S.; et al. Centrality Dependence of the Charged-Particle Multiplicity Density at Midrapidity in Pb-Pb Collisions at = 5.02 TeV. Phys. Rev. Lett. 2016, 116, 222302. [Google Scholar] [CrossRef] [PubMed]
- Parfenov, P.; Idrisov, D.; Luong, V.; Taranenko, A. Relating Charged Particle Multiplicity to Impact Parameter in Heavy-Ion Collisions at NICA Energies. Particles 2017, 4, 275–287. [Google Scholar] [CrossRef]
- CMS Collaboration; Khachatryan, V.; Sirunyan, A.; Tumasyan, A.; Adam, W.; Asilar, E.; Bergauer, T.; Brandstetter, J.; Brondolin, E.; Dragicevic, M.; et al. Evidence for collectivity in pp collisions at the LHC. Phys. Lett. B 2017, 765, 193–220. [Google Scholar] [CrossRef]
- CMS Collaboration; Khachatryan, V.; Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Bergauer, T.; Dragicevic, M.; Erö, J.; Fabjan, C.; Friedl, M.; et al. Charged particle multiplicities in pp interactions at = 0.9, 2.36, and 7 TeV. J. High Energy Phys. 2011, 01, 079. [Google Scholar] [CrossRef]
- ALICE Collaboration; Aamodt, K.; Abel, N.; Abeysekara, U.; Abrahantes Quintana, A.; Abramyan, A.; Adamová, D.; Aggarwal, M.M.; Aglieri Rinella, G.; Agocs, A.G.; et al. Charged-particle multiplicity measurement in proton–proton collisions at = 7 TeV with ALICE at LHC. Eur. Phys. J. C 2010, 68, 345–354. [Google Scholar] [CrossRef]
- ATLAS Collaboration; Aad, G.; Abbott, B.; Abdallah, J.; Abdelalim, A.A.; Abdesselam, A.; Abdinov, O.; Abi, B.; Abolins, M.; Abramowicz, H.; et al. Charged-particle multiplicities in pp interactions measured with the ATLAS detector at the LHC. New J. Phys. 2011, 13, 053033. [Google Scholar] [CrossRef]
- ALICE Collaboration; Acharya, S.; Adamová, D.; Adolfsson, J.; Aggarwal, M.M.; AglieriRinella, G.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahmad, N.; et al. Charged-particle multiplicity distributions over a wide pseudorapidity range in proton-proton collisions at = 0.9, 7, and 8 TeV. Eur. Phys. J. C 2017, 10, 852. [Google Scholar] [CrossRef]
- CMS Collaboration; Khachatryan, V.; Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Bergauer, T.; Dragicevic, M.; Ero, J.; Fabjan, C.; Friedl, M.; et al. Transverse momentum and pseudorapidity distributions of charged hadrons in pp collisions at = 7 TeV. Phys. Rev. Lett. 2010, 105, 022002. [Google Scholar] [CrossRef]
- ATLAS Collaboration; Aad, G.; Abat, E.; Abbott, B.; Abdallah, J.; Abdelalim, A.A.; Abdesselam, A.; Abdinov, O.; Abi, B.; Abolins, M.; et al. Charged-particle multiplicities in pp interactions at = 900 GeV measured with the ATLAS detector at the LHC. Phys. Lett. B 2010, 688, 21–42. [Google Scholar] [CrossRef]
- Aad, G.; Abbott, B.; Abdallah, J.; Abdinov, O.; Abeloos, B.; Aben, R.; Abolins, M.; AbouZeid, O.; Abraham, N.; Abramowicz, H.; et al. Charged-particle distributions in = 13 TeV pp interactions measured with the ATLAS detector at the LHC. Phys. Lett. B 2016, 758, 67–88. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahmad, S.; et al. Multiplicity dependence of π, K, and p production in pp collisions at = 13 TeV. Eur. Phys. J. C 2020, 80, 67–88. [Google Scholar] [CrossRef]
- Acharya, S.; ALICE Collaboration; Adamová, D.; Adhya, S.P.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; et al. Charged-particle production as a function of multiplicity and transverse spherocity in pp collisions at = 5.02 and 13 TeV. Eur. Phys. J. C 2019, 79, 67–88. [Google Scholar] [CrossRef]
- Sjöstrand, T.; Mrenna, S.; Skands, P. PYTHIA 6.4 physics and manual. J. High Energy Phys. 2006, 05, 026. [Google Scholar] [CrossRef]
- Buckley, A.; Butterworth, J.; Gieseke, S.; Grellscheid, D.; Höche, S.; Hoeth, H.; Krauss, F.; Lönnblad, L.; Nurse, E.; Richardson, P.; et al. General-purpose event generators for LHC physics. Phys. Rep. 2011, 504, 145–233. [Google Scholar] [CrossRef]
- Sjöstrand, T.; Skands, P.Z. Transverse-momentum-ordered showers and interleaved multiple interactions. Eur. Phys. J. C 2005, 39, 129–154. [Google Scholar] [CrossRef]
- Sjöstrand, T.; van Zijl, M. A multiple-interaction model for the event structure in hadron collisions. Phys. Rev. D 1987, 36, 2019. [Google Scholar] [CrossRef]
- Andersson, B.; Gustafson, G.; Ingelman, G.; Sjöstrand, T. Parton fragmentation and string dynamics. Phys. Rep. 1983, 97, 31–145. [Google Scholar] [CrossRef]
- Sjöstrand, T. Jet fragmentation of multiparton configurations in a string framework. Nucl. Phys. B 1984, 248, 469–502. [Google Scholar] [CrossRef]
- Corke, R.; Sjöstrand, T. Interleaved parton showers and tuning prospects. J. High Energy Phys. 2011, 3, 32. [Google Scholar] [CrossRef]
- Skands, P.; Carrazza, S.; Rojo, J. Tuning PYTHIA 8.1: The Monash 2013 tune. Eur. Phys. J. C 2014, 74, 3024. [Google Scholar] [CrossRef]
- Skands. Soft-QCD and UE spectra in pp collisions at very high CM energies (a Snowmass white paper). arXiv 2013, arXiv:1308.2813. [Google Scholar]
- Dobbs, M.; Hansen, J.B. The HepMC C++ Monte Carlo event record for High Energy Physics. Comput. Phys. Commun. 2001, 134, 41–46. [Google Scholar] [CrossRef]
- Aggarwal, R.; Kaur, M. Charged particle production in pp collisions at = 8, 7 and 2.76 TeV at the LHC—A case study. Int. J. Mod. Phys. E 2021, 30, 2150005. [Google Scholar] [CrossRef]
- Alexopoulos, T.; Anderson, E.W.; Biswas, N.N.; Bujak, A.; Carmony, D.D.; Erwin, A.R.; Gutay, L.J.; Hirsch, A.S.; Hojvat, C.; Kenney, V.P.; et al. The role of double parton collisions in soft hadron interactions. Phys. Lett. B 1998, 435, 453–457. [Google Scholar] [CrossRef]
- Giovannini, A.; Ugoccioni, R. Soft and semi-hard components structure in multiparticle production in high energy collisions. Nucl. Phys. Proc. Suppl. 1999, 71, 201. [Google Scholar] [CrossRef]
- Schegelsky, V.A.; Martinb, A.D.; Ryskin, M.G.; Khoze, V.A. Pomeron universality from identical pion correlations at the LHC. Phys. Lett. B 2011, 703, 288–291. [Google Scholar] [CrossRef][Green Version]
- Koba, Z.; Nielsen, H.B.; Olesen, P. Scaling of multiplicity distributions in high-energy hadron collisions. Nucl. Phys. B 1972, 40, 317–334. [Google Scholar] [CrossRef]
- Feynman, R.P. Very High-Energy Collisions of Hadrons. Phys. Rev. Lett. 1969, 23, 1415. [Google Scholar] [CrossRef]
- Thomé, W.; Eggert, K.; Giboni, K.; Lisken, H.; Darriulat, P.; Dittmann, P.; Holder, M.; McDonald, K.; Albrecht, H.; Modis, T.; et al. Charged particle multiplicity distributions in p p collisions at ISR energies. Nucl. Phys. B 1977, 129, 365. [Google Scholar] [CrossRef]
- Breakstone, A.; Campanini, R.; Crawley, H.B.; Dallavalle, G.M.; Deninno, M.M.; Doroba, K.; Drijard, D.; Fabbri, F.; Faessler, M.A.; Firestone, A.; et al. Charged multiplicity distribution in p p interactions at ISR energies. Phys. Rev. D 1984, 30, 528. [Google Scholar] [CrossRef]
- EHS/NA22 Collaboration; Adamus, M.; Agababyan, N.M.; Ajinenko, I.V.; Belokopytov, Y.A.; Białkowska, H.; Böttcher, H.; Chliapnikov, P.V.; Crijns, F.; de Roeck, A.; et al. Phase space dependence of the multiplicity distribution in π+ p and p p collisions at 250-GeV/c. Z. Phys. C 1988, 37, 215. [Google Scholar]
- NA22 Collaboration; Adamus, M.; Ajinenko, I.V.; De Almeida, F.M.L., Jr.; Belokopytov, Y.A.; Białkowska, H.; Boettcher, H.; Chliapnikov, P.V.; Crijns, F.; de Roeck, A.; et al. Rapidity dependence of negative and all charged multiplicities in nondiffractive π+ p and p p collisions at 250-GeV/c. Phys. Lett. B 1986, 177, 239. [Google Scholar] [CrossRef]
- CDF Collaboration. Soft and hard interactions in pp collisions at = 1800 GeV and 630 GeV. Phys. Rev. D 2002, 65, 072005. [Google Scholar]
- Capella, A.; Staar, A.; Van, J.T.T. KNO Scaling Violations in the Dual Parton Model. Phys. Rev. D 1985, 32, 2933. [Google Scholar] [CrossRef]
- Bleibel, J.; Bravina, L.V.; Zabrodin, E.E. How many of the scaling the scaling trends in pp collisions will be violated at = 14 TeV?—Predictions from Monte Carlo quark-gluon string model. Phys. Rev. D 2016, 93, 114012. [Google Scholar] [CrossRef]
- Alner, G.; Ansorge, R.; Åsman, B.; Böckmann, K.; Booth, C.; Burow, L.; Carlson, P.; Declercq, C.; Dewolf, R.; Eckart, B.; et al. Scaling violations in multiplicity distributions at 200-GeV and 900-GeV. Phys. Lett. B 1986, 167, 476–480. [Google Scholar] [CrossRef]
- Hegyi, S. KNO scaling 30 years later. Nucl. Phys. Proc. Suppl. 2001, 92, 122–129. [Google Scholar] [CrossRef]
- Bialas, A.; Derado, I.; Stodolsky, L. KNO Scaling Violation and the ’Bremsstrahlung’ Analogy. Phys. Lett. B 1985, 56, 421. [Google Scholar] [CrossRef]
- Matinyan, S.G.; Walker, W.D. Multiplicity distribution and mechanisms of the high energy hadron collisions. Phys. Rev. D 1999, 59, 034022. [Google Scholar] [CrossRef]
- Sjöstrand, T. The Development of MPI Modelling in PYTHIA. Adv. Ser. Direct. High Energy Phys. 2018, 29, 191–225. [Google Scholar]
- Gustafson, G. Multiple Interactions, Saturation and Final States in pp Collisions and DIS. Acta Phys. Pol. B 2009, 40, 1981. [Google Scholar]
- Weber, S.G.; Dubla, A.; Andronic, A.; Morsch, A. Elucidating the multiplicity dependence of J/Ψ production in proton–proton collisions with PYTHIA8. Eur. Phys. J. C 2019, 79, 36. [Google Scholar] [CrossRef]
- Vertesi, R.; Gemes, A.; Barnafoldi, G.G. Koba-Nielsen-Olesen-like scaling within a jet in proton-proton collisions at LHC energies. Phys. Rev. D 2021, 103, L051503. [Google Scholar] [CrossRef]
- Ortiz, A.; Palomo, L.V. Probing color reconnection with underlying event observables at the LHC energies. Phys. Rev. D 2019, 99, 034027. [Google Scholar] [CrossRef]
- Alner, G.; Alpgård, K.; Ansorge, R.; Åsman, B.; Böckmann, K.; Booth, C.; Burow, L.; Carlson, P.; Chevalley, J.-L.; DeClercq, C.; et al. Scaling violation favoring high multiplicity events at 540-GeV CMS energy. Phys. Lett. B 1984, 138, 304–310. [Google Scholar] [CrossRef]
- UA1 Collaboration; Albajar, C.; Albrow, M.G.; Allkofer, O.C.; Andrieu, B.; Ankoviak, K.; Apsimon, R.; Astbury, A.; Aubert, B.; Bacci, C.; et al. A study of the general characteristics of p collisions at = 0.2-TeV to 0.9-TeV. Nucl. Phys. B 1990, 335, 261–287. [Google Scholar] [CrossRef]



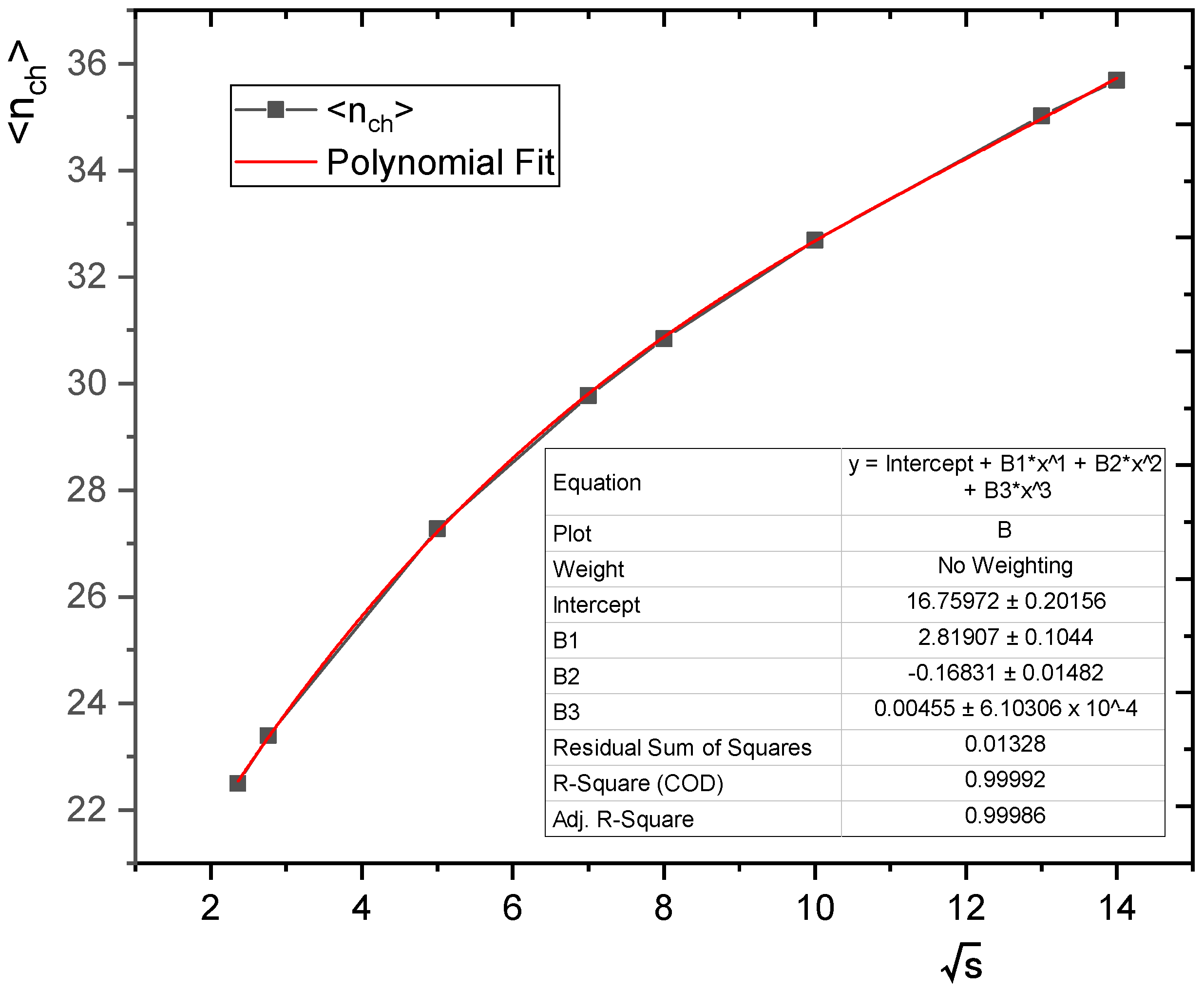
| (TeV) | Number of Generated Events × 107 | ||
|---|---|---|---|
| 2.36 | 22.49 | 22.9 ± 2.05 (stat. + syst.) | 1.396728 |
| 2.76 | 23.39 | 1.396718 | |
| 5 | 27.27 | - | 1.396264 |
| 7 | 29.77 | 30.4 ± 2.2 (stat. + syst.) | 1.39555 |
| 8 | 30.84 | 30.82 ± 0.35 (stat.) | 1.395548 |
| 10 | 32.68 | - | 1.395809 |
| 13 | 35.2 | 1.396184 | |
| 14 | 35.69 | - | 1.396307 |
| Parameter | Value |
|---|---|
| B | 16.75972 ± 0.20156 |
| B1 | 2.81907 ± 0.1044 |
| B2 | −0.16831 ± 0.01482 |
| B3 | 0.00455 ± 6.10306 × 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahmoud, M.A. Multiplicity Distribution and KNO Scaling of Charged Particles Production from pp Collisions at Different Energies. Particles 2022, 5, 96-109. https://doi.org/10.3390/particles5020009
Mahmoud MA. Multiplicity Distribution and KNO Scaling of Charged Particles Production from pp Collisions at Different Energies. Particles. 2022; 5(2):96-109. https://doi.org/10.3390/particles5020009
Chicago/Turabian StyleMahmoud, Mohammed Attia. 2022. "Multiplicity Distribution and KNO Scaling of Charged Particles Production from pp Collisions at Different Energies" Particles 5, no. 2: 96-109. https://doi.org/10.3390/particles5020009
APA StyleMahmoud, M. A. (2022). Multiplicity Distribution and KNO Scaling of Charged Particles Production from pp Collisions at Different Energies. Particles, 5(2), 96-109. https://doi.org/10.3390/particles5020009






