Rabi Frequency Management of Collapsing Quasi-Two-Dimensional Bose-Einstein Condensates with Pseudospin-1/2
Abstract
:1. Introduction
2. The Model and Main Parameters
3. Numerical Results and Discussion
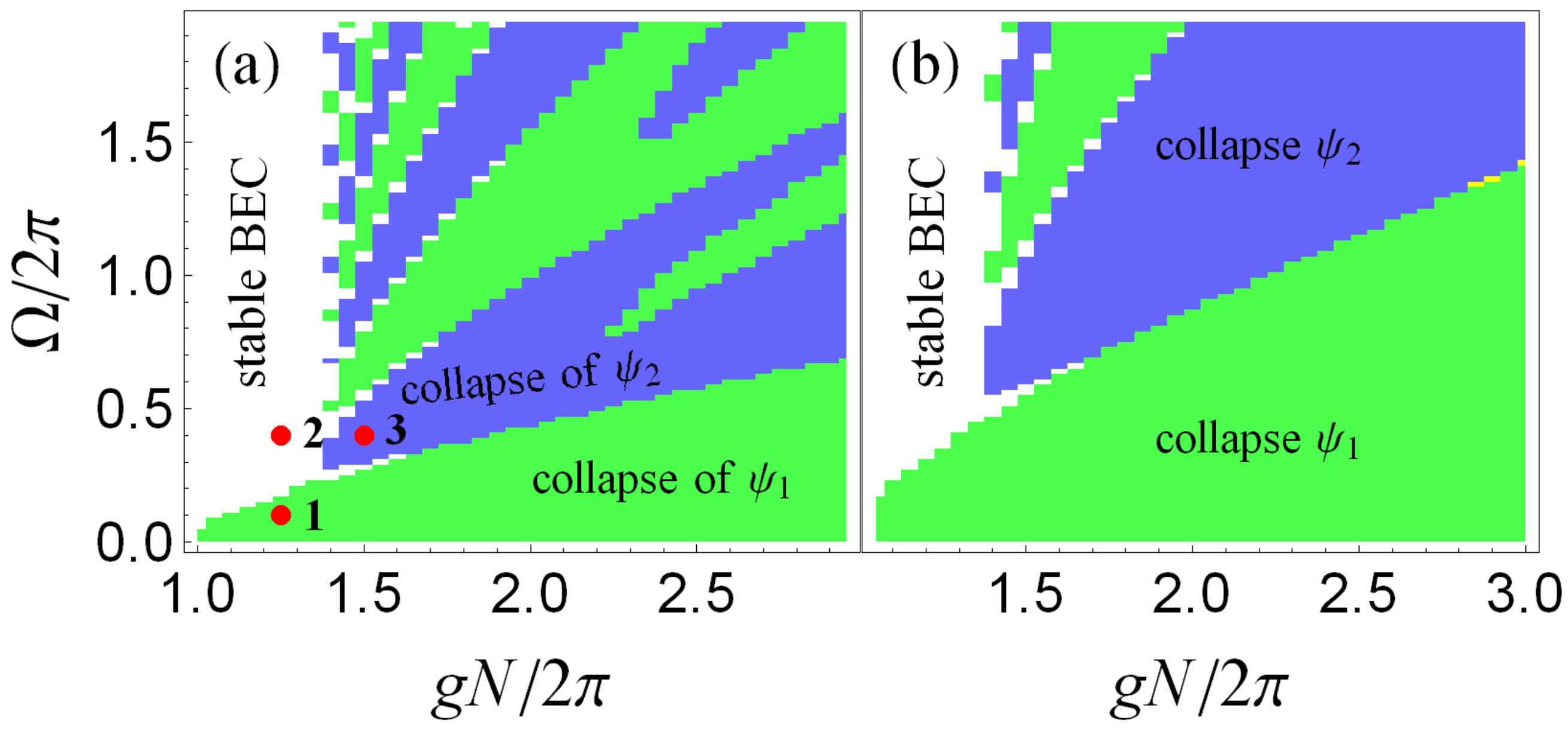
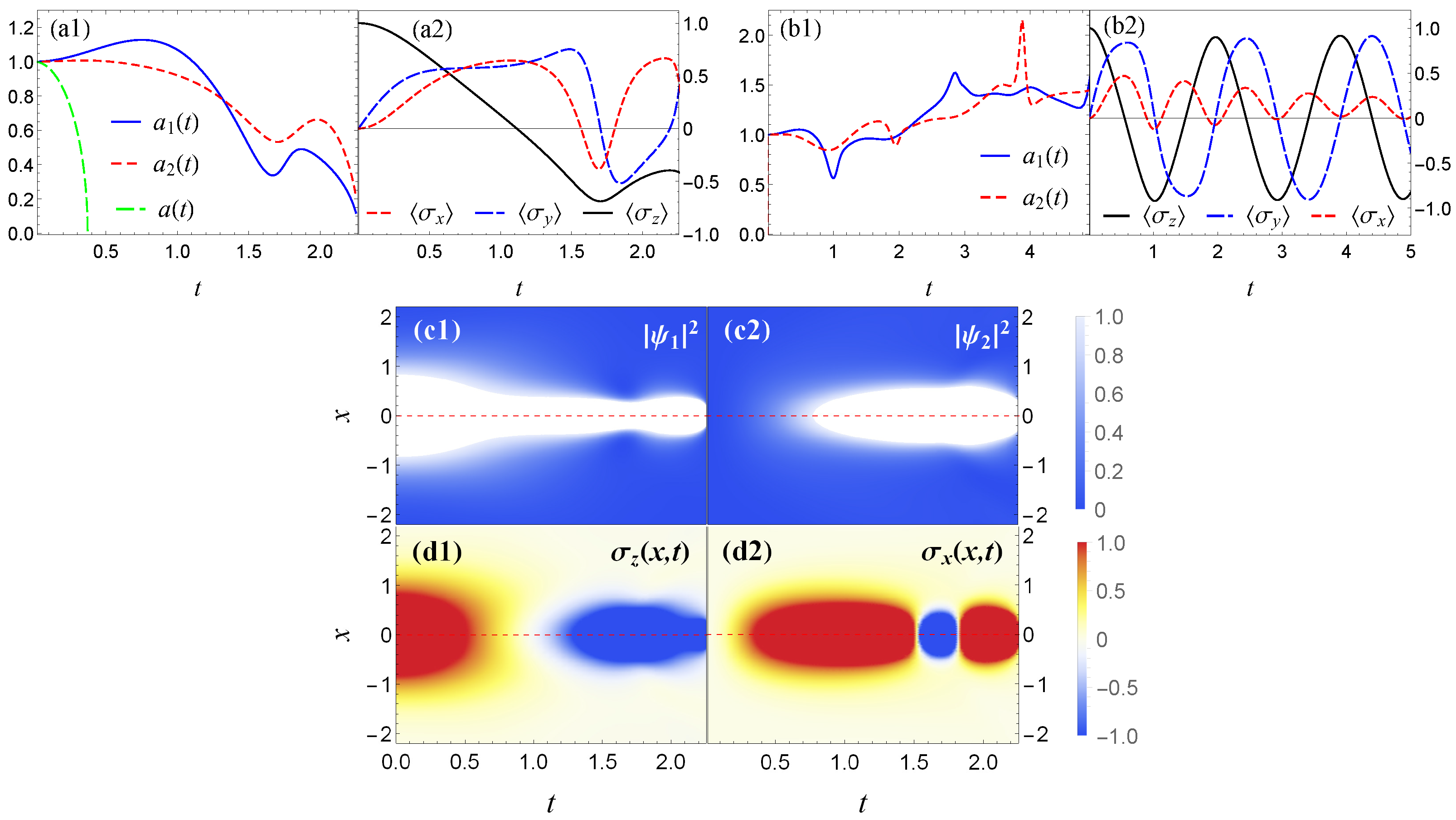
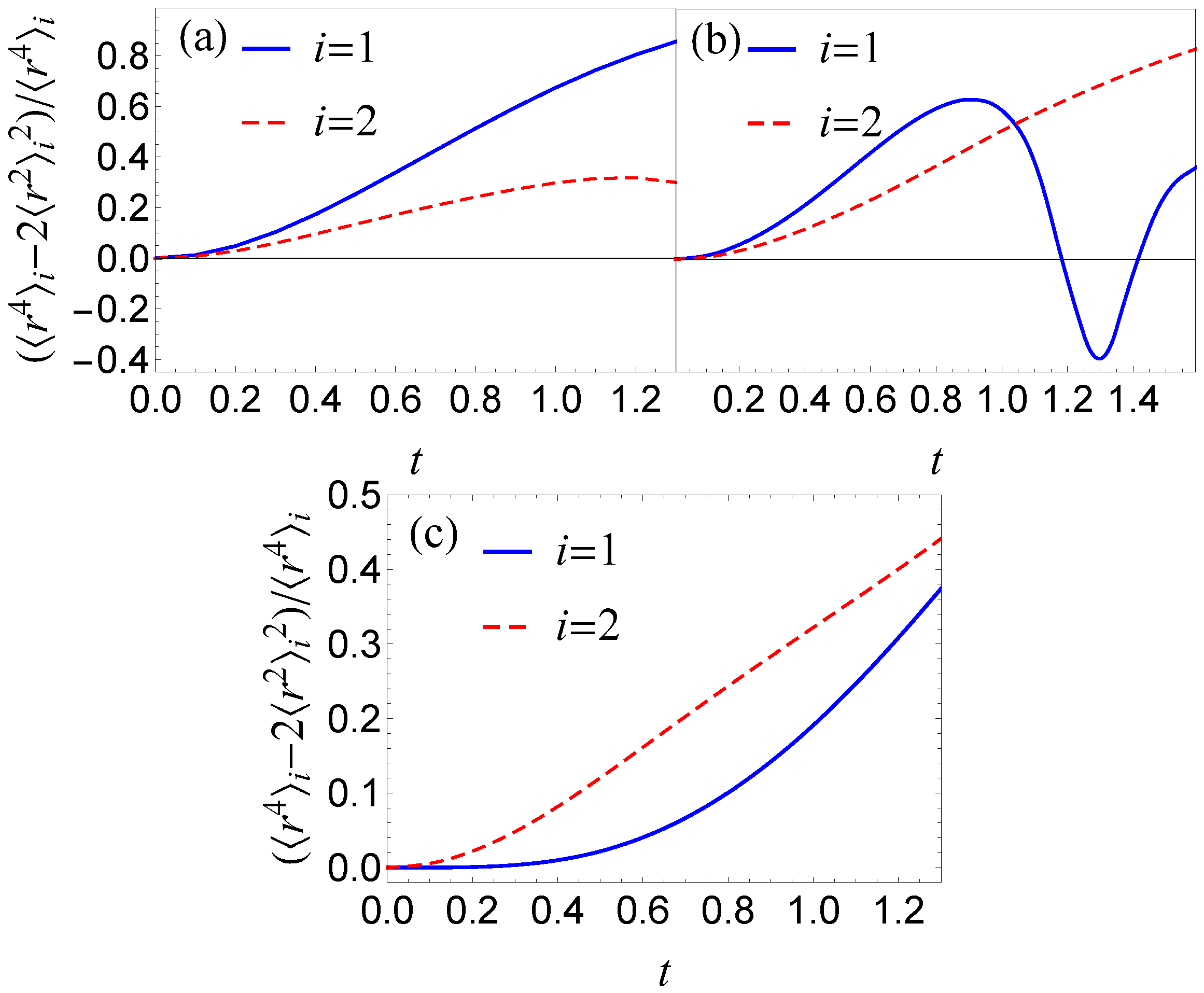
3.1. Intra-Spin Interaction
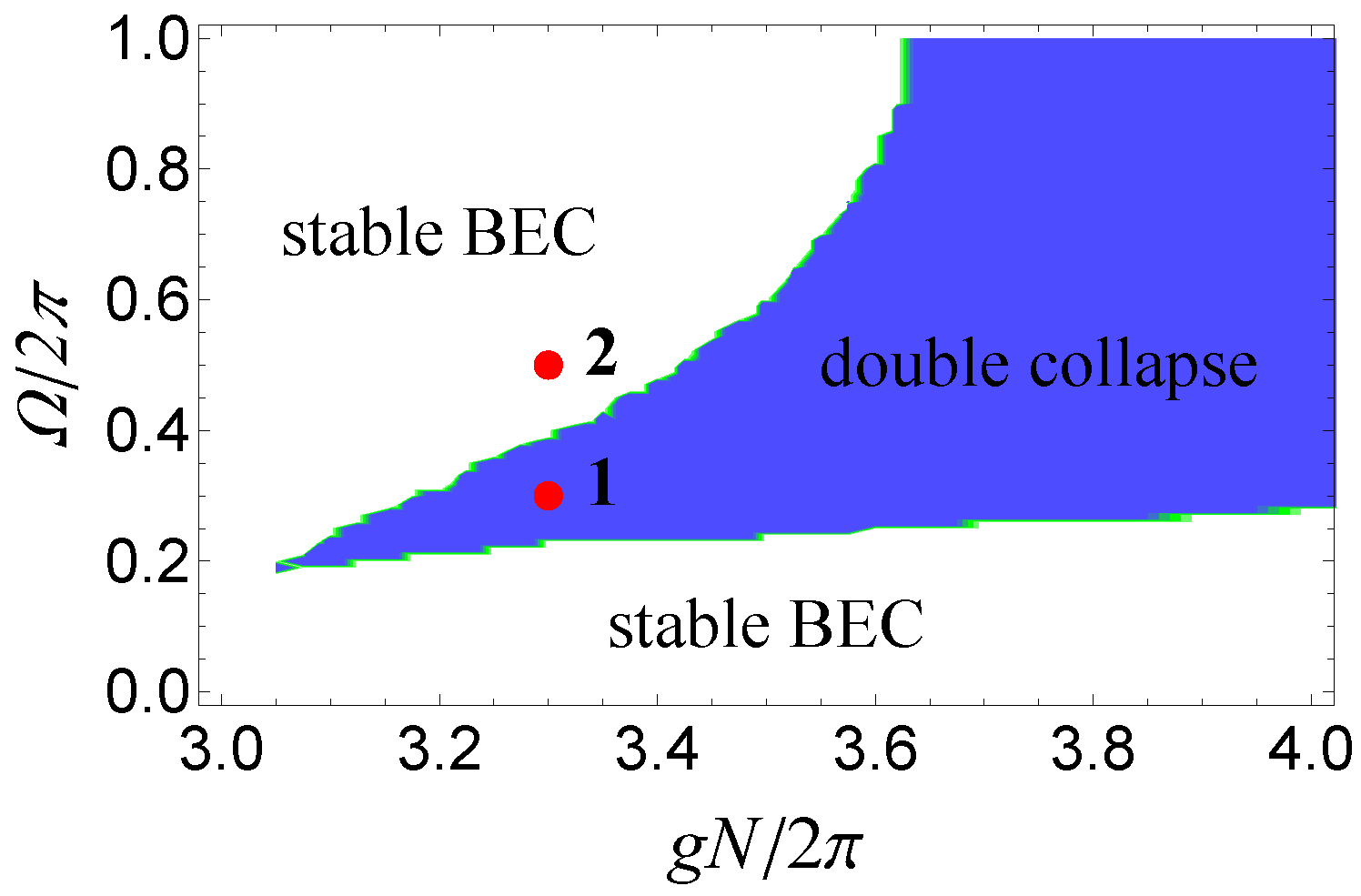
3.2. Cross-Spin Interaction
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BEC | Bose-Einstein condensate |
| GPE | Gross-Pitaevskii equation |
References
- Rajaraman, R. Solitons and Instantons: An Introduction to Solitons and Instantons in Quantum Field Theory; North-Holland Personal Library, North-Holland Publishing Company: Amsterdam, The Netherlands, 1982. [Google Scholar]
- Dalfovo, F.; Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of Bose-Einstein condensation in trapped gases. Rev. Mod. Phys. 1999, 71, 463. [Google Scholar] [CrossRef] [Green Version]
- Sikivie, P.; Yang, Q. Bose-Einstein Condensation of Dark Matter Axions. Phys. Rev. Lett. 2009, 103, 111301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chin, C.; Grimm, R.; Julienne, P.; Tiesinga, E. Feshbach resonances in ultracold gases. Rev. Mod. Phys. 2010, 82, 1225. [Google Scholar] [CrossRef]
- Giovanazzi, S.; Görlitz, A.; Pfau, T. Tuning the Dipolar Interaction in Quantum Gases. Phys. Rev. Lett. 2002, 89, 130401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kumar, R.K.; Tomio, L.; Gammal, A. Spatial separation of rotating binary Bose-Einstein condensates by tuning the dipolar interactions. Phys. Rev. A 2019, 99, 043606. [Google Scholar] [CrossRef] [Green Version]
- Kivshar, Y.S.; Malomed, B.A. Dynamics of solitons in nearly integrable systems. Rev. Mod. Phys. 1989, 61, 763. [Google Scholar] [CrossRef]
- Ferrier-Barbut, I.; Kadau, H.; Schmitt, M.; Wenzel, M.; Pfau, T. Observation of Quantum Droplets in a Strongly Dipolar Bose Gas. Phys. Rev. Lett. 2016, 116, 215301. [Google Scholar] [CrossRef]
- Semeghini, G.; Ferioli, G.; Masi, L.; Mazzinghi, C.; Wolswijk, L.; Minardi, F.; Modugno, M.; Modugno, G.; Inguscio, M.; Fattori, M. Self-Bound Quantum Droplets of Atomic Mixtures in Free Space. Phys. Rev. Lett. 2018, 120, 235301. [Google Scholar] [CrossRef] [Green Version]
- Bergé, L. Wave collapse in physics: Principles and applications to light and plasma waves. Phys. Rep. 1998, 303, 259. [Google Scholar] [CrossRef]
- Greiner, M.; Mandel, O.; Hänsch, T.W.; Bloch, I. Collapse and revival of the matter wave field of a Bose–Einstein condensate. Nature 2002, 419, 51. [Google Scholar] [CrossRef]
- Donley, E.A.; Claussen, N.R.; Cornish, S.L.; Roberts, J.L.; Cornell, E.A.; Wieman, C.E. Dynamics of collapsing and exploding Bose–Einstein condensates. Nature 2001, 412, 295. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sackett, C.A.; Stoof, H.T.C.; Hulet, R.G. Growth and Collapse of a Bose-Einstein Condensate with Attractive Interactions. Phys. Rev. Lett. 1998, 80, 2031. [Google Scholar] [CrossRef] [Green Version]
- Cornish, S.L.; Claussen, N.R.; Roberts, J.L.; Cornell, E.A.; Wieman, C.E. Stable 85Rb Bose-Einstein Condensates with Widely Tunable Interactions. Phys. Rev. Lett. 2000, 85, 1795. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mardonov, S.; Modugno, M.; Sherman, E.Y.; Malomed, B.A. Rabi-coupling-driven motion of a soliton in a Bose-Einstein condensate. Phys. Rev. A 2019, 99, 013611. [Google Scholar] [CrossRef] [Green Version]
- Abdullaev, F.K.; Salerno, M. Gap-Townes solitons and localized excitations in low-dimensional Bose-Einstein condensates in optical lattices. Phys. Rev. A 2005, 72, 033617. [Google Scholar] [CrossRef] [Green Version]
- Abdullaev, F.K.; Caputo, J.G.; Kraenkel, R.A.; Malomed, B.A. Controlling collapse in Bose-Einstein condensates by temporal modulation of the scattering length. Phys. Rev. A 2003, 67, 013605. [Google Scholar] [CrossRef] [Green Version]
- Kagan, Y.; Surkov, E.L.; Shlyapnikov, G.V. Evolution of a Bose-condensed gas under variations of the confining potential. Phys. Rev. A 1996, 54, R1753. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Petrov, D.S. Quantum Mechanical Stabilization of a Collapsing Bose-Bose Mixture. Phys. Rev. Lett. 2015, 115, 155302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yu, Z.F.; Zhang, A.X.; Tang, R.A.; Xu, H.P.; Gao, J.M.; Xue, J.K. Spin-orbit-coupling stabilization of a collapsing binary Bose-Einstein condensate. Phys. Rev. A 2017, 95, 033607. [Google Scholar] [CrossRef]
- Wang, L.X.; Dai, C.Q.; Wen, L.; Liu, T.; Jiang, H.F.; Saito, H.; Zhang, S.G.; Zhang, X.F. Dynamics of vortices followed by the collapse of ring dark solitons in a two-component Bose-Einstein condensate. Phys. Rev. A 2018, 97, 063607. [Google Scholar] [CrossRef]
- Kartashov, Y.V.; Sherman, E.Y.; Malomed, B.A.; Konotop, V.V. Stable two-dimensional soliton complexes in Bose–Einstein condensates with helicoidal spin–orbit coupling. New J. Phys. 2020, 22, 103014. [Google Scholar] [CrossRef]
- Zeng, L.; Zeng, J. Preventing critical collapse of higher-order solitons by tailoring unconventional optical diffraction and nonlinearities. Commun. Phys. 2020, 3, 26. [Google Scholar] [CrossRef]
- Sakaguchi, H.; Sherman, E.Y.; Malomed, B.A. Vortex solitons in two-dimensional spin-orbit coupled Bose-Einstein condensates: Effects of the Rashba-Dresselhaus coupling and Zeeman splitting. Phys. Rev. E 2016, 94, 032202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kartashov, Y.V.; Konotop, V.V.; Modugno, M.; Sherman, E.Y. Solitons in Inhomogeneous Gauge Potentials: Integrable and Nonintegrable Dynamics. Phys. Rev. Lett. 2019, 122, 064101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, Z.; Li, Y.; Malomed, B.A.; Salasnich, L. Spontaneous symmetry breaking of fundamental states, vortices, and dipoles in two- and one-dimensional linearly coupled traps with cubic self-attraction. Phys. Rev. A 2017, 96, 033621. [Google Scholar] [CrossRef] [Green Version]
- Mardonov, S.; Modugno, M.; Sherman, E.Y. Dynamics of a macroscopic spin qubit in spin–orbit coupled Bose–Einstein condensates. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 115302. [Google Scholar] [CrossRef]
- Mardonov, S.N. Collapse of quasi-two-dimensional symmetrized Dresselhaus spin-orbit coupled Bose–Einstein condensate. Arab. J. Math. 2021, 11. [Google Scholar] [CrossRef]
- Mardonov, S.; Sherman, E.Y.; Muga, J.G.; Wang, H.W.; Ban, Y.; Chen, X. Collapse of spin-orbit-coupled Bose-Einstein condensates. Phys. Rev. A 2015, 91, 043604. [Google Scholar] [CrossRef] [Green Version]
- Kartashov, Y.V.; Astrakharchik, G.E.; Malomed, B.A.; Torner, L. Frontiers in multidimensional self-trapping of nonlinear fields and matter. Nat. Rev. Phys. 2019, 1, 185. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mardonov, S.N.; Ahmedov, B.J. Rabi Frequency Management of Collapsing Quasi-Two-Dimensional Bose-Einstein Condensates with Pseudospin-1/2. Particles 2022, 5, 135-145. https://doi.org/10.3390/particles5020012
Mardonov SN, Ahmedov BJ. Rabi Frequency Management of Collapsing Quasi-Two-Dimensional Bose-Einstein Condensates with Pseudospin-1/2. Particles. 2022; 5(2):135-145. https://doi.org/10.3390/particles5020012
Chicago/Turabian StyleMardonov, Shukhrat N., and Bobomurat J. Ahmedov. 2022. "Rabi Frequency Management of Collapsing Quasi-Two-Dimensional Bose-Einstein Condensates with Pseudospin-1/2" Particles 5, no. 2: 135-145. https://doi.org/10.3390/particles5020012
APA StyleMardonov, S. N., & Ahmedov, B. J. (2022). Rabi Frequency Management of Collapsing Quasi-Two-Dimensional Bose-Einstein Condensates with Pseudospin-1/2. Particles, 5(2), 135-145. https://doi.org/10.3390/particles5020012






