Optimal and Quasi-Optimal Automatic Tuning of Vibration Neutralizers
Abstract
1. Introduction
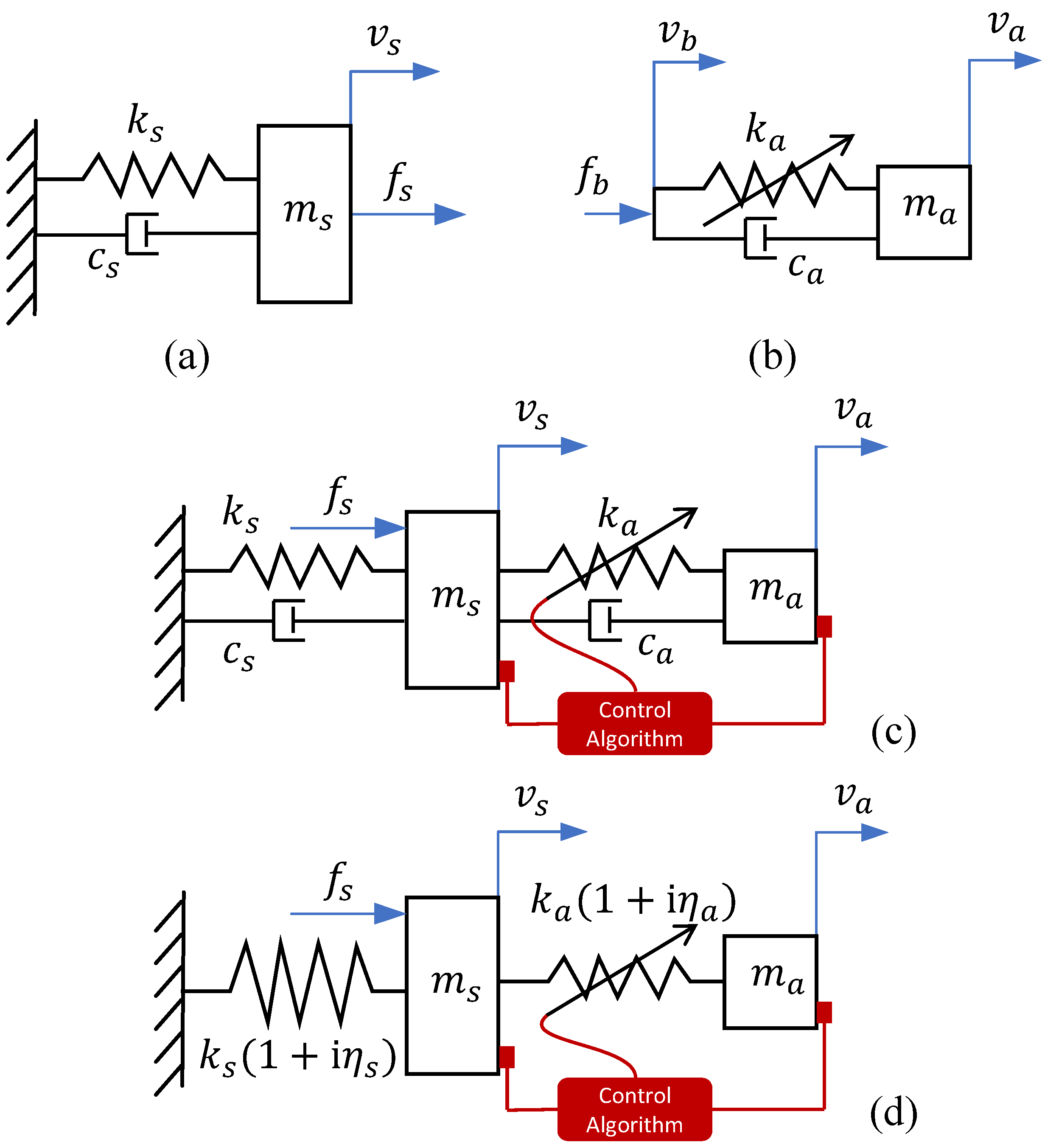
2. The Adaptive Tuned Vibration Neutralizer
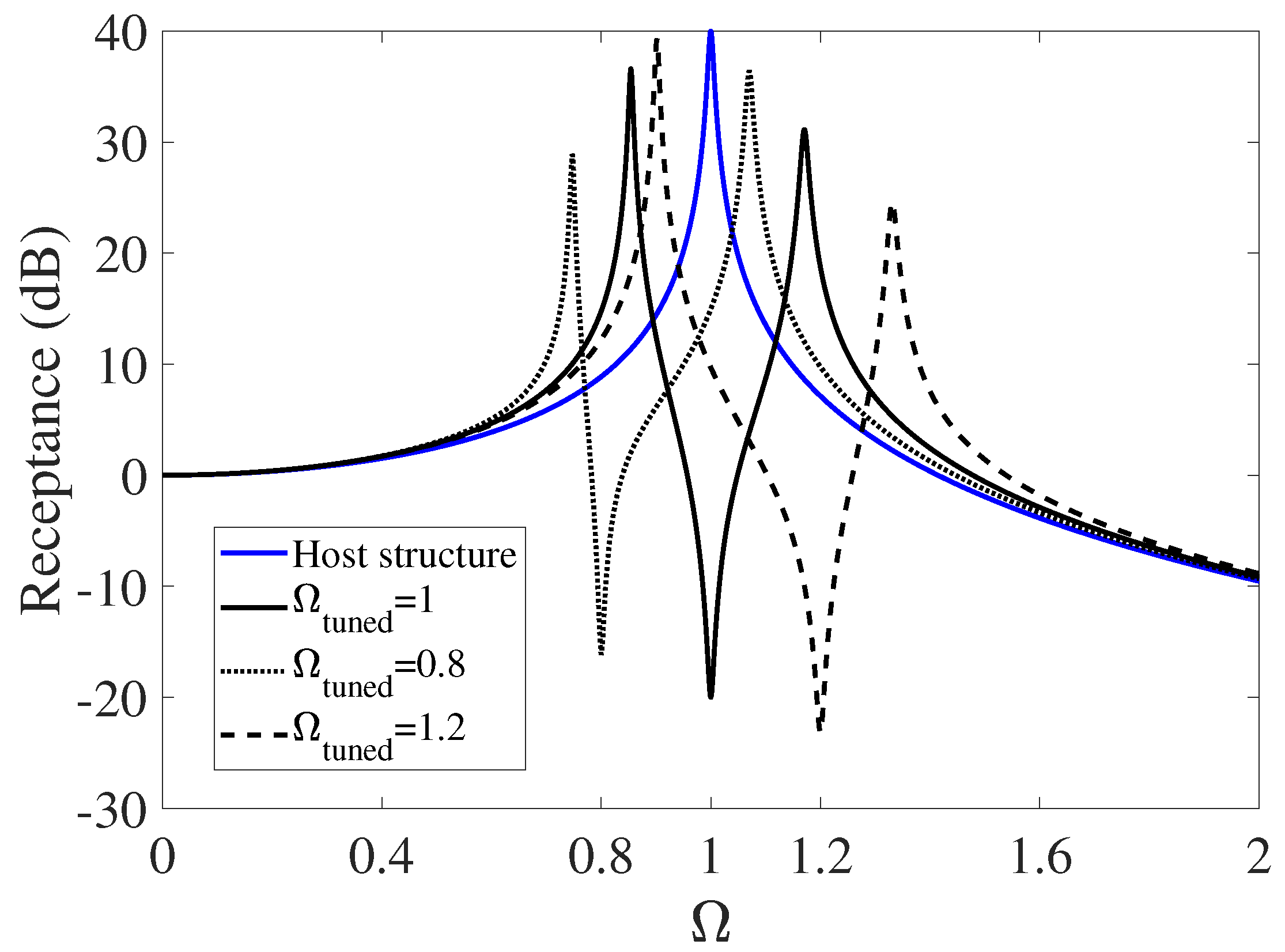
3. Optimal and Approximated Tuning of the ATVN
3.1. Viscous Damping
3.2. Hysteretic Damping
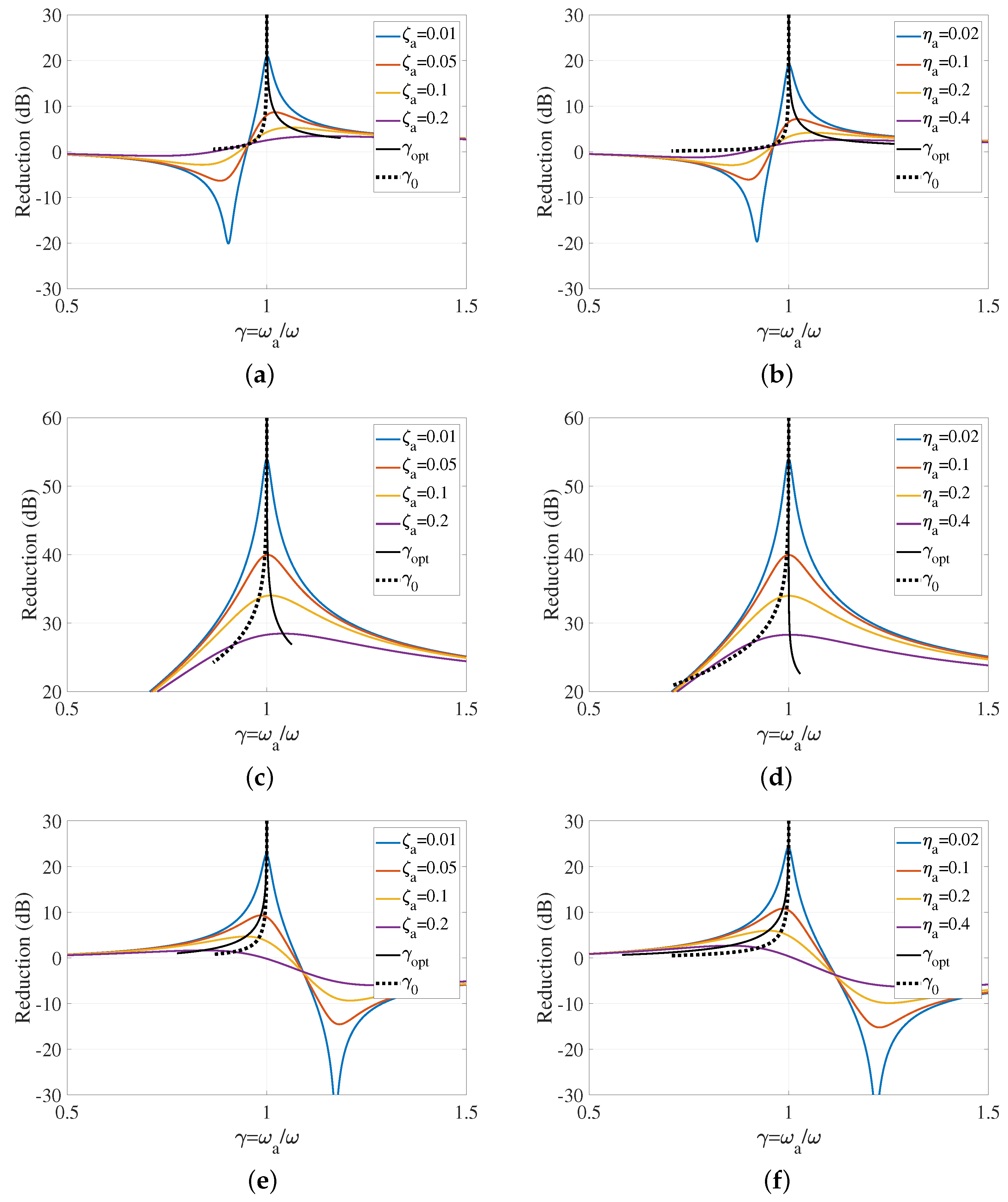
4. Quasi-Optimal Tuning of the ATVN
4.1. Viscous Damping
4.2. Structural Damping
5. Data Analysis and Discussion
6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ATVN | Adaptive Tuned Vibration Neutralizer |
References
- Bonello, P. Adaptive tuned vibration absorbers: Design principles, concepts and physical implementation. In Vibration Analysis and Control; Beltran-Carbajal, F., Ed.; IntechOpen: Rijeka, Croatia, 2011. [Google Scholar] [CrossRef]
- Franchek, M.A.; Ryan, M.W.; Bernhard, R.J. Adaptive passive vibration control. J. Sound Vib. 1996, 189, 565–585. [Google Scholar] [CrossRef]
- Bonello, P.; Groves, K.H. Vibration control using a beam-like adaptive tuned vibration absorber with an actuator-incorporated mass element. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2009, 223, 1555–1567. [Google Scholar] [CrossRef]
- Kidner, M.R.F.; Brennan, M.J. Varying the Stiffness of a Beam-Like Neutralizer Under Fuzzy Logic Control. J. Vib. Acoust. 2001, 124, 90–99. [Google Scholar] [CrossRef]
- Walsh, P.L.; Lamancusa, J.S. A variable stiffness vibration absorber for minimization of transient vibrations. J. Sound Vib. 1992, 158, 195–211. [Google Scholar] [CrossRef]
- Rustighi, E.; Brennan, M.J.; Mace, B.R. A shape memory alloy adaptive tuned vibration absorber: Design and implementation. Smart Mater. Struct. 2004, 14, 19–28. [Google Scholar] [CrossRef]
- Williams, K.A.; Chiu, G.T.-C.; Bernhard, R.J. Dynamic modelling of a shape memory alloy adaptive tuned vibration absorber. J. Sound Vib. 2005, 280, 211–234. [Google Scholar] [CrossRef]
- Carneal, J.P.; Charette, F.; Fuller, C.R. Minimization of sound radiation from plates using adaptive tuned vibration absorbers. J. Sound Vib. 2004, 270, 781–792. [Google Scholar] [CrossRef]
- Bonello, P.; Brennan, M.J.; Elliott, S.J. Vibration control using an adaptive tuned vibration absorber with a variable curvature stiffness element. Smart Mater. Struct. 2005, 14, 1055–1065. [Google Scholar] [CrossRef]
- Brennan, M.J.; Bonello, P.; Rustighi, E.; Mace, B.R.; Elliott, S.J. Designs of a variable stiffness element for a tunable vibration absorber. In Proceedings of the ICA2004 (The 18th International Congress on Acoustics), Kyoto, Japan, 4–9 April 2004; Volume IV, pp. 2915–2918. [Google Scholar]
- Fosdick, R.; Ketema, Y. A thermoviscoelastic dynamic vibration absorber. J. Appl. Mech. 1998, 65, 17–24. [Google Scholar] [CrossRef]
- Rustighi, E.; Beaugrand, M. A viscoelastic adaptive tuned vibration absorber. In Proceedings of the MoViC2014: The 12th International Conference on Motion and Vibration, Sapporo, Japan, 3–7 August 2014; p. 10069. [Google Scholar]
- Wang, Y.; Li, L.; Hofmann, D.; Andrade, J.E.; Daraio, C. Structured fabrics with tunable mechanical properties. Nature 2021, 596, 238–243. [Google Scholar] [CrossRef] [PubMed]
- Rustighi, E.; Gardonio, P.; Cignolini, N.; Baldini, S.; Malacarne, C.; Perini, M. Vibration response of tuneable structured fabrics. In Proceedings of the ISMA2022 Including USD2022, Leuven, Belgium, 12–14 September 2022; pp. 2429–2439. [Google Scholar]
- Davis, C.L.; Lesieutre, G.A. An Actively Tuned Solid-State Vibration Absorber Using Capacitive Shunting of Piezoelectric Stiffness. J. Sound Vib. 2000, 232, 601–617. [Google Scholar] [CrossRef]
- Morgan, R.A.; Wang, K.W. Active-Passive Piezoelectric Absorbers for Systems Under Multiple Non-Stationary Harmonic Excitations. J. Sound Vib. 2002, 255, 685–700. [Google Scholar] [CrossRef]
- Rustighi, E.; Brennan, M.J.; Mace, B.R. Real-time control of a shape memory alloy adaptive tuned vibration absorber. Smart Mater. Struct. 2005, 14, 1184–1195. [Google Scholar] [CrossRef]
- Williams, K.A.; Chiu, G.T.-C.; Bernhard, R.J. Nonlinear control of a shape memory alloy adaptive tuned vibration absorber. J. Sound Vib. 2005, 288, 1131–1155. [Google Scholar] [CrossRef]
- Brennan, M.J. Vibration control using a tunable vibration neutralizer. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1997, 211, 91–108. [Google Scholar] [CrossRef]
- Den Hartog, J.P. Mechanical Vibrations, 4th ed.; McGraw-Hill: New York, NY, USA, 1956. [Google Scholar]
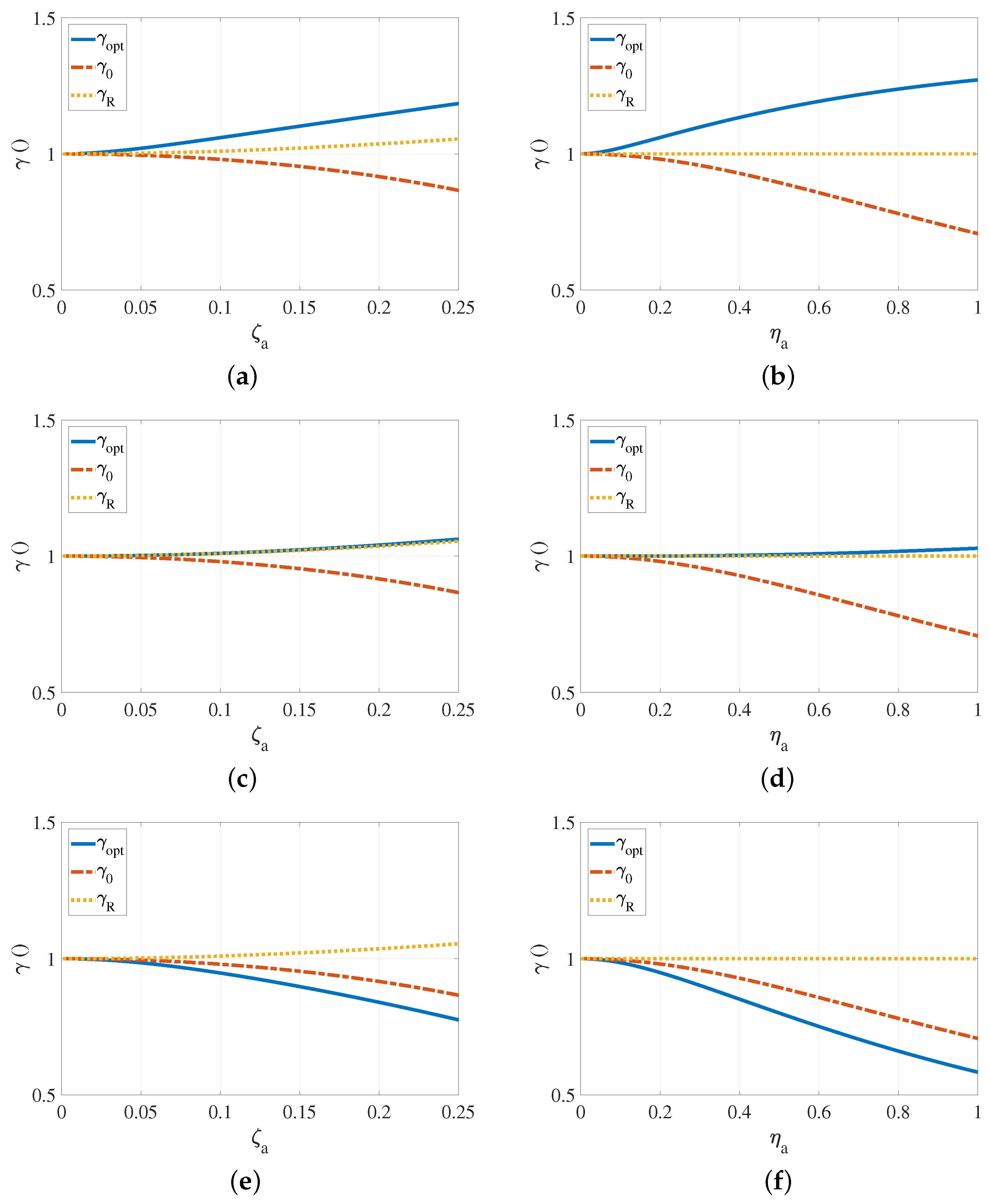

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rustighi, E. Optimal and Quasi-Optimal Automatic Tuning of Vibration Neutralizers. Vibration 2024, 7, 362-373. https://doi.org/10.3390/vibration7020018
Rustighi E. Optimal and Quasi-Optimal Automatic Tuning of Vibration Neutralizers. Vibration. 2024; 7(2):362-373. https://doi.org/10.3390/vibration7020018
Chicago/Turabian StyleRustighi, Emiliano. 2024. "Optimal and Quasi-Optimal Automatic Tuning of Vibration Neutralizers" Vibration 7, no. 2: 362-373. https://doi.org/10.3390/vibration7020018
APA StyleRustighi, E. (2024). Optimal and Quasi-Optimal Automatic Tuning of Vibration Neutralizers. Vibration, 7(2), 362-373. https://doi.org/10.3390/vibration7020018





