Fourier Series Approximation of Vertical Walking Force-Time History through Frequentist and Bayesian Inference
Abstract
1. Introduction
2. Extracting Fundamental Data
2.1. Experimental Data Collection
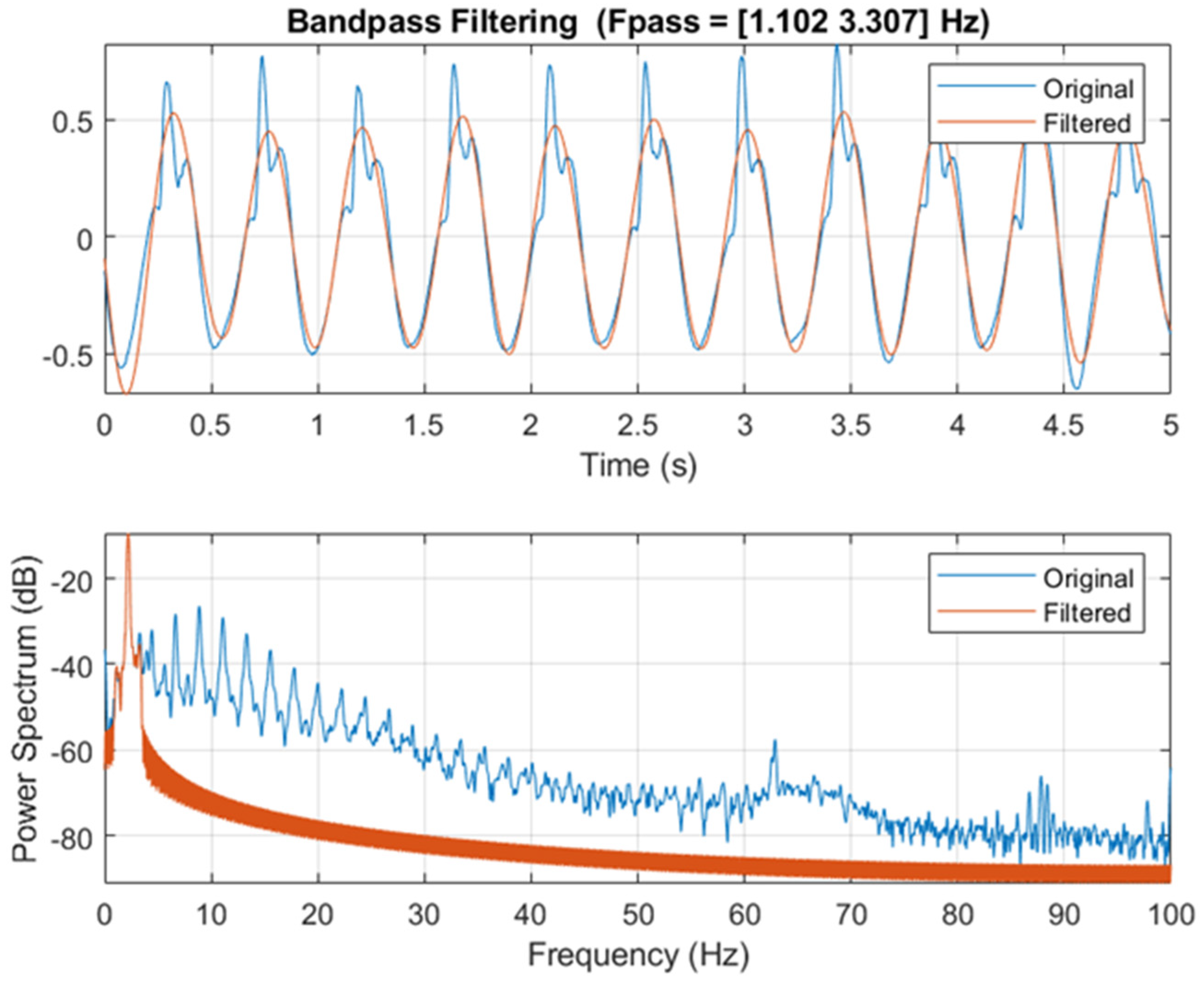
2.2. Data Pre-Processing
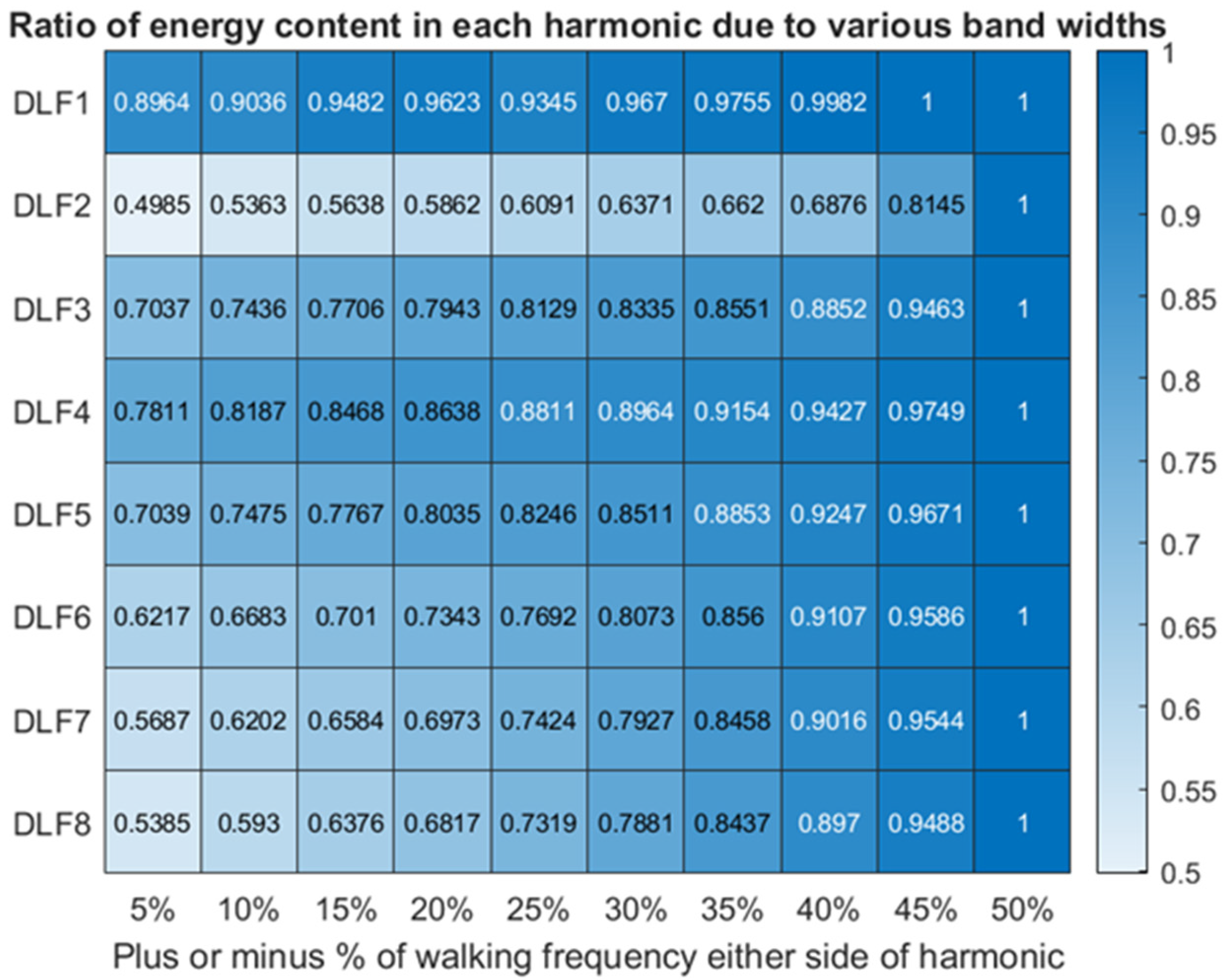
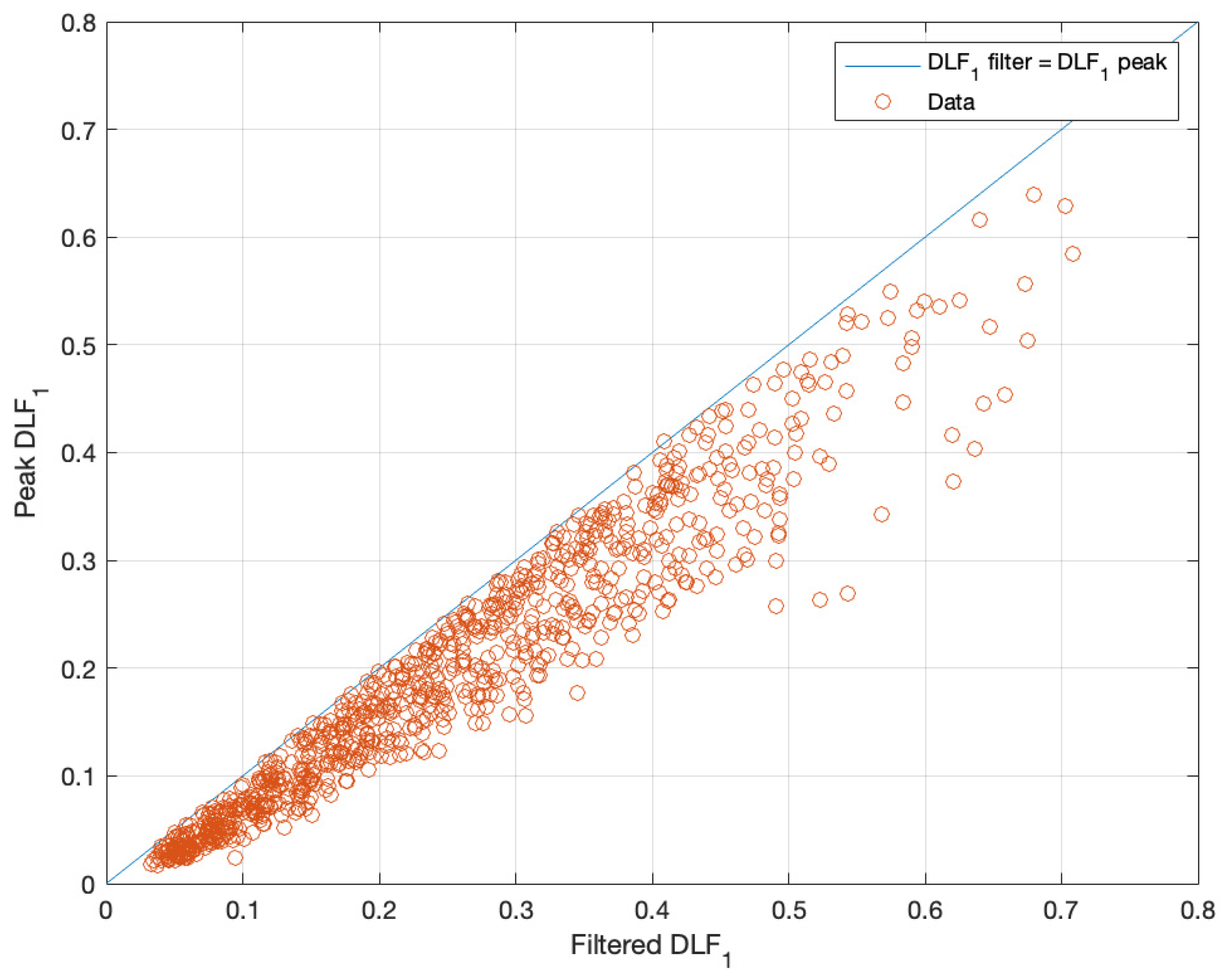
2.3. Extraction of DLFs and Walking Frequencies
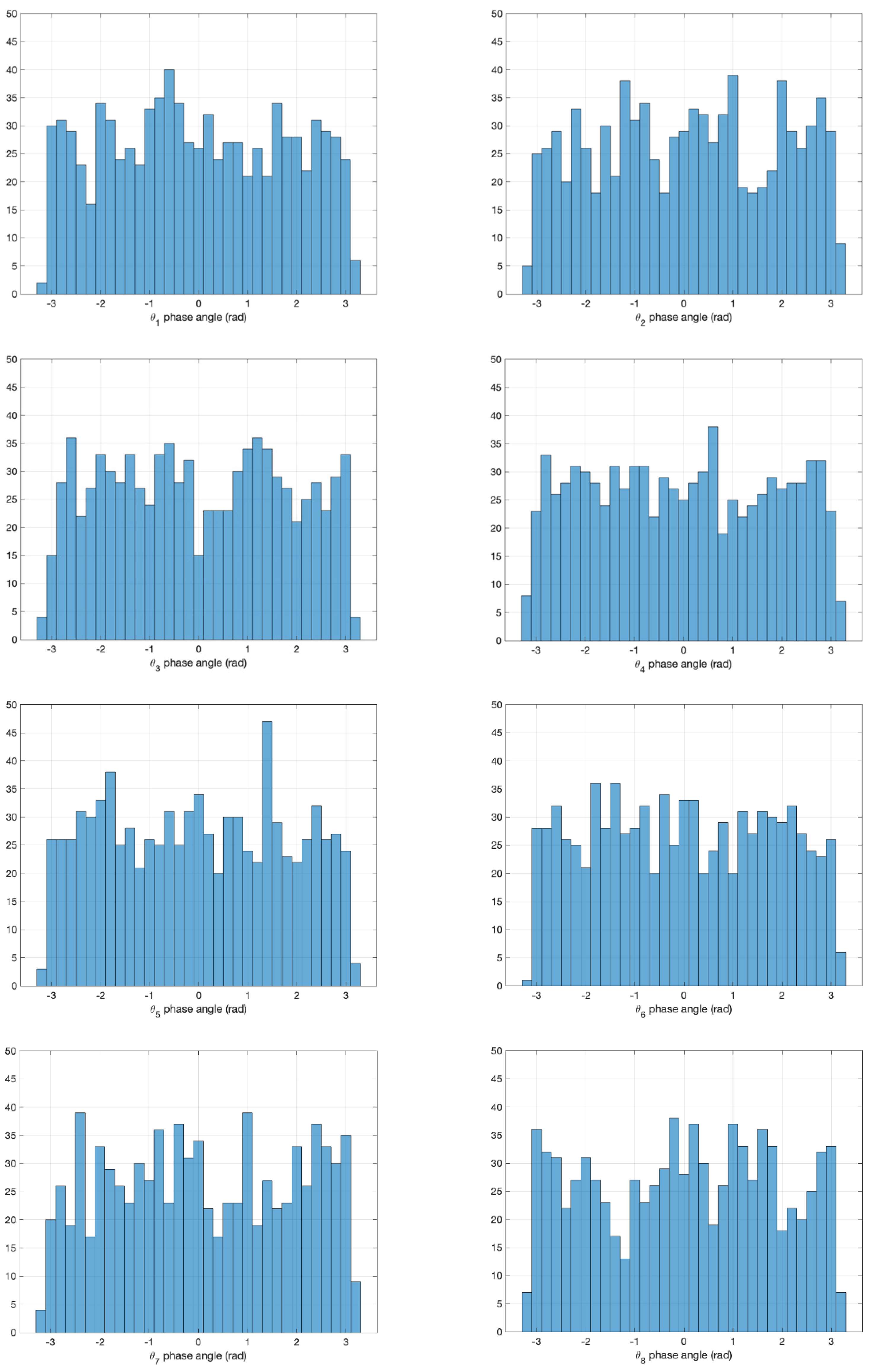
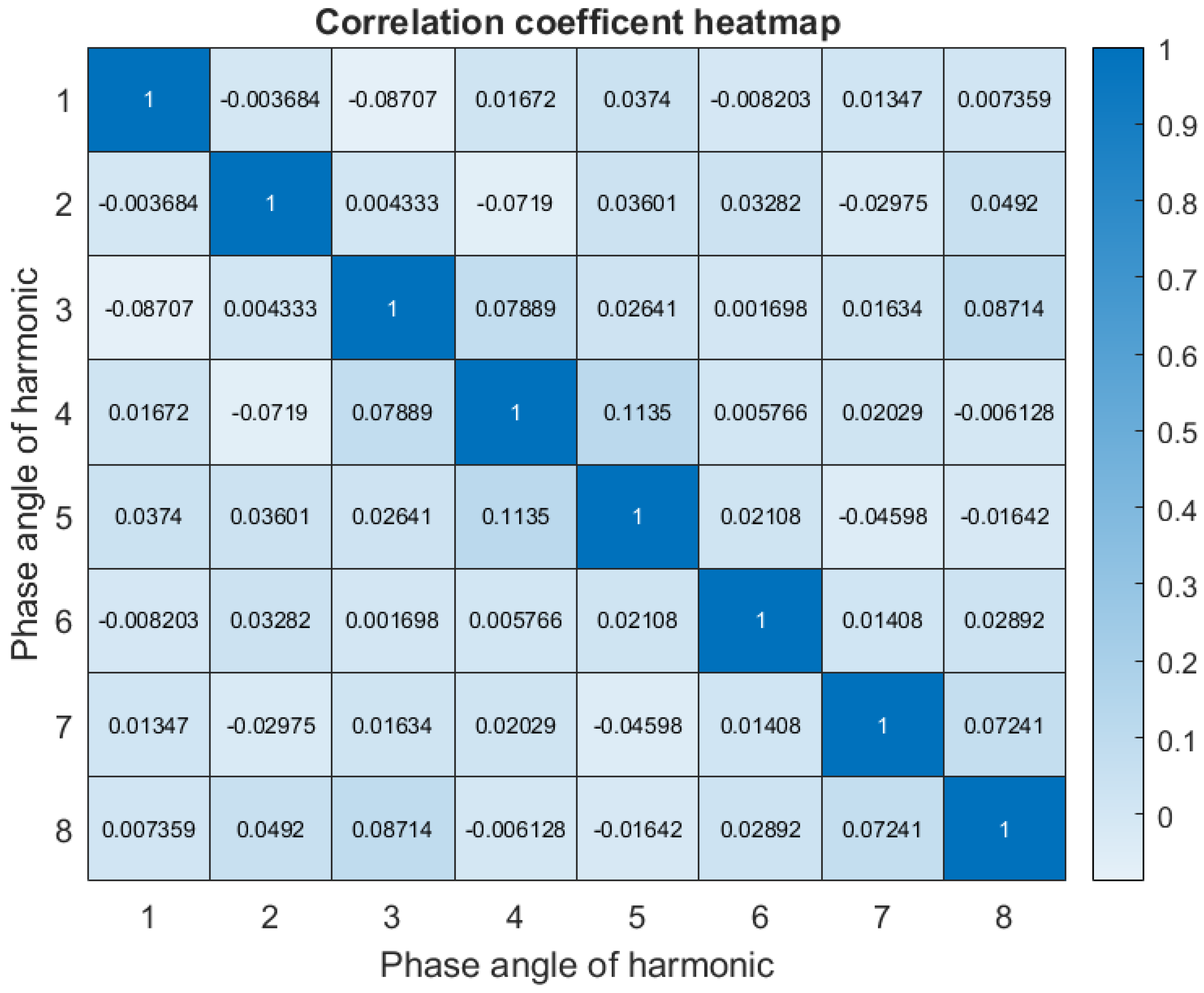
2.4. Phase Angles
3. Regression Inference of DLFs Given Walking Frequency
3.1. Frequentist Regression Model
- the regression models are represented through a linear combination of the parameters and error terms,
- errors are normally distributed,
- the error term has a condition mean of zero given the data,
- all independent terms are uncorrelated with the error term,
- observations of the error term are uncorrelated and non-auto-regressive,
- errors have constant variance, homoscedasticity.
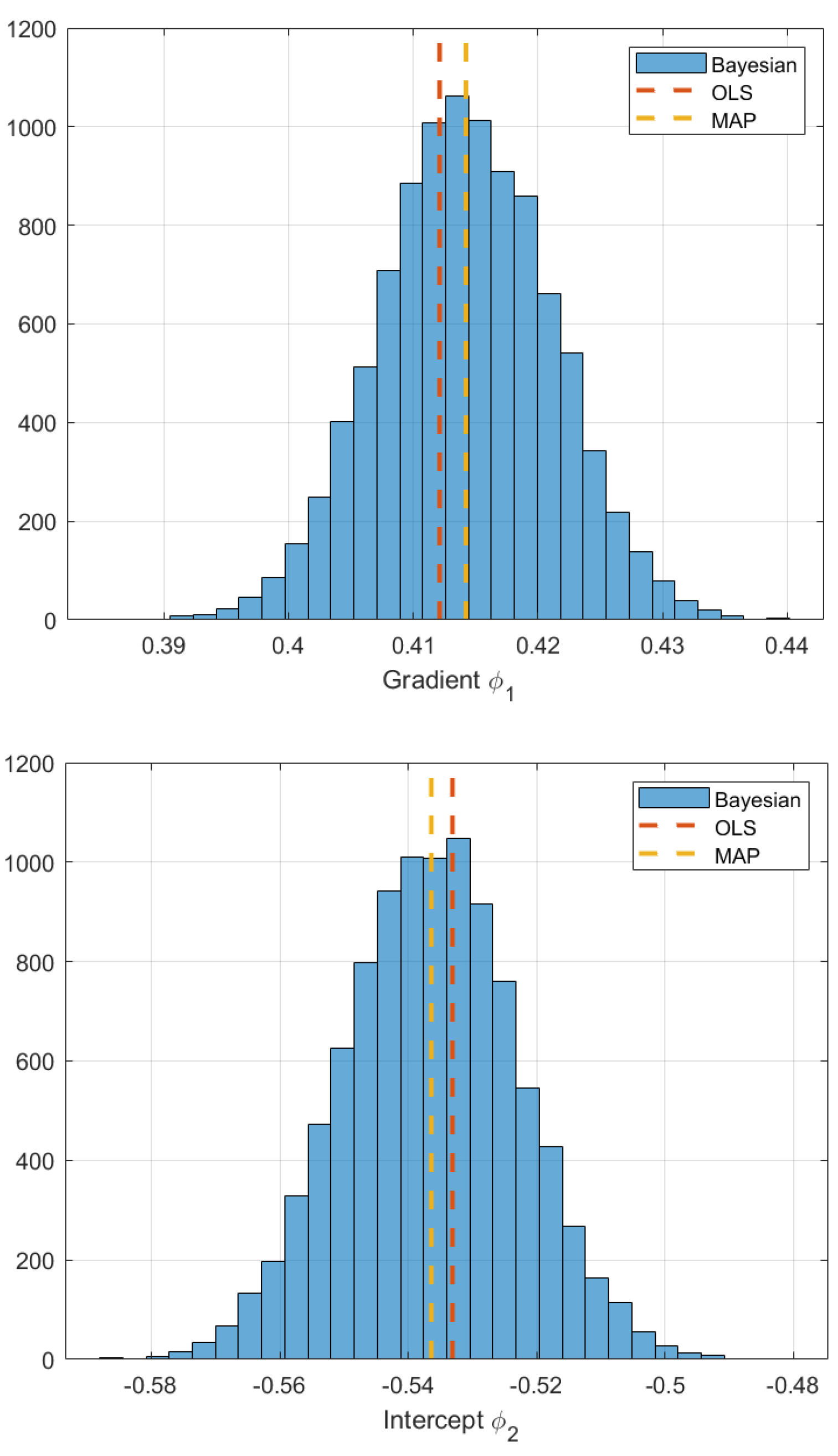
3.2. Bayesian Linear Regression Model
4. Discussion
4.1. Bayesian vs. Frequentist Approach to Fixed Parameter Values
4.2. Comparison of Proposed Models against the Current State of the Art Models
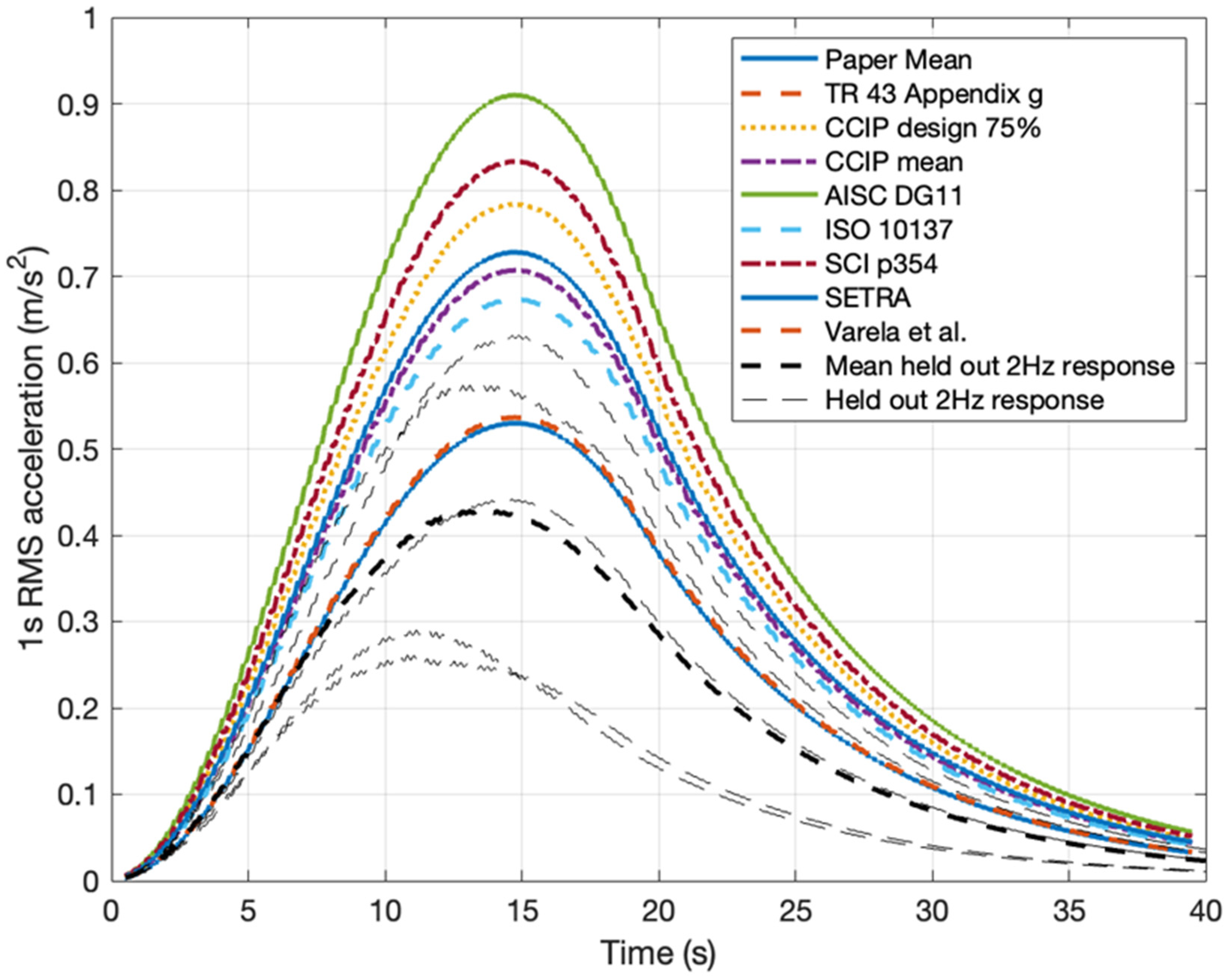
4.3. Comparison of Acceleration Response
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Živanović, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Fitzpatrick, T.; Dallard, P.; le Bourva, S.; Low, A.; Smith, R.; Willford, M. Linking London: The millennium bridge. R. Acad. Eng. 2001, 1, 1–28. [Google Scholar]
- Strogatz, S.; Abrams, D.; McRobie, A.; Eckhardt, B.; Ott, B. Crowd synchrony on the Millennium bridge. Nature 2005, 438, 43–44. [Google Scholar] [CrossRef]
- Newland, D.E. Vibration of the London Millennium Bridge: Cause and cure. Int. J. Acoust. Vib. 2003, 8, 9–14. [Google Scholar] [CrossRef]
- Ferdous, W.; Bai, Y.; Ngo, T.D.; Manalo, A.; Mendis, P. New advancements, challenges and opportunities of multi-storey modular buildings—A state-of-the-art review. Eng. Struct. 2019, 183, 883–893. [Google Scholar] [CrossRef]
- Pavic, A. Results of Istructe 2015 Survey of Practitioners on Vibration Serviceability IStructE Survey Questionnaire. SECED. 2019, pp. 1–8. Available online: https://ore.exeter.ac.uk/repository/handle/10871/120174 (accessed on 1 December 2022).
- Orr, J.; Drewniok, M.P.; Walker, I.; Ibell, T.; Copping, A.; Emmitt, S. Minimising energy in construction: Practitioners’ views on material efficiency. Resour. Conserv. Recycl. 2019, 140, 125–136. [Google Scholar] [CrossRef]
- Dunant, C.F.; Drewniok, M.P.; Eleftheriadis, S.; Cullen, J.M.; Allwood, J.M. Regularity and optimisation practice in steel structural frames in real design cases. Resour. Conserv. Recycl. 2018, 134, 294–302. [Google Scholar] [CrossRef]
- Kennedy, C. Innovative Thinker|Why Lean Design Creates Floor Vibration Challenge. New Civil Engineer. 2021. Available online: https://www.newcivilengineer.com/innovative-thinking/innovative-thinker-alex-pavic-on-why-lean-design-creates-floor-vibration-challenge-02-07-2021/ (accessed on 9 August 2021).
- Dunant, C.F.; Drewniok, M.P.; Orr, J.J.; Allwood, J.M. Good early stage design decisions can halve embodied CO2 and lower structural frames’ cost. Structures 2021, 33, 343–354. [Google Scholar] [CrossRef]
- Gonçalves, M.; Pavic, A. Environmental Impact of Structural Modifications in Office Floors to Satisfy Vibration Serviceability. In Proceedings of the EURODYN 2020 XI International Conference on Structural Dynamics, Athens, Greece, 23–26 November 2020; pp. 1924–1931. [Google Scholar] [CrossRef]
- Orr, J.; Cooke, M.; Ibell, T.; Smith, C.; Watson, N. Design for Zero; Institution of Structural Engineers: London, UK, 2021. [Google Scholar] [CrossRef]
- ISO 10137; Bases for Design of Structures-Serviceability of Buildings and Walkways against Vibrations. ISO: Geneva, Switzerland, 2007.
- Muhammad, Z.; Reynolds, P. Vibration Serviceability of Building Floors: Performance Evaluation of Contemporary Design Guidelines. J. Perform. Constr. Facil. 2019, 33, 1–17. [Google Scholar] [CrossRef]
- Muhammad, Z.; Reynolds, P.; Hudson, J. Evaluation of Contemporary Guidelines for Floor Vibration Serviceability Assessment. In Dynamics of Civil Structures, Volume 2, Proceedings of the 35th IMAC, A Conference and Exposition on Structural Dynamics 2017; Springer: Geneva, Switzerland, 2017; pp. 339–346. [Google Scholar]
- Muhammad, Z.; Reynolds, P.; Avci, O.; Hussein, M. Review of Pedestrian Load Models for Vibration Serviceability Assessment of Floor Structures. Vibration 2018, 2, 1–24. [Google Scholar] [CrossRef]
- Younis, A.; Avci, O.; Hussein, M.; Davis, B.; Reynolds, P. Dynamic Forces Induced by a Single Pedestrian: A Literature Review. Appl. Mech. Rev. 2017, 69, 020802. [Google Scholar] [CrossRef]
- Ohlsson, S.V. Ten years of floor vibration research—A review of aspects and some results. In Proceedings of the Symposium/Workshop on Serviceability of Buildings (Movements, Deformations, Vibrations); NRCC Institute for Research in Construction: New Orleans, LA, USA, 1988; pp. 419–434. [Google Scholar]
- Racic, V.; Morin, J.B. Data-driven modelling of vertical dynamic excitation of bridges induced by people running. Mech. Syst. Signal Process. 2014, 43, 153–170. [Google Scholar] [CrossRef]
- Racic, V.; Chen, J.; Pavic, A. Advanced fourier-based model of bouncing loads. In Dynamics of Civil Structures; Conference Proceedings of the Society for Experimental Mechanics Series; Springer: Geneva, Switzerland, 2019; pp. 367–376. [Google Scholar] [CrossRef]
- Whittle, M. Gait Analysis an Introduction, 4th ed.; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Murray, T.M.; Allen, D.E.; Ungar, E.E. Floor Vibrations due to Human Activity AISC DG 11; AISC American Institute of Steel Construction: Chicago, IL, USA, 2016. [Google Scholar]
- Pavic, A.; Willford, M. Technical Report 43 Post-tensioned floors Design Handbook—Appendix G, 2nd ed.; Concrete Society: Cinderford, UK, 2008. [Google Scholar]
- Willford, M.R.; Young, P. A Design Guide for Footfall Induced Vibration of Structures. The Concrete Society. 2006. Available online: www.concretecentre.com (accessed on 1 December 2022).
- Sétra (Services d’Études Techniques, des Routes et Autoroutes). Footbridges—Assessment of Vibrational Behavior of Footbridges under Pedestrian Loading; Ministére des Transports de l’Équipement du Tourisme et de la Mer: Paris, France, 2006. [Google Scholar]
- BSI. BS EN 1990 Eurocode: Basis of Structural Design; British Standards Institute: London, UK, 2002. [Google Scholar]
- Brownjohn, J.; Racic, V.; Chen, J. Universal response spectrum procedure for predicting walking-induced floor vibration. Mech. Syst. Signal Process. 2016, 70–71, 741–755. [Google Scholar] [CrossRef]
- Van Nimmen, K.; Broeck, P.V.D.; Lombaert, G.; Tubino, F. Pedestrian-Induced Vibrations of Footbridges: An Extended Spectral Approach. J. Bridg. Eng. 2020, 25, 04020058. [Google Scholar] [CrossRef]
- Racic, V.; Brownjohn, J.M.W. Stochastic model of near-periodic vertical loads due to humans walking. Adv. Eng. Informatics 2011, 25, 259–275. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A. Probabilistic Modeling of Walking Excitation for Building Floors. J. Perform. Constr. Facil. 2009, 23, 132–143. [Google Scholar] [CrossRef]
- Smith, A.L.; Hicks, S.J.; Devine, P.J. Design of Floors for Vibration: A New Approach; Steel Construction Institute Ascot: Berkshire, UK, 2007. [Google Scholar]
- Murray, T.; Allen, D.; Ungar, E. Floor Vibrations Due to Human Activity—DG-11 (10M797); American Institute of Steel Construction: Chicago, IL, USA, 2001. [Google Scholar]
- García-Diéguez, M.; Zapico-Valle, J.L. Statistical Modeling of the Relationships between Spatiotemporal Parameters of Human Walking and Their Variability. J. Struct. Eng. 2017, 143, 04017164. [Google Scholar] [CrossRef]
- Mohammed, A.; Pavic, A.; Racic, V. Improved model for human induced vibrations of high-frequency floors. Eng. Struct. 2018, 168, 950–966. [Google Scholar] [CrossRef]
- Kerr, S.C. Human Induced Loading on Staircases; University College London: London, UK, 1998; Available online: http://discovery.ucl.ac.uk/1318004/ (accessed on 1 December 2022).
- Muhammad, Z.O.; Reynolds, P. Probabilistic Multiple Pedestrian Walking Force Model including Pedestrian Inter- and Intrasubject Variabilities. Adv. Civ. Eng. 2020, 2020, 1–14. [Google Scholar] [CrossRef]
- Racic, V.; Pavic, A.; Brownjohn, J.M.W. Experimental identification and analytical modelling of human walking forces: Literature review. J. Sound Vib. 2009, 326, 1–49. [Google Scholar] [CrossRef]
- Brownjohn, J.; Middleton, C. Procedures for vibration serviceability assessment of high-frequency floors. Eng. Struct. 2008, 30, 1548–1559. [Google Scholar] [CrossRef]
- Liu, D. Vibration of Steel-Framed Floors Supporting Sensitive Equipment in Hospitals, Research Facilities, and Manufacturing Facilities. Ph.D. Thesis, University of Kentucky, Lexington, KY, USA, 2015. [Google Scholar] [CrossRef]
- Wang, J.; Chen, J. A comparative study on different walking load models. Struct. Eng. Mech. 2017, 63, 847–856. [Google Scholar] [CrossRef]
- Blanchard, J.; Davies, B.; Smith, W. Design criteria and analysis for dynamic loading of footbridges. In Proceedings of the a Symposium on Dynamic Behaviour of Bridges at the Transport and Road Research Laboratory, London, UK, 19 May 1977; pp. 90–106. [Google Scholar]
- Chen, J.; Ding, G.; Živanović, S. Stochastic Single Footfall Trace Model for Pedestrian Walking Load. Int. J. Struct. Stab. Dyn. 2019, 19, 1950029. [Google Scholar] [CrossRef]
- Chen, J.; Wang, J.; Brownjohn, J.M.W. Power Spectral-Density Model for Pedestrian Walking Load. J. Struct. Eng. 2019, 145, 05018014. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, B.; Wei, J.; Luo, P.; Cui, C. A Stochastic Study on the Distribution Parameters of Random Individual Walking Excitations. In AEI 2017: Resilience of the Integrated Building; American Society of Civil Engineers: Reston, VA, USA, 2017; pp. 495–505. [Google Scholar]
- García-Diéguez, M.; Racic, V.; Zapico-Valle, J. Complete statistical approach to modelling variable pedestrian forces induced on rigid surfaces. Mech. Syst. Signal Process. 2021, 159, 107800. [Google Scholar] [CrossRef]
- Ahmadi, E.; Caprani, C.; Živanović, S.; Heidarpour, A. Vertical ground reaction forces on rigid and vibrating surfaces for vibration serviceability assessment of structures. Eng. Struct. 2018, 172, 723–738. [Google Scholar] [CrossRef]
- Semaan, M.B.; Wallard, L.; Ruiz, V.; Gillet, C.; Leteneur, S.; Simoneau-Buessinger, E. Is treadmill walking biomechanically comparable to overground walking? A systematic review. Gait Posture 2022, 92, 249–257. [Google Scholar] [CrossRef]
- Toso, M.A.; Gomes, H.M.; da Silva, F.T.; Pimentel, R.L. Experimentally fitted biodynamic models for pedestrian–structure interaction in walking situations. Mech. Syst. Signal Process. 2016, 72–73, 590–606. [Google Scholar] [CrossRef]
- Bachmann, H.; Ammann, W. Vibrations in Structures: Induced by Man and Machines; IABSE: Zurich, Switzerland, 1987. [Google Scholar]
- Rainer, J.H.; Pernica, G.; Allen, D.E. Dynamic loading and response of footbridges. Can. J. Civ. Eng. 1988, 15, 66–71. [Google Scholar] [CrossRef]
- Petersen, C.; Werkle, H. Dynamik der Baukonstruktionen (Dynamics of Building Constructions); Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- BS 5400; Steel, Concrete and Composite Bridges—Part 2: Specification for Loads; Appendix C: Vibration Serviceability Requirements for Foot and Cycle Track Bridges. British Standards Association: British, UK, 1978.
- Ellis, B. On the response of long-span floors to walking loads generated by individuals and crowds. Struct. Eng. 2000, 78, 17–25. [Google Scholar]
- Architectural Institute of Japan. AIJ Recommendations for Loads on Buildings, Japan. 2004. [Google Scholar]
- Brownjohn, J.M.; Pavic, A.; Omenzetter, P. A spectral density approach for modelling continuous vertical forces on pedestrian structures due to walking. Can. J. Civ. Eng. 2004, 31, 65–77. [Google Scholar] [CrossRef]
- Willford, M. An investigation into crowd-induced vertical dynamic loads using available measurements. Struct. Eng. 2001, 79, 21–25. [Google Scholar]
- Živanović, S. Probability-Based Estimation of Vibration for Pedestrian Structures due to Walking; University of Sheffield: Sheffield, UK, 2006. [Google Scholar]
- Živanović, S.; Pavić, A.; Reynolds, P. Probability-based prediction of multi-mode vibration response to walking excitation. Eng. Struct. 2007, 29, 942–954. [Google Scholar] [CrossRef]
- Nguyen, H.A.U. Walking Induced Floor Vibration Design and Control. Ph.D. Thesis, Swinburne University of Technology, Hawthorn, Australia, 2013; p. 340. [Google Scholar]
- Chen, J.; Xu, R.; Zhang, M. Acceleration response spectrum for predicting floor vibration due to occupant walking. J. Sound. Vib. 2014, 333, 3564–3579. [Google Scholar] [CrossRef]
- Varela, W.D.; Pfeil, M.S.; de Paula A. da Costa, N. Experimental Investigation on Human Walking Loading Parameters and Biodynamic Model. J. Vib. Eng. Technol. 2020, 8, 883–892. [Google Scholar] [CrossRef]
- Butz, C. A Probabilistic Engineering Load Model for Pedestrian Streams. In Proceedings of the Third International Conference Footbridge, Porto, Portugal, 2–4 July 2008. [Google Scholar]
- Venuti, F.; Racic, V.; Corbetta, A. Modelling framework for dynamic interaction between multiple pedestrians and vertical vibrations of footbridges. J. Sound Vib. 2016, 379, 245–263. [Google Scholar] [CrossRef]
- Mohammed, A.; Pavic, A. Simulation of people’s movements on floors using social force model. In Dynamics of Civil Structures; Springer: Cham, Switzerland, 2019; Volume 2, pp. 39–46. [Google Scholar] [CrossRef]
- Helbing, D.; Molnár, P. Social force model for pedestrian dynamics. Phys. Rev. E 1995, 51, 4282–4286. [Google Scholar] [CrossRef]
- Feldmann, M.; Heinemeyer, C.; Butz, C.; Caetano, E.; Cunha, A.; Galanti, F.; Goldack, A.; Hechler, O.; Hicks, S.; Keil, A.; et al. Design of Floor Structures for Human Induced Vibrations Prepared under the JRC-ECCS Cooperation Agreement for the Evolution of Eurocode 3 (programme of CEN/TC 250) Design of Floor Structures for Human Induced Vibrations; Office for Official Publ. of the European Communities: Luxembourg, 2009. [Google Scholar]
- H.M. Development, User Manuals, 2009.
- Bishop, C. Pattern Recognition and Machine Learning; Springer: Cambridge, UK, 2006. [Google Scholar]
- Zivanovic, S.; Pavic, A. Probabilistic approach to subjective assessment of footbridge vibration. In Proceedings of the 42rd UK Conference on Human Responses to Vibration, Southampton, UK, 9–11 September 2007. [Google Scholar]
- Murphey, K.P. Machine Learning: A Probabilistic Perspective; MIT Press: Cambridge, MA, USA, 2012. [Google Scholar] [CrossRef]
- Snoek, J.; Larochelle, H.; Adams, R.P. Practical Bayesian optimization of machine learning algorithms. Adv. Neural. Inf. Process. Syst. 2012, 4, 2951–2959. [Google Scholar]
- Matlab. Kolmogorov-Smirnov Test for Normality, Matlab. 2021. Available online: https://uk.mathworks.com/help/stats/kstest.html (accessed on 3 September 2021).
- Strutz, T. Data fitting and uncertainty. In A Practical Introduction to Weighted Least Squares and Beyond; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Engle, R.F. Autoregressive Conditional Heteroscedasticity with Estimates of the Variance of United Kingdom Inflation. Econometrica 1982, 50, 987–1007. [Google Scholar] [CrossRef]
- Mathworks. Least Squares Fitting. 2021. Available online: https://uk.mathworks.com/help/curvefit/least-squares-fitting.html (accessed on 5 November 2021).
- Bayes, T. An essay towards solving a problem in the doctrine of chances, Philosophical Transactions of the Royal Society A: Mathematical. Phys. Eng. Sci. 1763, 53, 370–418. [Google Scholar]
- Van de Schoot, R.; Depaoli, S.; King, R.; Kramer, B.; Märtens, K.; Tadesse, M.G.; Vannucci, M.; Gelman, A.; Veen, D.; Willemsen, J.; et al. Bayesian statistics and modelling. Nat. Rev. Methods Prim. 2021, 1, 1–23. [Google Scholar] [CrossRef]
- Gonçalves, M.; Pavic, A.; Pimentel, R. Vibration serviceability assessment of office floors for realistic walking and floor layout scenarios: Literature review. Adv. Struct. Eng. 2020, 23, 1238–1255. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Nishioka, T.; Shiojiri, H.; Matsuzaki, K. Dynamic design of footbridges. IABSE Proc. 1978, 17, 1–15. [Google Scholar] [CrossRef]
- Pachi, A.; Ji, T. Frequency and velocity of people walking. Struct. Eng. 2005, 83, 36–40. [Google Scholar]
- Dang, H.V.; Živanović, S. Experimental characterisation of walking locomotion on rigid level surfaces using motion capture system. Eng. Struct. 2015, 91, 141–154. [Google Scholar] [CrossRef]
- Shahabpoor, E.; Pavic, A.; Racic, V. Structural vibration serviceability: New design framework featuring hu-man-structure interaction. Eng Struct. 2017, 136, 295–311. [Google Scholar] [CrossRef]
- Van Nimmen, K.; Pavic, A.; van den Broeck, P. A simplified method to account for vertical human-structure interaction. Structures 2021, 32, 2004–2019. [Google Scholar] [CrossRef]
- Ahmadi, E.; Caprani, C.; Zivanovic, S.; Heidarpour, A. Assessment of human-structure interaction on a lively light-weight GFRP footbridge. Eng Struct. 2019, 199, 109687. [Google Scholar] [CrossRef]
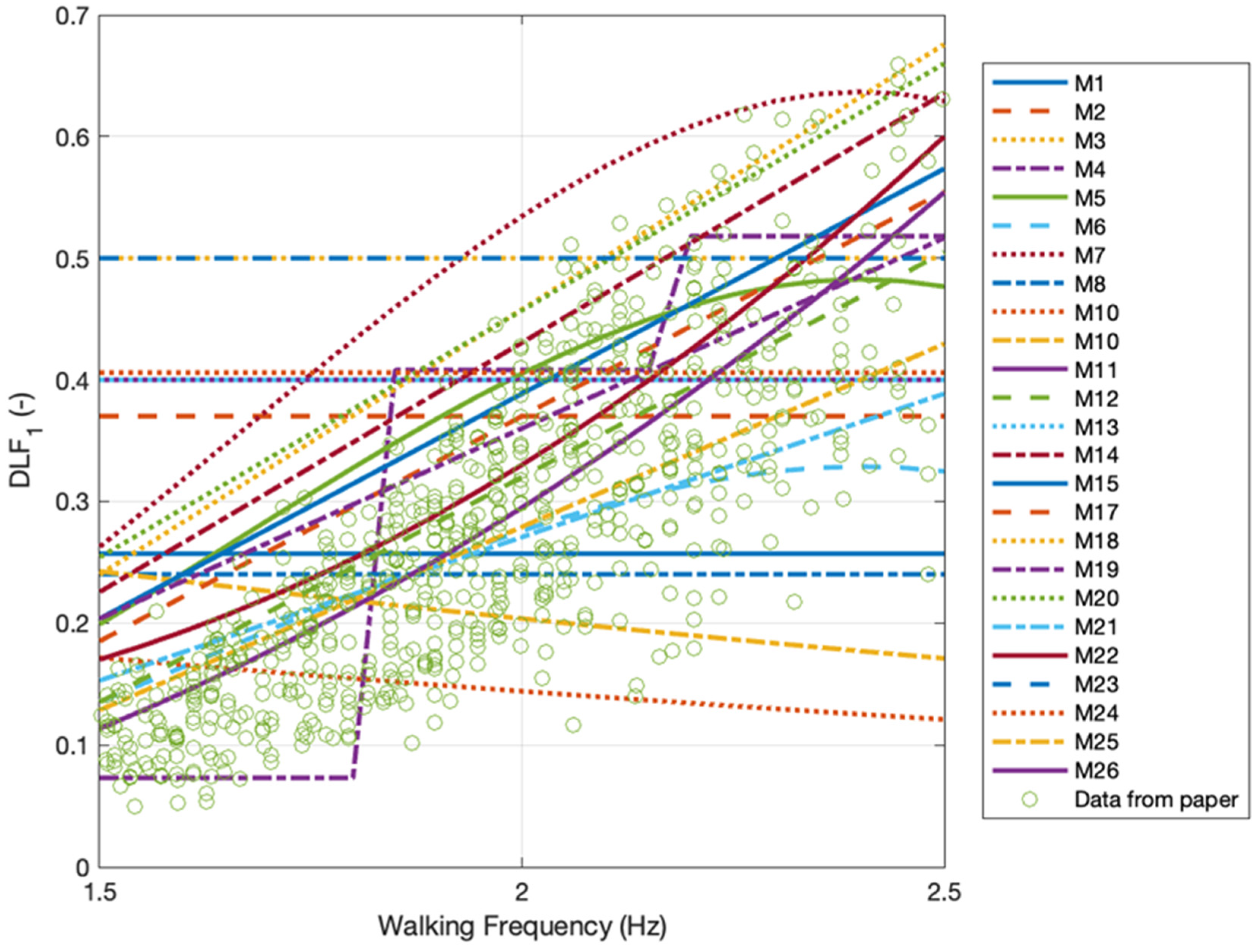
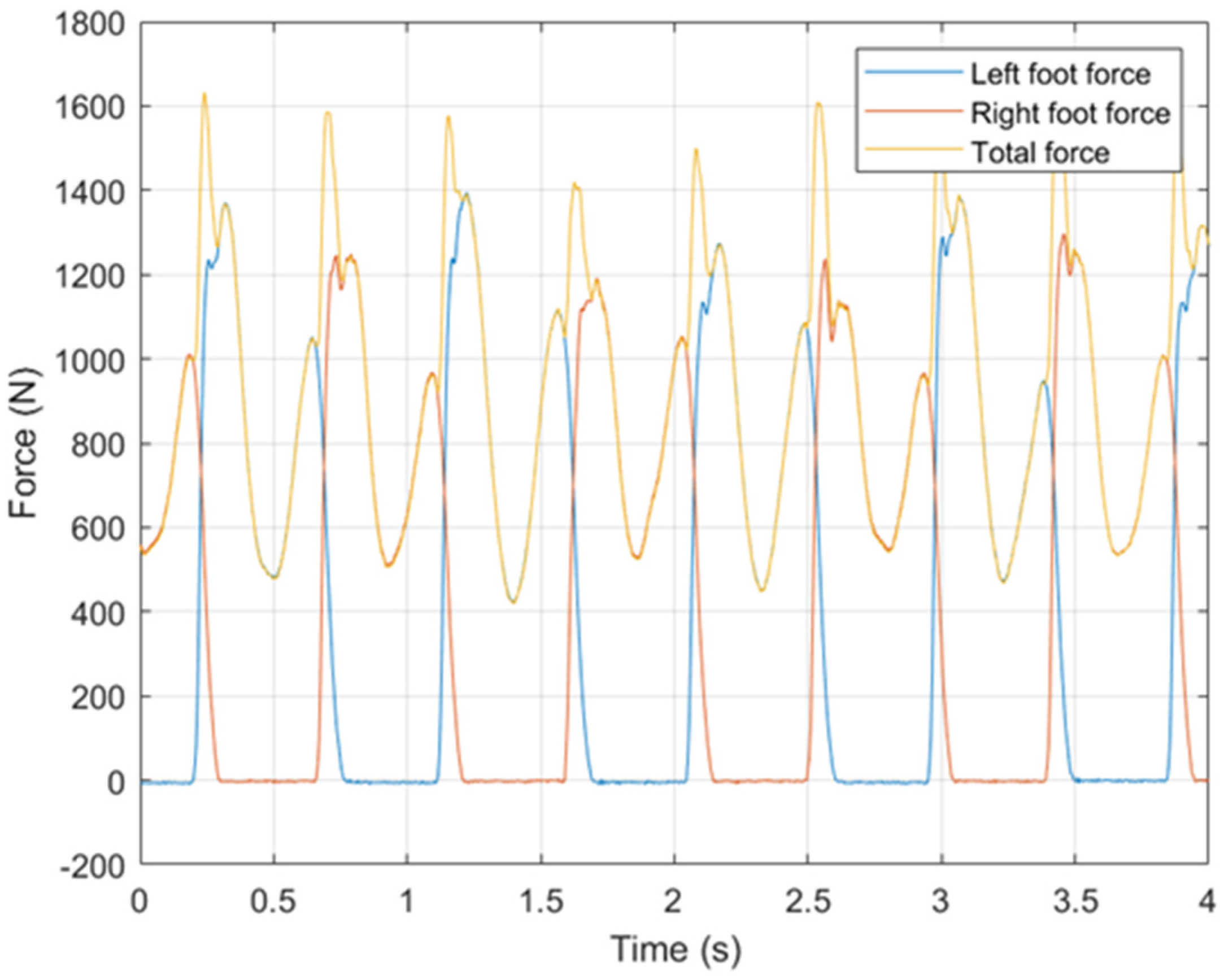
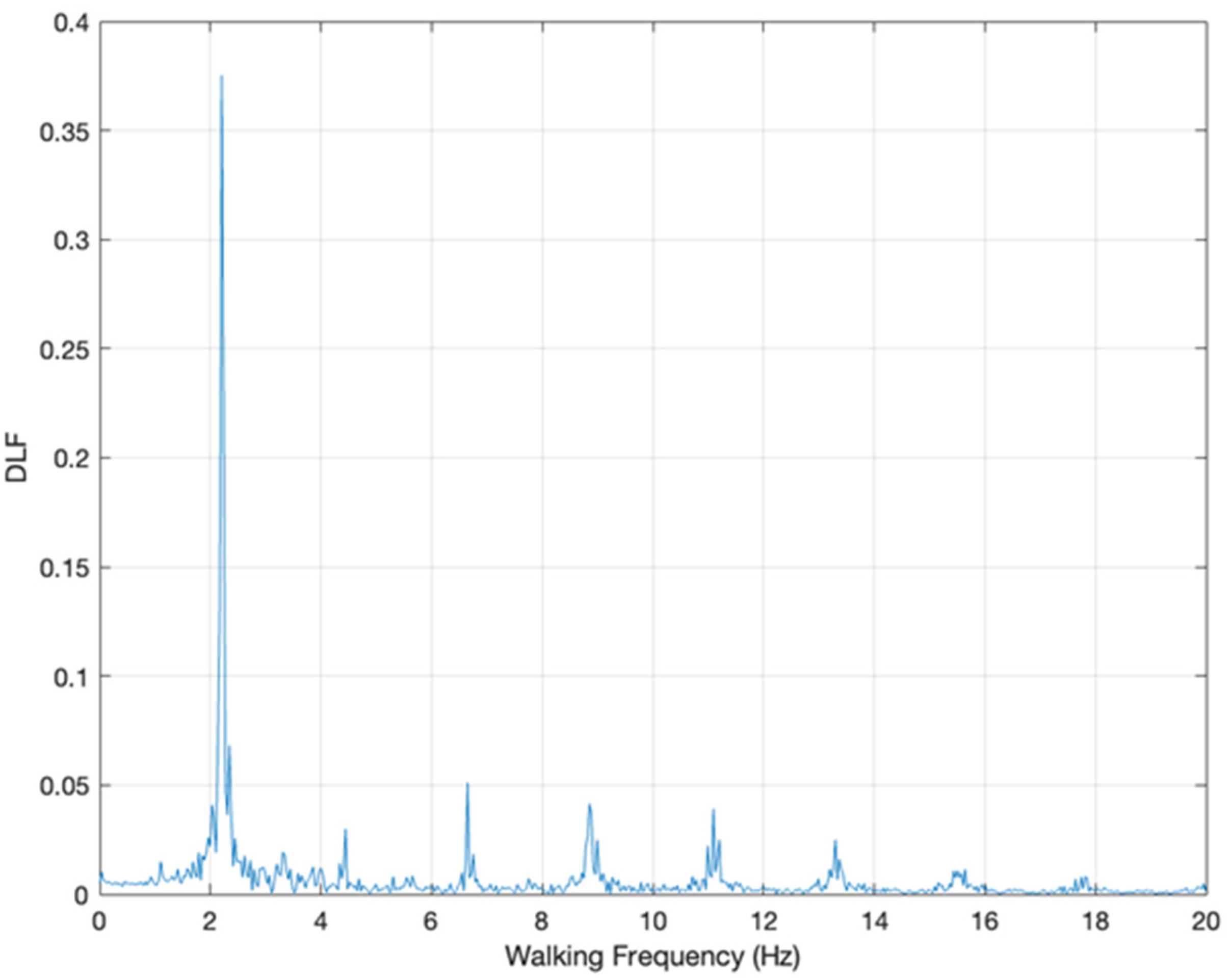
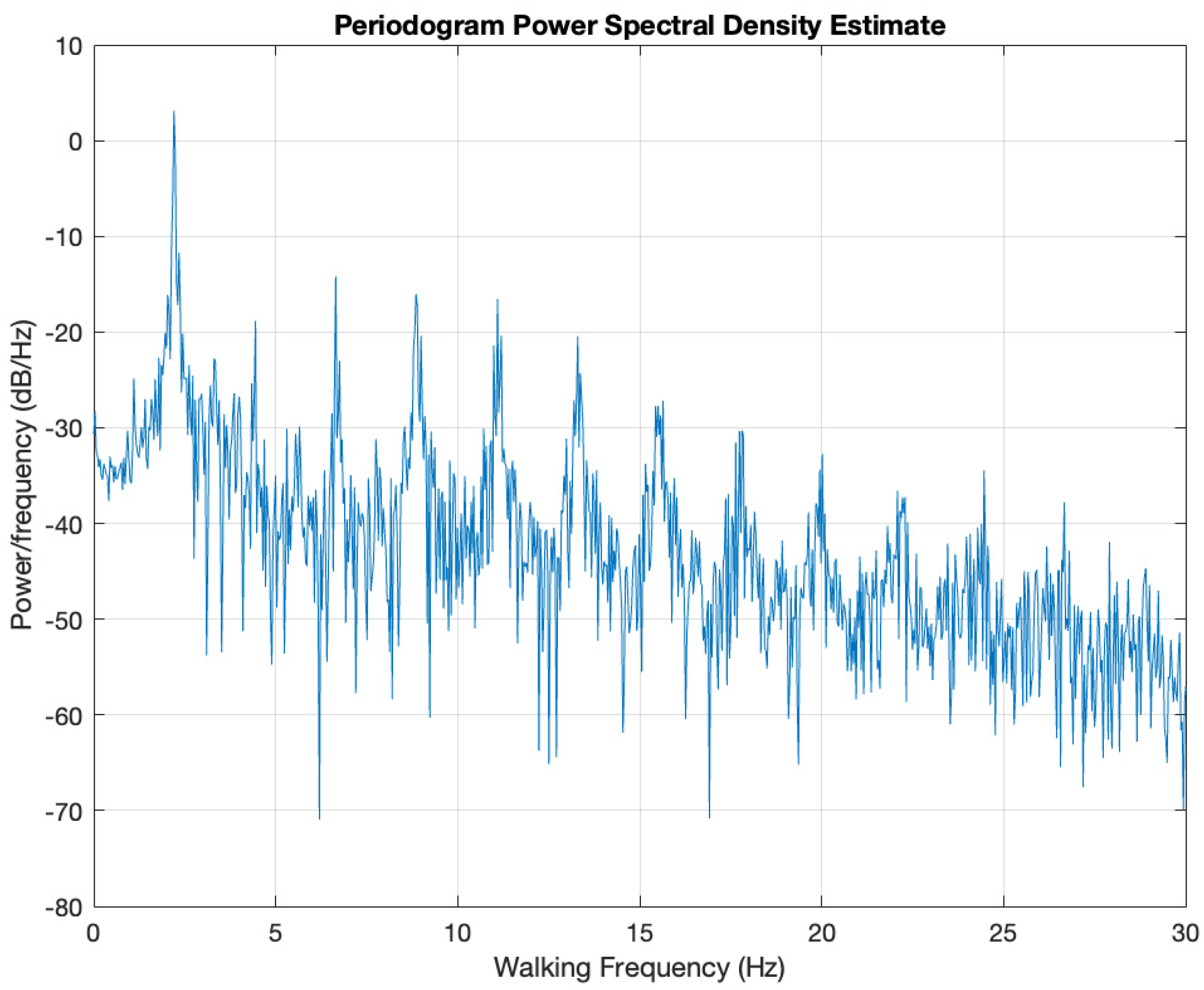
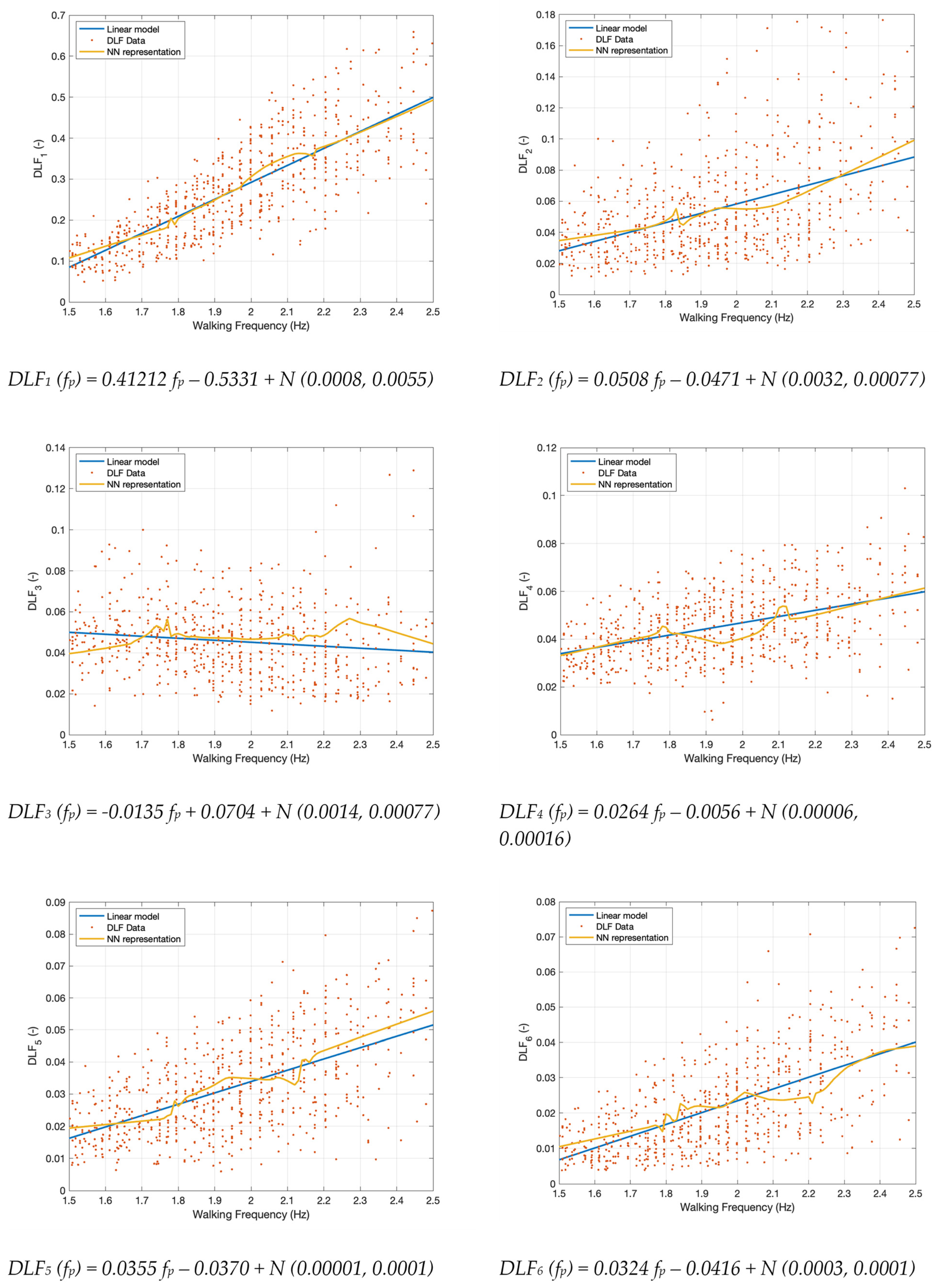


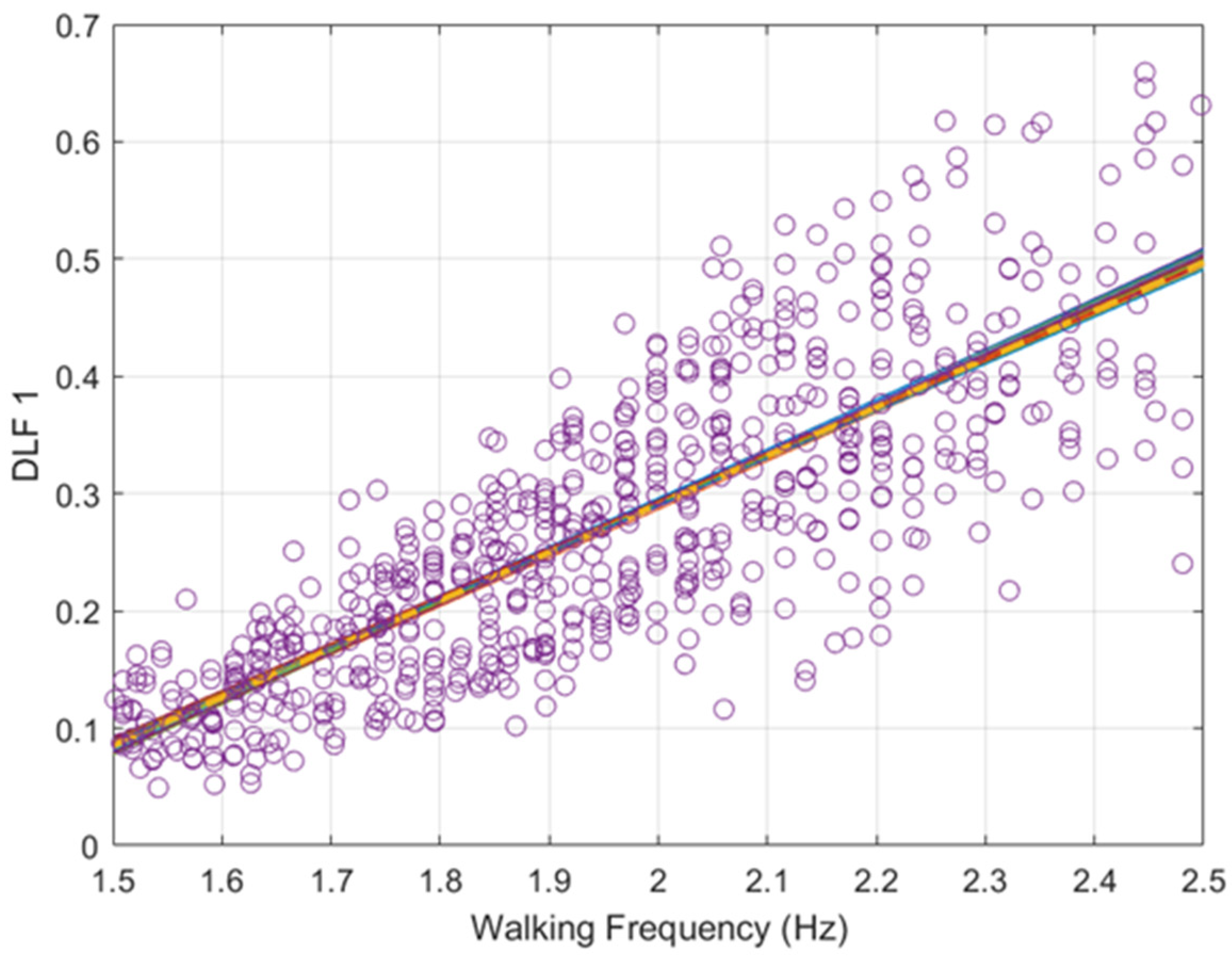




| Label | Author | Year | 1st Harmonic | 2nd Harmonic | 3rd Harmonic | 4th Harmonic |
|---|---|---|---|---|---|---|
| M1 | Blanchard [41] | 1977 | 0.257 | - | - | - |
| M2 | Bachmann [49] | 1987 | 0.37 | 0.1 | 0.12 | 0.04 |
| M3 | Allen et al. [50] | 1993 | 0.5 | 0.2 | 0.1 | 0.05 |
| M4 | Petersen [51] | 1996 | 0.073 0.408 0.518 | 0.138 0.079 0.058 | 0.018 (1.5 Hz) 0.018 (2 Hz) 0.041 (2.5 Hz) | - |
| M5 | Kerr [35] Mean | 1999 | −0.265 fp3 + 1.321 fp2 − 1.760 fp + 0.761 | 0.07 | 0.05 | - |
| M6 | Kerr [35] 5% | 1999 | −0.1801 fp3 + 0.898 fp2 − 1.1966 fp + 0.5177 | - | - | - |
| M7 | Kerr [35] 95% | 1999 | −0.3497 fp3 + 1.7432 fp2 − 2.3228 fp + 1.0049 | - | - | - |
| M8 | BS 5400 [52] | 1999 | 0.24 (180 N) | - | - | - |
| M9 | Ellis [53] | 2000 | - | - | 0.07 | 0.07 |
| M10 | Allen et al. [32] | 2001 | 290 e−0.35fp floors 410 e−0.35fp footbridges | - | - | - |
| M11 | Japanese load code [54] | 2004 | 0.4 | 0.2 | 0.06 | - |
| M12 | Brownjohn et al. [55] | 2004 | 0.37 fp − 0.42 | 0.053 | 0.042 | 0.041 |
| M13 | SETRA [25] | 2006 | 0.4 | 0.1 | 0.1 | - |
| M14 | Willford et al. [56] 75% | 2006 | 0.41 (fp − 0.95) | 0.069 + 0.0056 × 2 fp | 0.033 + 0.0064 × 3 fp | 0.013 + 0.0065 × 4 fp |
| M15 | Willford et al. [56] mean | 2006 | 0.37 (fp − 0.95) | 0.054 + 0.0044 × 2 fp | 0.026 + 0.005 × 3 fp | 0.010 + 0.0051 × 4 fp |
| M16 | Zivanovic [57,58] | 2006 | −0.2649 fp3 + 1.3206 fp2 − 1.7597 fp + 0.7613 Std. 0.16 | 0.07 s.d. 0.03 | 0.05 s.d. 0.02 | 0.05 s.d. 0.02 |
| M17 | ISO 10137 [13] | 2007 | 0.37 (fp − 1) | 0.1 | 0.06 | 0.06 |
| M18 | Smith [31] | 2007 | 0.436 (fp − 0.95) | 0.006 (2 fp + 12.3) | 0.007 (3 fp + 5.2) | 0.007 (4 fp + 2) |
| M19 | Nguyen [59] 90% | 2013 | 0.313 fp − 0.226 | 0.113 fp − 0.078 | 0.037 fp + 0.008 | 0.036 fp − 0.002 |
| M20 | Nguyen [59] 95% | 2013 | 0.406 fp − 0.355 | 0.126 fp − 0.084 | 0.031 fp + 0.027 | 0.047 fp − 0.014 |
| M21 | Chen et al. [60] | 2014 | 0.2358 fp − 0.2010 | 0.0949 | 0.0523 | 0.0461 |
| M22 | Toso et al. [48] | 2016 | 0.22 fp2 − 0.45 fp + 0.35 | 0.0243 + 6.87 × 10−5 c − 2.46 × 10−6 | −0.0638 + 0.0024 M − 1.09 × 10−6 K +1 × 10−8 MK − 1.38 × 10−5 M2 | - |
| M23 | AISC design guide 11 [22] | 2016 | 0.5 | 0.2 | 0.1 | 0.05 |
| M24 | Zhang et al. [44] | 2017 | Mean 0.4058 Std. 0.1663 | - | - | - |
| M25 | Chen et al. [42] | 2019 | 0.301 fp − 0.323 | 0.0301 fp + 0.053 | −0.054 fp + 0.264 | −0.1121 fp + 0.053 |
| M26 | Varela [61] | 2020 | 0.1556 fp2 − 0.1816 × fp + 0.0356 | 0.065 if fp < 2 0.1958 fp − 0.3266 if fp > 2 | - | - |
| Harmonic | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |
| Mean percentage of Energy compared to total (%) | 82.97 | 8.00 | 5.53 | 3.71 | 1.71 | 0.80 | 0.43 | 0.25 |
| Variance of Energy compared to total (%) | 1.6210 | 0.4650 | 0.3938 | 0.0752 | 0.01298 | 0.0031 | 0.0011 | 0.0005 |
| +/−Percentage of Walking frequency considered | 5% | 10% | 15% | 20% | 25% | 30% | 35% | 40% | 45% | 50% |
| Average percentage change in DLF values from selecting peak (%) | 23 | 31 | 33 | 34 | 34 | 36 | 36 | 37 | 37 | 39 |
| Model | 1st Order Polynomial | Fine Tree | Coarse Tree | Linear SVM | Cubic SVM | Ensemble Boosted Trees | Ensemble Bagged Trees | Gaussian Process- Squared Exponential Bases Function | Gaussian Process- Rational Quadratic Bases Function | Neural Network- Ten Hidden Layers-Relu Activation Function |
|---|---|---|---|---|---|---|---|---|---|---|
| RMSE | 0.074205 | 0.076378 | 0.074829 | 0.074393 | 0.074699 | 0.07515 | 0.074711 | 0.074255 | 0.074309 | 0.0556 |
| DLF | Mean Values (Intercept, Gradient) μ | Covariance (Intercept, Gradient) Λ | The Variance of Error (α, β | OLS | MAP |
|---|---|---|---|---|---|
| 1 | |||||
| 2 | |||||
| 3 | |||||
| 4 | |||||
| 5 | |||||
| 6 | |||||
| 7 | |||||
| 8 |
| Model | Walking Frequency Limits (Hz) |
|---|---|
| AISC Design Guide 11 [22] | 1.6–2.2 |
| ISO 10137 [13] | 1.2–2.4 |
| Technical report 43 Appendix G [23] | 1–2.8 |
| CCIP Mean value [24] | 1–2.8 |
| CCIP design value [24] | 1–2.8 |
| SCI P35 [31] | 1.6–2.2 |
| SETRA [25] | 1.6–2.4 |
| Varela et al. [61] | 1.4–2.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peters, A.E.; Racic, V.; Živanović, S.; Orr, J. Fourier Series Approximation of Vertical Walking Force-Time History through Frequentist and Bayesian Inference. Vibration 2022, 5, 883-913. https://doi.org/10.3390/vibration5040052
Peters AE, Racic V, Živanović S, Orr J. Fourier Series Approximation of Vertical Walking Force-Time History through Frequentist and Bayesian Inference. Vibration. 2022; 5(4):883-913. https://doi.org/10.3390/vibration5040052
Chicago/Turabian StylePeters, Angus Ewan, Vitomir Racic, Stana Živanović, and John Orr. 2022. "Fourier Series Approximation of Vertical Walking Force-Time History through Frequentist and Bayesian Inference" Vibration 5, no. 4: 883-913. https://doi.org/10.3390/vibration5040052
APA StylePeters, A. E., Racic, V., Živanović, S., & Orr, J. (2022). Fourier Series Approximation of Vertical Walking Force-Time History through Frequentist and Bayesian Inference. Vibration, 5(4), 883-913. https://doi.org/10.3390/vibration5040052







