Study on Hydraulic Dampers Using a Foldable Inverted Spiral Origami Structure
Abstract
1. Introduction
2. Materials and Methods
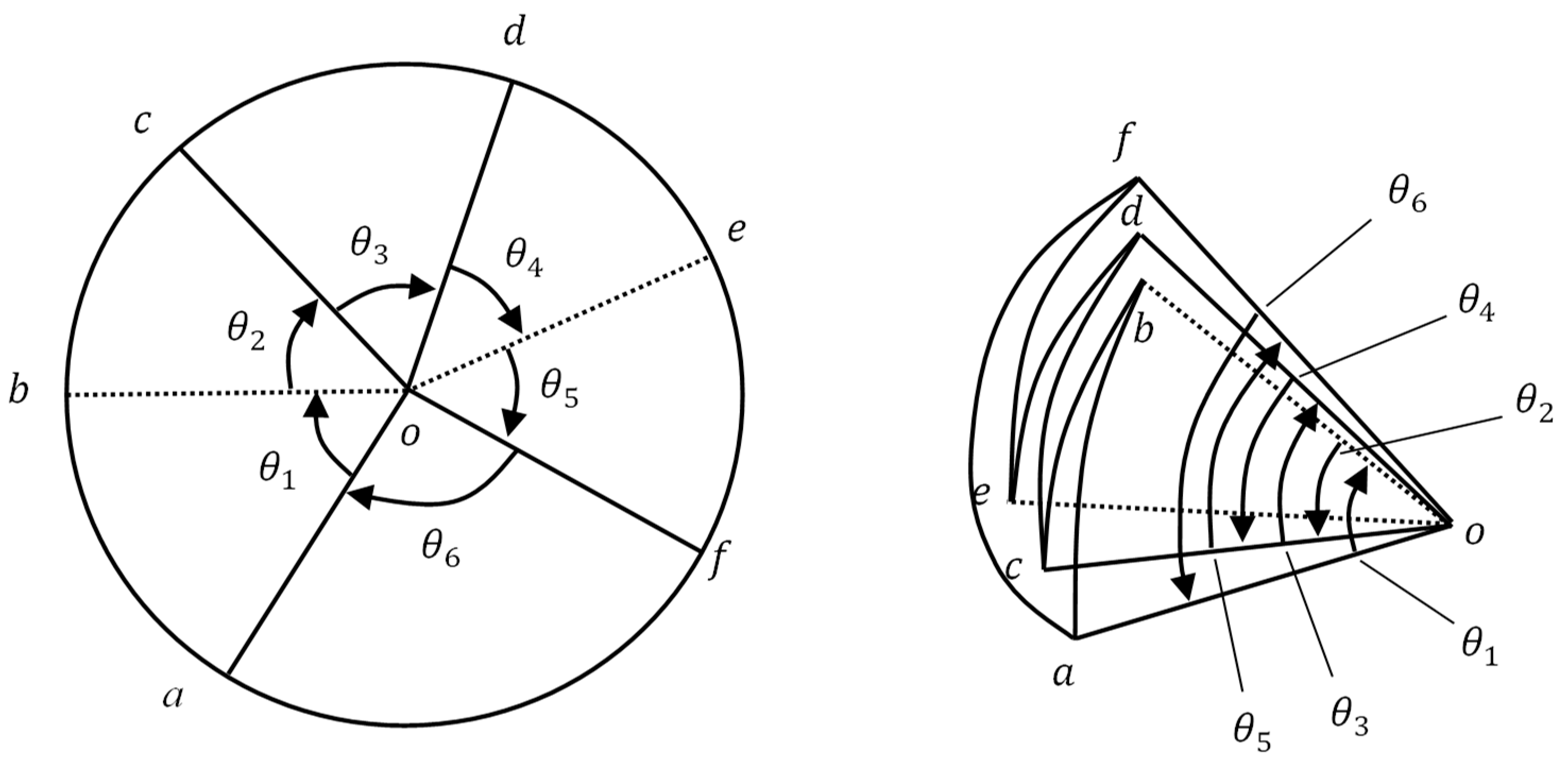
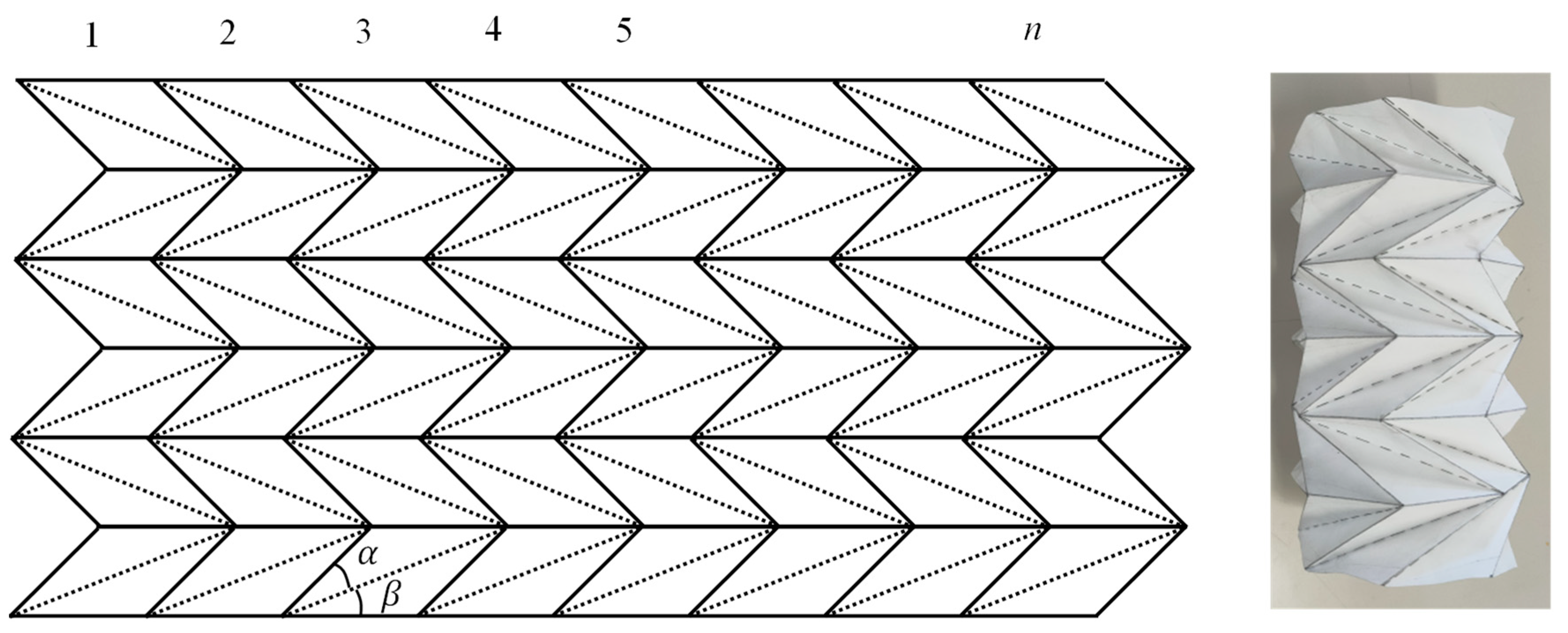
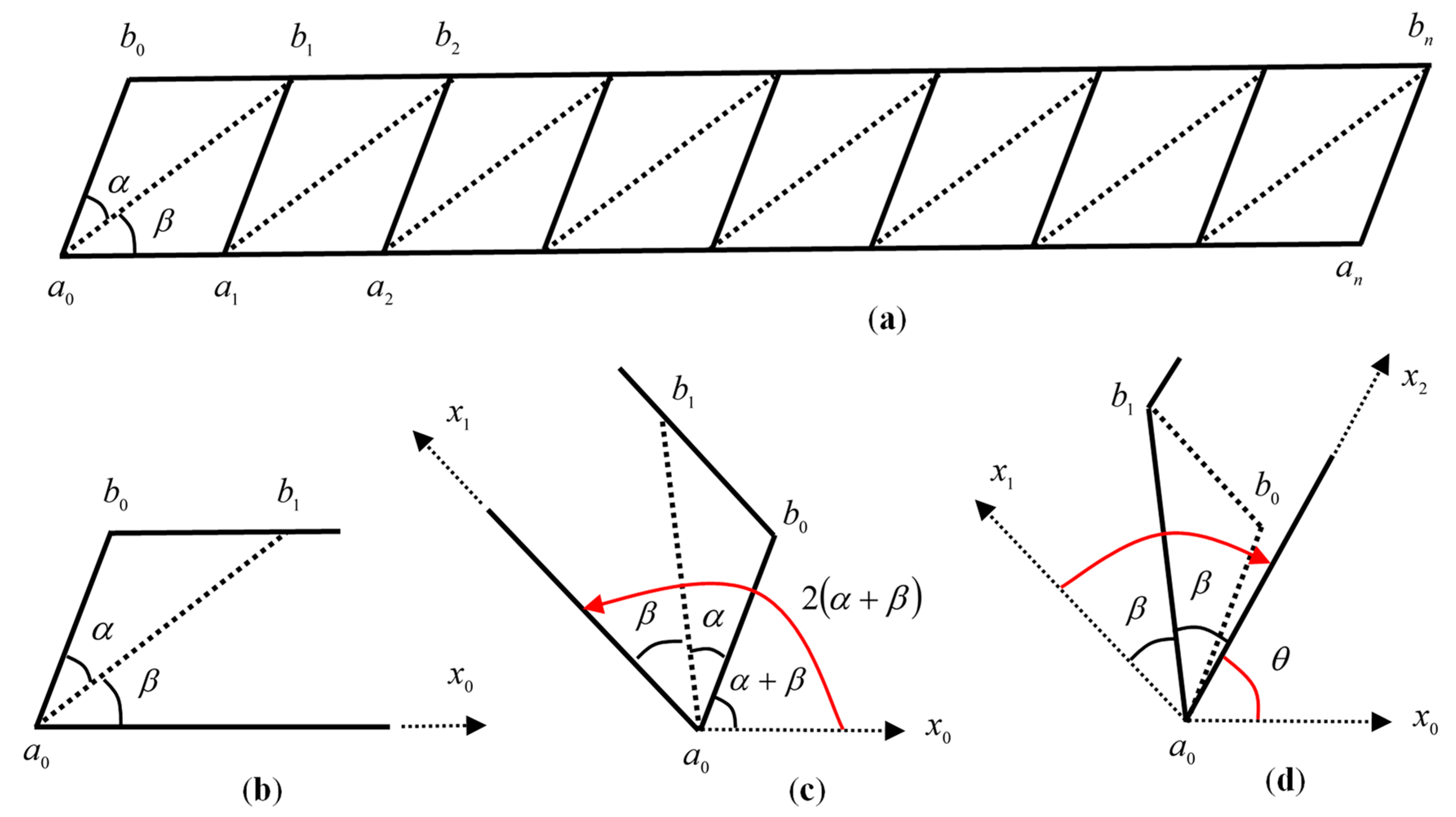
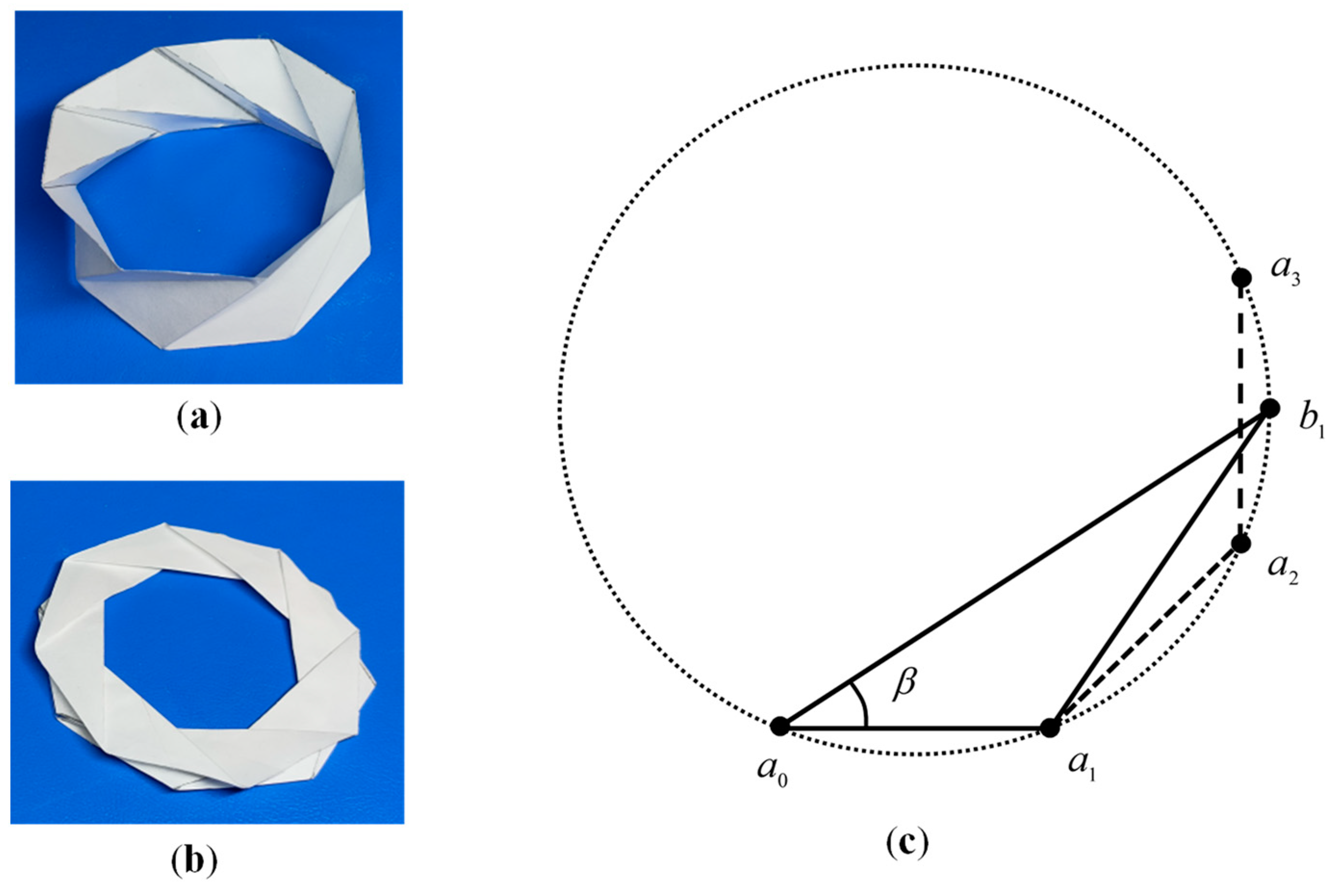
2.1. Inverted Spiral Origami Structure
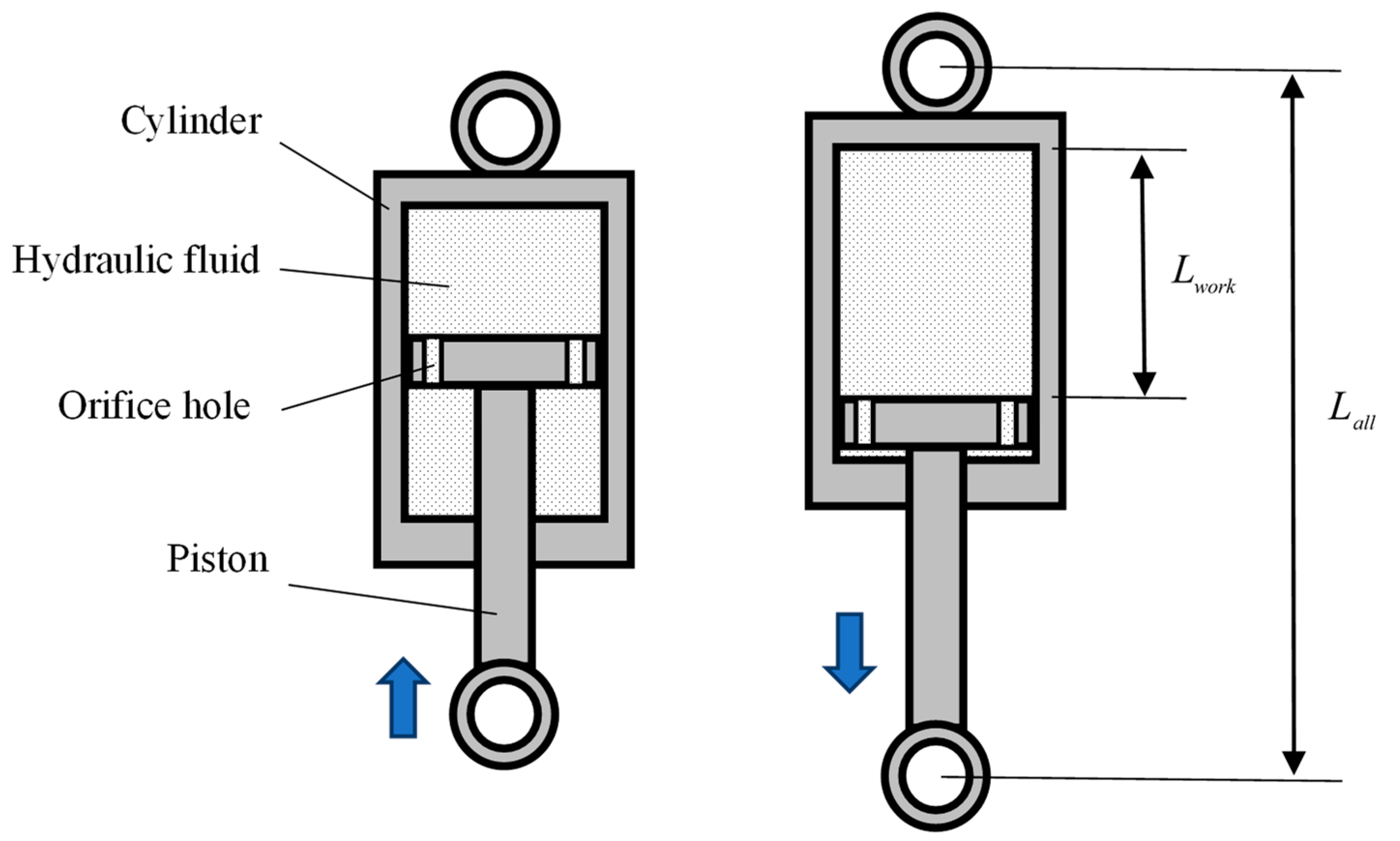
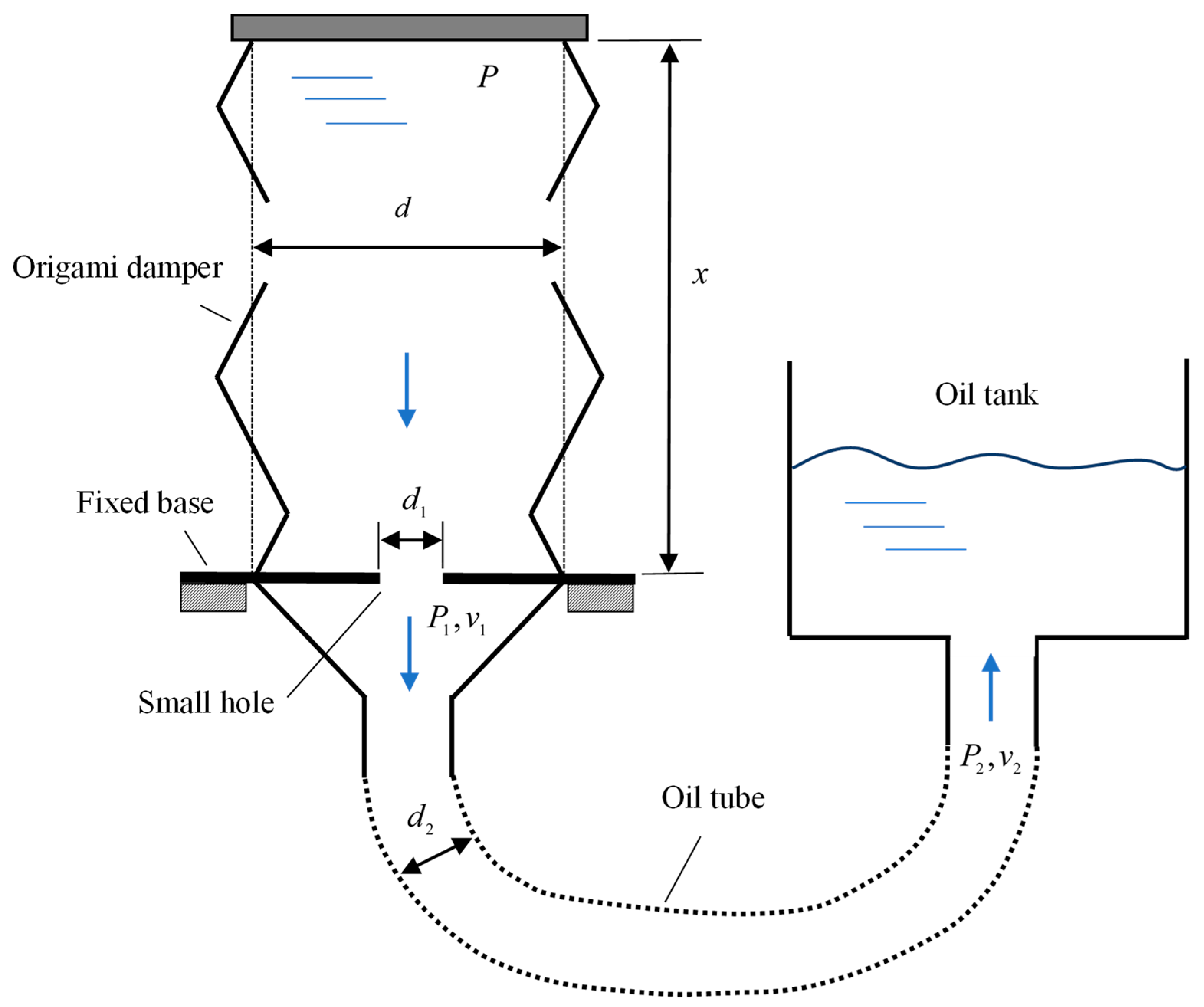
2.2. Inverted Spiral Origami Type Hydraulic Damper
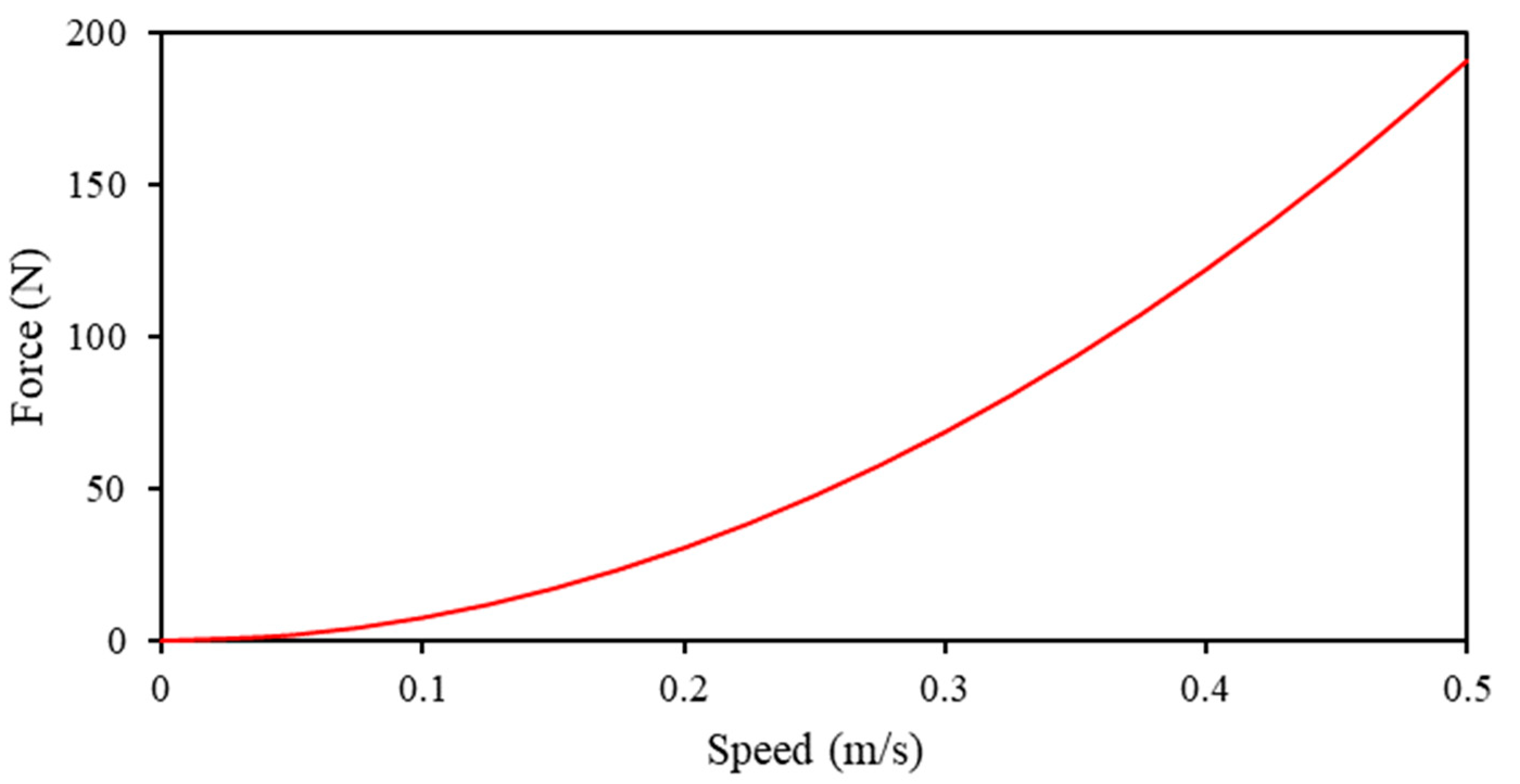
2.3. Damping Force of Origami Type Hydraulic Damper
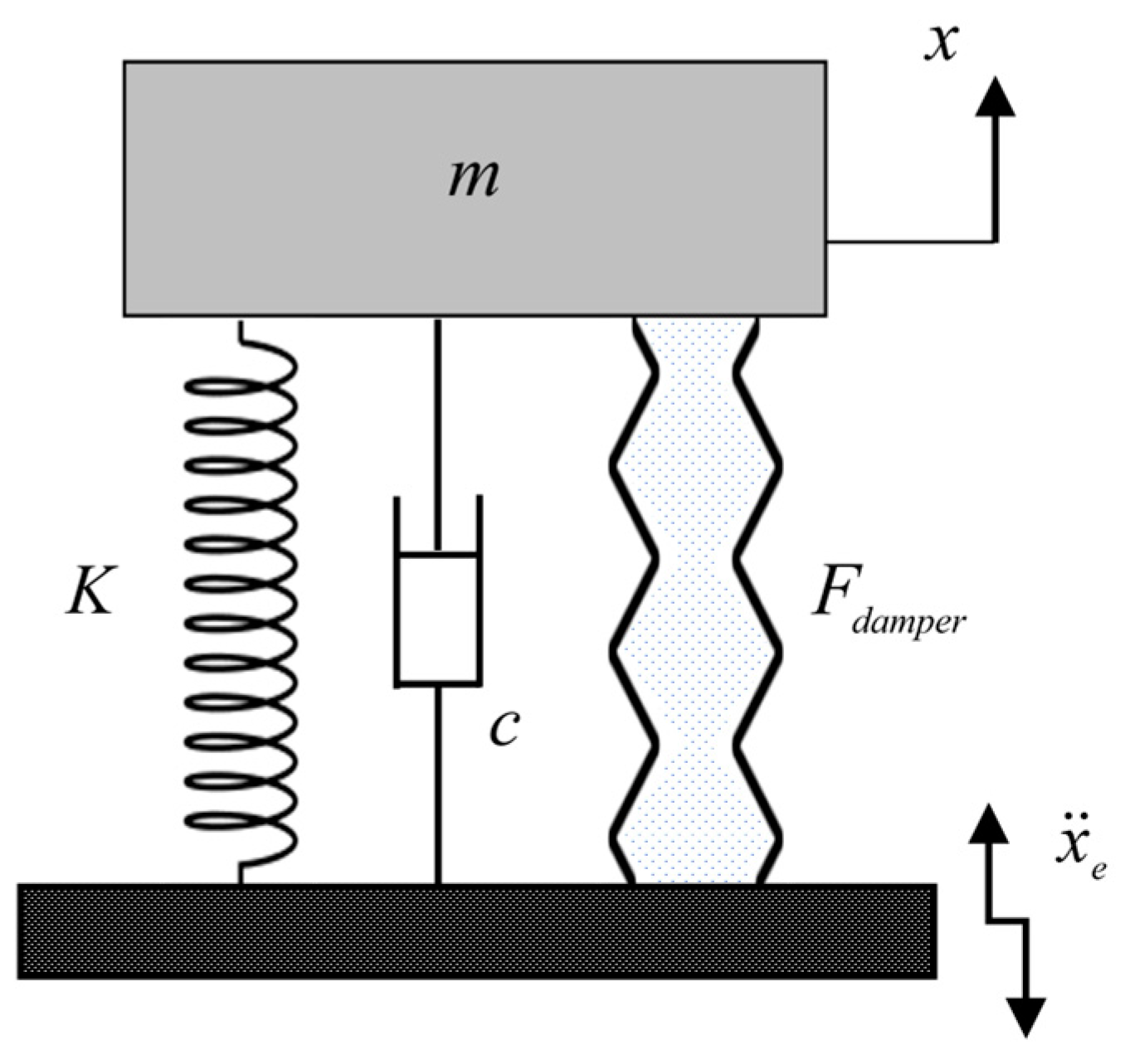
2.4. Vibration Analysis Method
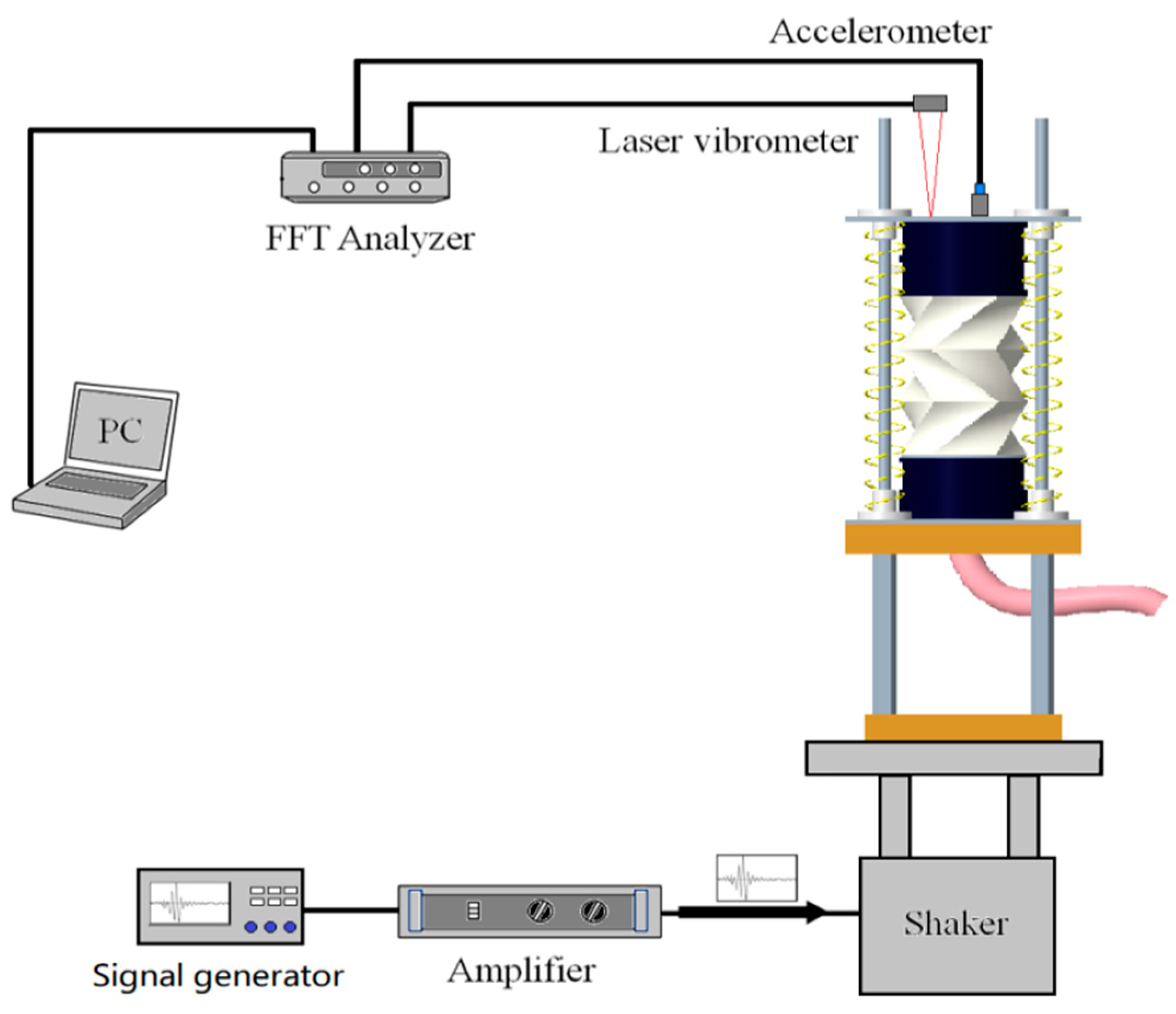
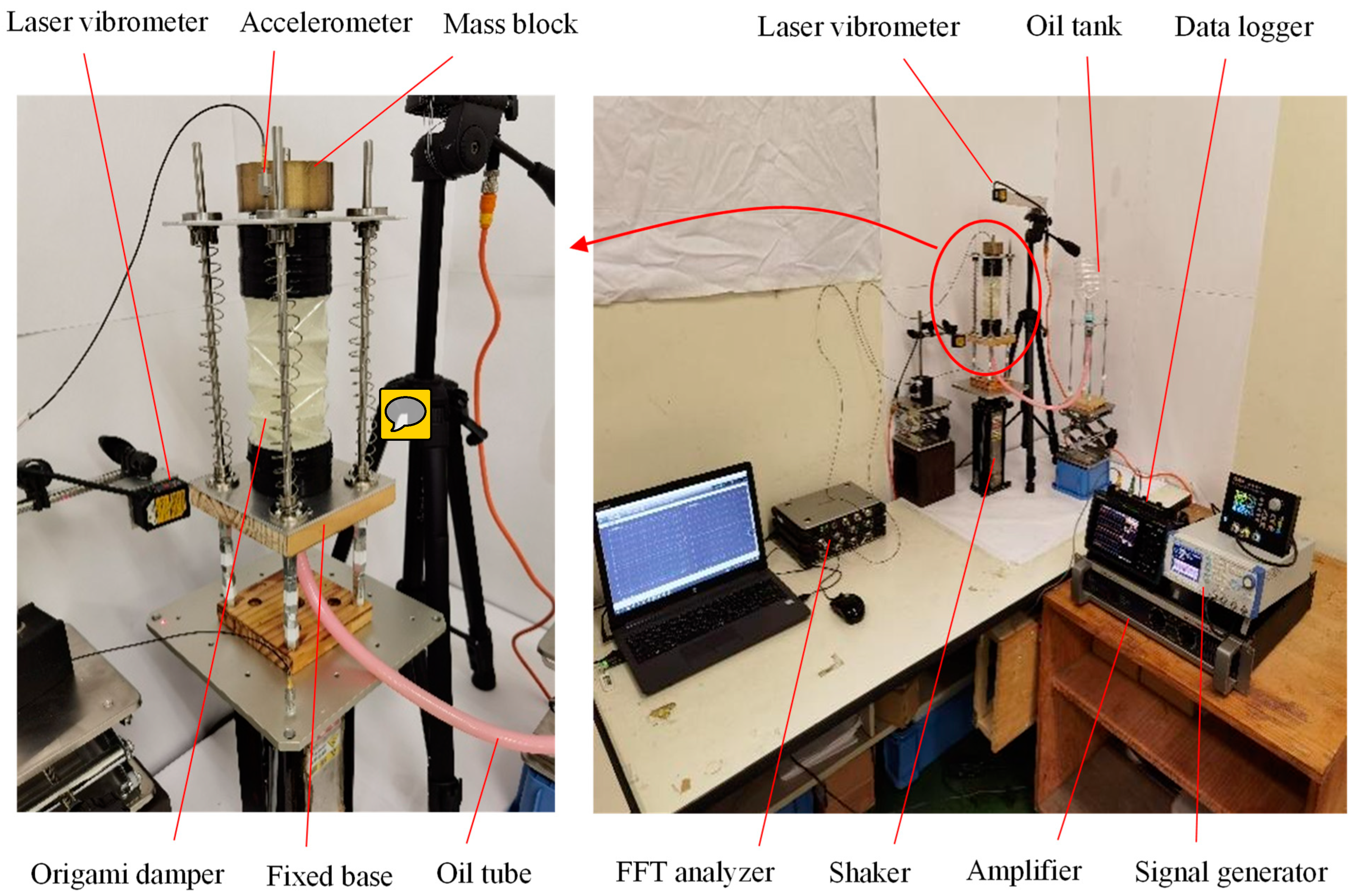
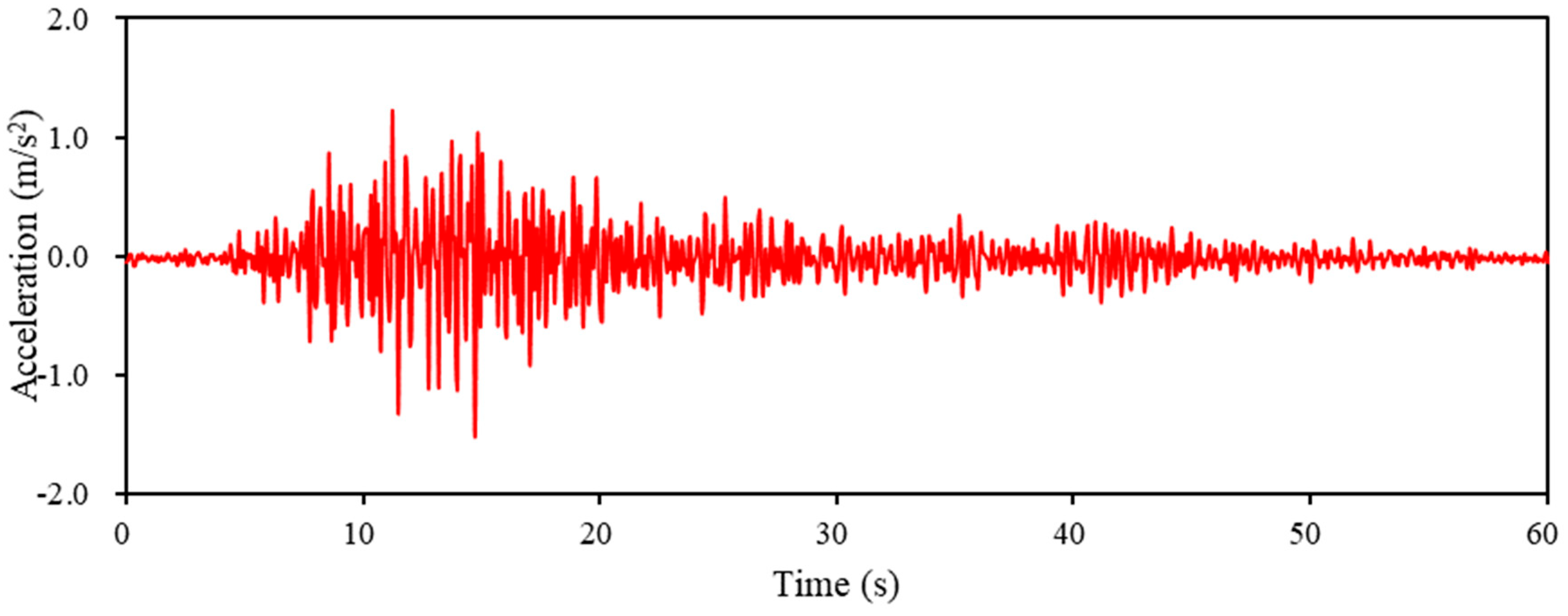
2.5. Vibration Verification Experiment
3. Results and Discussion
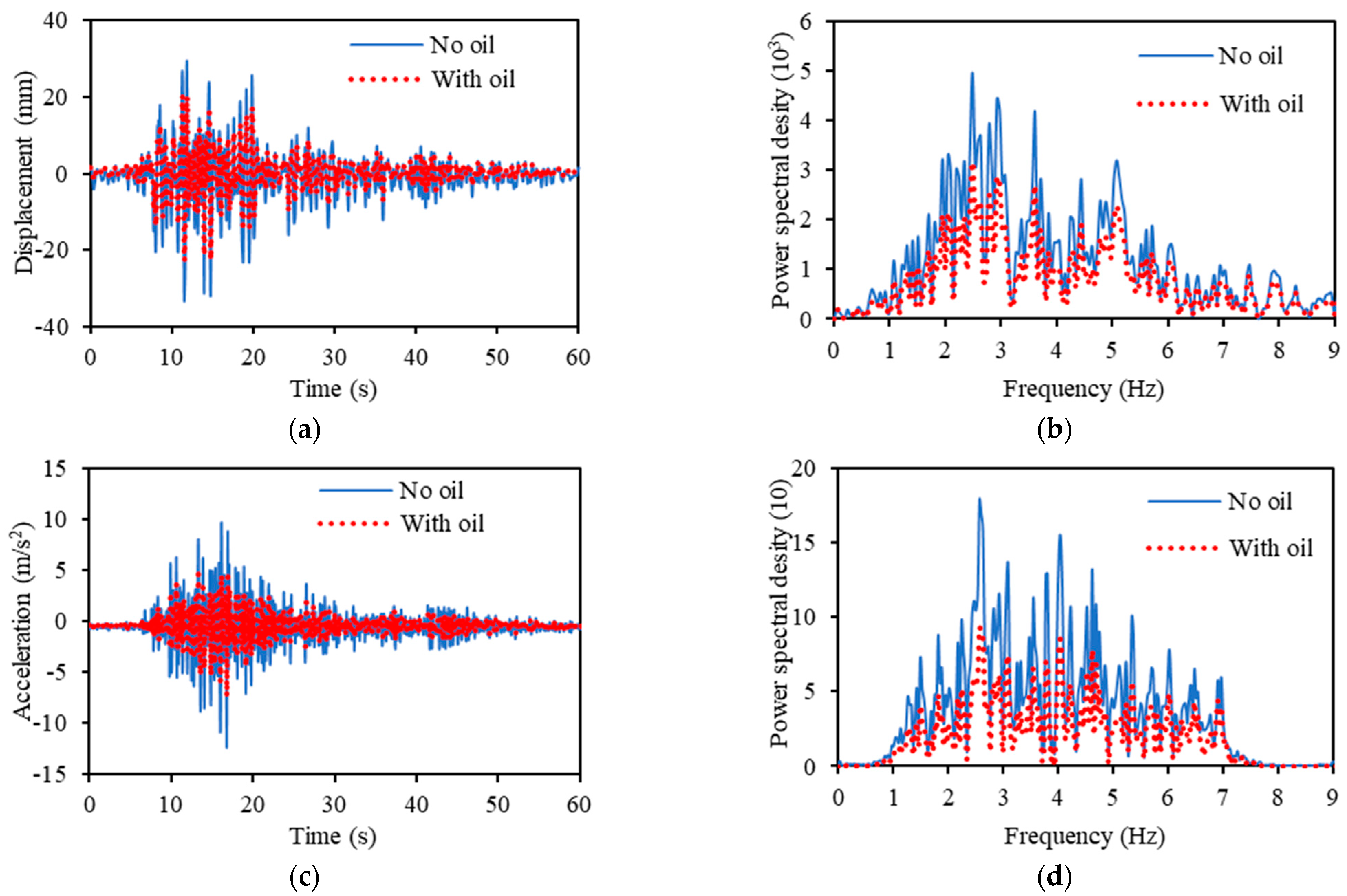
3.1. Vibration Verification Experiment
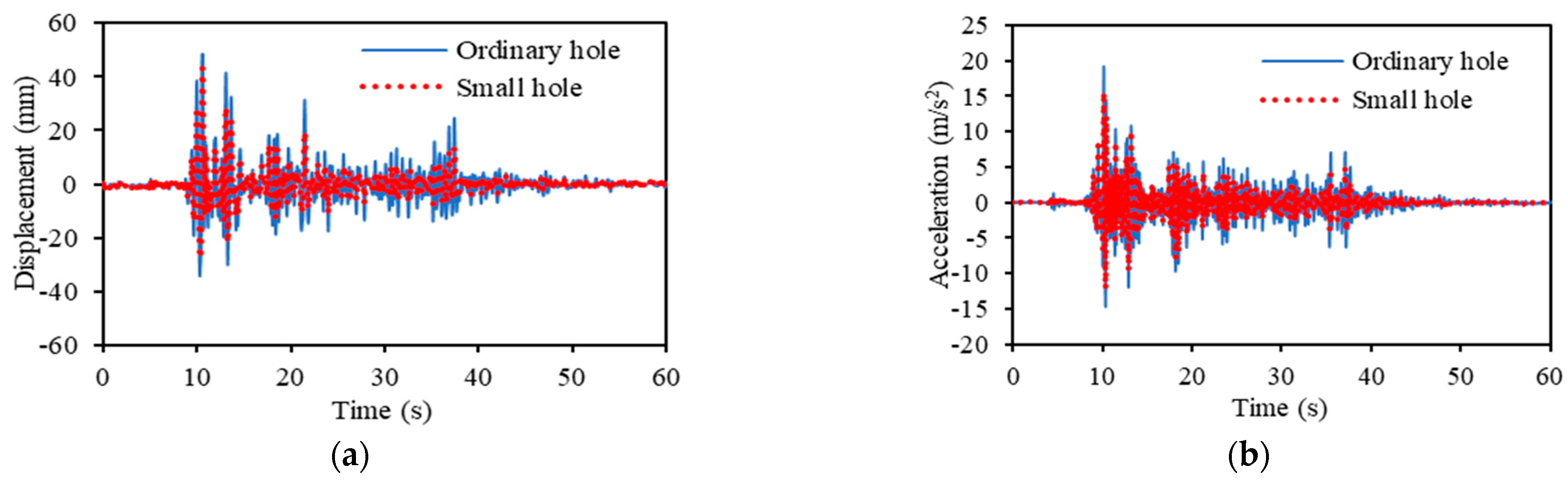
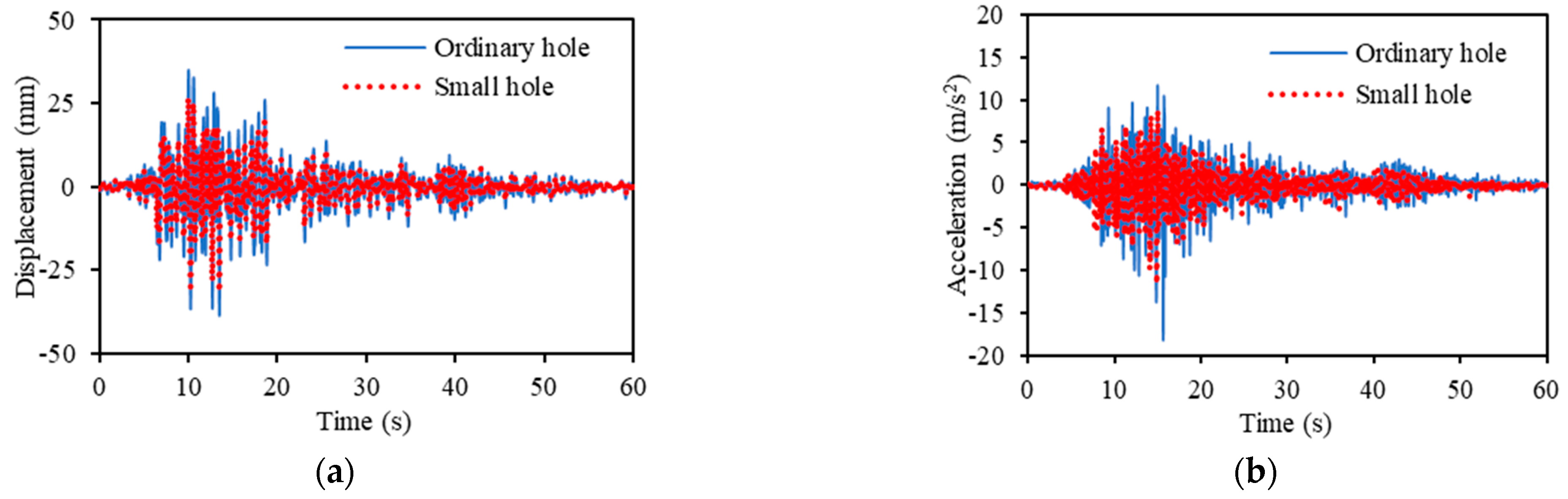
3.2. Effects of Orifice Hole
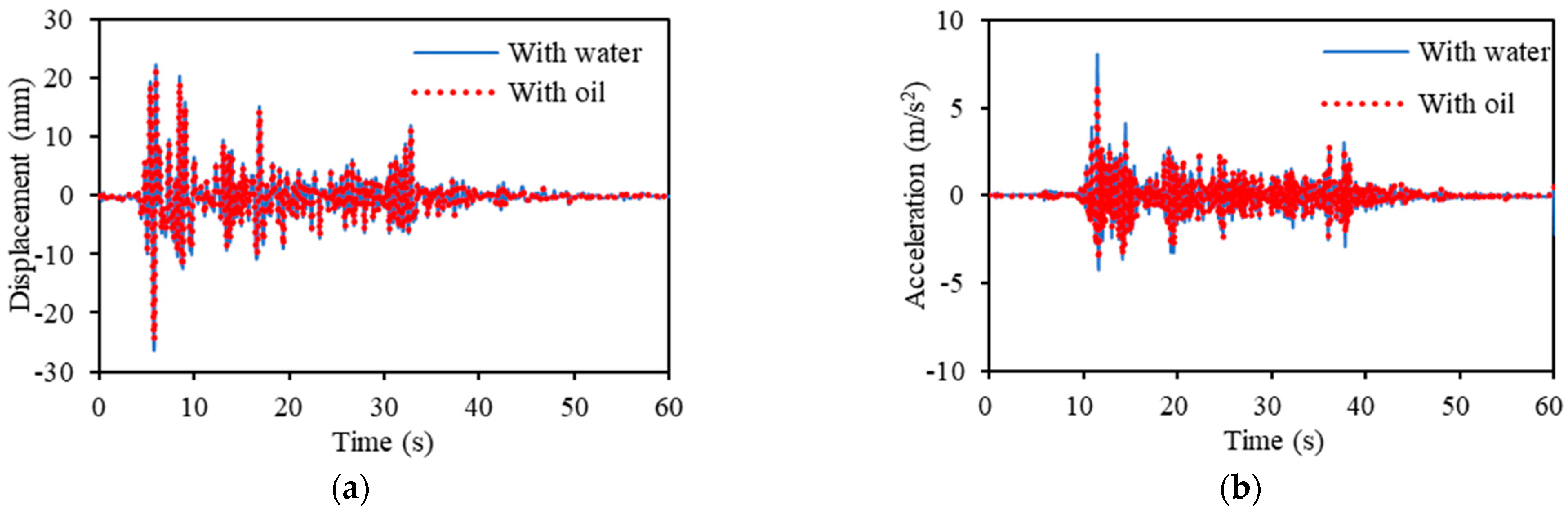
3.3. Effect of Fluid Type
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Balasubramanian, K.R.; Sivapirakasam, S.P.; Anand, R. Hydraulic Circuit in Damper: An Overview. Appl. Mech. Mater. 2014, 592–594, 2056–2065. [Google Scholar]
- Wu, Z.; Xu, G.; Yang, H.; Li, M. Analysis of Damping Characteristics of a Hydraulic Shock Absorber. Shock. Vib. 2021, 2021, 8883024. [Google Scholar] [CrossRef]
- Czop, P.; Slawik, D.; Wszolek, G. Development of an optimization method for minimizing vibrations of a hydraulic damper. Simulation 2013, 88, 1073–1086. [Google Scholar] [CrossRef]
- Sun, J.; Jiao, S.; Huang, X.; Hua, H. Investigation into the Impact and Buffering Characteristics of a Non-Newtonian Fluid Damper: Experiment and Simulation. Shock. Vib. 2014, 2014, 170464. [Google Scholar] [CrossRef]
- Gao, H.; Cui, M.; Dai, L.; Yang, J.; Zhou, X. Mathematical Modelling and Computational Simulation of the Hydraulic Damper during the Orifice-Working Stage for Railway Vehicles. Math. Prob. Eng. 2020, 2020, 1830150. [Google Scholar] [CrossRef]
- Jugulkar, L.M.; Shankar, S.; Sawant, S.M. Fluid flow modeling and experimental investigation on automobile damper. Construct. Build. Mater. 2016, 121, 760–772. [Google Scholar] [CrossRef]
- Dharankar, C.S.; Hada, M.K.; Chandel, S. Experimental identification of non-hysteresis algebraic force model of automotive hydraulic damper. Int. J. Vehicle Perform. 2019, 5, 2. [Google Scholar] [CrossRef]
- Ahmed, M.R.; Yusoff, A.R.; Romlay, F.R.M. Numerical Investigation of Continuous Damping of The Semi-Active Suspension System for Passenger Car. IOP Conf. Ser. Mater. Sci. Eng. 2019, 530, 012014. [Google Scholar] [CrossRef]
- Dobre, A. Modelling the dynamic behaviour of car hydraulic dampers. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1091, 012018. [Google Scholar] [CrossRef]
- Go, C.; Sui, C.; Shih, M.; Sung, W. A Linearization Model for the Displacement Dependent Semi-active Hydraulic Damper. J. Vib. Contr. 2010, 16, 2195–2214. [Google Scholar] [CrossRef]
- Azimi, M.; Rasoulnia, A.; Lin, Z.; Pan, H. Improved semi-active control algorithm for hydraulic damper-based braced buildings. Struct. Contr. Heal. Monit. 2017, 24, 11. [Google Scholar] [CrossRef]
- Hu, K.; Li, F.; Zhang, Z.; Wang, S.; Jiang, H. A Magnetoelectric Hybrid Hydraulic Damper for the Mining Robot Suspension System. Shock. Vib. 2021, 2021, 5479423. [Google Scholar] [CrossRef]
- Goldasz, J.; Sapinski, B.; Jastrzebski, L.; Kubik, M. Dual Hysteresis Model of MR Dampers. Front. Mater. 2020, 2021, 5479423. [Google Scholar] [CrossRef]
- Raut, L.B.; Khedkar, Y.M.; Salunke, S.Y.; Jadhav, S.V. Modification of Classical Hydraulic damper into Semi active damper using MR Approach. Int. J. Sci. Res. Publ. 2019, 9, 676–683. [Google Scholar] [CrossRef]
- Jin, G.; Zhao, Z.; Liu, B.; Cun, W.; Zhao, Z.; Hou, M.; Chen, G. Design of a particle damper and experimental study on vibration damping of the pipeline. Advan. Mech. Eng. 2021, 13, 9. [Google Scholar] [CrossRef]
- Wang, C.; Qiu, Z.; Zheng, Q.; Liu, Y.; Miao, G. Energy Dissipation Mechanism and Control Model of a Digital Hydraulic Damper. Shock. Vib. 2019, 2019, 1432910. [Google Scholar] [CrossRef]
- Lv, C.; Krishnaraju, D.; Konjevod, G.; Yu, H.; Jiang, H. Origami based Mechanical Metamaterials. Sci. Rep. 2014, 4, 5979. [Google Scholar] [CrossRef]
- Shen, T.; Nagai, Y. An Overview of Folding Techniques in Architecture Design. World J. Eng. Tech. 2017, 5, 12–19. [Google Scholar] [CrossRef]
- Dureisseix, D. An Overview of Mechanisms and Patterns with Origami. Int. J. Mech. Mater. Des. 2021, 17, 801–829. [Google Scholar] [CrossRef]
- Lebee, A. From Folds to Structures, a Review. Int. J. Space Struct. 2015, 30, 55–74. [Google Scholar] [CrossRef]
- Liu, X.; Gattas, J.M.; Chen, Y. One-DOF Superimposed Rigid Origami with Multiple States. Sci. Rep. 2016, 6, 36883. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Zhou, Z.; Kwok, K.W.; Sze, K.Y. Simulating flexible origami structures by finite element method. Int. J. Mech. Mater. Des. 2021, 17, 801–829. [Google Scholar] [CrossRef]
- Chen, Y.; Lv, W.; Li, J.; You, Z. An Extended Family of Rigidly Foldable Origami Tubes. J. Mech. Robot. 2017, 9, 021002. [Google Scholar] [CrossRef]
- Yue, W.; Qi, J.; Song, X.; Fan, S.; Fortino, G.; Chen, C.; Xu, C.; Ren, H. Origami-Inspired Structure with Pneumatic-Induced Variable Stiffness for Multi-DOF Force-Sensing. Sensors 2022, 22, 5370. [Google Scholar] [CrossRef] [PubMed]
- Guest, S.D.; Pellegrino, S. The Folding of Triangulated Cylinders, Part I: Geometric Considerations. J. Appl. Mech. 1994, 61, 773–777. [Google Scholar] [CrossRef]
- Shen, W.; Cao, Y.; Jiang, X.; Zhang, Z.; Kremer, G.E.O.; Qin, H. Experimental and Numerical Investigation on Radial Stiffness of Origami-Inspired Tubular Structures. J. Appl. Mech. 2022, 89, 031001. [Google Scholar] [CrossRef]
- Reid, A.; Lechenault, F.; Rica, S.; Adda-Bedia, M. Geometry and design of origami bellows with tunable response. Phys. Rev. E 2017, 95, 013002. [Google Scholar] [CrossRef]
- Agarwal, V.; Wang, K.W. On the nonlinear dynamics of a Kresling-pattern origami under harmonic force excitation. Extrem. Mech. Lett. 2022, 52, 101653. [Google Scholar] [CrossRef]
- Yang, Y.; Ishida, S.; Zhao, X.; Hagiwara, I. Vehicle energy absorbers consisting of foldable cylinders using response surface methodology. Int. J. Vehicle Perform. 2017, 3, 380–394. [Google Scholar] [CrossRef]
- Terada, K.; Kadoi, K.; Tokura, S.; Sushida, T.; Hagiwara, I. The deformation mechanism on origami-based foldable structures. Int. J. Vehicle Perform. 2017, 3, 334–346. [Google Scholar] [CrossRef]
- Zhao, X.; Hu, Y.; Hagiwara, I. Shape Optimization to Improve Energy Absorption Ability of Cylindrical Thin-Walled Origami Structure. J. Comput. Sci. Tech. 2011, 5, 148–162. [Google Scholar] [CrossRef]
- Huang, J.; Zhou, J.; Wang, Z.; Law, J.; Cao, H.; Li, Y.; Wang, H.; Liu, Y. Modular Origami Soft Robot with the CAD Perception of Interaction Force and Body Configuration. Adv. Intell. Syst. 2022, 4, 2200081. [Google Scholar] [CrossRef]
- Onal, C.D.; Wood, R.J.; Rus, D. An Origami-Inspired Approach to Worm Robots. IEEE/ASME Tran. Mech. 2013, 18, 430–438. [Google Scholar] [CrossRef]
- Wu, S.; Qiji Ze, Q.; Dai, J.; Udipi, N.; Paulino, G.H.; Zhao, R. Stretchable origami robotic arm with omnidirectional bending and twisting. Proc. Natl. Acad. Sci. USA 2021, 118, 2110023118. [Google Scholar] [CrossRef] [PubMed]
- Huang, Z.; Wei, C.; Dong, L.; Wang, A.; Yao, H.; Guo, Z.; Mi, S. Fluid-driven hydrogel actuators with an origami structure. iScience 2022, 25, 104674. [Google Scholar] [CrossRef]
- Ye, S.; Zhao, P.; Zhao, Y.; Kavousi, F.; Feng, H.; Hao, G. A Novel Radially Closable Tubular Origami Structure (RC-ori) for Valves. Actuators 2022, 11, 243. [Google Scholar] [CrossRef]
- Kawasaki, H. An Application of a Theorem of Alternatives to Origami. J. Oper. Res. Soc. Jpn. 2017, 60, 393–399. [Google Scholar] [CrossRef][Green Version]
- Cengel, Y.A.; Cimbala, J.M. Fluid Mechanics: Fundamentals and Applications; McGraw-Hill: New York, NY, USA, 2006; pp. 347–430. [Google Scholar]







| Items | Parameters |
|---|---|
| Hydraulic oil density | 860 kg/m3 |
| Orifice flow coefficient c | 0.61 |
| Coefficient of friction of oil tube | 0.005 |
| Pressure drop coefficient of rapid expansion tube Kc | 0.65 |
| Oil tube length L | 300 mm |
| Average diameter of cylindrical origami hydraulic damper d | 50 mm |
| Orifice hole diameter d1 | 12 mm |
| Oil tube diameter d2 | 15 mm |
| Items | Parameters |
|---|---|
| Spring length l0 | 110 mm |
| Spring coefficient K | 280 N/m |
| Mass of mass block m | 1.431 kg |
| Friction damping coefficient c | 0.35 Ns/m |
| FFT analyzer | Shimadzu Corporation DS-3000 |
| Laser vibrometer | Optex FA CD100 ± 50 |
| Accelerometer | Onosokki NP-3421 |
| Shaker | SAN-Esu SSV-60S |
| Signal generator | NF Corporation WF1973 |
| Data logger | HIOKI Corporation LR8431 |
| Displacement | Acceleration | |||
|---|---|---|---|---|
| Maximum value | No oil | 37.72 | No oil | 10.85 |
| With oil | 24.60 | With oil | 5.80 | |
| Change | −53.33% | Change | −87.07% | |
| Average value | No oil | 2.88 | No oil | 0.45 |
| With oil | 1.85 | With oil | 0.32 | |
| Change | −55.78% | Change | −39.05% | |
| Kurtosisvalue | No oil | 7.46 | No oil | 25.12 |
| With oil | 7.63 | With oil | 16.03 | |
| Change | 2.18% | Change | −56.73% | |
| Skewness Value | No oil | 2.54 | No oil | 3.97 |
| With oil | 2.56 | With oil | 3.14 | |
| Change | 0.98% | Change | −26.39% | |
| Displacement | Acceleration | |||
|---|---|---|---|---|
| Maximum value | No oil | 33.41 | No oil | 12.04 |
| With oil | 22.73 | With oil | 7.33 | |
| Change | −46.99% | Change | −64.26% | |
| Average value | No oil | 3.99 | No oil | 0.61 |
| With oil | 2.64 | With oil | 0.38 | |
| Change | −50.88% | Change | −59.02% | |
| Kurtosisvalue | No oil | 1.62 | No oil | 18.52 |
| With oil | 1.84 | With oil | 17.33 | |
| Change | −11.80% | Change | −6.91% | |
| Skewness Value | No oil | 1.42 | No oil | 3.61 |
| With oil | 1.45 | With oil | 3.38 | |
| Change | −2.13% | Change | −6.79% | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, J.; Zuo, J.; Zhao, W.; Gomi, N.; Zhao, X. Study on Hydraulic Dampers Using a Foldable Inverted Spiral Origami Structure. Vibration 2022, 5, 711-731. https://doi.org/10.3390/vibration5040042
Guan J, Zuo J, Zhao W, Gomi N, Zhao X. Study on Hydraulic Dampers Using a Foldable Inverted Spiral Origami Structure. Vibration. 2022; 5(4):711-731. https://doi.org/10.3390/vibration5040042
Chicago/Turabian StyleGuan, Jingchao, Jingshun Zuo, Wei Zhao, Nobuyuki Gomi, and Xilu Zhao. 2022. "Study on Hydraulic Dampers Using a Foldable Inverted Spiral Origami Structure" Vibration 5, no. 4: 711-731. https://doi.org/10.3390/vibration5040042
APA StyleGuan, J., Zuo, J., Zhao, W., Gomi, N., & Zhao, X. (2022). Study on Hydraulic Dampers Using a Foldable Inverted Spiral Origami Structure. Vibration, 5(4), 711-731. https://doi.org/10.3390/vibration5040042







