A Shake Table Frequency-Time Control Method Based on Inverse Model Identification and Servoactuator Feedback-Linearization
Abstract
1. Introduction
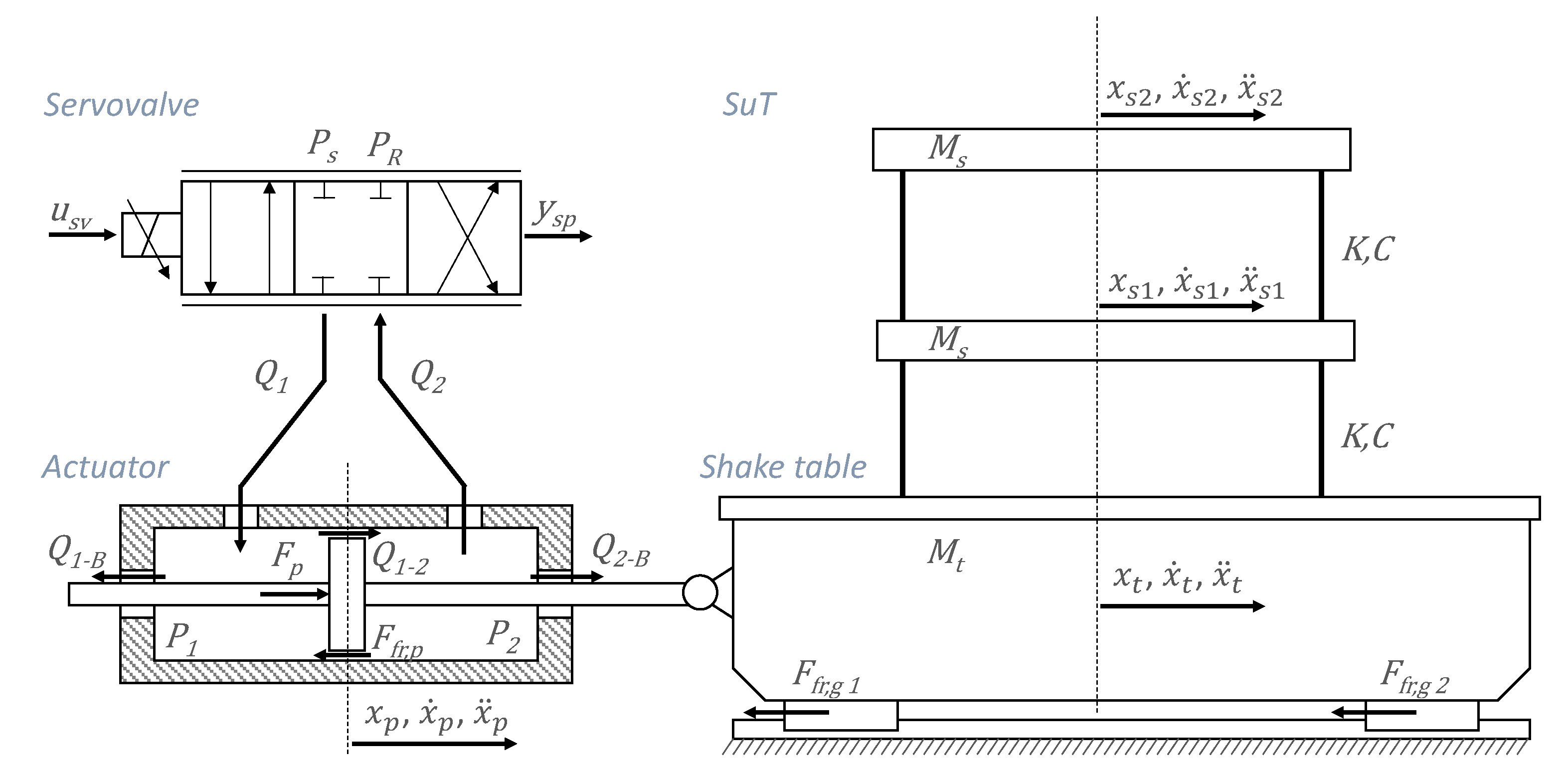
2. Shake Table System Modeling
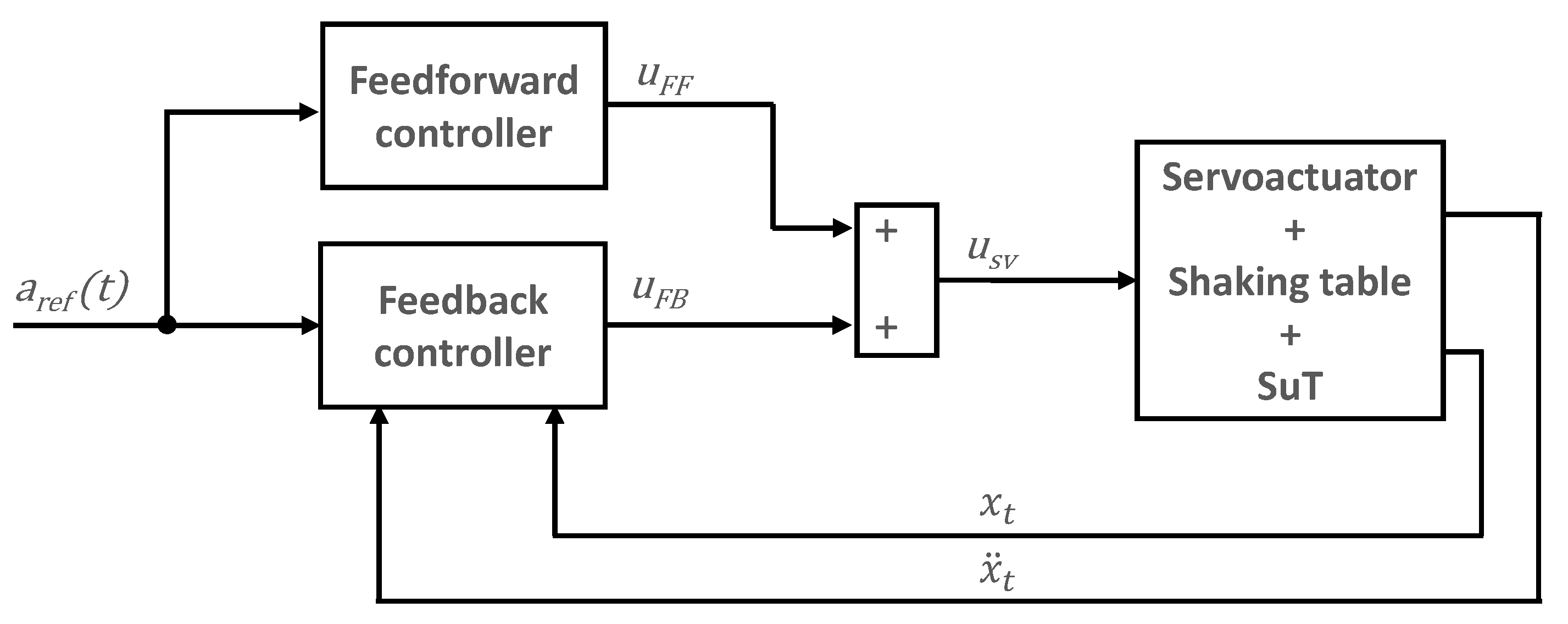
3. Description of the Proposed Control Methodology
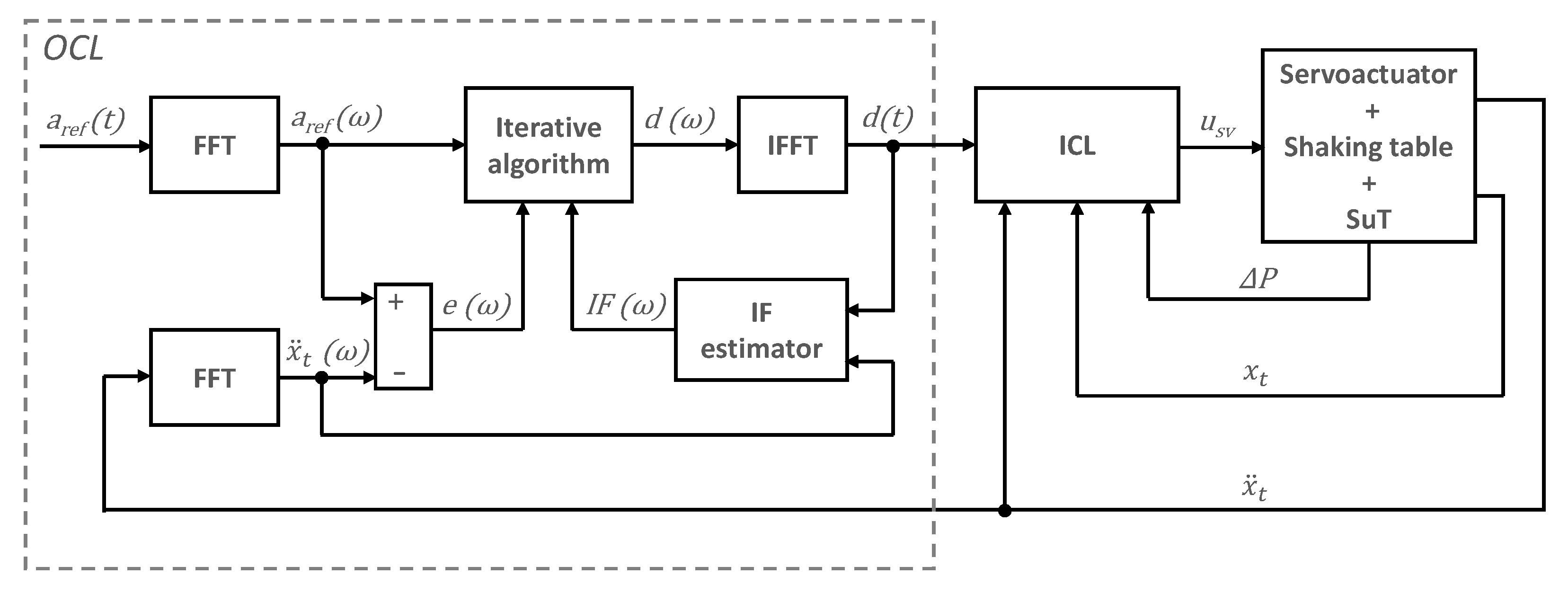
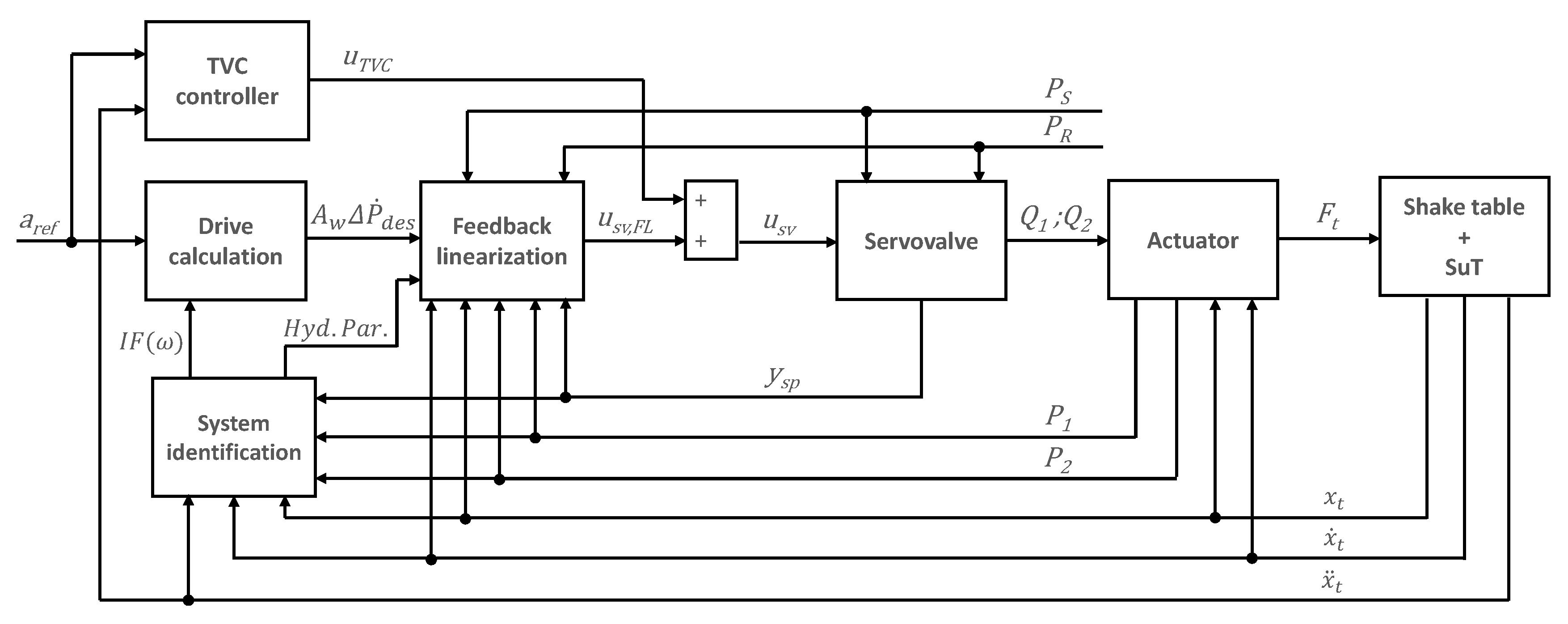
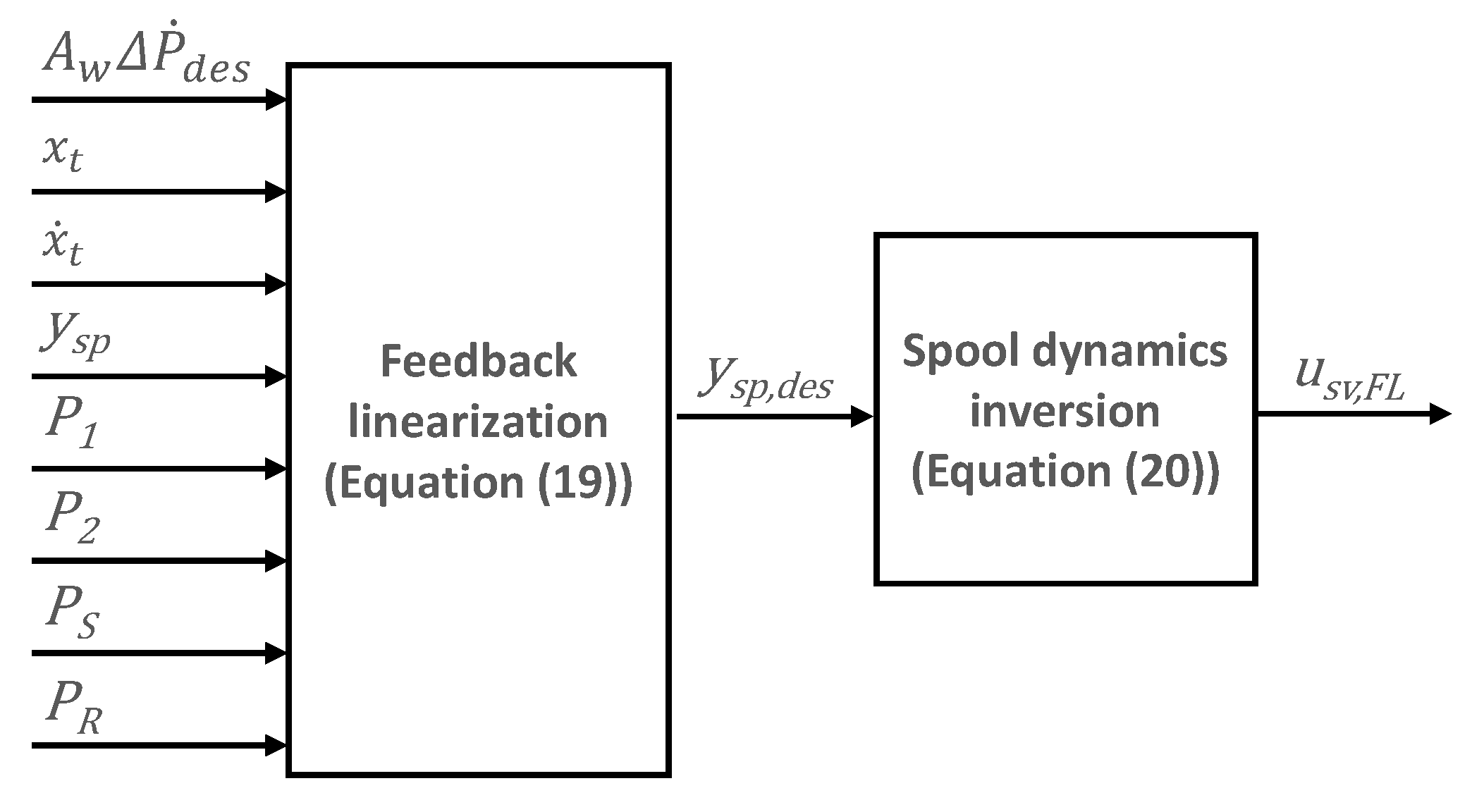
- Feedback linearization. The purpose of this block is to cancel out, at least approximately, the non-linearities inherent to the servovalve-actuator system, leading to a control scheme where the time derivative of the pressure force exerted on the servoactuator’s piston rod can be directly imposed.
- System identification. This module operates when the system is in identification mode, prior to the test itself. It is in charge of: (i) estimating and inverting the Accelerance Function (AF), which later is transformed into a more suitable IF representing the inverse model of the shake table-SuT system, and (ii) obtaining approximations for the values of the hydraulic parameters required by the feedback linearization scheme. It can also be implemented to operate, on a signal block basis, refining identification of IF and system parameters between one signal block and the following, as the test proceeds.
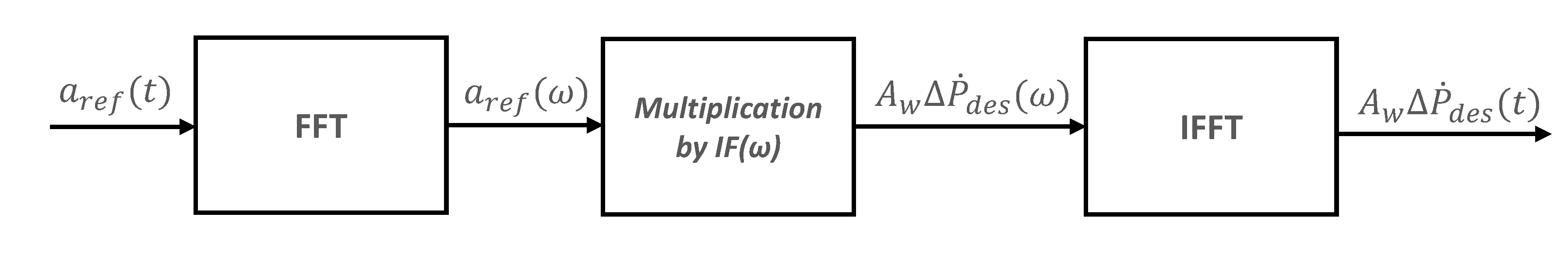
- Drive calculation. This algorithm operates when the system is in test mode, on a signal block basis. It calculates the necessary pressure force time derivative to be applied on servoactuator’s rod by multiplying the IF from the system identification module by the desired acceleration output, in frequency domain, and transforming the result back into time domain.
- TVC controller. This feedback controller is necessary to compensate for the unavoidable imperfections present in the identified inverse model and to ensure overall system stability. It is implemented in parallel with the abovementioned architecture and accounts for errors in displacement, velocity and acceleration tracking in real-time.
3.1. Feedback Linearization
3.2. System Identification
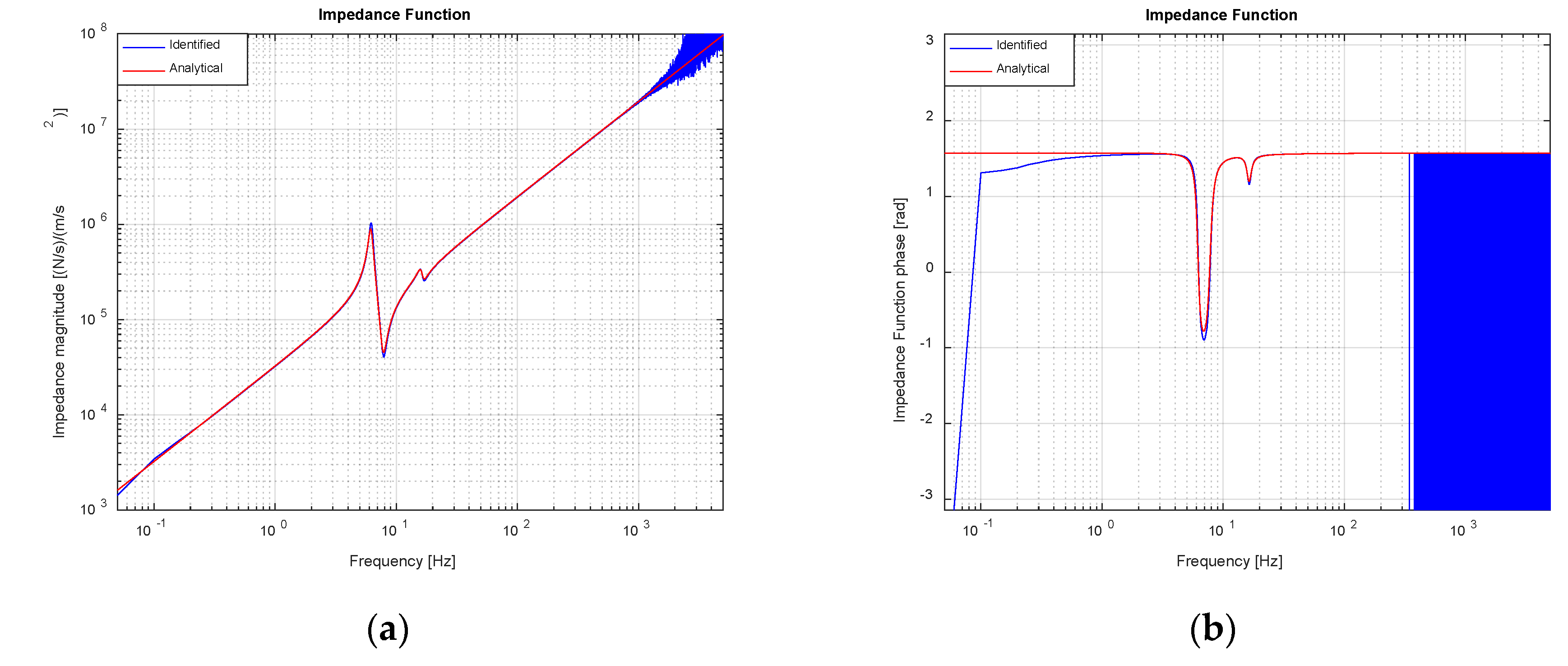
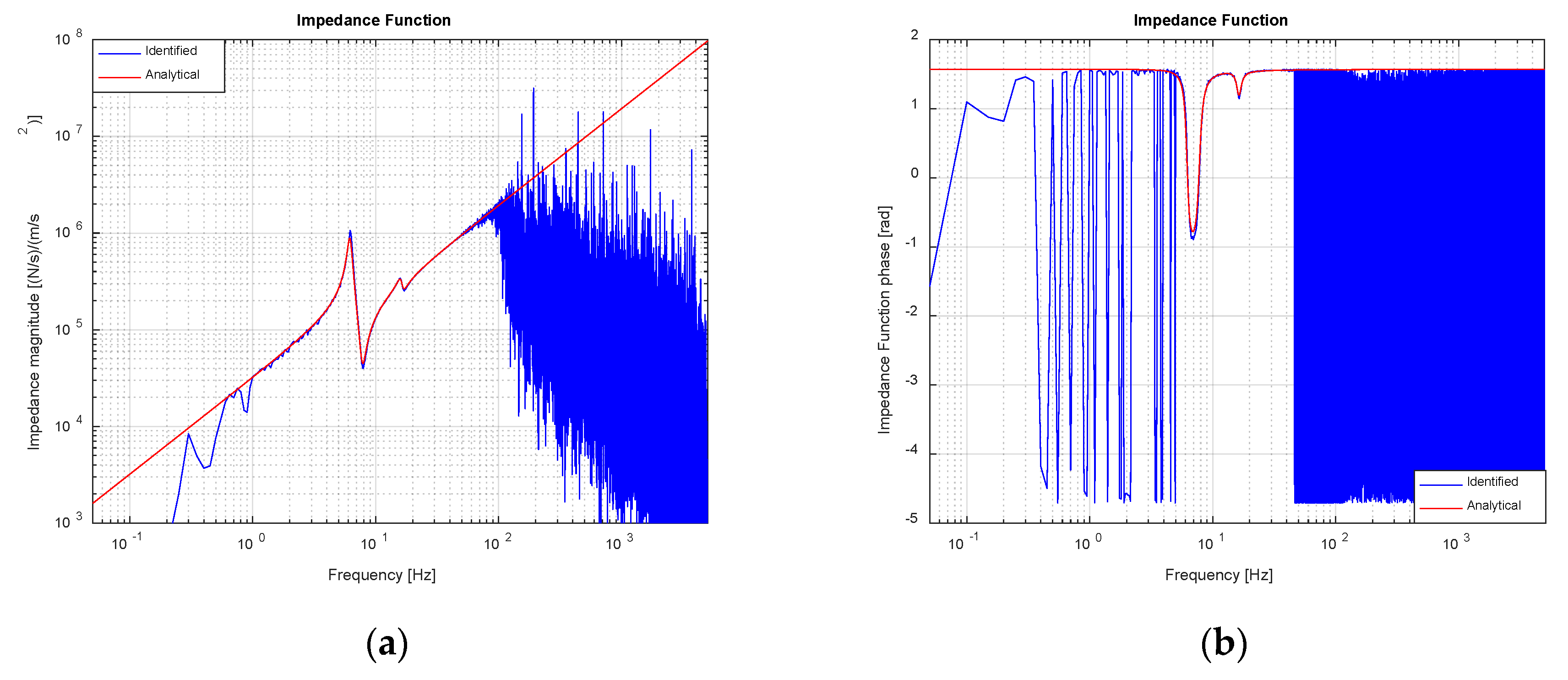
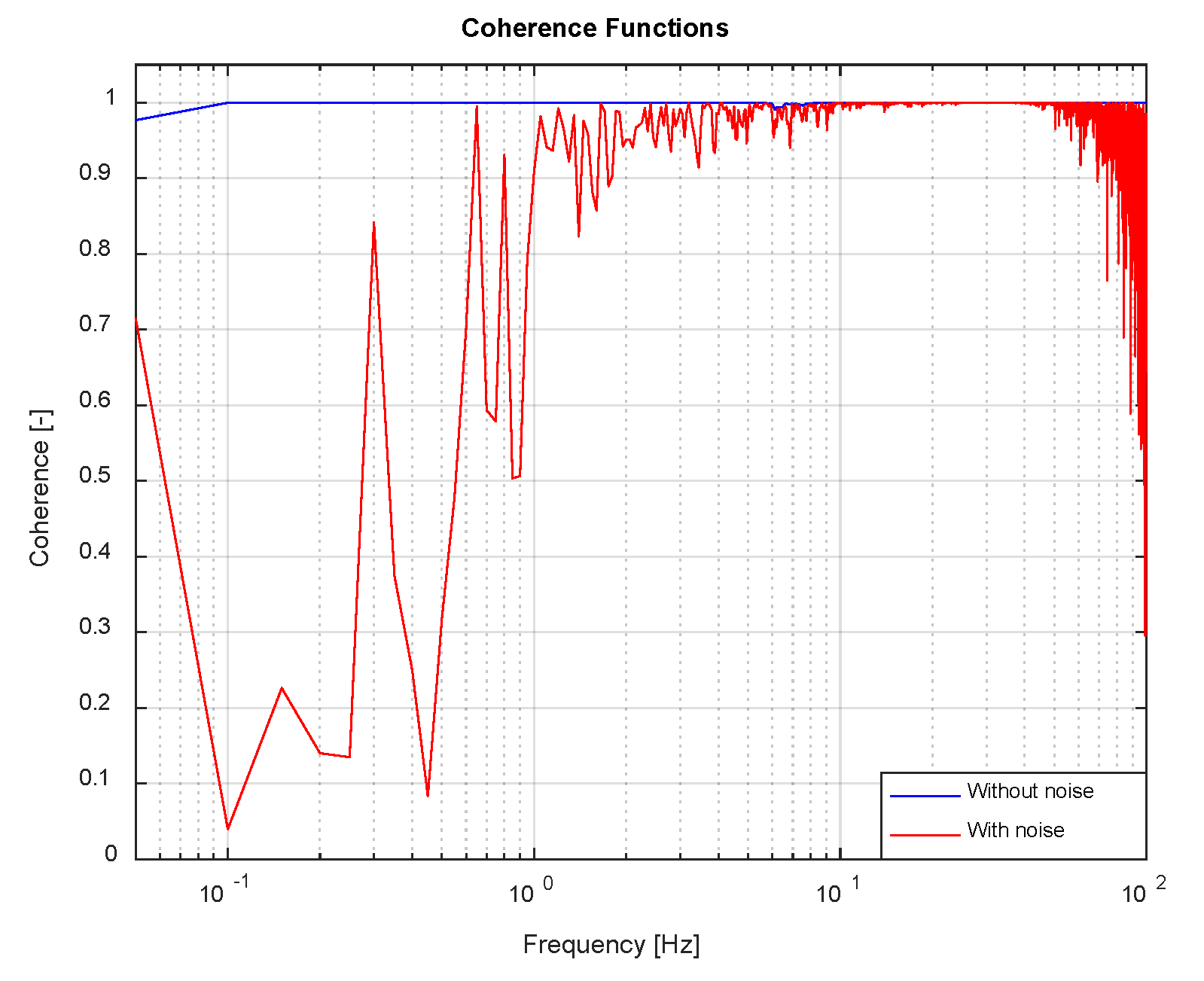
3.2.1. Impedance Function Identification Procedure
3.2.2. Hydraulic Parameters Identification Procedure
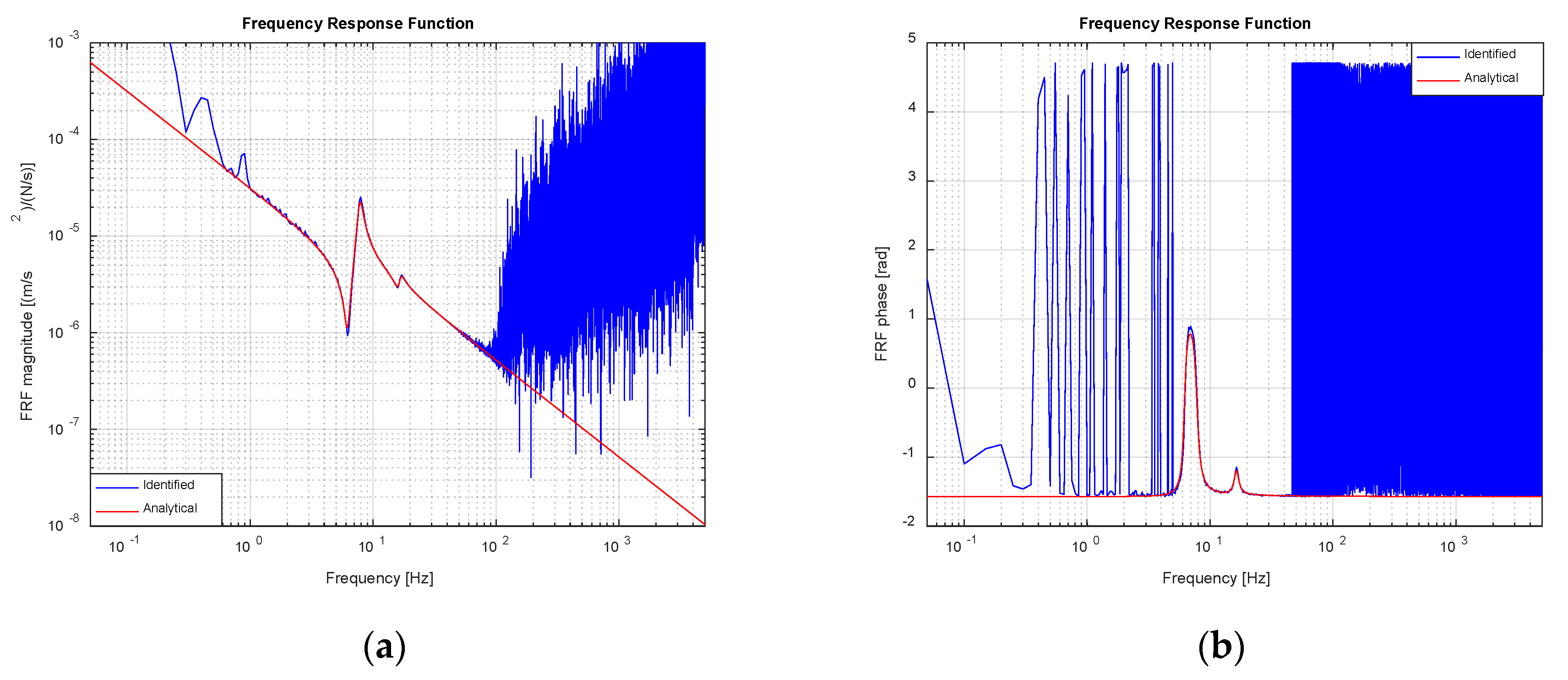
3.3. Drive Calculation Module
3.4. Three Variable Controller
4. Numerical Simulations Results and Control Methods Comparison
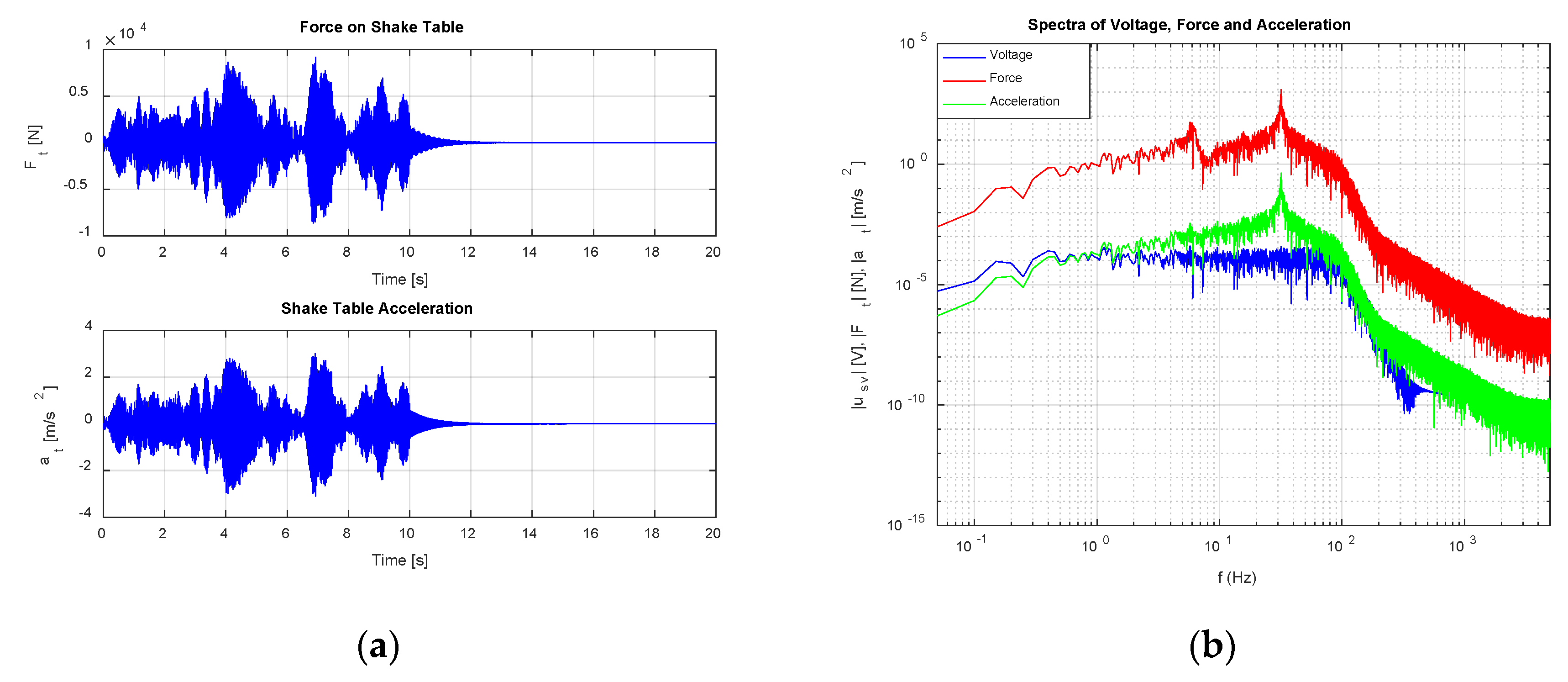
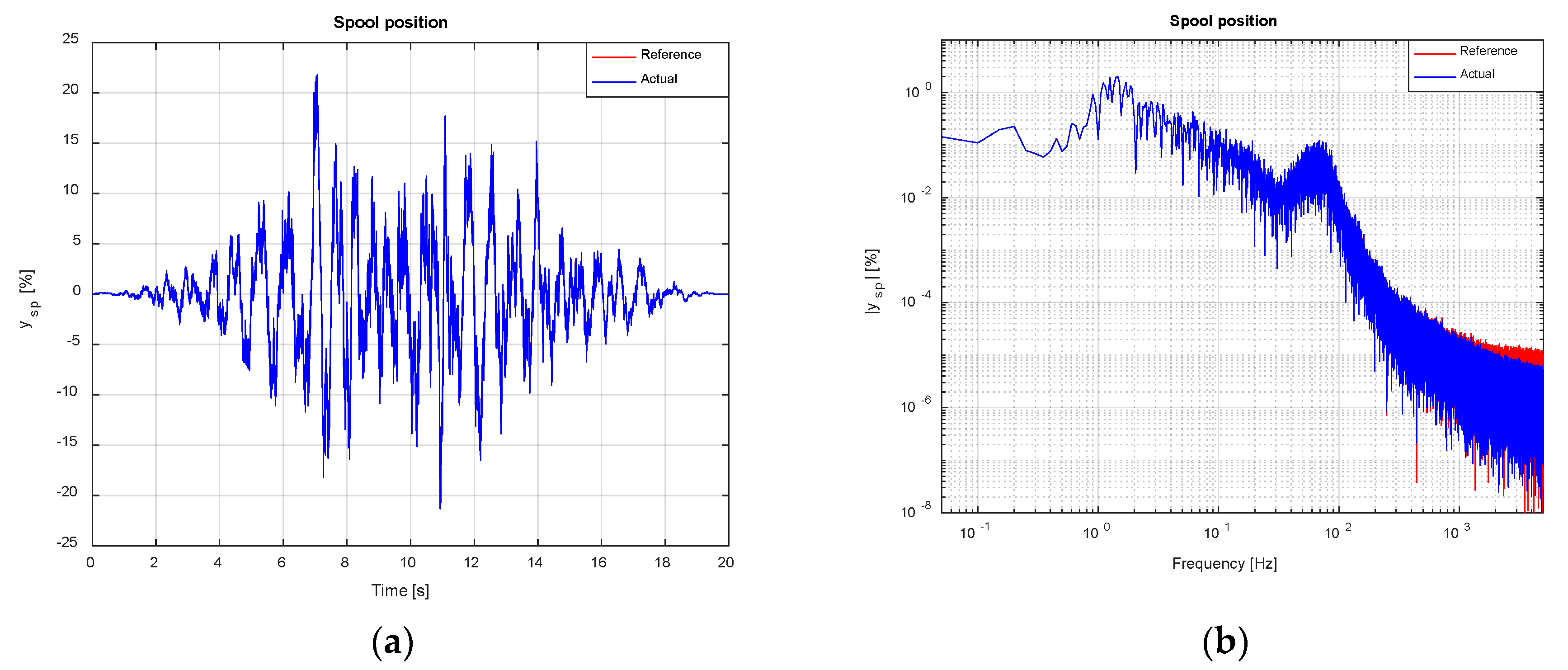
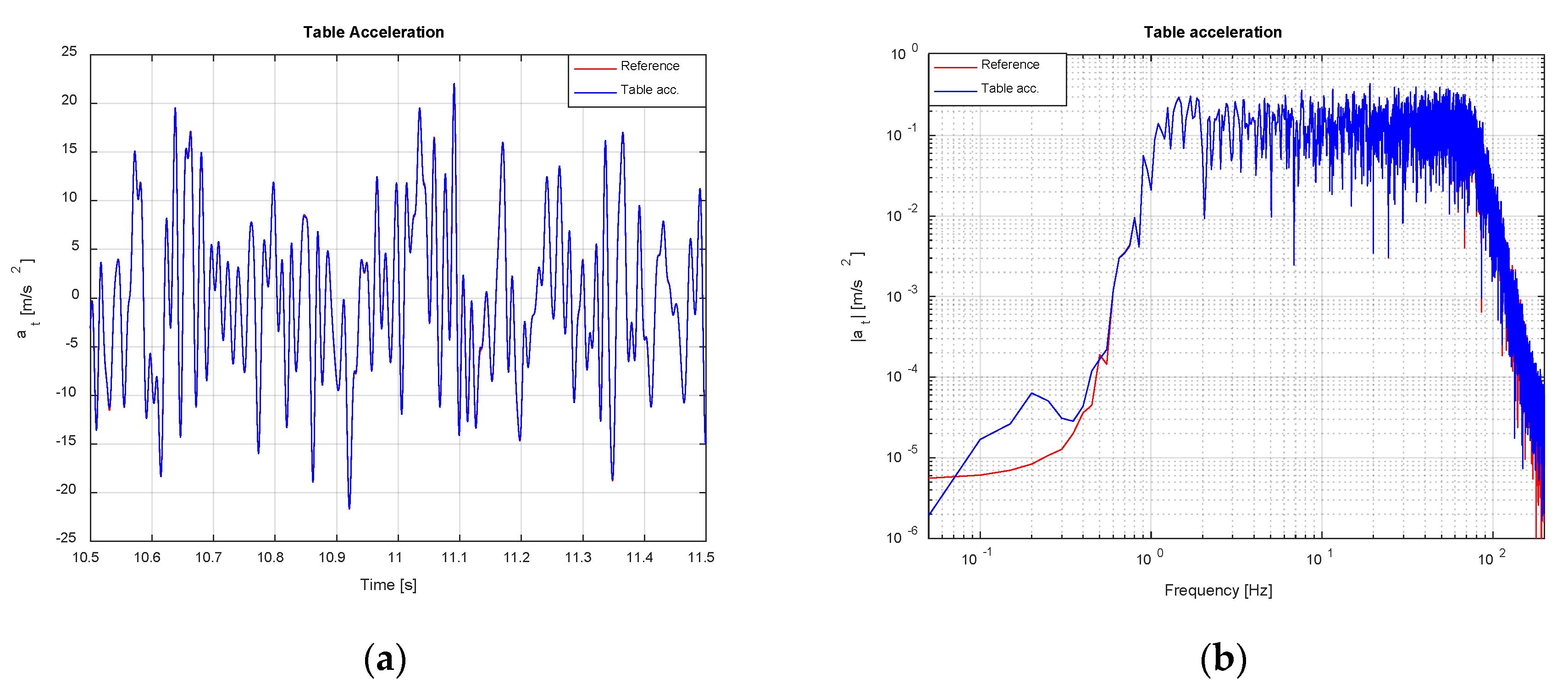
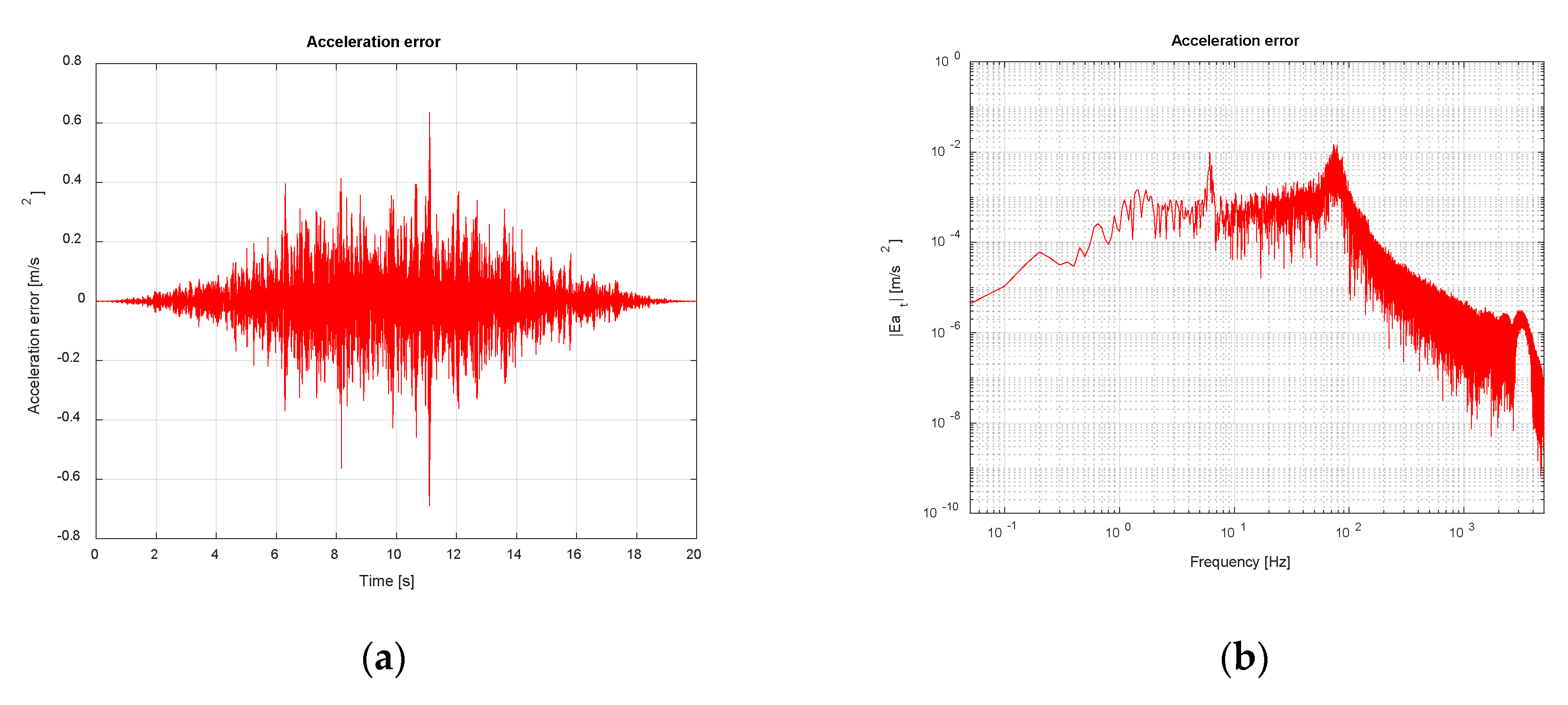
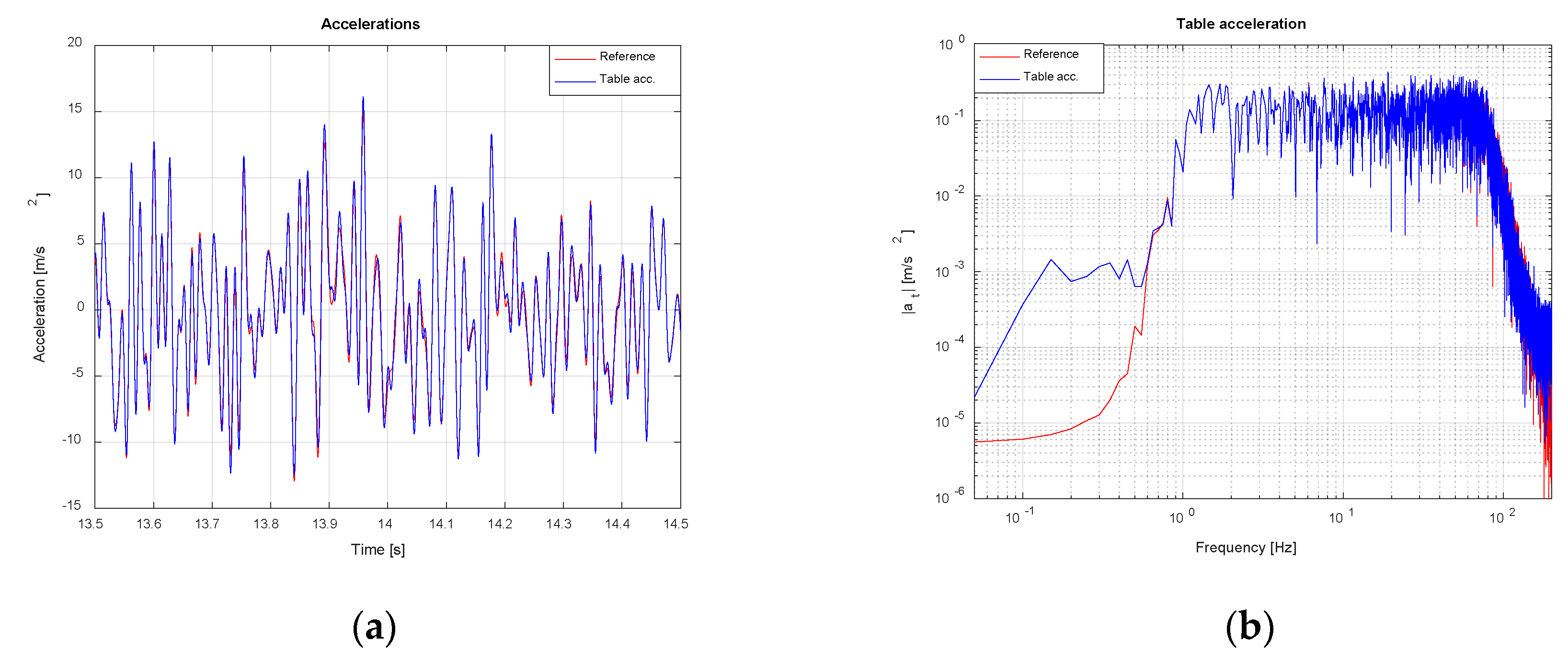
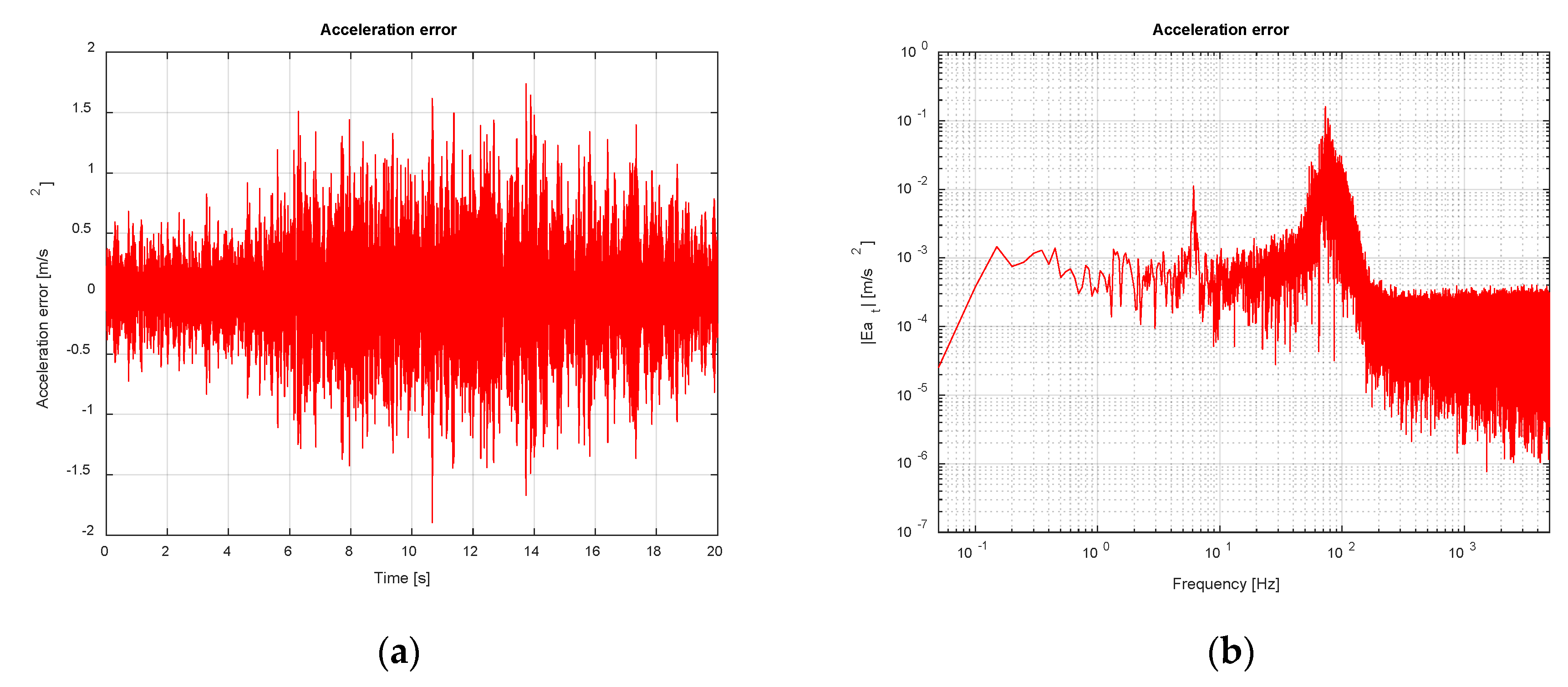
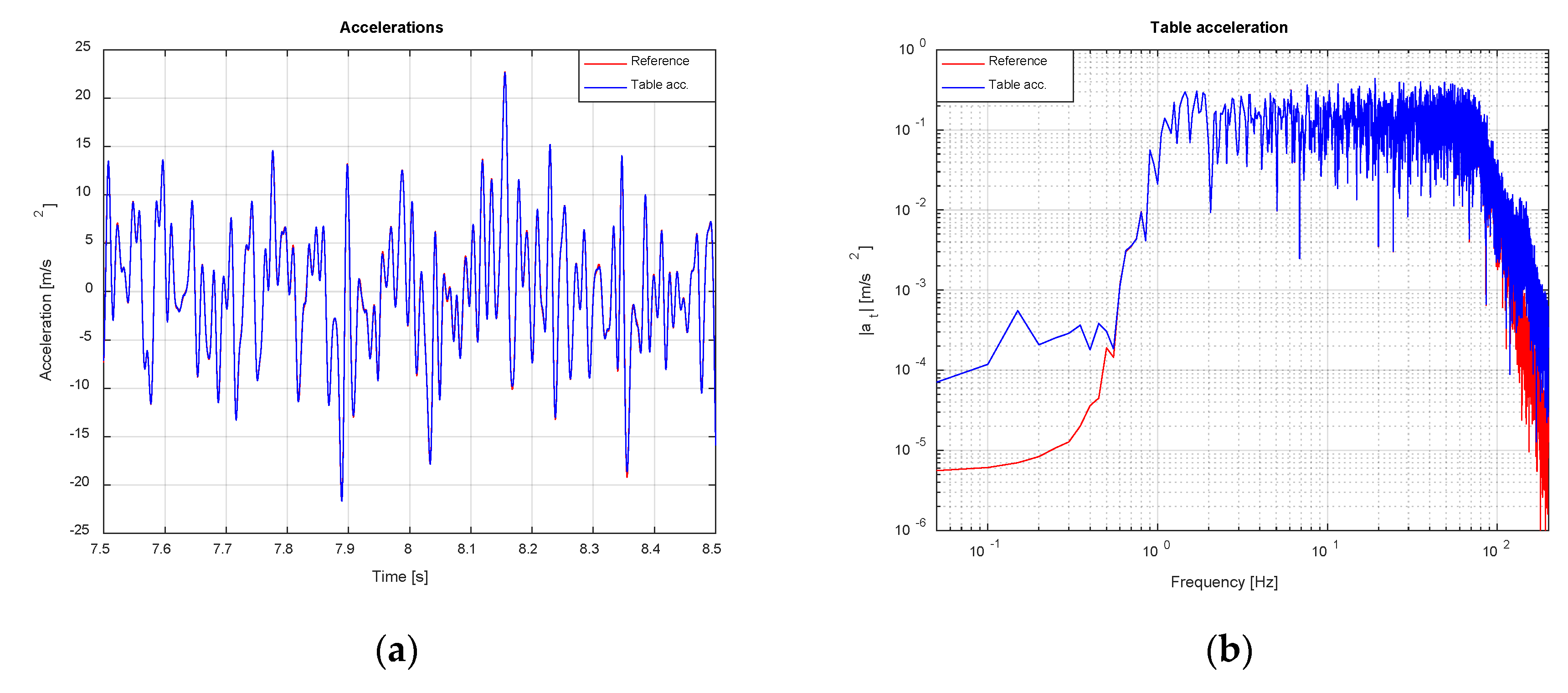
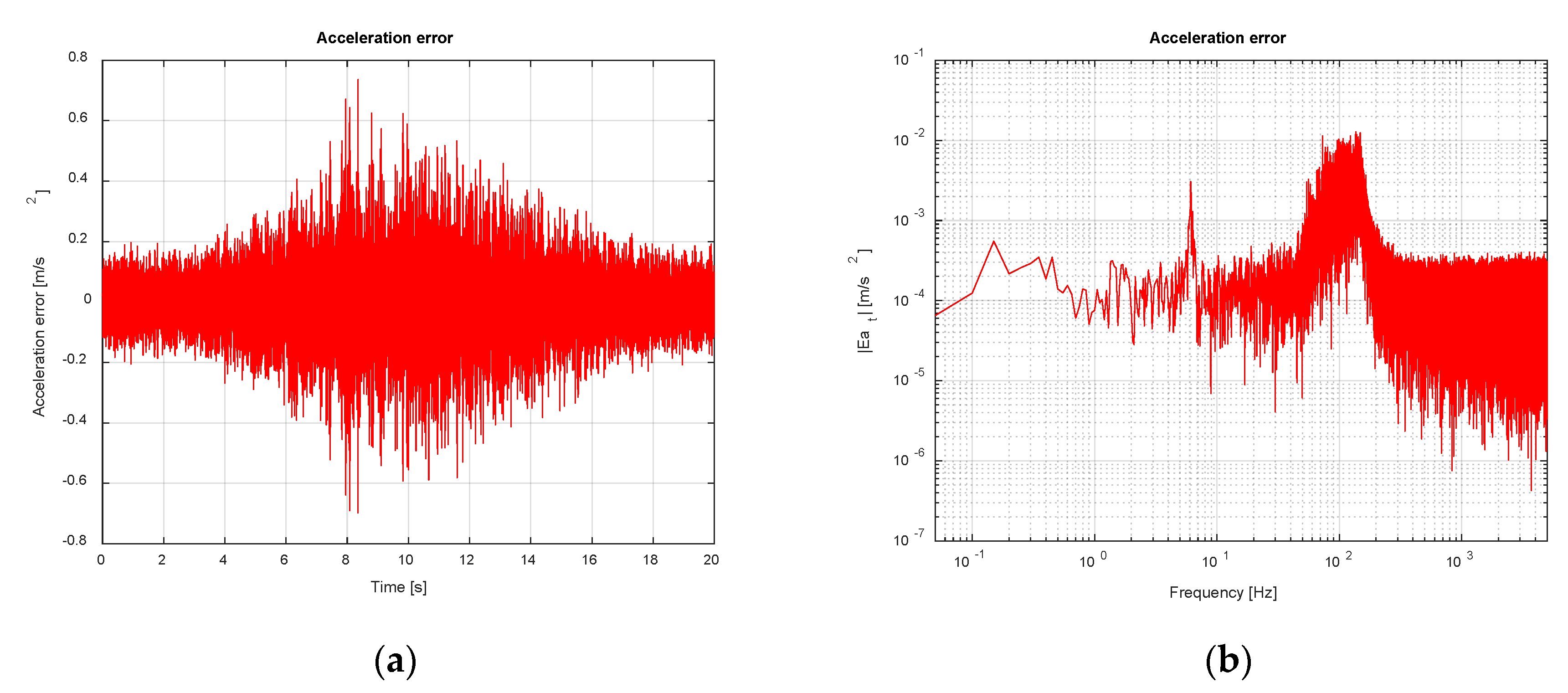
4.1. Numerical Simulations Results
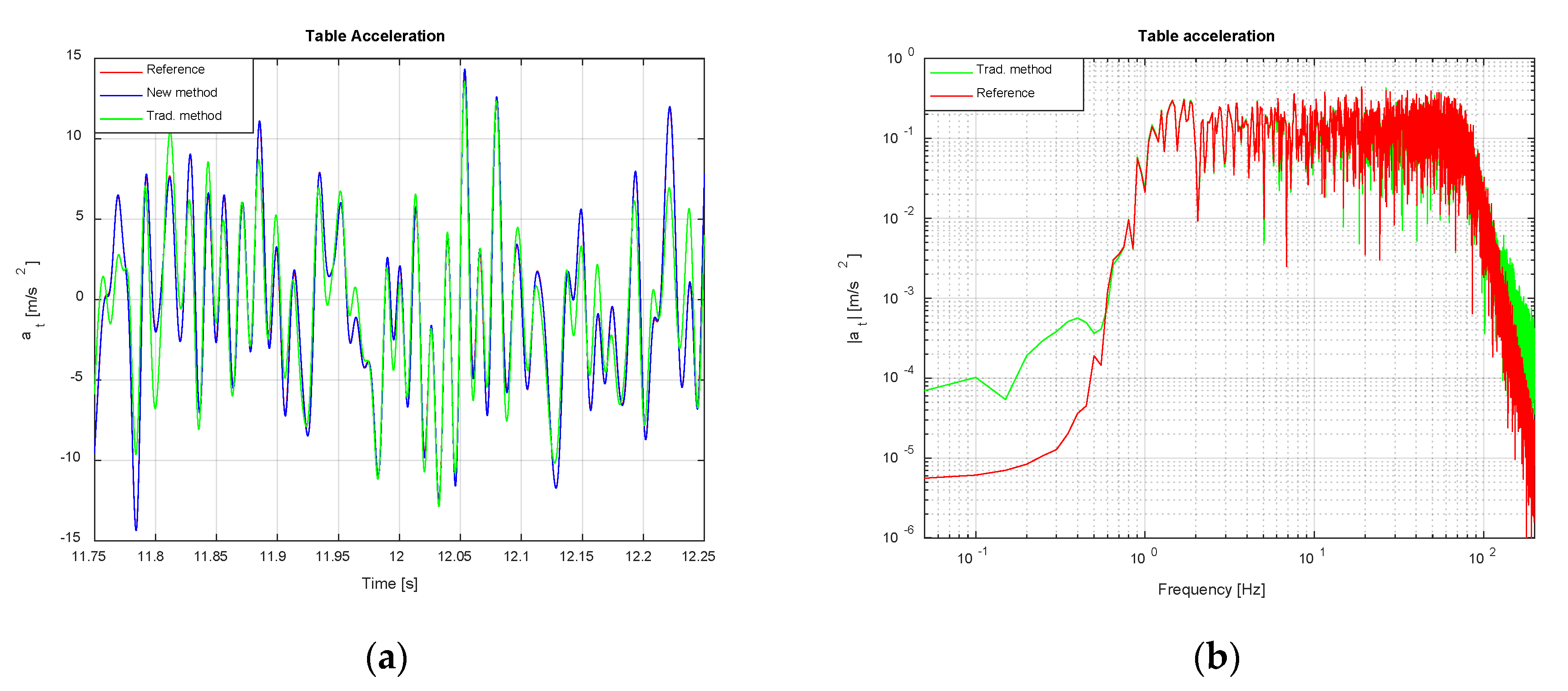
4.2. Comparison between Control Methods
5. Conclusions
- without the parallel TVC feature enabled in an electrical noise free environment;
- with the parallel TVC feature enabled in a noise-free environment;
- with the parallel TVC feature enabled in an electrical noise contaminated environment;
- with the same conditions as in 3. but with a better tuning of TVC parameters.
- Non-linearities present in the purely mechanical system.
- Rigorous studies on the uncertainty in IF and hydraulic parameters estimation and on the effect of noise present in sensors measurements.
- Development of differentiation schemes robust against noise in signals.
- Assessment of the effects of the delay due to control loop and sensors and their effect on feedback linearization scheme.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Williams, M.S.; Blakeborough, A. Laboratory testing of structures under dynamic loads: An introductory review. Philos. Trans. R. Soc. Lond. Ser. A 2001, 359, 1651–1669. [Google Scholar] [CrossRef]
- Füllekrug, U. Utilization of multi-axial shaking tables for the modal identification of structures. Philos. Trans. R. Soc. Lond. Ser. A 2001, 359, 1753–1770. [Google Scholar] [CrossRef]
- Severn, R. The development of shaking tables—A historical note. Earthq. Eng. Struct. Dyn. 2011, 40, 195–213. [Google Scholar] [CrossRef]
- Plummer, A.R. Control techniques for structural testing: A review. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2007, 221, 139–169. [Google Scholar] [CrossRef]
- Bairrão, R.; Vaz, C.T. Shaking Table Testing of Civil Engineering Structures-The LNEC 3D Simulator Experience. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000. [Google Scholar]
- Yao, J.; Dietz, M.; Xiao, R.; Yu, H.; Wang, T.; Yue, D. An overview of control schemes for hydraulic shaking tables. J. Vib. Control 2014, 22, 2807–2823. [Google Scholar] [CrossRef]
- Hanh, D.L.; Ahn, K.K.; Kha, N.B.; Jo, W.K. Trajectory control of electro-hydraulic excavator using fuzzy self tuning algorithm with neural network. J. Mech. Sci. Technol. 2009, 23, 149–160. [Google Scholar] [CrossRef]
- Phillips, B.M.; Wierschem, N.E.; Spencer, B.F. Model based multi-metric control of uniaxial shake tables. Earthq. Eng. Struct. Dyn. 2014, 43, 681–699. [Google Scholar] [CrossRef]
- Najafi, A.; Spencer, B.F. Modified model-based control of shake tables for online acceleration tracking. Earthq. Eng. Struct. Dyn. 2020. [Google Scholar] [CrossRef]
- Plummer, A. Model-based motion control for multi-axis servohydraulic shaking tables. Control Eng. Pract. 2016, 53, 109–122. [Google Scholar] [CrossRef]
- Shen, G.; Zheng, S.T.; Ye, Z.M.; Yang, Z.D.; Zhao, Y.; Han, J.W. Tracking control of an electro-hydraulic shaking table system using a combined feedforward inverse model and adaptive inverse control for real-time testing. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2011, 225, 647–666. [Google Scholar] [CrossRef]
- Nakata, N. Acceleration trajectory tracking control for earthquake simulators. Eng. Struct. 2014, 32, 2229–2236. [Google Scholar] [CrossRef]
- Seki, K.; Iwasaki, M.; Kawafuku, M.; Hirai, H.; Yasuda, K. Improvement of control performance in shaking-tables by feedback compensation for reaction force. In Proceedings of the 34th Annual Conference of the IEEE Industrial Electronics Society, Orlando, FL, USA, 10–13 November 2008; IEEE: New York, NY, USA, 2008; pp. 2551–2556. [Google Scholar] [CrossRef]
- Yao, J.; Di, D.T.; Jiang, G.L.; Gao, S. Acceleration amplitude-phase regulation for electro-hydraulic servo shaking table based on LMS adaptive filtering algorithm. Int. J. Control 2012, 85, 1581–1592. [Google Scholar] [CrossRef]
- Yao, J.; Hu, S.H.; Fu, W.; Han, J.W. Harmonic cancellation for electro-hydraulic servo shaking table based on LMS adaptive algorithm. J. Vib. Control 2011, 17, 1862–1868. [Google Scholar] [CrossRef]
- Nowak, R.F.; Kusner, D.A.; Larson, R.L.; Thoen, B.K. Utilizing modern digital signal processing for improvement of large scale shaking table performance. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000; pp. 2035–2042. [Google Scholar]
- Tagawa, Y.; Kajiwara, K. Controller development for the E-Defense shaking table. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2007, 221, 171–181. [Google Scholar] [CrossRef]
- Stoten, D.P.; Gómez, E.G. Adaptive control of shaking tables using the minimal control synthesis algorithm. Philos. Trans. R. Soc. Lond. Ser. A 2001, 359, 1697–1723. [Google Scholar] [CrossRef]
- Stoten, D.P.; Shimizu, N. The feedforward minimal control synthesis algorithm and its application to the control of shaking-tables. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2007, 221, 423–444. [Google Scholar] [CrossRef]
- De Cuyper, J.; Coppens, D.; Liefooghe, C.; Debille, J. Advanced system identification methods for improved service load simulation on multi-axial test rigs. Eur. J. Mech. Environ. Eng. 1999, 44, 27–39. [Google Scholar]
- Zhao, J.; Catharine, C.S.; Posbergh, T. Nonlinear system modeling and velocity feedback compensation for effective force testing. J. Eng. Mech. 2005, 131, 244–253. [Google Scholar] [CrossRef]
- Merrit, H.E. Hydraulic Control. Systems, 1st ed.; John Wiley & Sons: New York, NY, USA, 1991; pp. 76–100. [Google Scholar]
- Servovalves with Integrated Electronics D791 and D792 Series. Available online: www.moog.com/content/dam/moog/literature/ICD/Moog-Valves-D791_D792-Catalog-en.pdf (accessed on 24 September 2020).
- Stoten, D.P. Fusion of kinetic data using composite filters. Proc. Inst. Mech. Eng. Part. I J. Syst. Control. Eng. 2001, 215, 483–497. [Google Scholar] [CrossRef]
- Plummer, A.R. Optimal complementary filters and their application in motion measurement. Proc. Inst. Mech. Eng. Part. I J. Syst. Control. Eng. 2006, 6, 489–507. [Google Scholar] [CrossRef]
- Kwatny, H.G.; Blankenship, G.L. Nonlinear Control and Analytical Mechanics: A Computational Approach, 1st ed.; Birkenhäuser: Boston, MA, USA, 2000; pp. 185–201. [Google Scholar]
- Slotine, J.E.; Li, W. Applied Nonlinear Control., 1st ed.; Prentice-Hall, Inc.: Englewood Cliffs, NJ, USA, 1991; pp. 207–265. [Google Scholar]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes in Fortran. In The Art of Scientific Computing, 2nd ed.; Cambridge University Press: New York, NY, USA, 1992; pp. 180–184. [Google Scholar]
- Leclere, Q.; Roozen, B.; Sandier, C. On the use of the Hs estimator for the experimental assessment of transmissibility matrices. Mech. Syst. Signal. Process. 2014, 43, 237–245. [Google Scholar] [CrossRef]



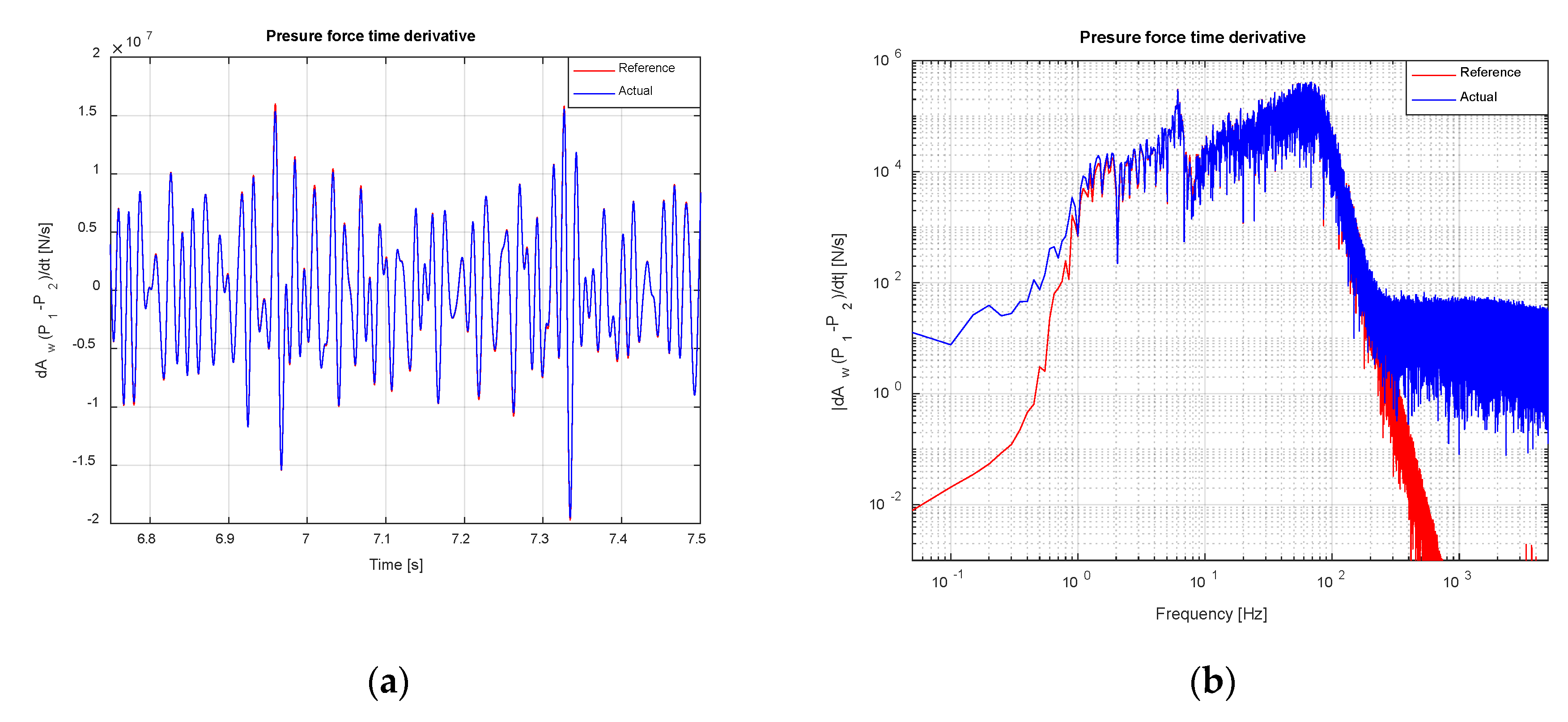
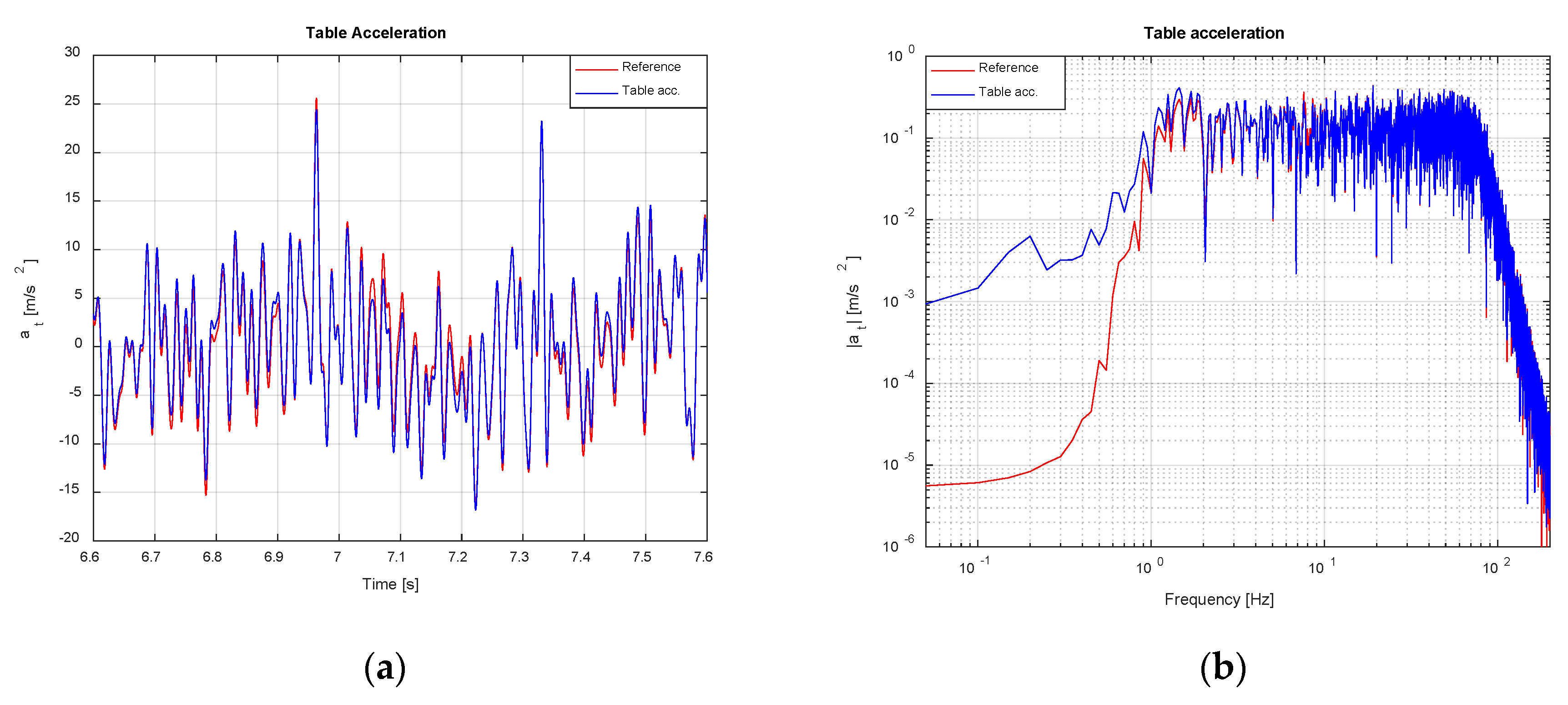
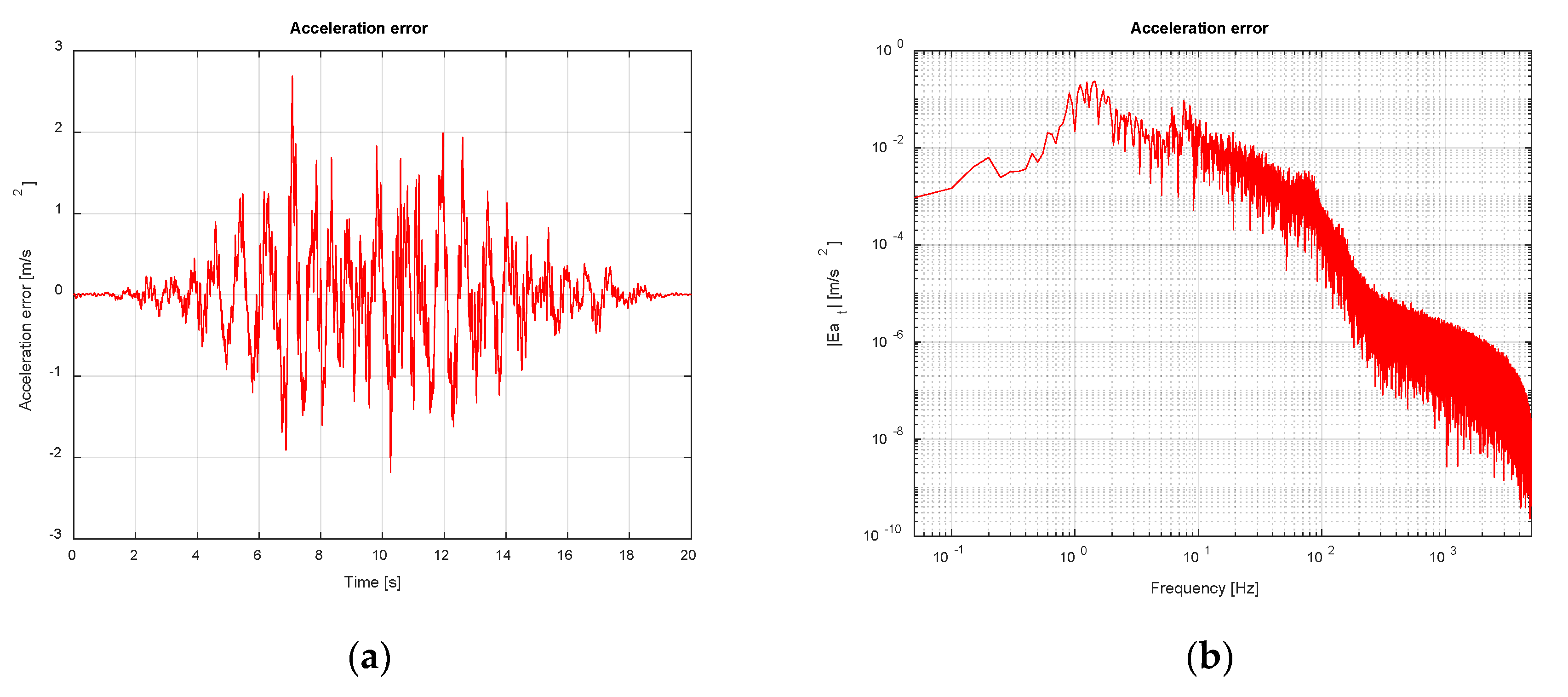
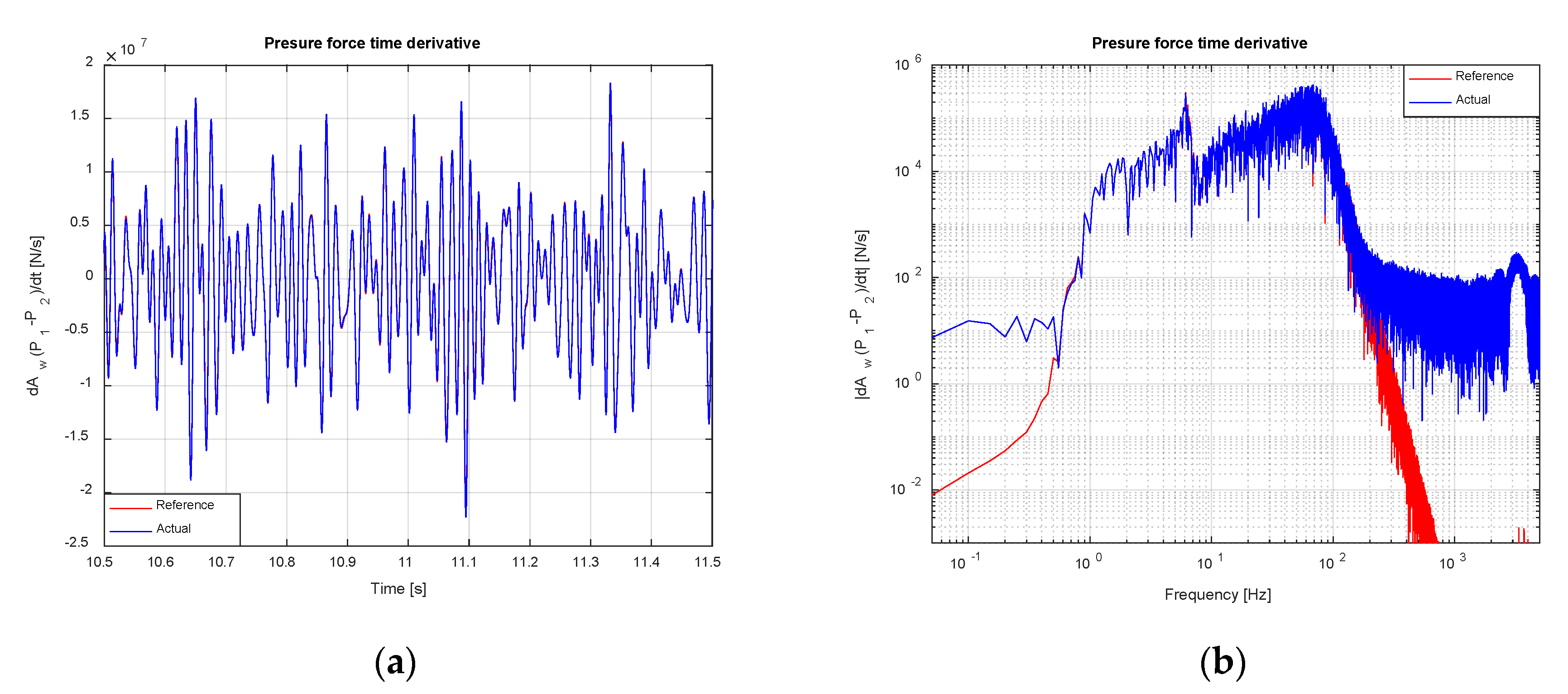


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| (m2) | (m) | ||
| (MPa) 1 | K (N/m) | ||
| (Ns/m) | (kg) | 8.0000 | |
| (-) | (kg) | ||
| (m/V) | (kg) | ||
| (s) | (MPa) | ||
| (m/s2/V) | (MPa) | 0 | |
| (m/V) | (kg/m3) | ||
| (Pa/V) | (s) | ||
| (%/V) | (V) | ||
| (V/V) | (V) | ||
| (-) | (m3) 1 |
| Parameter | Model Value (S.I. Units) | Identified Value without Noise (S.I. Units) | Relative Error without Noise (%) | Identified Value with Noise (S.I. Units) | Relative Error with Noise (%) |
|---|---|---|---|---|---|
| 3.5635 | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Senent, J.R.; García-Palacios, J.H.; Díaz, I.M. A Shake Table Frequency-Time Control Method Based on Inverse Model Identification and Servoactuator Feedback-Linearization. Vibration 2020, 3, 425-447. https://doi.org/10.3390/vibration3040027
Senent JR, García-Palacios JH, Díaz IM. A Shake Table Frequency-Time Control Method Based on Inverse Model Identification and Servoactuator Feedback-Linearization. Vibration. 2020; 3(4):425-447. https://doi.org/10.3390/vibration3040027
Chicago/Turabian StyleSenent, José Ramírez, Jaime H. García-Palacios, and Iván M. Díaz. 2020. "A Shake Table Frequency-Time Control Method Based on Inverse Model Identification and Servoactuator Feedback-Linearization" Vibration 3, no. 4: 425-447. https://doi.org/10.3390/vibration3040027
APA StyleSenent, J. R., García-Palacios, J. H., & Díaz, I. M. (2020). A Shake Table Frequency-Time Control Method Based on Inverse Model Identification and Servoactuator Feedback-Linearization. Vibration, 3(4), 425-447. https://doi.org/10.3390/vibration3040027







