Effect of Initial Temperature and Pressure on the Explosion Characteristics and Intermediate Reaction Products of Formic Acid Mixtures: A Theoretical Study
Abstract
1. Introduction
| HCOOH(l) ↔ H2(g) + CO2(g) | ΔG° = −32.9 kJ/mol |
| HCOOH(l) ↔ H2O(l) + CO(g) | ΔG° = −12.4 kJ/mol |
2. Numerical Studies
3. Results and Discussion
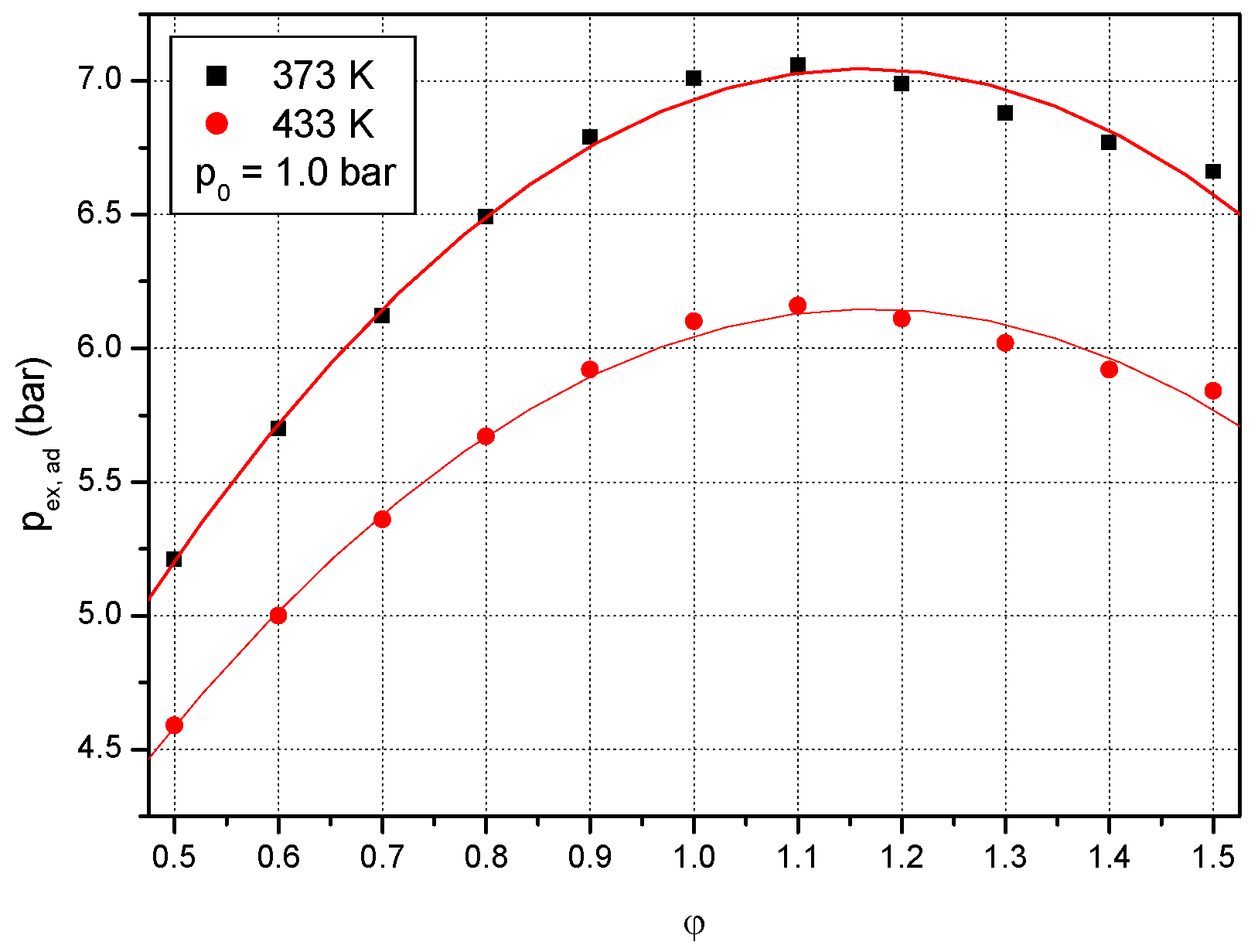
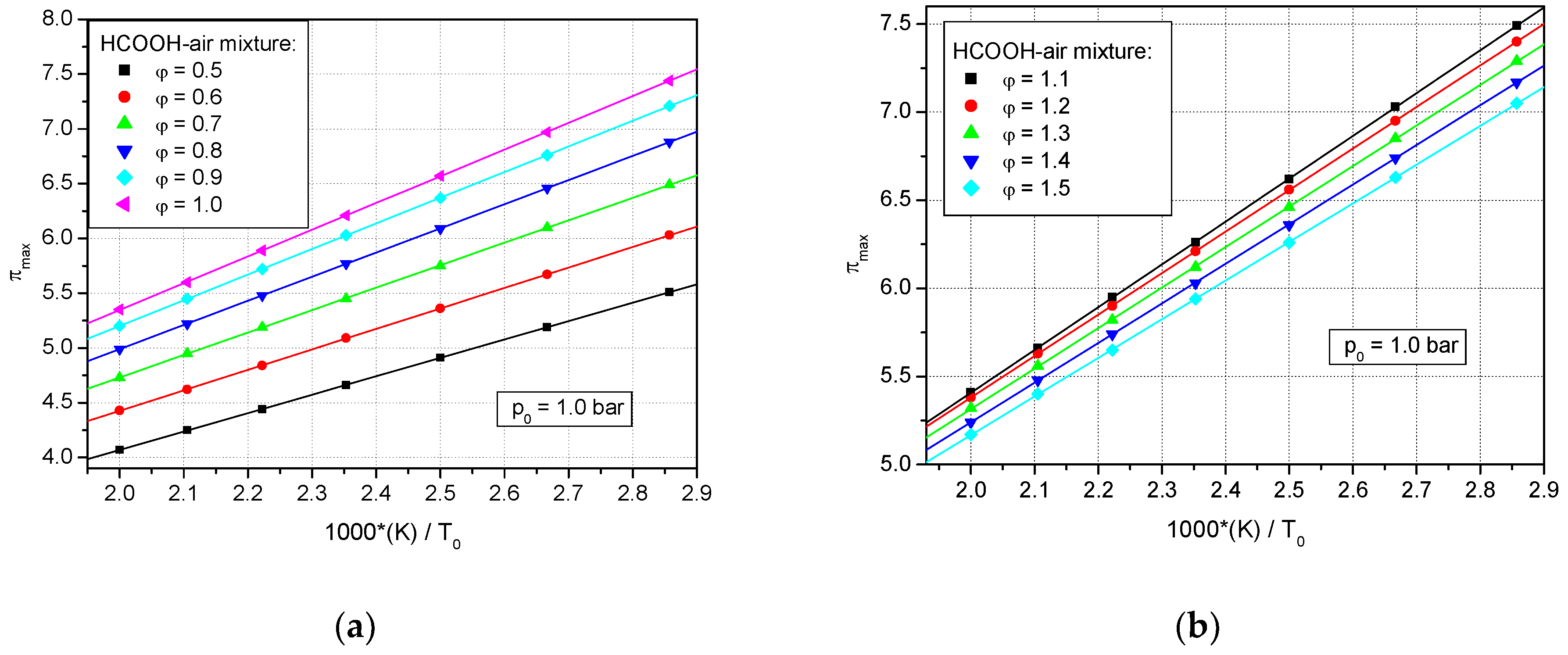
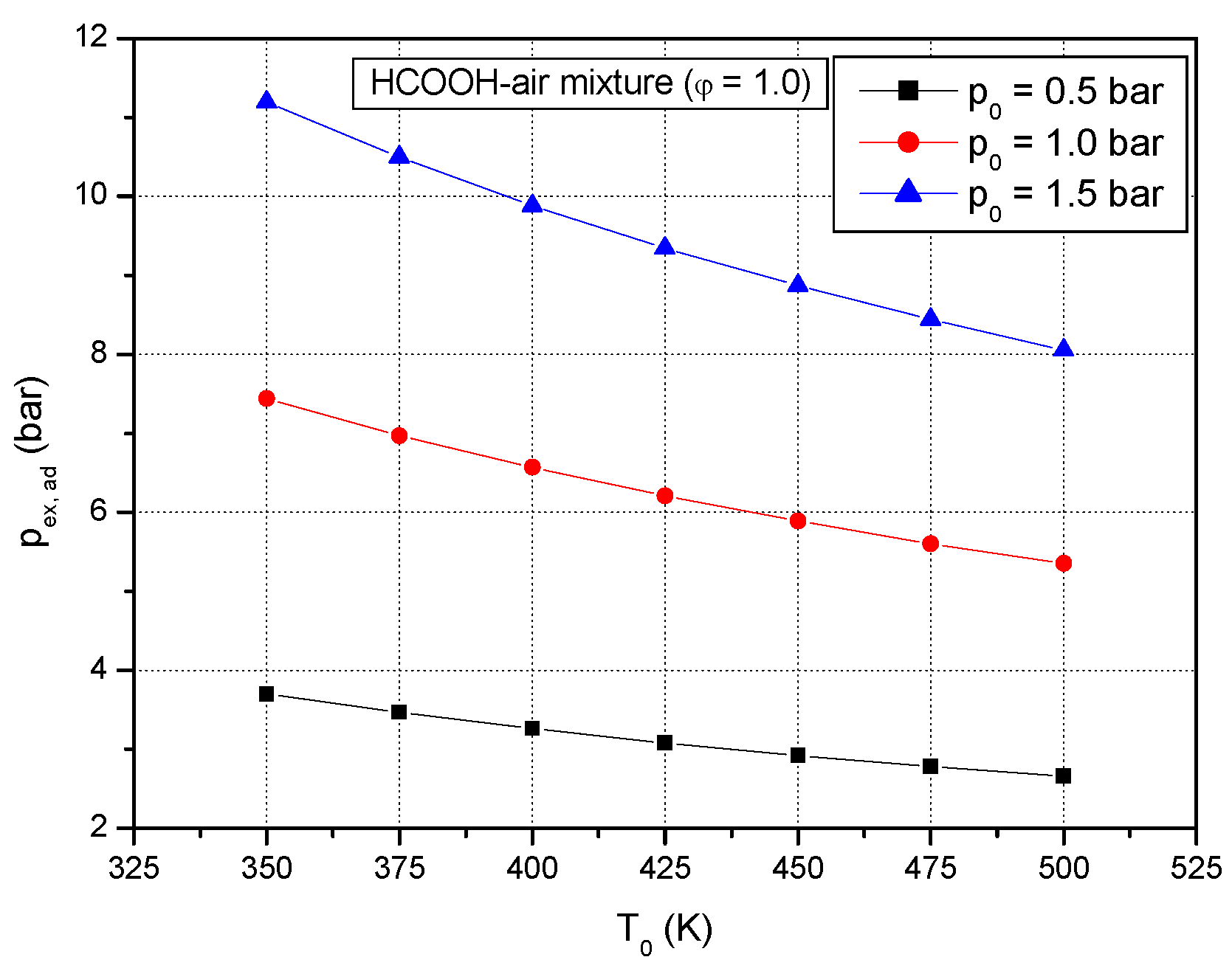
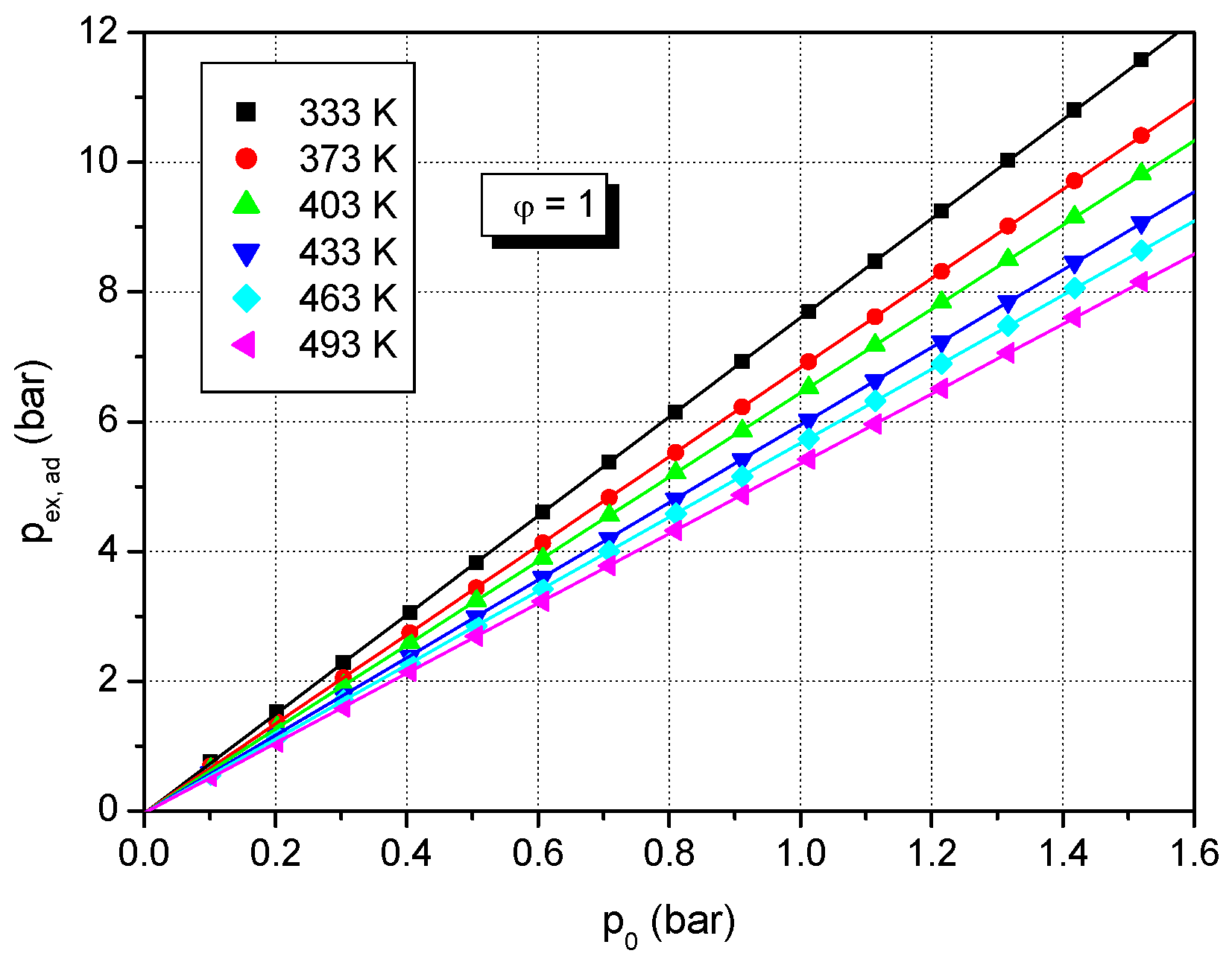
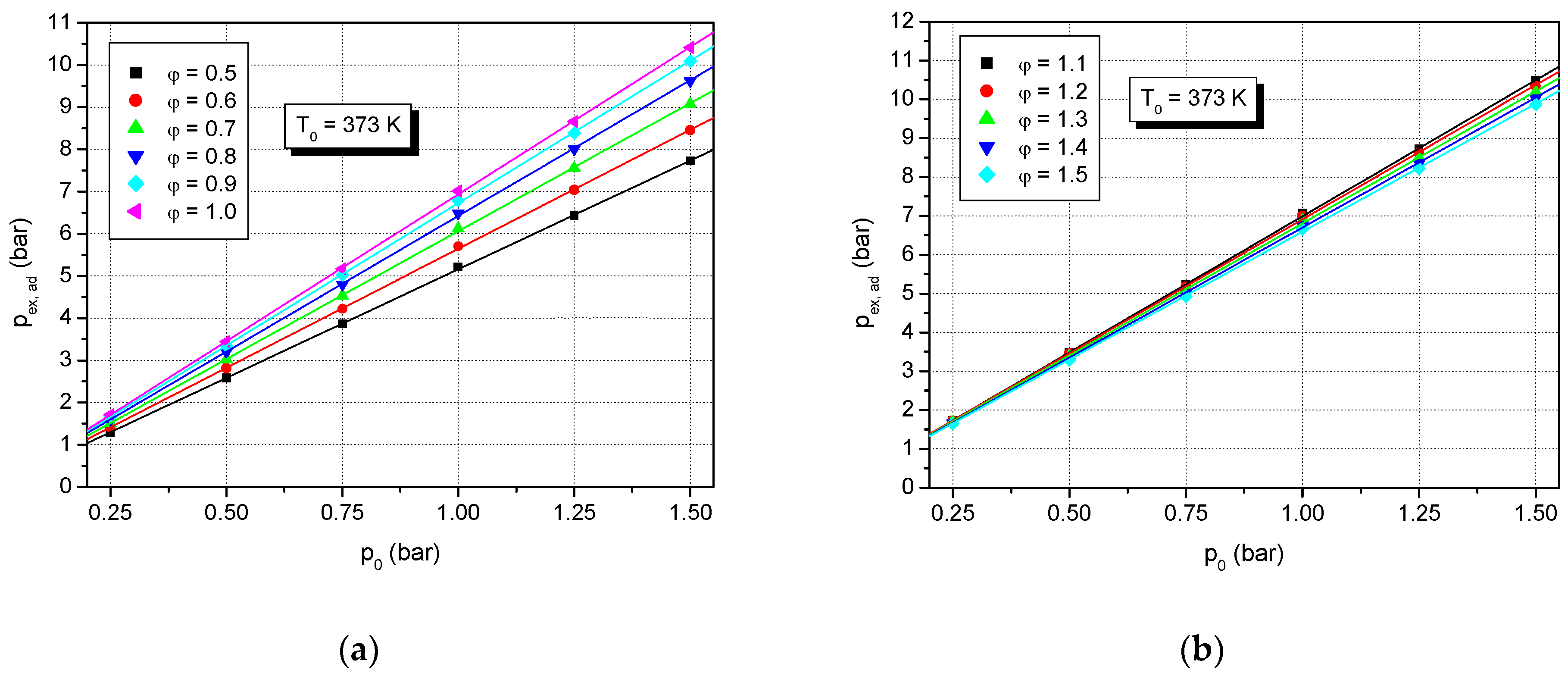
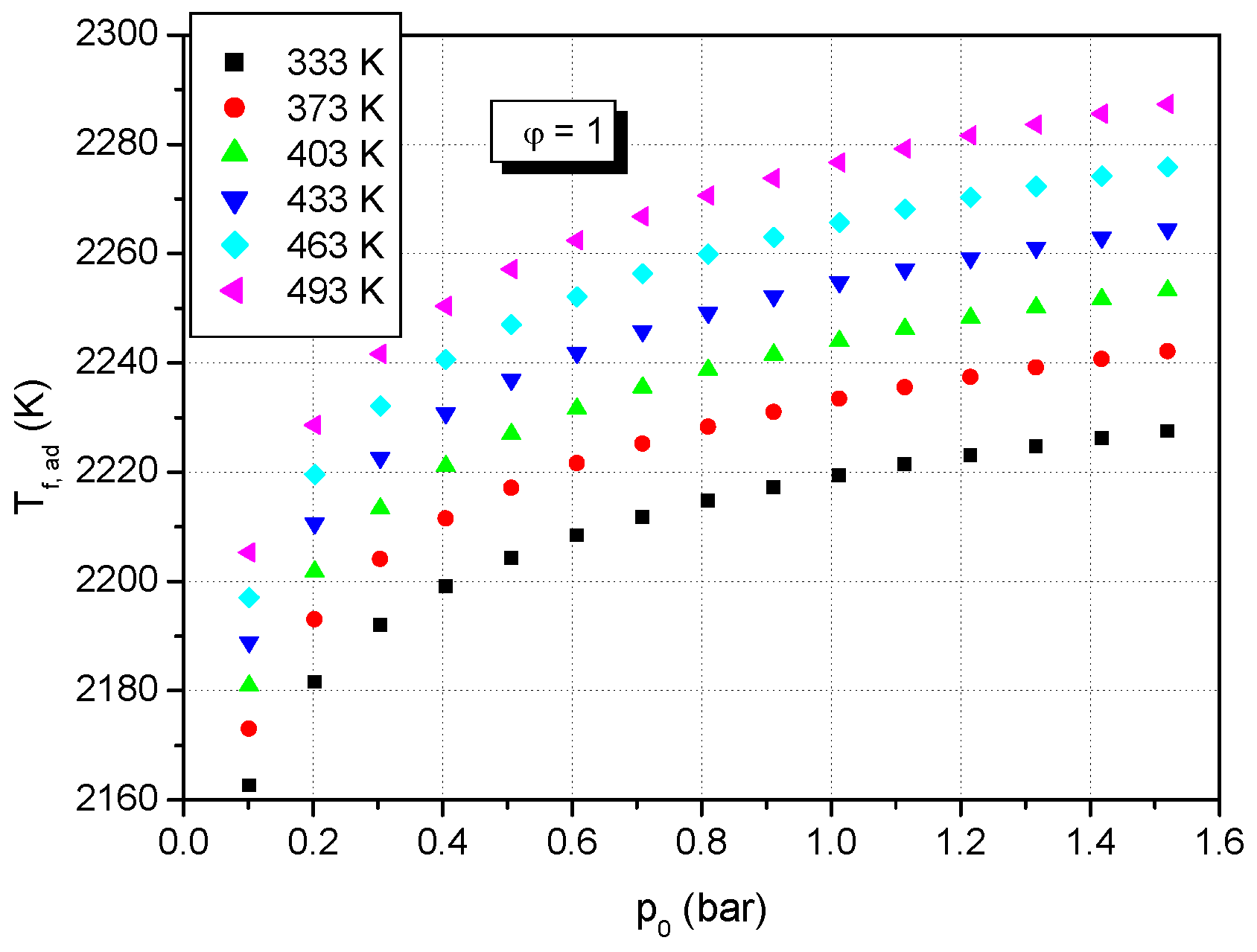
3.1. Influence of the Initial Pressure, Temperature, and Concentration on the Adiabatic Peak Explosion Pressures

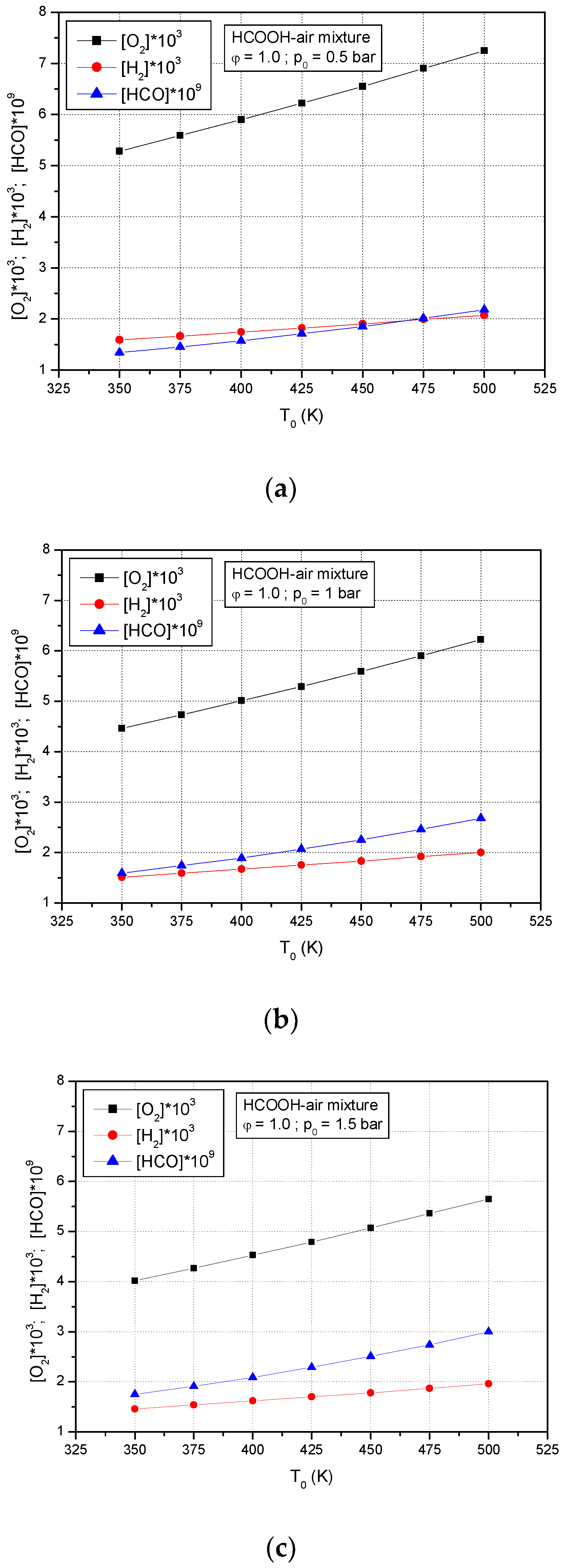
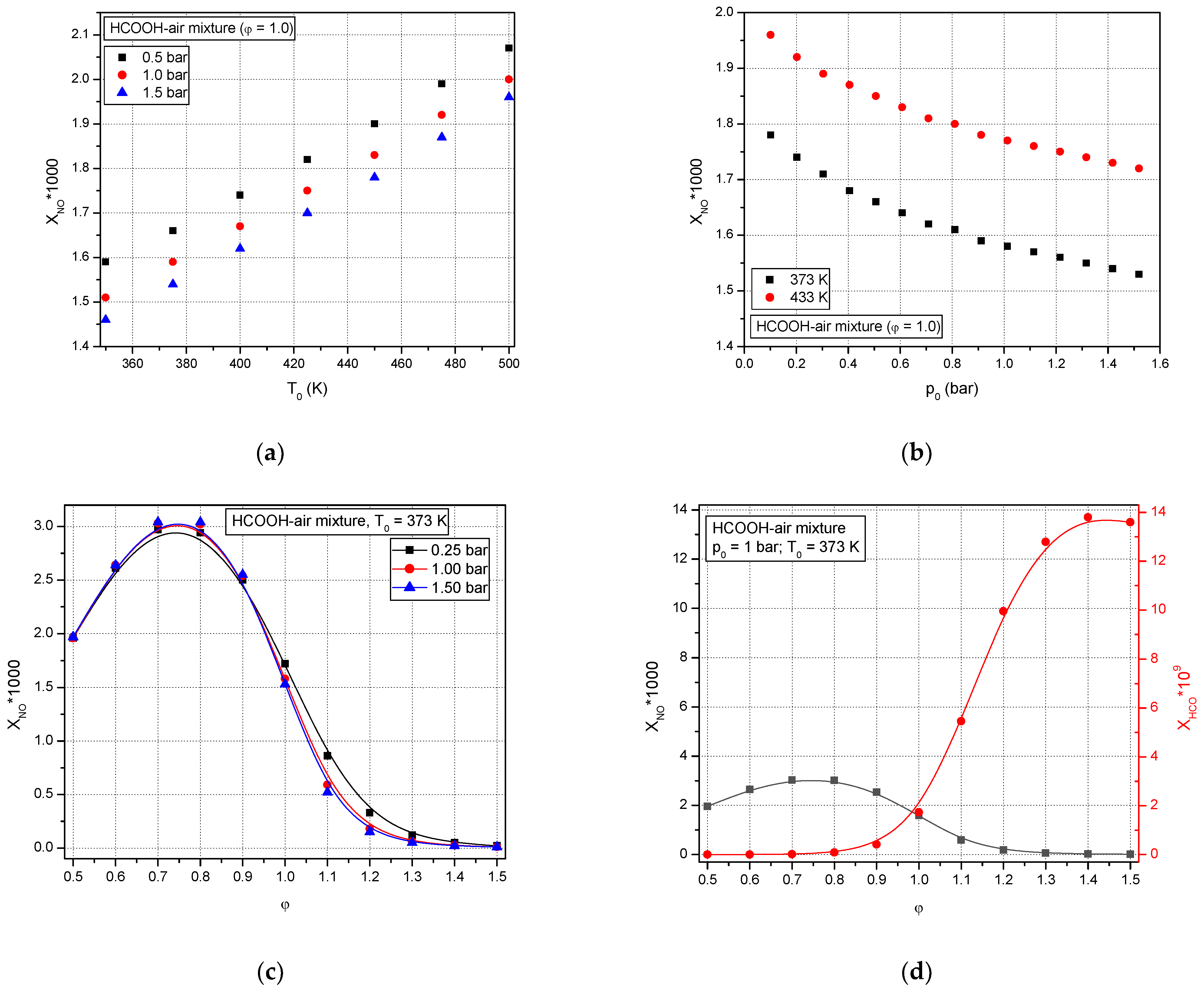
3.2. Structure of Premixed Formic Acid–Air Flames
4. Conclusions
- -
- The adiabatic peak pressures of the formic acid–air mixture increased with increasing initial pressures and decreased with increasing initial temperatures.
- -
- The adiabatic peak explosion pressures versus the total initial pressure (or initial temperature) of the flammable formic acid–air mixture are correlated by linear dependences for all examined mixtures, with both the slope and intercept of such correlations being influenced by the composition of the flammable mixtures.
- -
- For all investigated flammable formic acid–air mixtures, the maximum adiabatic peak pressures occur around equivalence ratio φ = 1.1.
- -
- This study showed that for rich concentrations of formic acid–air mixtures, at the constant initial temperature, a decrease in combustion pressure is efficient for lowering NO concentrations in the burned gas.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koumi Ngoh, S.; Njomo, D. An overview of hydrogen gas production from solar energy. Renew. Sustain Energy Rev. 2021, 16, 6782–6792. [Google Scholar] [CrossRef]
- Dutta, I.; Chatterjee, S.; Cheng, H.; Parsapur, R.K.; Liu, Z.; Li, Z.; Ye, E.; Low, J.S.C.; Lai, Z.; Loh, X.J.; et al. Formic Acid to Power towards Low-Carbon Economy. Adv. Energy Mater. 2022, 12, 2103799. [Google Scholar] [CrossRef]
- Mellmann, D.; Sponholz, P.; Junge, H.; Beller, M. Formic acid as a hydrogen storage material–development of homogeneous catalysts for selective hydrogen release. Chem. Soc. Rev. 2016, 45, 3954–3988. [Google Scholar] [CrossRef] [PubMed]
- Loges, B.; Boddien, A.; Gärtner, F.; Junge, H.; Beller, M. Catalytic generation of hydrogen from formic acid and its derivatives: Useful hydrogen storage materials. Top. Catal. 2010, 53, 902–914. [Google Scholar] [CrossRef]
- Wang, X.; Meng, Q.; Gao, L.; Jin, Z.; Ge, J.; Liu, C.; Xing, W. Recent progress in hydrogen production from formic acid decomposition. Int. J. Hydrogen Energy 2018, 43, 7055–7071. [Google Scholar] [CrossRef]
- Eversole, J.F. Manufacture of Formic acid. Patent US2160064 A, 30 May 1939. [Google Scholar]
- Jogunola, O.; Salmi, T.; Warna, J.; Mikkola, J.P.; Tirronen, E. Kinetics of methyl formate hydrolysis in the absence and presence of a complexing agent. Ind. Eng. Chem. Res. 2011, 50, 267–276. [Google Scholar] [CrossRef]
- Kim, C.; Lee, Y.; Kim, K.; Lee, U. Implementation of Formic Acid as a Liquid Organic Hydrogen Carrier (LOHC): Techno-Economic Analysis and Life Cycle Assessment of Formic Acid Produced via CO2 Utilization. Catalysts 2022, 12, 1113. [Google Scholar] [CrossRef]
- BASF. Applications of Formic Acid. 2022. Available online: https://products.basf.com/global/en/ci/formic-acid.html (accessed on 8 November 2022).
- Eppinger, J.; Huang, K.W. Formic acid as a hydrogen energy carrier. ACS Energy Lett. 2017, 2, 188–195. [Google Scholar] [CrossRef]
- Alvarez, A.; Bansode, A.; Urakawa, A.; Bavykina, A.V.; Wezendonk, T.A.; Makkee, M.; Gascon, J.; Kapteijn, F. Challenges in the greener production of formates/formic acid, methanol, and DME by heterogeneously catalyzed CO2 hydrogenation processes. Chem. Rev. 2017, 117, 9804–9838. [Google Scholar] [CrossRef]
- Sordakis, K.; Tang, C.; Vogt, L.K.; Junge, H.; Dyson, P.J.; Beller, M.; Laurenczy, G. Homogeneous catalysis for sustainable hydrogen storage in formic acid and alcohols. Chem. Rev. 2018, 118, 372–433. [Google Scholar] [CrossRef]
- Guan, C.; Pan, Y.; Zhang, T.; Ajitha, M.J.; Huang, K.W. An update on formic acid dehydrogenation by homogeneous catalysis. Chem.–Asian J. 2020, 15, 937–946. [Google Scholar] [CrossRef]
- Valentini, F.; Kozell, V.; Petrucci, C.; Marrocchi, A.; Gu, Y.; Gelman, D.; Vaccaro, L. Formic acid, a biomass-derived source of energy and hydrogen for biomass upgrading. Energy Environ. Sci. 2019, 12, 2646–2664. [Google Scholar] [CrossRef]
- Maharjan, S.; Elbaz, A.M.; Roberts, W.L. Investigation on the formic acid evaporation and ignition of formic acid/octanol blend at elevated temperature and pressure. Fuel 2022, 313, 122636. [Google Scholar] [CrossRef]
- Rice, C.; Ha, S.; Masel, R.I.; Waszczuk, P.; Wieckowski, A.; Barnard, T. Direct formic acid fuel cells. J. Power Sources 2002, 111, 83–89. [Google Scholar] [CrossRef]
- Müller, K.; Brooks, K.; Autrey, T. Hydrogen storage in formic acid: A comparison of process options. Energy Fuels 2017, 31, 12603–12611. [Google Scholar] [CrossRef]
- Onishi, N.; Laurenczy, G.; Beller, M.; Himeda, Y. Recent progress for reversible homogeneous catalytic hydrogen storage in formic acid and in methanol. Coord. Chem. Rev. 2018, 373, 317–332. [Google Scholar] [CrossRef]
- Yin, G.; Xu, J.; Hu, E.; Gao, Q.; Zhan, H.; Huang, Z. Experimental and kinetic study on the low temperature oxidation and pyrolysis of formic acid in a jet-stirred reactor. Combust. Flame 2021, 223, 77–87. [Google Scholar] [CrossRef]
- van Putten, R.; Wissink, T.; Swinkels, T.; Pidko, E.A. Fuelling the hydrogen economy: Scale-up of an integrated formic acid-to-power system. Int. J. Hydrogen Energy 2019, 44, 28533–28541. [Google Scholar] [CrossRef]
- Lavadera, M.L.; Konnov, A.A. Laminar burning velocities of methane + formic acid + air flames: Experimental and modeling study. Combust. Flame 2021, 225, 65–73. [Google Scholar] [CrossRef]
- Osipova, K.N.; Sarathy, S.M.; Korobeinichev, O.P.; Shmakov, A.G. Chemical structure of atmospheric pressure premixed laminar formic acid/hydrogen flames. Proc. Combust. Inst. 2021, 38, 2379–2386. [Google Scholar] [CrossRef]
- Sarathy, S.M.; Brequigny, P.; Katoch, A.; Elbaz, A.M.; Roberts, W.L.; Dibble, R.W.; Foucher, F. Laminar burning velocities and kinetic modeling of a renewable e-fuel: Formic acid and its mixtures with H2 and CO2. Energy Fuels 2020, 34, 7564–7572. [Google Scholar] [CrossRef]
- Yin, G.; Gao, Q.; Hu, E.; Xu, J.; Zhou, M.; Huang, Z. Experimental and kinetic study on laminar flame speeds of formic acid. Combust. Flame 2020, 220, 73–81. [Google Scholar] [CrossRef]
- Wako, F.M.; Pio, G.; Salzano, E. Modeling formic acid combustion. Energy Fuels 2022, 36, 14382–14392. [Google Scholar] [CrossRef]
- CHEMSAFE. 2024. Available online: https://www.chemsafe.ptb.de/ (accessed on 10 June 2024).
- de Wilde, E.V.; van Tiggelen, A. Burning velocities in mixtures of methyl alcohol, formaldehyde or formic acid with oxygen. Bulletin des Sociétés Chimiques Belges 1968, 77, 67–75. [Google Scholar] [CrossRef]
- Morley, C. Gaseq—A Chemical Equilibrium Program for Windows. 2004. Available online: http://www.gaseq.co.uk (accessed on 10 June 2024).
- Mitu, M.; Brandes, E. Sicherheitstechnische Kenngroßen von Alkohol/Luft-Gemischen-Explosionsdruck, zeitlicher Druckanstieg, Verbrennungsgeschwindigkeit. In Proceedings of the Proceedings 13. BAM-PTB-Kolloquium zur Chemischen und Physikalischen Sicherheitstechnik, Braunschweig, Germany, 18–19 June 2013. [Google Scholar]
- Mitu, M.; Brandes, E.; Hirsch, W. Mitigation effects on the explosion safety characteristic data of ethanol/air mixtures in closed vessel. Process Saf. Environ. Prot. 2018, 117, 190–199. [Google Scholar] [CrossRef]
- Mitu, M.; Brandes, E.; Zakel, S.; Hirsch, W. Explosion regions and limiting oxygen concentrations of methyl propionate, methyl acetate, dimethyl carbonate with air and inert gas mixtures. J. Loss Prev. Process Ind. 2021, 69, 104384. [Google Scholar] [CrossRef]
- Steen, H.; Hattwig, M. (Eds.) Handbook of Explosion Prevention and Control; Wiley-VCH: London, UK; New York, NY, USA, 2004; Chapter 3. [Google Scholar]
- Razus, D.; Brinzea, V.; Mitu, M.; Oancea, D. Temperature and pressure influence on explosion pressures of closed vessel propane–air deflagrations. J. Hazard. Mater. 2010, 174, 548–555. [Google Scholar] [CrossRef] [PubMed]
- Pekalski, A.A.; Schildberg, H.P.; Smallegange, P.S.D.; Lemkowitz, S.M.; Zevenbergen, J.F.; Braithwaite, M.; Pasman, H.J. Determination of the explosion behaviour of methane and propene in air or oxygen at standard and elevated conditions. Procces Saf. Environ. Prot. 2005, 83, 421–429. [Google Scholar] [CrossRef]
- Mitu, M.; Giurcan, V.; Razus, D.; Oancea, D. Temperature and pressure influence on ethane–air deflagration parameters in a spherical closed vessel. Energy Fuels 2012, 26, 4840–4848. [Google Scholar] [CrossRef]
- Desoky, A.A.; Abdel-Ghafar, Y.A.; El-Badrawy, R.M. Hydrogen, propane and gasoline laminar flame development in a spherical vessel. Int. J. Hydrogen Energy 1990, 15, 895–905. [Google Scholar] [CrossRef]
- Mitu, M.; Brandes, E. Explosion parameters of methanol–air mixtures. Fuel 2015, 158, 217–223. [Google Scholar] [CrossRef]
- Mitu, M.; Brandes, E. Influence of pressure, temperature and vessel volume on explosion characteristics of ethanol/air mixtures in closed spherical vessels. Fuel 2017, 203, 460–468. [Google Scholar] [CrossRef]
- Gieras, M.; Klemens, R.; Rarata, G.; Wolański, P. Determination of explosion parameters of methane-air mixtures in the chamber of 40 dm3 at normal and elevated temperature. J. Loss Prev. Process. Ind. 2006, 19, 263–270. [Google Scholar] [CrossRef]
- Razus, D.; Oancea, D.; Chirila, F.; Ionescu, N.I. Transmission of an explosion between linked vessels. Fire Safety J. 2003, 38, 147–163. [Google Scholar] [CrossRef]
- Cui, G.; Wang, S.; Liu, J.; Bi, Z.; Li, Z. Explosion characteristics of a methane/air mixture at low initial temperatures. Fuel 2018, 234, 886–893. [Google Scholar] [CrossRef]
- Li, Q.; Cheng, Y.; Huang, Z. Comparative assessment of the explosion characteristics of alcohol-air mixtures. J. Loss Prev. Process. Ind. 2015, 37, 91–100. [Google Scholar] [CrossRef]
- Oppong, F.; Zhongyang, L.; Li, X.; Song, Y.; Xu, C.; Diaby, A.L. Methyl pentanoate laminar burning characteristics: Experimental and numerical analysis. Renewable Energy 2022, 197, 228–236. [Google Scholar] [CrossRef]
- Zhang, B.; Xiu, G.; Bai, C. Explosion characteristics of argon/nitrogen diluted natural gas-air mixtures. Fuel 2014, 124, 125–132. [Google Scholar] [CrossRef]
- Kamran, M.; Shahani, N.M. Decision Support System for the Prediction of Mine Fire Levels in Underground Coal Mining Using Machine Learning Approaches. Min. Metall. Explor. 2022, 39, 591–601. [Google Scholar] [CrossRef]
- Kamran, M.; Wattimena, R.K.; Armaghani, D.J.; Asteris, P.G.; Jiskani, I.M.; Mohamad, E.T. Intelligent based decision-making strategy to predict fire intensity in subsurface engineering environments. Process Saf. Environ. Prot. 2023, 171, 374–384. [Google Scholar] [CrossRef]
- Kamran, M.; Chaudhry, W.; Wattimena, R.K.; Rehman, H.; Martyushev, D.A. A multi-criteria decision intelligence framework to predict fire danger ratings in underground engineering structures. Fire 2023, 6, 412. [Google Scholar] [CrossRef]



| Empirical formula | CH2O2 |
| Chemical structure | HCOOH |
| Relative molar mass | 46 |
| Relative gas density | 1.59 |
| Density (g/cm3) | 1.218 |
| Melting point (°C) | 8.4 |
| Boiling point (°C) | 100.5 |
| Autoignition temperature (°C) | 510 |
| Flash point (closed cup) (°C) | 45 |
| Lower explosion limit (LEL), (vol%); (temperature of LEL: 100 °C) | 16.4 |
| Upper explosion limit (UEL), (vol%) | 45.5 |
| Maximum Experimental Safe Gap (MESG), (mm) | 1.76 |
| Molar stoichiometric fraction of combustible in mixture with air (Mol%) | 29.5 |
| Molar stoichiometric fraction of combustible in mixture with oxygen (Mol%) | 66.7 |
| φ | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1.0 | 1.1 | 1.2 | 1.3 | 1.4 | 1.5 |
| Concentration of formic acid (vol%) | 17.32 | 20.09 | 22.68 | 25.11 | 27.38 | 29.53 | 31.55 | 33.46 | 35.26 | 36.97 | 38.59 |
| φ | a | b*1000 (K) |
|---|---|---|
| 0.5 | 0.71 | 1.68 |
| 0.6 | 0.69 | 1.87 |
| 0.7 | 0.63 | 2.05 |
| 0.8 | 0.58 | 2.21 |
| 0.9 | 0.52 | 2.34 |
| 1.0 | 0.47 | 2.44 |
| 1.1 | 0.55 | 2.43 |
| 1.2 | 0.66 | 2.36 |
| 1.3 | 0.71 | 2.30 |
| 1.4 | 0.74 | 2.25 |
| 1.5 | 0.78 | 2.19 |
| T0 (K) | −α (bar) | β | rn |
|---|---|---|---|
| 333 | 0.037 ± 0.004 | 7.640 ± 0.004 | 1.0 |
| 373 | 0.033 ± 0.005 | 6.877 ± 0.005 | 1.0 |
| 403 | 0.037 ± 0.005 | 6.479 ± 0.005 | 1.0 |
| 433 | 0.033 ± 0.004 | 5.983 ± 0.004 | 1.0 |
| 463 | 0.035 ± 0.004 | 5.703 ± 0.005 | 1.0 |
| 493 | 0.034 ± 0.004 | 5.385 ± 0.005 | 0.9999 |
| [HCOOH] (vol%) | φ | −α (bar) | β | rn |
|---|---|---|---|---|
| 0.173 | 0.5 | −0.010 ± 0.027 | 5.149 ± 0.027 | 0.9999 |
| 0.201 | 0.6 | −0.001 ± 0.029 | 5.642 ± 0.030 | 0.9999 |
| 0.227 | 0.7 | 0.003 ± 0.030 | 6.064 ± 0.031 | 0.9999 |
| 0.251 | 0.8 | 0.009 ± 0.035 | 6.431 ± 0.036 | 0.9999 |
| 0.274 | 0.9 | 0.025 ± 0.034 | 6.751 ± 0.035 | 0.9999 |
| 0.295 | 1.0 | 0.033 ± 0.038 | 6.971 ± 0.039 | 0.9999 |
| 0.315 | 1.1 | 0.021 ± 0.037 | 7.010 ± 0.038 | 0.9999 |
| 0.335 | 1.2 | 0.002 ± 0.039 | 6.912 ± 0.040 | 0.9999 |
| 0.353 | 1.3 | 0.007 ± 0.037 | 6.801 ± 0.038 | 0.9999 |
| 0.370 | 1.4 | 0.001 ± 0.036 | 6.699 ± 0.037 | 0.9999 |
| 0.386 | 1.5 | 0.008 ± 0.035 | 6.585 ± 0.035 | 0.9999 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mitu, M. Effect of Initial Temperature and Pressure on the Explosion Characteristics and Intermediate Reaction Products of Formic Acid Mixtures: A Theoretical Study. Fire 2024, 7, 290. https://doi.org/10.3390/fire7080290
Mitu M. Effect of Initial Temperature and Pressure on the Explosion Characteristics and Intermediate Reaction Products of Formic Acid Mixtures: A Theoretical Study. Fire. 2024; 7(8):290. https://doi.org/10.3390/fire7080290
Chicago/Turabian StyleMitu, Maria. 2024. "Effect of Initial Temperature and Pressure on the Explosion Characteristics and Intermediate Reaction Products of Formic Acid Mixtures: A Theoretical Study" Fire 7, no. 8: 290. https://doi.org/10.3390/fire7080290
APA StyleMitu, M. (2024). Effect of Initial Temperature and Pressure on the Explosion Characteristics and Intermediate Reaction Products of Formic Acid Mixtures: A Theoretical Study. Fire, 7(8), 290. https://doi.org/10.3390/fire7080290






