Study on the Fire Characteristics of Dual Fire Sources and the Difference in Power Temperature of Different Fire Sources in Tunnel
Abstract
1. Introductory
2. Dual-Source Fire Experiment
2.1. Determination of Model Construction Parameters
2.2. Numerical Simulation Experimental Modeling
3. Analysis of Experimental Results
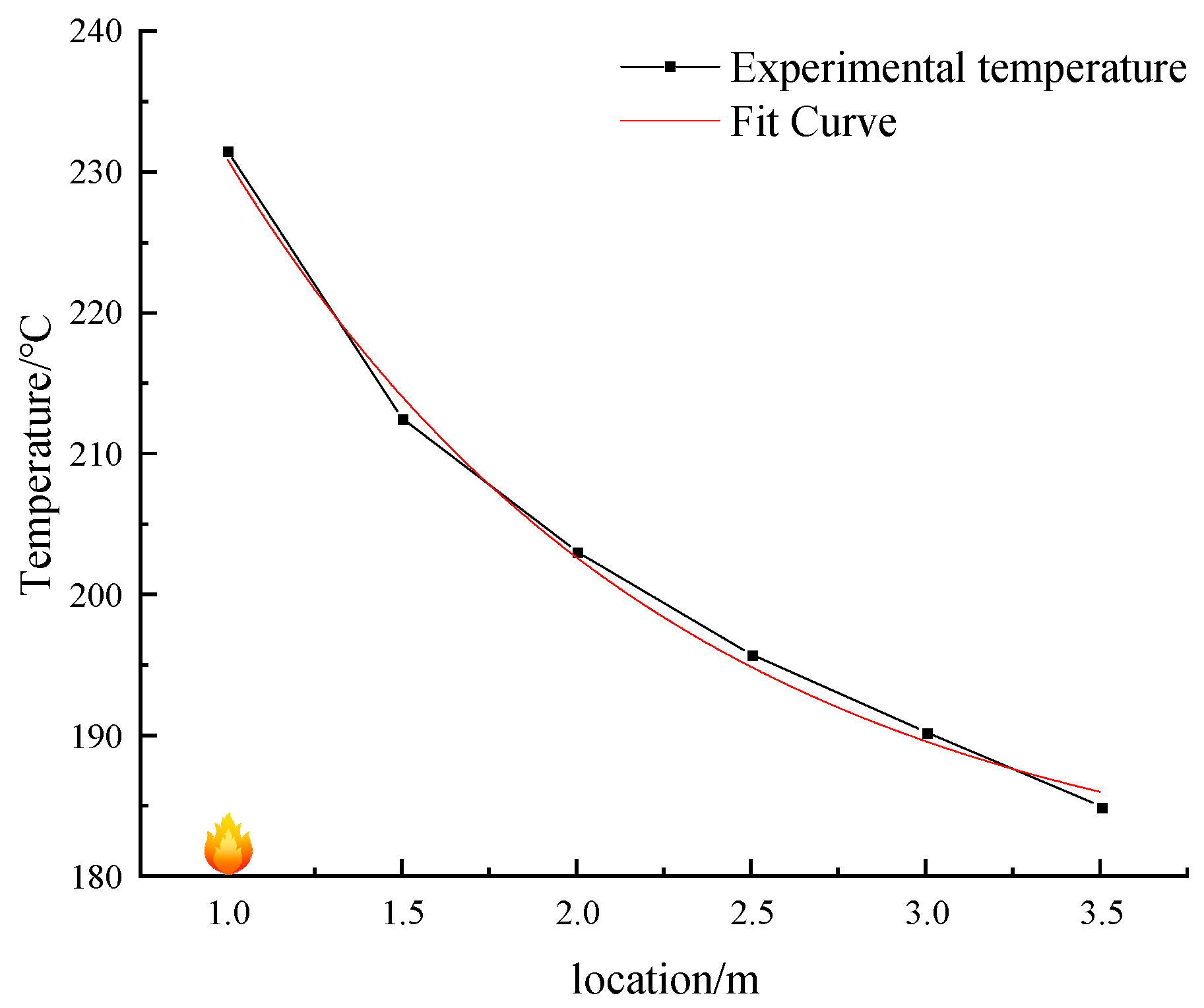
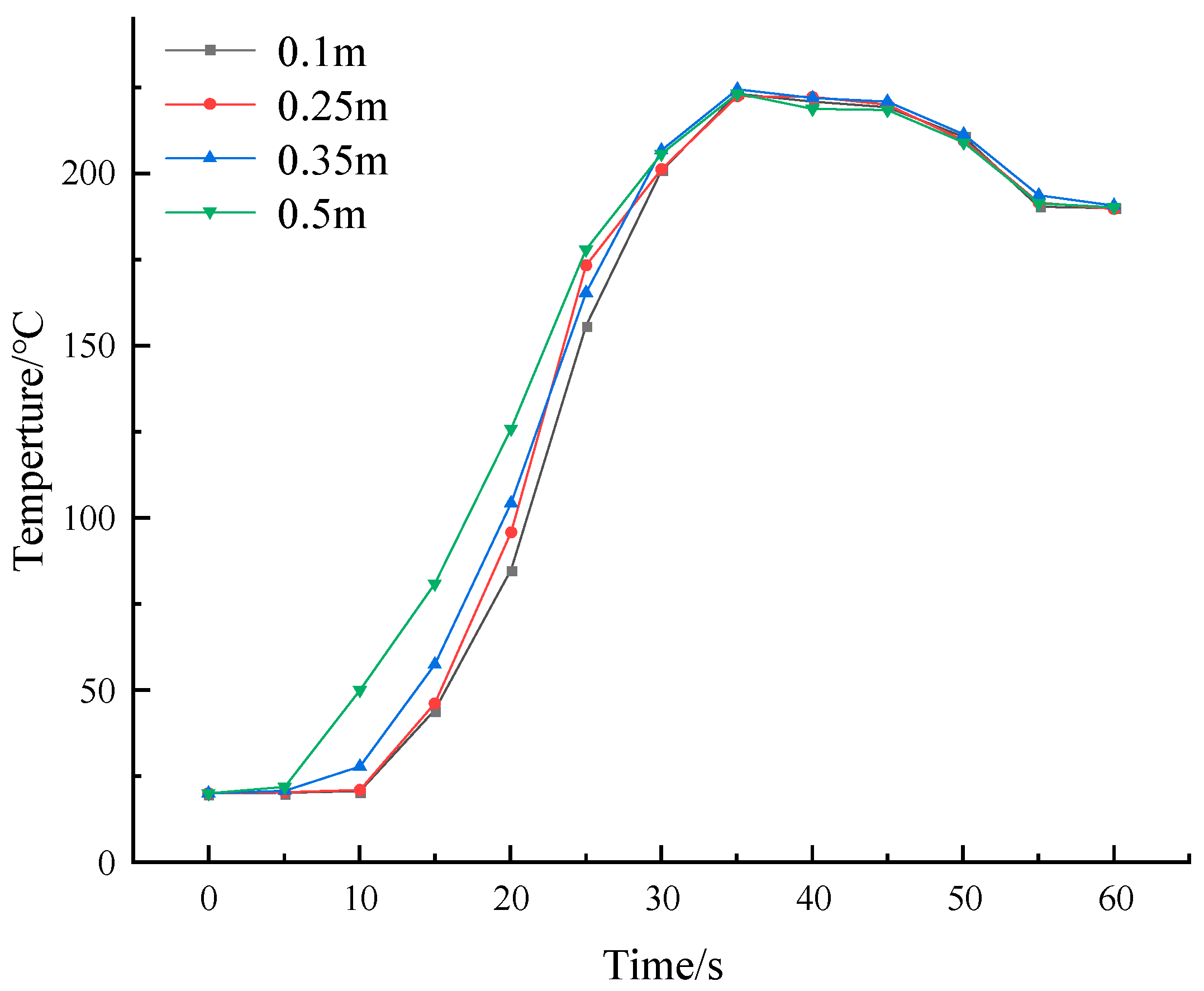
3.1. Research on the Change Rule of Temperature Change of Double Fire Source
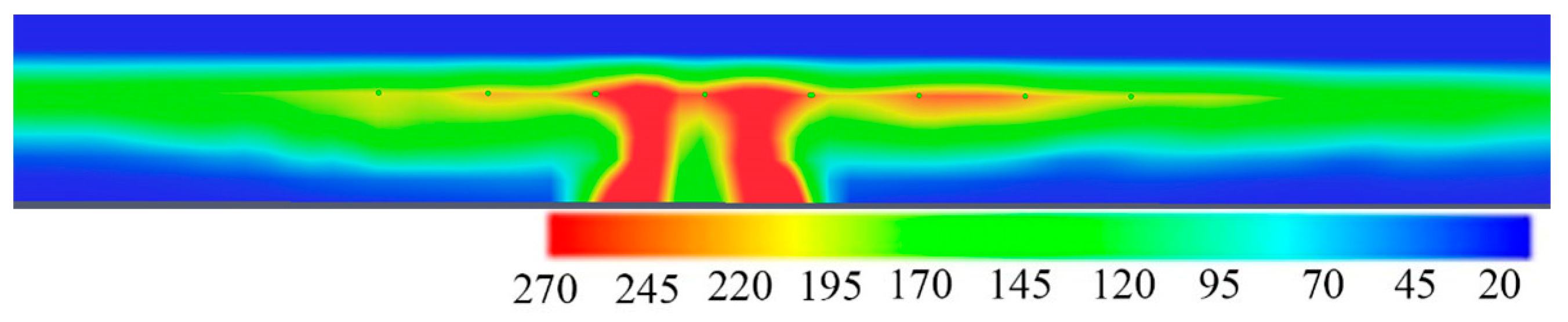
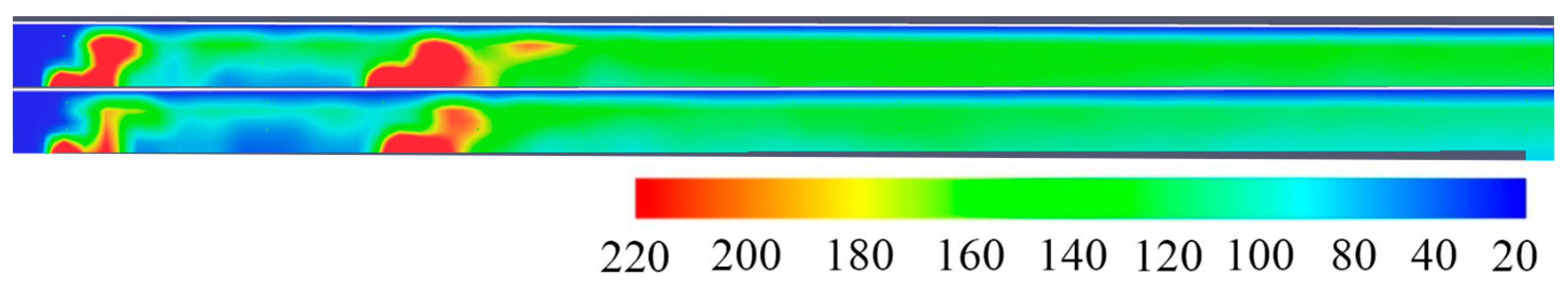
3.2. Study of the Phenomenon of Dual Source Fires
4. Numerical Simulation and Analysis of Double-Source Fire in Full-Size Tunnel
4.1. Establishment of Full-Size Tunnel Model
4.2. Mesh Irrelevance Test
4.3. Fire Source and Other Boundary Conditions
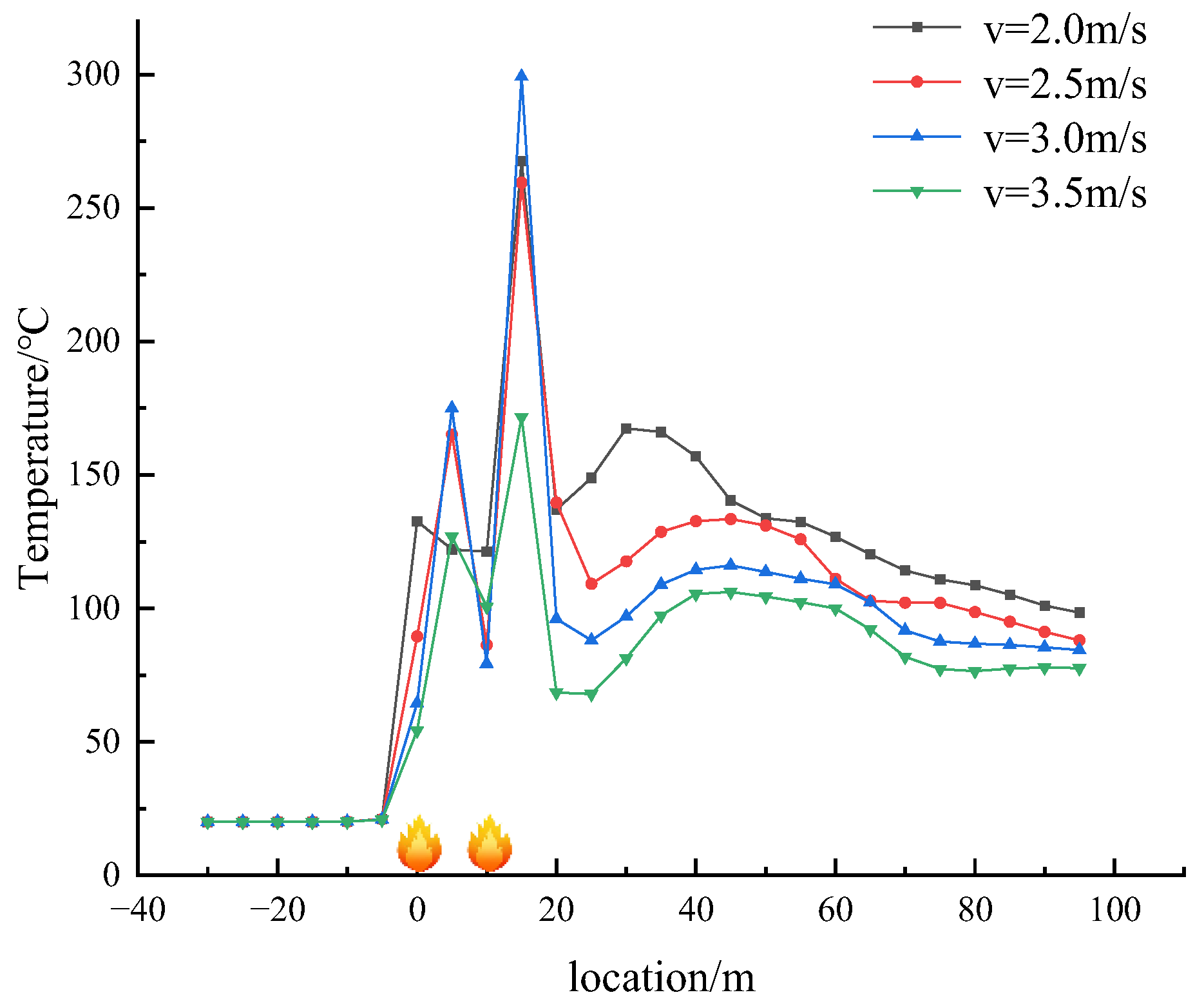
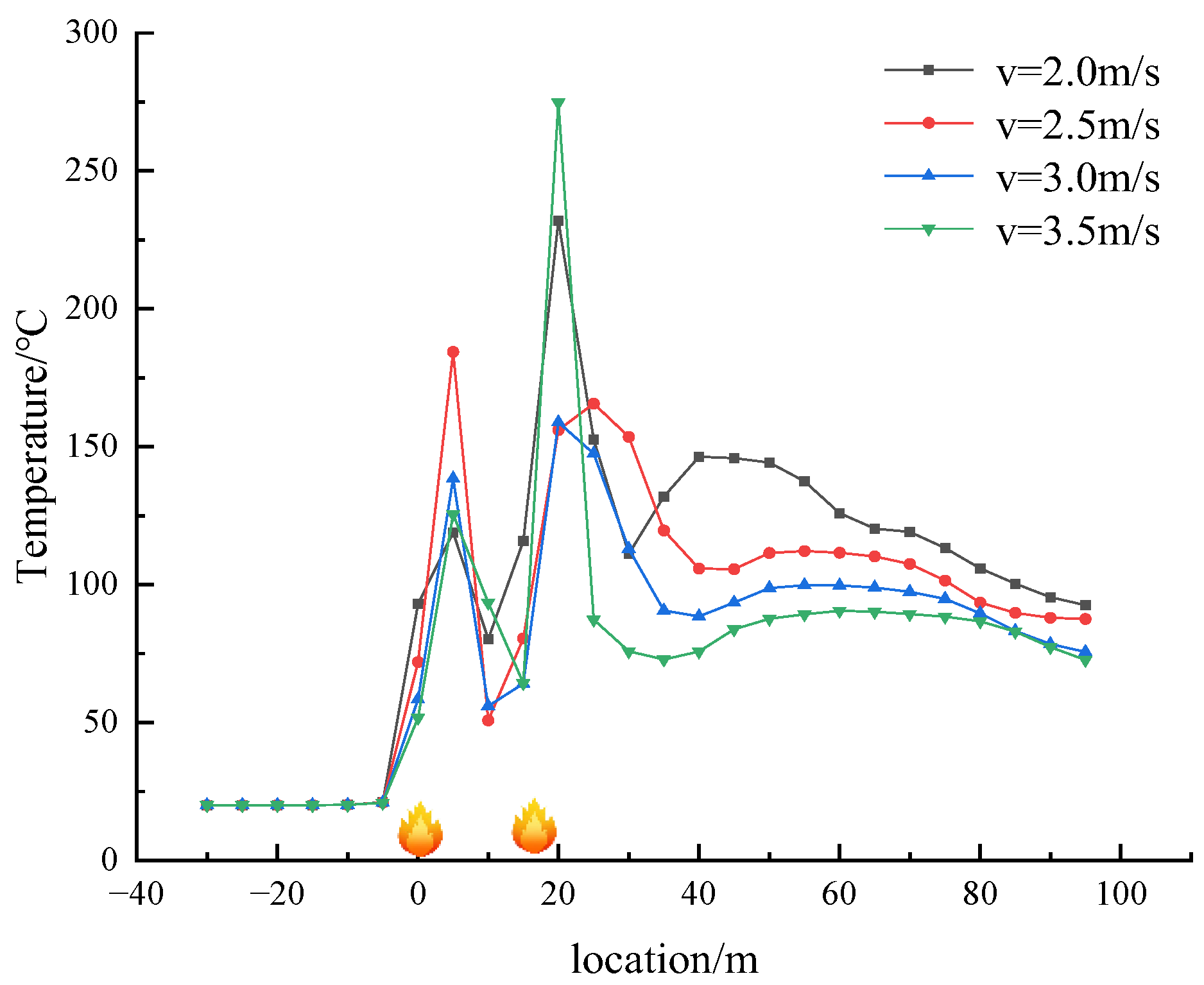
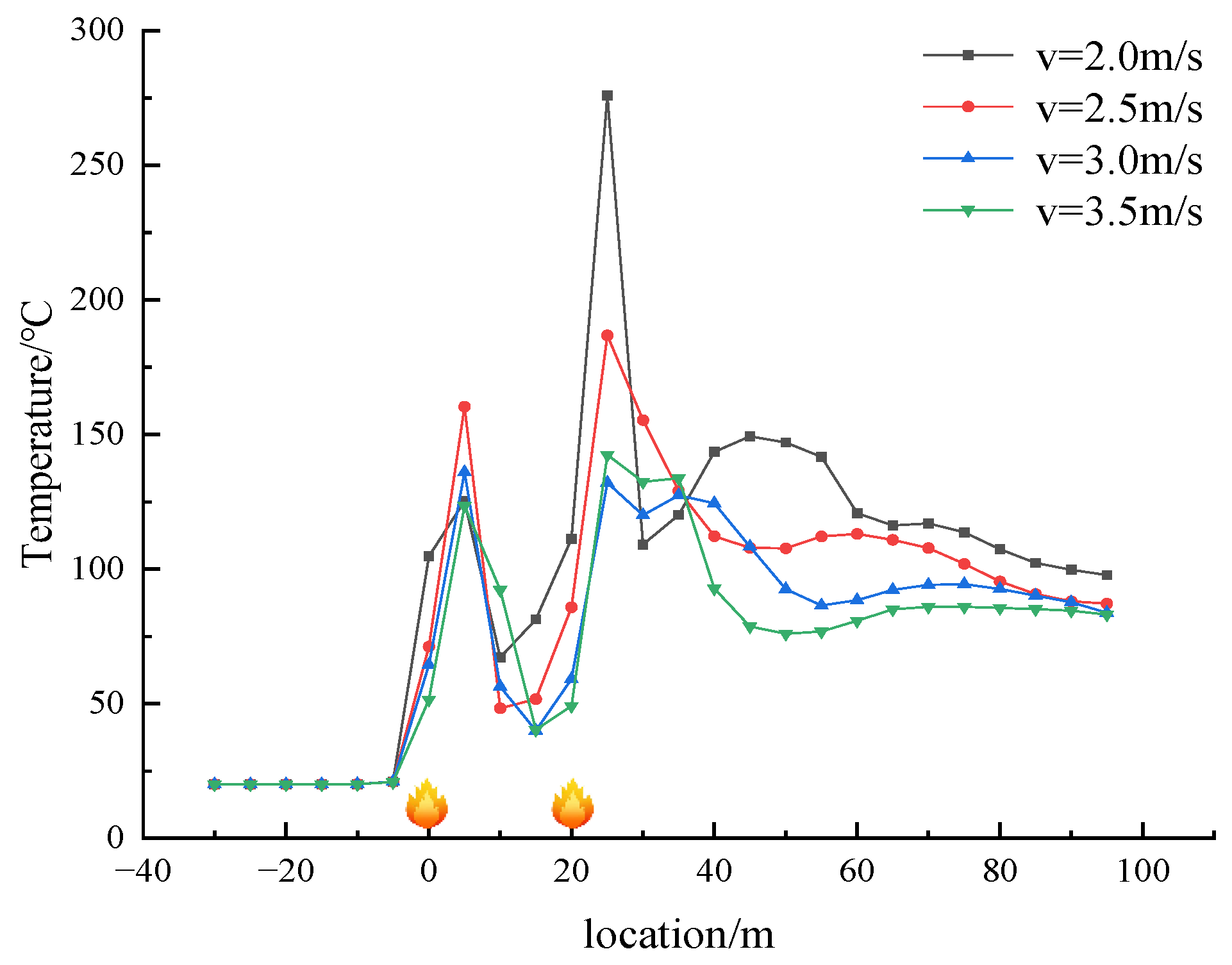
4.4. Determination of Critical Wind Speed for Twin Source Fires
4.5. Fire Characterization of Different Ignition Powers
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| physical quantity | marginal notes |
| Relative ambient temperature rise of high-temperature smoke at distance x from the fire source, °C | |
| Ambient temperature rise at fire source location, °C | |
| k | Temperature decay coefficient |
| x | Reference position from the source of the fire, m |
| x0 | fire source distance, m |
| α | Combined heat transfer coefficient of the flue gas layer, W/(m2·K) |
| D | the length of the smoke layer in contact with the wall of the tunnel, m |
| cp | constant-pressure specific heat, KJ/(kg·K) |
| m | the mass flow rate of the fire smoke stream, kg/s |
| hc | the convective heat transfer coefficient, W/(m2·K) |
| hr | the radiative heat transfer coefficient in W/(m2·K) |
| B | the length of the cross-section of the flue gas layer in the part that does not come into contact with the tunnel wall, m. |
| u | the horizontal propagation velocity of the flue gas, m/s. |
| K′ | the empirical relationship constant, kCal/m2°C |
| ε | the emissivity |
| σ | Boltzmann’s constant |
| Ts | the temperature of the smoke stream, K |
| Tsur | the temperature of the wall surface, Kc |
| s | Cross-sectional area of the flue gas layer, m2 |
| ρ | Ambient air density, kg/m3 |
| Q* | the dimensionless heat release rate |
| Q | the heat release rate of the fire source, kW, °C |
| ρ0 | the air density, kg/m3 |
| T0 | the ambient temperature of the air, K |
| g | the acceleration of gravity, m/s2; |
| H | the hydraulic diameter, m |
| v* | Dimensionless wind speed |
| v | critical wind speed |
References
- Yue, N.; Cai, G.; Gao, W.; Quan, Y.; Wang, F. Research on influencing factors of water mist suppression for belt spreading fire in mine. J. Saf. Sci. Technol. 2022, 18, 92–97. [Google Scholar]
- Ji, J.W.; Cheng, Y.P. Fire Dynamics; China University of Mining and Technology Press: Xuzhou, China, 2018. [Google Scholar]
- Hu, L.H.; Peng, W.; Yang, R.X. Fundamentals of Tunnel Fire Dynamics and Prevention Technology; Science Press: Beijing, China, 2014. [Google Scholar]
- Fukuda, Y.; Kamikawa, D.; Hasemi, Y.; Kagiya, K. Flame Characteristics of group fires. Fire Sci. Technol. 2004, 23, 164–169. [Google Scholar] [CrossRef]
- Liu, N.; Liu, Q.; Lozano, J.S.; Shu, L.; Zhang, L.; Zhu, J.; Deng, Z.; Satoh, K. Global burning rate of square fire arrays: Experimental correlation and interpretation. Proc. Combust. Inst. 2009, 32, 2519–2526. [Google Scholar] [CrossRef]
- Yu, M.; Su, G.; Chen, J. Numerical studies on the effect of double fire sources upon critical ventilation velocity in tunnel fires. Fire Saf. Sci. 2017, 26, 20–28. [Google Scholar]
- Liu, Q.; Zheng, F. Study on Variation Rules of Critical Ventilation Velocity for Double-source Tunnel Fires. J. Disaster Prev. Mitig. Eng. 2018, 38, 137–143. [Google Scholar]
- Cui, X.; Zhao, J.; Yao, R.; Yuan, S.; Wu, B.; Huang, H. Numerical simulation on double fire source tunnel. J. Cent. South Univ. (Sci. Technol.) 2022, 53, 2255–2267. [Google Scholar]
- Tsai, K.C.; Chen, H.H.; Lee, S.K. Cortical ventilation velocity for multi-source tunnel fires. J. Wind. Eng. Ind. Aerodyn. 2010, 98, 650–660. [Google Scholar] [CrossRef]
- Guo, C.; Yan, Z.; Li, W.; Zhou, X. A Full-scale Experimental Study on the Fire Characteristics of Dual-source Tunnel Fire. Mod. Tunn. Technol. 2023, 60, 247–259. [Google Scholar]
- Xu, H.; Zhao, W.; Lv, S. Experimental Study on the Combustion Characteristics of two Fire Sources in a Tunnel. J. Eng. Thermophys. 2020, 41, 1254–1260. [Google Scholar]
- Zhong, M.; Shi, C.; He, L.; Shi, J.; Liu, C.; Tian, X. Smoke development in full-scale sloped long and large curved tunnel fires under natural ventilation. Appl. Therm. Eng. 2016, 108, 857–865. [Google Scholar] [CrossRef]
- Yao, Y.; Cheng, X.; Shi, L.; Zhang, S.; He, K.; Peng, M.; Zhang, H. Experimental study on the effects of initial sealing time on fire behaviors in channel fires. Int. J. Therm. Sci. 2018, 125, 273–282. [Google Scholar] [CrossRef]
- Ji, J.; Wan, W.; Gao, Z.; Fu, Y.; Sun, J.; Zhang, Y.; Li, K.; Hostikka, S. Experimental study on flame merging behaviors from two pool fires along the longitudinal centerline of model tunnel with natural ventilation. Combust. Flame 2016, 173, 307–318. [Google Scholar] [CrossRef]
- Molkens, T. FDS Versus En-Models. A Comparison between the Heskestad Model from EN and CFD Results. J. Struct. Fire Eng. 2015, 6, 3. [Google Scholar] [CrossRef]
- Qinghua, G.; Zhen, Y.L.; Haukur, I.; Yan, Z.; Zhu, H. Numerical study on thermally driven smoke flow characteristics in long tunnels under natural ventilation. Int. J. Therm. Sci. 2023, 192, 108379. [Google Scholar]
- Liu, N.A.; Zhang, S.J.; Luo, X.S.; Lei, J.; Chen, H.; Xie, X.; Zhang, L.; Tu, R. Interaction of two parallel rectangular fires. Proc. Combust. Inst. 2019, 37, 3833–3841. [Google Scholar] [CrossRef]
- Haukur, I.; Ying, Z. Model scale tunnel fire tests with longitudinal ventilation. Fire Saf. J. 2010, 45, 371–384. [Google Scholar]
- Oerkan, K. An Experimental study on the effects of blockage ratio and ventilation velocity on the heat release rate of tunnel fires. J. Fire Sci. 2011, 29, 555–575. [Google Scholar]
- Wan, H.; Gao, Z.; Ji, J.; Fang, J.; Zhang, Y. Experimental study on horizontal gas temperature distribution of two propane diffusion flames impinging on an unconfined ceiling. Int. J. Therm. Sci. 2019, 136, 1–8. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, S.; Liu, H.; Shen, J.; Shang, F.; Shi, C.; Tang, F. Characterization of ceiling smoke temperature profile and maximum temperature rise induced by double fires in a natural ventilation tunnel. Tunn. Undergr. Space Technol. Technol. 2020, 96, 103233. [Google Scholar] [CrossRef]
- Zhang, Y.; Xing, S.; Chen, R.; Chen, L.; Li, T.; Mao, P. Experimental study on maximum temperature beneath tunnel ceiling under the condition of double fire sources. Tunn. Undergr. Space Technol. Technol. 2020, 106, 103624. [Google Scholar] [CrossRef]
- Chin, D.; Joaquim, P. Analysis of Fire Throttling in Longitudinally Ventilated Tunnels with a One-dimensional Model. Fire Technol. 2022, 58, 2925–2947. [Google Scholar]
- Song, Y.; Jiang, H. Pyrosim-based Simulation of Differentiated Sprinkler System for Terminal Fire. Sci. Technol. Eng. 2021, 21, 10998–11004. [Google Scholar]
- Hu, L.H.; Huo, R.; Li, Y.Z.; Wang, H.B.; Chow, W.K. Full-scale burning tests on studying smoke temperature and velocity along a corridor. Tunneling Undergr. Space Technol. 2005, 20, 223–229. [Google Scholar] [CrossRef]
- Hu, L.H. Studies on Thermal Physics of Smoke Movement in Tunnel Fires. Ph.D. Thesis, University of Science and Technology of China, Hefei, China, 2006. [Google Scholar]
- Zhao, X.K.; Ni, M.H. Research on fire characteristics of different curvature curved tunnels based on PyroSim. Fire Sci. Technol. 2024, 43, 309–313. [Google Scholar]
- Wu, Y.; Baker, M.Z.A. Control of smoke flow in tunnel fires using longitudinal ventilation systems—a study of the critical velocity. Fire Saf. J. 2000, 35, 363–390. [Google Scholar] [CrossRef]
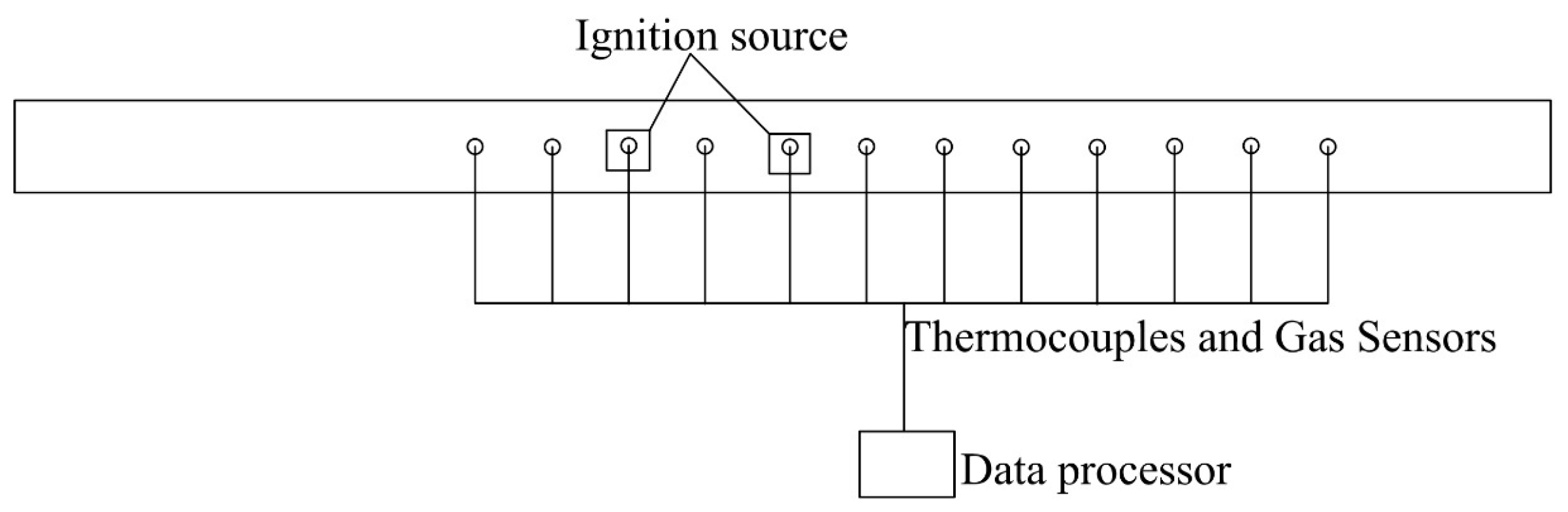

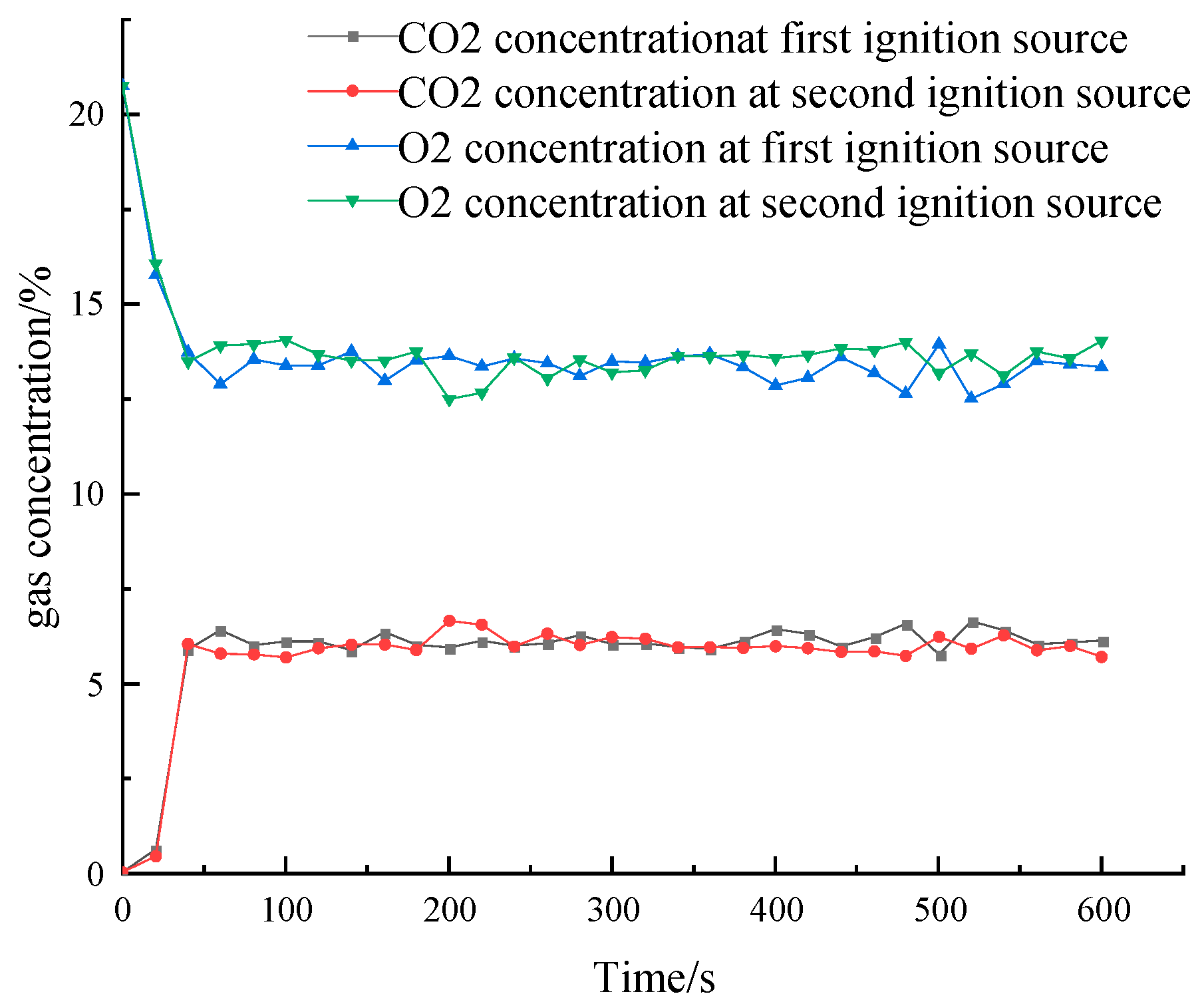

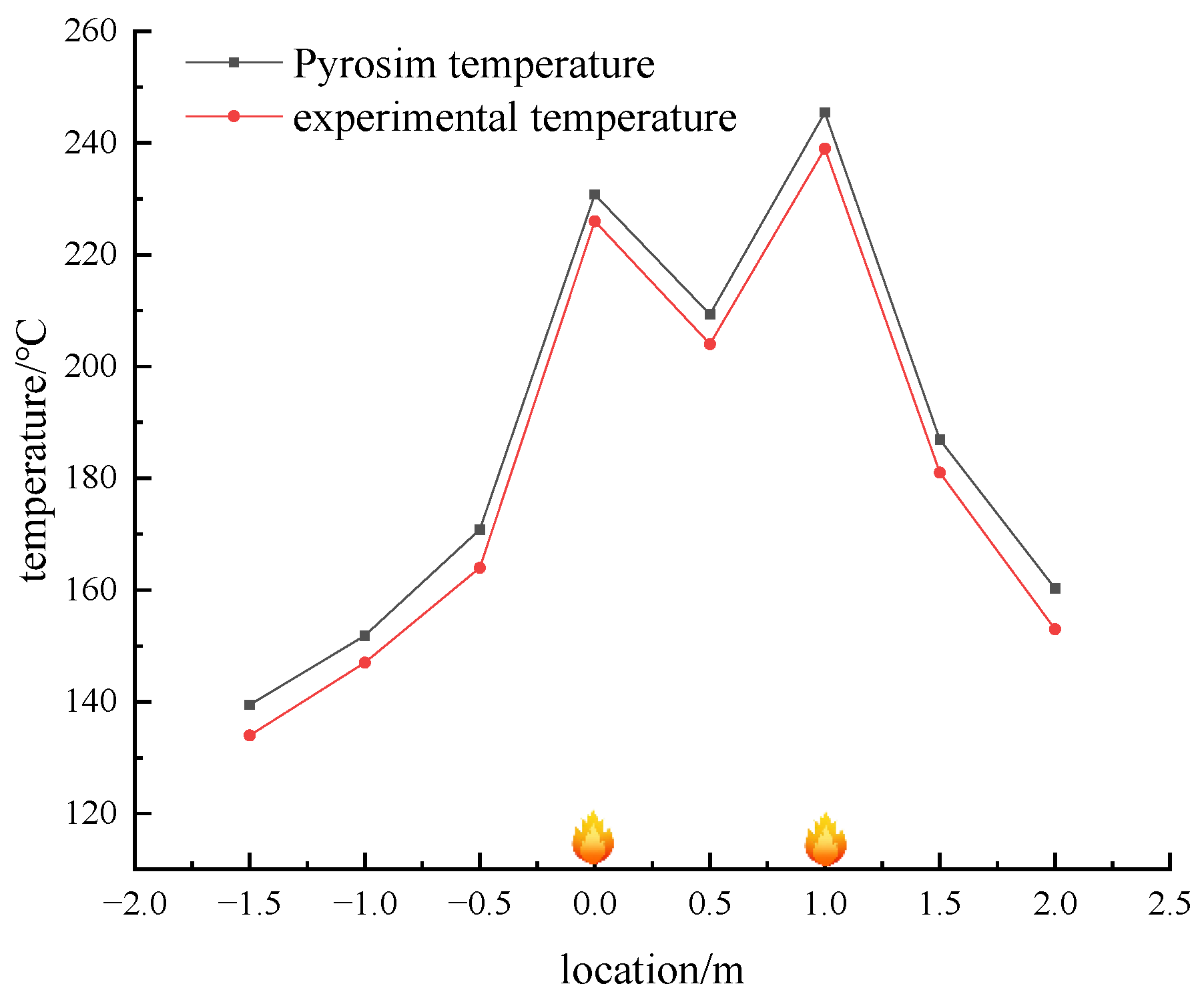
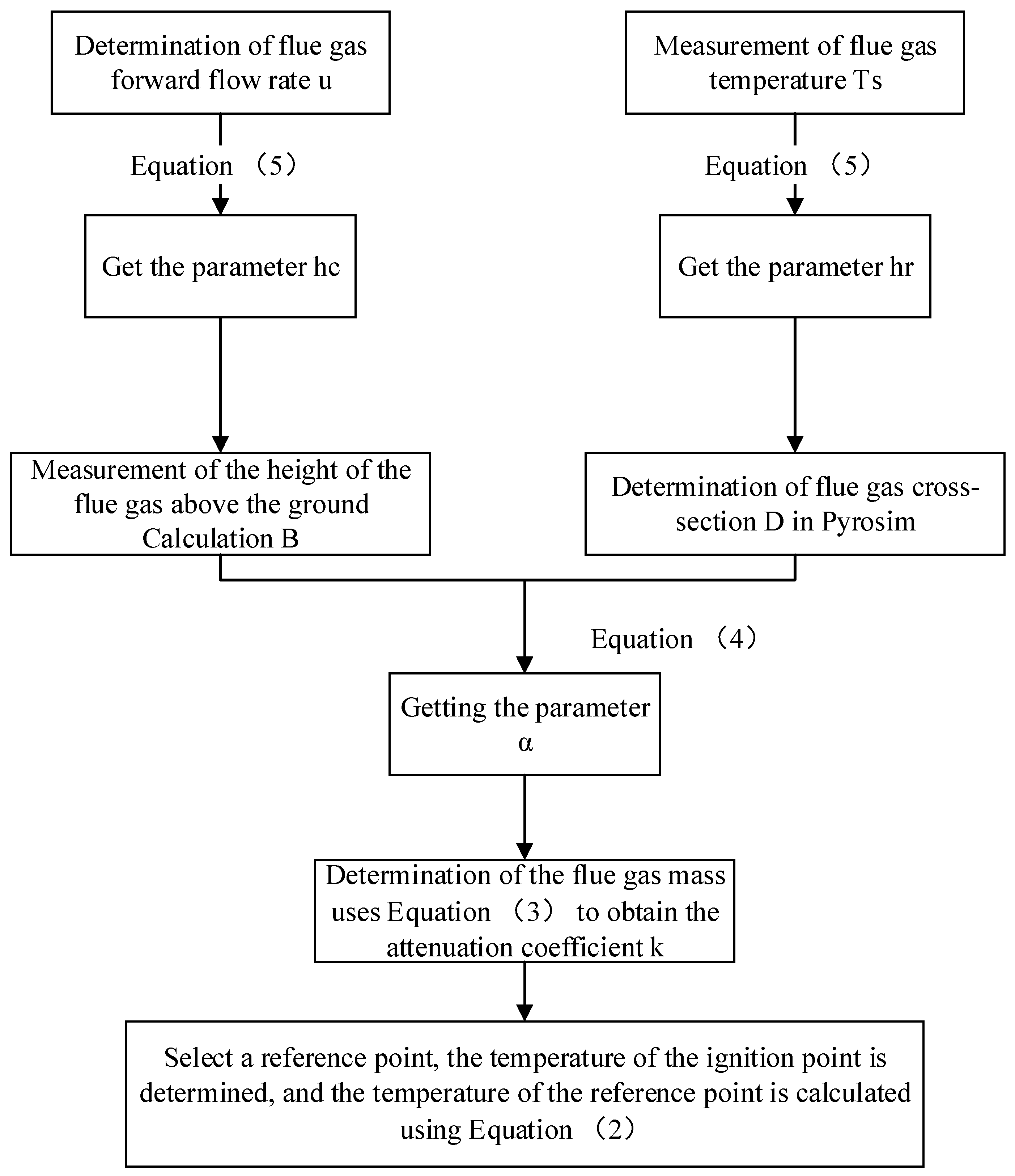


| Model Part | Sizes (cm) | Material |
|---|---|---|
| Bottom and Top | 80 × 1000 | steel |
| sidewall | 60 × 100 | reinforced glass |
| frame | 3.8 × 3.8 | Square Steel Pipe |
| hibachi | 20 × 30 × 1.5 | aluminum |
| Characteristic | Parameters |
|---|---|
| density/(kg/m3) | 800 |
| flash point/°C | 12 |
| combustion heat/(KJ/kg) | 29,710 |
| boiling/°C | 78.4 |
| latent heat of evaporation/(KJ/kg) | 845.2 |
| Boundary Condition | Setting Parameters |
|---|---|
| wall | TITLE MATERIAL |
| bottom and top surfaces | STEEL |
| Fire Source Size | 20 cm × 20 cm |
| Type of reaction | C2H6O |
| Fire source power | 10.9 kW |
| Measured Physical Quantity | Measurement Settings |
|---|---|
| Temperature | thermocouple |
| oxygen concentration | Gas-phase Device (Species: Oxygen) |
| Carbon dioxide concentration | Gas-phase Device (Species: Carbon Dioxide) |
| air velocity | Gas-phase Device (Velocity) |
| heat release rate | Heat Release Rate Device |
| ventilation resistance | Gas-phase Device(pressure) |
| Flue gas height | Layer zoning Device |
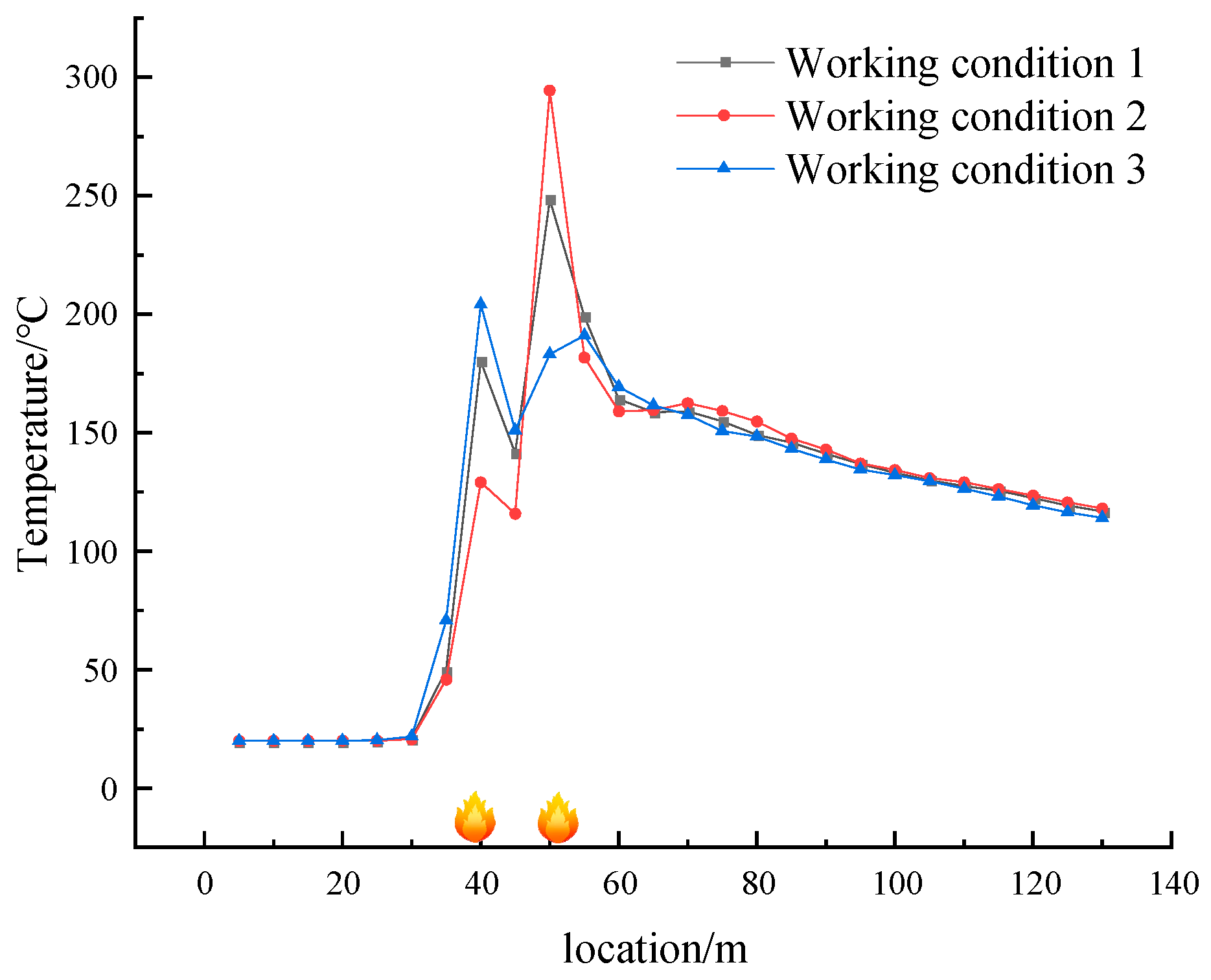
| Serial Number | Distance between Fire Sources D | HRR | Critical Wind Speed |
|---|---|---|---|
| single origin of fire | / | 3 MW | 1.8 m/s |
| working condition 1 | 10 m | 3 MW + 3 MW | 2.3 m/s |
| working condition 2 | 10 m | 3 MW + 4 MW | 2.1 m/s |
| working condition 3 | 10 m | 4 MW + 3 MW | 2.1 m/s |
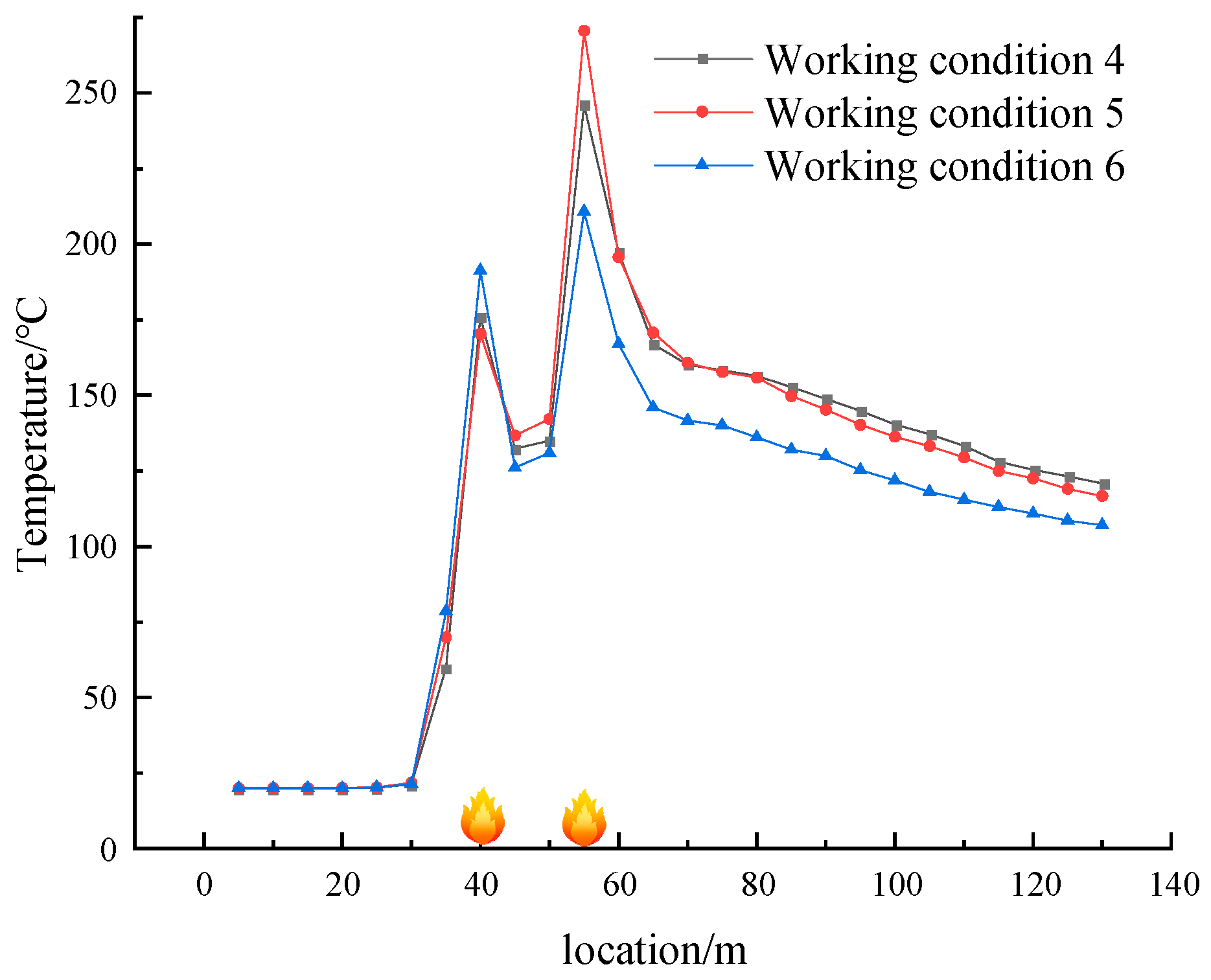
| working condition 4 | 15 m | 3 MW + 3 MW | 2.2 m/s |
| working condition 5 | 15 m | 3 MW + 4 MW | 2.0 m/s |
| working condition 6 | 15 m | 4 MW + 3 MW | 2.0 m/s |
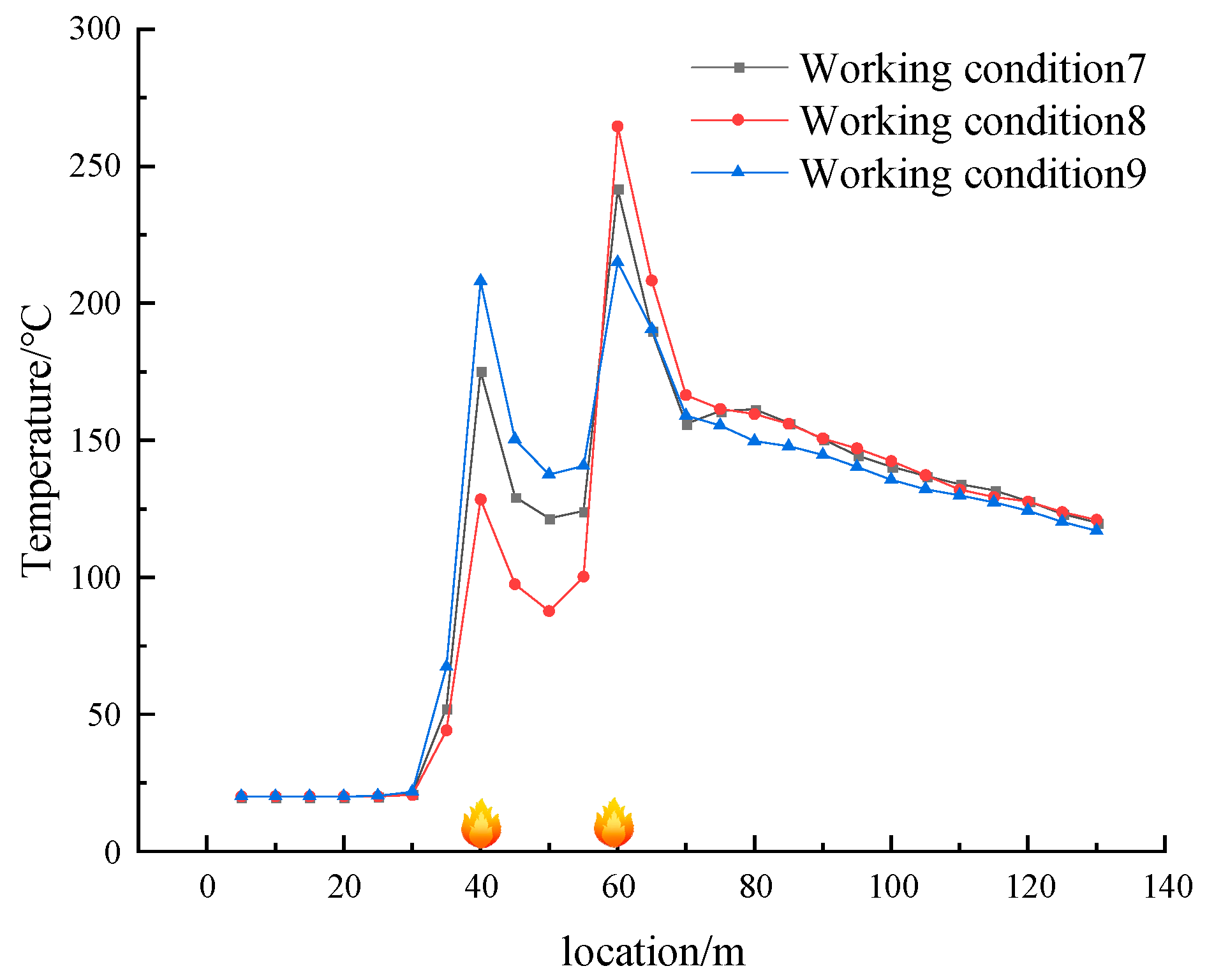
| working condition 7 | 20 m | 3 MW + 3 MW | 1.9 m/s |
| working condition 8 | 20 m | 3 MW + 4 MW | 1.9 m/s |
| working condition 9 | 20 m | 4 MW + 3 MW | 1.9 m/s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; Ni, M.; Wang, W.; Wang, H.; Wang, J. Study on the Fire Characteristics of Dual Fire Sources and the Difference in Power Temperature of Different Fire Sources in Tunnel. Fire 2024, 7, 273. https://doi.org/10.3390/fire7080273
Zhao X, Ni M, Wang W, Wang H, Wang J. Study on the Fire Characteristics of Dual Fire Sources and the Difference in Power Temperature of Different Fire Sources in Tunnel. Fire. 2024; 7(8):273. https://doi.org/10.3390/fire7080273
Chicago/Turabian StyleZhao, Xiaokun, Minghao Ni, Wencai Wang, Hongwei Wang, and Jianing Wang. 2024. "Study on the Fire Characteristics of Dual Fire Sources and the Difference in Power Temperature of Different Fire Sources in Tunnel" Fire 7, no. 8: 273. https://doi.org/10.3390/fire7080273
APA StyleZhao, X., Ni, M., Wang, W., Wang, H., & Wang, J. (2024). Study on the Fire Characteristics of Dual Fire Sources and the Difference in Power Temperature of Different Fire Sources in Tunnel. Fire, 7(8), 273. https://doi.org/10.3390/fire7080273








