Mechanism of Spontaneous Acceleration of Slow Flame in Channel
Abstract
1. Introduction
2. Problem Setup
3. Results and Discussion
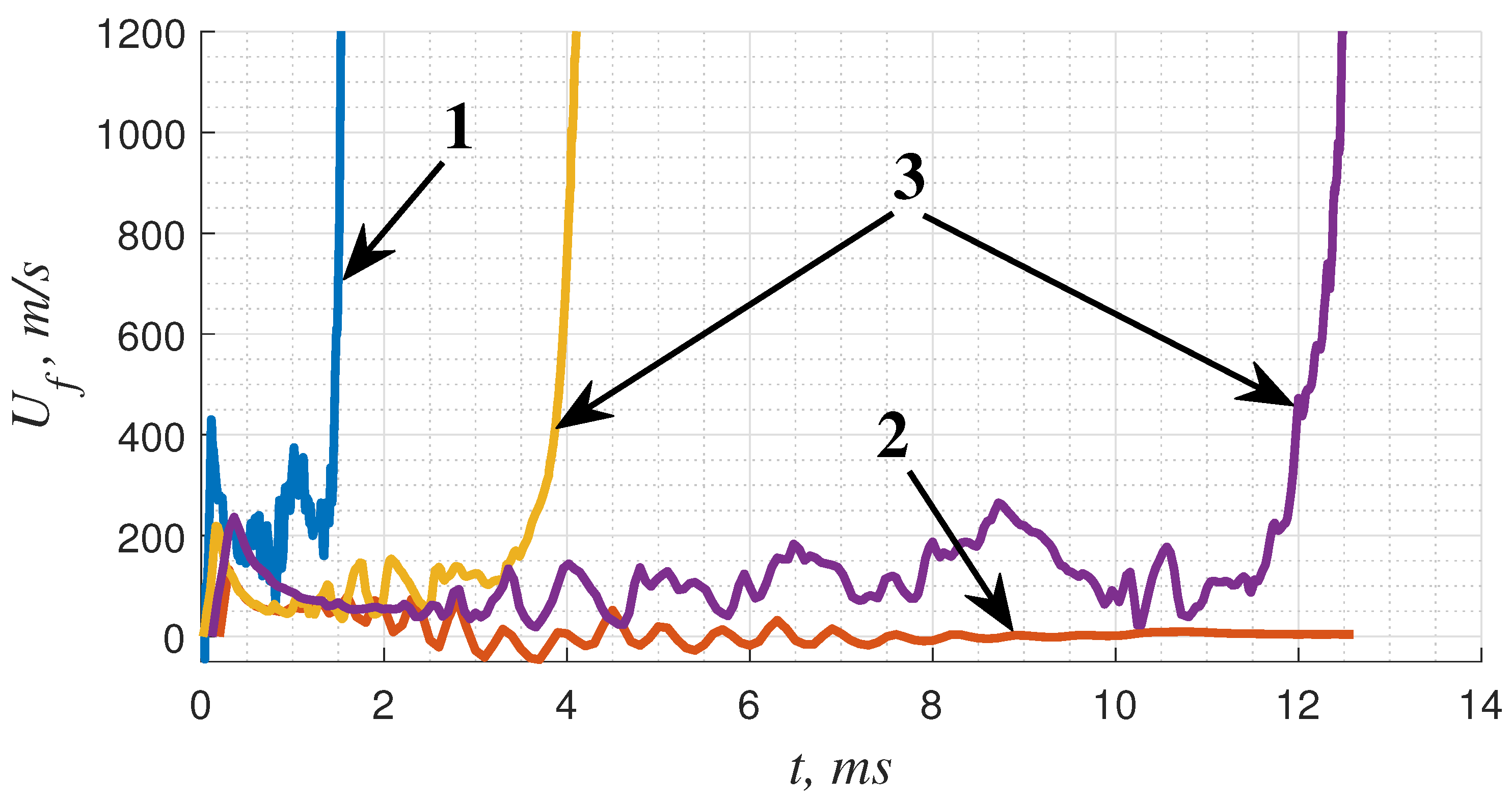
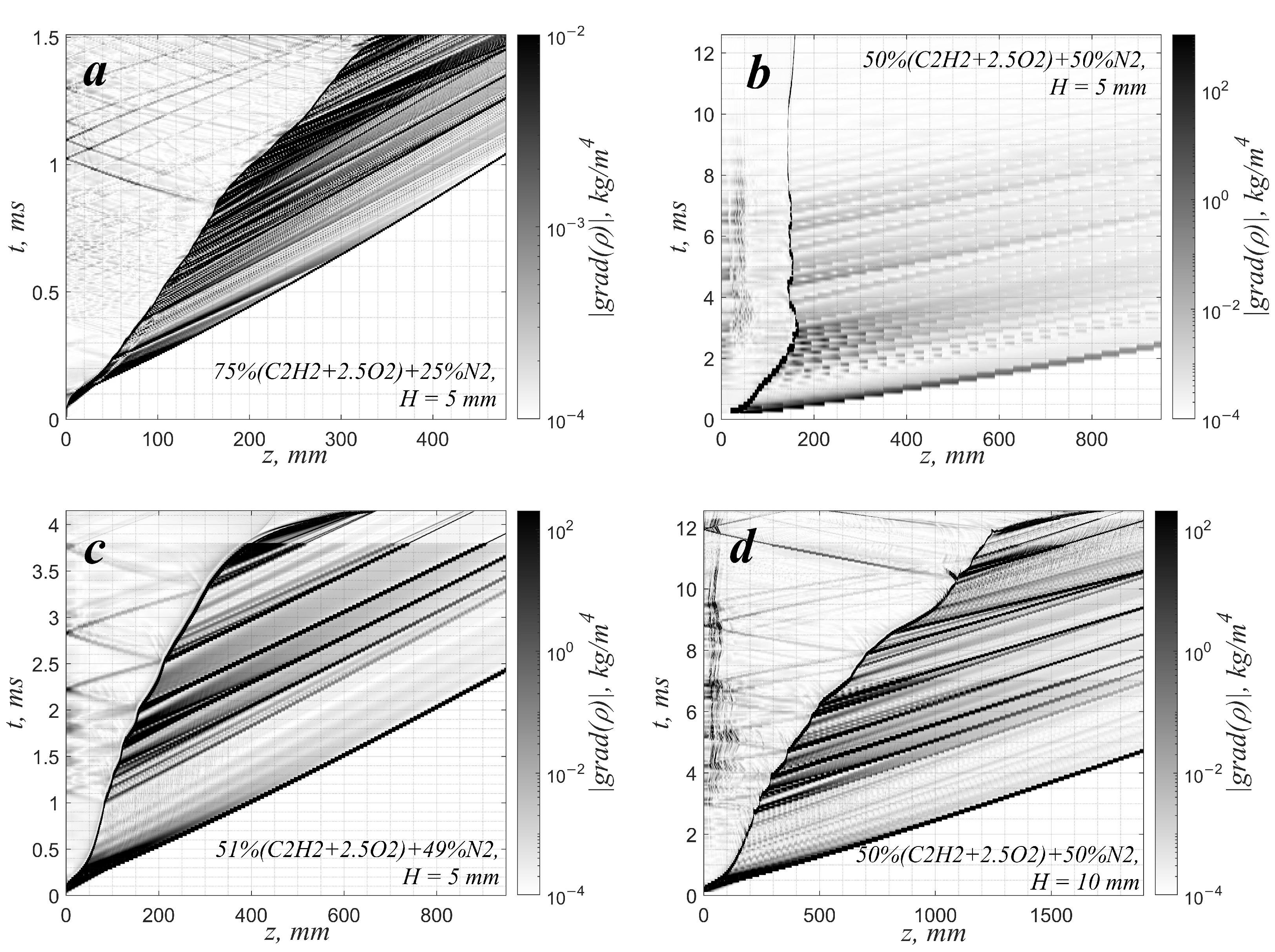
3.1. General Pattern of Flame Dynamics in Narrow Channel
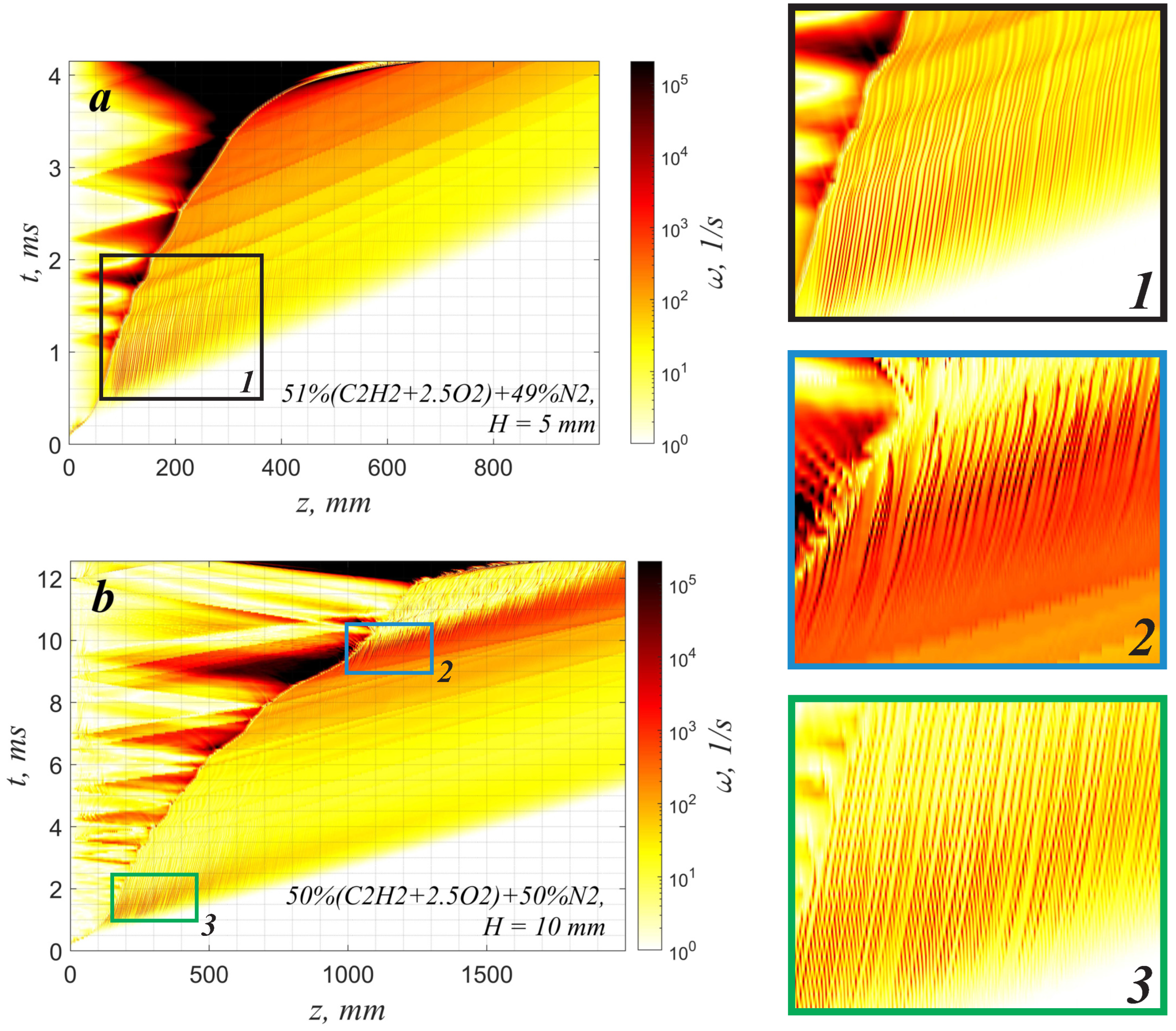
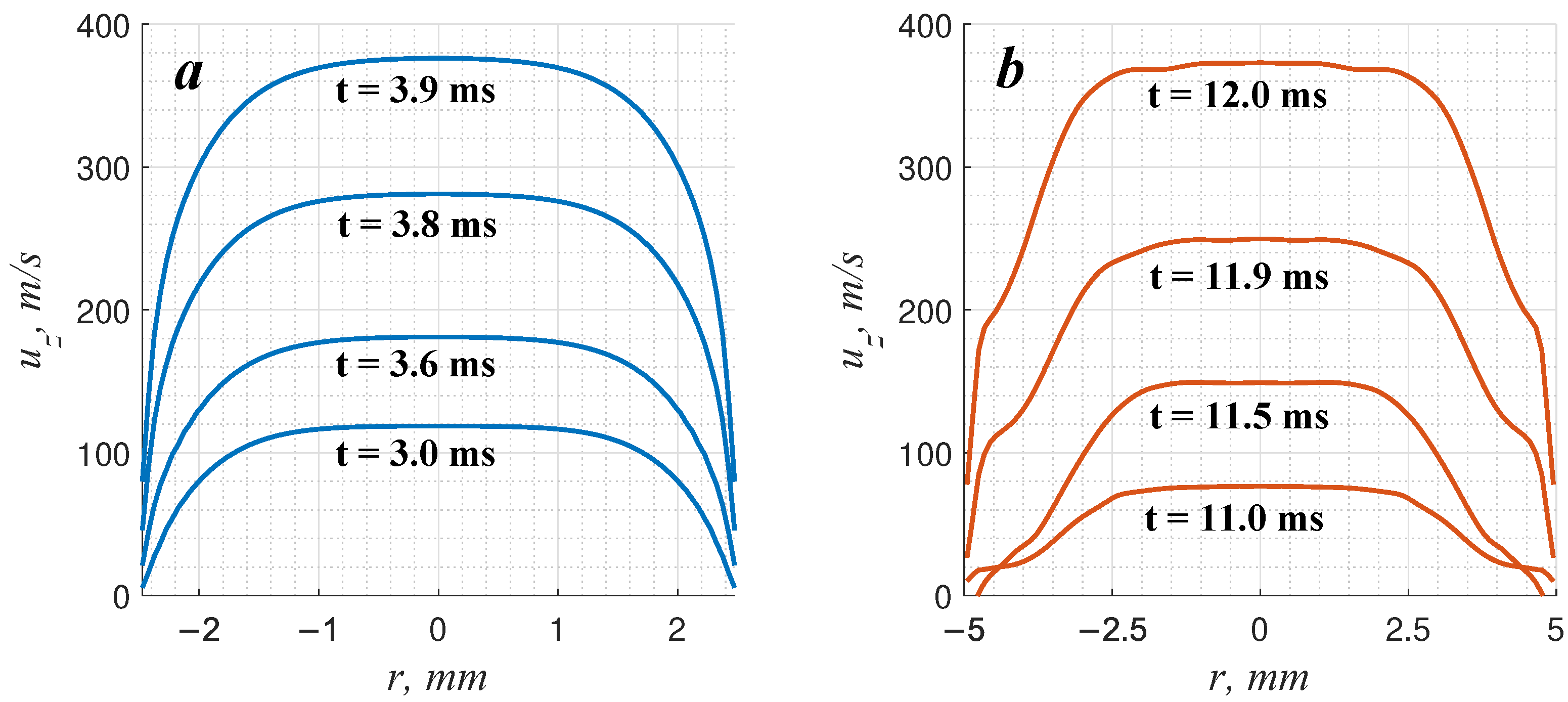
3.2. Boundary-Layer Evolution and Spontaneous Flame Acceleration
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Venetsanos, A.G.; Huld, T.; Adams, P.; Bartzis, J.G. Source, dispersion and combustion modelling of an accidental release of hydrogen in an urban environment. J. Hazard. Mater. 2003, 105, 1–25. [Google Scholar] [CrossRef]
- Wang, X.; Li, B.; Han, B.; Jin, X.; Zhang, D.; Bi, M. Explosion of high pressure hydrogen tank in fire: Mechanism, criterion, and consequence assessment. J. Energy Storage 2023, 72, 108455. [Google Scholar] [CrossRef]
- Xu, Y.; Huang, Y.; Ma, G. A review on effects of different factors on gas explosions in underground structures. Undergr. Space 2020, 5, 298–314. [Google Scholar] [CrossRef]
- International Atomic Energy Agency. Mitigation of Hydrogen Hazards in Severe Accidents in Nuclear Power Plants; Technical Report IAEA-TECDOC-1661; International Atomic Energy Agency: Vienna, Austria, 2011. [Google Scholar]
- Efremov, V.P.; Ivanov, M.F.; Kiverin, A.D.; Utkin, A.V. Shock-wave dynamics during oil-filled transformer explosions. Shock Waves 2017, 27, 517–522. [Google Scholar] [CrossRef]
- He, L.; Lee, J.H. The dynamical limit of one-dimensional detonations. Phys. Fluids 1995, 7, 1151–1158. [Google Scholar] [CrossRef]
- Zeldovich, Y. Regime classification of an exothermic reaction with nonuniform initial conditions. Combust. Flame 1980, 39, 211–214. [Google Scholar] [CrossRef]
- Kiverin, A.; Yakovenko, I. Ignition and detonation onset behind incident shock wave in the shock tube. Combust. Flame 2019, 204, 227–236. [Google Scholar] [CrossRef]
- Zel’dovich, Y.B.; Rozlovskii, A.I. On the onset of unstable normal burning: Transition of a spherical flame to detonation. Dokl. Akad. Nauk SSSR 1947, 57, 365–368. (In Russian) [Google Scholar]
- Liberman, M.A.; Ivanov, M.F.; Peil, O.E.; Valiev, D.M.; Eriksson, L.E. Self-acceleration and fractal structure of outward freely propagating flames. Phys. Fluids 2004, 16, 2476–2482. [Google Scholar] [CrossRef]
- Kiverin, A.; Yakovenko, I. Mechanism of transition to detonation in unconfined volumes. Acta Astronaut. 2020, 176, 647–652. [Google Scholar] [CrossRef]
- Clanet, C.; Searby, G. On the “tulip flame” phenomenon. Combust. Flame 1996, 105, 225–238. [Google Scholar] [CrossRef]
- Qian, C.; Liberman, M.A. On the mechanism of “tulip flame” formation: The effect of ignition sources. Phys. Fluids 2023, 35, 116122. [Google Scholar] [CrossRef]
- Yarkov, A.; Kiverin, A.; Yakovenko, I. Effect of channel geometry on the flame acceleration and transition to detonation in acetylene-oxygen-nitrogen mixtures. Acta Astronaut. 2024, 217, 273–279. [Google Scholar] [CrossRef]
- Urtiew, P.A.; Oppenheim, A.K. Experimental Observations of the Transition to Detonation in an Explosive Gas. Proc. R. Soc. London Ser. A 1966, 295, 13–28. [Google Scholar] [CrossRef]
- Kuznetsov, M.; Alekseev, V.; Matsukov, I.; Dorofeev, S. DDT in a smooth tube filled with a hydrogen–oxygen mixture. Shock Waves 2005, 14, 205–215. [Google Scholar] [CrossRef]
- Krivosheyev, P.; Novitski, A.; Penyazkov, O. Flame front dynamics, shape and structure on acceleration and deflagration-to-detonation transition. Acta Astronaut. 2023, 204, 692–704. [Google Scholar] [CrossRef]
- Wu, M.H.; Kuo, W.C. Accelerative expansion and DDT of stoichiometric ethylene/oxygen flame rings in micro-gaps. Proc. Combust. Inst. 2013, 34, 2017–2024. [Google Scholar] [CrossRef]
- Ciccarelli, G.; Johansen, C.; Kellenberger, M. High-speed flames and DDT in very rough-walled channels. Combust. Flame 2013, 160, 204–211. [Google Scholar] [CrossRef]
- Rudy, W.; Teodorczyk, A. Numerical Simulations of DDT Limits in Hydrogen-Air Mixtures in Obstacle Laden Channel. Energies 2021, 14, 24. [Google Scholar] [CrossRef]
- Shamshin, I.O.; Aksenov, V.S.; Kazachenko, M.V.; Gusev, P.A.; Frolov, S.M. Fast Deflagration-to-Detonation Transition in Helical Tubes. Processes 2023, 11, 1719. [Google Scholar] [CrossRef]
- Bivol, G.Y.; Golovastov, S.; Golub, V. Prechamber initiation of gaseous detonation in a channel. Combust. Sci. Technol. 2016, 188, 1165–1179. [Google Scholar] [CrossRef]
- Shamshin, I.O.; Kazachenko, M.V.; Frolov, S.M.; Basevich, V.Y. Deflagration-to-Detonation Transition in Stochiometric Propane–Hydrogen–Air Mixtures. Fuels 2022, 3, 667–681. [Google Scholar] [CrossRef]
- Kuznetsov, M.; Denkevits, A.; Veser, A.; Friedrich, A. Flame propagation regimes and critical conditions for flame acceleration and detonation transition for hydrogen-air mixtures at cryogenic temperatures. Int. J. Hydrogen Energy 2022, 47, 30743–30756. [Google Scholar] [CrossRef]
- Johansen, C.; Ciccarelli, G. Numerical simulations of the flow field ahead of an accelerating flame in an obstructed channel. Combust. Theory Model. 2010, 14, 235–255. [Google Scholar] [CrossRef]
- Boeck, L.; Lapointe, S.; Melguizo-Gavilanes, J.; Ciccarelli, G. Flame propagation across an obstacle: OH-PLIF and 2-D simulations with detailed chemistry. Proc. Combust. Inst. 2017, 36, 2799–2806. [Google Scholar] [CrossRef]
- Smirnov, N.; Nikitin, V.; Phylippov, Y.G. Deflagration-to-detonation transition in gases in tubes with cavities. J. Eng. Phys. Thermophys. 2010, 83, 1287–1316. [Google Scholar] [CrossRef]
- Cross, M.; Ciccarelli, G. DDT and detonation propagation limits in an obstacle filled tube. J. Loss Prev. Process. Ind. 2015, 36, 380–386. [Google Scholar] [CrossRef]
- Ivanov, M.; Kiverin, A.; Liberman, M. Hydrogen-oxygen flame acceleration and transition to detonation in channels with no-slip walls for a detailed chemical reaction model. Phys. Rev. E 2011, 83, 056313. [Google Scholar] [CrossRef]
- Smirnov, N.N.; Tyurnikov, M.V. Experimental investigation of deflagration to detonation transition in hydrocarbon-air gaseous mixtures. Combust. Flame 1995, 100, 661–668. [Google Scholar] [CrossRef]
- Thomas, G.; Ward, S.; Williams, R.; Bambrey, R. On critical conditions for detonation initiation by shock reflection from obstacles. Shock Waves 2002, 12, 111–119. [Google Scholar] [CrossRef]
- Medvedev, S.P.; Polenov, A.N.; Khomik, S.V.; Gel’fand, B.E. Deflagration-to-detonation transition in air-binary fuel mixtures in an obstacle-laden channel. Russ. J. Phys. Chem. B 2010, 4, 70–74. [Google Scholar] [CrossRef]
- Kiverin, A.D.; Yakovenko, I.S. Estimation of critical conditions for deflagration-to-detonation transition in obstructed channels filled with gaseous mixtures. Math. Model. Nat. Phenom. 2018, 13, 54. [Google Scholar] [CrossRef]
- Rakotoarison, W.; Vilende, Y.; Pekalski, A.; Radulescu, M.I. Model for Chapman-Jouguet deflagrations in open ended tubes with varying vent ratios. Combust. Flame 2024, 260, 113212. [Google Scholar] [CrossRef]
- Wang, C.; Wu, S.; Zhao, Y.; Addai, E.K. Experimental investigation on explosion flame propagation of H2-O2 in a small scale pipeline. J. Loss Prev. Process. Ind. 2017, 49, 612–619. [Google Scholar] [CrossRef]
- Bykov, V.; Koksharov, A.; Kuznetsov, M.; Zhukov, V. Hydrogen-oxygen flame acceleration in narrow open ended channels. Combust. Flame 2022, 238, 111913. [Google Scholar] [CrossRef]
- Kuo, K.K.; Acharya, R. Fundamentals of Turbulent and Multiphase Combustion, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Kiverin, A.; Yarkov, A.; Yakovenko, I. On the Features of Numerical Simulation of Hydrogen Self-Ignition under High-Pressure Release. Computation 2024, 12, 103. [Google Scholar] [CrossRef]
- Varatharajan, B.; Williams, F. Chemical-kinetic descriptions of high-temperature ignition and detonation of acetylene-oxygen-diluent systems. Combust. Flame 2001, 124, 624–645. [Google Scholar] [CrossRef]
- McBridge, B.; Gordon, S.; Reno, M. Coefficients for calculating thermodynamic and transport properties of individual species. In NASA Technical Memorandum; NASA Langley Research Center: Hampton, VA, USA, 1993; Volume 4513. [Google Scholar]
- Karabasov, S.A.; Goloviznin, V.M. Compact Accurately Boundary-Adjusting high-REsolution Technique for fluid dynamics. J. Comput. Phys. 2009, 228, 7426–7451. [Google Scholar] [CrossRef]
- Yakovenko, I.; Kiverin, A. Numerical Modeling of Hydrogen Combustion: Approaches and Benchmarks. Fire 2023, 6, 239. [Google Scholar] [CrossRef]
- Han, W.; Huang, J.; Gu, G.; Wang, C.; Law, C.K. Surface heat loss and chemical kinetic response in deflagration-to-detonation transition in microchannels. Phys. Rev. Fluids 2020, 5, 053201. [Google Scholar] [CrossRef]
- Kurylo, J.; Dwyer, H.A.; Oppenheim, A.K. Numerical Analysis of Flowfields Generated by Accelerating Flames. AIAA J. 1980, 18, 302–308. [Google Scholar] [CrossRef][Green Version]
- Deshaies, B.; Joulin, G. Flame-speed sensitivity to temperature changes and the deflagration-to-detonation transition. Combust. Flame 1989, 77, 201–212. [Google Scholar] [CrossRef]
- Kiverin, A.D.; Yakovenko, I.S. Evolution of wave patterns and temperature field in shock-tube flow. Phys. Rev. Fluids 2018, 3, 053201. [Google Scholar] [CrossRef]
- Ellzey, J.L.; Picone, M.; Oran, E.S. The Interaction of a Shock with a Compressible Vortex; Naval Research Laboratory, Senior Science for Reactive Flow Physics Branch: Washington, DC, USA, 1992. [Google Scholar]
- Kuznetsov, M.; Yanez, J.; Grune, J. Flame acceleration and DDT in a torus geometry. In Proceedings of the 30th International Symposium on Shock Waves 1: ISSW30-Volume 1, Tel-Aviv, Israel, 19–24 July 2015; Springer: Berlin/Heidelberg, Germany, 2017; pp. 385–389. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yarkov, A.; Yakovenko, I.; Kiverin, A. Mechanism of Spontaneous Acceleration of Slow Flame in Channel. Fire 2024, 7, 362. https://doi.org/10.3390/fire7100362
Yarkov A, Yakovenko I, Kiverin A. Mechanism of Spontaneous Acceleration of Slow Flame in Channel. Fire. 2024; 7(10):362. https://doi.org/10.3390/fire7100362
Chicago/Turabian StyleYarkov, Andrey, Ivan Yakovenko, and Alexey Kiverin. 2024. "Mechanism of Spontaneous Acceleration of Slow Flame in Channel" Fire 7, no. 10: 362. https://doi.org/10.3390/fire7100362
APA StyleYarkov, A., Yakovenko, I., & Kiverin, A. (2024). Mechanism of Spontaneous Acceleration of Slow Flame in Channel. Fire, 7(10), 362. https://doi.org/10.3390/fire7100362




