Application of Analytic Hierarchy Process to Rank Fire Safety Factors for Assessing the Fire Probabilistic Risk in School for the Blind Building: A Case Study in Thailand
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Design and Setting
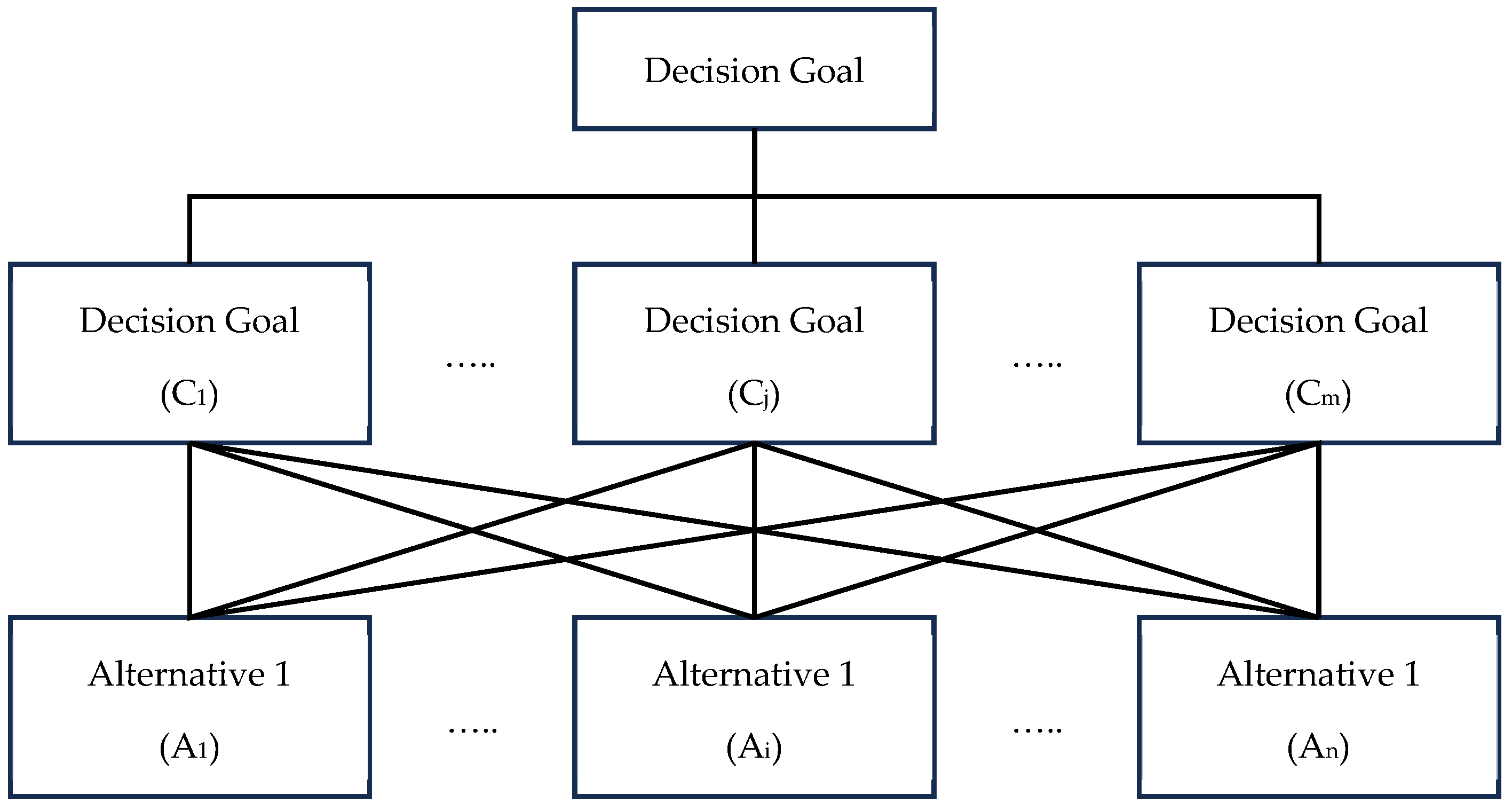
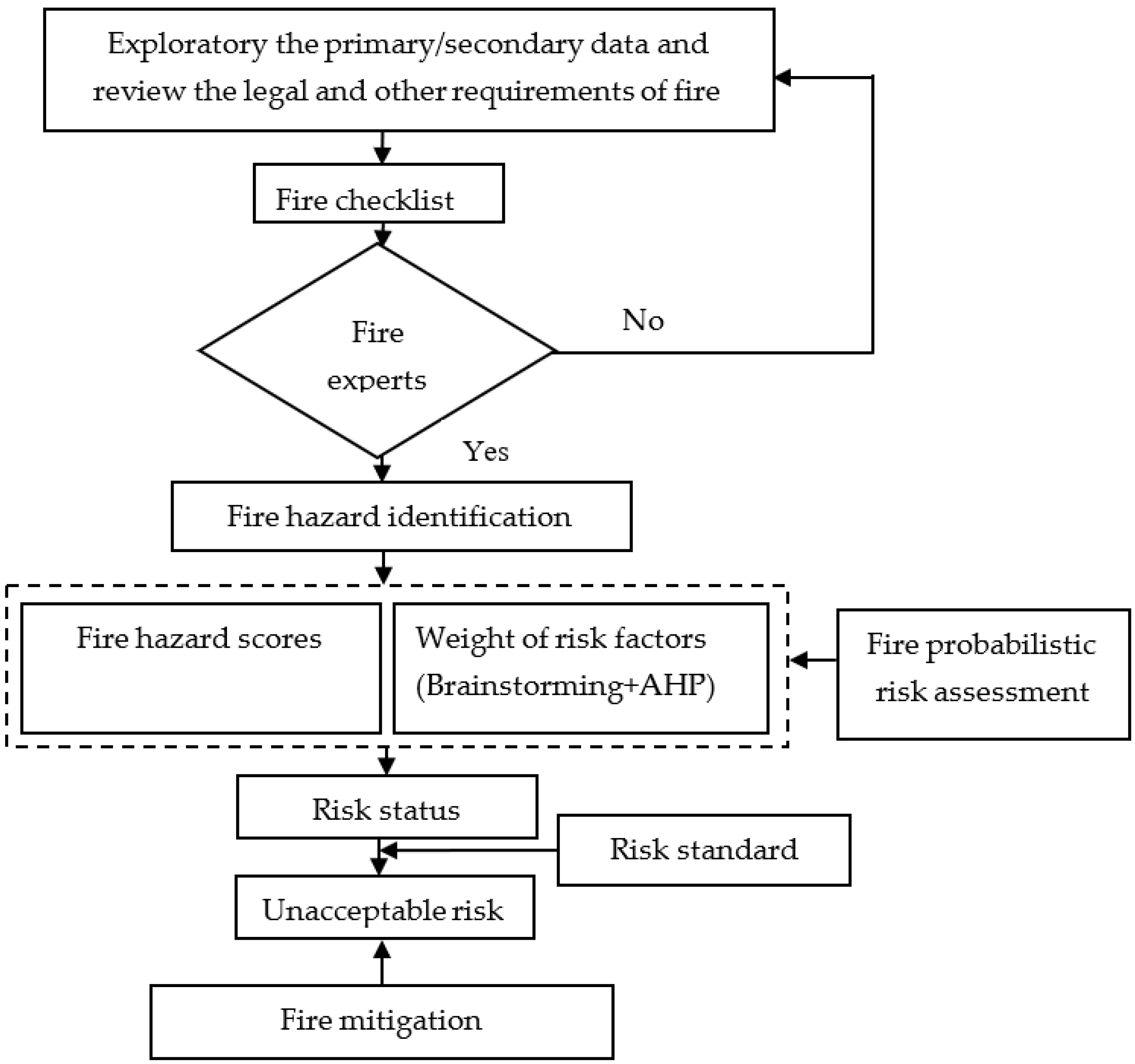
2.2. Instrument Development
2.3. Order of Operations Steps
- R = Fire probabilistic risk scores.
- n = The number of fire probabilistic risk assessment index.
- Wci = Weight of fire probabilistic risk assessment indicator i, ranging from 0 to 1.
- Pci = Scores of fire probabilistic risk assessment indicator i, ranging from 1 to 5.
3. Results
- (1)
- Relative weight calculation and the maximum eigenvalue (λmax) for each matrix based on the number of decision criteria (n).
- (2)
- Consistency index calculation for each matrix based on the number of decision criteria (n) CI = (λmax − n)/(n − 1) = (3.06 − 3)/(3 − 1) ≈ 0.03.
- (3)
- Consistency ratio calculation, which was C.R. = CI/RI = 0.03/0.58 ≈ 0.06.
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fire Accident Reports during 2015–2022. 2022. Available online: http://www.disaster.go.th/ (accessed on 5 December 2022).
- Prashant, T. The Essential Aspects of Fire Safety Management in High-Rise Buildings; Universiti Teknologi Malaysia: Shah Alam, Malaysia, 2007. [Google Scholar]
- Moore, J. Assessment of Fire Safety and Evacuation Management in Nursing Homes; Dublin Institute of Technology: Dublin, Ireland, 2012. [Google Scholar]
- Yeung, C.H. A Study on the Fire Safety Management of Public Rental Housing in Hong Kong; The City University of Hong Kong: Kowloon Tong, Hong Kong, 2012. [Google Scholar]
- Tanima, A.W. Impact of Facility Management on Fire Safety Crisis in Bangladesh’s AEC Industry; Metropolia University of Applied Sciences: Helsinki, Finland, 2018. [Google Scholar]
- Ayyappa, T.M. Risk Acceptance in Fire Safety Engineering: Development of Reference Case Studies; Ghent University: Ghent, Belgium, 2019. [Google Scholar]
- Saaty, T.L. How to make a decision: The analytic hierarchy process. Eur. J. Occup. Res. 1990, 48, 9–26. [Google Scholar] [CrossRef]
- Contreras, F.; Hanaki, K.; Aramaki, T.; Connors, S. Application of analytical hierarchy process to analyze stakeholders’ preferences for municipal solid waste management plans. Resour. Conserv. Recycl. 2008, 52, 979–991. [Google Scholar] [CrossRef]
- Geneletti, D. Combining stakeholder analysis and spatial multicriteria evaluation to select and rank inert landfill sites. Waste Manag. 2010, 30, 328–337. [Google Scholar] [CrossRef] [PubMed]
- Achillas, C.H.; Vlachokostas, C.H.; Moussiopoulos, N.; Banias, G. Prioritize strategies to confront environmental deterioration in urban areas: Multicriteria assessment of public opinion and experts’ views. Cities 2011, 28, 414–423. [Google Scholar] [CrossRef]
- Jirattinart, T.; Saowanee, W.; Thavivongse, S.; Chiyuth, S. A review of the analytical hierarchy process (AHP): An approach to water resource management in Thailand. Appl. Environ. Res. 2015, 37, 13–32. [Google Scholar]
- Li, G.-F.; Xiang, X.Y.; Tong, Y.Y.; Wang, H.M. Impact assessment of urbanization on flood risk in the Yangtze River Delta. Stoch. Environ. Res. Risk Assess. 2013, 27, 1683–1693. [Google Scholar] [CrossRef]
- Yang, X.; Ding, J.-H.; Hou, H. Application of a triangular fuzzy AHP approach for flood risk evaluation and response measures analysis. Nat. Hazards 2013, 68, 657–674. [Google Scholar] [CrossRef]
- Bottero, M.; Comino, E.; Riggio, V. Application of the analytic hierarchy process and the analytic network process for the assessment of different wastewater treatment systems. Environ. Model. Softw. 2011, 26, 1211–1224. [Google Scholar] [CrossRef]
- Aminbakhsh, S.; Gunduz, M.; Sonmez, R. Safety risk assessment using analytic hierarchy process (AHP) during planning and budgeting of construction projects. J. Saf. Res. 2013, 46, 95–105. [Google Scholar] [CrossRef]
- Hoscan, O.; Cetinyokus, S. Determination of emergency assembly point for industrial accidents with AHP analysis. J. Loss Prev. Process Ind. 2021, 69, 104386. [Google Scholar] [CrossRef]
- Saaty, T.L. The Analytic Hierarchy Process; Mc Graw Hill: New York, NY, USA, 1980; ISBN 978-0-070-54371-3. [Google Scholar]
- Chan, F.T.S.; Chan, H.K. An AHP model for selection of suppliers in the fast-changing fashion market. Int. J. Adv. Manuf. Technol. 2010, 51, 9–12. [Google Scholar] [CrossRef]
- Najafi, A.; Afrazeh, A. Analysis of the environmental projects risk management success using Analytical Network Process Approach. Int. J. Environ. Res. 2011, 5, 277–284. [Google Scholar]
- Yatsalo, B.I.; Kiker, G.A.; Kim, J.; Bridges, T.S.; Seager, T.P.; Gardner, K.; Satterstrom, F.K.; Linkov, I. Application of multicriteria decision analysis tools to two contaminated sediment case studies. Integr. Environ. Assess Manag. 2007, 3, 223–233. [Google Scholar] [CrossRef] [PubMed]
- Saracoglu, B.O. Selecting industrial investment locations in master plans of countries. Eur. J. Ind. Eng. 2013, 7, 416–441. [Google Scholar] [CrossRef]
- Pažek, K.; Rozman, Č.; Bavec, F.; Borec, A.; Bavec, M. A multi-criteria decision analysis framework tool for the selection of farm business models on organic mountain farms. J. Sustain. Agric. 2010, 34, 778–799. [Google Scholar] [CrossRef]
- French, S.; Xu, D.L. Comparison study of multi-attribute decision analytic software. J. Multi-Criteria Decis. Anal. 2005, 13, 65–80. [Google Scholar] [CrossRef]
- Hyun, K.C.; Min, S.; Choi, H.; Park, J.; Lee, I.M. Risk analysis using fault-tree analysis (FTA) and analytic hierarchy process (AHP) applicable to shield TBM tunnels. Tunn. Undergr. Space Technol. 2015, 49, 121–129. [Google Scholar] [CrossRef]
- Alberto, P.; Evanthia, G.; Ewan, D.; Jie, G.; Maria, C.L. Applying analytic hierarchy process (AHP) to choose a human factors technique: Choosing the suitable human reliability analysis technique for the automotive industry. Saf. Sci. 2019, 119, 229–239. [Google Scholar]
- Ali, K.; Ulviye, P.; Cansu, D. A new approximation for risk assessment using the AHP and Fine Kinney methodologies. Saf. Sci. 2017, 91, 24–32. [Google Scholar]
- Maria, G.S.; Reinhard, M. AHP-based risk analysis of energy performance contracting projects in Russia. Energy Policy 2016, 97, 559–581. [Google Scholar]
- Emre, A.; Oacan, A.; Osman, T. Application of fuzzy logic to fault tree and event tree analysis of the risk for cargo liquefaction on board ship. Appl. Ocean. Res. 2020, 101, 102238. [Google Scholar]
- Valeo, M.; Nassif, H.; Issa, L.; Capers, H., Jr.; Ozbay, K. Analytic hierarchy process to improve simple bridge security checklist. Transp. Res. Rec. J. Transp. Res. Board 2012, 2313, 201–207. [Google Scholar] [CrossRef]
- Bahrami, B.; Ghorbani Pour, R.; Rahimi, H.; Memari Nashalji, M. Occupational medicine status of Kashan industries by weighted checklist to analytic hierarchy process method 2019. Occup. Hyg. Health Promot. 2021, 5, 200–207. [Google Scholar]
- Memari Nashalji, M.; Bahrami, B.; Rahimi, H. Weighting the checklist for evaluating the performance of industrial occupational health experts using the analytic hierarchy process. Health Dev. J. 2020, 9, 254–262. [Google Scholar]
- Li, W.; Li, H.; Liu, Y.; Wang, S.; Pei, X.; Li, Q. Fire risk assessment of high-rise buildings under construction based on unascertained measure theory. PLoS ONE 2020, 15, 1–17. [Google Scholar] [CrossRef]
- Roshan, S.A.; Daneshvar, S. Fire risk assessment and its economic loss estimation in Tehran subway, Applying Event Tree Analysis. Iran. J. Health 2015, 2, 229–234. [Google Scholar]
- Lau, C.K.; Lai, K.K.; Yan, P.L.; Du, J. Fire risk assessment with scoring system, using the support vector machine approach. Fire Saf. J. 2015, 78, 188–195. [Google Scholar] [CrossRef]
- Omidvari, M.; Mansouri, N.; Nouri, J. A pattern of fire risk assessment and emergency management in educational center laboratories. Saf. Sci. 2015, 73, 34–42. [Google Scholar] [CrossRef]
- NFPA. NFPA 10: Standard for Portable Fire Extinguishers; NFPA: Quincy, MA, USA, 2018; ISBN 978-1-455-91659-7. [Google Scholar]

| Numerical Rating | Description for Risk Factor Evaluation |
|---|---|
| 1 | Equivalently |
| 2 | Equivalently to neutrally more |
| 3 | Neutrally more |
| 4 | Neutrally to greatly more |
| 5 | Greatly more |
| 6 | Greatly to very greatly more |
| 7 | Very greatly more |
| 8 | Very greatly to extremely more |
| 9 | Extremely more |
| Order (n) | Random Inconsistency Index (RI) |
|---|---|
| 1 | 0 |
| 2 | 0 |
| 3 | 0.58 |
| 4 | 0.90 |
| 5 | 1.12 |
| 6 | 1.24 |
| 7 | 1.32 |
| 8 | 1.41 |
| 9 | 1.45 |
| 10 | 1.49 |
| Score | Description |
|---|---|
| 1 | Remote possibility |
| 2 | Possible but not great possibility |
| 3 | Moderate possibility |
| 4 | Important possibility |
| 5 | Most possible |
| Criteria | Inherent Fire Hazard Factor | Grade Assessment (A) | Weighting Attribute (B) | Scores Attribute (A × B) |
|---|---|---|---|---|
| Building structure | ||||
| 1 | Height of the blind school building | 4 | 0.0625 | 0.2500 |
| 2 | Multilayer | 4 | 0.1375 | 0.5500 |
| 3 | Fire resistance rating | 3 | 0.3250 | 0.9750 |
| 4 | Hazard classification | 1 | 0.4750 | 0.4750 |
| Sum of A × B | (C) | 2.2500 | ||
| Relative weight of building structure | (D) | 0.1880 | ||
| Building structure scores | (C) × (D) | 0.4230 | ||
| Fire load | ||||
| 1 | Location area | 3 | 0.1700 | 0.5100 |
| 2 | Interior decoration | 3 | 0.8300 | 2.4900 |
| Sum of A × B | (C) | 3.0000 | ||
| Relative weight of fire load | (D) | 0.0810 | ||
| Fire load scores | (C) × (D) | 0.2430 | ||
| Ignition sources | ||||
| 1 | Electrical equipment | 3 | 0.6330 | 1.8990 |
| 2 | Type of combustible gas supply | 3 | 0.1070 | 0.3210 |
| 3 | External fire | 5 | 0.6330 | 1.3600 |
| Sum of A × B | (C) | 3.5800 | ||
| Relative weight of ignition sources | (D) | 0.7310 | ||
| Ignition sources scores | (C) × (D) | 2.6170 | ||
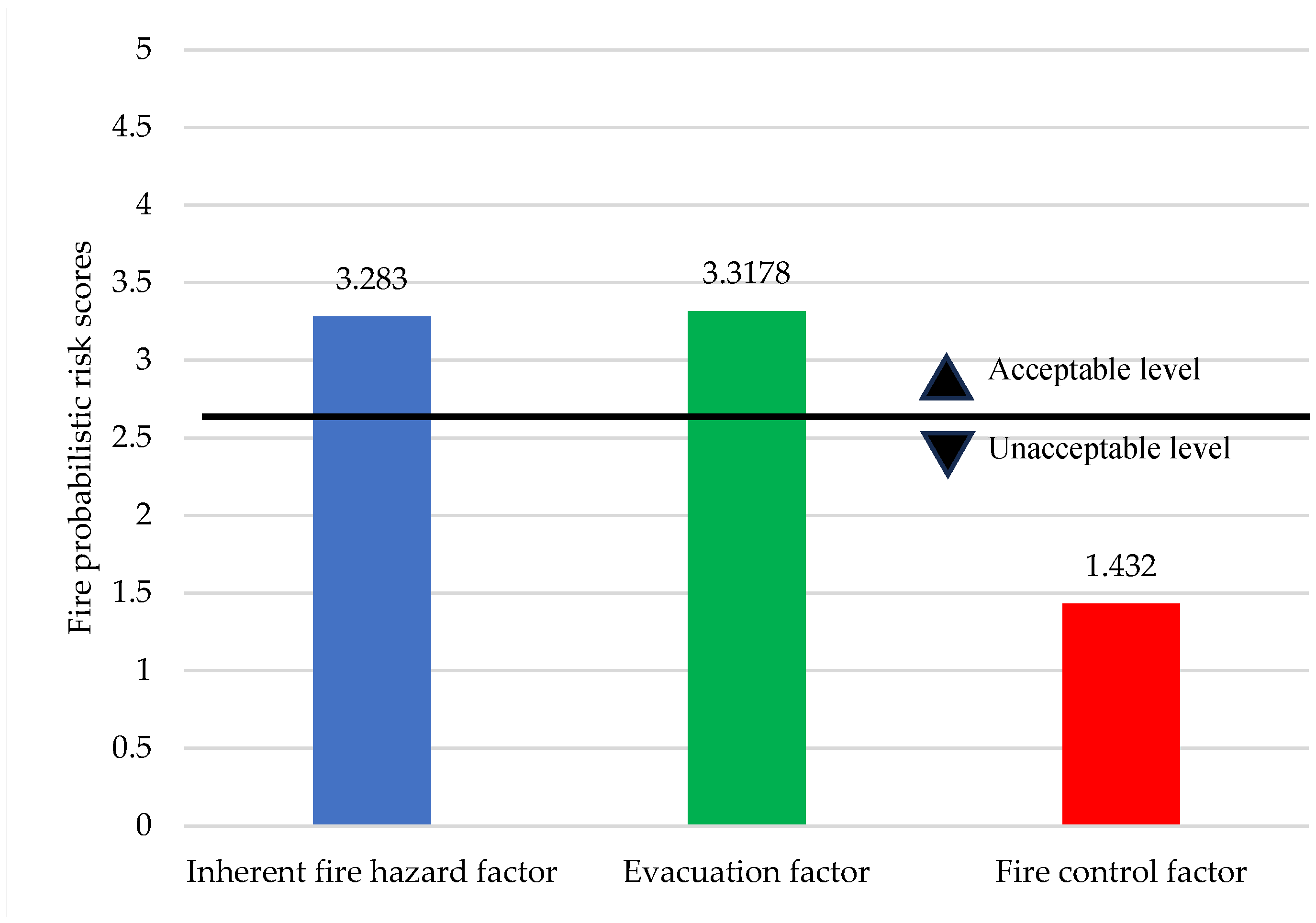
| Building structure scores + fire load scores + ignition sources scores | 3.2830 | |||
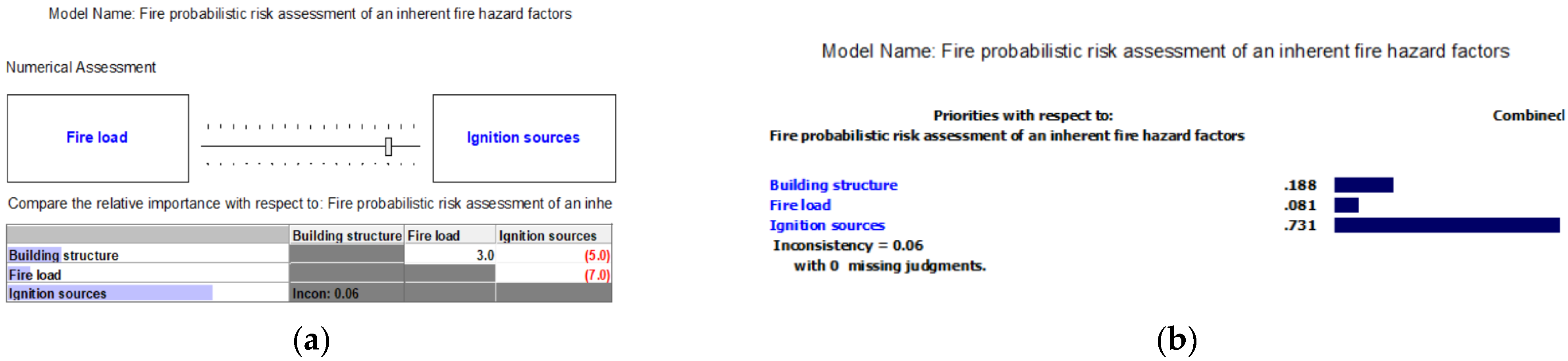
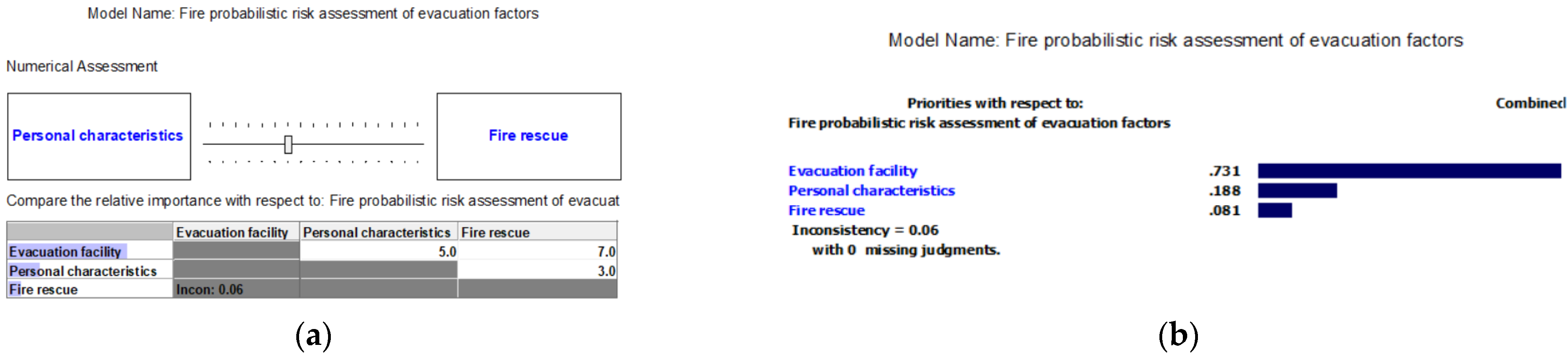
| Criteria (C.R. = 0.06 < 0.1) | Building Structure | Fire Load | Ignition Sources | Priority Value |
|---|---|---|---|---|
| Building structure | 1 | 3 | 1/5 | 0.188 |
| Fire load | 1/3 | 1 | 1/7 | 0.081 |
| Ignition sources | 5 | 7 | 1 | 0.731 |
| Sum | 6.33 | 11.00 | 1.34 | 1.000 |
| Calculation Guidelines | Sum of Value | Total | ||
|---|---|---|---|---|
| Vertical sum of value | 6.33 | 11.00 | 1.34 | |
| Horizontal sum of value | 0.188 | 0.081 | 0.731 | |
| Maximum eigenvalue (λmax) | 1.19 | 0.89 | 0.98 | 3.06 |
| Criteria | Fire Control Factor | Grade Assessment (A) | Weighting Attribute (B) | Scores Attribute (A × B) |
|---|---|---|---|---|
| Fire facility | ||||
| 1 | Fire alarm and fire control linkage system | 1 | 0.3520 | 0.3520 |
| 2 | Portable fire extinguisher | 3 | 0.2020 | 0.6060 |
| 3 | Floor plan | 1 | 0.0930 | 0.0930 |
| 4 | Safety sign | 1 | 0.1780 | 0.1780 |
| 5 | Emergency lighting | 1 | 0.1320 | 0.1320 |
| 6 | Lightning protection system | 5 | 0.0430 | 0.2150 |
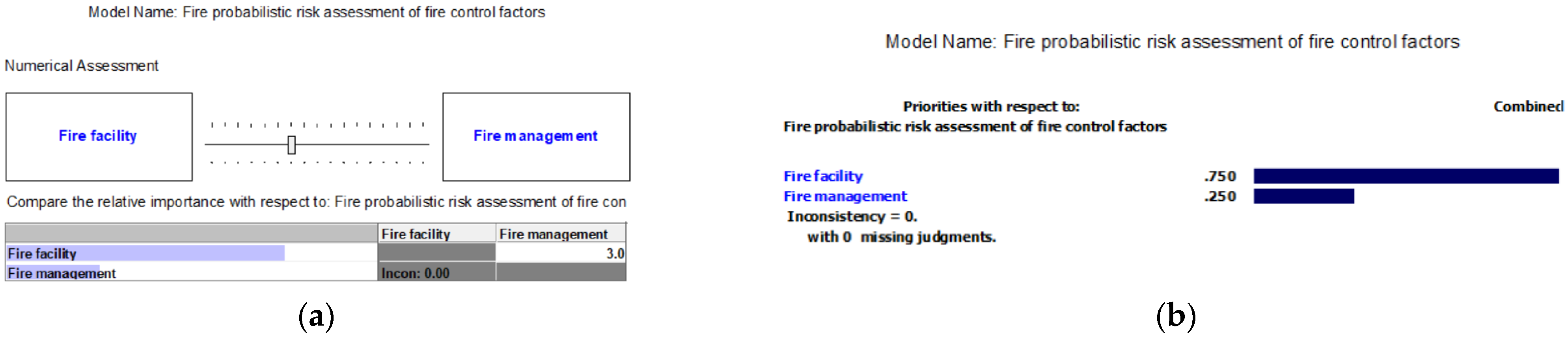
| Sum of A × B | (C) | 1.5760 | ||
| Relative weight of fire facility | (D) | 0.7500 | ||
| Fire facility scores | (C) × (D) | 1.1820 | ||
| Fire management | ||||
| 1 | Fire safety inspection | 1 | 0.5400 | 0.5400 |
| 2 | Basic firefighting | 1 | 0.2300 | 0.2300 |
| 3 | Fire drill | 1 | 0.0725 | 0.0725 |
| 4 | Fire emergency plan | 1 | 0.1575 | 0.1575 |
| Sum of A × B | (C) | 1.0000 | ||
| Relative weight of fire management | (D) | 0.2500 | ||
| Fire management scores | (C) × (D) | 0.2500 | ||
| Fire facility scores + fire management scores | 1.4320 | |||
| Criteria | Evacuation Factor | Grade Assessment (A) | Weighting Attribute (B) | Scores Attribute (A × B) |
|---|---|---|---|---|
| Evacuation facility | ||||
| 1 | Indoor fire exit stair | 5 | 0.1300 | 0.6500 |
| 2 | Fire exit door | 2 | 0.1040 | 0.2080 |
| 3 | Fire exit discharge | 1 | 0.0830 | 0.0830 |
| 4 | Emergency radio | 3 | 0.0390 | 0.1170 |
| 5 | Capacity of means of egress | 4 | 0.4540 | 1.8160 |
| 6 | Dead-end corridors | 5 | 0.1630 | 0.1630 |
| 7 | Escape equipment | 1 | 0.0270 | 0.0270 |
| Sum of A × B | (C) | 3.0640 | ||
| Relative weight of evacuation facility | (D) | 0.7310 | ||
| Evacuation facility scores | (C) × (D) | 2.2398 | ||
| Personal characteristics | ||||
| 1 | Crowd density | 3 | 0.1400 | 0.4200 |
| 2 | Number of blind students per teacher | 5 | 0.2900 | 1.4500 |
| 3 | Degree of familiarity with building | 3 | 0.5700 | 1.7100 |
| Sum of A × B | (C) | 3.5800 | ||
| Relative weight of personal characteristics | (D) | 0.1880 | ||
| Personal characteristics scores | (C) × (D) | 0.6730 | ||
| Fire rescue | ||||
| 1 | Distance from fire brigade | 5 | 0.5730 | 2.865 |
| 2 | Distance from hospital | 5 | 0.1400 | 0.700 |
| 3 | First aid kit | 5 | 0.2870 | 1.435 |
| Sum of A × B | (C) | 5.000 | ||
| Relative weight of fire rescue | (D) | 0.081 | ||
| Fire rescue scores | (C) × (D) | 0.4050 | ||
| Evacuation facility + personal characteristics + fire rescue | 3.3178 | |||
| Fire Probabilistic Risk Scores | 1 ≤ R ≤ 1.5 | 1.5 < R ≤ 2.5 | 2.5 < R ≤ 3.5 | 3.5 < R ≤ 4.5 | 4.5 < R ≤ 5 |
|---|---|---|---|---|---|
| Risk ranking | Class 1 | Class 2 | Class 3 | Class 4 | Class 5 |
| Risk status | worst | worse | good | better | best |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ketsakorn, A.; Phangchandha, R. Application of Analytic Hierarchy Process to Rank Fire Safety Factors for Assessing the Fire Probabilistic Risk in School for the Blind Building: A Case Study in Thailand. Fire 2023, 6, 354. https://doi.org/10.3390/fire6090354
Ketsakorn A, Phangchandha R. Application of Analytic Hierarchy Process to Rank Fire Safety Factors for Assessing the Fire Probabilistic Risk in School for the Blind Building: A Case Study in Thailand. Fire. 2023; 6(9):354. https://doi.org/10.3390/fire6090354
Chicago/Turabian StyleKetsakorn, Arroon, and Rujipun Phangchandha. 2023. "Application of Analytic Hierarchy Process to Rank Fire Safety Factors for Assessing the Fire Probabilistic Risk in School for the Blind Building: A Case Study in Thailand" Fire 6, no. 9: 354. https://doi.org/10.3390/fire6090354
APA StyleKetsakorn, A., & Phangchandha, R. (2023). Application of Analytic Hierarchy Process to Rank Fire Safety Factors for Assessing the Fire Probabilistic Risk in School for the Blind Building: A Case Study in Thailand. Fire, 6(9), 354. https://doi.org/10.3390/fire6090354





