1. Introduction
For the designing and assessment of the structural fire resistance, the EN 1991-1-2 (2002) [
1] defines five performance levels, based on the building importance. In order to satisfy the fixed performance level, different design solutions based on prescriptive or performance-based approaches can be adopted. The main difference between the two approaches is that the first one is based on standard fire resistance tests or empirical calculation methods, using nominal fire curves. In particular, the code provides three types of conventional fire curves (standard ISO834 [
2], hydrocarbon, and external nominal curve), selected according to the nature of the combustible materials in the compartment. On the other hand, the performance-based approach considers the complexity of structures and the inter-relationship between the various fire safety measures and systems, using specific natural fire curves, generally obtained by advanced thermo-fluid-dynamic analyses.
The first step of the performance design approach consists in the thermal input evaluation through the choice of the design fire scenarios, which represents qualitative description of the fire evolution, based on real fire key aspects (e.g., compartment dimension, ventilation, fire loads...). The choice of fire model is a fundamental aspect for many uses, such as open car parks, which are increasing, due to the growing number of vehicles in recent decades. The most effective way, in terms of performance, cost and simplicity to build these open car parks involves the use of a composite steel-concrete structure. During a fire, the flash-over condition is rarely reached in an open compartment, so the localized fires should be considered, which leads to the heating some elements. For the structural fire design, different models can be used, such as simplified ones or advanced numerical modelling. The EN 1991-1-2 (2002) [
1] suggests different simple models for localized fires, e.g., the models proposed by Heskestad [
3], Hasemi [
4] and the LOCAFI [
5] models, which can be applied in specific situations, and advanced numerical modelling as the computational fluid dynamics (CDF). As also said before, to assess the fire safety of an open car parks requires an accurate representation of the fire events. The modelling of the fire source is essential to better understand the evolution of the gas temperatures of a car park and for the following structural verification phases. In addition, the choice of an appropriate heat release rate, HRR, for vehicles and a realistic fire propagation are necessary to a reliable fire safety design of structural elements. In this context, the fire source modelling can influence the evolution of the gas temperature both in terms of maximum temperature θ
MAX reached, of heating rate and of time at which θ
MAX is reached. These aspects can influence not only the structural fire checks, but also the verification of human life safety and their evacuation. Indeed, steel car parks show high vulnerability mainly to the maximum temperatures of the fire, due to the steel mechanical properties degradation caused by the heating. While the concrete elements are also influenced by the time of exposure to the fire. In fact, as stated in a previous research [
6], the concrete structure is sensitive not only to the temperature peak reached during the fire, but also to the duration of each temperature level. So, to assess the structural elements exposed to fire is essential to create a reliable model that returns the gas temperatures closest to reality. Furthermore, a more accurate evaluation of the structural element temperature can lead to different indirect actions inside the structure, according to the reduction in material stiffness.
Several were carried out on the different fire modelling, focusing on the open car park. Yan et al. [
7] proposed a numerical study on the thermal exposure on steel framing members in open car park fire, comparing the steel temperatures computed by the coupling of computational fluid dynamics and finite element modelling, and by analytical models from the Eurocodes. In addition, the influence of galvanization on the steel temperature evolution is assessed. Results show that temperatures in unprotected beams and columns are influenced by the section geometry, car fire scenario, modelling approach, and use of galvanization. Regarding the different models, CFD-FEM (CFD: computational fluid dynamics, FEM: finite-element method) coupled models predict lower temperatures than the Hasemi model, because the latter conservatively assumes that the fire flame continuously touches the ceiling. Nigro et al., [
8], investigated the behaviour of open and closed car parks, under different fire scenarios, applying the aspects of the Fire Safety Engineering (FSE) for the structural safety checks in case of fire, with reference to Italian and European standards. However, very few paper investigated the source fire modelling and its effects on the structural element temperatures. About this topic, Wang et al. [
9] highlighted the influence of using different fuel geometrical shapes on flame extension, temperature distributions and gas species concentrations during different tunnel fire development phases. The results have shown that the use of the geometrical shapes causes significant differences in flame extension lengths during the fully developed fire phase. Another relevant aspect in the case of open car park can be represented by the wind effect, that can influence the ventilation effect; this aspect was investigated by Ghodrat et al. [
10].
Khan et al. [
11] studied the differences between conventional localized fires and the localized burning in large compartments, introducing the concept of semi-confined fire and considering the compartment effects into localized fire models. Hidalgo et al. [
12] considered the transition of fire modes in a full-scale fire test carried out in an open-plan industrial building. Three different fire modes were identified including a traveling fire, a growing fire, and a fully developed fire, by the ratio between the flame front velocity and burnout front velocity. Nadjai et al. [
13] conducted large scale fire tests, investigating the development of a traveling fire in open structures in the frame of the European RFCS-TRAFIR project and concluded that the positioning of the traveling fire band and the ceiling height directly influenced the temperatures reached in the surrounding structures. However, no researcher investigated how the fire source influences the temperature gas evolution and therefore the temperature inside the structural element. In addition, limited research investigated the fire response of car park structures.So, the main objective of this study is to perform several fire analyses, comparing the results in terms of gas temperature and structural elements temperature, with different localised fire models. The main novelty consists in studying the effect of the fire source modelling in CFD analyses, changing its geometrical and combustion properties.
3. Description of Modelling Approach
During a fire in opened compartments, the flash-over condition is rarely reached, so the localized fires should be considered, heating only some structural elements. In the case of a localized fire, the temperatures in the flame, in the smoke and in the surrounding gas are not uniform, unlike in the generalized fire, in which the gas temperature can be considered constant with a good approximation. The thermal action of a localized fire on the structural elements can be evaluated through different models, e.g., the Heskestad model [
3] or Hasemi one [
4] proposed in the Annex C of the EN 1991-1-2 (2002) [
1]. The difference between the two approaches regards the relative height of the flame to the ceiling. In addition, the LOCAFI [
5] simplified localised fire model was proposed by the EN 1991-1-2 (2002) [
1] for calculating the radiative heat flux received by a vertical member not engulfed in the fire area (e.g., a column). This last method was directly applied in this work, as localized fire scenarios related to the main beam were considered (see
Section 2.2). These models were implemented also in the thermo-mechanical dedicated software SAFIR [
21], which is used in this work. In particular, to define the design fire, the equivalent area diameter, the ceiling height and the car heat release rate curve were required. The burning car was modelled by a circular plan area with a 3.25 m diameter. The axis of the localized fire was at the centre of the primary beam, which corresponds to the driving lane. The composite steel-concrete beam temperatures were investigated with the simple models described below, which are valid if the diameter of the fire is limited up to 10 m and HRR of the fire is limited up to 50 MW. Indeed, one of the most relevant advantages of this models is their easy application. Finally, the computational fluid dynamics (CFD) [
22] was used for modelling the localised fires. The CFD is an extremely powerful tool, which allows the detailed study of fluid motion in complex geometries.
3.1. Heskestad Model
The Heskestad method [
3], described in Annex C of the Eurocode EN1991-1-2 (2002) [
1], is applicable when the flame is not impacting the ceiling of a compartment (L
f < H, see
Figure 5) or for an open-air fire.
The method provides the temperature along the vertical centreline in the fire plume and the heat flux. To calculate the flame length [m] of a localized fire is given by:
The temperature in the plume θ
f (°C) along the symmetrical vertical flame axis can be calculated as follows:
where D is the diameter of the fire [m], Q is the heat release rate [W], Qc is the convective part of the heat release rate [W], z is the height along the flame axis [m], H is the distance between the fire source and the ceiling [m]. Instead z
0 is the virtual origin of the axis [m] given by:
3.2. Hasemi Model
When the flame is impacting the ceiling (L
f < H) the Hasemi model [
4] can be used. This method provides the trend of the heat flow impacting the structural elements (see the
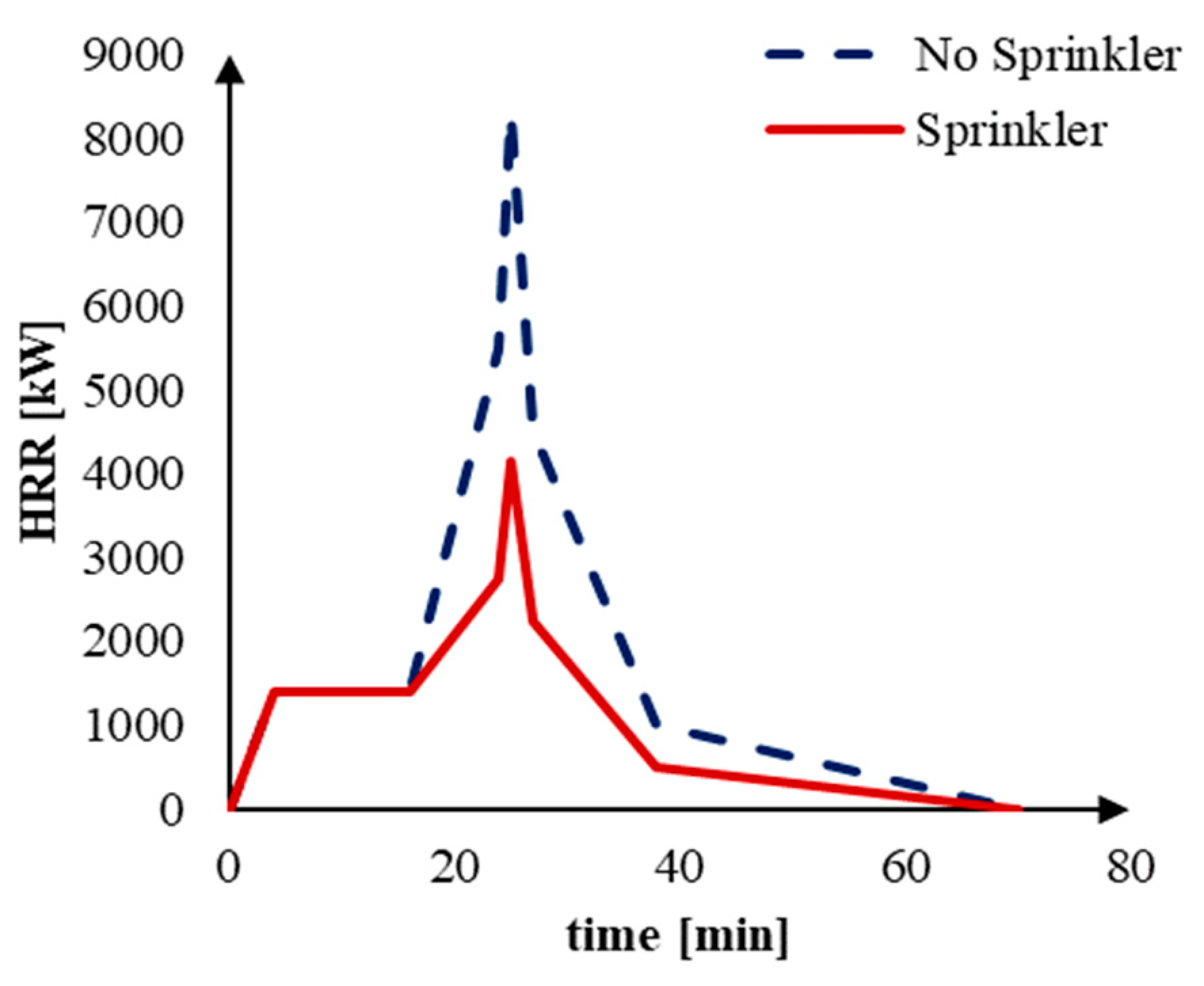
Figure 6), as a function of the fire position, source fire diameter and the heat release rate over time (HRR) of each type of vehicle (see the
Figure 4).
This model is included in EN1991-1-2 (2002) Annex C [
1] which provides the following equations for the calculation of the flux:
in Equation (4),
y is a non-dimensional parameter given by:
where
r is the horizontal distance [m] between the vertical axis of the fire plume and the point along the ceiling where the thermal flux is calculated,
H is the distance between the fire source and the ceiling [m] and
is the vertical position of the virtual heat source [m]. The net heat flux received by the fire exposed unit surface area at the level of the ceiling is given by:
where
is the convection coefficient,
is the surface emissivity of the member,
is the emissivity of the flame and
is the configuration factor,
is the Stefan-Boltzmann constant,
is the member temperature [°C].
In this work, the Hasemi model was applied directly through SAFIR, which implements the Hasemi methodology for localised fires.
3.3. CFD Models
The previous analyses were carried out also with advanced numerical modelling as the computational fluid dynamics (CDF). Computational fluid dynamics (CFD) is an extremely powerful tool, which allows the detailed study of fluid motion in complex geometries. In the CDF models, to predict the development of the fire, the environment is divided into a large number of elementary volumes in which the balance equations are solved, taking into account the variations that occur in each of them due to the changes in the adjacent ones, acting in an iterative way.
Among the computational fluid dynamics models, the Fire Dynamics Simulator (FDS) software [
22], developed by the Fire Research Division at the Building and Fire Research Laboratory (BFRL) of the National Institute of Standards and Technology, is one of the most widespread. FDS is a computational software used to predict and assess the fire evolution. The software numerically solves the Navier-Stokes equations for low-speed flows generated by thermal gradients, considering the smoke/heat transport phenomena typical of fires. To transfer the thermal information from FDS models to finite element analysis, two approaches are generally adopted, the adiabatic surface temperature (AST) and a dedicated FDS-FEM interface. FDS includes a calculation of the adiabatic surface temperature (AST), a quantity that is representative of the heat flux to a solid surface. Following the idea proposed by Ulf Wickström [
23], TAST is the surface temperature for which the net heat flux is zero, so it considers that the surface is a perfect insulator. The usefulness of the AST is that it represents an effective exposure temperature that can be passed on to a more detailed model of the solid object. It provides the gas phase thermal boundary condition in a single quantity, and it is not affected by the uncertainty associated with the solid phase heat conduction model within FDS. The second approach is the FDS-FEM interface. In this case a file containing the gas temperatures and radiant intensities at various positions and from different direction in the compartment is created.
FDS software was used also in this work to study the evolution of gas temperature in an open car park. In particular, in order to evaluate the gas temperatures, the FDS-AST approach was used. Two models were developed: (i) the fire without the sprinkler system and (ii) the fire with sprinkler system activation. In addition, the modelling of the fire source was developed according to two different approaches. All the details are described below.
3.3.1. Fuel Source
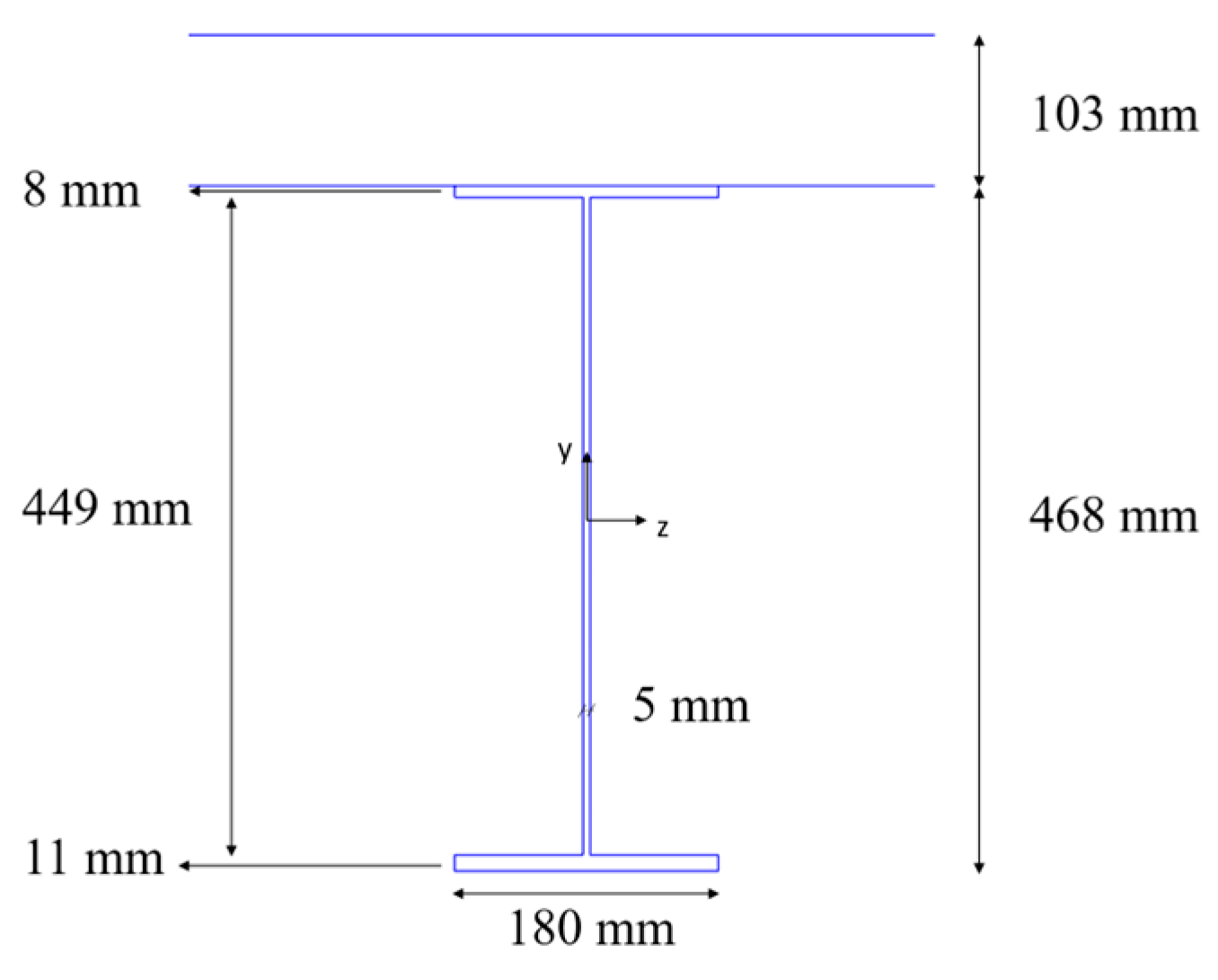
The fuel source was modelled with two approaches: the 3D car flame simulation (FDS MODEL A) and the flat car flame one (FDS MODEL B). In both cases, the compartment was modelled in FDS using computational domain of 8.75 m × 8.75 m × 2.75 m. The mesh size was 0.125 m in X, Y and Z direction. The steel beam was modelled with its space as 50 cm × 25 cm, with the bottom flange at +2.125 m from the slab domain and +2.625 m from the domain floor. To model the fire action, the pre-set reaction in FDS corresponding to the polyurethane combustion was used. Thermal properties of the concrete-steel beam were considered variable with temperature, according to the EN 1994 1-2 (2005) [
16].
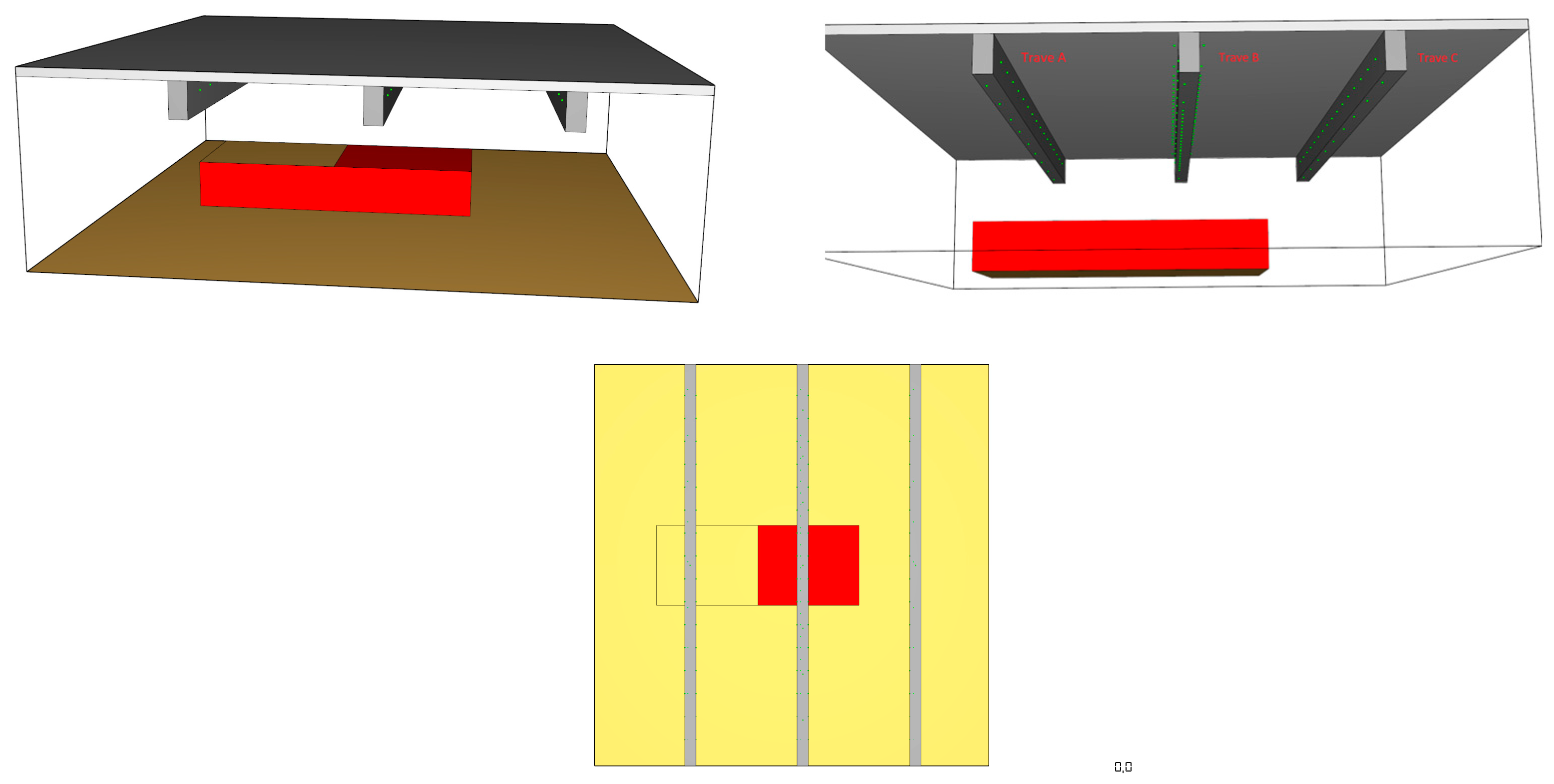
3.3.2. 3D Flame Car FDS Simulation
In the 3D flame car simulation (see the
Figure 7), the volume of the car was considered equal to 4.5 × 1.75 × 0.75 m
3. The HRR/m
2 was considered equal to 778 W/m
2. The main burning car surfaces were 2 × 4.5 × 0.75 m
3, while on the top, a half surface was modelled with 0.5 × 4.5 × 1.75 m
3 dimensions.
3.3.3. Flat Flame Car FDS Simulation
In the second approach, which considers the flat flame car simulation (shown in the
Figure 8), the volume of the car was considered equal to 4.75 × 1.75 × 0.25 m
3.
In this case, the HRR/m2 was equal to 1000 W/m2 and the burning car surface was the top surface 4.75 × 1.75 m2.
4. Results and Discussion
To compare both the localised fire models and the FDS fire source modelling effects, a series of thermomechanical analyses were conducted. In addition, the sprinkler effect was considered (see
Table 1). All the analyses were performed for 60 min, consistently with the duration of the car heat release rate curve, which represents the input of the fire analyses, as described before.
In this section all the results were discussed, both in terms of input fire curves for FDS models and of steel profile temperature for both Hasemi/SAFIR and FDS models. Moreover, for each part of the cross section, the steel temperatures were compared considering the different models. In all the cases the thermal analyses of the steel profile were analysed using the SAFIR software [
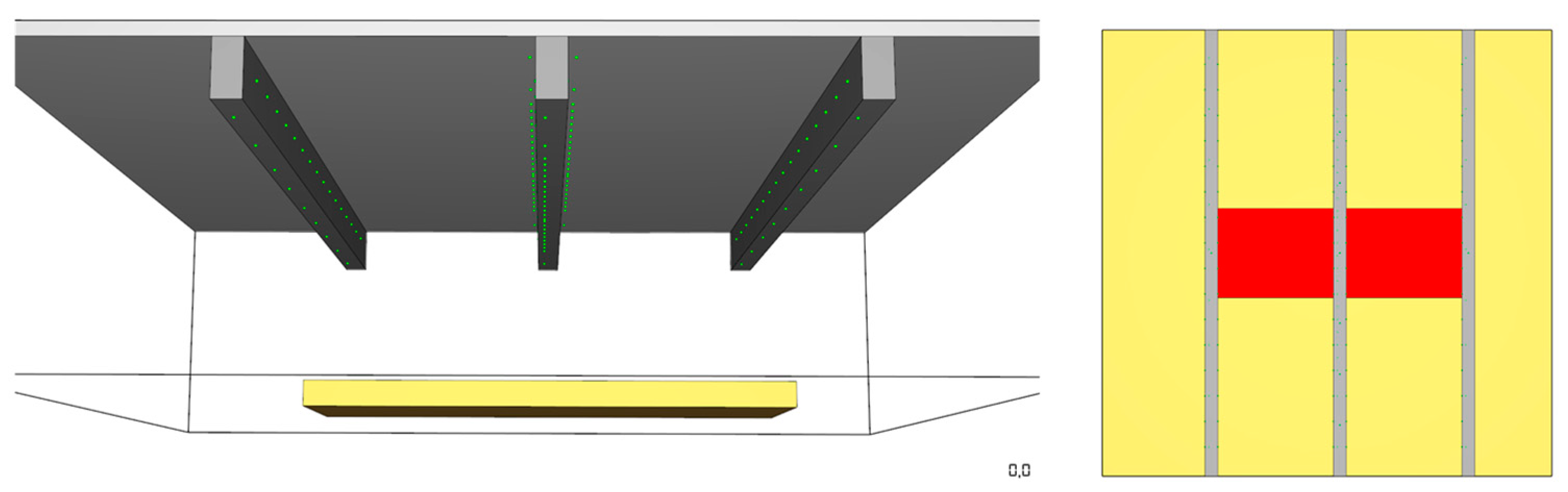
21]. The FDS-AST temperatures, recorded by thermocouples, were applied to the exposed surfaces of the steel cross section (top flange, web and bottom flange), as shown in the
Figure 9.
The rate of increase in temperature of a steel cross-section is determined by the ratio of the heated surface area (A
m) to the volume (V). This ratio, (A
m/V), has units of m
−1 and is known as section factor. The section factor indicates the heating rate of a steel element during a fire; steel members with low section factors will heat up more slowly. In the
Figure 9, the section factors A
m/V for all the profile parts (top flange web and bottom flange) were shown, emerging that their values are very different. Therefore, the temperature evolution inside these three parts was investigated.
4.1. Comparison between Adiabatic Surface Temperature, Flame Evolution and Gas Temperature in FDS Models
The temperature in the steel profile, which directly influences the fire behaviour of a structure, is clearly linked to the input fire curve. The input temperature in this case were analysed both in terms of ambient temperature and in terms of adiabatic surface temperature (AST), obtained by the FDS simulation described before.
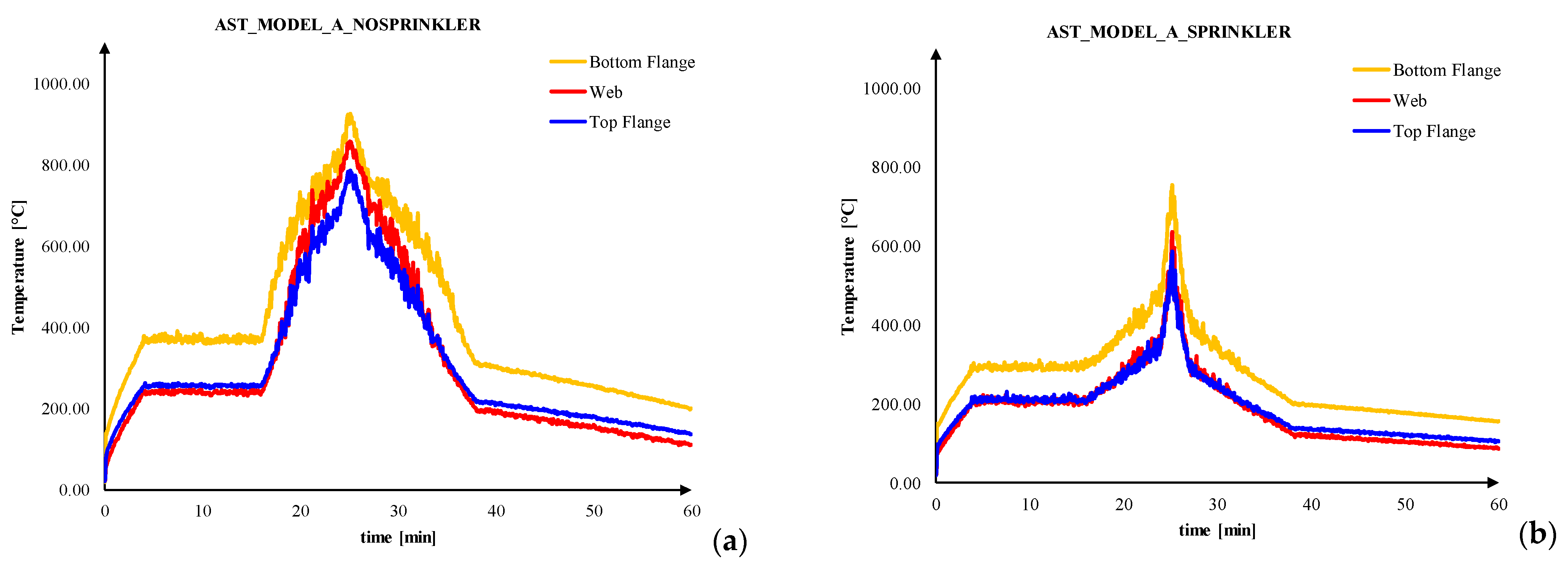
Therefore, a useful comparison is the one between the AST in the case of model_A_nosprinkler, model_A_sprinkler (see
Figure 10), model_B_nosprinkler and model_B_sprinkler (see
Figure 11), at different compartment locations. In particular, the AST, placed close to the analysed beam, were considered (see). Observing the results some considerations can be stated:
- -
in all the cases the maximum AST was recorded beside the lower flange, especially in presence of sprinkler, this is due to the greater proximity to the fire source;
- -
the presence of sprinkler reduced the temperatures;
- -
the temperatures recorded beside web and top flange are very similar to each other;
- -
the model with 3D fire source returned higher temperatures than flat flame modelling, in the case of absence of sprinkler.
Other general comparisons were made in terms of flame evolution (see the
Figure 12) and gas temperature evolutions (see the
Figure 13). For brevity reasons, the latter results were reported only for the cases without sprinkler system, as it returns higher temperatures.
The
Figure 12 shows the flame evolution for both model_A and model_B at several time steps; the influence of the fire source modelling is very clear, indeed, fixing t = 1500 s time at which the HRR peak is reached, the flame appeared different with a ceiling impact, in the case of model_A, due to the modelling of the real size of the car. The differences in fire source modelling appeared also observing the gas temperature evolution (see the
Figure 13), indeed the model_A returns more realistic temperature, not only in terms of values, but also in the case of temperature distribution around the burned car.
4.2. Comparison of Temperature in Steel Profile
The temperature evolution inside the cross section of structural element is one of the most relevant aspects that influences the fire behaviour of the whole structure. Therefore, the thermal analysis of the element is one of the crucial steps. In the following, the thermal analyses of the composite steel-concrete beam described in the
Section 2 was conducted, under the fire inputs described before. The
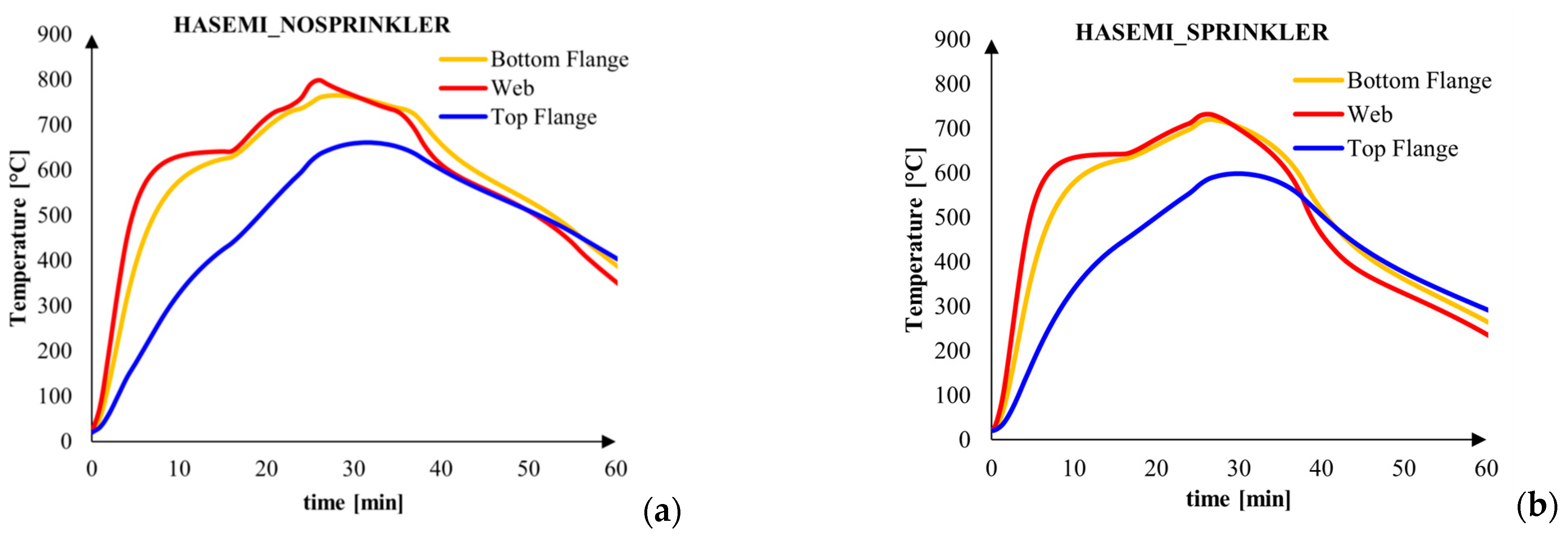
Figure 14 shows the steel temperature in the bottom flange, web and top flange, under the Hasemi model. In particular, it can be observed that the sprinkler system reduced the temperature and the peak from about 800 °C, to about 750 °C. As shown in both
Figure 14a and
Figure 14b, the web heated up more than the bottom flange, since its A
m/V is greater than the bottom flange one.
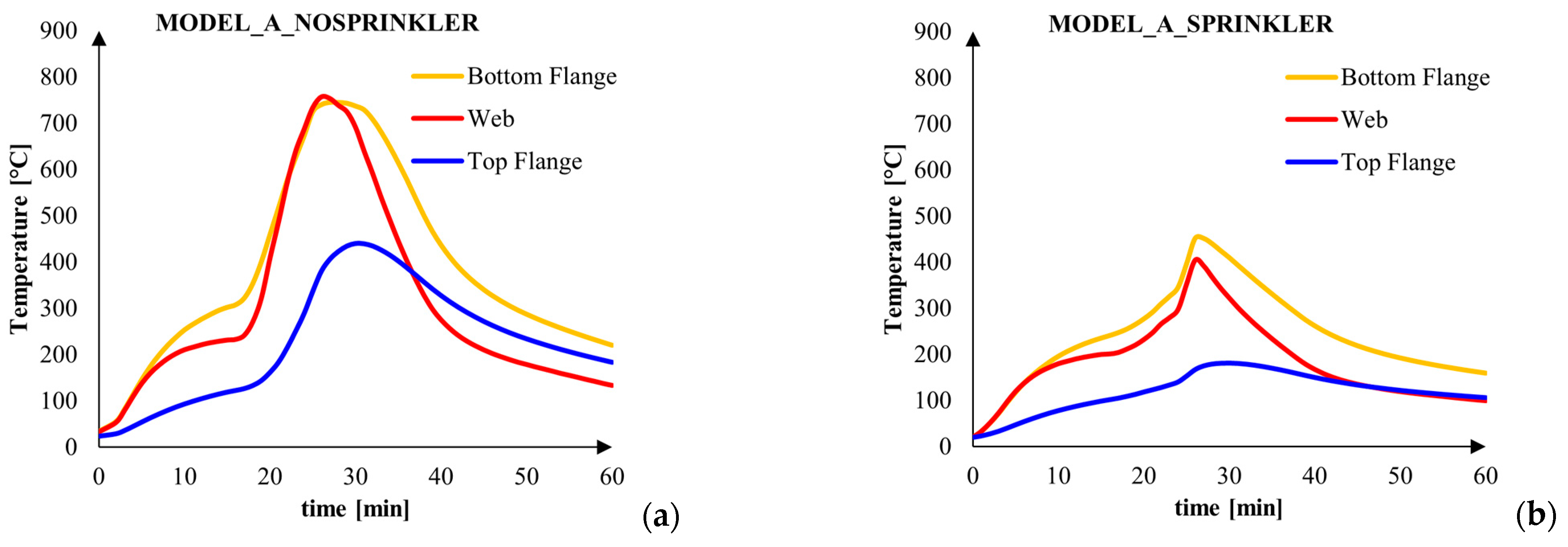
Moving on the
Figure 15, which represents the steel temperature under the Model_A, the same consideration of the previous case can be done; however, in the case of sprinkler activation (the
Figure 15b), the section factor effect became less evident and the bottom flange was more heated because the input AST is greater.
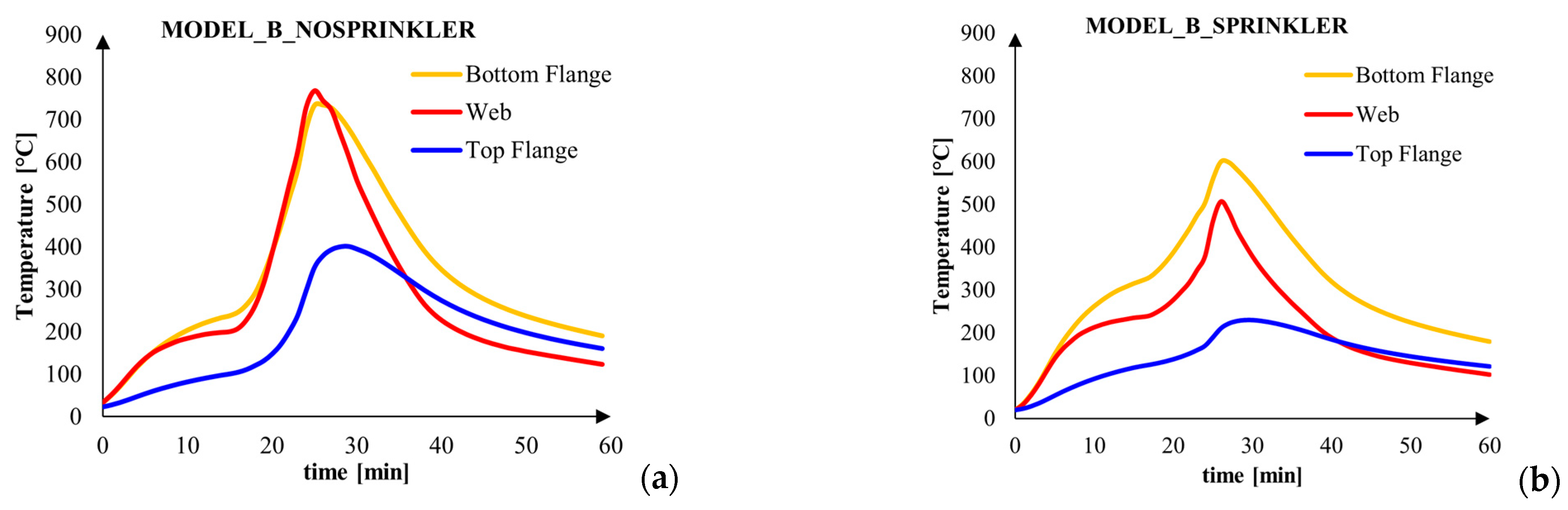
All the same considerations about the Model_A can be conducted also on the Model_B, observing the
Figure 16.
In all the cases, comparing the web and the top flange temperature, the effect of the slower cooling of the top flange emerges; indeed, this section part is the slowest to heat up since its smallest Am/V and the concrete slab subtracts heat by conduction from the steel flange. In addition, in the cooling phase, the top flange becomes cold more slowly because the concrete, with its high thermal inertia, delays the steel cooling.
4.3. Comparison between Fire Models
The steel temperature evolutions were also compared for each element of the cross section (bottom flange, web ad bottom flange) as function of the localized fire model. As seen in the
Figure 17, for all three analysed elements, the comparison leads to the same results, i.e., more accurate is the model, lower are the temperature and the heating rate of the steel profile.
Very similar results were also achieved by other previous researchers. In particular, Yan et al. obtained the same result analysing an open car park [
7] and its steel beam [
24] under a localised fire. Indeed, comparing the results of the simple localised fire models and the FDS analysis, the Hasemi model results overconservative in terms of temperatures evolution in the structural elements at the ceiling level during the fire, because in this model, the flames touching ceiling during the whole fire duration is assumed.
In the case of absence of sprinkler, the Hasemi temperature overestimation was more evident in the heating and cooling phase, but the temperature peak is very similar in all the three models for bottom flange and web. This result was not confirmed for the top flange, where the different heating condition leaded to different temperature between Hasemi and FDS. In all the cases, the model_A returned temperature slightly higher than the model_B. For the analyses with sprinkler (see the
Figure 18), the HASEMI model overestimates the temperature during all the thermal transient, while Model_A and Model_B returned very similar temperature, even if in this case the higher one is related to Model_B. This result is related to the different AST registered by FDS.
4.4. Sprinkler Effect on Steel Temperature
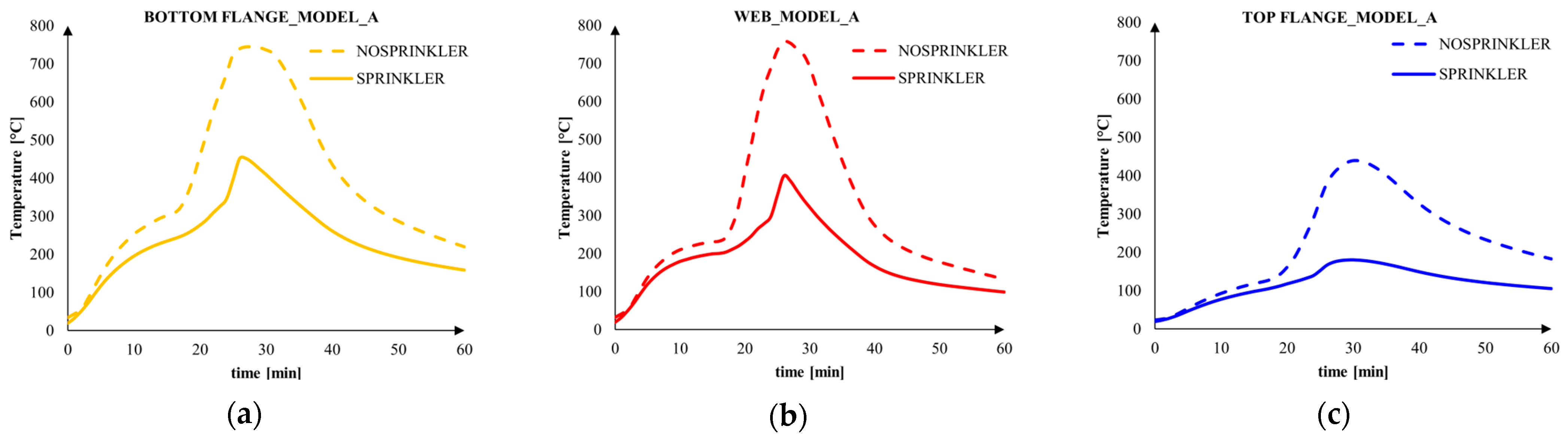
Focusing on Model_A, i.e., the 3D flame models in FDS., the comparison between steel temperature with and without sprinkler system was conducted (see
Figure 19). This comparison was conducted also by Poon, obtaining similar results. In particular, the performance of sprinkler protection offers the ability to control the fire before it becomes fully developed, a measure which passive protection is not able to provide [
25].
Reducing the potential development of a fire offers also other advantages such as reducing potential damage and allowing a much easier access for fire rescue and intervention teams. In all the cases the steel temperature benefitted from the sprinkler.
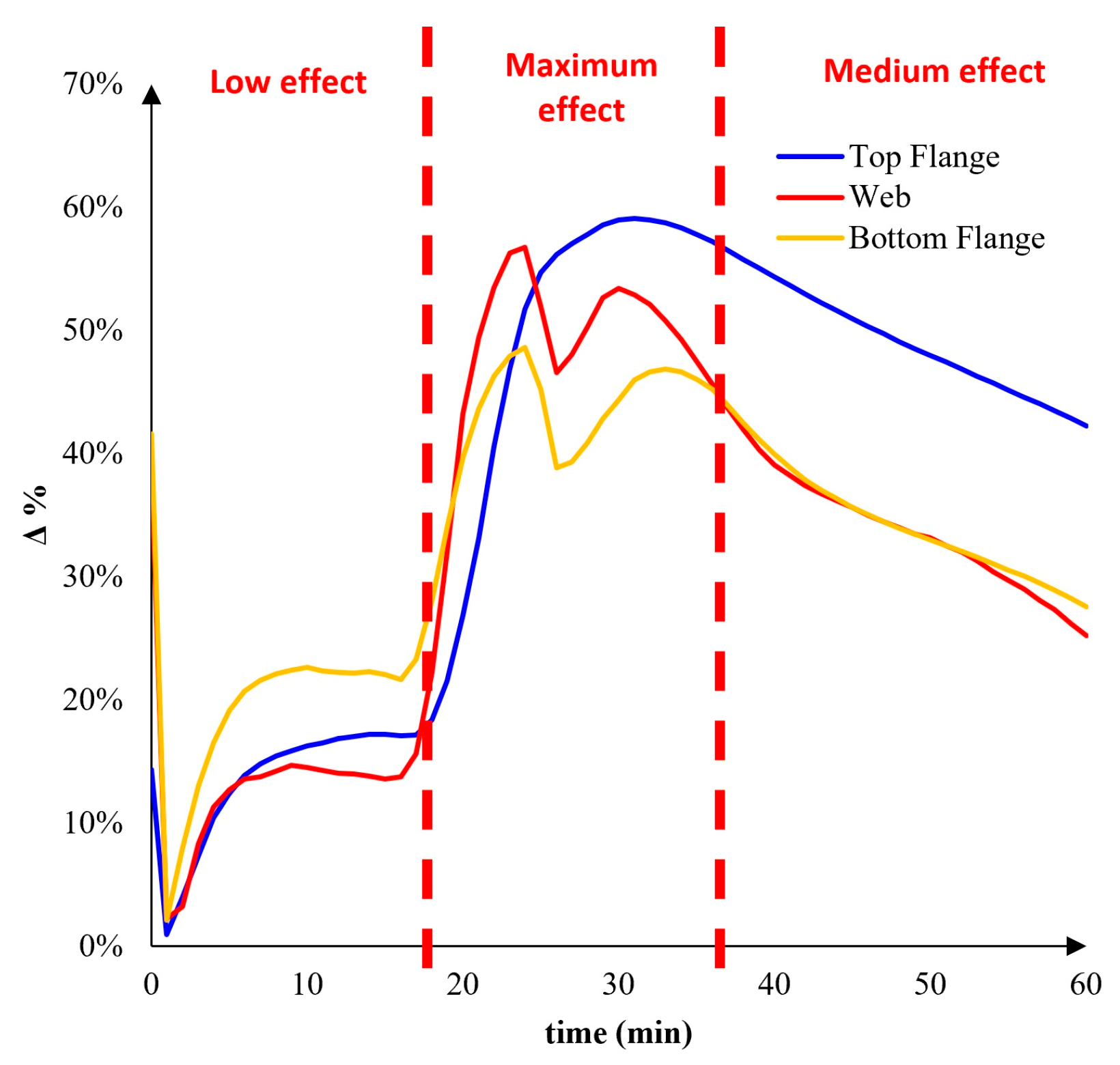
To have a better estimation of the sprinkler effect during the thermal transient, the value of Δ% was considered:
where
θSPRINKLER is the temperature with sprinkler effect, while
θNO_SPRINKLER is the steel temperature without sprinkler effect (see
Figure 20).
- -
between 0 and about 20 min the effect of the sprinkler is low, since Δ% is less than 30%;
- -
the maximum effect is reached between about 20 and 40 min, reaching also Δ% about 60% for the top flange;
- -
between 40 and 60 min the sprinkler effect decreased especially for web and bottom flange.
5. Conclusions
The main objective of the paper is to study the effect of the different localised fire models on the flame evolution, gas temperature and steel temperature, with reference to a localized fire due to a car burning in an open car park.
Assessing the simulation results in terms of fire effects, the following conclusions can be pointed out:
in all the cases the maximum adiabatic surface temperature was recorded beside the lower flange; this is due to the greater proximity to the fire source;
the presence of sprinkler reduced the adiabatic surface temperatures;
the adiabatic surface temperature recorded beside web and top flange are very similar, demonstrating a comparable thermal input flux;
the model with 3D fire source returned higher temperatures than flat flame modelling in the case of absence of sprinkler.
All these effects were considered also with reference to the steel temperatures of a typological steel-concrete beam, and the main evidences can be summarized as following:
generally, the web is more heated than the bottom flange, since its section factor Am/V is greater;
the top flange is the coolest one, since it has the smallest Am/V and the concrete slab subtracts heat by conduction;
comparing the web and the top flange temperature, the effect of the slower cooling of the top flange emerges. Indeed, the top flange becomes cold more slowly because the concrete, with its high thermal inertia, delays the cooling;
more accurate is the localised fire model, lower are the temperature and the heating of the steel profile;
the maximum effect of the sprinkler is reached between about 20 and 40 min.
The main results of this paper show the importance of using the most accurate model to simulate the natural fire, if a performance-based approach is used. Indeed, one relevant aspect is to obtain a correct estimation of both the fire parameters (e.g., flame and temperature evolution) and the temperature in the structural elements. Both aspects can affect the fire safety objectives: protection of life, protection of property/structure and continuity of operation.
In future, the modelling of more fire scenarios, e.g., involving more cars, will be carried out and the wind effect, that could be relevant in the case of ventilated open car park will be also considered in the CFD analyses.