Risk Assessment of Explosion Accidents in Hydrogen Fuel-Cell Rooms Using Experimental Investigations and Computational Fluid Dynamics Simulations
Abstract
:1. Introduction
2. Materials and Methods
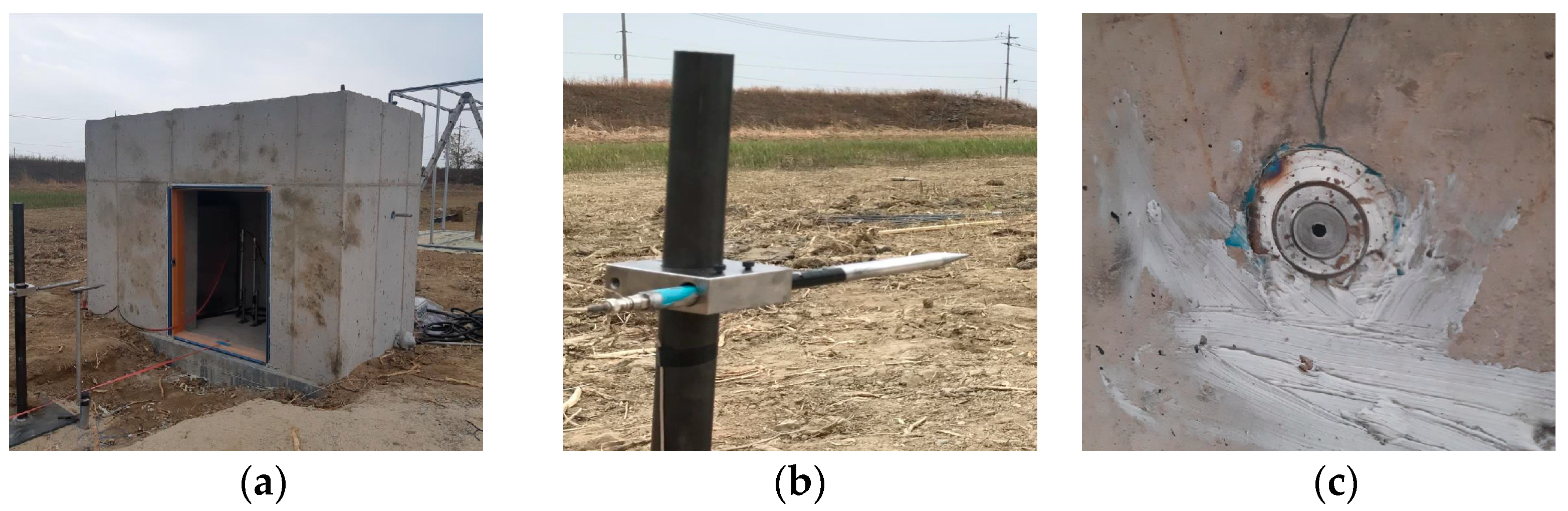
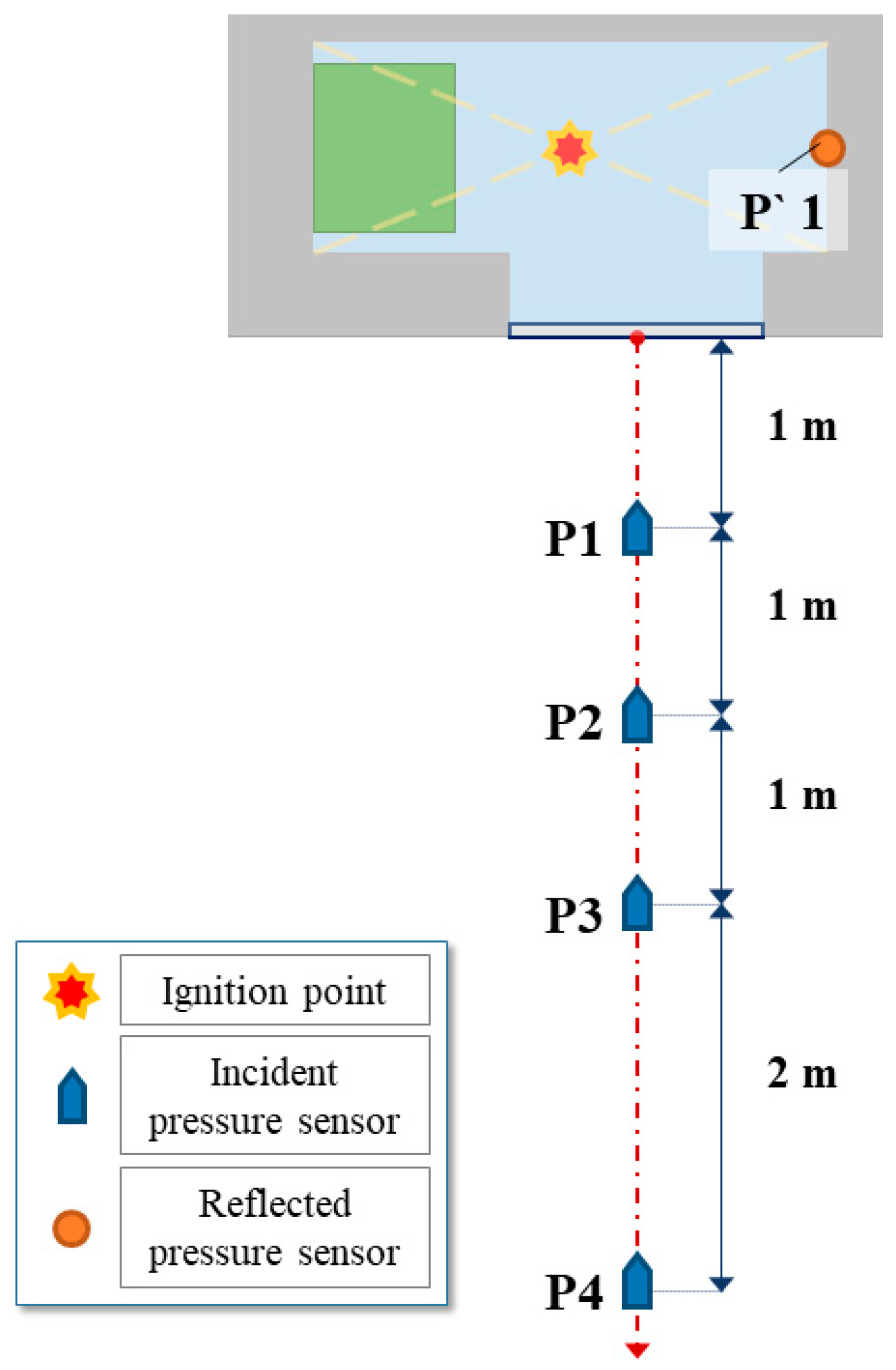
2.1. Experimental Setup and Materials
2.2. Characterization of Materials
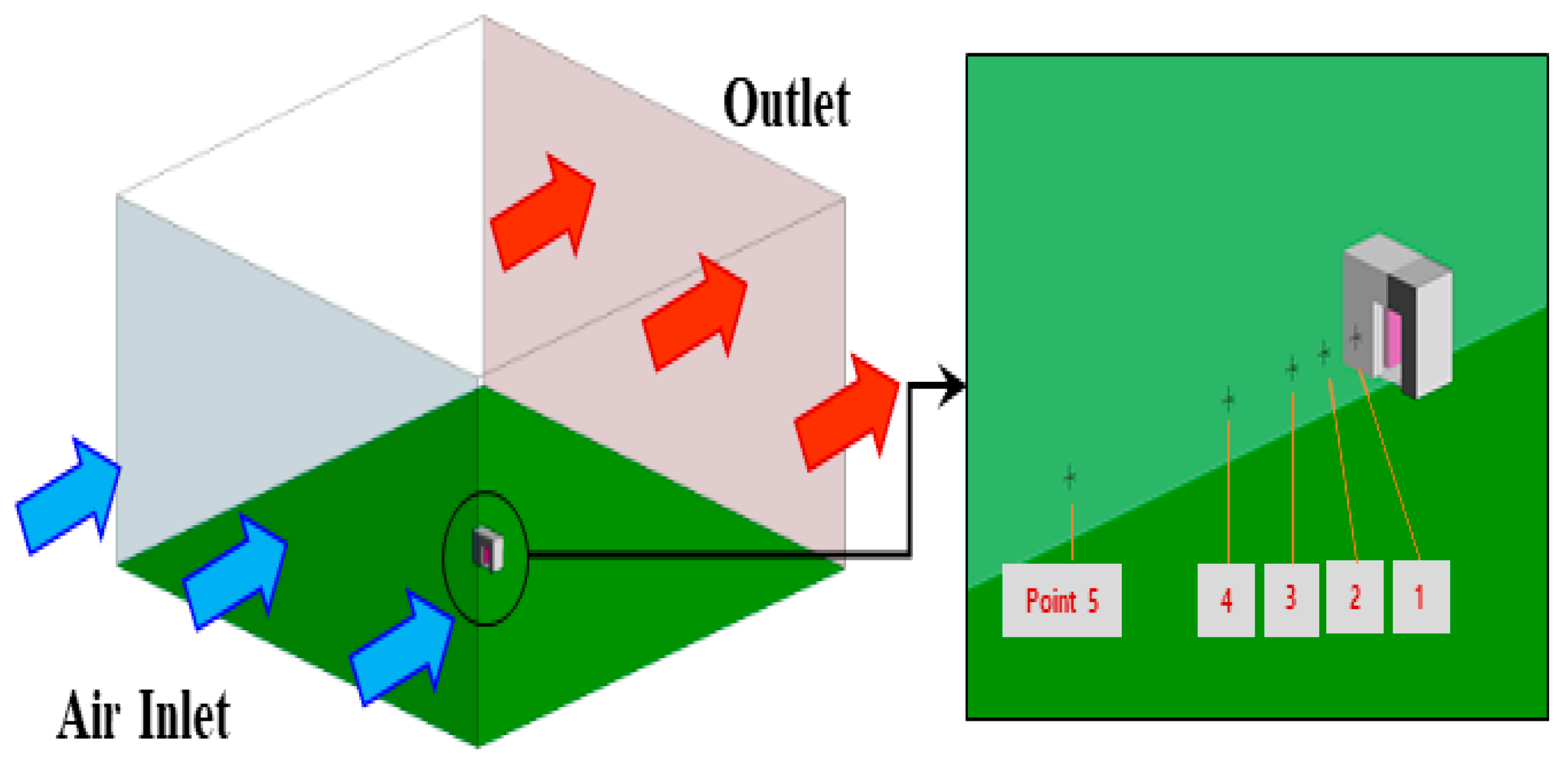
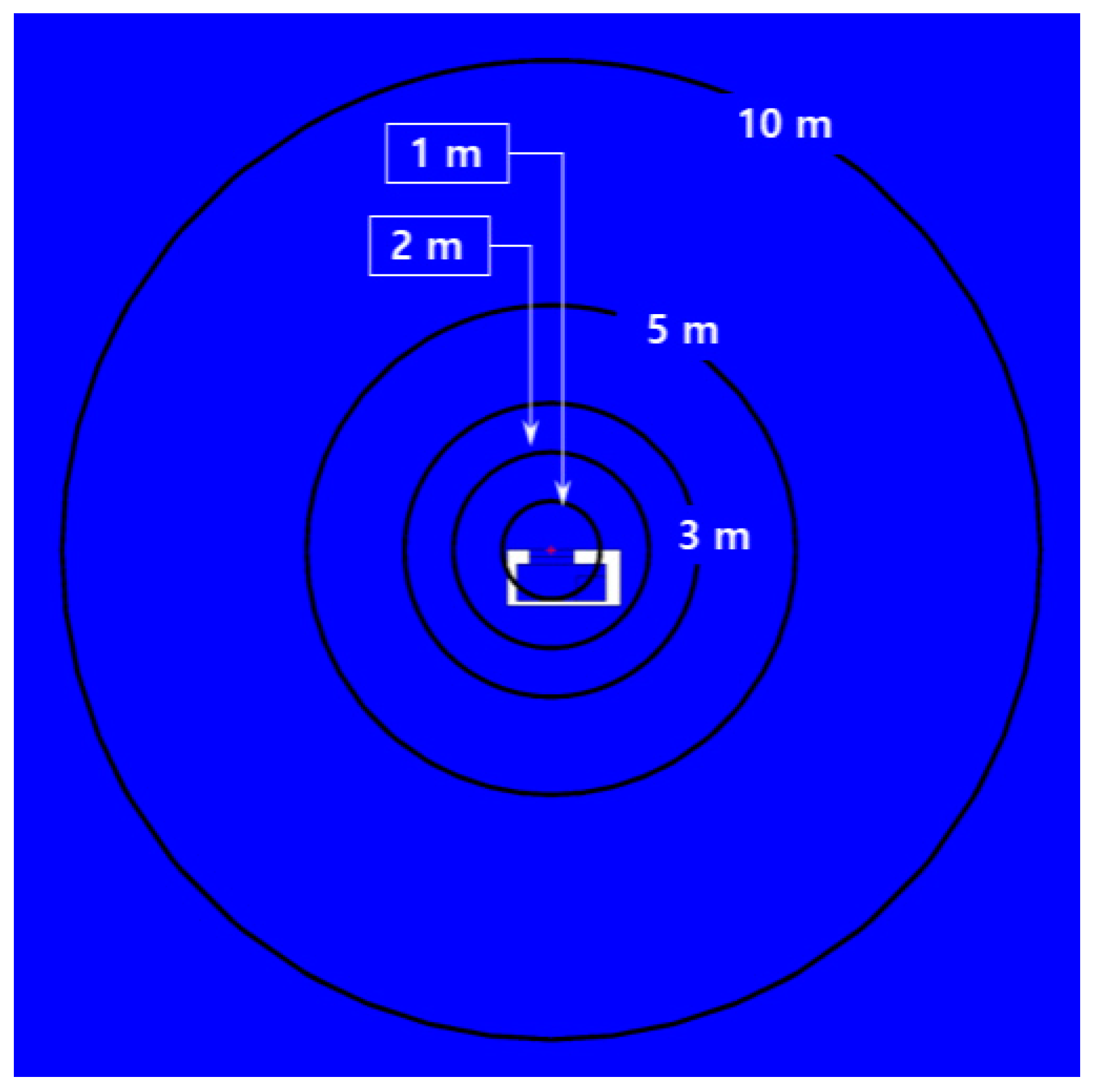
2.3. CFD Numerical Setup
3. Results and Discussion
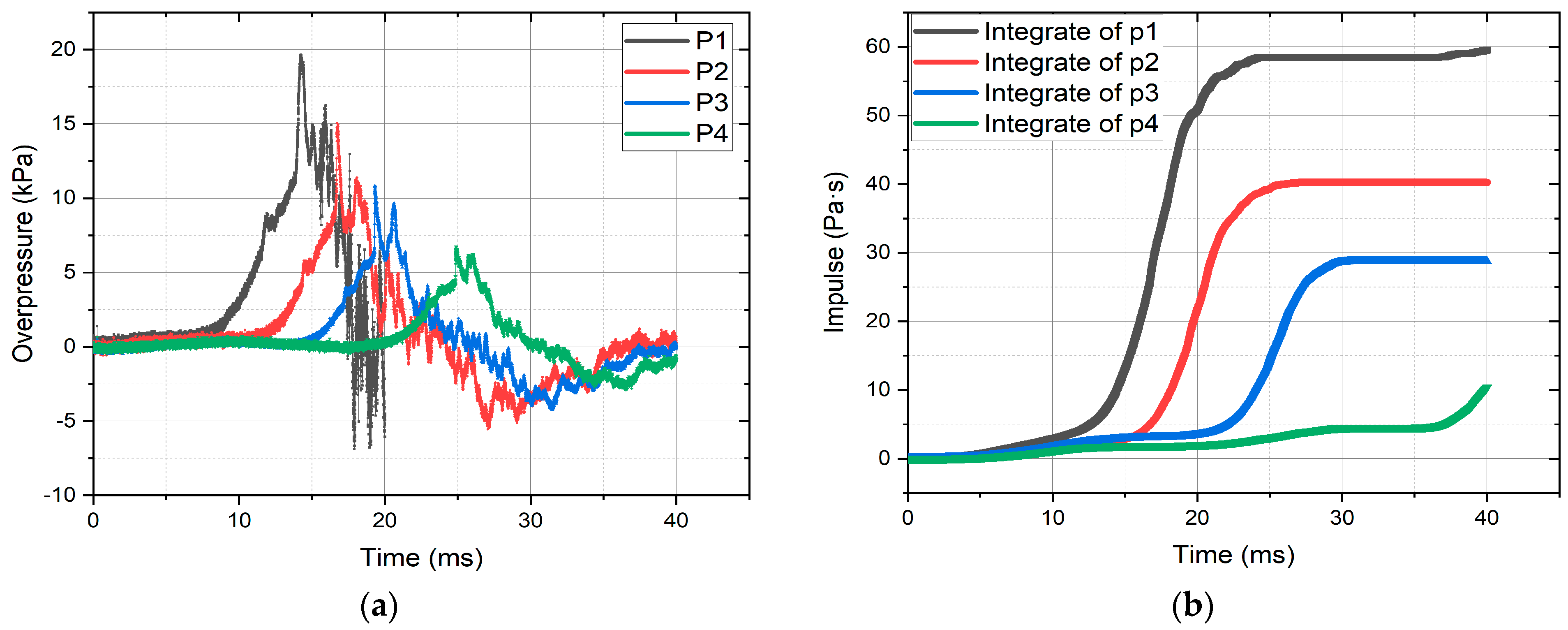
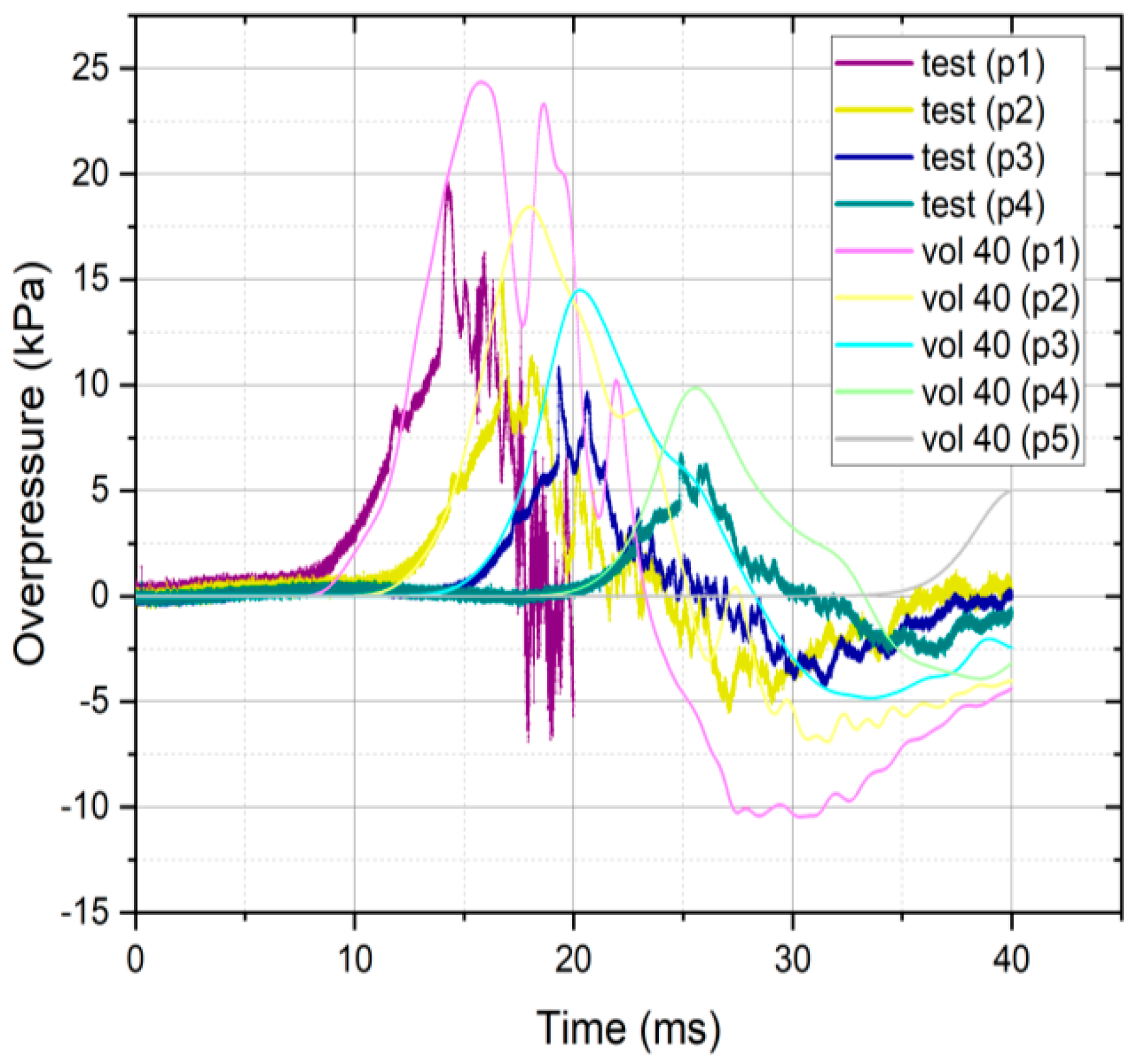
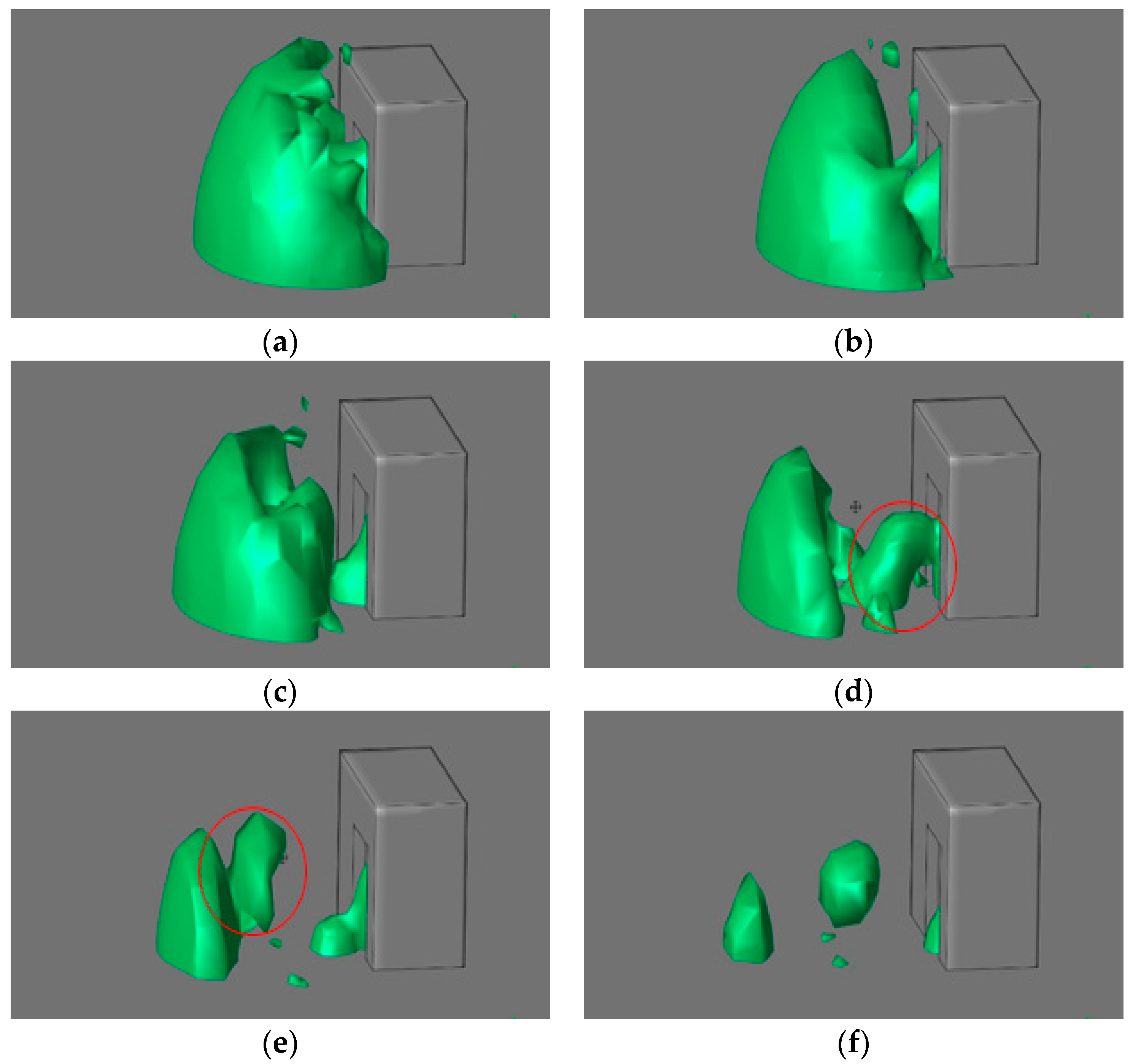
3.1. Validation Results
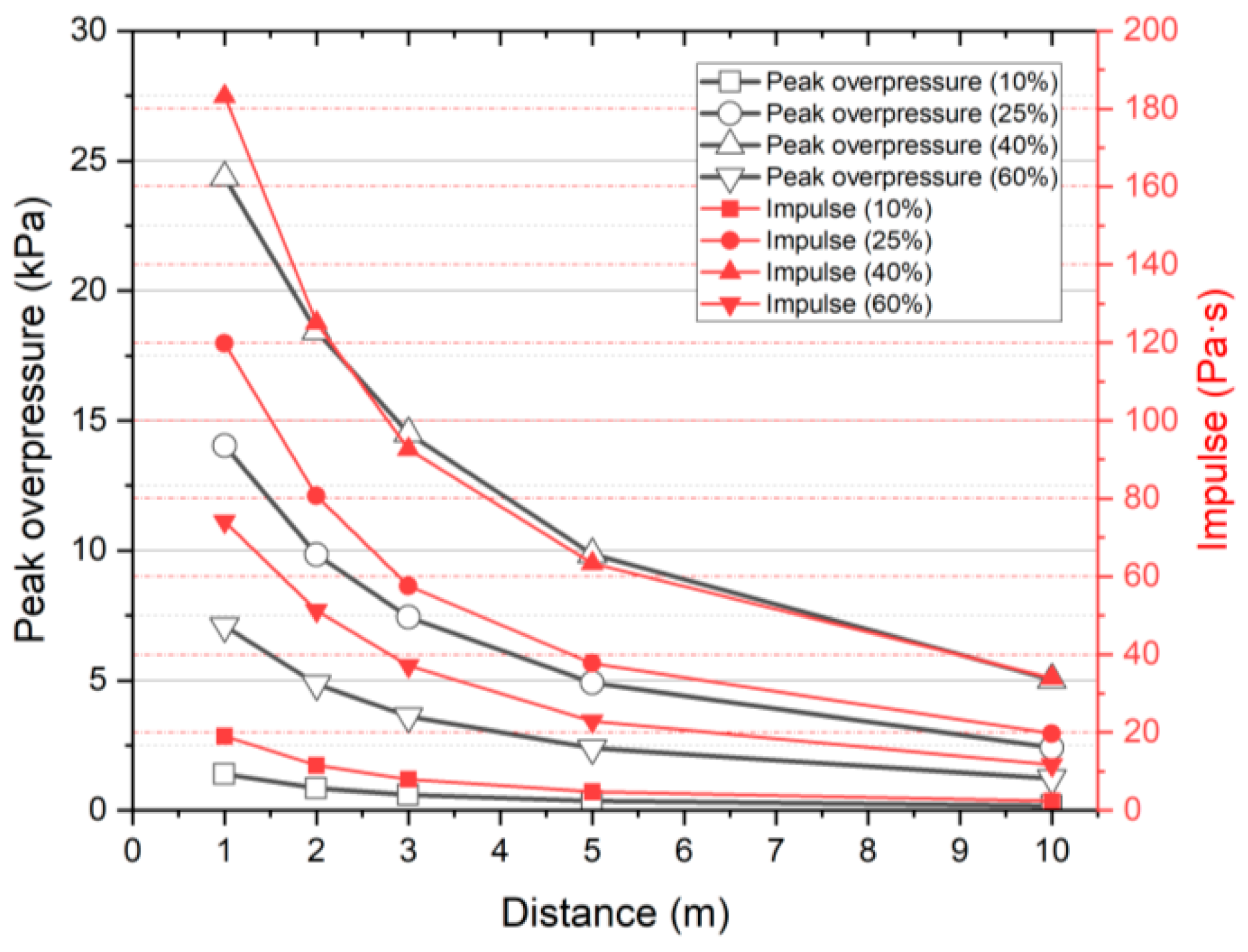
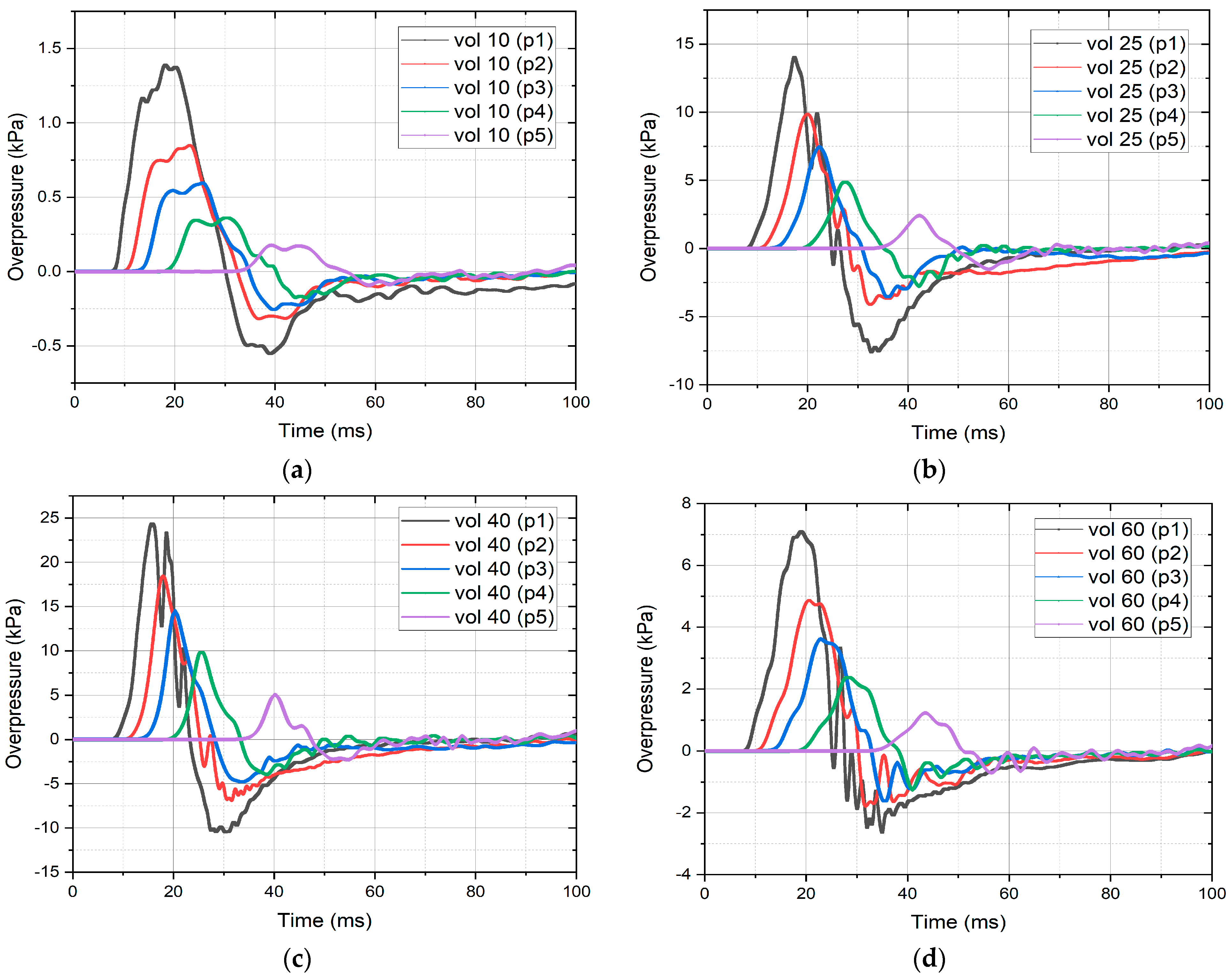
3.2. Validation CFD Modeling Results
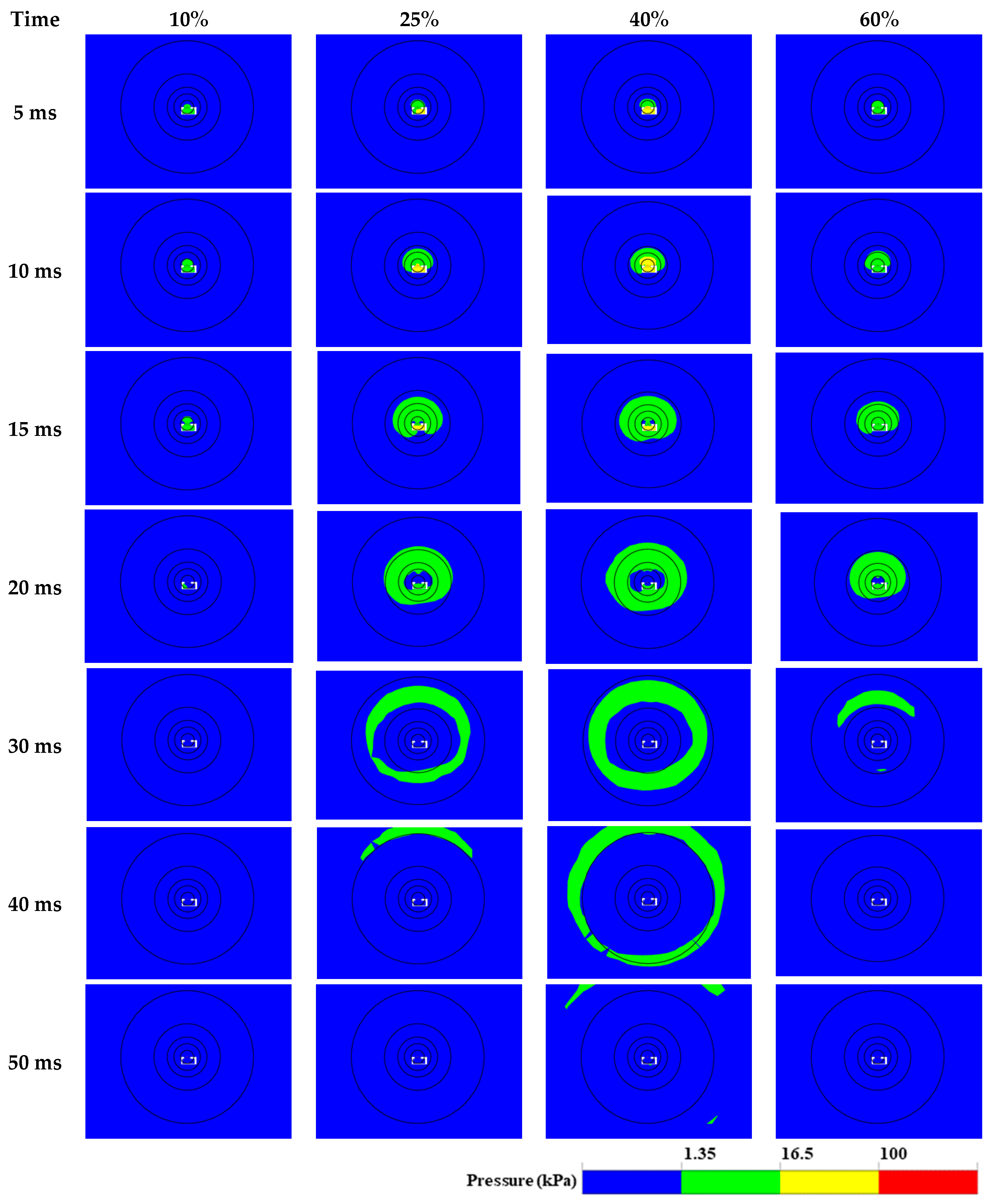
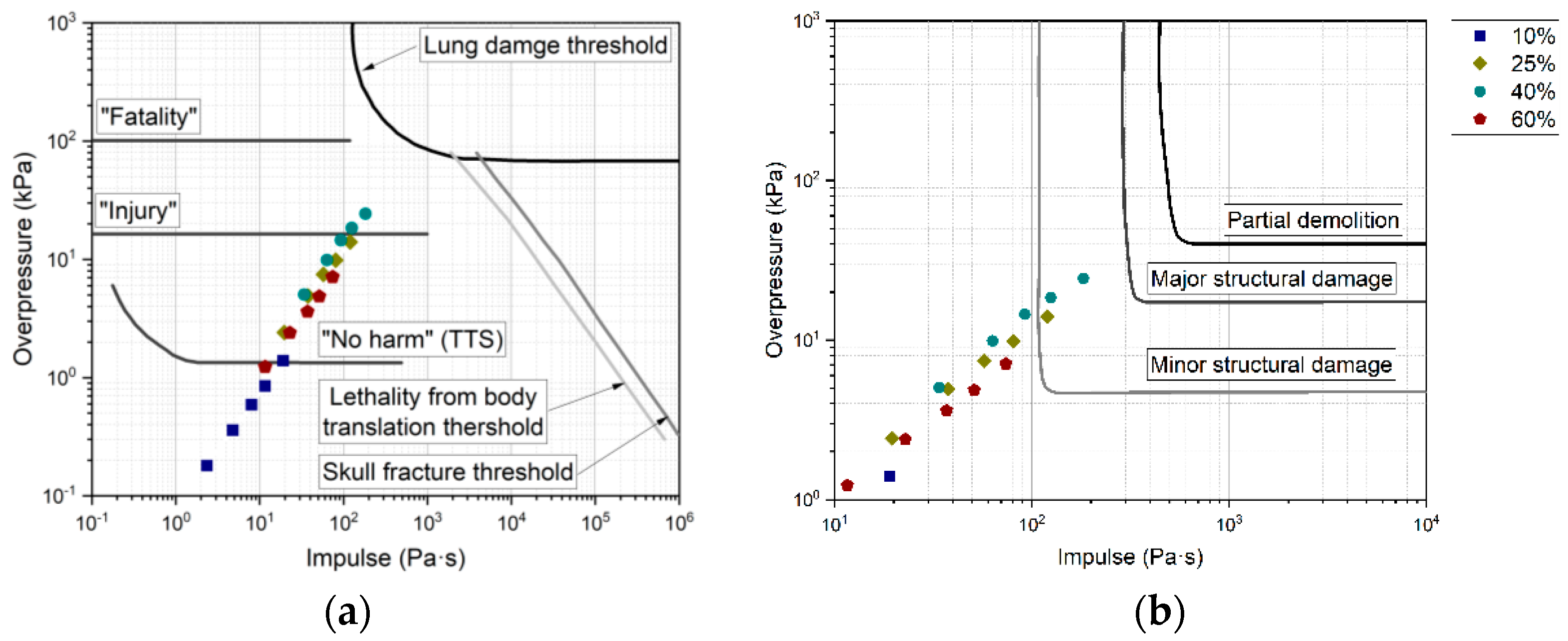
3.3. Risk Level Depending on Overpressure
| Incident Pressure (kPa) | Harm Level |
|---|---|
| 13.8 | Eardrum rupture threshold [46] |
| 16.5 | 1% eardrum rupture probability [42] |
| 23 | 1% eardrum rupture [47] |
| 25~35 | 1% fatality probability [48] |
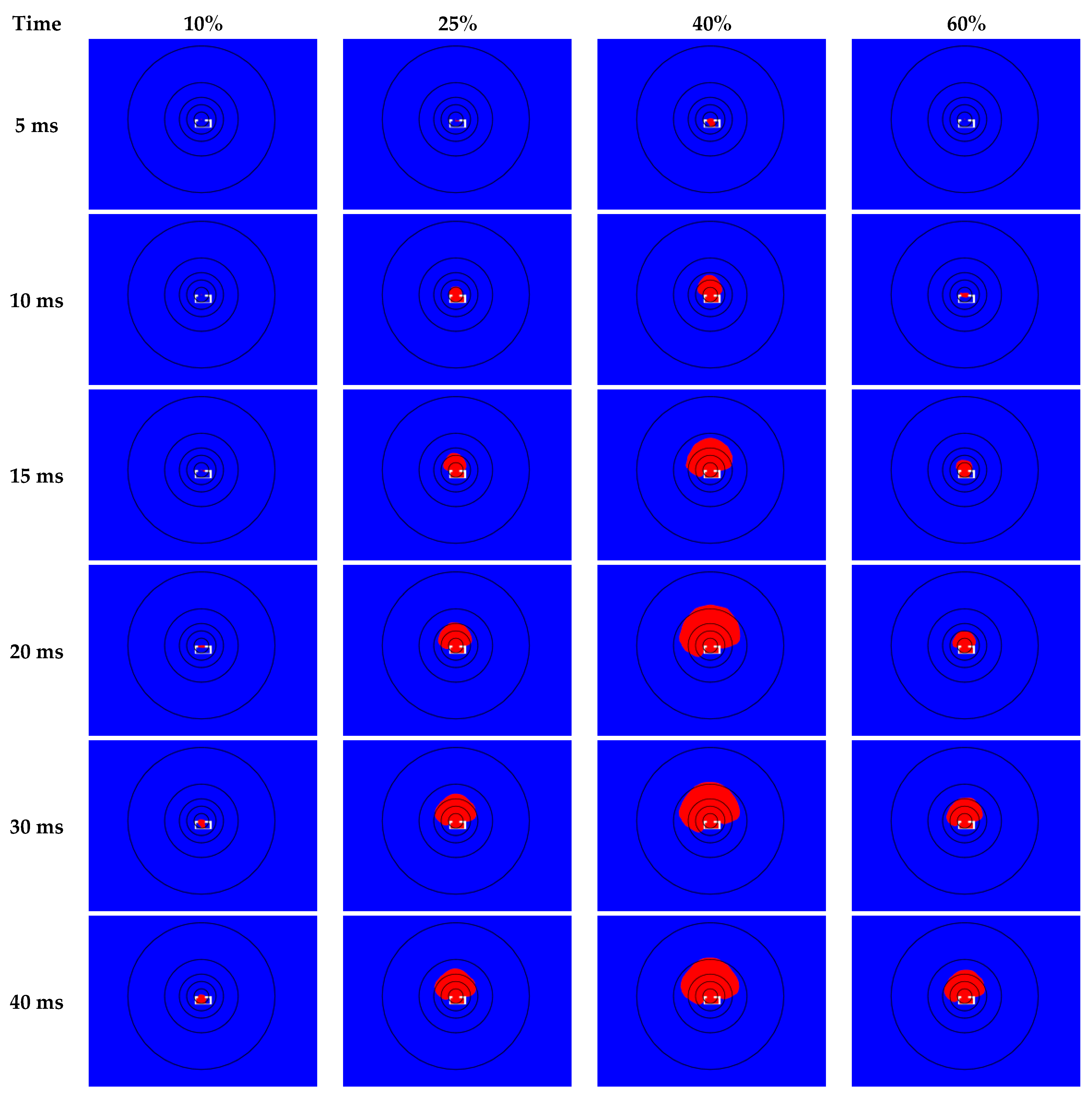
3.4. Risk Level Depending on Heat Flux
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schleussner, C.F.; Rogelj, J.; Schaeffer, M.; Lissner, T.; Licker, R.; Fischer, E.M.; Kuetti, R.; Levermann, A.; Frieler, K.; Hare, W. Science and Policy Characteristics of the Paris Agreement Temperature Goal. Nat. Clim. Chang. 2016, 6, 827–835. [Google Scholar] [CrossRef]
- Jensen, M.; Swedish Public Housing Project Goes Off-Grid with Solar, Hydrogen, and Fuel Cells. LinkedIn. 2019. Available online: https://www.linkedin.com/pulse/swedish-public-housing-project-goes-off-grid-solar-h2-michael-jensen (accessed on 2 December 2019).
- Northern Gas Networks. H21 Leeds City Gate Project. 2016. Available online: https://h21.green/projects/h21-leeds-city-gate/ (accessed on 1 March 2016).
- IRD Fuel Cells. Vestenskov—The First Hydrogen Village in the World. 2011. Available online: https://stateofgreen.com/en/solutions/hydrogen-community-lolland/ (accessed on 1 March 2016).
- Japan for Sustainability. World’s First Community Level Hydrogen Town Project Starts in Kitakyushu. 2011. Available online: https://www.japanfs.org/en/news/archives/news_id030826.html (accessed on 17 October 2011).
- Hydrogen House. Welcome to the Hydrogen Future. 2022. Available online: https://www.hydrogenhouseproject.org/index.html (accessed on 1 January 2021).
- Abedini, M.; Mutalib, A.A.; Raman, S.N.; Alipour, R.; Akhlagh, E. Pressure–Impulse (P–I) Diagrams for Reinforced Concrete (RC) Structures. Arch. Comput. Methods Eng. 2019, 26, 733–767. [Google Scholar] [CrossRef]
- Wesevich, J.W.; Oswal, C.J. Empirical Based Concrete Masonry Pressure-Impulse Diagrams for Varying Degrees of Damage; Baker Engineering and Risk Consultants; ASCE Structures Congress: Reston, VA, USA, 2005. [Google Scholar]
- Syed, Z.I.; Mendis, P.; Lam, N.T.K.; Ngo, T. Concrete Damage Assessment for Blast Load Using Pressure-Impulse Diagrams. In Earthquake Engineering in Australia; ACT: Canberra, Australia, 2006. [Google Scholar]
- Lobato, J. A Comparison of Hydrogen Cloud Explosion Models and the Study of the Vulnerability of the Damage Caused by an Explosion of H2. Int. J. Hydrogen Energy 2006, 31, 1780–1790. [Google Scholar] [CrossRef]
- Goswami, R.; Biao, S. Study on Vapour Dispersion and Explosion from Compressed Hydrogen Spill: Risk Assessment on a Hydrogen Plant. Int. J. Hydrogen Energy 2022, 47, 41195–41207. [Google Scholar] [CrossRef]
- Groethe, M.; Merilo, E.; Colton, J.; Chiba, S.; Sato, Y.; Iwabuchi, H. Large-Scale Hydrogen Deflagrations and Detonations. Int. J. Hydrogen Energy 2007, 32, 2025–2133. [Google Scholar] [CrossRef]
- Sato, Y.; Iwabuchi, H.; Groethe, M.; Merilo, E.; Chiba, S. Experiments on Hydrogen Deflagration. J. Power Sources 2006, 159, 144–148. [Google Scholar] [CrossRef]
- Royle, M.; Shirvil, L.C.; Roberts, T.A. Vapour Cloud Explosions from the Ignition of Methane/Hydrogen/Air Mixtures in a Congested Region. In Proceedings of the 2nd ICHS Conference, San Sebastian, Spain, 11–13 September 2007. [Google Scholar]
- Kai, Z.; Saifeng, D.; Hao, C.; Jingui, W.; Jiaqing, Z.; Yi, G.; Jin, G. Effect of Hydrogen Concentration on the Vented Explosion of Hydrogen–Air Mixtures in a 5-m-Long Duct. Process Saf. Environ. Prot. 2022, 162, 978–986. [Google Scholar]
- Matthijs, W.; Trygve, S.; Dirk, R.; Antoine, D.; Andrzej, P. Chemical Inhibition of Hydrogen-Air Explosions: Literature Review, Simulations and Experiments. Process Saf. Environ. Prot. 2023, 176, 1120–1129. [Google Scholar]
- Tanaka, T.; Azuma, T.; Evans, J.A.; Cronin, P.M.; Johnson, D.M.; Cleaver, R.P. Experimental Study on Hydrogen Explosions in a Full-Scale Hydrogen Filling Station Model. Int. J. Hydrogen Energy 2007, 32, 2162–2170. [Google Scholar] [CrossRef]
- Lowesmith, B.J.; Hankinson, G.; Johnson, D.M. Vapour Cloud Explosions in a Long Congested Region Involving Methane/Hydrogen Mixtures. Process Saf. Environ. Prot. 2011, 89, 234–247. [Google Scholar] [CrossRef]
- Shuhao, Z.; Hongtao, M.; Xiaomei, H.; Shini, P. Numerical Simulation on Methane-Hydrogen Explosion in Gas Compartment in Utility Tunnel. Process Saf. Environ. Prot. 2020, 140, 100–110. [Google Scholar]
- Li, Y.; Bi, M.; Li, B.; Zhou, Y.; Huang, L.; Gao, W. Explosion Hazard Evaluation of Renewable Hydrogen/Ammonia/Air Fuels. Energy 2018, 159, 252–263. [Google Scholar] [CrossRef]
- Li, Y.; Bi, M.; Zhou, Y.; Zhang, Z.; Zhang, K.; Zhang, C.; Gao, W. Characteristics of Hydrogen-Ammonia-Air Cloud Explosion. Process Saf. Environ. Prot. 2021, 148, 1207–1216. [Google Scholar] [CrossRef]
- Ji, W.; Wang, Y.; Yang, J.; He, J.; Lu, C.; Wen, X.; Wang, Y. Explosion Overpressure Behavior and Flame Propagation Characteristics in Hybrid Explosions of Hydrogen and Magnesium Dust. Fuel 2023, 332, 125801. [Google Scholar] [CrossRef]
- Xiong, X.; Gao, K.; Mu, J.; Ji, C.; Li, B.; Zhang, D.; Xu, Y.; Xie, L. Experimental Study on the Explosion Destructive Ability of Magnesium Powder/Hydrogen Hybrids in Large Space. Process Saf. Environ. Prot. 2023, 173, 237–248. [Google Scholar] [CrossRef]
- Yu, X.; Zhang, Z.; Yan, X.; Wang, Z.; Yu, J.; Gao, W. Explosion Characteristics and Combustion Mechanism of Hydrogen/Tungsten Dust Hybrid Mixtures. Fuel 2023, 332, 126017. [Google Scholar] [CrossRef]
- Kim, Y.G.; Park, B.J. Experimental and Analytical Study on Hydrogen-Air Deflagrations in Open Atmosphere. J. Korean Soc. Saf. 2021, 36, 64–71. [Google Scholar]
- Park, B.J.; Kim, Y.G.; Paik, P.W.; Kang, C.K. Numerical and Experimental Analysis of Jet Release and Jet Flame Length for Qualitative Risk Analysis at Hydrogen Refueling Station. Process Saf. Environ. Prot. 2021, 155, 145–154. [Google Scholar] [CrossRef]
- Park, B.J.; Kim, Y.G.; Lee, K.W.; Paik, P.W.; Kang, C.K. Risk Assessment Method Combining Independent Protection Layers (IPL) of Layer of Protection Analysis (LOPA) and RISKCURVES Software. Energies 2021, 14, 4043. [Google Scholar] [CrossRef]
- Piotr, M.; Eugeniusz, M. CFD Analysis of Large-Scale Hydrogen Detonation and Blast Wave Overpressure in Partially Confined Spaces. Process Saf. Environ. Prot. 2022, 158, 537–546. [Google Scholar]
- Hansen, O.R. Hydrogen Infrastructure—Efficient Risk Assessment and Design Optimization Approach to Ensure Safe and Practical Solutions. Process Saf. Environ. Prot. 2020, 143, 164–176. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, Z.; Wei, J.; Lan, Y.; Yang, S.; Jin, T. Evaluation and Prediction of the Safe Distance in Liquid Hydrogen Spill Accident. Process Saf. Environ. Prot. 2021, 146, 1–8. [Google Scholar] [CrossRef]
- Kashkarov, S.; Li, Z.; Molkov, V. Blast Wave from a Hydrogen Tank Rupture in a Fire in the Open: Hazard Distance Nomograms. Int. J. Hydrogen Energy 2020, 45, 2429–2446. [Google Scholar] [CrossRef]
- Molkov, V.; Kashkarov, S. Blast Wave from a High-Pressure Gas Tank in a Fire: Stand-Alone and Under-Vehicle Hydrogen Tanks. Int. J. Hydrogen Energy 2015, 40, 12581–12603. [Google Scholar] [CrossRef]
- Brock, V.; Javad, M.; Fatemeh, S.; Rouzbeh, A. Safety Assessment of Hydrogen Jet Fire Scenarios within Semi-Confined Spaces. Fire 2023, 6, 29. [Google Scholar]
- Xirui, Y.; Depeng, K.; Xu, H.; Ping, P. Risk Analysis of Fire and Explosion of Hydrogen-Gasoline Hybrid Refueling Station Based on Accident Risk Assessment Method for Industrial System. Fire 2023, 6, 181. [Google Scholar]
- Korea Land & Housing Corporation. 2023. Available online: www.rorenhouse.co.kr (accessed on 1 January 2023).
- Park, B.J.; Kim, Y.G.; Hwang, I.J. An Experimental Study on the Explosion Hazards in the Fuel Cell Room of Residential House. J. Korean Soc. Saf. 2021, 36, 71–79. [Google Scholar]
- Center for Chemical Process Safety at the American Institute of Chemical Engineers. Guidelines for Chemical Process Quantitative Risk Analysis, 2nd ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2000; pp. 157–179. [Google Scholar]
- Center for Chemical Process Safety at the American Institute of Chemical Engineers. Guidelines for Evaluating the Characteristics of Vapor Cloud Explosions, Flash Fires, and BLEVEs; American Institute of Chemical Engineers: New York, NY, USA, 1994; pp. 347–357. [Google Scholar]
- ANSYS Inc. ANSYS Fluent Theory Guide Release 2020 R2. 2020. Available online: https://www.ansys.com (accessed on 1 January 2023).
- Holtappels, K. Report on the Experimentally Determined Explosion Limits, Explosion Pressures and Rates of Explosion Pressure Rise—Part 1: Methane, Hydrogen, and Propylene. Available online: https://www.morechemistry.com/SAFEKINEX/deliverables/44.Del.%20No.%208.pdf (accessed on 1 January 2002).
- Kuznetsov, M.; Friedrich, A.; Stern, G.; Kotchourko, N.; Jallais, S.; L’Hostis, B. Medium-Scale Experiments on Vented Hydrogen Deflagration. J. Loss Prev. Process Ind. 2015, 36, 416–428. [Google Scholar] [CrossRef]
- Solomos, G.; Larcher, M.; Valsamos, G.; Karlos, V.; Casadei, F. A Survey of Computational Models for Blast Induced Human Injuries for Security and Defence Applications; Joint Research Centre Technical Reports; Publications office of the European Union, EU: Luxembourg, 2020. [Google Scholar]
- Crowl, D.A.; Louvar, J.F. Chemical Process Safety Fundamentals with Applications, 4th ed.; Pearson Education Inc.: London, UK, 2019; pp. 264–282. [Google Scholar]
- Li, B.; Han, B.; Li, Q.; Gao, W.; Guo, C.; Lv, H.; Liu, Y.; Jin, X.; Bi, M. Study on Hazards from High-Pressure On-Board Type III Hydrogen Tank in Fire Scenario: Consequences and Response Behaviours. Int. J. Hydrogen Energy 2022, 47, 2759–2770. [Google Scholar] [CrossRef]
- Debroey, J. Probit Function Analysis of Blast Effects on Human Beings; Royal Military Academy: Leuven, Bergium, 2016. [Google Scholar]
- Jeffries, R.M.; Hunt, S.J.; Gould, L. Derivation of Fatality Probability Function for Occupants’ Buildings Subject to Blast Loads; WS Atkins Sci Technol 1997; Contract Research Report for HSE 147; HSE Books: Norwich, UK, 1997. [Google Scholar]
- NFPA. NFPA 2 Hydrogen Technologies Code; NFPA: Quincy, MA, USA, 2020; pp. 2169–7471. [Google Scholar]
- Health and Safety Executive. Methods of Approximation and Determination of Human Vulnerability for Offshore Major Accident Hazard Assessment. Supporting Document to SPC 30. 2010. Available online: https://www.hse.gov.uk/foi/internalops/hid_circs/technical_osd/spc_tech_osd_30/spctecosd30.pdf (accessed on 1 January 2023).
- LaChance, J.; Tchouvelev, A.; Engebo, A. Development of Uniform Harm Criteria for Use in Quantitative Risk Analysis of the Hydrogen Infrastructure. Int. J. Hydrogen Energy 2011, 36, 2381–2388. [Google Scholar] [CrossRef]





| Incident Pressure (kPa) | Damage Level |
|---|---|
| 0.15 | Annoying noise |
| 0.2 | Occasional breaking of large windowpanes already under strain |
| 0.3 | Loud noise; sonic boom glass failure |
| 0.7 | Breakage of small windows under strain |
| 1 | Threshold for glass breakage |
| 2 | “Safe distance”, probability of 0.95 of no serious damage beyond this value; some damage to house ceilings; 10% window glass broken |
| 3 | Limited minor structural damage |
| 3.5–7 | Large and small windows usually shattered; occasional damage to window frames |
| 5 | Minor damage to house structures |
| 8 | Partial demolition of houses, made uninhabitable |
| 9.5 | Second-degree burns after 20 s |
| 12.5~15 | First-degree burns after 10 s, 1% fatality within 1 min |
| 25 | Significant injury in 10 s, 100% fatality within 1 min |
| 35~37.5 | 1% fatality within 10 s |
| Radiant Heat Flux Intensity (kW/m2) | Effects on People |
|---|---|
| 1.5 | No harm; safe for the general public and for stationery personnel |
| 2.5 | Intensity tolerable for five min; severe pain above this exposure time |
| 3 | Intensity tolerable for non-frequent emergency situations for 30 min |
| 5 | Pain for 20 s exposure, first-degree burns. Intensity tolerable for those performing emergency operations |
| 6 | Intensity tolerable for escaping emergency personnel |
| 9.5 | Second-degree burns after 20 s |
| 12.5~15 | First-degree burns after 10 s, 1% fatality within 1 min |
| 25 | Significant injury in 10 s, 100% fatality within 1 min |
| 35~37.5 | 1% fatality within 10 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, B.; Kim, Y.; Hwang, I.-J. Risk Assessment of Explosion Accidents in Hydrogen Fuel-Cell Rooms Using Experimental Investigations and Computational Fluid Dynamics Simulations. Fire 2023, 6, 390. https://doi.org/10.3390/fire6100390
Park B, Kim Y, Hwang I-J. Risk Assessment of Explosion Accidents in Hydrogen Fuel-Cell Rooms Using Experimental Investigations and Computational Fluid Dynamics Simulations. Fire. 2023; 6(10):390. https://doi.org/10.3390/fire6100390
Chicago/Turabian StylePark, Byoungjik, Yangkyun Kim, and In-Ju Hwang. 2023. "Risk Assessment of Explosion Accidents in Hydrogen Fuel-Cell Rooms Using Experimental Investigations and Computational Fluid Dynamics Simulations" Fire 6, no. 10: 390. https://doi.org/10.3390/fire6100390
APA StylePark, B., Kim, Y., & Hwang, I.-J. (2023). Risk Assessment of Explosion Accidents in Hydrogen Fuel-Cell Rooms Using Experimental Investigations and Computational Fluid Dynamics Simulations. Fire, 6(10), 390. https://doi.org/10.3390/fire6100390




