Experimental Study of a Nonthermal DBD-Driven Plasma Jet System Using Different Supply Methods
Abstract
:1. Introduction
2. Materials and Methods
2.1. System Specifications and Reactor Setup
2.1.1. Plasma Jet Requirements for Wound Disinfection
- Gas temperature is a principal indicator of applicability; if the temperature of the jet differs substantially from body temperature, treatment will be painful. Temperatures above 42 °C denature proteins, and exposed tissues die. The power injected into the discharge determines the temperature of the gas in the plasma.
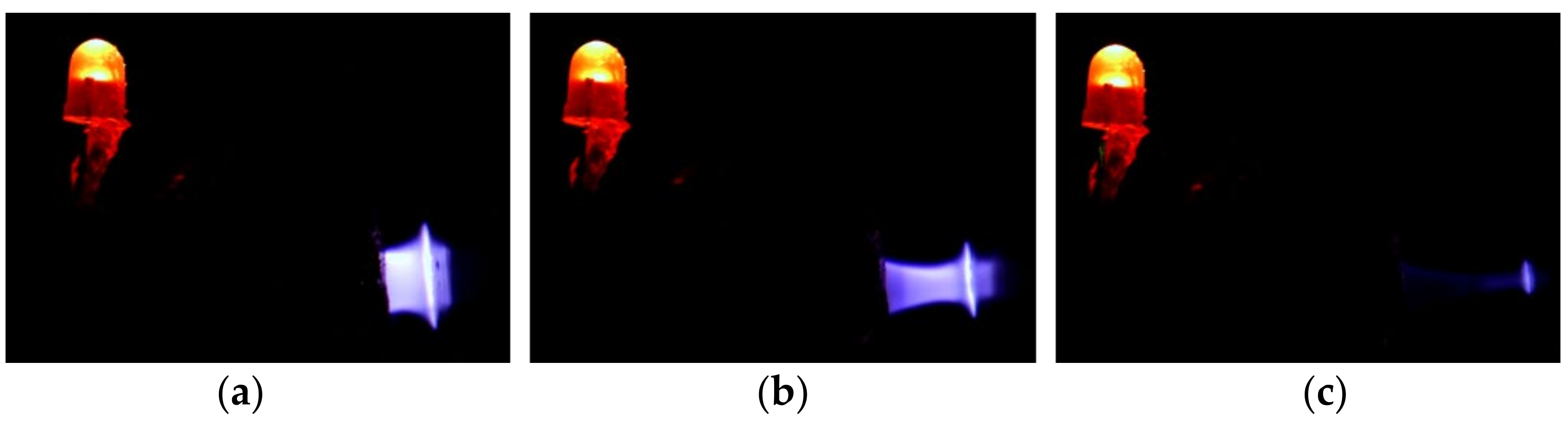
- Plasma plume length is the distance from the end of the reactor to the treated surface. It is related to the electrical isolation requirements, to prevent the patient and the caregiver from receiving an electric shock. A longer plasma plume length guarantees noncontact treatment and decreases the probability of high-voltage breakdown with the treated surface. Long plasma plumes are also convenient when processing surfaces with complex shapes.
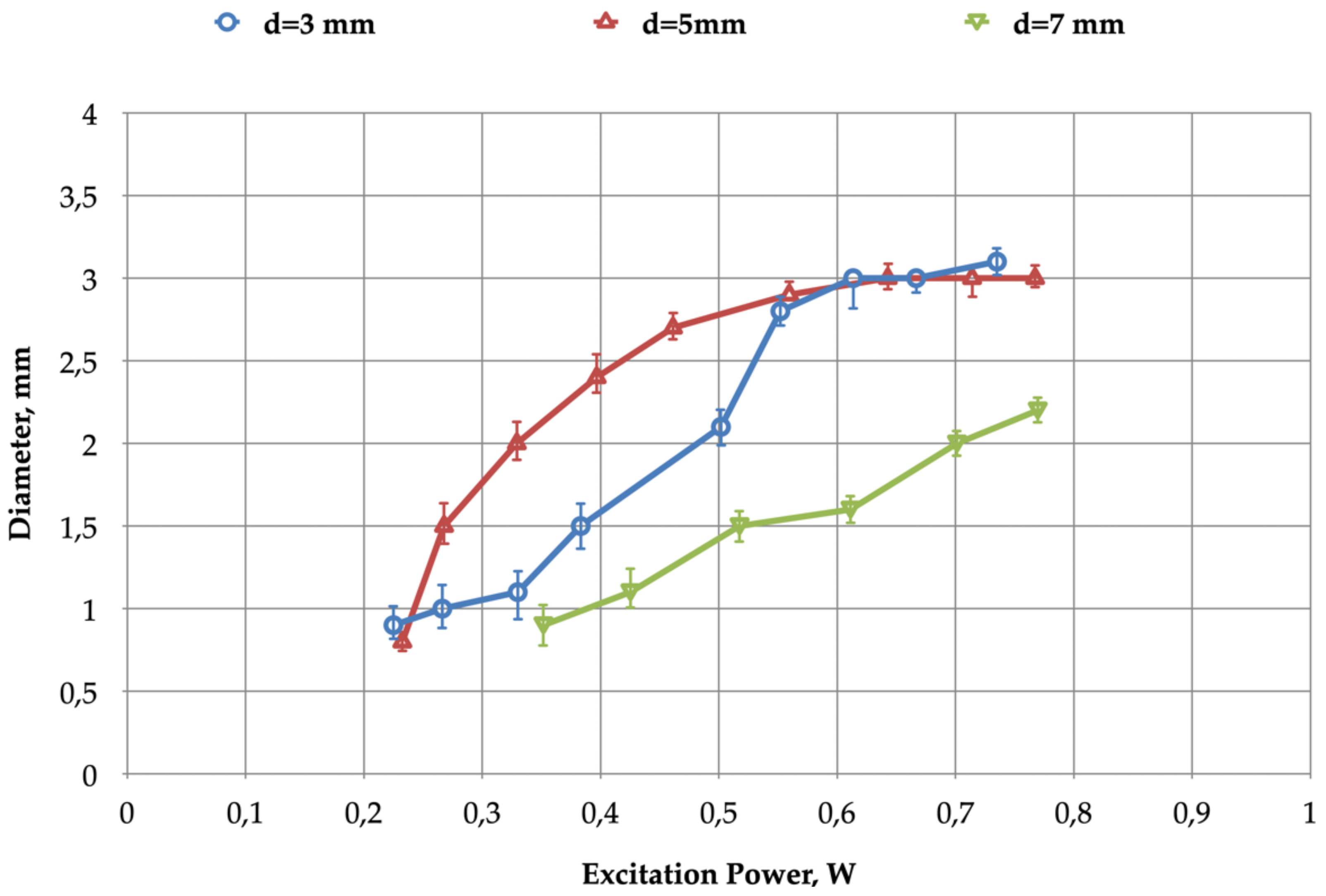
- Plasma jet diameter. The shape of the plume is generally conical; the diameter of the spot should match the surface of the wound. A wide plasma–surface contact area shortens treatment time. An increase in the plasma jet diameter reduces current density in the jet and ensures a more uniform gas temperature distribution.
2.1.2. Plasma Jet Reactor Setup
2.2. Power Supplies
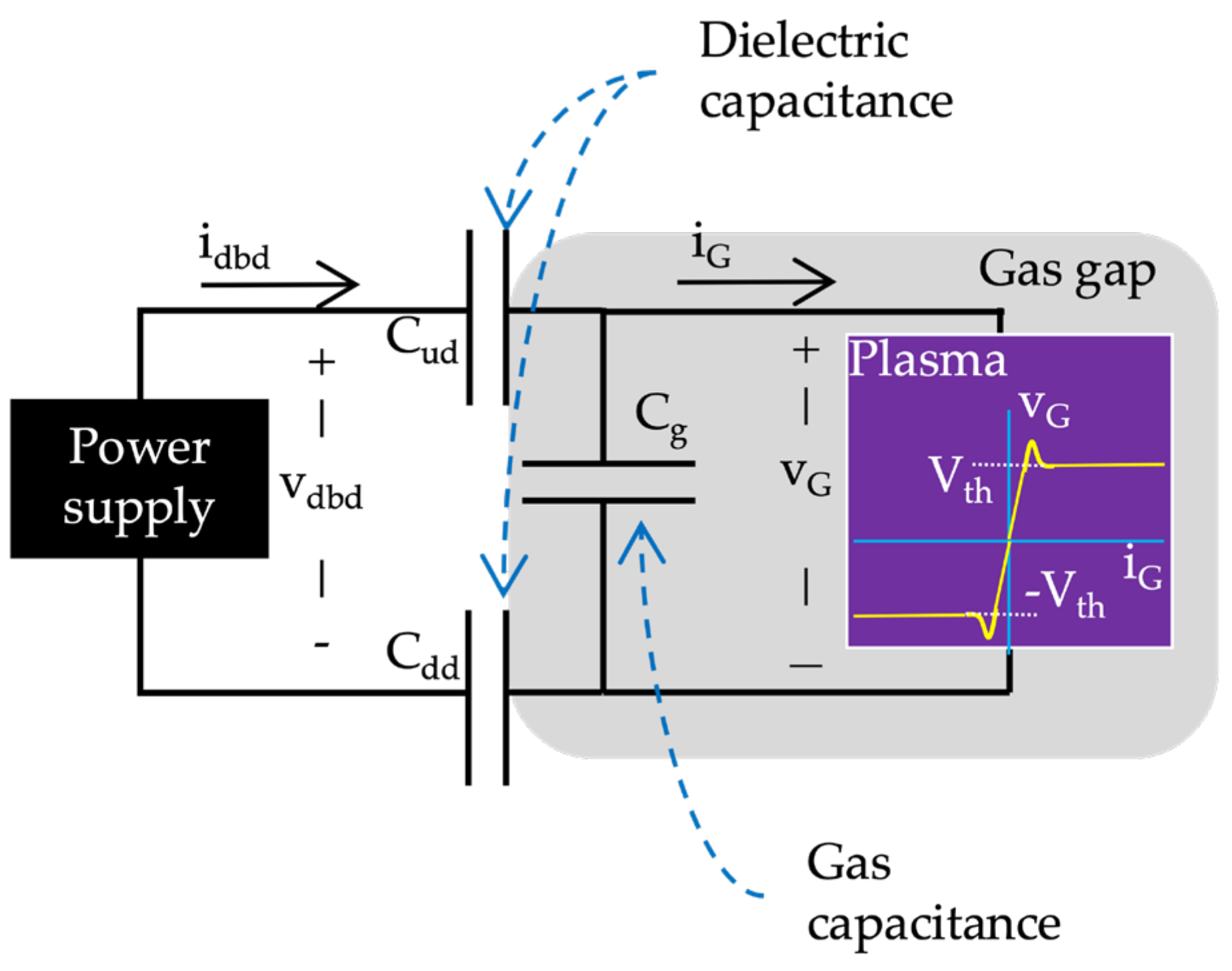
2.2.1. Equivalent Circuit of the Plasma Jet (DBD)
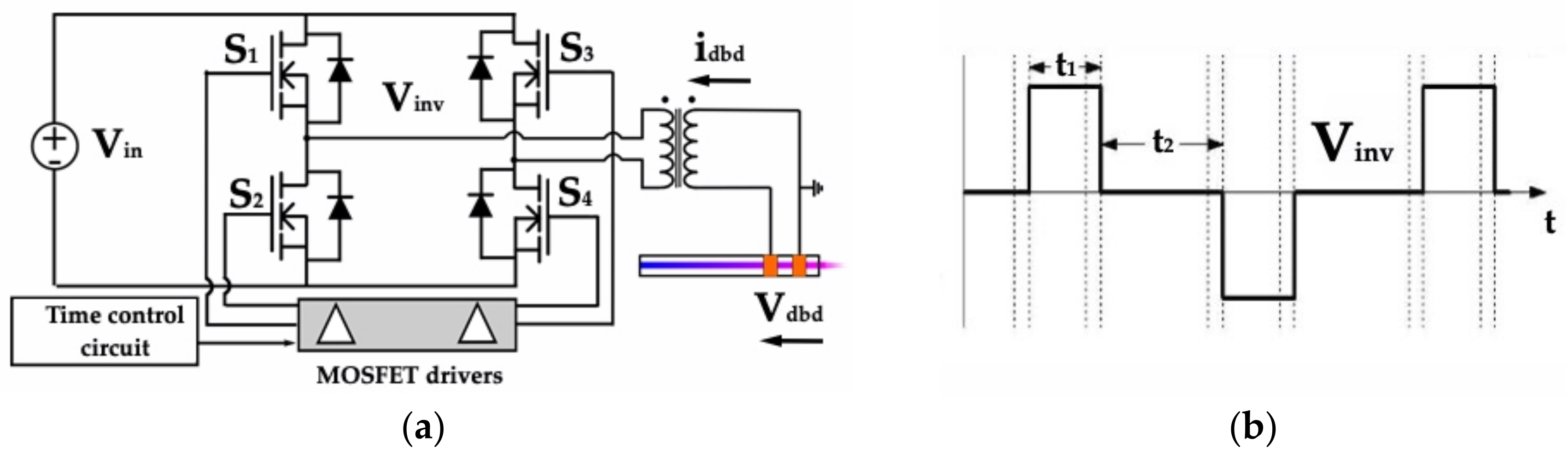
2.2.2. Bipolar Short-Pulse (BSP) Voltage Supply
- The magnitude of the positive and negative levels is linearly adjusted using the Vin voltage; the typical range is 100–300 V.
- The pulse duration (t1) is adjustable in the range of 0.75–1.5 µs.
- Frequency varies in the range of 5–20 kHz, considering the limitations of the step-up transformer. The latter provides an additional degree of freedom with regard to designing the system through its turns ratio; the selected turns ratio is 1:10.
2.2.3. Series Resonant Inverter (SRI) Current Supply
2.2.4. Power Injection, Energy Transfers, and Control of Supply Operation
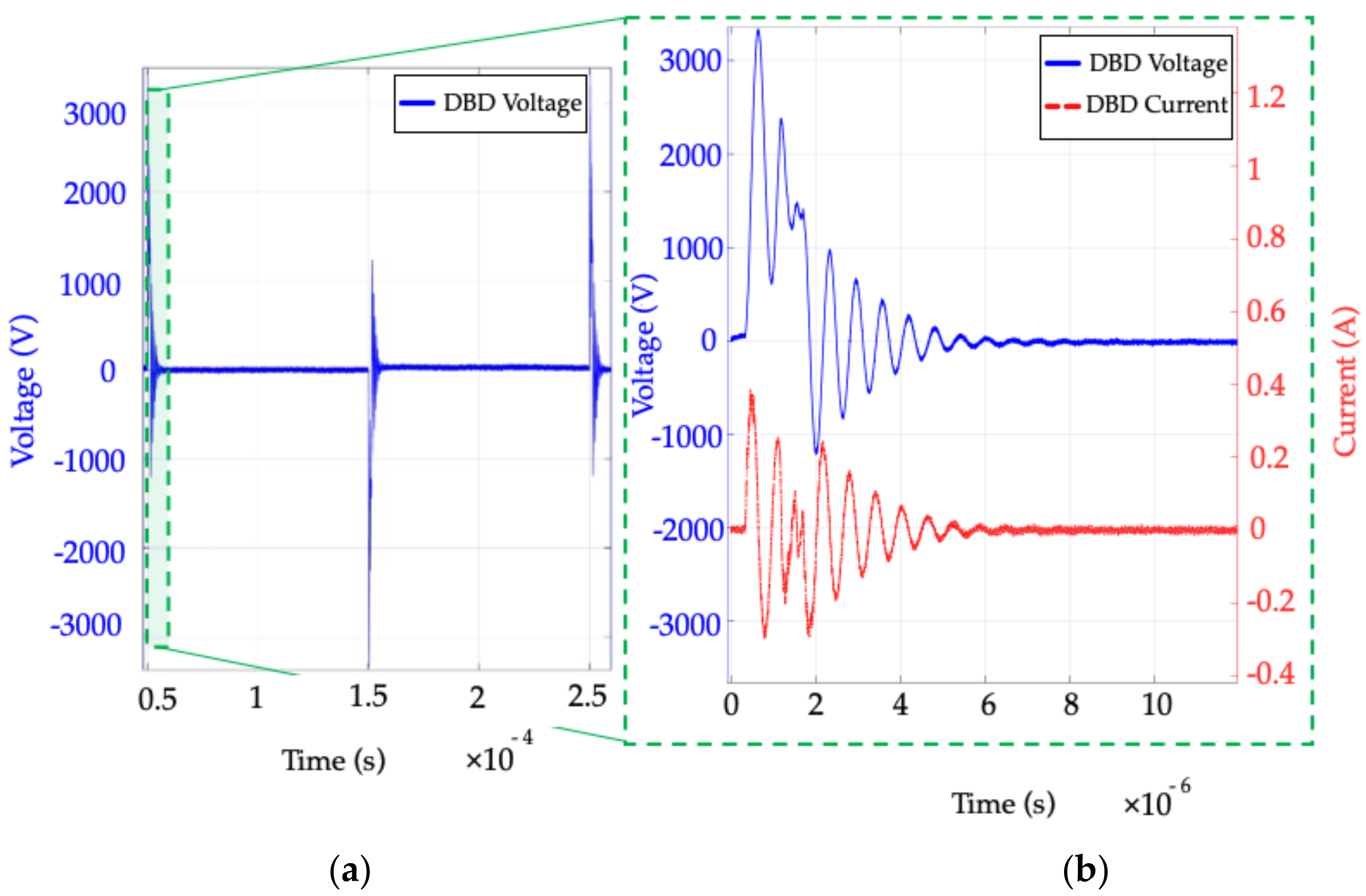
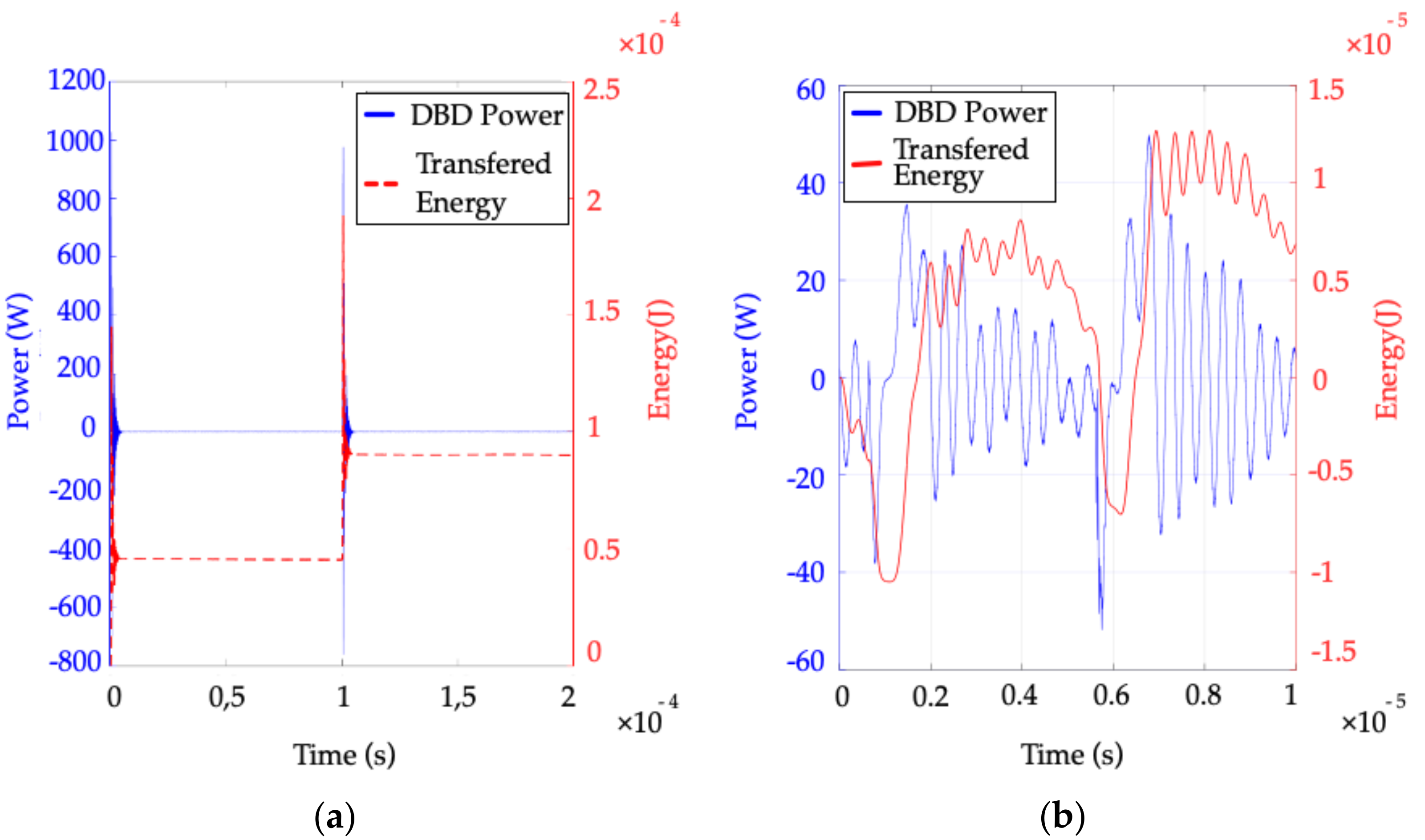
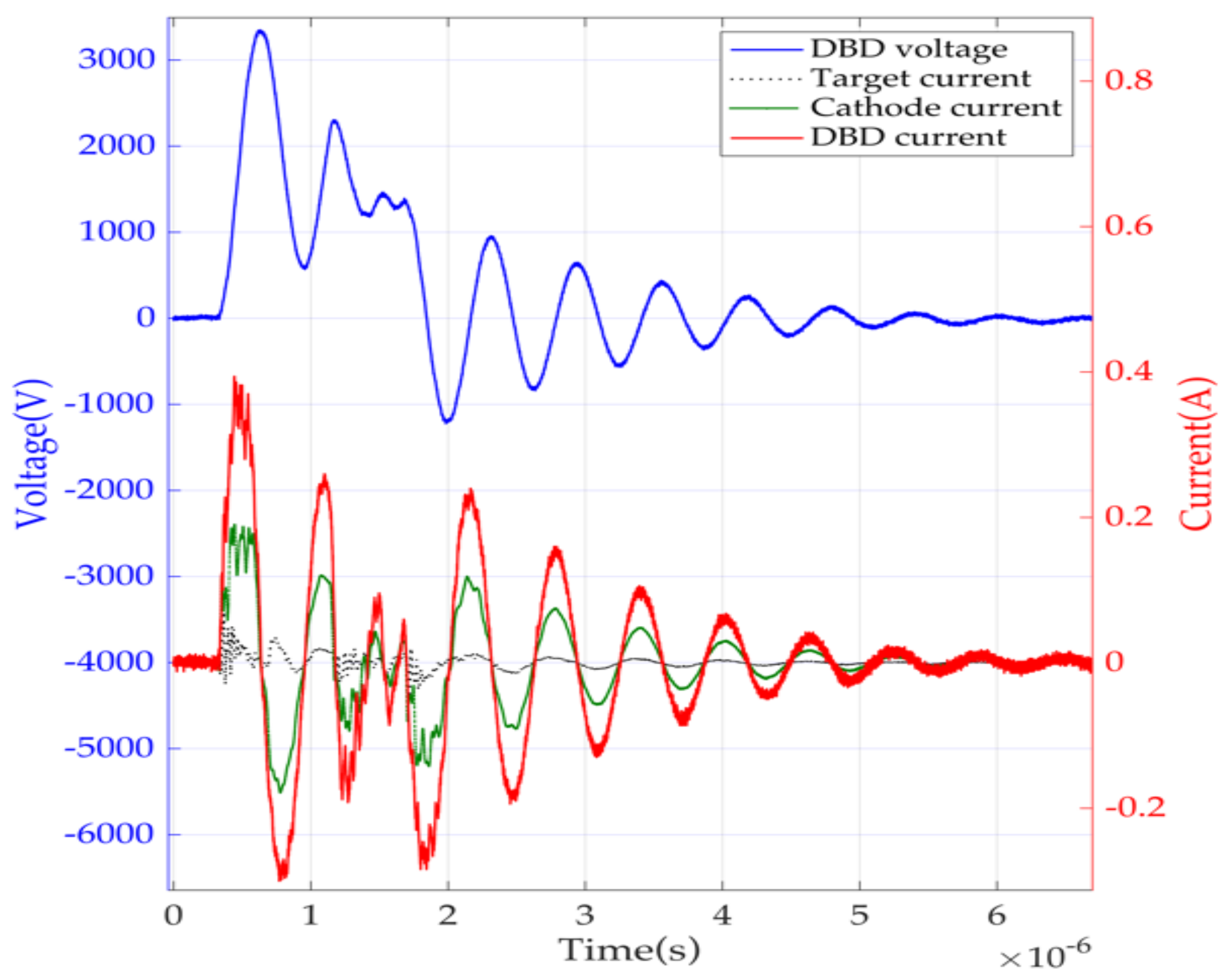
- The BSP voltage supply allows adjustment of the jet power, using three degrees of freedom (DOF): the input voltage, Vin, the bridge switching frequency, fsw, and the pulse duration, t1. As indicated previously, the DBD jet is capacitive; thus, its current depends on the rise and fall times of the voltage pulses, as does the jet power, Pdbd. The switch response times and transformer parasitic elements affect the rise and fall times of the voltage pulses delivered to the jet, making it difficult to determine the output power analytically [13]. The output power, however, can be estimated using Manley’s diagram or Equation (6) to find out the energy transferred to the plasma over one period. The average power is then calculated using Equation (5). In practice, due to parasitic elements of the circuit, the DBD peak voltage used in Equation (5) is difficult to define accurately. The input voltage, Vin, is adjusted to obtain the desired average power. The power injection pause (the time interval during which no energy is injected) plays an important role in the efficiency of the DBD-driven process [12]. In the BSP, t2 (see Figure 4) is set directly by the inverter’s control board.
- As the previous section shows, two DOFs are available in the SRI for operating condition control: the input voltage, Vin, and the bridge switching frequency, fsw. However, the current pulse duration is fixed and depends on the L value. As stated in the comment for Figure 8, power delivery is much longer (throughout the current pulse), and the power injection pause interval is the difference between the operating period and the duration of the current pulse; this is a much lower percentage of the Tsw period than for the BSP converter.
- With the short Tsw timescale of the power supply operation (high-frequency fsw), Edbd is controlled by the parameters shown in Equations (5) and (7).
- At average values, considering TBurst = 1/fBurst time (much longer than Tsw; see Equation (10)), the injected power is adjusted using the MBurst and Toff variables, with Equations (9) and (10) in the low-frequency domain, at fBurst.
3. Results
3.1. Test Bench
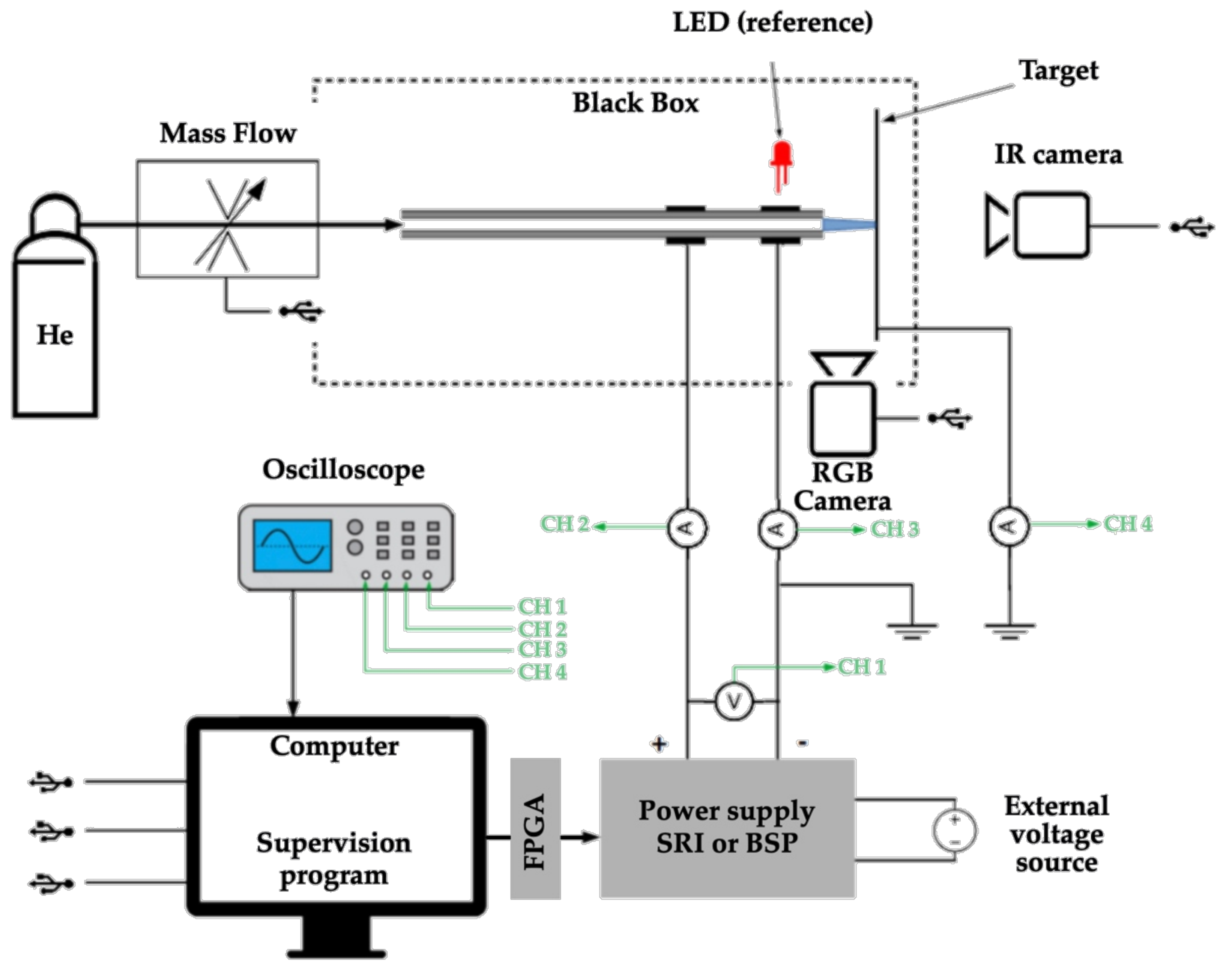
3.1.1. Overall Setup
- Good ability to reproduce tests with selected operating conditions;
- Full storage of the experimental records and conditions under which these records were obtained.
- An oscilloscope provided full measurements of the electrical conditions: Vdbd (channel 1 in Figure 10) and Idbd, measured on both electrodes (channels 2 and 3). The current flowing from the screen target (copper foil connected to the ground) was also measured for jet electrical diagnostics (channel 4).
- An IR camera focused on the rear side of the copper foil target measured the temperature.
- A digital RGB camera took pictures of the jet to measure its dimensions. The jet and the camera were installed in a closed box painted black to enhance the images.
3.1.2. Measurements
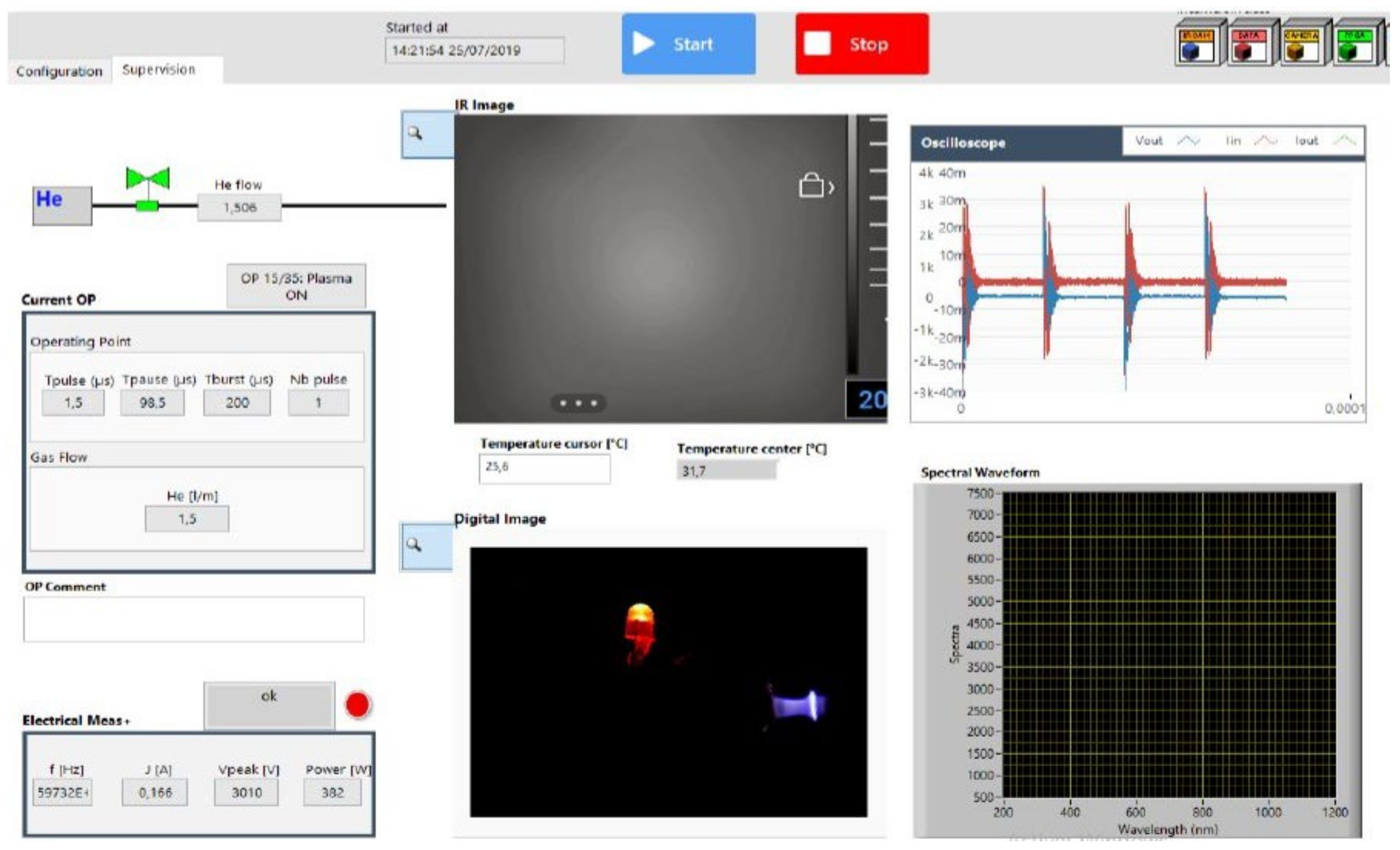
3.1.3. Supervision and Post-Treatments
- Configuration of all the devices;
- Monitoring of data acquisition. Each operating condition was characterized by a series of at least five different sets of measurements, allowing experimental identification of margins of error;
- The setup and monitoring of measurement batches with variations in the operating conditions: gas flow and common and specific parameters to the BSP or SRI power supply (Vin, fsw).
- Storage of a whole set of data for each operating point as follows:
- ○
- The configuration of each device, making it possible to reproduce any experiment;
- ○
- Storage of all the electrical waveforms (raw numerical data and oscilloscope screen copies);
- ○
- Storage of all jet measurements (temperature, geometry, and spectroscopy results).
- Start of post-treatment calculation scripts;
- Organization of all the parameters and measurements into a database.
3.2. Experimental Results
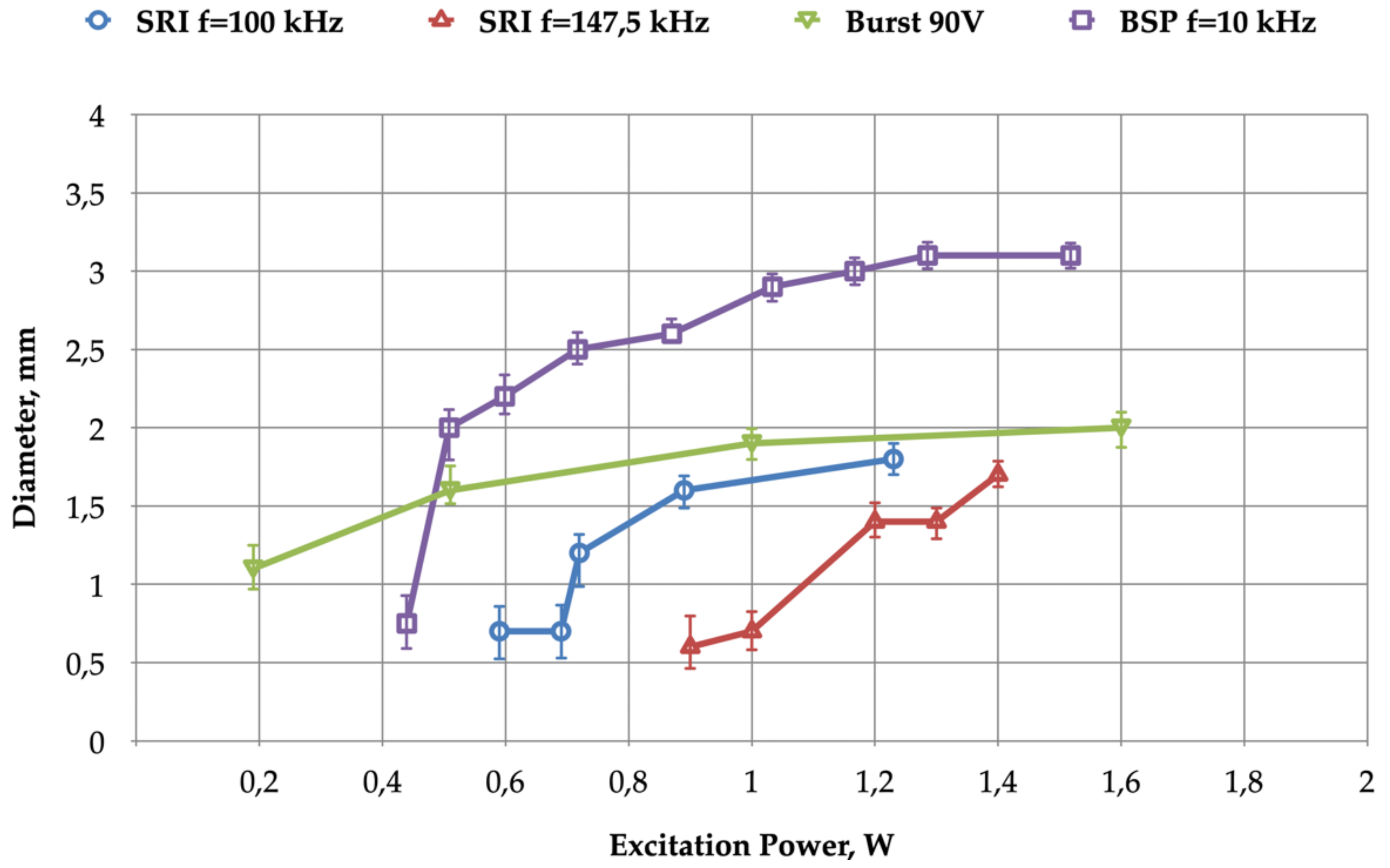
3.2.1. Power Supply Evaluation
- Performance obtained at lower frequencies (100 kHz for SRI, 5 kHz and 10 kHz for BSP) is much smoother and more controllable.
- A lower operating frequency of the supply means lower switching losses.
- From an electrical design perspective, higher frequencies are associated with more compact and lighter solutions.
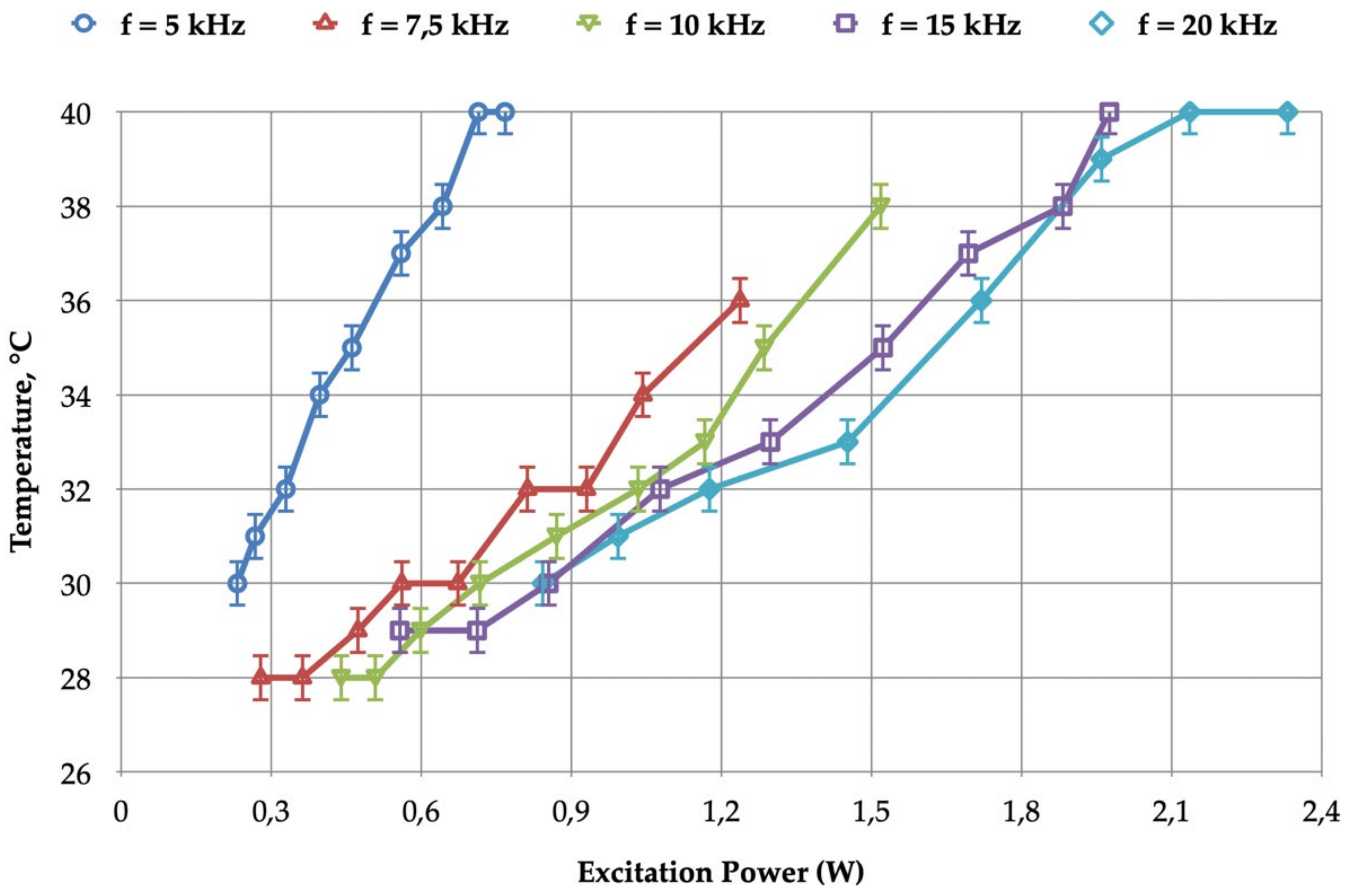
3.2.2. BSP Power Supply Investigations
4. Discussion
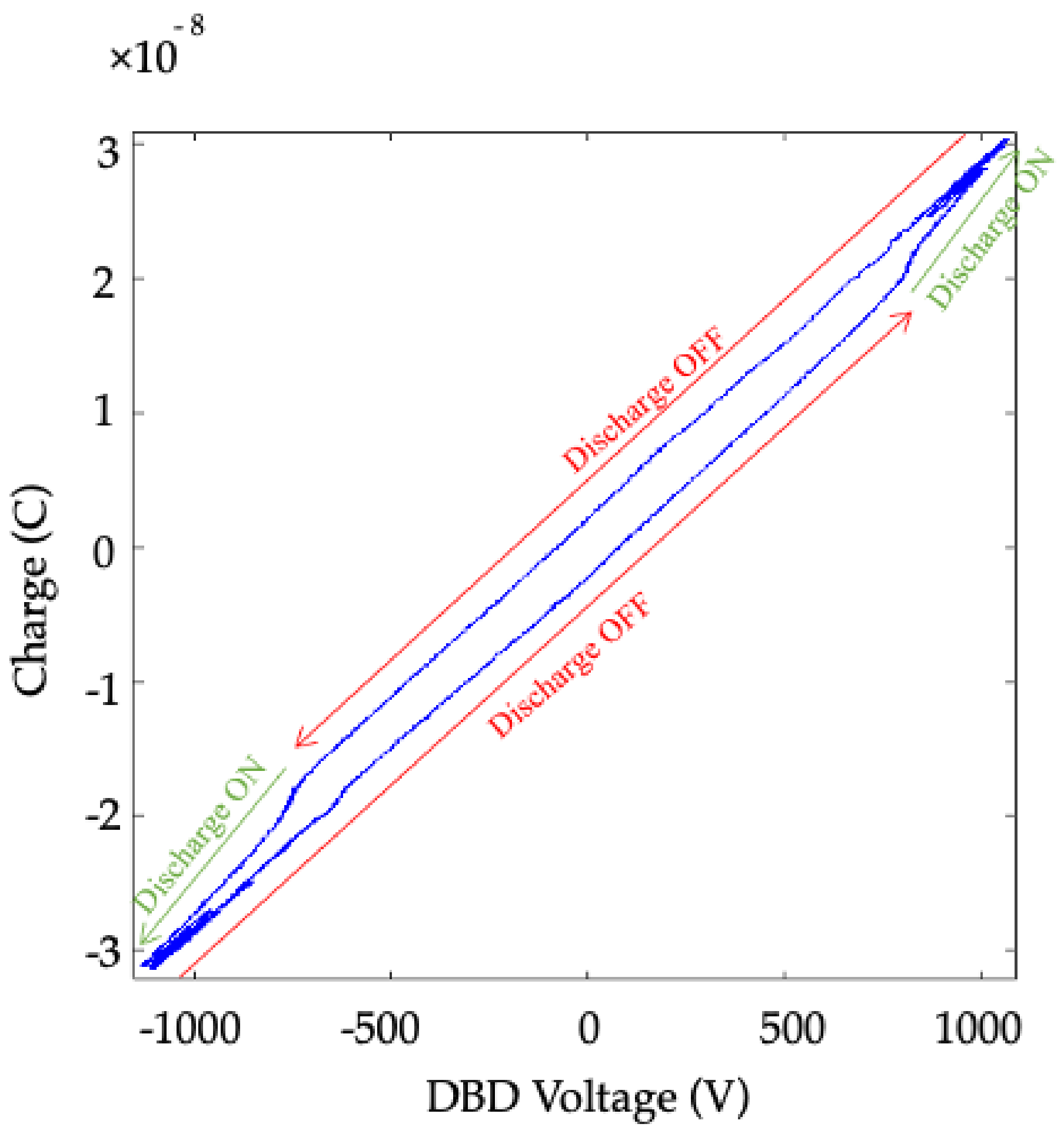
4.1. Plasma Jet Equivalent Circuit Used for Supply Design
4.2. Comparison with Behavior Described in the Literature
- Our jet setup has many similarities with the configuration described in [4]. For the latter, different power supplies were considered: USP (unipolar short voltage pulses) and sine excitation voltage supplies. The gas temperature of the plasma, measured with a bimetal thermometer as shown in [4], had a variation trend with regard to the gas flow rate very similar to that presented here in Figure 18 (considering the operating points observed, once the gas flow was high enough to obtain plume contact with the target). Moreover, the experimental parameters of both supplies used in [4] were set in a range compatible with our investigations: frequency of 33 kHz and excitation power of 4.2 W.
- The authors of [24] described experiments on a plasma jet generated using helium as a working gas. The authors used an AC power supply unit (providing 8 to 10 kVpp voltages at a frequency of 20 kHz), operating in a range comparable to ours. The temperature measurements in [24] were carried out using a method different from ours, with a mercury thermometer placed at the end of the plasma jet, and not at a set distance; in our case, the temperature was measured on the rear side of the target (a thin copper foil) using an IR camera. The authors of [24] presented the following results with similar trends to ours, especially:
- ○
- The dependence of the plasma temperature versus the flow rate (corresponding to Figure 18);
- ○
4.3. Power Injection Pause
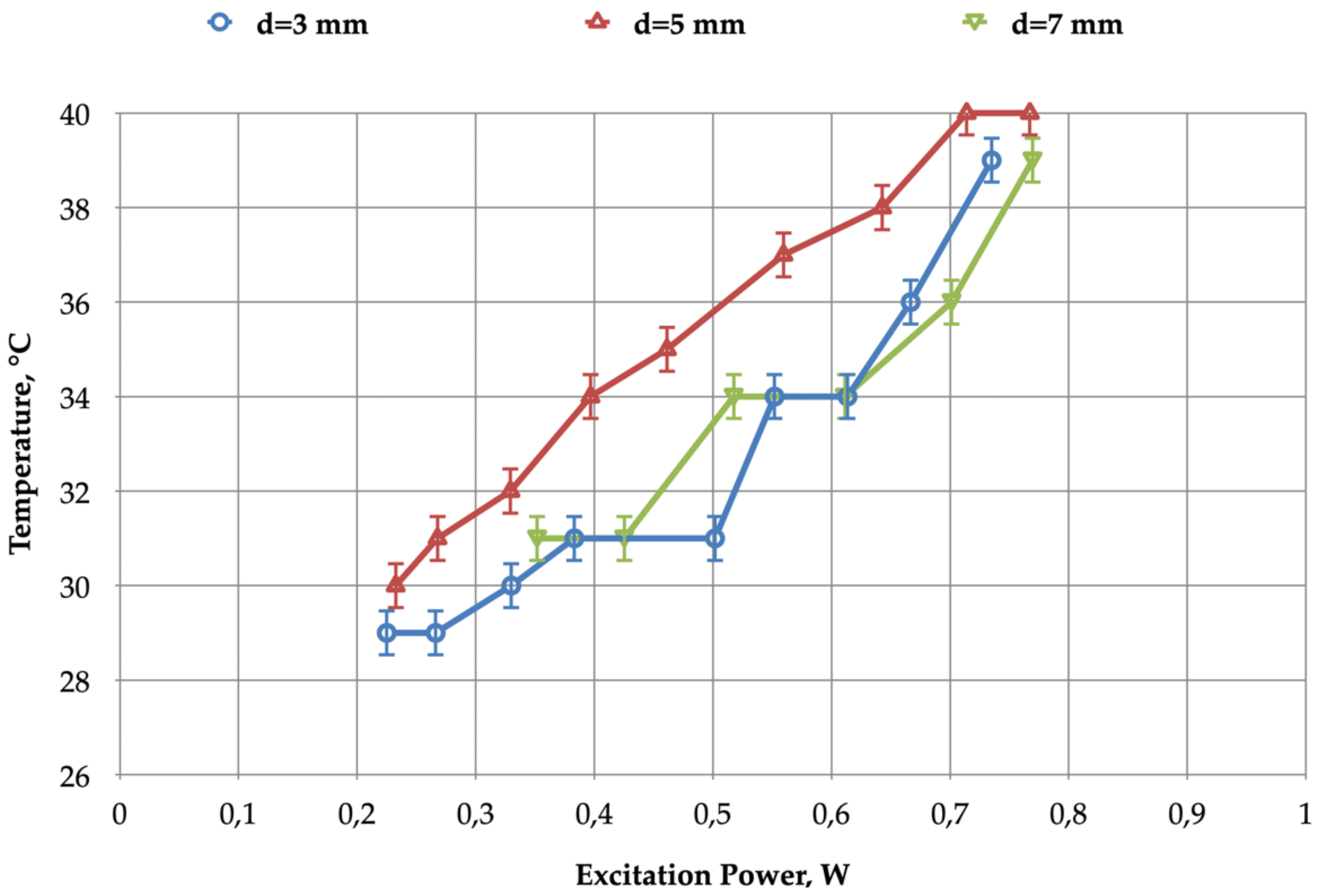
4.4. Applicability of the Investigated System
- According to our communications with healthcare professionals, it is necessary to start by ensuring that the configuration is financially feasible; thus, a maximum gas flow has to be defined;
- Subsequently, it must be ensured that the configuration will allow operation at a safe and comfortable distance from the application area, i.e., a distance of at least 5 mm;
- From this step, temperature should be considered, in order to ensure comfortable and safe use of the device, without exceeding the limit (human body temperature);
- The diameter of the plasma will determine both efficacy of use and the speed of the treatment procedure; this will also affect the operating cost.
- According to the results of the previous four steps, the plots provided in this paper can be used (provided the jet configuration is the same as it is here) to select the necessary excitation level. Accordingly, the power supply and its operating conditions that offer best performance matching can be selected.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Machala, P.Z.; Hensel, K.; Akishev, Y. Plasma for Bio-Decontamination, Medicine and Food Security; NATO Science for Peace and Security Series A: Chemistry and Biology; Springer: Berlin, Germany, 2012. [Google Scholar] [CrossRef]
- Laroussi, M. Nonthermal decontamination of biological media by atmospheric-pressure plasmas: Review, analysis, and prospects. IEEE Trans. Plasma Sci. 2002, 30, 1409–1415. [Google Scholar] [CrossRef]
- Zhdanova, O.S.; Kuznetsov, V.S.; Panarin, V.A.; Skakun, V.S.; Sosnin, É.A.; Tarasenko, V.F. A Planar Source of Atmospheric-Pressure Plasma Jet. Plasma Phys. Rep. 2018, 44, 153–156. [Google Scholar] [CrossRef]
- Schitz, D.; Ivankov, A.; Pismennyi, V. Nonthermal Plasma Jet for Biomedical Applications. KnE Energy 2018, 3, 456–461. [Google Scholar] [CrossRef]
- Isbary, G.; Zimmermann, J.; Shimizu, T.; Li, Y.-F.; Morfill, G.; Thomas, H.; Steffes, B.; Heinlin, J.; Karrer, S.; Stolz, W. Non-thermal plasma—More than five years of clinical experience. Clin. Plasma Med. 2013, 1, 19–23. [Google Scholar] [CrossRef]
- Heinlin, J.; Morfill, G.; Landthaler, M.; Stolz, W.; Isbary, G.; Zimmermann, J.L.; Shimizu, T.; Karrer, S. Plasma medicine: Possible applications in dermatology. JDDG J. Deutsch. Dermatol. Ges. 2010, 8, 968–976. [Google Scholar] [CrossRef] [PubMed]
- Lopes, B.B.; Kraft, M.B.D.P.L.; Rehder, J.; Batista, F.R.X.; Puzzi, M.B. The Interactions between Non-thermal Atmospheric Pressure Plasma and Ex-vivo Dermal Fibroblasts. Procedia Eng. 2013, 59, 92–100. [Google Scholar] [CrossRef] [Green Version]
- Kolb, J.F.; Mohamed, A.-A.; Price, R.O.; Swanson, R.J.; Bowman, A.; Chiavarini, R.L.; Stacey, M.; Schoenbach, K.H. Cold atmospheric pressure air plasma jet for medical applications. Appl. Phys. Lett. 2008, 92, 241501. [Google Scholar] [CrossRef] [Green Version]
- Gerling, T.; Brandenburg, R.; Wilke, C.; Weltmann, K.-D. Power measurement for an atmospheric pressure plasma jet at different frequencies: Distribution in the core plasma and the effluent. Eur. Phys. J. Appl. Phys. 2017, 78, 10801. [Google Scholar] [CrossRef] [Green Version]
- Asenjo, J.; Mora, J.; Vargas, A.; Brenes, L.; Montiel, R.; Arrieta, J.; Vargas, V. Atmospheric-Pressure Non-Thermal Plasma Jet for biomedical and industrial applications. J. Phys. Conf. Ser. 2015, 591, 012049. [Google Scholar] [CrossRef] [Green Version]
- Friedman, P.C.; Miller, V.; Fridman, G. Use of cold atmospheric pressure plasma to treat warts: A potential therapeutic option. Clin. Exp. Dermatol. 2019, 44, 459–461. [Google Scholar] [CrossRef]
- Nicol, M.J.; Brubaker, T.R.; Ii, B.J.H.; Simmons, A.N.; Kazemi, A.; Geissel, M.A.; Whalen, C.T.; Siedlecki, C.A.; Bilén, S.G.; Knecht, S.D.; et al. Antibacterial effects of low-temperature plasma generated by atmospheric-pressure plasma jet are mediated by reactive oxygen species. Sci. Rep. 2020, 10, 3066. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Florez, D.; Schitz, D.; Piquet, H.; Diez, R. Efficiency of an Exciplex DBD Lamp Excited Under Different Methods. IEEE Trans. Plasma Sci. 2017, 46, 140–147. [Google Scholar] [CrossRef] [Green Version]
- Stepanova, O.; Rybalchenko, O.; Pinchuk, M.; Astafiev, A.; Orlova, O.; Spodobin, V.A.; Kudryavtsev, A. Bactericidal Effect of a Dielectric Barrier Discharge Plasma Jet Generated in Laminar and Preturbulent Helium Flows. Plasma Med. 2017, 7, 187–200. [Google Scholar] [CrossRef]
- Sosnin, E.A.; Stoffels, E.; Erofeev, M.V.; Kieft, I.E.; Kunts, S.E. The Effects of UV Irradiation and Gas Plasma Treatment on Living Mammalian Cells and Bacteria: A Comparative Approach. IEEE Trans. Plasma Sci. 2004, 32, 1544–1550. [Google Scholar] [CrossRef]
- Joh, H.M.; Kang, H.R.; Chung, T.H.; Kim, S.J. Electrical and Optical Characterization of Atmospheric-Pressure Helium Plasma Jets Generated With a Pin Electrode: Effects of the Electrode Material, Ground Ring Electrode, and Nozzle Shape. IEEE Trans. Plasma Sci. 2014, 42, 3656–3667. [Google Scholar] [CrossRef]
- Lee, H.W.; Nam, S.H.; Mohamed, A.-A.H.; Kim, G.C.; Lee, J.K. Atmospheric Pressure Plasma Jet Composed of Three Electrodes: Application to Tooth Bleaching. Plasma Process. Polym. 2010, 7, 274–280. [Google Scholar] [CrossRef]
- Fang, Z.; Shao, T.; Yang, J.; Zhang, C. Discharge processes and an electrical model of atmospheric pressure plasma jets in argon. Eur. Phys. J. D 2016, 70, 1–8. [Google Scholar] [CrossRef]
- Daeschlein, G.; Scholz, S.; Ahmed, R.; von Woedtke, T.; Haase, H.; Niggemeier, M.; Kindel, E.; Brandenburg, R.; Weltmann, K.-D.; Juenger, M. Skin decontamination by low-temperature atmospheric pressure plasma jet and dielectric barrier discharge plasma. J. Hosp. Infect. 2012, 81, 177–183. [Google Scholar] [CrossRef]
- Florez, D. Power Supplies for Dielectric Barrier Discharges—Toward an Efficient Use of Ultraviolet Exciplex Lamps; Editorial Pontificia Universidad Javeriana: Bogota, Colombia, 2018. [Google Scholar]
- Choi, J.H.; Song, Y.S.; Song, K.; Lee, H.J.; Hong, J.W.; Kim, G.C. Skin renewal activity of non-thermal plasma through the activation of β-catenin in keratinocytes. Sci. Rep. 2017, 7, 6146. [Google Scholar] [CrossRef] [Green Version]
- Manley, T.C. The Electric Characteristics of the Ozonator Discharge. Trans. Electrochem. Soc. 1943, 84, 83. [Google Scholar] [CrossRef]
- Nguyen, D.B.; Trinh, Q.H.; Hossain, M.; Lee, W.G.; Mok, Y.S. Improvement of Electrical Measurement of a Dielectric Barrier Discharge Plasma Jet. IEEE Trans. Plasma Sci. 2019, 47, 2004–2010. [Google Scholar] [CrossRef]
- Hammoodi, R.; Khalaf, M.; Abbas, A. Construction of the Non-Thermal Helium Plasma Jet using Two Electrodes and studying its Characteristics. Int. J. Sci. Res. IJSR 2018, 8, 1372–1378. [Google Scholar] [CrossRef]
- Bouzidi, M.C.; Bonnin, X.; Naudé, N.; Piquet, H.; Belinger, A.; Gherardi, N. Maximization of the working domain of an Atmospheric Pressure Townsend Discharge (APTD) using a current-source static converter. J. Phys. Conf. Ser. 2014, 550, 012044. [Google Scholar] [CrossRef] [Green Version]
- Belinger, A.; Naudé, N.; Gherardi, N. Transition from diffuse to self-organized discharge in a high frequency dielectric barrier discharge. Eur. Phys. J. Appl. Phys. 2017, 79, 10802. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z.; Li, J. Repetitively pulsed gas discharges: Memory effect and discharge mode transition. High Volt. 2020, 5, 569–582. [Google Scholar] [CrossRef]
- Starikovskiy, A.; Aleksandrov, N. Plasma-assisted ignition and combustion. Prog. Energy Combust. Sci. 2013, 39, 61–110. [Google Scholar] [CrossRef] [Green Version]
- Piquet, H.; Bhosle, S.; Diez, R.; Erofeev, M.V. Pulsed Current-Mode Supply of Dielectric Barrier Discharge Excilamps for the Control of the Radiated Ultraviolet Power. IEEE Trans. Plasma Sci. 2010, 38, 2531–2538. [Google Scholar] [CrossRef] [Green Version]
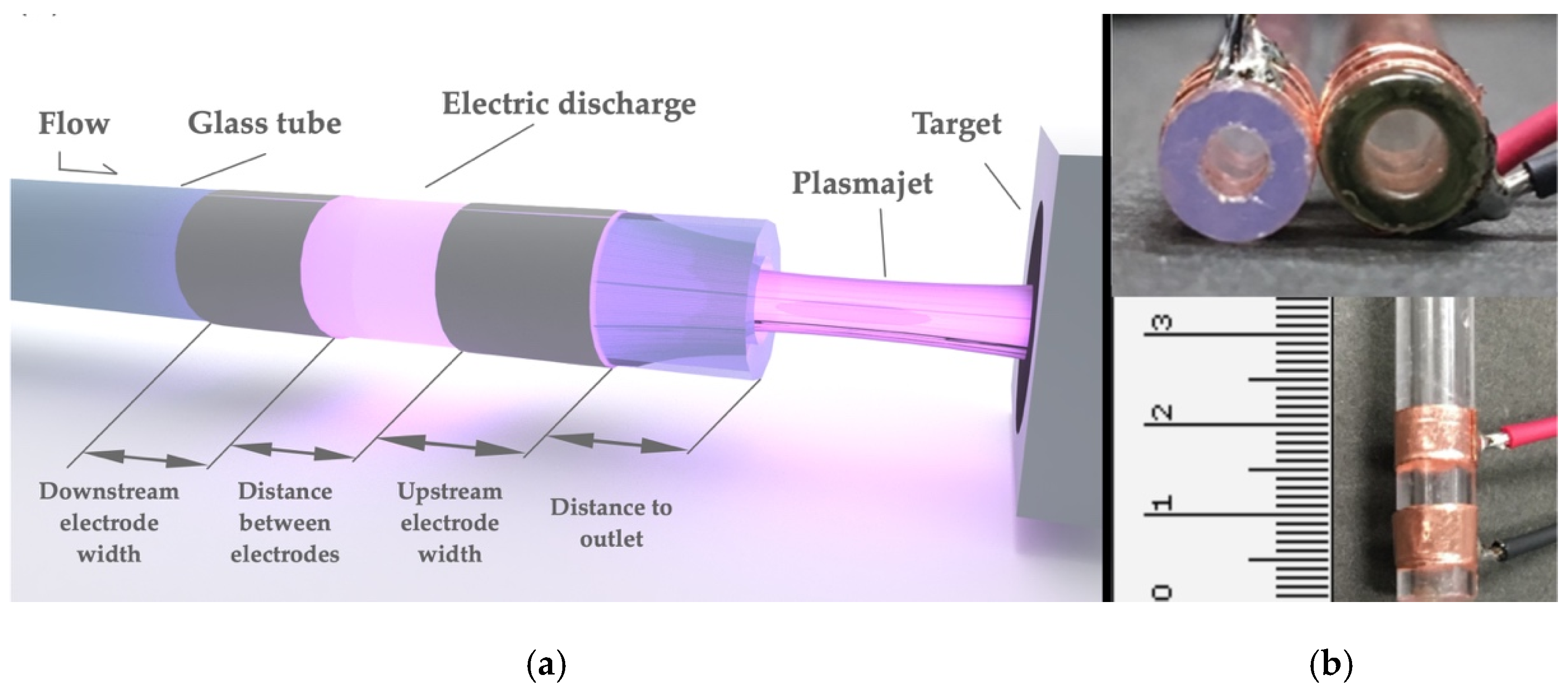





| Pipe (Borosilicate) | Electrodes (Copper Rings) | ||
|---|---|---|---|
| Permittivity | 7 | Width | 5 mm |
| External diameter | 4.5 mm | Distance between electrodes | 3 mm |
| Thickness | 0.8 mm | Distance to outlet | 5 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ivankov, A.; Capela, T.; Rueda, V.; Bru, E.; Piquet, H.; Schitz, D.; Florez, D.; Diez, R. Experimental Study of a Nonthermal DBD-Driven Plasma Jet System Using Different Supply Methods. Plasma 2022, 5, 75-97. https://doi.org/10.3390/plasma5010007
Ivankov A, Capela T, Rueda V, Bru E, Piquet H, Schitz D, Florez D, Diez R. Experimental Study of a Nonthermal DBD-Driven Plasma Jet System Using Different Supply Methods. Plasma. 2022; 5(1):75-97. https://doi.org/10.3390/plasma5010007
Chicago/Turabian StyleIvankov, Anton, Tony Capela, Vanesa Rueda, Eric Bru, Hubert Piquet, Dmitry Schitz, David Florez, and Rafael Diez. 2022. "Experimental Study of a Nonthermal DBD-Driven Plasma Jet System Using Different Supply Methods" Plasma 5, no. 1: 75-97. https://doi.org/10.3390/plasma5010007
APA StyleIvankov, A., Capela, T., Rueda, V., Bru, E., Piquet, H., Schitz, D., Florez, D., & Diez, R. (2022). Experimental Study of a Nonthermal DBD-Driven Plasma Jet System Using Different Supply Methods. Plasma, 5(1), 75-97. https://doi.org/10.3390/plasma5010007







