Non-Uniform Drying Shrinkage in Robocasted Green Body Ceramic Products
Abstract
1. Introduction
2. Materials and Methods
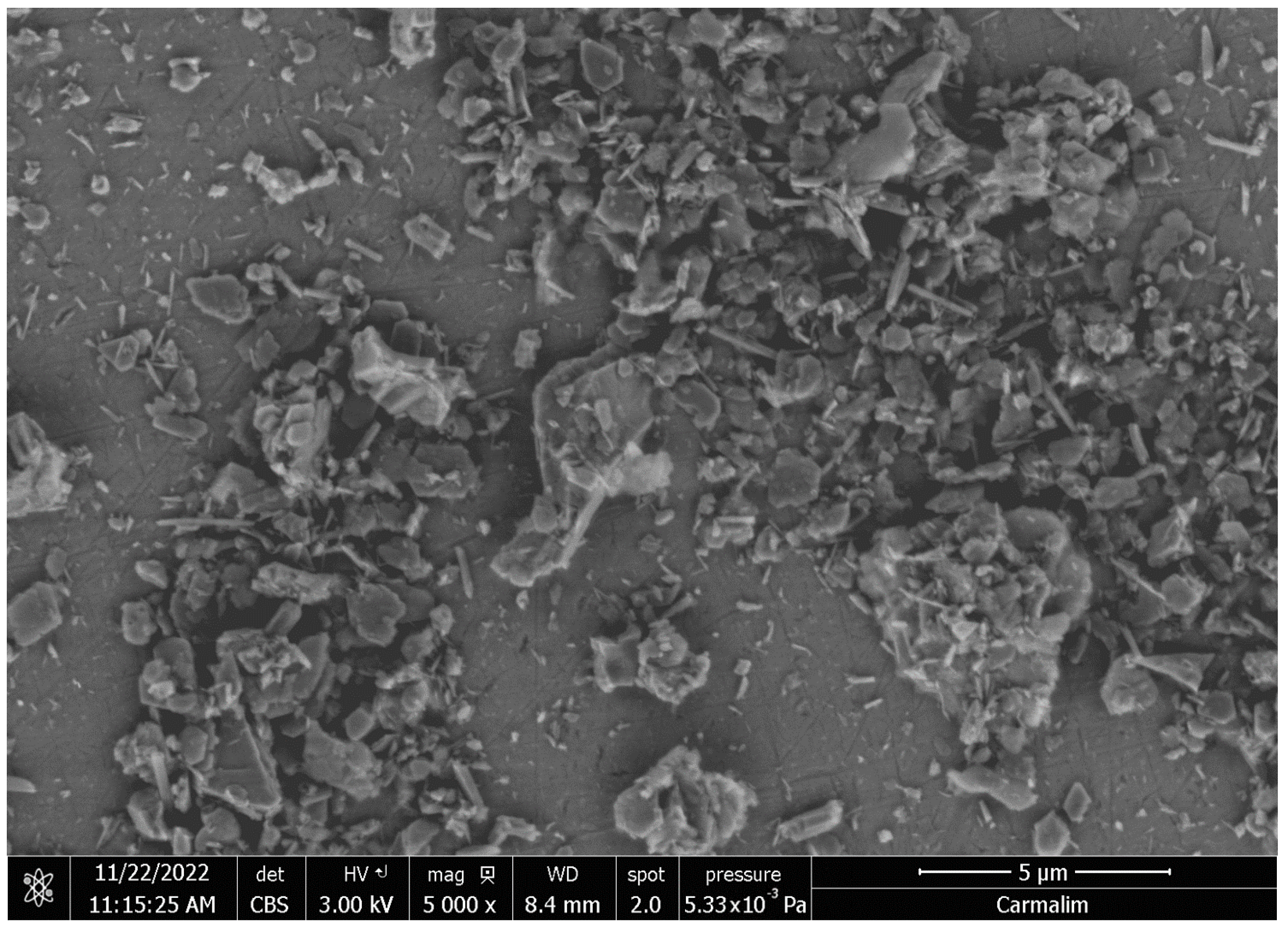
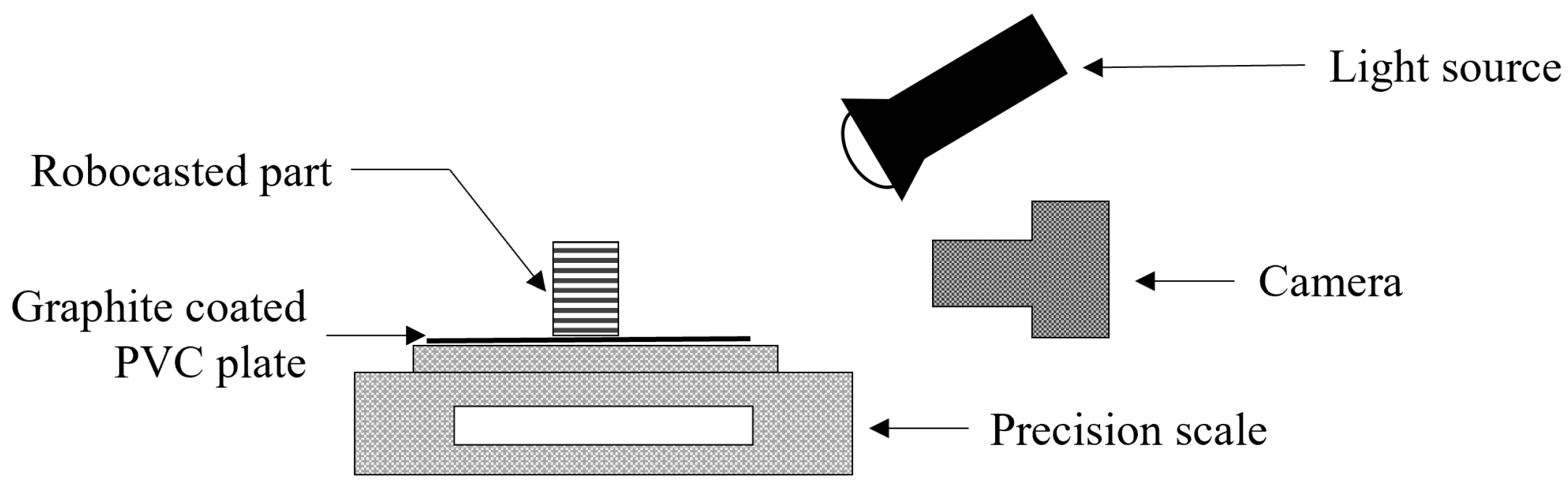
2.1. Materials and Devices
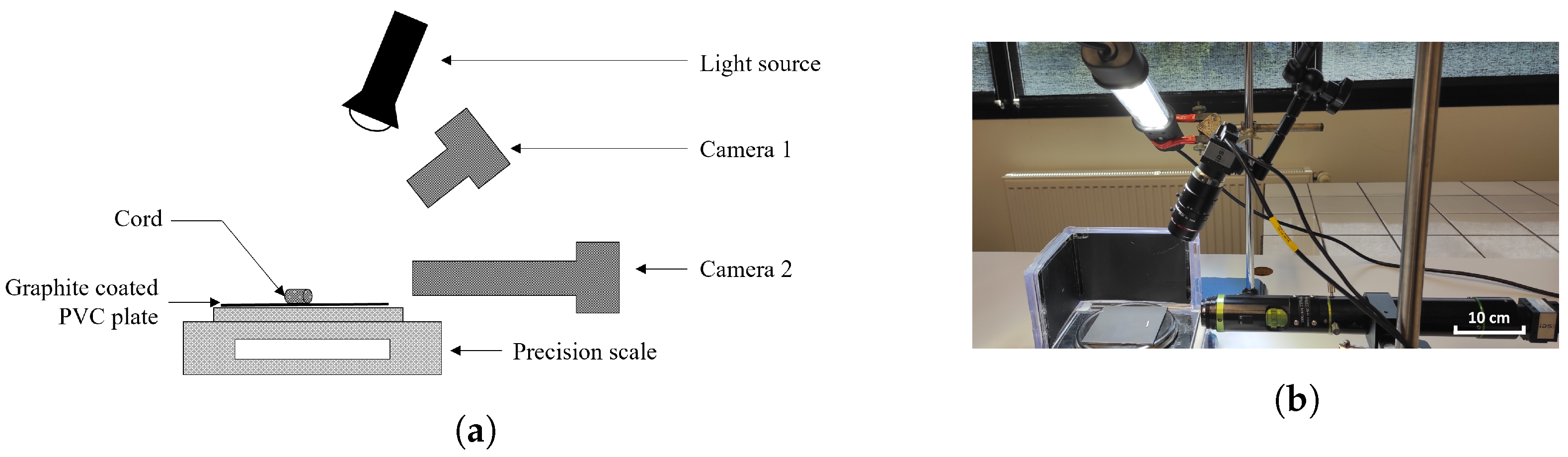
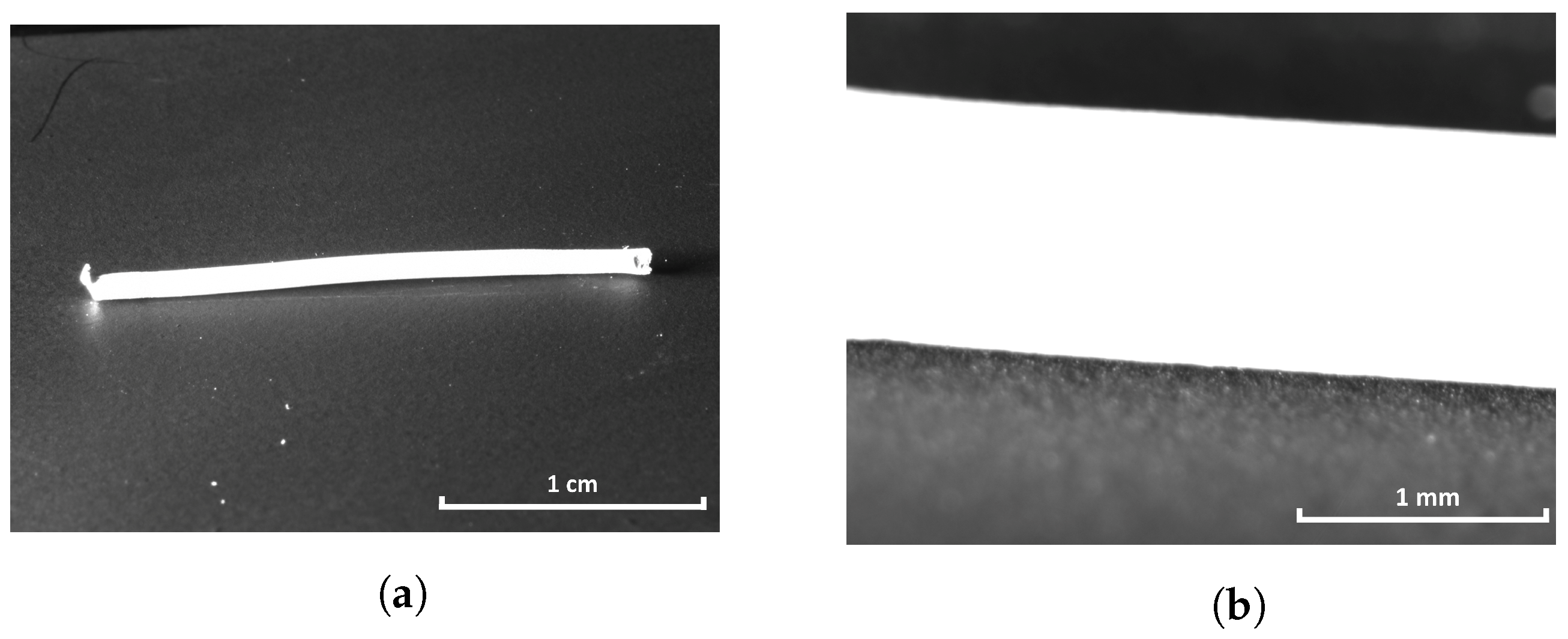
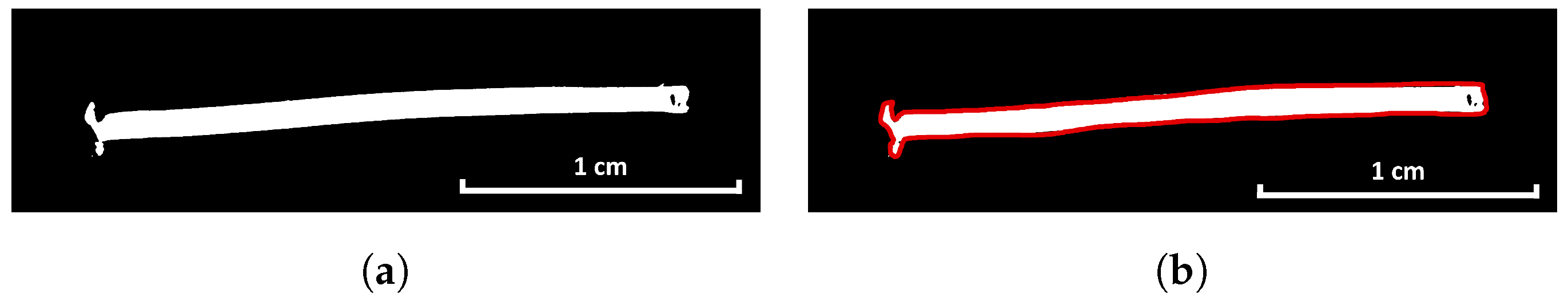
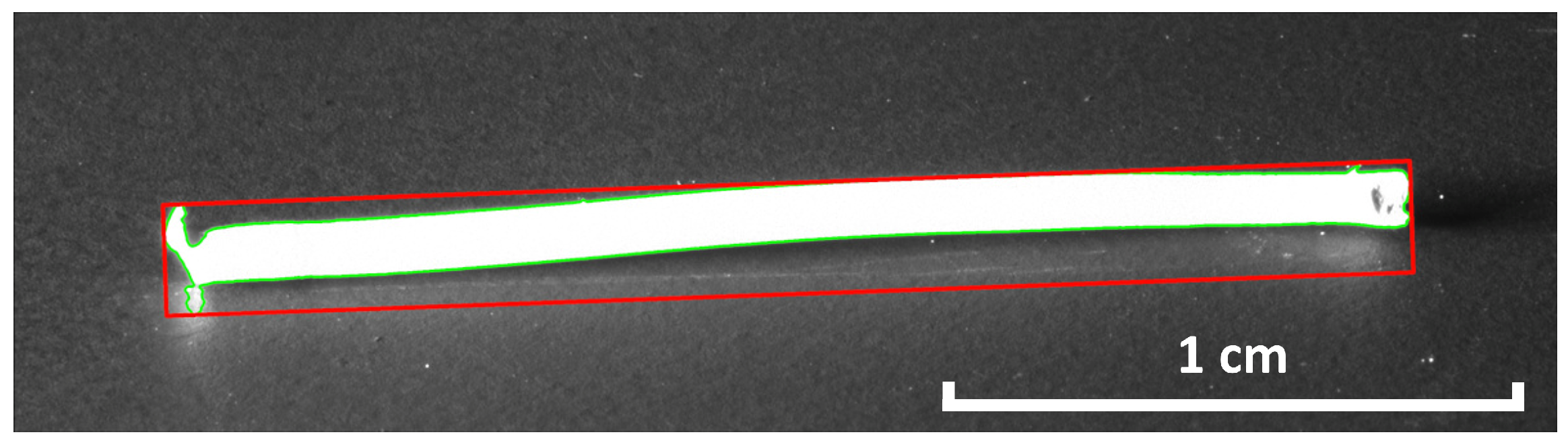
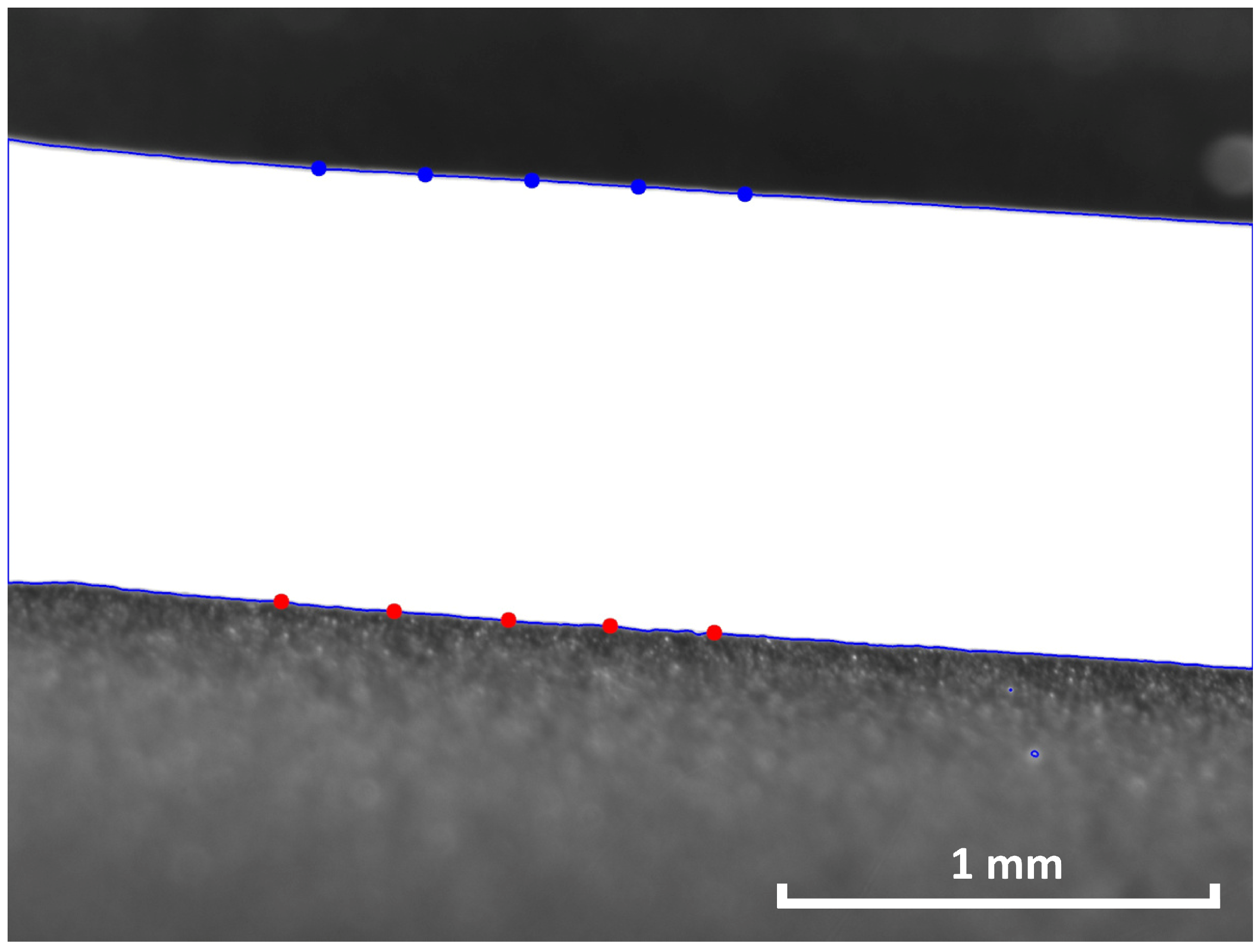
2.2. Measuring the Drying Shrinkage of an Individual Robocasted Ceramic Cord
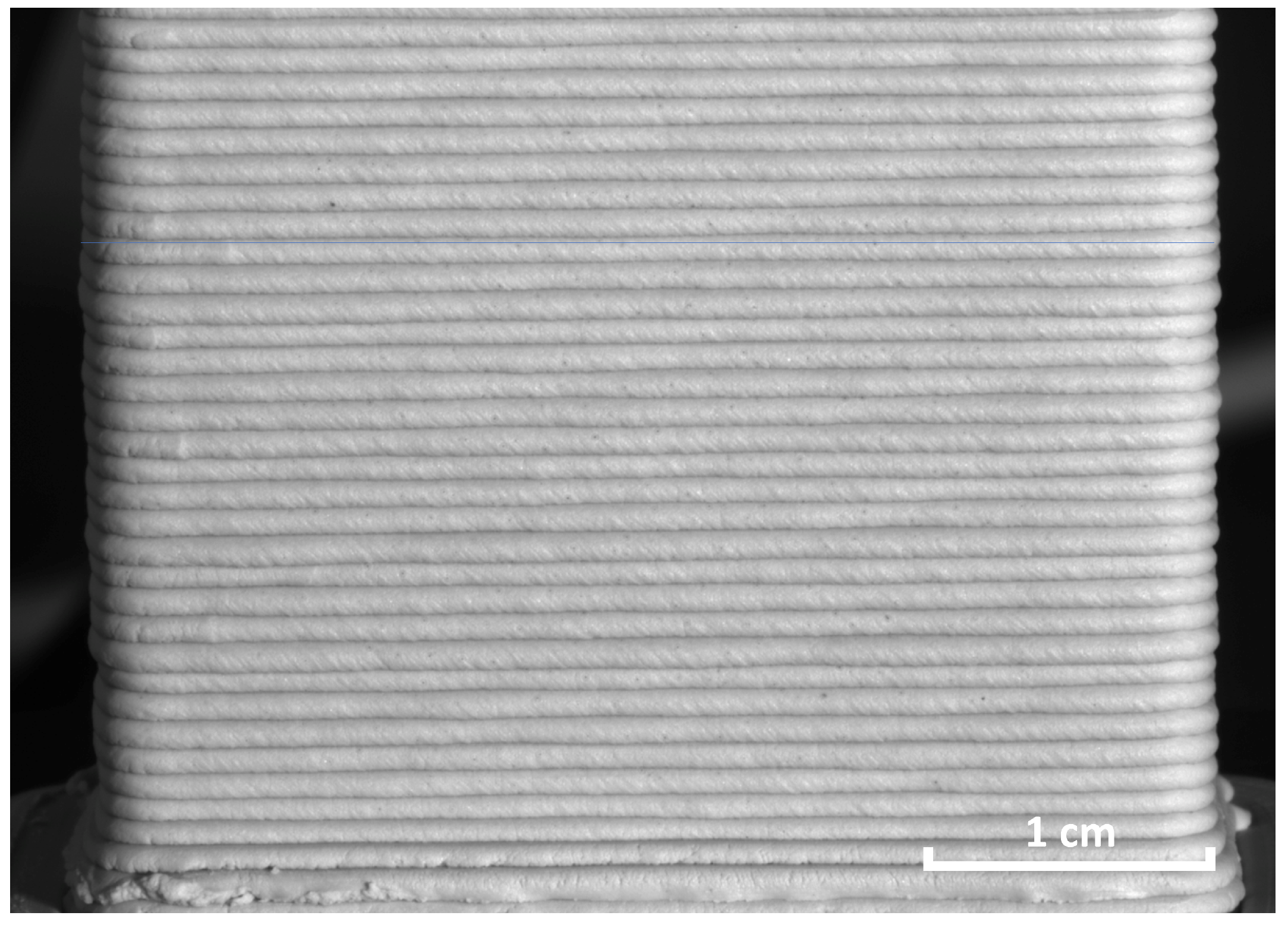
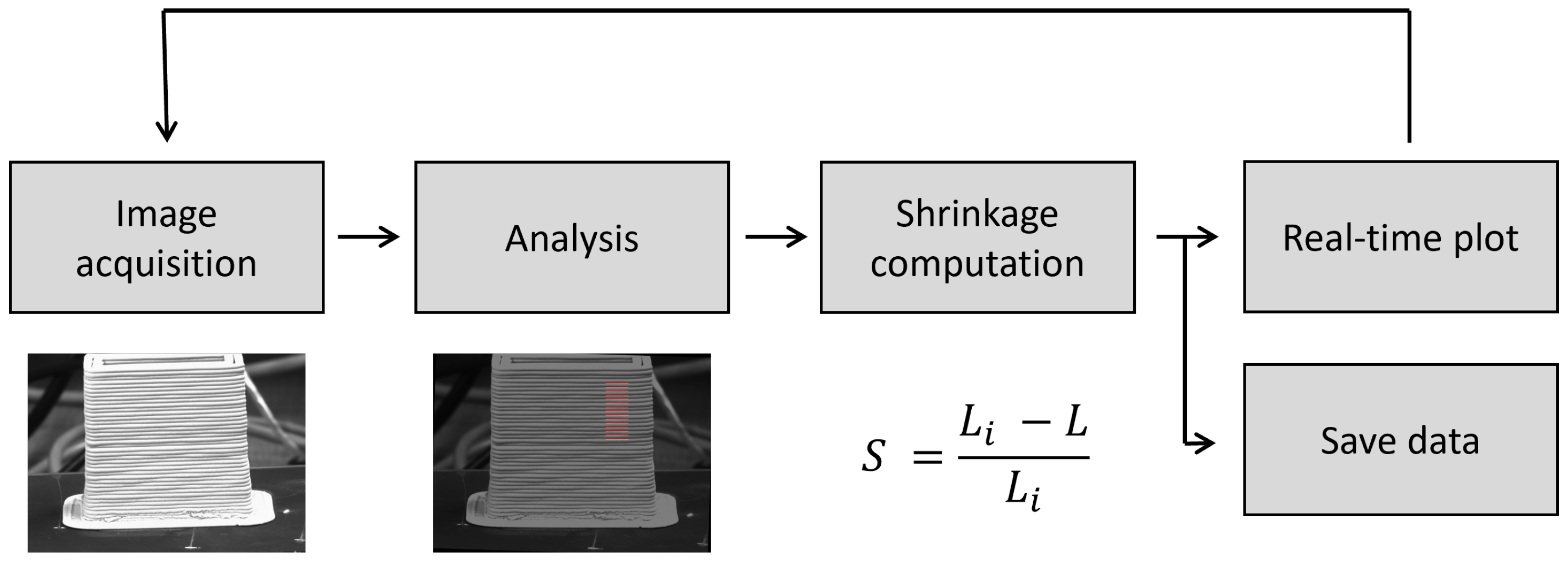
2.3. Measuring the Drying Shrinkage of a Robocasted Ceramic Product
3. Results and Discussion
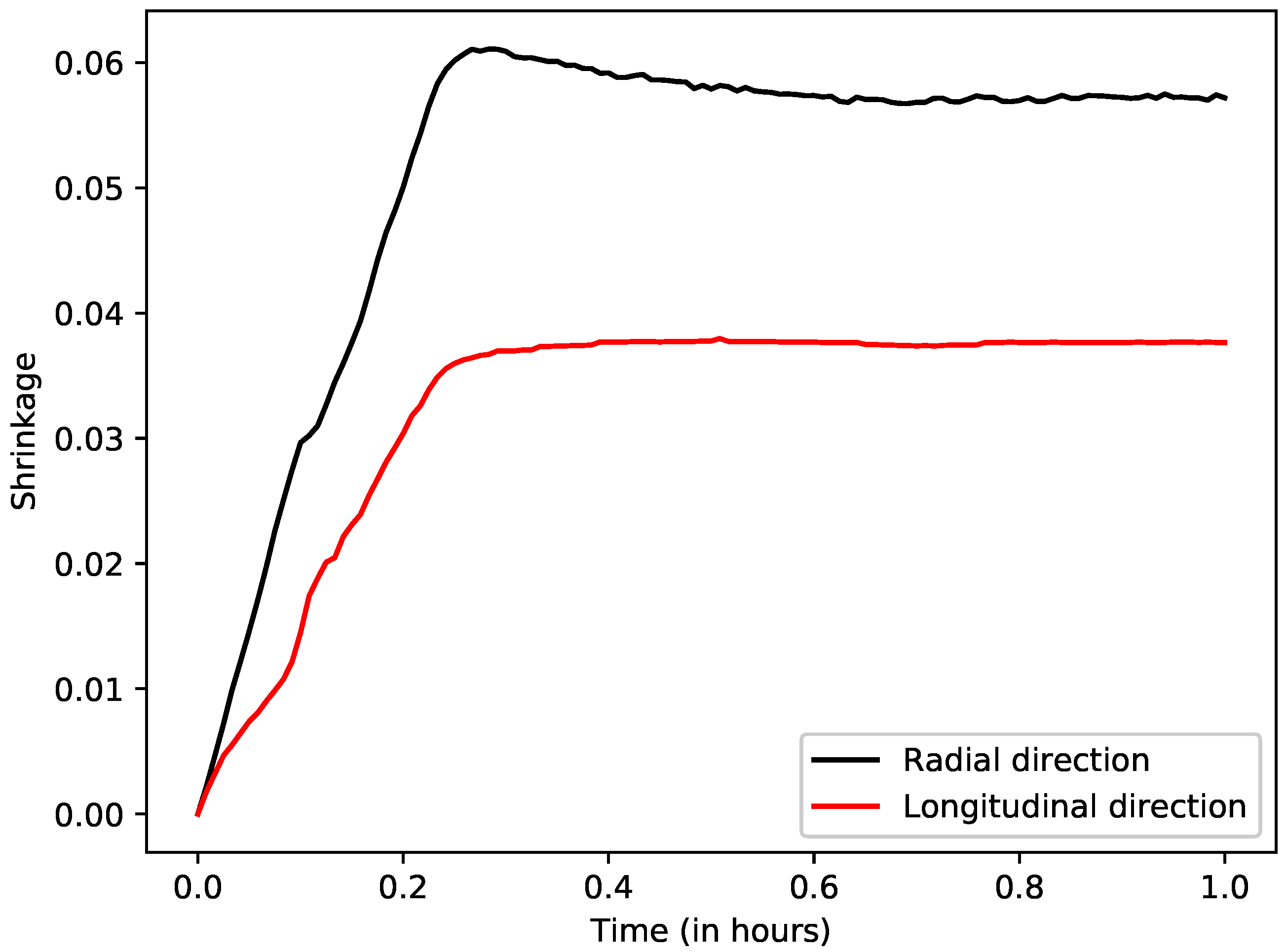
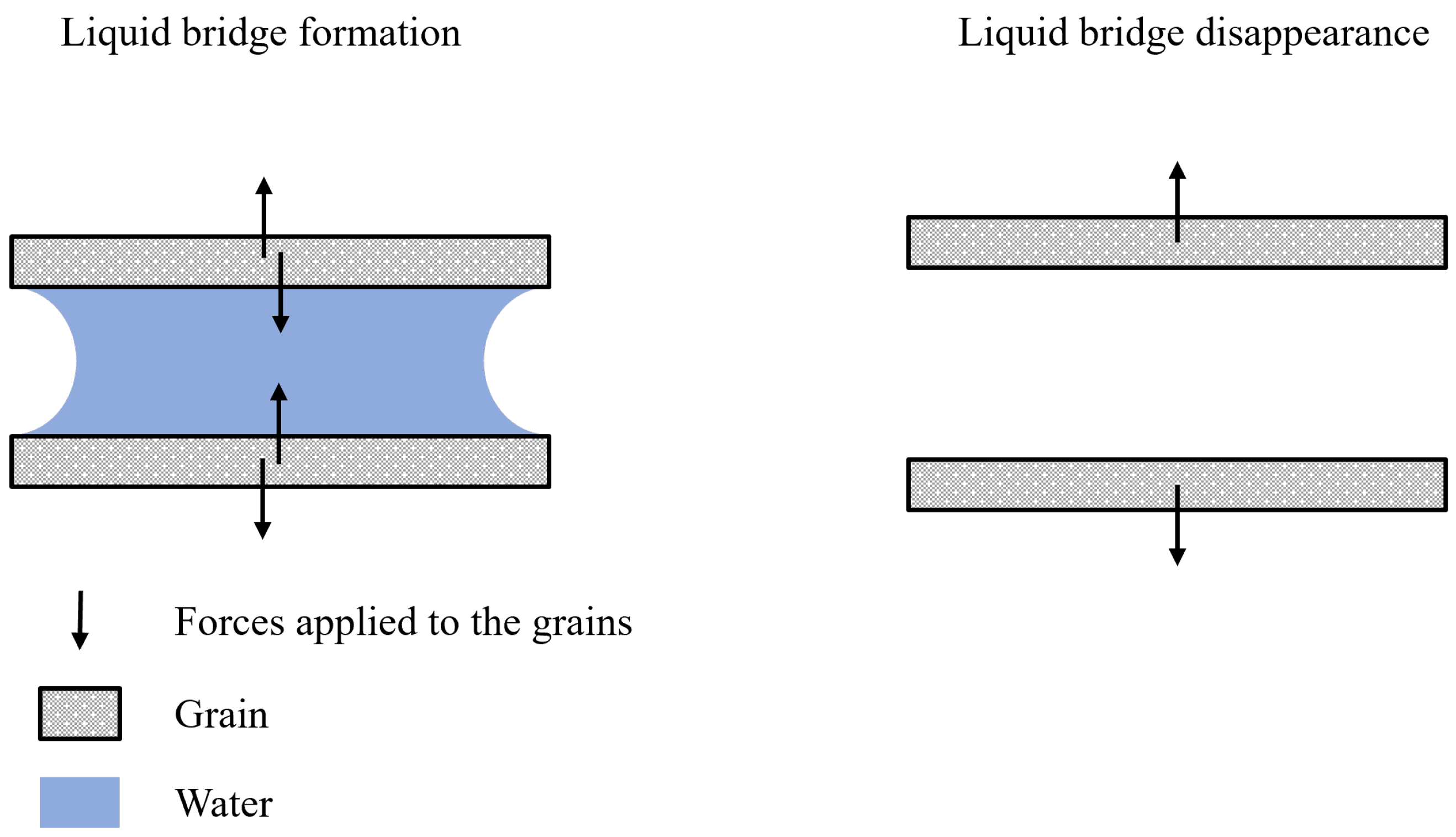
3.1. Drying Shrinkage of a Robocasted Ceramic Cord
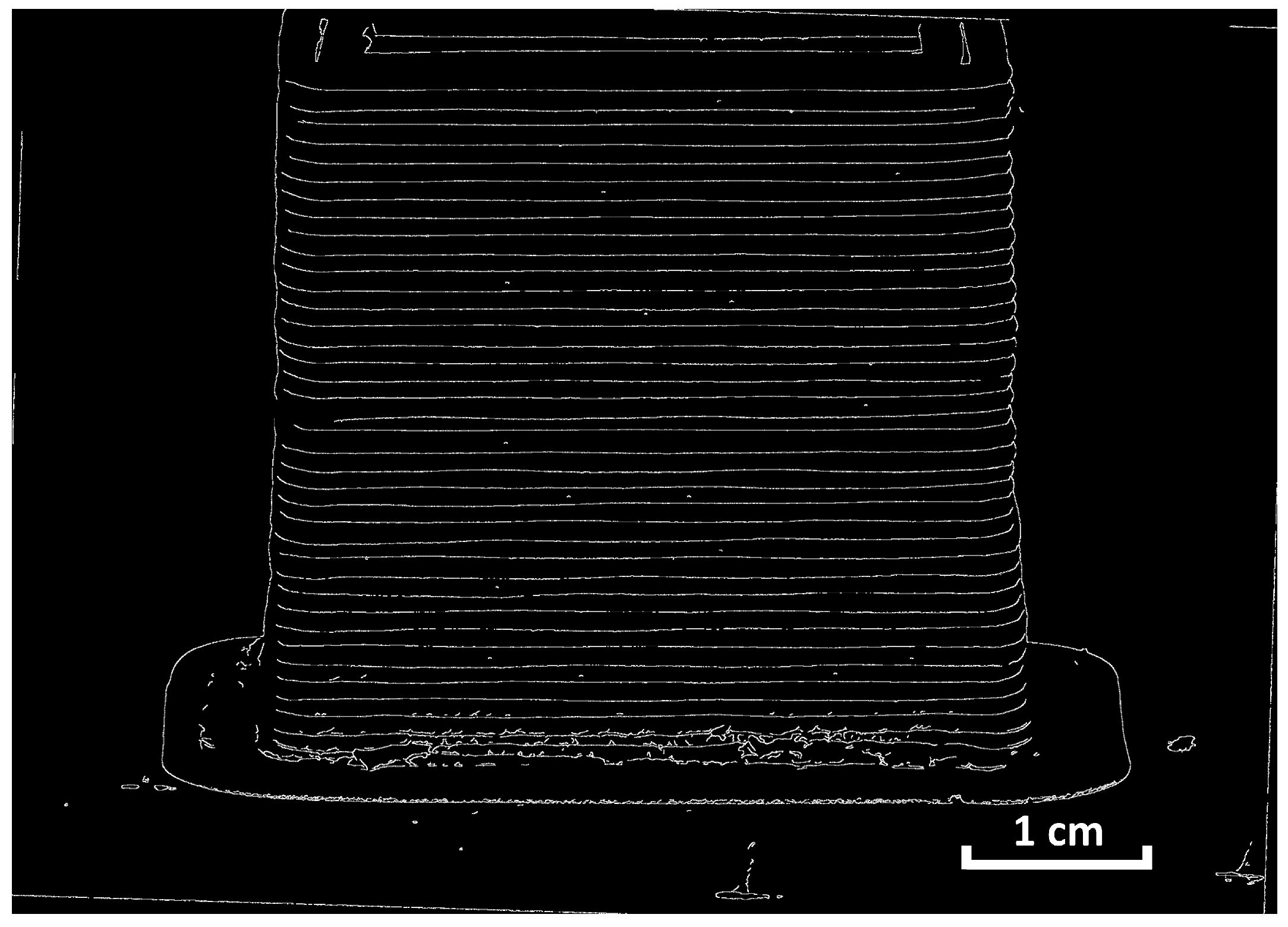
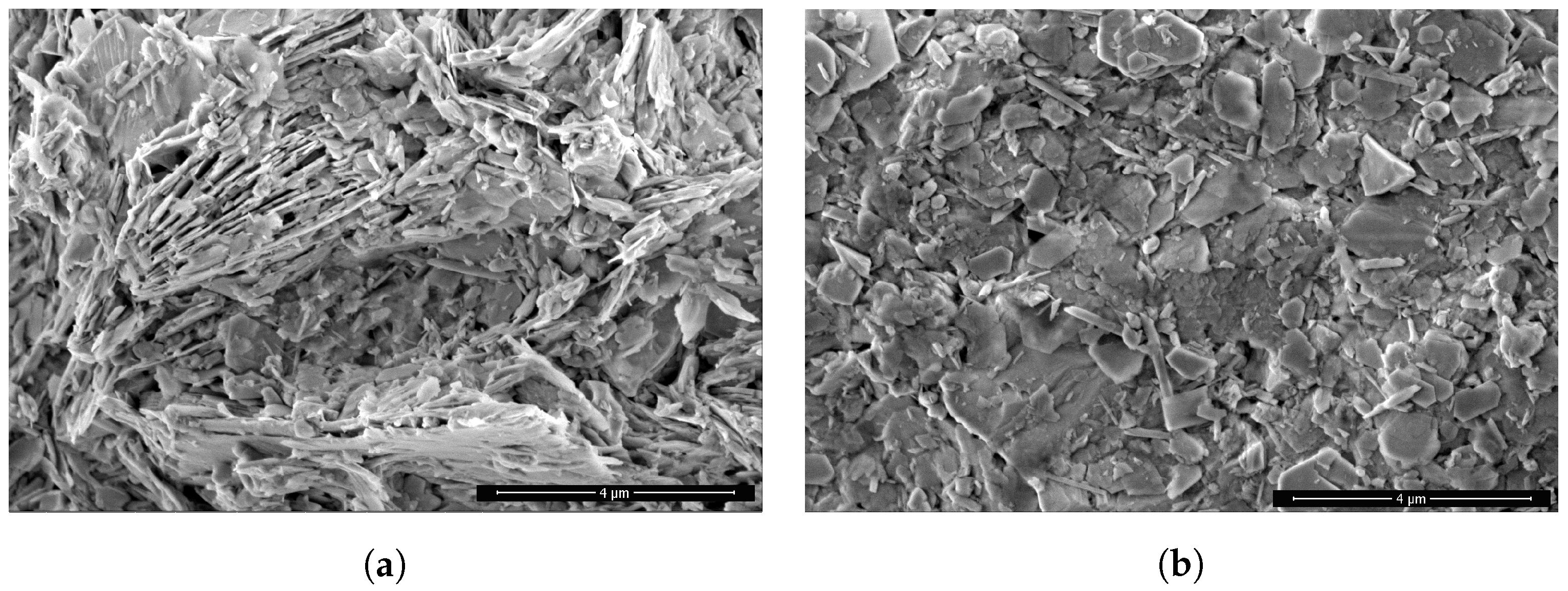
3.2. Drying of a Complete Robocasted Product
4. Perspectives on Real-Time Measurements of Drying Shrinkage for Robocasted Products
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deckers, J.; Vleugels, J.; Kruthl, J. Additive Manufacturing of Ceramics: A Review. J. Ceram. Sci. Technol. 2014, 5, 245–260. [Google Scholar] [CrossRef]
- Chen, Z.; Li, Z.; Li, J.; Liu, C.; Lao, C.; Fu, Y.; Liu, C.; Li, Y.; Wang, P.; He, Y. 3D printing of ceramics: A review. J. Eur. Ceram. Soc. 2019, 39, 661–687. [Google Scholar] [CrossRef]
- Zocca, A.; Franchin, G.; Colombo, P.; Günster, J. Additive Manufacturing. Encycl. Mater. Tech. Ceram. Glas. 2021, 1, 203–221. [Google Scholar] [CrossRef]
- Cesarano, J.; Denham, H.; Stuecker, J.; Baer, T.; Griffith, M. Freeforming of Ceramics and Composites from Colloidal Slurries; U.S. Department of Energy, Office of Scientific and Technical Information: Oak Ridge, TN, USA, 1999. [CrossRef]
- Paterlini, A.; Le Grill, S.; Brouillet, F.; Combes, C.; Grossin, D.; Bertrand, G. Robocasting of self-setting bioceramics: From paste formulation to 3D part characteristics. Open Ceram. 2021, 5, 100070. [Google Scholar] [CrossRef]
- Mirkhalaf, M.; Men, Y.; Wang, R.; No, Y.; Zreiqat, H. Personalized 3D printed bone scaffolds: A review. Acta Biomater. 2022, 156, 110–124. [Google Scholar] [CrossRef]
- Monfared, M.H.; Nemati, A.; Loghman, F.; Ghasemian, M.; Farzin, A.; Beheshtizadeh, N.; Azami, M. A deep insight into the preparation of ceramic bone scaffolds utilizing robocasting technique. Ceram. Int. 2022, 48, 5939–5954. [Google Scholar] [CrossRef]
- Boretti, A.; Castelletto, S. A perspective on 3D printing of silicon carbide. J. Eur. Ceram. Soc. 2024, 44, 1351–1360. [Google Scholar] [CrossRef]
- Gourdonnaud, D.; Pateloup, V.; Junger, A.; Bourret, J.; Chartier, T.; Geffroy, P.M. Correlation between filament deposition path, microstructural and mechanical properties of dense alumina parts printed by robocasting. J. Eur. Ceram. Soc. 2024, 44, 1027–1035. [Google Scholar] [CrossRef]
- Maillard, M.; Chevalier, J.; Gremillard, L.; Baeza, G.P.; Courtial, E.J.; Marion, S.; Garnier, V. Optimization of mechanical properties of robocast alumina parts through control of the paste rheology. J. Eur. Ceram. Soc. 2023, 43, 2805–2817. [Google Scholar] [CrossRef]
- Belmonte, M.; Lopez-Navarrete, G.; Osendi, M.I.; Miranzo, P. Heat dissipation in 3D printed cellular aluminum nitride structures. J. Eur. Ceram. Soc. 2021, 41, 2407–2414. [Google Scholar] [CrossRef]
- Pires, L.; Luís, J.; Fernandes, M.; Oliveira, M. Controlling properties of ceramic formulations for porcelain robocasting. Ceram. Int. 2023, 49, 4764–4774. [Google Scholar] [CrossRef]
- Wu, Y.; Lan, J.; Wu, M.; Zhou, W.; Zhou, S.; Yang, H.; Zhang, M.; Li, Y. Rheology and Printability of a Porcelain Clay Paste for DIW 3D Printing of Ceramics with Complex Geometric Structures. ACS Omega 2024, 9, 26450–26457. [Google Scholar] [CrossRef]
- Cesarano, J.; Baer, T.A.; Calvert, P. Recent developments in freeform fabrication of dense ceramics from slurry deposition. In Proceedings of the 1997 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 11–13 August 1997. [Google Scholar]
- Lamnini, S.; Elsayed, H.; Lakhdar, Y.; Baino, F.; Smeacetto, F.; Bernardo, E. Robocasting of advanced ceramics: Ink optimization and protocol to predict the printing parameters—A review. Heliyon 2022, 8, e10651. [Google Scholar] [CrossRef]
- Feilden, E.; Glymond, D.; Saiz, E.; Vandeperre, L. High temperature strength of an ultra high temperature ceramic produced by additive manufacturing. Ceram. Int. 2019, 45, 18210–18214. [Google Scholar] [CrossRef]
- Scherer, G.W. Theory of Drying. J. Am. Ceram. Soc. 1990, 73, 3–14. [Google Scholar] [CrossRef]
- Brosnan, D.; Robinson, G. Introduction to Drying of Ceramics: With Laboratory Exercises; Wiley: Hoboken, NJ, USA, 2003. [Google Scholar]
- Ford, R.W. Ceramics Drying; Elsevier: Amsterdam, The Netherlands, 1986. [Google Scholar]
- Ghazanfari, A.; Li, W.; Leu, M.; Hilmas, G. A novel extrusion-based additive manufacturing process for ceramic parts. In Proceedings of the 27th Annual International Solid Freeform Fabrication Symposium, Austin, TX, USA, 8–10 August 2016. [Google Scholar]
- Baltazar, J.; Torres, P.; de Oliveira, J.D.; da Cruz, J.P.; Gouveia, S.; Olhero, S. Influence of filament patterning in structural properties of dense alumina ceramics printed by robocasting. J. Manuf. Process. 2021, 68, 569–582. [Google Scholar] [CrossRef]
- Oummadi, S.; Nait-Ali, B.; Alzina, A.; Paya, M.C.; Gaillard, J.M.; Smith, D.S. Optical method for evaluation of shrinkage in two dimensions during drying of ceramic green bodies. Open Ceram. 2020, 2, 100016. [Google Scholar] [CrossRef]
- Itseez. Open Source Computer Vision Library. 2015. Available online: https://github.com/itseez/opencv (accessed on 1 January 2020).
- Shaji, S.; Radhakrishnan, V. An investigation on surface grinding using graphite as lubricant. Int. J. Mach. Tools Manuf. 2002, 42, 733–740. [Google Scholar] [CrossRef]
- Suzuki, S.; be, K. Topological structural analysis of digitized binary images by border following. Comput. Vis. Graph. Image Process. 1985, 30, 32–46. [Google Scholar] [CrossRef]
- Canny, J. A Computational Approach to Edge Detection. In Readings in Computer Vision; Fischler, M.A., Firschein, O., Eds.; Morgan Kaufmann: San Francisco, CA, USA, 1987; pp. 184–203. [Google Scholar]
- Gayathri Monicka, S.; Manimegalai, D.; Karthikeyan, M. Detection of microcracks in silicon solar cells using Otsu-Canny edge detection algorithm. Renew. Energy Focus 2022, 43, 183–190. [Google Scholar] [CrossRef]
- Meng, Y.; Zhang, Z.; Yin, H.; Ma, T. Automatic detection of particle size distribution by image analysis based on local adaptive canny edge detection and modified circular Hough transform. Micron 2018, 106, 34–41. [Google Scholar] [CrossRef]
- Dietemann, B.; Wahl, L.; Travitzky, N.; Kruggel-Emden, H.; Kraft, T.; Bierwisch, C. Reorientation of Suspended Ceramic Particles in Robocasted Green Filaments during Drying. Materials 2022, 15, 2100. [Google Scholar] [CrossRef]
- Feilden, E.; Ferraro, C.; Zhang, Q.; García-Tuñón, E.; D’Elia, E.; Giuliani, F.; Vandeperre, L.; Saiz, E. 3D Printing Bioinspired Ceramic Composites. Sci. Rep. 2017, 7, 13759. [Google Scholar] [CrossRef]
- Rossetti, D.; Pepin, X.; Simons, S.J. Rupture energy and wetting behavior of pendular liquid bridges in relation to the spherical agglomeration process. J. Colloid Interface Sci. 2003, 261, 161–169. [Google Scholar] [CrossRef]
- Norton, F.H. Fundamental Study of Clay: VIII, a New Theory for the Plasticity of Clay-Water Masses. J. Am. Ceram. Soc. 1948, 31, 236–240. [Google Scholar] [CrossRef]
- Mielniczuk, B.; Hueckel, T.; El Youssoufi, M.S. Laplace pressure evolution and four instabilities in evaporating two-grain liquid bridges. Powder Technol. 2015, 283, 137–151. [Google Scholar] [CrossRef]
- Tarì, G.; Ferreira, J.M.F.; Lyckfeldt, O. Influence of the Stabilising Mechanism and Solid Loading on Slip Casting of Alumina. J. Eur. Ceram. Soc. 1998, 18, 479–486. [Google Scholar] [CrossRef]
- Onoda, G.Y., Jr. Specific Volume Diagrams for Ceramic Processing. J. Am. Ceram. Soc. 1983, 66, 297–301. [Google Scholar] [CrossRef]
- Lauro, N.; Oummadi, S.; Alzina, A.; Nait-Ali, B.; Smith, D.S. Computer model of drying behaviour of ceramic green bodies with particular reference to moisture content dependent properties. J. Eur. Ceram. Soc. 2021, 41, 7321–7329. [Google Scholar] [CrossRef]
- Lauro, N. Caractérisation et Modélisation des Retraits de Séchage dans le Procédé de Microextrusion d’une Pâte Céramique. Ph.D. Thesis, IRCER—Institut de Recherche sur les CERamiques, Limoges, France, 2023. [Google Scholar]
- ASTM C326-09; Standard Test Method for Drying and Firing Shrinkages of Ceramic Whiteware Clays ASTM C326-09. ASTM: West Conshohocken, PA, USA, 2014.
- Pardo, F.; Jordan, M.M.; Montero, M.A. Ceramic behaviour of clays in Central Chile. Appl. Clay Sci. 2018, 157, 158–164. [Google Scholar] [CrossRef]
- Kiennemann, J.; Chartier, T.; Pagnoux, C.; Baumard, J.; Huger, M.; Lamérant, J. Drying mechanisms and stress development in aqueous alumina tape casting. J. Eur. Ceram. Soc. 2005, 25, 1551–1564. [Google Scholar] [CrossRef]
- Mauroux, T.; Benboudjema, F.; Turcry, P.; Aït-Mokhtar, A.; Deves, O. Study of cracking due to drying in coating mortars by digital image correlation. Cem. Concr. Res. 2012, 42, 1014–1023. [Google Scholar] [CrossRef]
- Ylmaztürk, F.; Kulur, S.; Pekmezci, B.Y. Measurement of shrinkage in concrete samples by using digital photogrammetric methods. In Proceedings of the International Archives of the Photogrammetry, Remote Sensing and Spatial Information Sciences—ISPRS Archives, Istanbul, Turkey, 12–23 July 2004; Volume 35, pp. 258–261. [Google Scholar]
- Nait-Ali, B.; Alzina, A.; Lauro, N.; Smith, D.S. Perspectives in drying of ceramics. Open Ceram. 2024, 17, 100554. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lauro, N.; Alzina, A.; Nait-Ali, B.; Smith, D.S. Non-Uniform Drying Shrinkage in Robocasted Green Body Ceramic Products. Ceramics 2024, 7, 1122-1136. https://doi.org/10.3390/ceramics7030073
Lauro N, Alzina A, Nait-Ali B, Smith DS. Non-Uniform Drying Shrinkage in Robocasted Green Body Ceramic Products. Ceramics. 2024; 7(3):1122-1136. https://doi.org/10.3390/ceramics7030073
Chicago/Turabian StyleLauro, Nicolas, Arnaud Alzina, Benoit Nait-Ali, and David S. Smith. 2024. "Non-Uniform Drying Shrinkage in Robocasted Green Body Ceramic Products" Ceramics 7, no. 3: 1122-1136. https://doi.org/10.3390/ceramics7030073
APA StyleLauro, N., Alzina, A., Nait-Ali, B., & Smith, D. S. (2024). Non-Uniform Drying Shrinkage in Robocasted Green Body Ceramic Products. Ceramics, 7(3), 1122-1136. https://doi.org/10.3390/ceramics7030073






