Investigation of the Quartz Distribution in Electro-Porcelain Materials
Abstract
1. Introduction
2. Materials and Methods
3. Results
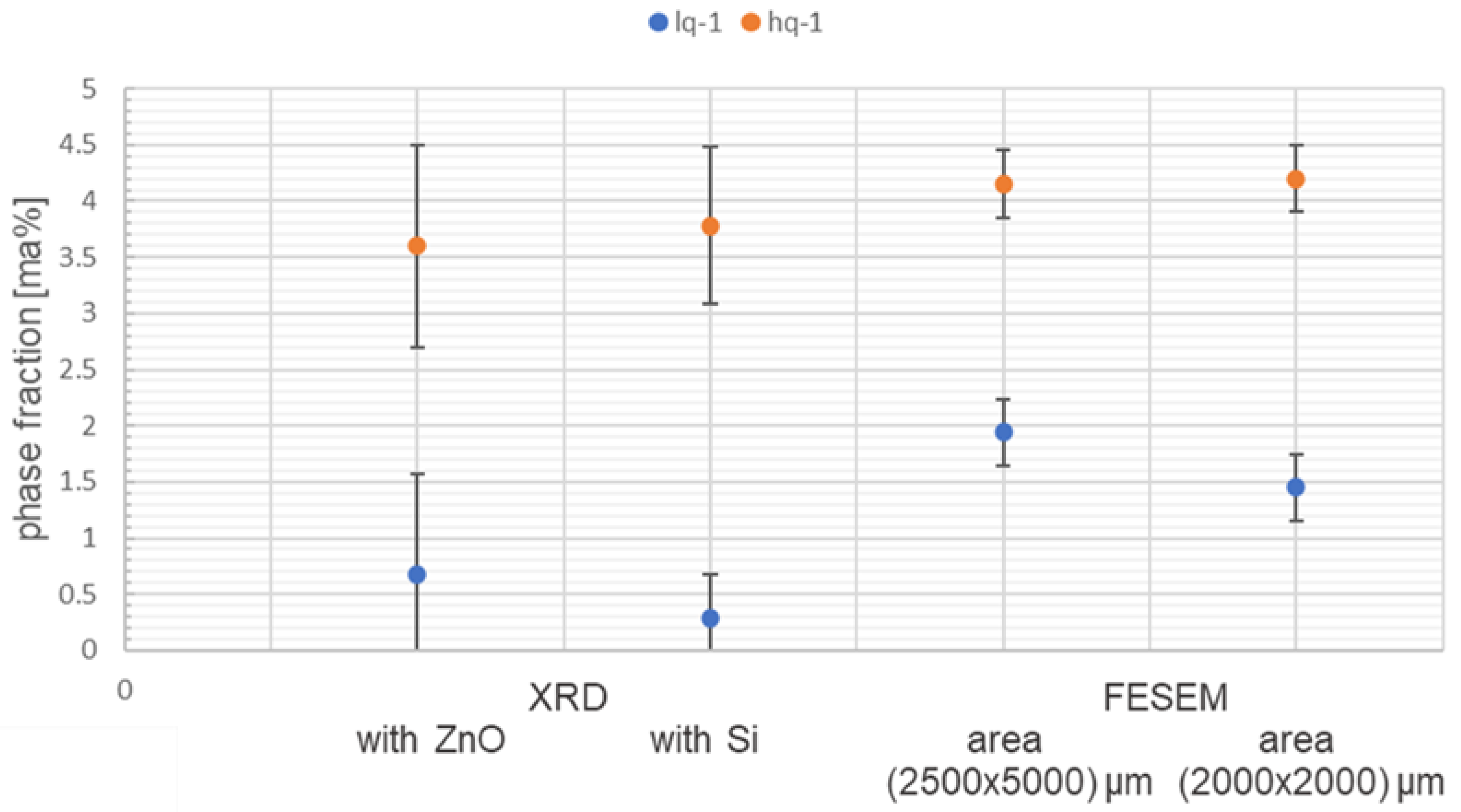
3.1. Phase Content Determined by X-ray Diffraction
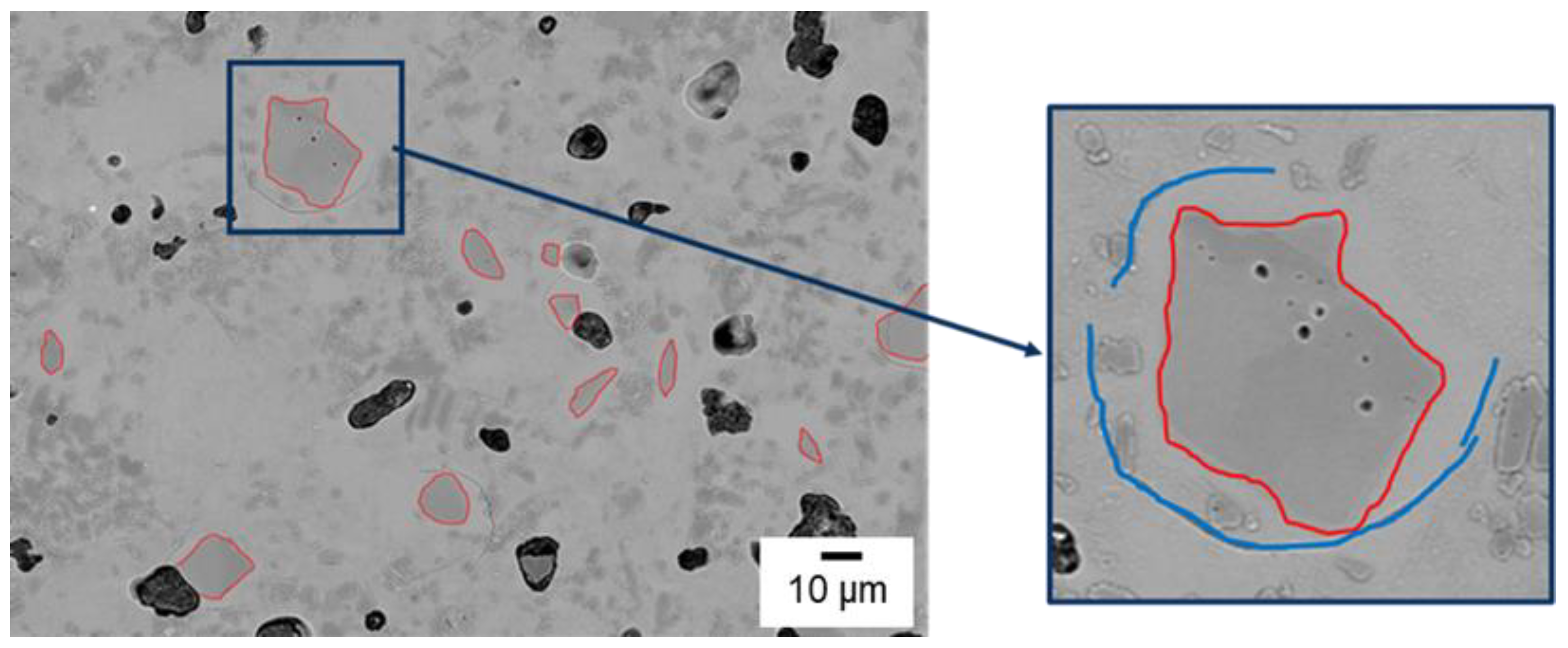
3.2. Microstructure Analysis
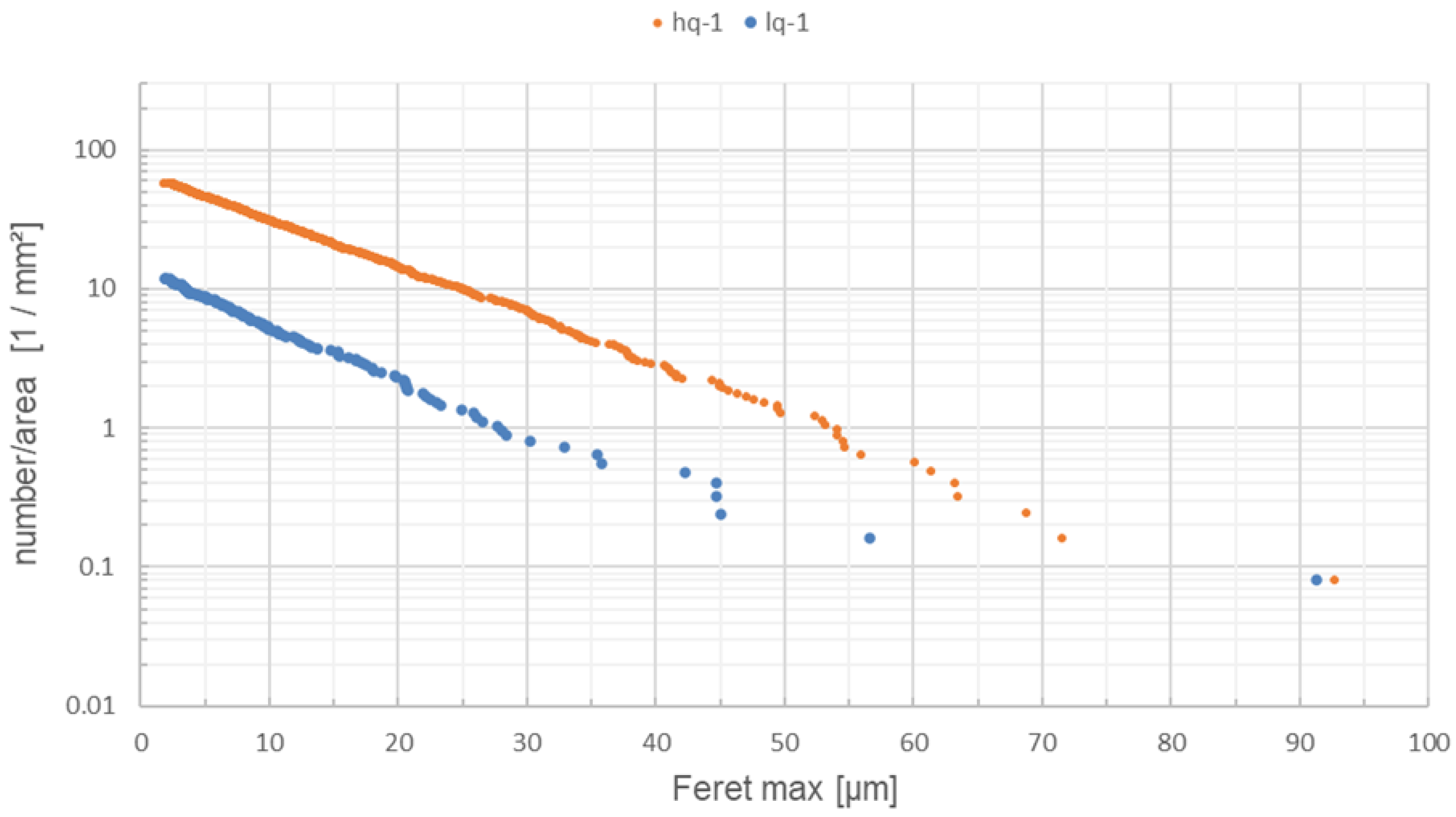
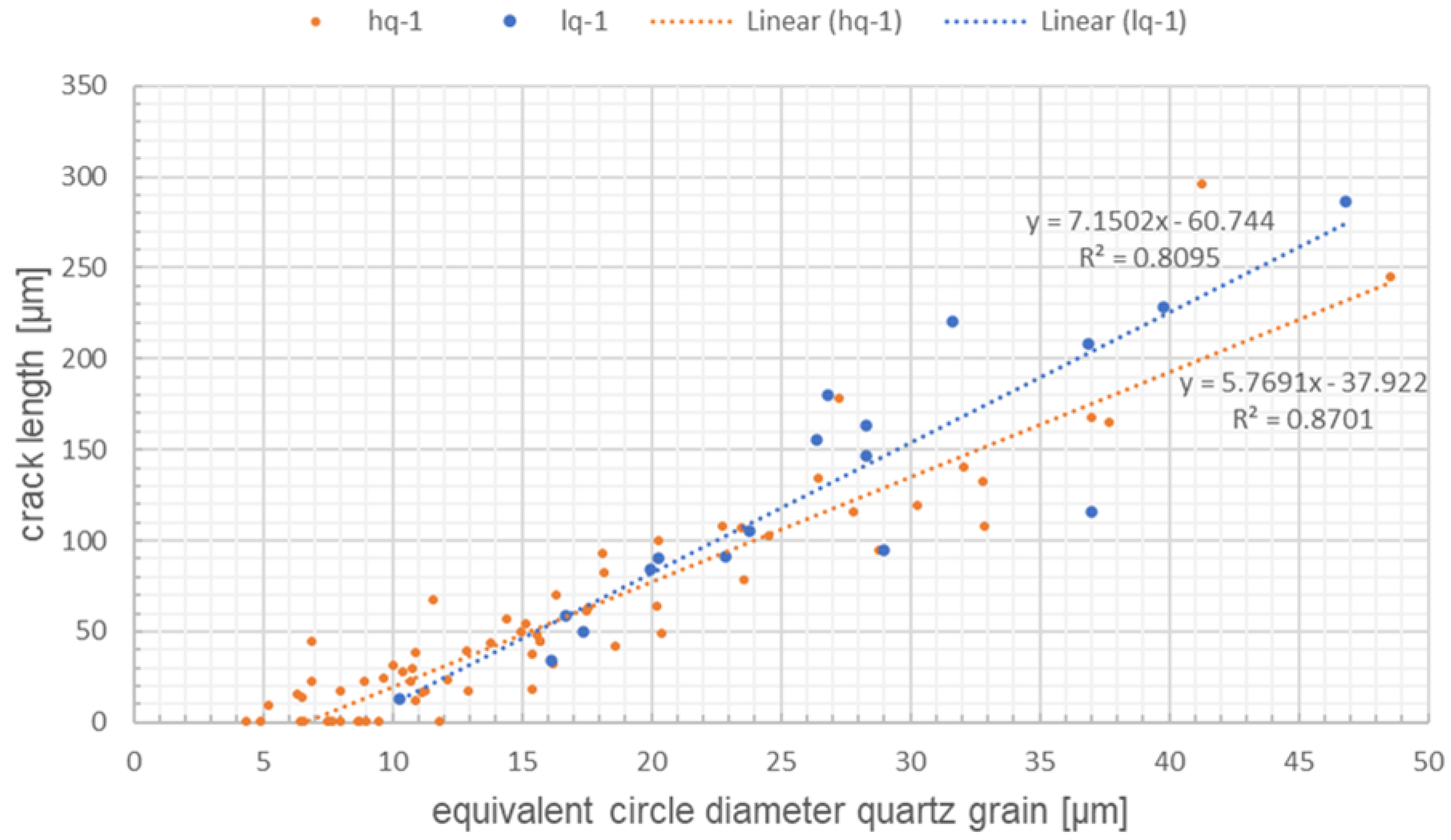
3.3. Relationship between Quartz Grain Size and Crack Length
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liebermann, J. Hochspannungsisolatoren—Grundlagen und Trends für Hersteller, Anwender und Studierende, 2nd ed.; H.O. Schulze KG: Lichtenfels, Germany, 2008. [Google Scholar]
- Kollenberg, W. Technische Keramik—Grundlagen Werkstoffe Verfahrenstechnik, 2nd ed.; Vulkan-Verlag GmbH: Essen, Germany, 2009; p. 192. [Google Scholar]
- Schulte-Fischedick, J.; Lehretz, F.; Seifert, J.M.; Hettich, P.; Schell, K.G.; Hoffmann, M.J.; Marthen, W.; Wekenborg, H.; Pohlmann, H. Materials Based Lifetime Assessment of Porcelain Insulators. In Proceedings of the 20th International Symposium on High Voltage Engineering, Buenos Aires, Argentina, 27 August–1 September 2017. [Google Scholar]
- Schulte-Fischedick, J.; Singh, P.; Lehretz, F.; Hettich, P.; Bucharsky, C.; Schell, G.; Ho, M.J.; Marthen, W.; Wekenborg, H.; Pohlmann, H. Materials based lifetime assessment of porcelain insulators. In Proceedings of the 21st International Symposium on High Voltage Engineering (ISH 2019), Budapest, Hungary, 26–30 August 2019; Lecture Notes in Electrical Engineering. Nemeth, B., Ed.; Springer Nature: Cham, Switzerland, 2020; Volume 599, pp. 1–12. [Google Scholar] [CrossRef]
- Szibor, H.; Hennicke, H.W. On the Correlation between Microstructural Data and Mechanical Properties of Porcelain Materials—Part II; cfi/Ber. Dt. Keram. Ges. 59: Köln, Germany, 1982; Volume 3, pp. 170–175. [Google Scholar]
- Szibor, H.; Hennicke, H.W. On the Correlation between Microstructural Data and Mechanical Properties of Porcelain Materials—Part I; cfi/Ber. DKG 58, No. 4/5: Köln, Germany, 1981; pp. 263–269. [Google Scholar]
- Salmang, H.; Scholze, H. Keramik, 7th ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2007; pp. 227, 401, 692ff, 709ff. [Google Scholar]
- Ochen, W.; D’ujanga, F.M.; Oruru, B. Influence of residual stress on the mechanical behavior of ceramics with various quartz sizes. Sci. Afr. 2020, 11, e00648. [Google Scholar] [CrossRef]
- Brevier Technische Keramik, 4th ed.; Verband der Keramischen Industrie e.V.: Verlag Hans Carl: Nürnberg, Germany, 2003.
- Davidge, R.W.; Green, T.J. The strength of two-phase ceramic/glass materials. J. Mat. Sci. 1968, 3, 629–634. [Google Scholar] [CrossRef]
- Weyl, D. Über den Einfluss innerer Spannungen auf das Gefüge und die mechanische Festigkeit des Porzellan. DKG 1959, 36, 319–352. [Google Scholar]
- Eshelby, J.D. The force on an elastic singularity. Series A, Mathematical and Physical Sciences. Phil. Trans. Roy. Soc. 1951, 244, 87–112. [Google Scholar] [CrossRef]
- Weinberger, C.R.; Cai, W.; Barnett, D.M. Lecture Notes—Elasticity of Microscopic Structures; Stanford University: Stanford, CA, USA, 2005; Available online: https://micro.stanford.edu/~caiwei/me340b/content/me340b-notes_v01.pdf (accessed on 15 April 2023).
- Serbena, F.C.; Zanotto, E. Internal Residual Stresses in Glass-Ceramics: A Review. J. Non-Cryst. Solids 2012, 358, 975–984. [Google Scholar] [CrossRef]
- Whipkey, S.C.; Modugno, M.C.; Lee, H.; Carty, W.M. Optimized etching of porcelain and polycristalline alumnia with a glass phase. J. Eur. Ceram. Soc. 2021, 41, 3761–3768. [Google Scholar] [CrossRef]
- DIN EN ISO 18754:2022-06; Hochleistungskeramik—Bestimmung der Dichte und der scheinbaren Porosität. Deutsches Institut für Normung e. V., Beuth-Verlag: Berlin, Germany, 2022.
- SciGlass Professional; Version 7.11; ITC Inc.: Hudsonville, MI, USA.
- Mendelson, M.I. Average Grain Size in Polycrystalline Ceramics. J. Am. Ceram. Soc. 1969, 52, 443–446. [Google Scholar] [CrossRef]
- Gerlt, A.R.C.; Criner, A.K.; Semiatin, L.; Payton, E.J. On the grain size proportionality constants calculated in M.I. Mendelson’s Average Grain Size in Polycrystalline Ceramics. J. Am. Ceram. Soc. 2018, 10, 37–41. [Google Scholar] [CrossRef]
- Roebuck, B.; Phatak, C.; Birks-Agnew, I. A Comparison of the Linear Intercept and Equivalent Circle Methods for Grain Size Measurement in WC/Co Hardmetals. In NPL Report MATC(A)149, 03/2004; NPL: Teddington, UK, 2004. [Google Scholar]
- ASTM International E:112-96; Standard Test Methods for Determining Average Grain Size. International Organization for Standardization: West Conshohocken, PA, USA, 2006.
- Schulte-Fischedick, J. Private communication: LAPP Insulators GmbH, Bahnhofstr. 5, 95632 Wunsiedel, Germany, July 2021.
- Quinn, G.D. NIST Recommended Practice Guide: Fractography of Ceramics and Glasses, 2nd ed.; Special Publication (NIST SP); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2016; pp. 7–21. [CrossRef]

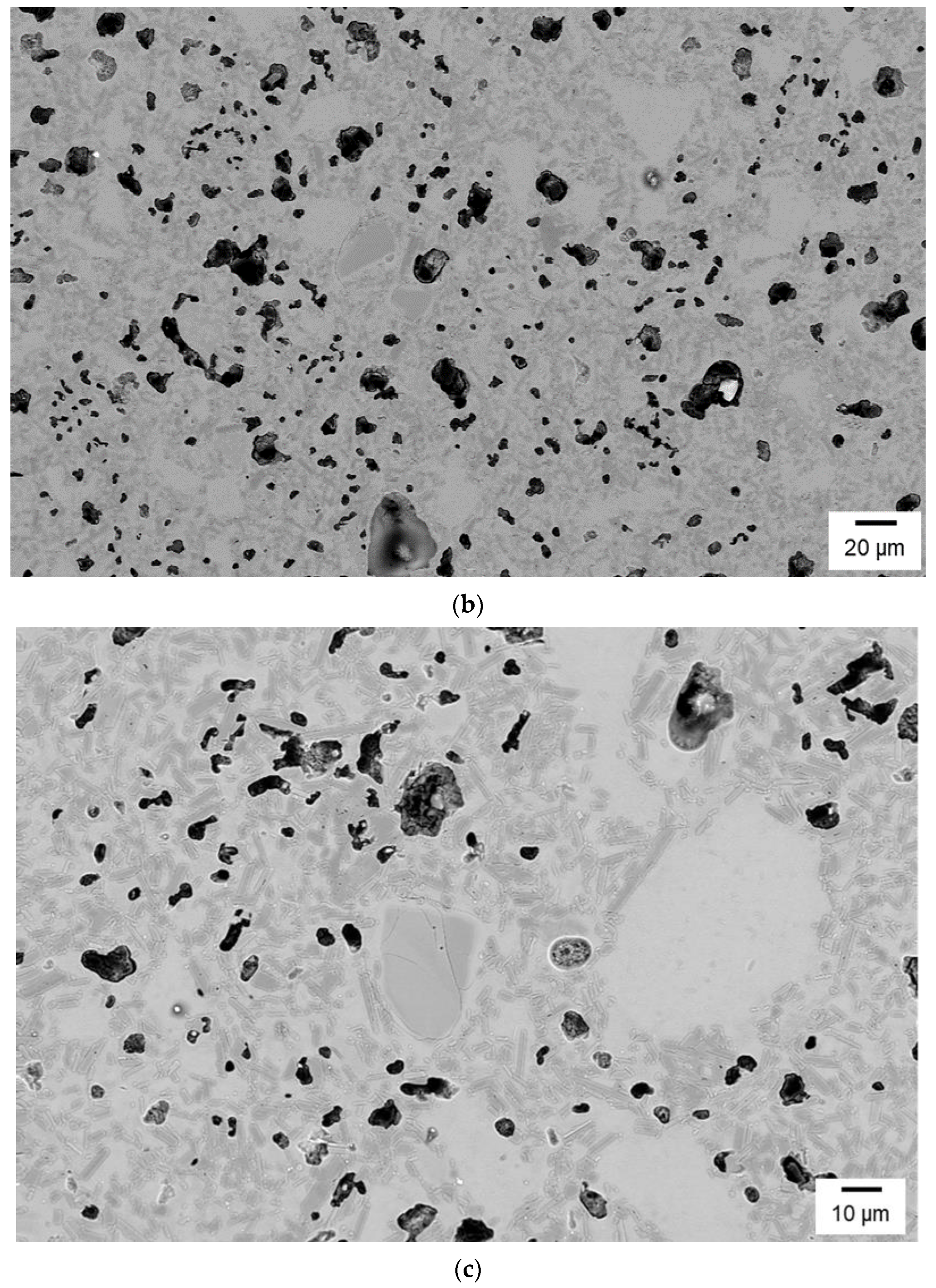
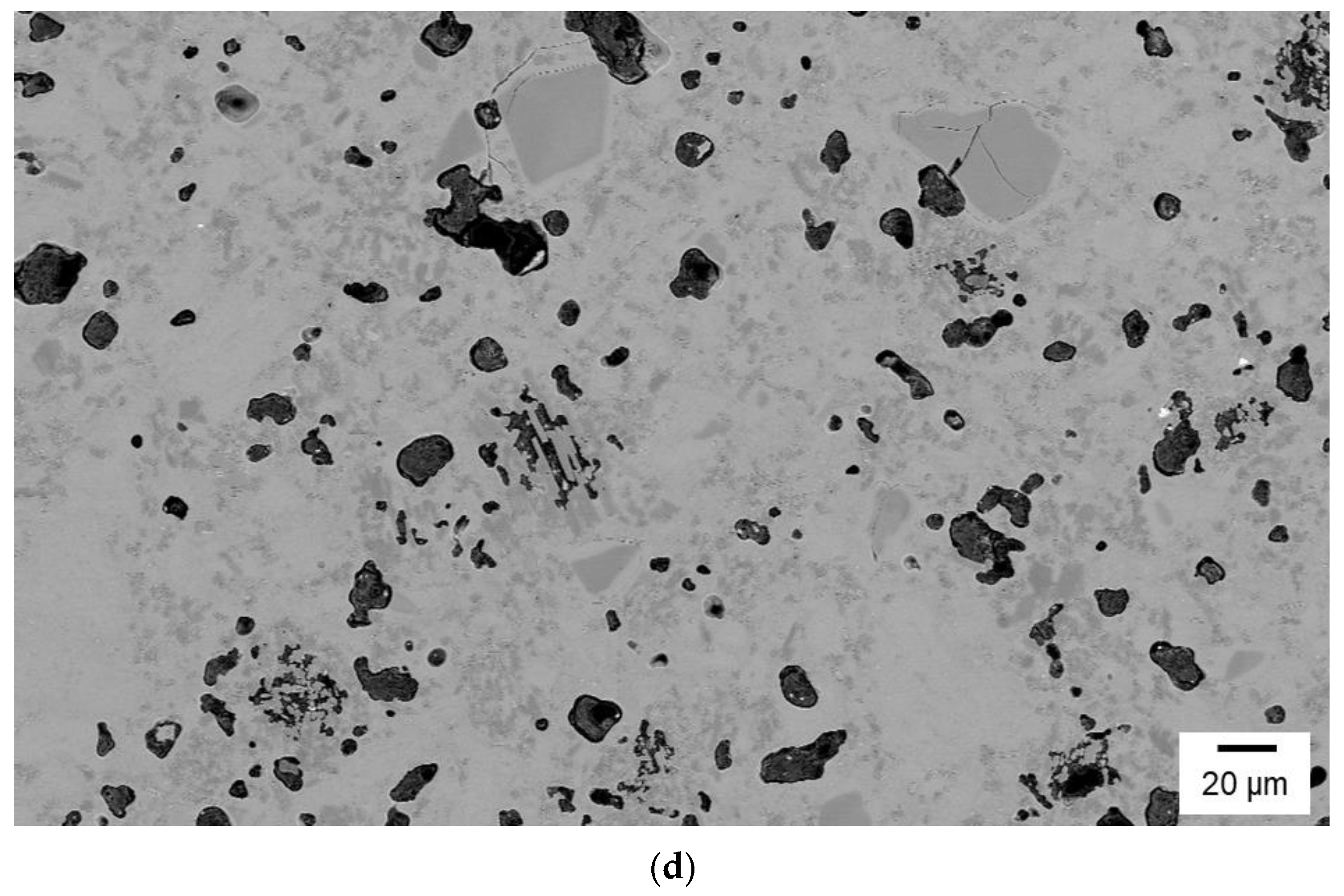


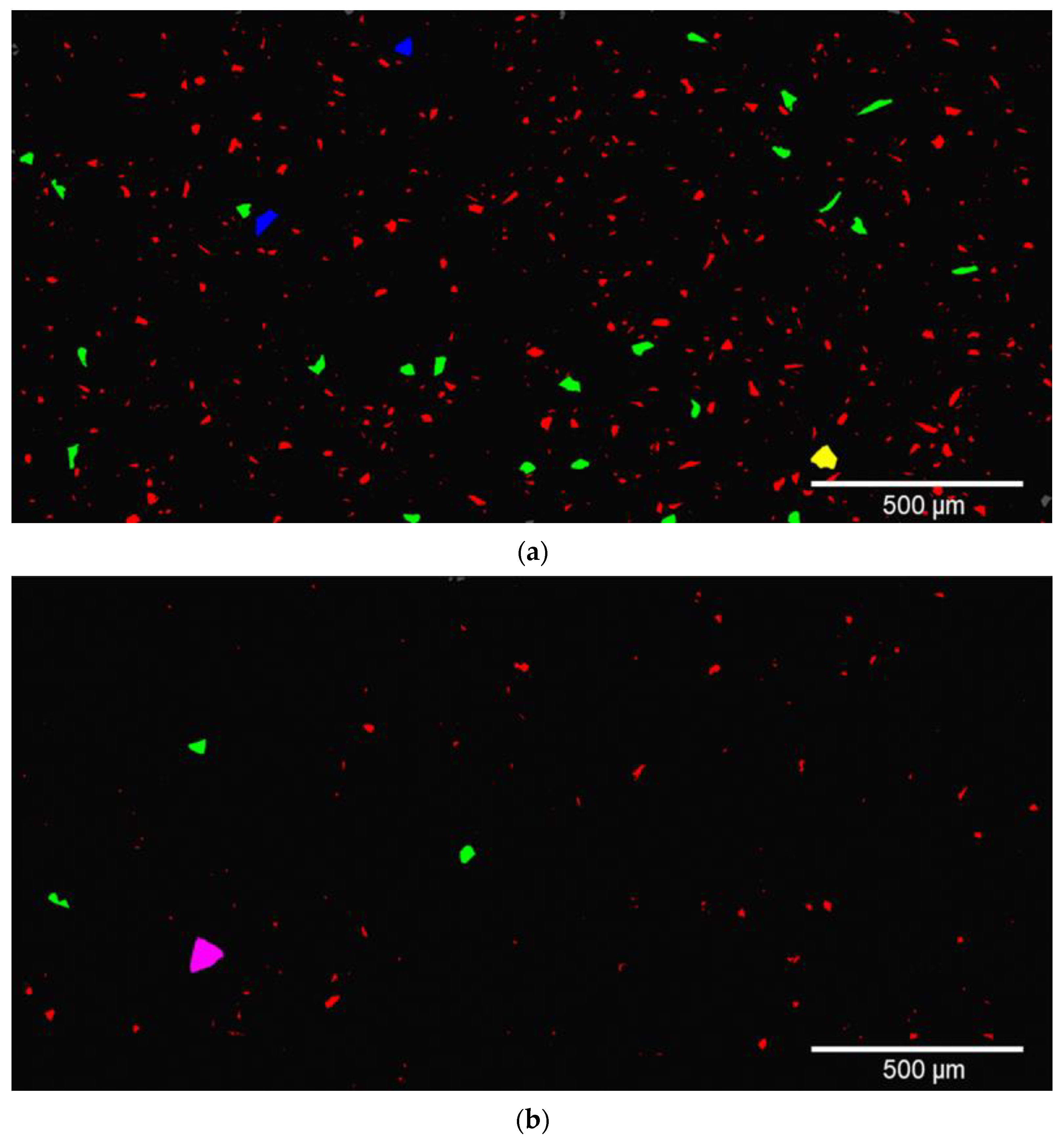


| Sample | Standard | Quartz [wt.%] | Corundum [wt.%] | Mullite [wt.%] | Amorphous Phase [wt.%] | Bulk Density |
|---|---|---|---|---|---|---|
| lq-1 | Si | 0.3 ± 0.4 | 46.2 ± 0.4 | 10.7 ± 0.2 | 42.8 ± 0.5 | 2.70 ± 0.01 |
| ZnO | 0.7 ± 0.9 | 43.8 ± 0.5 | 11.2 ± 0.5 | 44.4 ± 0.8 | ||
| hq-1 | Si | 3.8 ± 0.7 | 31.7 ± 0.4 | 14.4 ± 0.3 | 50.2 ± 0.6 | 2.56 ± 0.01 |
| ZnO | 3.6 ± 0.9 | 26.9 ± 0.6 | 13.2 ± 0.5 | 56.2 ± 0.8 |
| SiO2 | Al2O3 | MgO | Na2O | K2O | ||
|---|---|---|---|---|---|---|
| Content | [mol%] | 92.0 ± 0.9 | 6.8 ± 0.8 | 0.64 ± 0.14 | 0.55 ± 0.35 | 0.03 ± 0.04 |
| [wt.%] | 87.9 | 11.1 | 0.41 | 0.55 | 0.05 |
| Sample | Quartz Content [vol.-%] | Total Number of Grains Measured | [µm] | [µm] | [µm] | [µm] |
|---|---|---|---|---|---|---|
| hq-1 | 4.2 ± 0.3 | 716 | 3.6 | 11.1 ± 0.9 | 31.9 | 92.7 |
| lq-1 | 2.0 ± 0.3 | 150 | 2.9 | 8.5 ± 1.9 | 26.1 | 91.3 |
| Material | Bulk Density | Quartz Content XRD [wt.%] | Quartz Content Image Analysis [wt.%] | Maximal Measured Quartz Grain Size (1) [µm] | Measured Critical Grain Size (1) [µm] | Recalculated to Volume Critical Grain Size [µm] |
|---|---|---|---|---|---|---|
| lq-1 with ZnO | 2.70 ± 0.01 | 0.7 ± 0.9 | 1.94 | 36.6 | 8.5 ± 4.4 | 12.0 |
| hq-1 with ZnO | 2.56 ± 0.01 | 3.6 ± 0.9 | 4.30 | 46.1 | 6.6 ± 1.2 | 9.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Senze, F.; Höhn, S.; Matthey, B.; Schulte-Fischedick, J.; Herrmann, M. Investigation of the Quartz Distribution in Electro-Porcelain Materials. Ceramics 2023, 6, 1277-1290. https://doi.org/10.3390/ceramics6020078
Senze F, Höhn S, Matthey B, Schulte-Fischedick J, Herrmann M. Investigation of the Quartz Distribution in Electro-Porcelain Materials. Ceramics. 2023; 6(2):1277-1290. https://doi.org/10.3390/ceramics6020078
Chicago/Turabian StyleSenze, Fanni, Sören Höhn, Björn Matthey, Jan Schulte-Fischedick, and Mathias Herrmann. 2023. "Investigation of the Quartz Distribution in Electro-Porcelain Materials" Ceramics 6, no. 2: 1277-1290. https://doi.org/10.3390/ceramics6020078
APA StyleSenze, F., Höhn, S., Matthey, B., Schulte-Fischedick, J., & Herrmann, M. (2023). Investigation of the Quartz Distribution in Electro-Porcelain Materials. Ceramics, 6(2), 1277-1290. https://doi.org/10.3390/ceramics6020078








